Damage Simulation Study of Composite-to-Metal Interference-Fit with Multiple Structural Parameters

Abstract

Composite-to-metal interference-fit is widely used in the aerospace field, where the problem of the damage and failure of composite materials is particularly critical. A numerical study was conducted on the damage and failure of carbon fiber composite sleeves with multiple structural parameters during interference-fit assembly. ABAQUS/Explicit simulation of interference-fit was performed with the three-dimensional finite element method, and the VUMAT subroutine with three-dimensional Hashin damage was utilized to investigate the initiation and evolution of composite component damage. The effects of different relative wall thicknesses and layup sequences on the selection of maximum interference were analyzed. The results reveal that the stress distribution on the inner surface of the sleeve is more uniform with the condition of radial layup. Radial layup enables 0.2% interference at 25% wall thickness, a 100% increase over axial layup. This study contributes to addressing long-standing issues in the aerospace field, such as connection failure and insufficient fatigue life. It is of great significance for improving structural performance, reducing costs, and promoting technological innovation.

1. Introduction

Hybrid composite materials played a critical role in aerospace, automotive, and biomedical applications [1]. Specifically, T800/924C carbon fiber composites and TC4 titanium alloy were extensively employed in aerospace structures due to their superior mechanical performance [2]. Compared to alternatives such as glass fiber composites, carbon fiber composites offered a significantly higher modulus, enabling them to endure complex loading conditions typical in aerospace environments [3]. Furthermore, TC4 titanium alloy, characterized by its high strength-to-weight ratio, effectively reduced plastic deformation of metallic shafts during interference-fit assembly, thereby ensuring precise control of the interference. In aircraft structures, interference-fit connection technology of composite was adopted widely due to its advantages of high load-bearing capacity [4,5]. An appropriate interference-fit could effectively adjust load distribution and reduce the stress concentration to a certain extent by increasing the contact area between shaft and sleeve, decreasing the stress amplitude under alternating loads, delaying fatigue failure, and enhancing load capacity and fatigue life [6,7,8,9,10,11,12]. However, composite materials were brittle and highly sensitive to assembly gaps. Selecting a proper interference could significantly enhance the connection strength of composite structures, thereby improving the service life of the aircraft [13,14].

Binner Goren Kiral found that the fit pattern did not affect the failure mode, and that interference-fit improved the compressive strength of composite pin joints [15]. Wang et al. proposed that the hole–axis interference significantly influenced the fatigue life of CFRP/Al-alloy bolted joints. Initially, the fatigue life increased with an increase in hole–axis interference, but subsequently, began to decline [16]. However, in the process of forming an interference-fit assembly, the hole edge material was subjected to higher residual compressive stress, which was more susceptible to damage under external loads. Current research on interference-fit has been conducted under the assumption of proper structural installation, focusing on the effect of interference, but lacks investigation into damage mechanisms during the fitting process [17,18]. In addition to the traditional hard squeeze interference-fit connection method, Raju et al. found that the bush bolt could effectively improve the load-carrying capacity of the hybrid joint structure [19]. Domestic researchers made a brief introduction to the structure, but the lack of systematic research on the installation mechanism, and the lack of an effective basis for the selection of reliable interference in the installation process, limited the structure to applications [20,21]. Cheng et al. conducted research on the tensile properties of composite–metal joints with bush countersunk bolts, and found that the interference between the bolt and the bush was an important factor affecting the strength of the joint [22]. The moderate increase in the amount of interference improved both the stiffness and strength of the joint. It was of great engineering significance to reasonably design the amount of interference to improve the tensile properties of the joint, but the factors of damage caused by installation were not considered in the simulation process, and no clear recommendations were made for the amount of interference. Kang et al. investigated the damage of composite laminates fabricated using prepregs of different thicknesses in interference-fit, finding that ultra-thin laminates can effectively reduce damage to the hole walls during the installation of interference-fit. Compared to thick laminate, hybrid laminate and ultra-thin laminate have significantly less damage at the exit, such as matrix cracking, delamination and burr formation, and ultra-thin laminate have more significant effects [23]. Wang et al. conducted a detailed experimental study of the strength of single-lap composite joints at different layer thicknesses and interference sizes, finding that the installation force of thin-layer joints with the same interference size was less than that of thick-layer joints, and thick-layer joints showed the optimum bearing strength at 1.00% interference [24]. However, the effect of the wall thickness of the hole and the layup sequences of the composite on the damage of the composite structure and the choice of the amount of interference is not investigated.

This article simulated the interference-fit between the TC4 shaft and the composite sleeve based on a three-dimensional Hashin failure model of composite materials. Taking into account the influence of component size and composite layup sequence on interference-fit, this paper explored the mechanism of how different structural parameters affected interference-fit and the range of reliable interference selection. The work provided a basis for setting the interference in an actual composite-to-metal interference-fit.

2. Simulation Basis

2.1. Material Parameters

The sleeve material is T800/924C carbon fiber composite material, and the shaft material is TC4 titanium alloy.

Table 1. Material parameters of T800/924C [25].

Material Parameter	Numeric Value
Longitudinal elastic modulus $E_{11}$ (MPa)	145,000
Tensile modulus $E_{22}$, $E_{33}$ (MPa)	9500
Modulus of shear $G_{12}$, $G_{13}$ (MPa)	5000
Modulus of shear $G_{23}$ (MPa)	3700
Poisson ratio ${\nu }_{12}$, ${\nu }_{13}$	0.3
Poisson ratio ${\nu }_{23}$	0.21
Longitudinal tensile strain effect Xt	0.0186
longitudinal compression loss effect Xc	0.0114
Horizontal tensile strain effect Yt	0.0058
Horizontal compression loss effect Yc	0.0237
Shear failure strain $S_{12}$, $S_{13}$	0.02
Shear failure strain $S_{23}$	0.0054
Density $\mathsf{\rho }$ (t/mm3)	$1.45\times {10}^{-9}$

presents the properties of T800/924C carbon fiber composite and the properties of TC4 titanium alloy are shown in

Table 2. Material parameters of TC4 titanium alloy [26].

Material Parameter	Numeric Value
Elastic modulus E (MPa)	110,000
Poisson ratio $\nu$	0.34
Density $\mathsf{\rho }$ (t/mm3)	$4.51\times {10}^{-9}$

.

2.2. Damage Initiation Criteria

Damage initiation criteria refer to the standards for determining the stress state under which a material begins to experience damage or degradation. For composite materials, due to their complex microstructure and mechanical properties, damage initiation criteria typically need to consider multiple stress states and failure modes. There are five commonly used damage initiation criteria: the Puch criterion, the Chang–Chang criterion, the Hoffman failure criterion, the Tsai–Wu failure criterion, and the Hashin failure criterion [27,28,29,30,31,32,33].

During high-speed impact, composite material layers can experience various failure modes, including matrix tensile failure, matrix compressive failure, fiber tensile failure, and others. To more accurately simulate the failure behavior of composite material layers, this study employs the three-dimensional Hashin damage initiation criterion based on strain [34], which includes six failure forms: fiber tension, fiber compression, matrix tension, matrix compression, in-plane shear, and out-of-plane shear, with the following expressions:

Fiber stretching (${\epsilon }_{11}\ge 0$):

$$f_{ft}={({\displaystyle \frac{{\epsilon }_{11}}{{\epsilon }_{11T}}})}^2+{({\displaystyle \frac{{\epsilon }_{12}}{{\epsilon }_{12f}}})}^2+{({\displaystyle \frac{{\epsilon }_{13}}{{\epsilon }_{13f}}})}^2$$

(1)

Fiber compression (${\epsilon }_{11}<0$):

$$f_{fc}={({\displaystyle \frac{{\epsilon }_{11}}{{\epsilon }_{11C}}})}^2$$

(2)

Matrix stretching (${\epsilon }_{22}+{\epsilon }_{33}\ge 0$):

$$f_{mt}={({\displaystyle \frac{{\epsilon }_{11}+{\epsilon }_{33}}{{\epsilon }_{22T}}})}^2+{({\displaystyle \frac{{\epsilon }_{12}}{{\epsilon }_{12f}}})}^2+{({\displaystyle \frac{{\epsilon }_{13}}{{\epsilon }_{13f}}})}^2+{\displaystyle \frac{{\epsilon }_{23}^2-{\epsilon }_{22}{\epsilon }_{33}}{{\epsilon }_{23f}}}$$

(3)

Matrix compression (${\epsilon }_{22}+{\epsilon }_{33}<0$):

$$f_{mc}={({\displaystyle \frac{{\epsilon }_{22}+{\epsilon }_{33}}{{\epsilon }_{22C}}})}^2$$

(4)

In-plane shear (1–2 plane):

$$f_{ip}={({\displaystyle \frac{{\epsilon }_{12}}{{\epsilon }_{12f}}})}^2$$

(5)

Exterior shear (1–3 and 2–3 plane):

$$f_{op}={({\displaystyle \frac{{\epsilon }_{13}}{{\epsilon }_{13f}}}+{\displaystyle \frac{{\epsilon }_{23}}{{\epsilon }_{23f}}})}^2$$

(6)

Among these, 1, 2, and 3 represent the fiber direction (X), the in-plane perpendicular direction (Y), and the out-of-plane perpendicular direction (Z), respectively. ${\epsilon }_{11T}$ and ${\epsilon }_{11C}$ are the tensile and compressive failure strains in the fiber direction, while ${\epsilon }_{22T}$ and ${\epsilon }_{22C}$ are the tensile and compressive failure strains in the matrix direction. ${\epsilon }_{ijf}$ (i, j = 1, 2, 3, i ≠ j) represents the shear failure strain. Composite materials can simultaneously exhibit multiple damage modes. When any damage parameter ($f_{ft}$, $f_{fc}$, $f_{mt}$, $f_{mc}$, $f_{ip}$, $f_{op}$) reaches 1, the corresponding damage initiation criterion is satisfied for the element, followed by stiffness degradation.

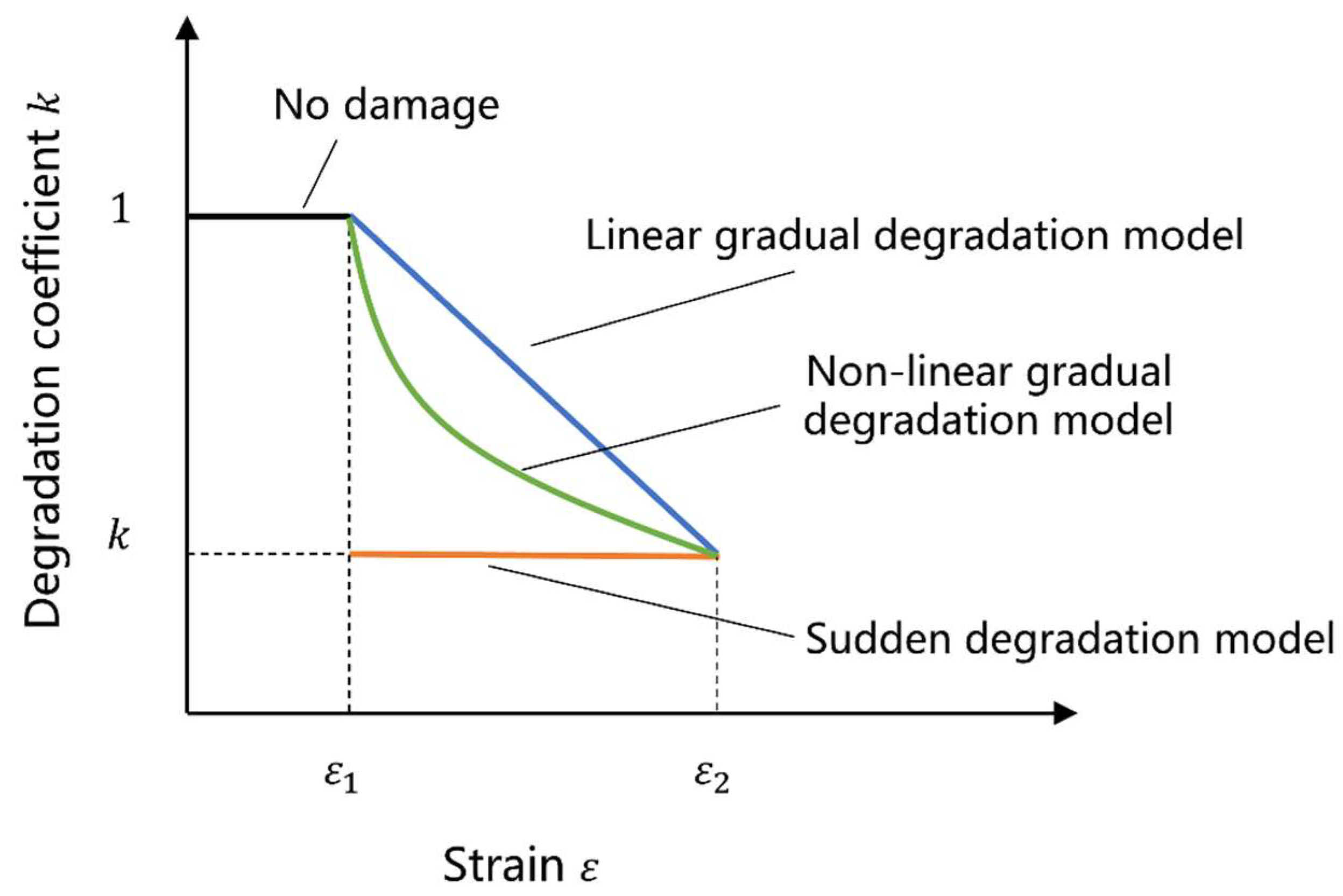
2.3. Damage Evolution Model

Damage evolution describes the rate of material stiffness degradation after reaching the corresponding damage initiation criteria. When the material satisfies the damage initiation criterion, the element is deemed to begin damage, losing its load-bearing capacity and experiencing stiffness degradation [35]. Figure 1 shows three degradation models for the mechanical properties of composite materials. The sudden degradation model represents that when composite materials reach a certain stress or strain threshold, their mechanical properties suddenly degrade; the linear gradual degradation model indicates that as stress or strain increases, the material’s load-bearing capacity decreases linearly, with a constant rate of performance degradation; the nonlinear gradual degradation model indicates that as stress or strain increases, the rate of capacity decrease gradually increases.

The definition of damage evolution consists of two components. The first component involves the effective displacement at complete failure relative to the effective displacement at the onset of damage or the energy dissipated due to failure (also referred to as fracture energy). The second component of the damage evolution definition specifies the nature of the evolution of the damage variable $D$ between the onset of damage and final failure. This can be achieved by defining linear or exponential softening laws or directly specifying $D$ as a tabular function of the effective displacement relative to the effective displacement at the onset of damage.

This paper considers the brittleness of carbon fiber reinforced composites, where the degradation of stiffness in composites macroscopically manifests as a reduction in the material’s elastic modulus, with the reduction scheme shown in

Table 3. Stiffness degradation scheme.

Damage Pattern	E11	E22	E33	G12	G23	G13
Fiber stretching	0.01	1	1	0.01	1	0.01
Fiber compression	0.01	1	1	0.01	1	0.01
Matrix stretching	1	0.01	0.01	0.01	0.01	0.01
Matrix compression	1	0.01	0.01	0.01	0.01	0.01
In-plane shear	1	1	1	0.01	1	1
Exterior shear	1	1	1	1	0.01	0.01

. If a composite unit experiences matrix failure, fiber failure, or shear failure, the unit is then deleted.

3. Modeling and Simulation

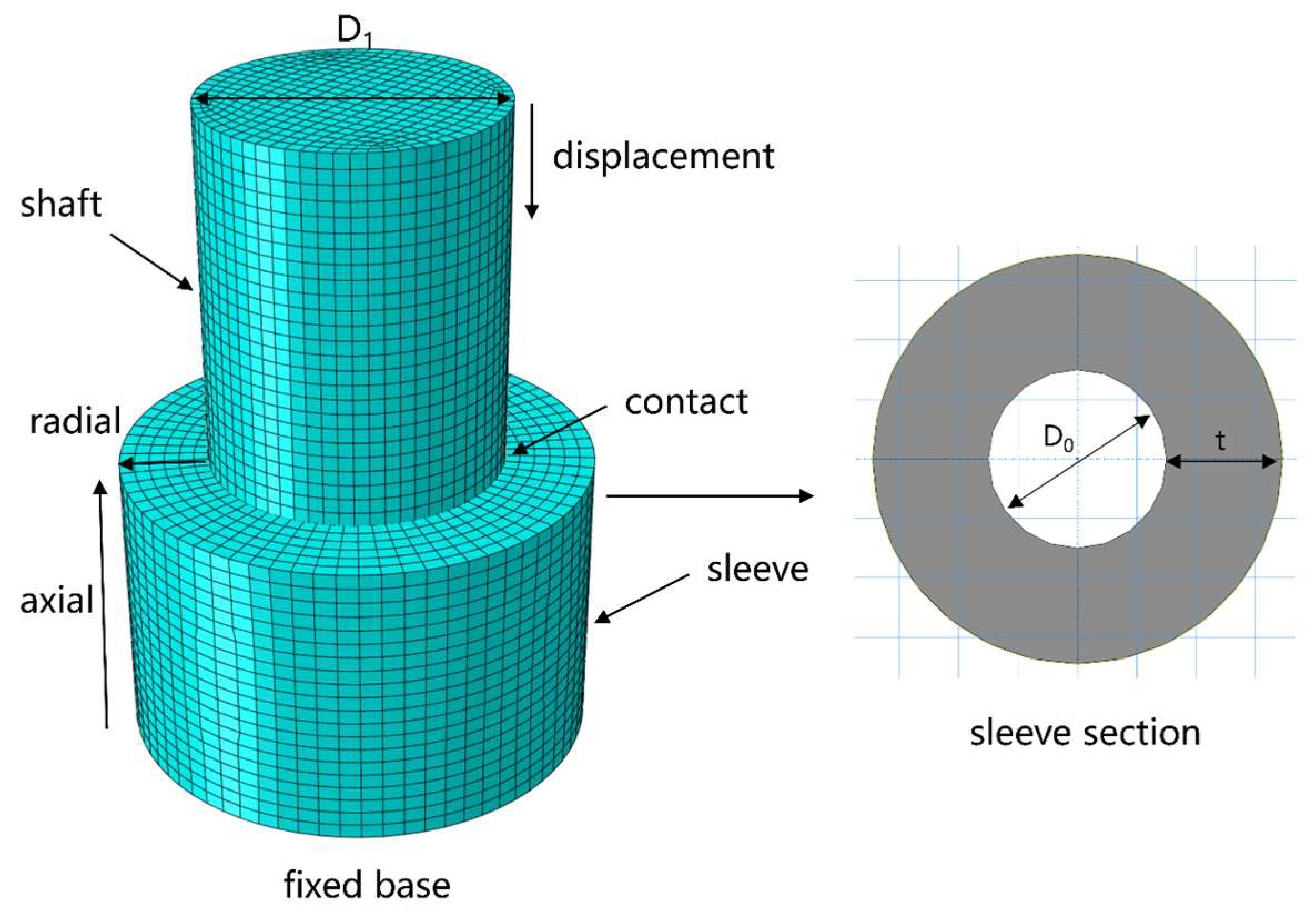
3.1. Interference-Fit Numerical Model

A three-dimensional finite element model of the interference-fit between the T800/924C composite material shaft and the TC4 titanium alloy shaft is established, as shown in Figure 2. The shaft height is 25 mm, the sleeve height is 20 mm, the inner diameter of the sleeve $D_0$ is 20 mm, and the sleeve has two layup sequences: axial and radial. The interference is altered by changing the shaft diameter. To effectively simulate the progressive damage process, the ABAQUS 2022 finite element analysis software is used to model the problem using explicit dynamics, employing the three-dimensional deformable solid C3D8R. Considering both computational time and result accuracy, the element size is selected as 1 mm.

Interference $\mathrm{I}$ is defined as follows:

$$\mathrm{I}={\displaystyle \frac{D_1-D_0}{D_0}}\times 100\%$$

(7)

In the formula presented, $D_1$ indicates the diameter of the shaft.

Relative wall thickness $\mathsf{\delta }$ is defined as follows:

$$\mathsf{\delta }={\displaystyle \frac{t}{D_0}}\times 100\%$$

(8)

In the formula presented, $t$ indicates the wall thickness of the sleeve.

Figure 2 gives the boundary conditions for the finite element model. The contact type between the TC4 shaft body and the inner surface of the composite material sleeve is set as friction contact, the outer surface of the pin sleeve shaft is set as contact surface, the inner surface of the base cylinder is set as target surface, and the friction coefficient f is 0.3 [36,37]. The interference is achieved by controlling the contact offset. The damage mechanical behavior of composites is simulated by using the Hashin failure criterion and the progressive damage model. During the numerical calculation, the VUMAT sub-program is called to evaluate the onset and evolution of damage in the simulation model.

3.2. Simulation of Interference-Fit with Different Relative Wall Thicknesses and Layup Sequences of the Sleeve

Based on the aforementioned modeling to study the effect of different wall thicknesses on the interference. Considering that different sleeve wall thicknesses directly affect the interference, and due to weight restrictions, the relative wall thickness δ of the sleeve generally does not exceed 25%. Therefore, δ is taken as 5%, 10%, 15%, 20%, and 25% to simulate the damage conditions of the sleeve under interference of 0.1%, 0.2%, and 0.3%.

In addition to the relative wall thickness of the composite sleeve, the layup sequences of the composite prepreg during sleeve fabrication also impact the interference. Two different layup sequences, axial and radial, were set up and simulations were conducted separately for each.

3.3. Validation of Structural Parameters in Composite Structure Design

To ensure the accuracy of the influence patterns of the structural parameters derived from simulations, the sleeve was reconfigured with an inner diameter D₀ of 40 mm and subjected to the same simulations as described above.

4. Results and Discussion

This section examines the damage conditions of composite sleeves under various structural parameters during interference-fit. Through the analysis of damage patterns, this study summarizes the selection rules for interference under different structural parameters and validates the model.

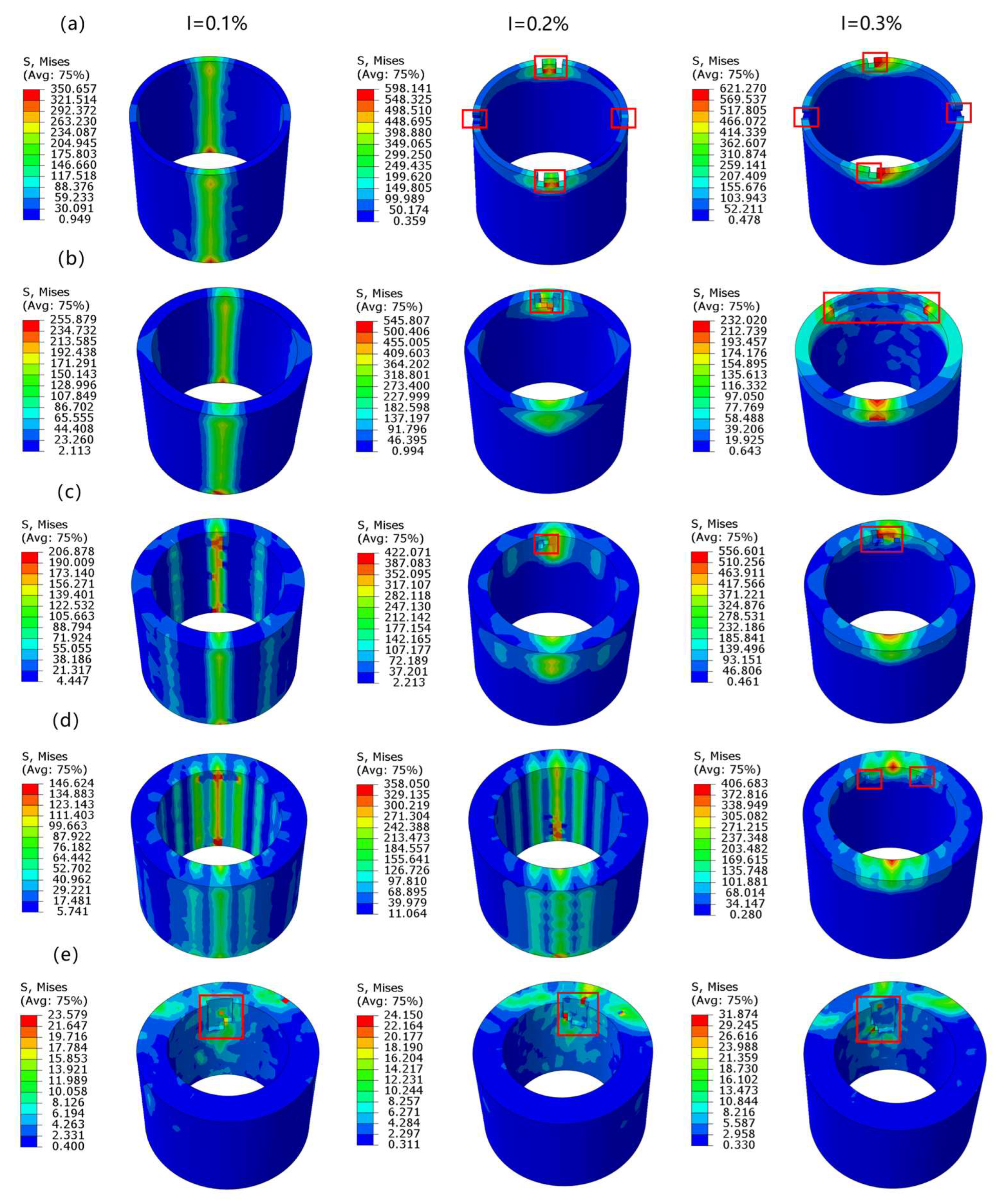
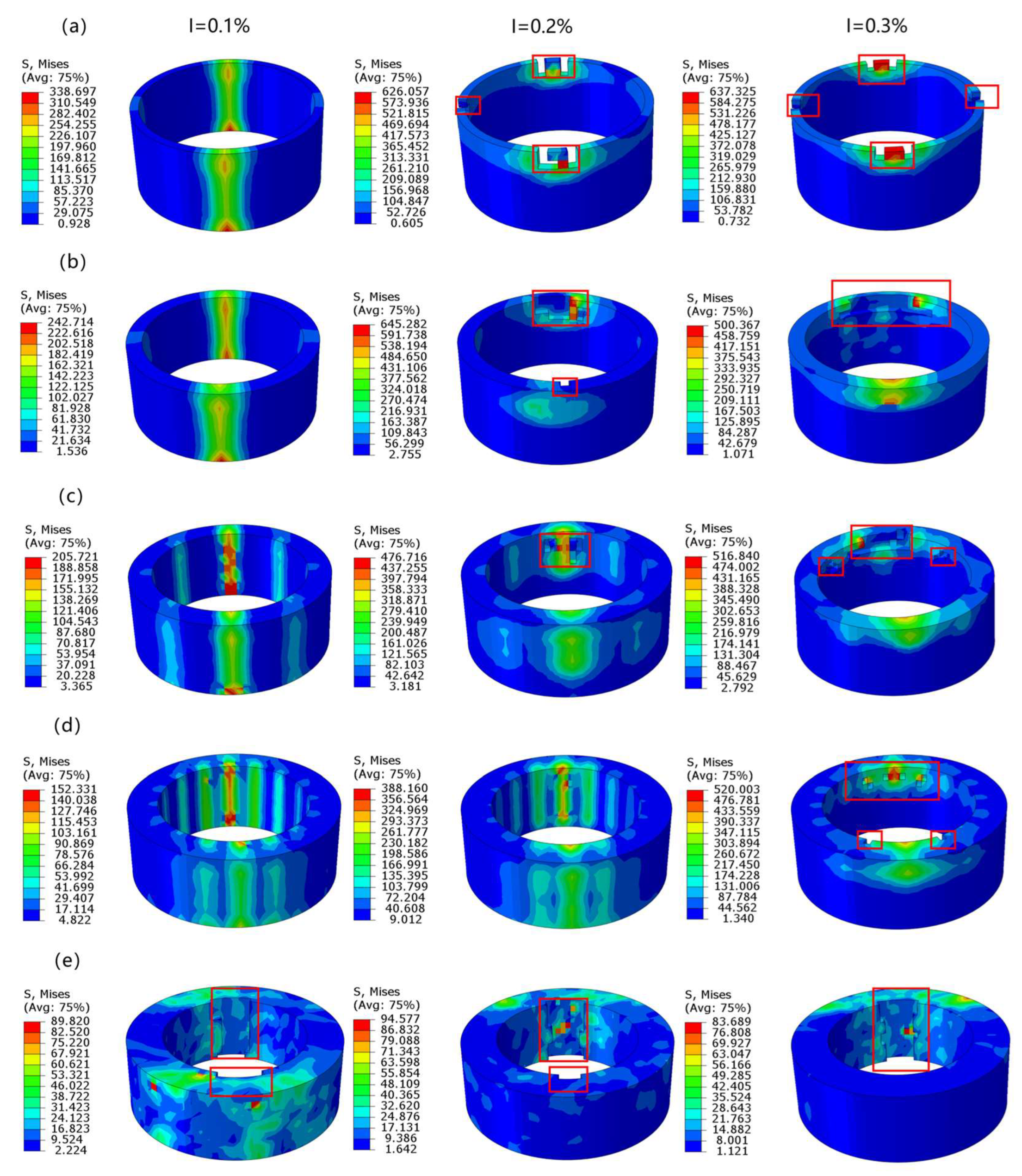
4.1. Simulation Results Under Axial Layup

When the relative wall thickness $\mathsf{\delta }$ is 5%, 10%, 15%, 20%, and 25%, respectively, the damage conditions of the composite sleeve in the shaft-sleeve interference-fit model under 0.1%, 0.2%, and 0.3% interference are shown in Figure 3. The red frame in the figure indicates the area of the injury. As shown in the figure, when the relative wall thickness is 5%, 10%, and 15%, the sleeve can only complete the interference-fit at an interference of 0.1%, which is due to the excessively low strength of the sleeve caused by the small wall thickness. When the relative wall thickness is 20%, the sleeve can further complete the interference-fit at an interference of 0.2%. However, when the relative wall thickness is 25%, the sleeve fails in all three interference conditions. This is because, under the axial layup sequence, the stress direction on the inner surface of the sleeve during interference-fit is perpendicular to the direction of the fibers, which has poor ductility. When the sleeve wall thickness is too large, the stress accumulation during the interference-fit process is more likely to cause damage to the inner surface of the sleeve. From the above results, it can be concluded that for composite sleeves with axial layup, both excessively large or small relative wall thicknesses are detrimental to interference-fit, and the maximum range of selectable interference is achieved when the relative wall thickness is 20%.

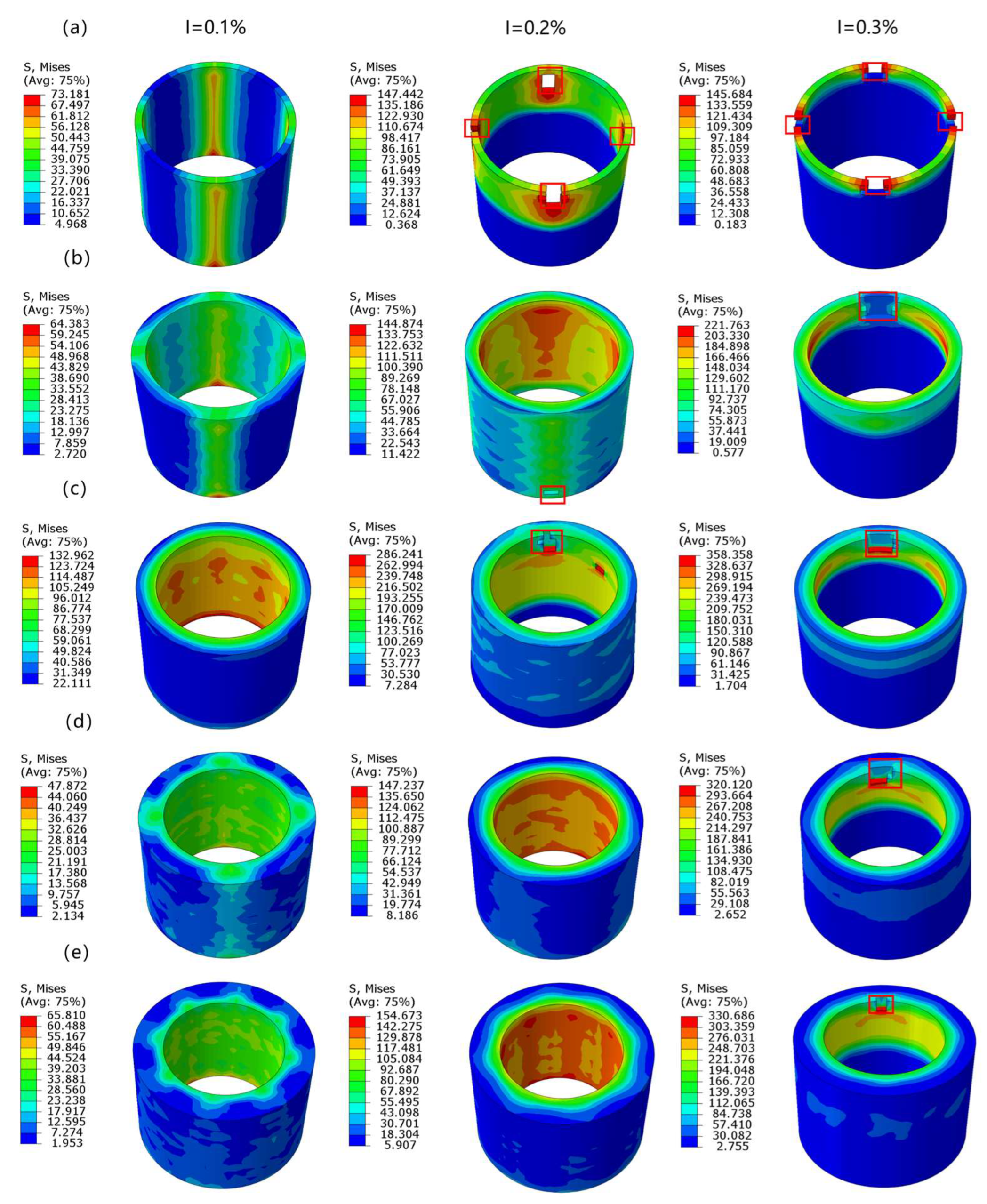
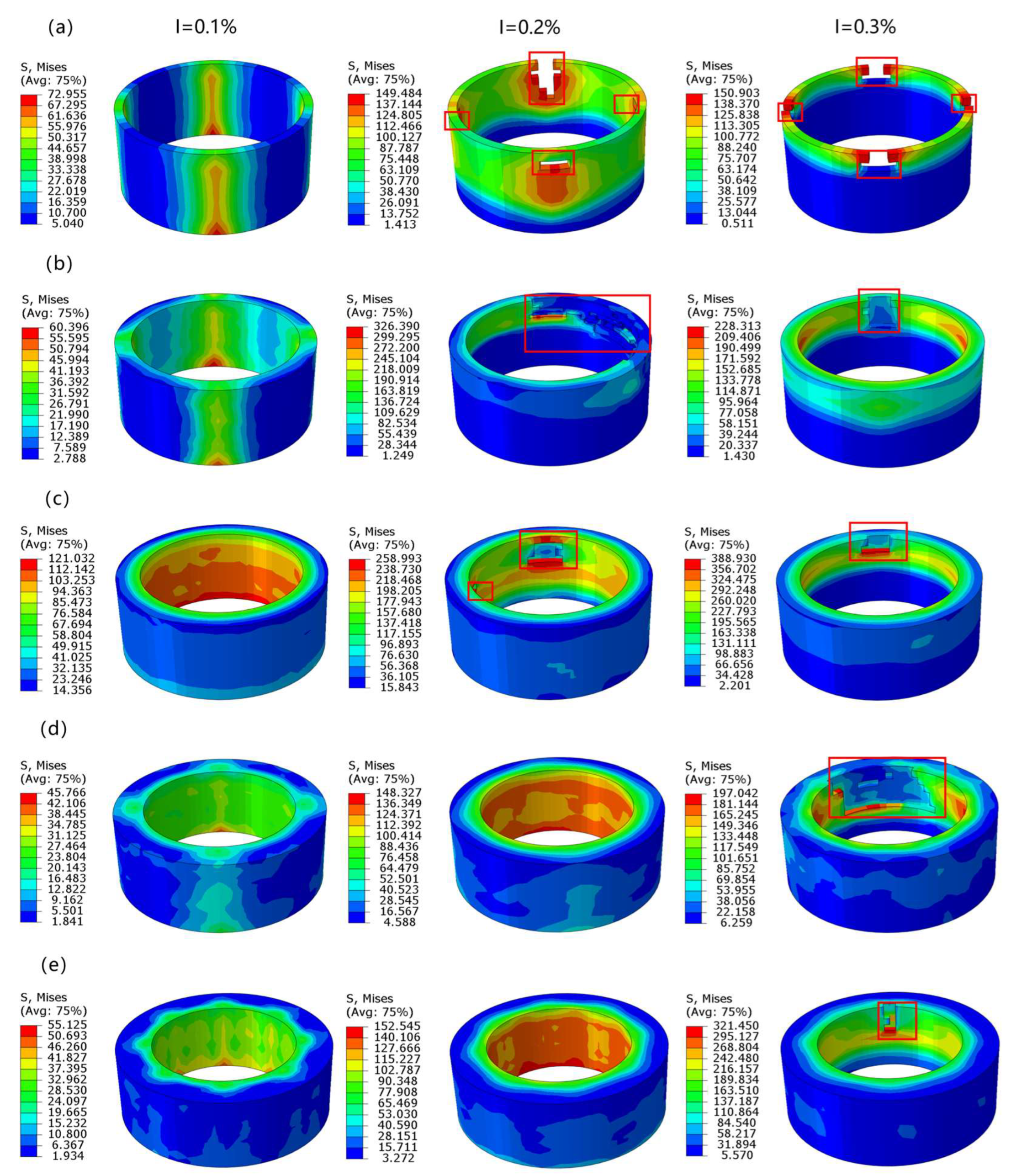
4.2. Simulation Results Under Radial Layup

The damage conditions of the sleeve during interference-fit under radial layup with relative wall thickness δ of 5%, 10%, 15%, 20%, and 25% at interference of 0.1%, 0.2%, and 0.3% are shown in Figure 4. As can be seen from the figure, when the relative wall thickness is 5%, 10%, and 15%, the sleeve can complete the interference-fit at an interference of 0.1% without damage failure. When the relative wall thickness is 20% and 25%, the sleeve can further complete the interference-fit at an interference of 0.2%. This is because, during interference-fit, the primary loading direction of the sleeve is perpendicular to the inner wall of the sleeve and outward. Radial layup allows the fiber direction with the highest strength to bear the load, while axial layup bears the load in the weaker matrix direction. Therefore, while axial layup achieves interference-fit at relative wall thicknesses of 5%, 10%, 15%, and 20%, radial layup further achieves interference-fit at a relative wall thickness of 25%. Combining the results from both layup sequences, it is observed that the interference does not exceed 0.2% in all successful interference-fit experiments. This is due to the material properties of T800/924C limiting the selection of maximum interference. Additionally, comparing the stress contour plots from different layup sequences reveals that the stress distribution on the inner surface of the sleeve during interference-fit differs. Under radial layup, the stress distribution on the inner surface of the sleeve is more uniform.

4.3. Verification of Accuracy of Simulation Results

The damage conditions of the shaft-sleeve interference-fit model under axial and radial layup sequences at 0.1%, 0.2%, and 0.3% interference with an inner diameter $D_0$ of 40 mm and relative wall thickness δ of 5%, 10%, 15%, 20%, and 25% are shown in Figure 5 and Figure 6. As seen from Figure 5, when the relative wall thickness is 5%, 10%, and 15%, the sleeve can only complete the interference-fit at 0.1% interference, which is due to the excessively small wall thickness leading to insufficient strength of the sleeve. When the relative wall thickness is 20%, the sleeve can further complete the interference-fit at 0.2% interference. However, when the relative wall thickness is 25%, the sleeve fails in all three interference conditions. As seen in Figure 6, when the relative wall thickness is 5%, 10%, and 15%, the sleeve can complete the interference-fit at 0.1% interference without damage failure. When the relative wall thickness is 20% and 25%, the sleeve can further complete the interference-fit at 0.2% interference.

Figure 7 shows the variation in the maximum interference with relative wall thickness δ under axial and radial layups for two sizes $D_0$ = 20 mm and $D_0$ = 40 mm. It can be seen that, under axial layup, the maximum interference $I_{max}$ for both sizes reaches 0.1% when the relative wall thickness is 5%, 10%, and 15%; $I_{max}$ reaches 0.2% when the relative wall thickness is 20%; interference-fit cannot be achieved when the relative wall thickness is 25%. Under radial layup, the maximum interference $I_{max}$ reaches 0.1% when the relative wall thickness is 5%, 10%, and 15%; and $I_{max}$ reaches 0.2% when the relative wall thickness is 20% and 25%. These results validate the accuracy of the simulation results, indicating that, under two different layup methods, the maximum interference $I_{max}$ is uniquely determined once the relative wall thickness δ of the sleeve is fixed, regardless of the specific dimensions of the sleeve.

5. Conclusions

Based on the three-dimensional Hashin failure model, the paper simulated the interference-fit between the TC4 titanium alloy shaft and T800/924C composite sleeve with different relative wall thicknesses and layup sequences using 3D finite element analysis. The damage results of the sleeve were analyzed with various structural parameters and the following conclusions were drawn:

(1)

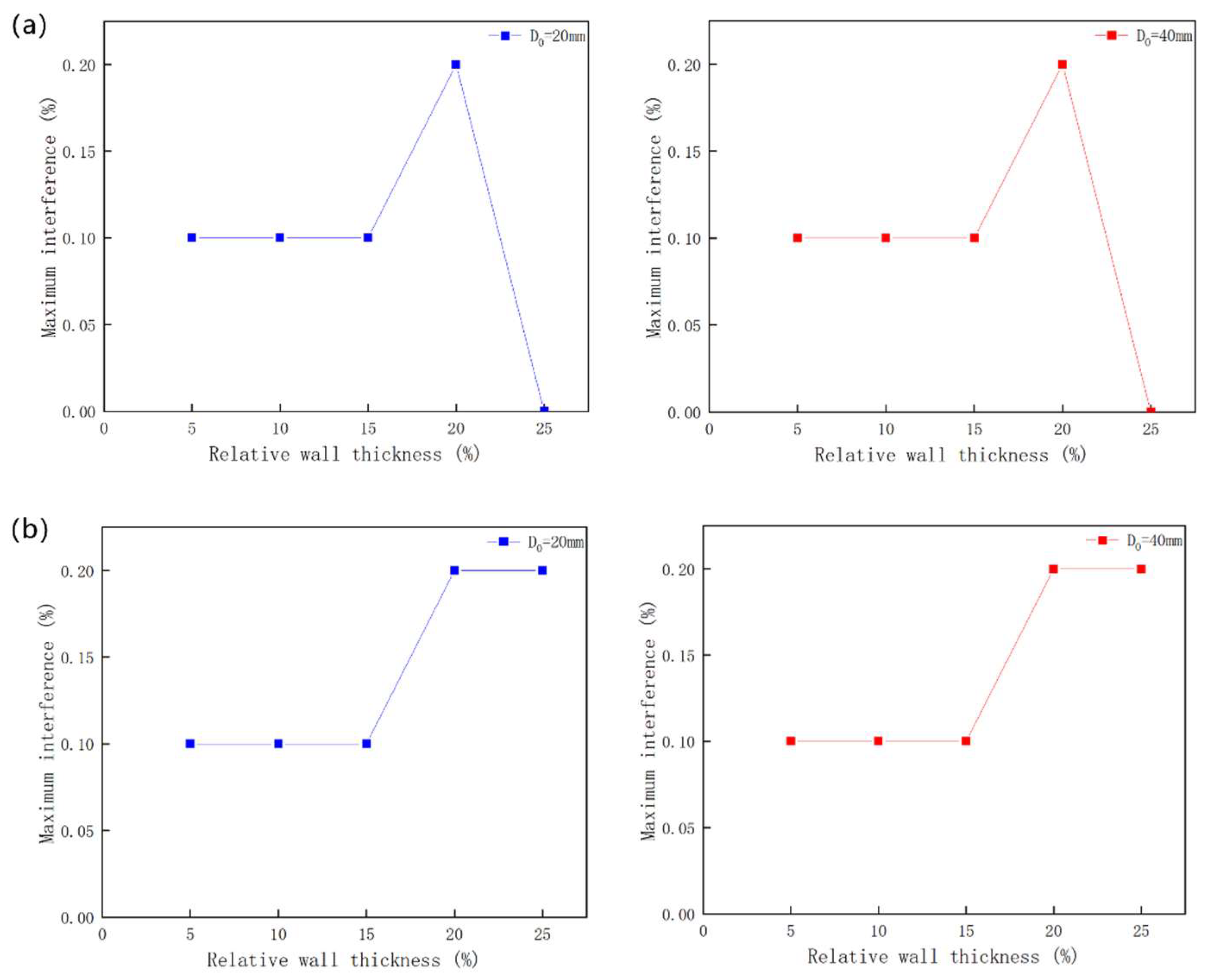
For the T800/924C carbon fiber composite sleeve under axial layup, the maximum interference is 0.1% at relative wall thicknesses of 5%, 10%, and 15%, reaches 0.2% at a relative wall thickness of 20%, and an interference-fit is not possible when the relative wall thickness is 25%.

(2)

With the condition of radial layup, the maximum interference is 0.1% at relative wall thicknesses of 5%, 10%, and 15%, and reaches 0.2% at relative wall thicknesses of 20% and 25%.

(3)

With the condition of radial layup, the stress distribution on the inner surface of the sleeve is more uniform. Radial layup effectively avoids local stress concentration compared to axial layup. Radial layup can effectively address the issue of the inability to achieve interference-fit when the relative wall thickness is too large in the axial layup, enabling a maximum interference of 0.2% at a relative wall thickness of 25%.