Parametric Analyses of the Influence of Temperature, Load Duration, and Interlayer Thickness on a Laminated Glass Structure Exposed to Out-of-Plane Loading
Abstract
1. Introduction
1.1. Glass as Material
1.2. Laminated Glass—Type of Interlayers
1.3. Influence of Interlayers’ Behavior on the Capacity of Laminated Glass Plates
| PVB (Structural) | EVA [34] | Ionoplast | |
|---|---|---|---|
| Density | 1070 kg/m3 | 970 kg/m3 | 950 kg/m3 |
| Poisson’s ratio | 0.476 | 0.32 | 0.458 |
| Glass transition temperature [24] | 12–25 °C | −28 °C | 55 °C |
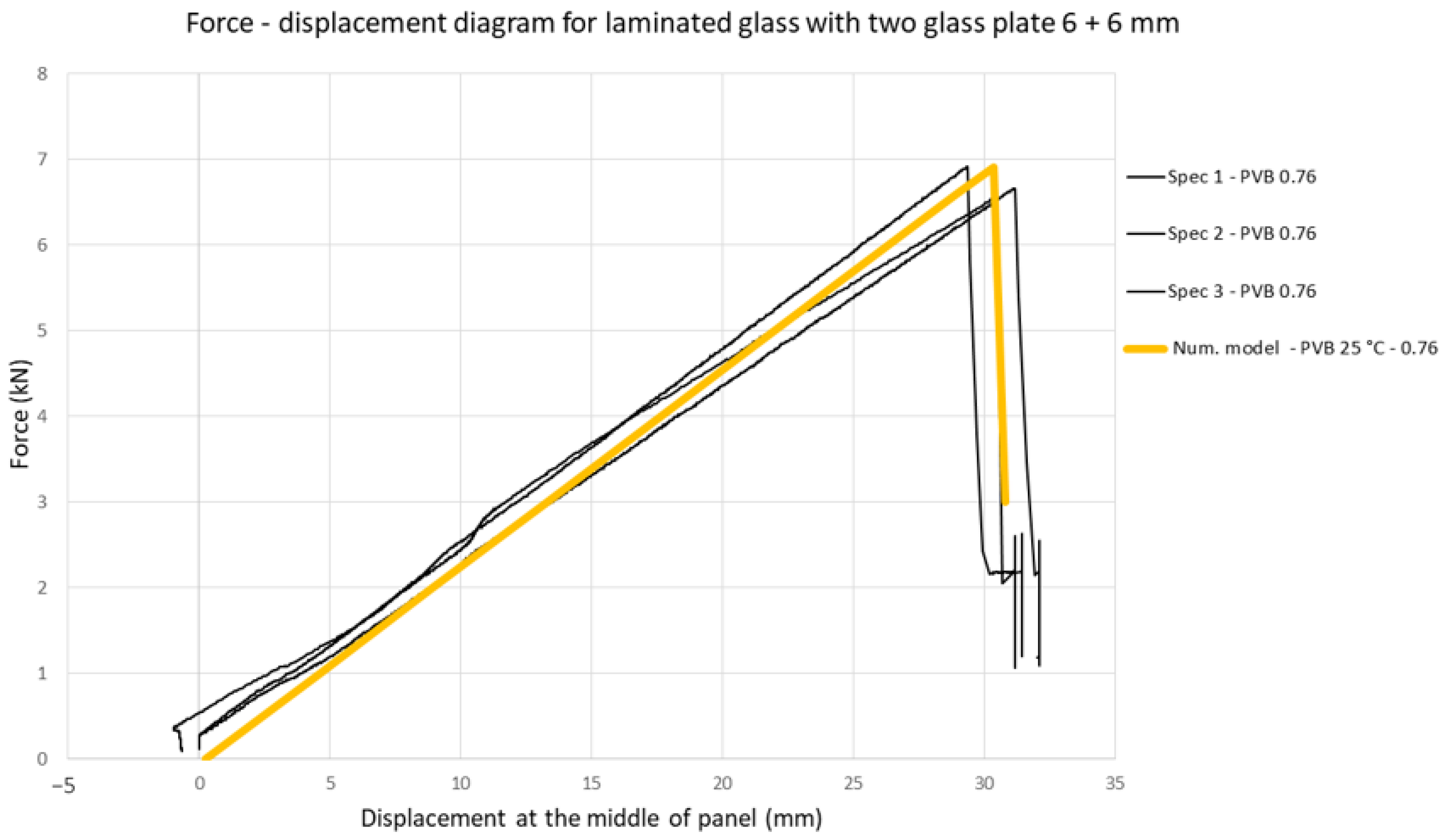
2. Experimental Tests
3. Numerical Model
4. A Parametric Study of Interlayer Properties on Bearing Capacity of Laminated Glass Panels
+ F10 ∙0z + F11 ∙1x ∙ z + F12 ∙ y ∙ z + F13 ∙ x2 ∙xz + F14 ∙ x ∙xy ∙ z + F15 ∙ y2 ∙yz + F16 ∙6x2
∙6y ∙ z + F17 ∙7x ∙ y2 ∙ z+ F18 ∙8x2 ∙8y2 ∙8z + F19 ∙z2 + F20 ∙0x ∙ z2 + F21 ∙1y ∙ z2 + F22
∙2x2 ∙2z2 + F23 ∙3x ∙ y ∙ z2 + F24 ∙4F2 ∙4F2 +F25 ∙ x2 ∙ y ∙ z2 + F26 ∙ x ∙ y2 ∙ z2 + F27 ∙7 2 ∙
y2 ∙ z2
5. Conclusions
- Comparing deflections, laminated glass panels with ionoplast interlayers provide generally higher stiffness than the same model with PVB (Saflex DG41) interlayers does. This behavior is expected, because an ionoplast interlayer is an ionomer-based material that provides the highest level of structural performance [23], which contributes to increasing the stiffness of laminated glass and thus causes less deflection.
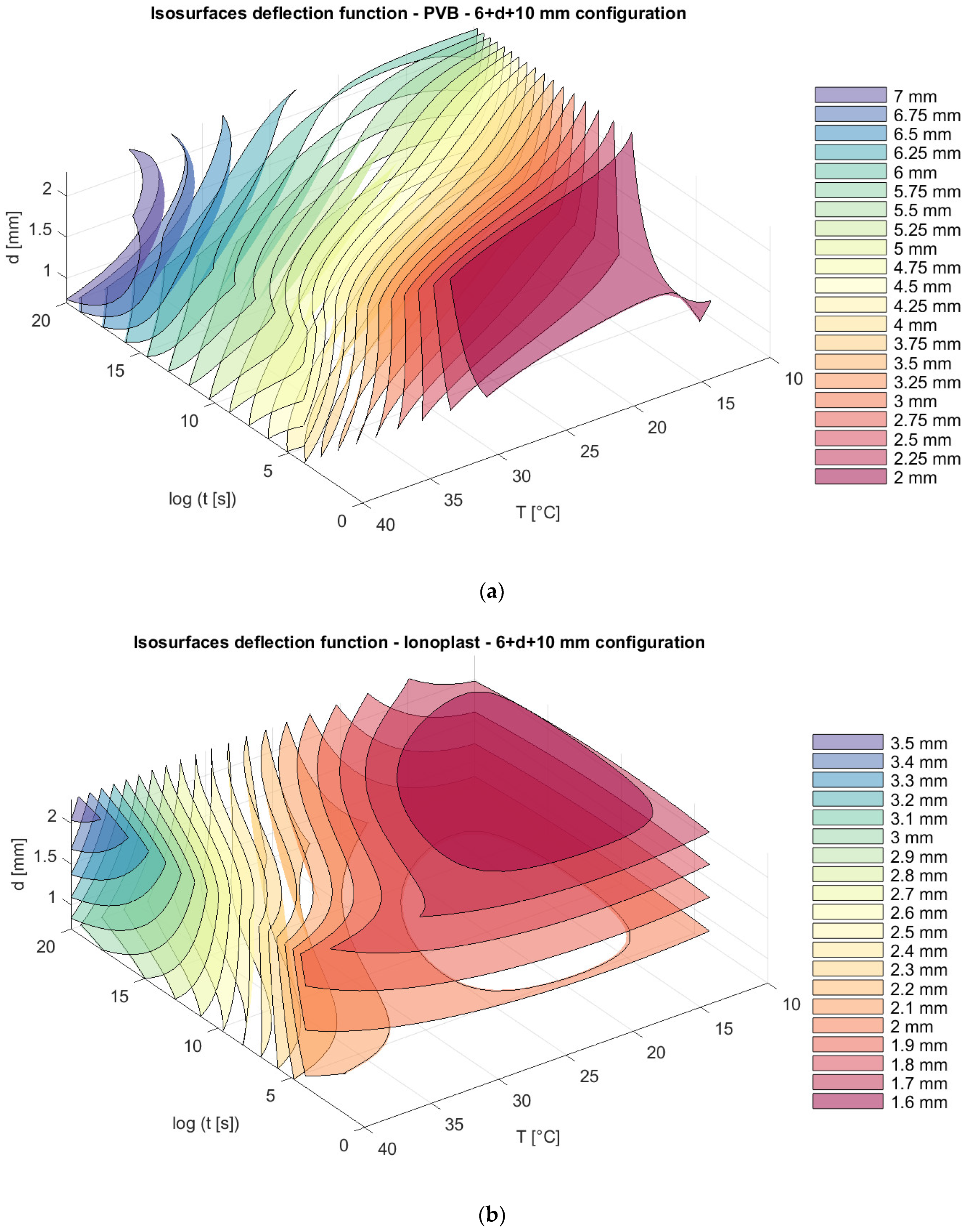
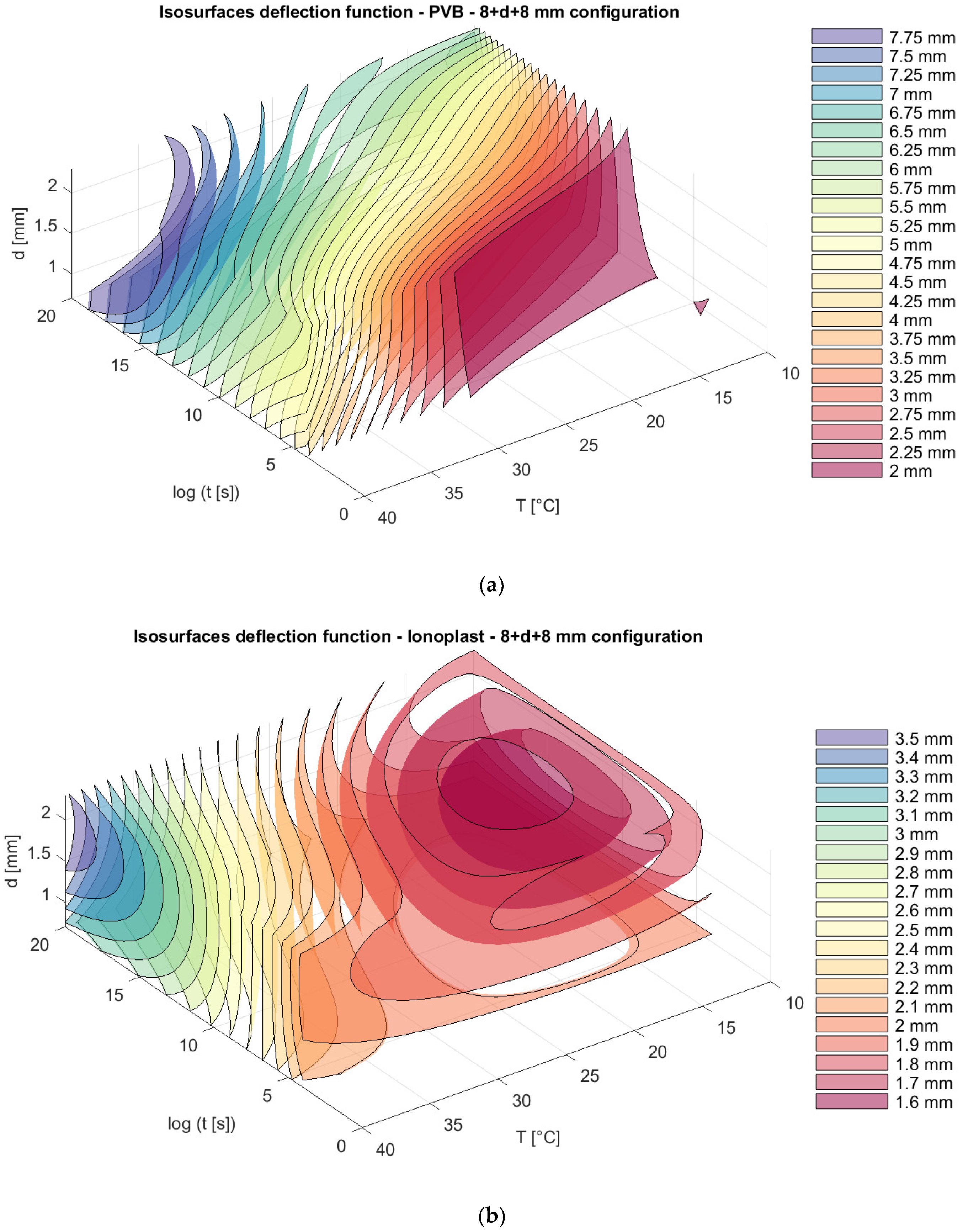
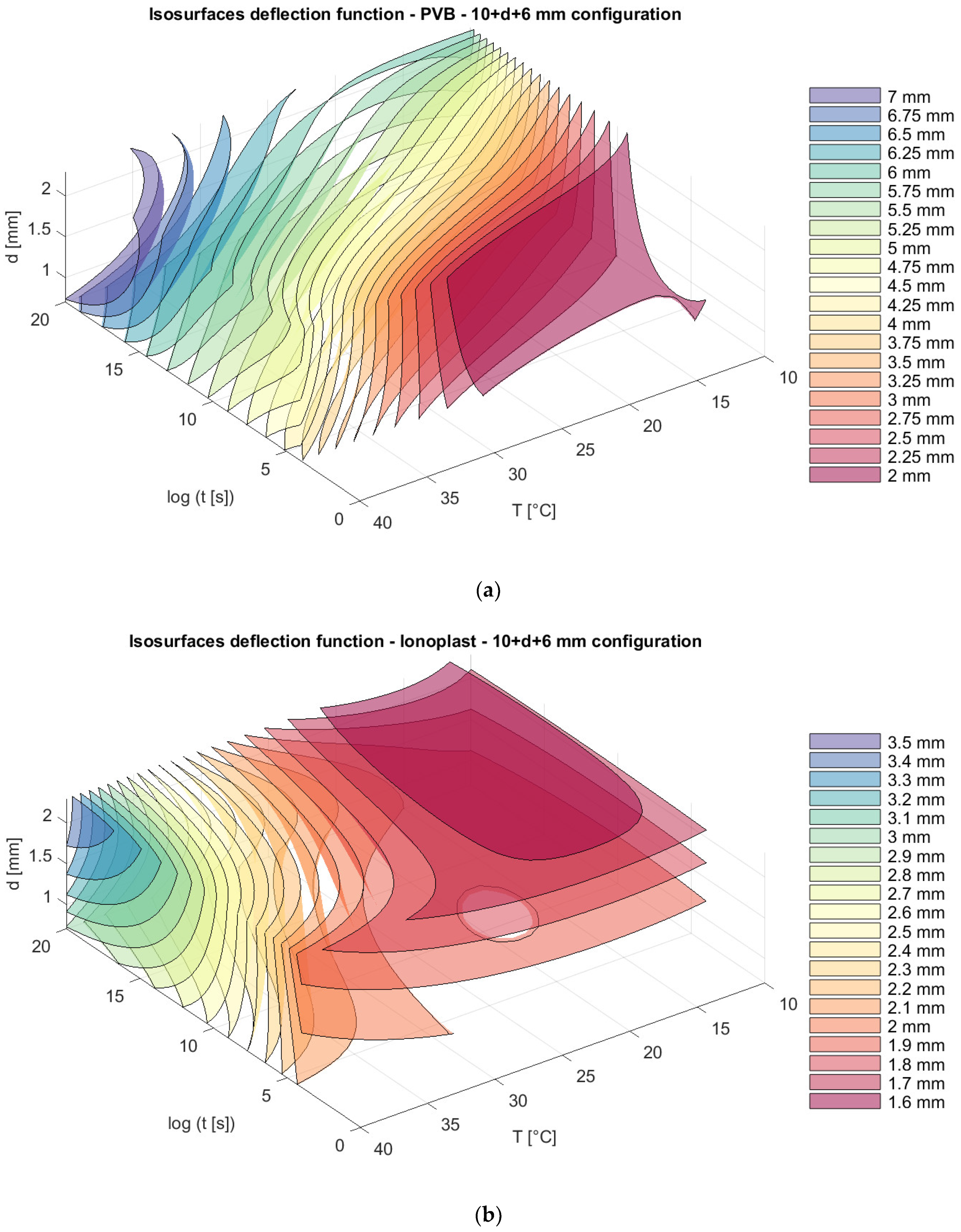
- Taking into account that the total thickness of glass in all of the models is 16 mm, we can see that, for nonsymmetrical panels, the position of thinner and thicker plates (6 mm + 10 mm and 10 mm + 6 mm) does not provide a difference in the total deflection of the panels, and these values are slightly lower than those from symmetrical dispositions of plates (8 mm + 8 mm). The dominant influence on the size of the deflection is the total thickness of the panels, which justifies the different approaches of calculating the effective thickness of the panels.
- When comparing the stresses on the bottom plate, we can see that due to the asymmetric dispositions of the plate, higher stresses occur in cases where the plate is thicker at the bottom. This behavior is expected, because that part of the panel has a higher bending stiffness, withstanding higher load levels, resulting in higher stresses in that part. In the case of symmetrical dispositions (8 mm + 8 mm) of the panel, the expected stress values are between the previously mentioned two load limits for laminated glass with a panel disposition of 6 mm + 10 mm and 10 mm + 6 mm.
- For lower temperatures (up to 20 °C), an increase in the interlayer thickness, for the ionoplast model, shows the positive effect of increasing the panel stiffness, resulting in lower deflection. However, for temperatures above 35 °C, that effect disappears, and for long-term loading, the increase in the interlayer thickness brings a slightly higher deflection of the panel. A different trend is observed in the model with PVB (Saflex DG41, EASTMAN, Kingsport, TN, USA) interlayers, where it can be seen that at lower temperatures and shorter loadings, the increased thickness of the interlayers does not provide any influence on the deflection. The PVB (Saflex DG41) will be able to transfer a significant amount of shear force between the glass plates, and in this case the laminated panel has a bending stiffness of a similar magnitude to an equivalent monolithic panel of the same overall thickness.
- For higher temperatures and longer loadings, the increase in the interlayer thickness shows an unfavorable effect, where deflection is increased with an increased interlayer thickness. The same effect is visible for the load duration, where we observe greater deflections for longer load durations in both models, but this trend is more pronounced in models with PVB (Saflex DG41) interlayers. When this type of PVB is exposed to higher temperatures, its stiffness decreases, which is clearly manifested by the fall of Young’s modulus, and it begins to behave in a similar manner to rubber, which results in an increased deflection.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Deflection 10 + 2.28 + 6 (mm) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 1.642 | 1.960 | 4.185 | 5.737 | 1.645 | 1.652 | 1.657 | 1.660 |
| 25 | 1.673 | 4.255 | 5.586 | 6.141 | 1.660 | 1.690 | 1.739 | 1.820 |
| 30 | 1.920 | 5.580 | 6.048 | 6.753 | 1.684 | 1.770 | 2.232 | 2.081 |
| 40 | 5.200 | 6.320 | 6.996 | 7.421 | 1.865 | 2.964 | 3.329 | 3.467 |
| Bottom panel stress 10 + 2.28 + 6 (MPa) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 11.820 | 12.273 | 14.893 | 21.577 | 11.809 | 11.821 | 11.834 | 11.840 |
| 25 | 11.865 | 15.169 | 20.949 | 23.349 | 11.834 | 11.885 | 11.963 | 12.090 |
| 30 | 12.218 | 20.897 | 22.930 | 25.903 | 11.874 | 12.012 | 12.605 | 13.226 |
| 40 | 19.276 | 24.102 | 26.977 | 28.790 | 12.150 | 13.382 | 13.739 | 13.882 |
| Deflection 10 + 1.52 + 6 (mm) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 1.835 | 2.077 | 3.909 | 5.403 | 1.837 | 1.843 | 1.847 | 1.852 |
| 25 | 1.858 | 3.963 | 5.245 | 5.827 | 1.848 | 1.872 | 1.907 | 1.971 |
| 30 | 2.040 | 5.234 | 5.724 | 6.500 | 1.866 | 1.931 | 2.279 | 2.732 |
| 40 | 4.860 | 6.015 | 6.778 | 7.279 | 2.000 | 2.858 | 3.158 | 3.274 |
| Bottom panel stress 10 + 1.52 + 6 (MPa) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 12.614 | 12.910 | 14.676 | 19.998 | 12.605 | 12.612 | 12.621 | 12.624 |
| 25 | 12.641 | 14.731 | 19.339 | 21.891 | 12.620 | 12.652 | 12.703 | 12.783 |
| 30 | 12.886 | 19.266 | 21.429 | 24.810 | 12.646 | 12.732 | 13.159 | 13.615 |
| 40 | 17.619 | 22.710 | 26.000 | 28.180 | 12.822 | 13.733 | 14.000 | 14.113 |
| Deflection 10 + 0.76 + 6 (mm) | Deflection 10 + 0.89 + 6 (mm) | |||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 2.064 | 2.210 | 3.457 | 4.736 | 1.978 | 1.981 | 1.984 | 1.988 |
| 25 | 2.077 | 3.447 | 4.561 | 5.157 | 1.984 | 2.000 | 2.023 | 2.065 |
| 30 | 2.181 | 4.525 | 5.031 | 5.907 | 1.996 | 2.038 | 2.266 | 2.576 |
| 40 | 4.177 | 5.323 | 6.241 | 6.922 | 2.083 | 2.664 | 2.881 | 2.967 |
| Bottom panel stress 10 + 0.76 + 6 (MPa) | Bottom panel stress 10 + 0.89 + 6 (MPa) | |||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 13.508 | 13.630 | 14.740 | 16.885 | 13.133 | 13.138 | 13.140 | 13.145 |
| 25 | 13.516 | 14.725 | 16.105 | 18.756 | 13.142 | 13.161 | 13.185 | 13.232 |
| 30 | 13.605 | 15.964 | 18.195 | 22.075 | 13.157 | 13.203 | 13.481 | 13.813 |
| 40 | 15.320 | 19.515 | 23.544 | 26.557 | 13.256 | 13.898 | 14.103 | 14.182 |
| Deflection 6 + 2.28 + 10 (mm) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 1.640 | 1.960 | 4.183 | 5.733 | 1.645 | 1.652 | 1.657 | 1.660 |
| 25 | 1.670 | 4.253 | 5.582 | 6.135 | 1.660 | 1.690 | 1.739 | 1.825 |
| 30 | 1.919 | 5.575 | 6.042 | 6.742 | 1.680 | 1.770 | 2.232 | 2.810 |
| 40 | 5.201 | 6.312 | 6.982 | 7.395 | 1.865 | 2.964 | 3.329 | 3.467 |
| Bottom panel stress 6 + 2.28 + 10 (MPa) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 11.440 | 12.784 | 19.698 | 24.349 | 11.444 | 11.490 | 11.522 | 11.559 |
| 25 | 11.619 | 19.910 | 23.896 | 25.557 | 11.536 | 11.710 | 11.940 | 12.301 |
| 30 | 12.631 | 23.876 | 25.274 | 27.401 | 11.674 | 12.083 | 13.761 | 15.617 |
| 40 | 22.746 | 26.094 | 28.146 | 29.511 | 12.461 | 16.096 | 17.201 | 17.612 |
| Deflection 6 + 1.52 + 10 (mm) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 1.835 | 2.077 | 3.907 | 5.402 | 1.837 | 1.843 | 1.847 | 1.852 |
| 25 | 1.858 | 3.962 | 5.242 | 5.824 | 1.848 | 1.872 | 1.907 | 1.971 |
| 30 | 2.042 | 5.231 | 5.720 | 6.493 | 1.866 | 1.931 | 2.279 | 2.732 |
| 40 | 4.855 | 6.010 | 6.768 | 7.260 | 2.001 | 2.857 | 3.158 | 3.274 |
| Bottom panel stress 6 + 1.52 + 10 (MPa) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 12.362 | 13.416 | 19.086 | 23.483 | 12.365 | 12.403 | 12.429 | 12.459 |
| 25 | 12.506 | 19.249 | 23.014 | 24.730 | 12.439 | 12.579 | 12.761 | 13.044 |
| 30 | 13.292 | 22.984 | 24.426 | 26.707 | 12.549 | 12.873 | 14.176 | 15.649 |
| 40 | 21.833 | 25.279 | 27.528 | 29.051 | 13.166 | 16.037 | 16.947 | 17.291 |
| Deflection 6 + 0.76 + 10 (mm) | Deflection 6 + 0.89 + 10 (mm) | |||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 2.063 | 2.203 | 3.421 | 4.707 | 2.022 | 2.026 | 2.029 | 2.032 |
| 25 | 2.076 | 3.440 | 4.540 | 5.133 | 2.029 | 2.045 | 2.068 | 2.110 |
| 30 | 2.180 | 4.520 | 5.013 | 5.888 | 2.041 | 2.083 | 2.312 | 2.624 |
| 40 | 4.174 | 5.317 | 6.230 | 6.907 | 2.129 | 2.713 | 2.931 | 3.018 |
| Bottom panel stress 6 + 0.76 + 10 (MPa) | Bottom panel stress 6 + 0.89 + 10 (MPa) | |||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 13.400 | 14.093 | 17.921 | 21.643 | 13.218 | 13.247 | 13.267 | 13.290 |
| 25 | 13.498 | 17.992 | 21.160 | 22.867 | 13.273 | 13.376 | 13.509 | 13.712 |
| 30 | 14.006 | 21.102 | 22.528 | 25.042 | 13.354 | 13.589 | 14.504 | 15.552 |
| 40 | 20.108 | 23.399 | 26.025 | 27.994 | 13.797 | 15.833 | 16.507 | 16.767 |
| Deflection 8 + 2.28 + 8 (mm) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 1.614 | 1.971 | 4.223 | 6.060 | 1.815 | 1.821 | 1.825 | 1.831 |
| 25 | 1.647 | 4.621 | 5.851 | 6.597 | 1.827 | 1.852 | 1.892 | 1.963 |
| 30 | 1.918 | 6.266 | 6.460 | 7.800 | 1.846 | 1.918 | 2.308 | 2.823 |
| 40 | 5.790 | 7.224 | 7.834 | 8.691 | 1.996 | 2.966 | 3.314 | 3.450 |
| Bottom panel stress 8 + 2.28 + 8 (MPa) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 11.458 | 12.591 | 18.121 | 22.108 | 12.360 | 12.388 | 12.409 | 12.434 |
| 25 | 11.600 | 18.718 | 21.654 | 23.277 | 12.417 | 12.527 | 12.675 | 12.907 |
| 30 | 12.449 | 22.383 | 22.975 | 25.827 | 12.504 | 12.767 | 13.872 | 15.103 |
| 40 | 21.317 | 24.517 | 25.973 | 27.980 | 13.010 | 15.424 | 16.212 | 16.514 |
| Deflection 8 + 1.52 + 8 (mm) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 1.812 | 2.084 | 4.223 | 6.061 | 1.815 | 1.821 | 1.258 | 1.831 |
| 25 | 1.837 | 4.265 | 5.851 | 6.597 | 1.827 | 1.852 | 1.892 | 1.963 |
| 30 | 2.040 | 5.827 | 6.458 | 7.470 | 1.846 | 1.918 | 2.308 | 2.822 |
| 40 | 5.356 | 6.826 | 7.834 | 8.501 | 1.996 | 2.966 | 3.314 | 3.449 |
| Bottom panel stress 8 + 1.52 + 8 (MPa) | ||||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 12.361 | 13.225 | 18.121 | 22.108 | 12.360 | 12.390 | 12.409 | 12.435 |
| 25 | 12.471 | 18.217 | 21.654 | 23.227 | 12.417 | 12.528 | 12.675 | 12.907 |
| 30 | 13.111 | 21.603 | 22.975 | 25.171 | 12.504 | 12.767 | 13.872 | 15.103 |
| 40 | 20.580 | 23.776 | 25.973 | 27.534 | 13.010 | 15.424 | 16.212 | 16.514 |
| Deflection 8 + 0.76 + 8 (mm) | Deflection 8 + 0.89 + 8 (mm) | |||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 2.049 | 2.211 | 3.644 | 5.197 | 2.007 | 2.011 | 2.014 | 2.019 |
| 25 | 2.063 | 3.460 | 4.979 | 5.721 | 2.015 | 2.032 | 2.058 | 2.105 |
| 30 | 2.018 | 4.939 | 5.564 | 6.681 | 2.028 | 2.075 | 2.333 | 2.688 |
| 40 | 4.514 | 5.937 | 7.119 | 8.027 | 2.125 | 2.789 | 3.042 | 3.142 |
| ottom panel stress 8 + 0.76 + 8 (MPa) | Bottom panel stress 8 + 0.89 + 8 (MPa) | |||||||
| Temp (°C) | PVB | IONOPLAST | ||||||
| 1 min | 24 h | 1 month | 10 years | 1 min | 24 h | 1 month | 10 years | |
| 10 | 13.385 | 13.931 | 17.225 | 20.514 | 13.200 | 13.222 | 13.236 | 13.258 |
| 25 | 13.456 | 17.224 | 20.060 | 21.615 | 13.241 | 13.321 | 13.425 | 13.590 |
| 30 | 13.850 | 19.971 | 21.285 | 23.633 | 13.303 | 13.490 | 14.244 | 15.131 |
| 40 | 19.071 | 22.057 | 24.554 | 26.474 | 13.659 | 15.365 | 15.926 | 16.147 |
Appendix B
| Coefficients for Displacement Function | ||||||
|---|---|---|---|---|---|---|
| Coef. | 10 + d + 6 mm PVB | 6 + d + 10 mm PVB | 8 + d + 8 mm PVB | 10 + d + 6 mm Ionoplast | 6 + d + 10 mm Ionoplast | 8 + d + 8 mm Ionoplast |
| F1 | 2.791 × 100 | 2.871 × 100 | 2.910 × 100 | 2.263 × 100 | 1.992 × 100 | −2.809 × 10−1 |
| F2 | 3.290 × 10−2 | 1.531 × 10−2 | 1.750 × 10−1 | −5.358 × 10−2 | 6.979 × 10−2 | 6.894 × 10−1 |
| F3 | 8.810 × 10−3 | 2.529 × 10−3 | 1.416 × 10−2 | −1.987 × 10−2 | 2.648 × 10−2 | 1.708 × 10−1 |
| F4 | −2.880 × 10−3 | −2.442 × 10−3 | −1.257 × 10−2 | 4.104 × 10−3 | −2.826 × 10−3 | −2.654 × 10−2 |
| F5 | −2.783 × 10−2 | −2.660 × 10−2 | −4.955 × 10−2 | 5.285 × 10−3 | −8.877 × 10−3 | −4.392 × 10−2 |
| F6 | −1.292 × 10−3 | −1.168 × 10−3 | −1.699 × 10−3 | 2.399 × 10−4 | −6.416 × 10−4 | −2.636 × 10−3 |
| F7 | 1.836 × 10−3 | 1.787 × 10−3 | 3.079 × 10−3 | −4.501 × 10−4 | 3.457 × 10−4 | 1.675 × 10−3 |
| F8 | 1.030 × 10−3 | 1.007 × 10−3 | 1.553 × 10−3 | −5.441 × 10−5 | 2.151 × 10−4 | 6.741 × 10−4 |
| F9 | −5.742 × 10−5 | −5.634 × 10−5 | −8.522 × 10−5 | 7.458 × 10−6 | −7.622 × 10−6 | −2.530 × 10−5 |
| F10 | 5.708 × 100 | 5.599 × 100 | 7.015 × 100 | −1.170 × 100 | −5.342 × 10−1 | 3.249 × 100 |
| F11 | −1.458 × 100 | −1.436 × 100 | −1.959 × 100 | 3.391 × 10−1 | 9.983 × 10−2 | −9.423 × 10−1 |
| F12 | −7.799 × 10−1 | −7.695 × 10−1 | −9.473 × 10−1 | 1.290 × 10−1 | 3.911 × 10−2 | −1.964 × 10−1 |
| F13 | 7.167 × 10−2 | 7.108 × 10−2 | 1.003 × 10−1 | −1.755 × 10−2 | −4.043 × 10−3 | 3.575 × 10−2 |
| F14 | 1.785 × 10−1 | 1.767 × 10−1 | 2.359 × 10−1 | −4.042 × 10−2 | −1.288 × 10−2 | 4.278 × 10−2 |
| F15 | 1.828 × 10−2 | 1.805 × 10−2 | 2.206 × 10−2 | −2.657 × 10−3 | −9.483 × 10−4 | 2.119 × 10−3 |
| F16 | −3.968 × 10−3 | −3.928 × 10−3 | −5.153 × 10−3 | 8.385 × 10−4 | 3.154 × 10−4 | −3.478 × 10−4 |
| F17 | −8.110 × 10−3 | −8.036 × 10−3 | −1.095 × 10−2 | 2.063 × 10−3 | 5.083 × 10−4 | −1.595 × 10−3 |
| F18 | 1.814 × 10−4 | 1.796 × 10−4 | 2.395 × 10−4 | −4.088 × 10−5 | −1.143 × 10−5 | 1.449 × 10−5 |
| F19 | −1.353 × 100 | −1.321 × 100 | −1.760 × 100 | 3.169 × 10−1 | 1.684 × 10−2 | −1.157 × 100 |
| F20 | 3.504 × 10−1 | 3.443 × 10−1 | 5.087 × 10−1 | −1.093 × 10−1 | −5.482 × 10−3 | 3.262 × 10−1 |
| F21 | 1.748 × 10−1 | 1.714 × 10−1 | 2.248 × 10−1 | −4.197 × 10−2 | −2.992 × 10−3 | 7.059 × 10−2 |
| F22 | −1.779 × 10−2 | −1.763 × 10−2 | −2.709 × 10−2 | 6.027 × 10−3 | 1.461 × 10−4 | −1.245 × 10−2 |
| F23 | −4.278 × 10−2 | −4.220 × 10−2 | −6.037 × 10−2 | 1.270 × 10−2 | 7.323 × 10−4 | −1.615 × 10−2 |
| F24 | −4.177 × 10−3 | −4.100 × 10−3 | −5.248 × 10−3 | 8.110 × 10−4 | 7.145 × 10−5 | −8.424 × 10−4 |
| F25 | 9.932 × 10−4 | 9.803 × 10−4 | 1.350 × 10−3 | −2.447 × 10−4 | −1.785 × 10−5 | 1.653 × 10−4 |
| F26 | 2.078 × 10−3 | 2.055 × 10−3 | 2.963 × 10−3 | −6.981 × 10−4 | −2.049 × 10−5 | 6.098 × 10−4 |
| F27 | −4.897 × 10−5 | −4.843 × 10−5 | −6.678 × 10−5 | 1.328 × 10−5 | 4.615 × 10−7 | −6.677 × 10−6 |
| Coefficients for Stress Function | ||||||
|---|---|---|---|---|---|---|
| Coef. | 10 + d + 6 mm PVB | 6 + d + 10 mm PVB | 8 + d + 8 mm PVB | 10 + d + 6 mm Ionoplast | 6 + d + 10 mm Ionoplast | 8 + d + 8 mm Ionoplast |
| F1 | 2.791 × 100 | 2.871 × 100 | 2.910 × 100 | 2.263 × 100 | 1.992 × 100 | −2.809 × 10−1 |
| F2 | 3.290 × 10−2 | 1.531 × 10−2 | 1.750 × 10−1 | −5.358 × 10−2 | 6.979 × 10−2 | 6.894 × 10−1 |
| F3 | 8.810 × 10−3 | 2.529 × 10−3 | 1.416 × 10−2 | −1.987 × 10−2 | 2.648 × 10−2 | 1.708 × 10−1 |
| F4 | −2.880 × 10−3 | −2.442 × 10−3 | −1.257 × 10−2 | 4.104 × 10−3 | −2.826 × 10−3 | −2.654 × 10−2 |
| F5 | −2.783 × 10−2 | −2.660 × 10−2 | −4.955 × 10−2 | 5.285 × 10−3 | −8.877 × 10−3 | −4.392 × 10−2 |
| F6 | −1.292 × 10−3 | −1.168 × 10−3 | −1.699 × 10−3 | 2.399 × 10−4 | −6.416 × 10−4 | −2.636 × 10−3 |
| F7 | 1.836 × 10−3 | 1.787 × 10−3 | 3.079 × 10−3 | −4.501 × 10−4 | 3.457 × 10−4 | 1.675 × 10−3 |
| F8 | 1.030 × 10−3 | 1.007 × 10−3 | 1.553 × 10−3 | −5.441 × 10−5 | 2.151 × 10−4 | 6.741 × 10−4 |
| F9 | −5.742 × 10−5 | −5.634 × 10−5 | −8.522 × 10−5 | 7.458 × 10−6 | −7.622 × 10−6 | −2.530 × 10−5 |
| F10 | 5.708 × 100 | 5.599 × 100 | 7.015 × 100 | −1.170 × 100 | −5.342 × 10−1 | 3.249 × 100 |
| F11 | −1.458 × 100 | −1.436 × 100 | −1.959 × 100 | 3.391 × 10−1 | 9.983 × 10−2 | −9.423 × 10−1 |
| F12 | −7.799 × 10−1 | −7.695 × 10−1 | −9.473 × 10−1 | 1.290 × 10−1 | 3.911 × 10−2 | −1.964 × 10−1 |
| F13 | 7.167 × 10−2 | 7.108 × 10−2 | 1.003 × 10−1 | −1.755 × 10−2 | −4.043 × 10−3 | 3.575 × 10−2 |
| F14 | 1.785 × 10−1 | 1.767 × 10−1 | 2.359 × 10−1 | −4.042 × 10−2 | −1.288 × 10−2 | 4.278 × 10−2 |
| F15 | 1.828 × 10−2 | 1.805 × 10−2 | 2.206 × 10−2 | −2.657 × 10−3 | −9.483 × 10−4 | 2.119 × 10−3 |
| F16 | −3.968 × 10−3 | −3.928 × 10−3 | −5.153 × 10−3 | 8.385 × 10−4 | 3.154 × 10−4 | −3.478 × 10−4 |
| F17 | −8.110 × 10−3 | −8.036 × 10−3 | −1.095 × 10−2 | 2.063 × 10−3 | 5.083 × 10−4 | −1.595 × 10−3 |
| F18 | 1.814 × 10−4 | 1.796 × 10−4 | 2.395 × 10−4 | −4.088 × 10−5 | −1.143 × 10−5 | 1.449 × 10−5 |
| F19 | −1.353 × 100 | −1.321 × 100 | −1.760 × 100 | 3.169 × 10−1 | 1.684 × 10−2 | −1.157 × 100 |
| F20 | 3.504 × 10−1 | 3.443 × 10−1 | 5.087 × 10−1 | −1.093 × 10−1 | −5.482 × 10−3 | 3.262 × 10−1 |
| F21 | 1.748 × 10−1 | 1.714 × 10−1 | 2.248 × 10−1 | −4.197 × 10−2 | −2.992 × 10−3 | 7.059 × 10−2 |
| F22 | −1.779 × 10−2 | −1.763 × 10−2 | −2.709 × 10−2 | 6.027 × 10−3 | 1.461 × 10−4 | −1.245 × 10−2 |
| F23 | −4.278 × 10−2 | −4.220 × 10−2 | −6.037 × 10−2 | 1.270 × 10−2 | 7.323 × 10−4 | −1.615 × 10−2 |
| F24 | −4.177 × 10−3 | −4.100 × 10−3 | −5.248 × 10−3 | 8.110 × 10−4 | 7.145 × 10−4 | −8.424 × 10−4 |
| F25 | 9.932 × 10−4 | 9.803 × 10−4 | 1.350 × 10−3 | −2.447 × 10−4 | −1.785 × 10−5 | 1.653 × 10−4 |
| F26 | 2.078 × 10−3 | 2.055 × 10−3 | 2.963 × 10−3 | −6.981 × 10−4 | −2.049 × 10−5 | 6.098 × 10−4 |
| F27 | −4.897 × 10−5 | −4.843 × 10−5 | −6.678 × 10−5 | 1.328 × 10−5 | 4.615 × 10−7 | −6.677 × 10−6 |
References
- FprEN 16612; Glass in Building—Determination of the Lateral Load Resistance of Glass Panes by Calculation. EUROPEAN STANDARDS s.r.o.: Pilsen, Czech Republic, 2019.
- Grozdanić, G.; Galić, M.; Marović, P. Some Aspects of the Analyses of Glass Structures Exposed to Impact Load. Couple. Syst. Mech. 2021, 10, 475–490. [Google Scholar] [CrossRef]
- Schmidt, J.; Zemanová, A.; Zeman, J.; Šejnoha, M. Phase-Field Fracture Modelling of Thin Monolithic and Laminated Glass Plates under Quasi-Static Bending. Materials 2020, 13, 5153. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, H.; Maharaj, C.; Zheng, M.; Mohagheghian, I.; Zhang, G.; Yan, Y.; Dear, J.P. Impact Response of Laminated Glass with Varying Interlayer Materials. Int. J. Impact Eng. 2020, 139, 103505:1–103505:15. [Google Scholar] [CrossRef]
- Timmel, M.; Kolling, S.; Osterrieder, P.; Du Bois, P.A. A Finite Element Model for Impact Simulation with Laminated Glass. Int. J. Impact Eng. 2007, 34, 1465–1478. [Google Scholar] [CrossRef]
- Serafinavicius, T.; Kvedaras, A.K.; Sauciuvenas, G. Bending Behavior of Structural Glass Laminated with Different Interlayers. Mech. Compos. Mater. 2013, 49, 437–446. [Google Scholar] [CrossRef]
- Pelfrene, J.; Kuntsche, J.; Van Dam, S.; Van Paepegem, W.; Schneider, J. Critical Assessment of the Post-breakage Performance of Blast Loaded Laminated Glazing: Experiments and Simulations. Int. J. Impact Eng. 2016, 88, 61–71. [Google Scholar] [CrossRef]
- Pankhardt, K.; Balázs, G.L. Temperature Dependent Load Bearing Capacity of Laminated Glass Panes. Period. Polytech. Civil Engng. 2010, 54, 11–22. [Google Scholar] [CrossRef][Green Version]
- Galuppi, L.; Royer-Carfagni, G.F. Effective Thickness of Laminated Glass Beams: New Expression via a Variational Approach. Eng. Struct. 2012, 38, 53–67. [Google Scholar] [CrossRef]
- Van Duser, A.; Jagota, A.; Bennison, S.J. Analysis of Glass/Polyvinyl Butyral Laminates Subjected to Uniform Pressure. ASCE J. Eng. Mech. 1999, 125, 435–442. [Google Scholar] [CrossRef]
- Louter, C.; Belis, J.; Veer, F.; Lebet, J.-P. Durability of SG-Laminated Reinforced Glass Beams: Effects of Temperature, Thermal Cycling, Humidity and Load-duration. Constr. Build. Mater. 2012, 27, 280–292. [Google Scholar] [CrossRef]
- Schwarzl, F.R. Polymer-Mechanik: Struktur und Mechanisches Verhalten von Polymeren; Springer: Berlin/Heidelberg, Germany, 1990; ISBN 978-3-642-64858-8. [Google Scholar]
- Brinson, H.F.; Brinson, L.C. Polymer Engineering Science and Viscoelasticity: An Introduction, 2nd ed.; Springer: New York, NY, USA, 2015; ISBN 978-1-4899-7484-6. [Google Scholar]
- Hána, T.; Janda, T.; Schmidt, J.; Zemanova, A.; Šejnoha, M.; Eliášová, M.; Vokáč, M. Experimental and Numerical Study of Viscoelastic Properties of Polymeric Interlayers Used for Laminated Glass: Determination of Material Parameters. Materials 2019, 12, 2241. [Google Scholar] [CrossRef]
- Hána, T.; Eliášová, M.; Machalická, K.; Vokáč, M. Determination of PVB Interlayer’s Shear Modulus and its Effect on Normal Stress Distribution in Laminated Glass Panels. Mater. Sci. Engng. 2017, 251, 012076:1–012076:8. [Google Scholar] [CrossRef]
- Biolzi, L.; Cattaneo, S.; Orlando, M.; Piscitelli, L.R.; Spinelli, P. Constitutive Relationships of Different Interlayer Materials for Laminated Glass. Compos. Struct. 2020, 244, 112221:1–112221:16. [Google Scholar] [CrossRef]
- Iwasaki, R.; Sato, C.; Lataillade, J.L.; Viot, P. Experimental Study on the Interface Fracture Toughness of PVB (Polyvinyl Butyral)/Glass at High Strain Rates. Int. J. Crashworthiness 2007, 12, 293–298. [Google Scholar] [CrossRef]
- Molnár, G.; Vigh, L.G.; Stocker, G.; Dunai, L. Finite Element Analysis of Laminated Structural Glass Plates with Polyvinyl Butyral ( PVB ) Interlayer. Period. Polytech. Civil Engng. 2012, 56, 35–42. [Google Scholar] [CrossRef]
- EASTMAN-Material Properties of PVB Interlayers Used in Saflex DG41, Product Sheets; Saflex DG: Springfield, MA, USA, 2015.
- SentryGlas® Ionoplast Interlayer-Elastic Properties (SG5000). Available online: https://www.trosifol.com/fileadmin/user_upload/technical_information/downloads/sentryglas/150129_Kuraray_TM_Datenblatt_SG.pdf (accessed on 29 January 2022).
- Hooper, P.A.; Blackman, B.R.K.; Dear, J.P. The Mechanical Behaviour of Poly (Vinyl Bbutyral ) at Different Strain Magnitudes and Strain Rates. J. Mater. Sci. 2012, 47, 3564–3576. [Google Scholar] [CrossRef]
- Zhang, H.; Hao, H.; Shi, Y.; Cui, J. The Mechanical Properties of Polyvinyl Butyral (PVB ) at High Strain Rates. Constr. Build. Mater. 2015, 93, 404–415. [Google Scholar] [CrossRef]
- Centelles, X.; Martin, M.; Solé, A.; Castro, J.R.; Cabeza, L.F. Tensile Test on Interlayer Materials for Laminated Glass under Diverse Ageing Conditions and Strain Rates. Constr. Build. Mater. 2020, 243, 118230. [Google Scholar] [CrossRef]
- Novotný, M.; Poot, B. Influence of temperature on laminated glass performances assembled with various interlayers. In Proceedings of the Conference on Architectural and Structural Applications of Glass (Challeging Glass 5), Ghent, Belgium, 16–17 June 2016. [Google Scholar]
- Aenlle-Lopez, M.; Noriega, A.; Pelayo, F. Mechanical Characterization of Polyvinil Butyral from Static and Modal Tests on Laminated Glass Beams. Compos. Part B Eng. 2019, 169, 9–18. [Google Scholar] [CrossRef]
- Liene, S.; Kinsella, D.; Kozłowski, M. Influence of EVA, PVB and Ionoplast Interlayers on the Structural Behaviour and Fracture Pattern of Laminated Glass. Int. J. Struct. Glas. Adv. Mater. Res. 2019, 3, 62–78. [Google Scholar] [CrossRef]
- Schuster, M.; Kraus, M.; Schneider, J.; Siebert, G. Investigations on the Thermorheologically Complex Material Behaviour of the laminated Safety Glass Interlayer Ethylene-Vinyl-Acetate. Glass Struct. Eng. 2018, 3, 373–388. [Google Scholar] [CrossRef]
- Kraus, M.A.; Schuster, M.; Kuntsche, J.; Siebert, G.; Schneider, J. Parameter Identification Methods for Visco- and Hyperelastic Material Models. Glass Struct. Eng. 2017, 2, 147–167. [Google Scholar] [CrossRef]
- Hána, T.; Vokáč, M.; Eliášová, M.; Machalická, K.V. Experimental Investigation of Temperature and Loading Rate Effects on the Initial Shear Stiffness of Polymeric Interlayers. Eng. Struct. 2020, 223, 110728:1–110728:16. [Google Scholar] [CrossRef]
- Biolzi, L.; Cagnacci, E.; Orlando, M.; Piscitelli, L.; Rosati, G. Long Term Response of Glass-PVB double-lap joints. Compos. Part B Eng. 2014, 41–49. [Google Scholar] [CrossRef]
- Andreozzi, L.; Briccoli Bati, S.; Fagone, M.; Ranocchiai, G.; Zulli, F. Dynamic Torsion Tests to Characterize the Termo-viscoelastic Properties of Polymeric Interlayers for Laminated Glass. Constr. Build. Mater. 2014, 65, 1–13. [Google Scholar] [CrossRef]
- Botz, M.; Kraus, M.; Siebert, G. Experimental determination of the shear modulus of polymeric interlayers used in laminated glass. In Proceedings of the Glass Con. Global, Chicago, IL, USA, 5–7 September 2018. [Google Scholar]
- Chen, S.; Lu, Y.; Zhang, Y.; Shao, X. Experimental and Analytical Study on Uniaxial Tensile Property of Ionomer Interlayer at Different Temperatures and Strain Rates. Constr. Build. Mater. 2020, 262, 120058:1–120058:18. [Google Scholar] [CrossRef]
- Castori, G.; Speranzini, E. Structural Analysis of Failure Behavior of Laminated Glass. Compos. Part B Eng. 2017, 125, 89–99. [Google Scholar] [CrossRef]
- EN 1288-3:2002; Glass in Building-Determination of the Bending Strength of Glass-Part 3: Test with Specimen Supported at Two Points (Four-Point Bending). European Committee for Standardization, CEN: Brussels, Belgium, 2000.
- Asik, M.Z. Laminated Glass Plates: Revealing of Nonlinear Behavior. Comput. Struct. 2003, 81, 2659–2671. [Google Scholar] [CrossRef]
- Gao, W.; Zang, M. The Simulation of Laminated Glass Beam Impact Problem by Developing Fracture Model of Spherical DEM. Eng. Anal. Bound. Elem. 2014, 42, 2–7. [Google Scholar] [CrossRef]
- Engineering Simulation Software ANSYS, Release 16.2; Ansys Inc.: Canonsburg, DC, USA, 2015.
- Mathematical Computing Software MATLAB, version 2021a; The MathWorks Inc.: Natick, MA, USA, 2021.
- Seber, G.A.F.; Wild, C.J. Nonlinear Regression; John Wiley & Sons: Hoboken, NJ, USA, 2003; ISBN 0-471-47135-6. [Google Scholar]
- Serafinavičius, T.; Lebet, J.-P.; Louter, C.; Lenkimas, T.; Kuranovas, A. Long-term Laminated Glass Four Point Bending Test with PVB, EVA and SG Interlayers at Different Temperatures. Procedia Eng. 2013, 57, 996–1004. [Google Scholar] [CrossRef]

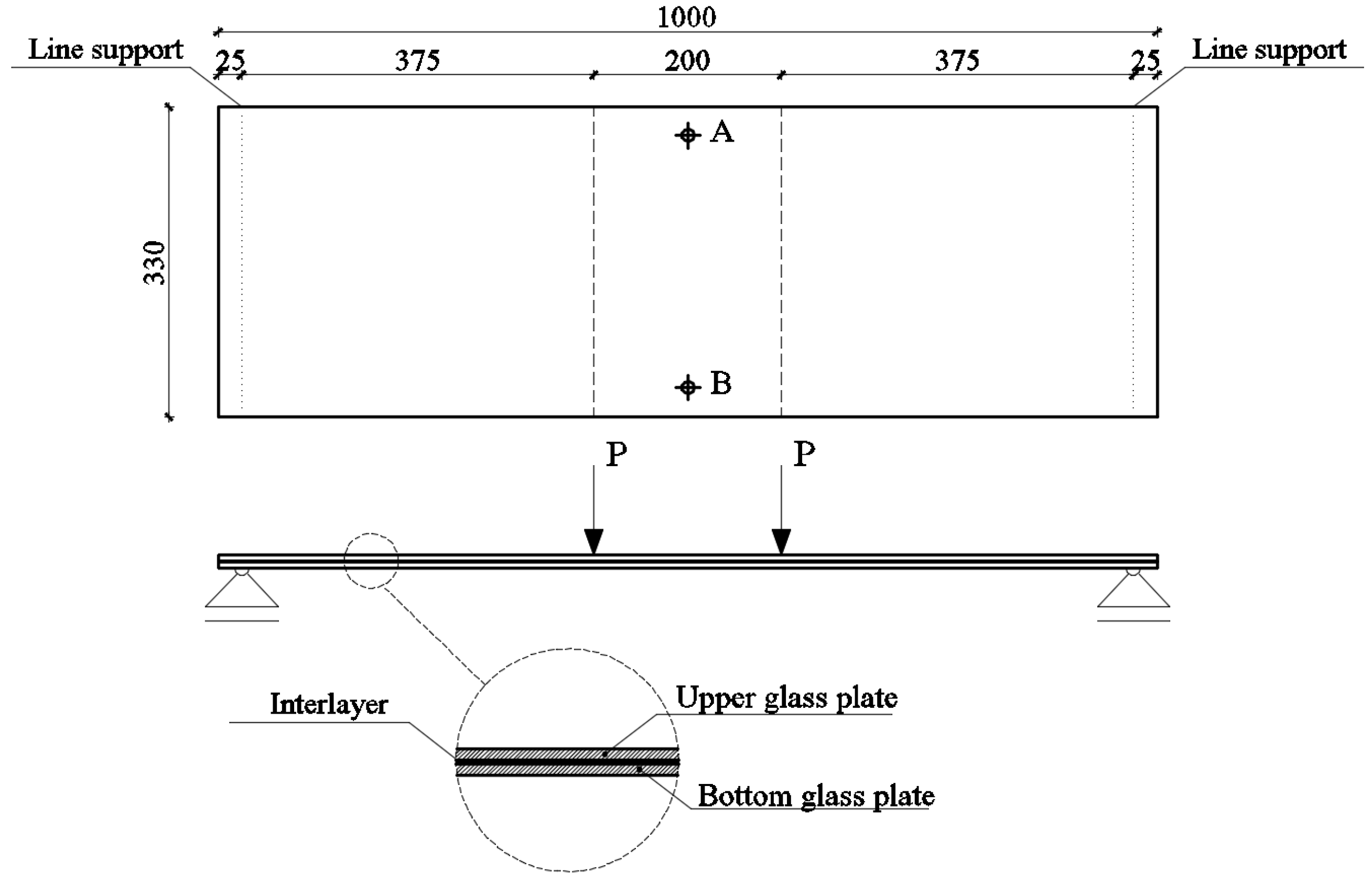

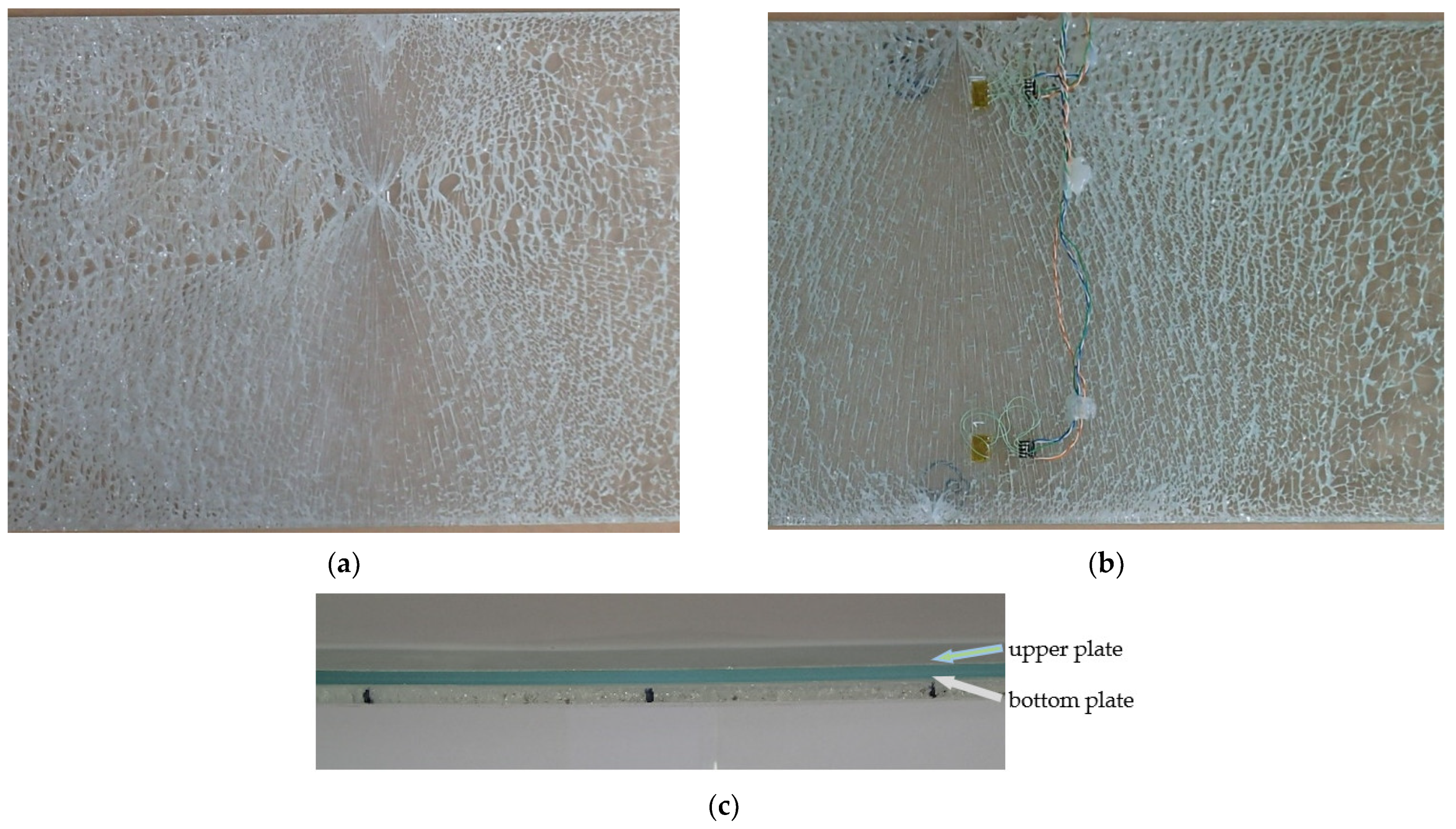
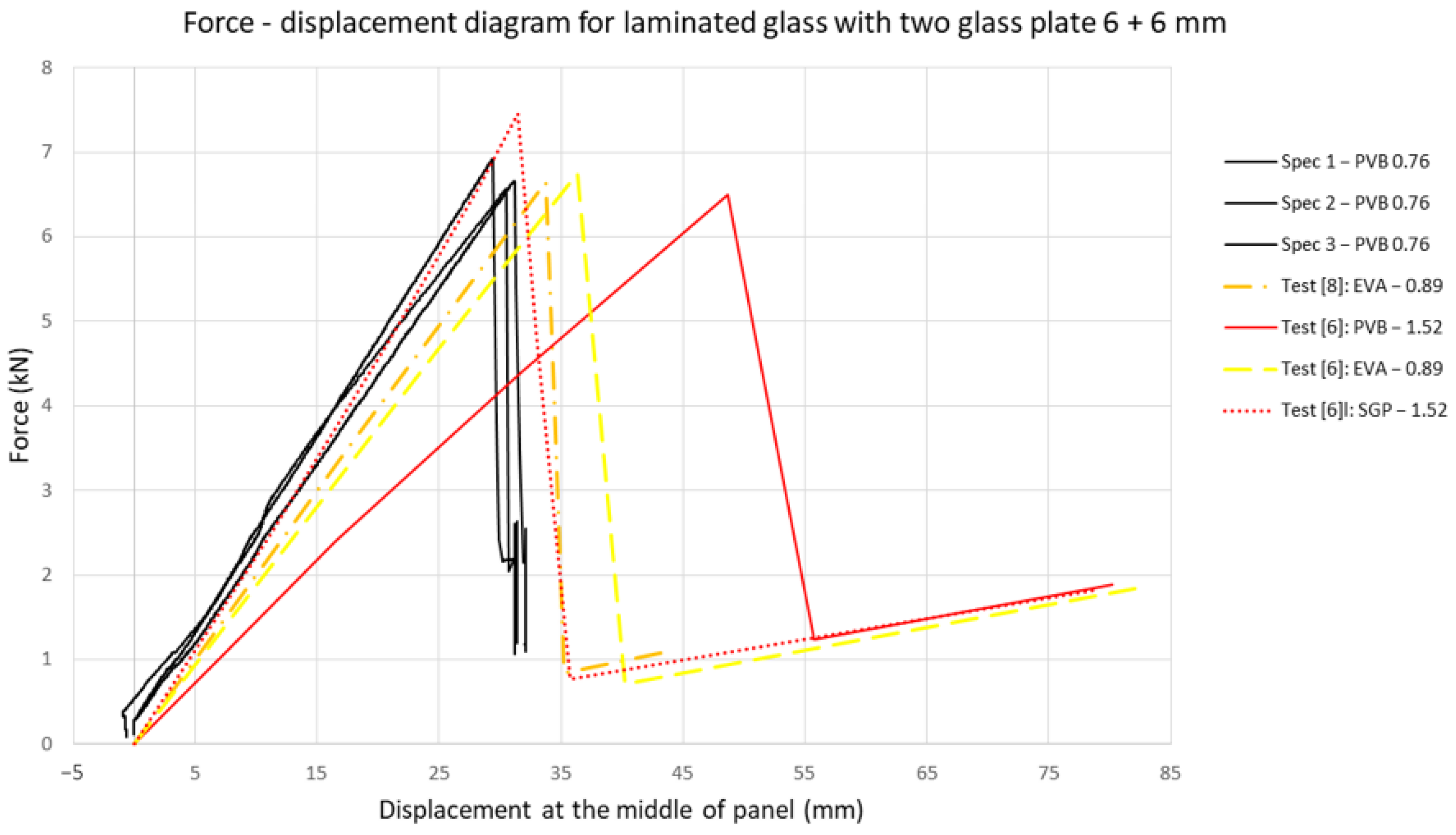


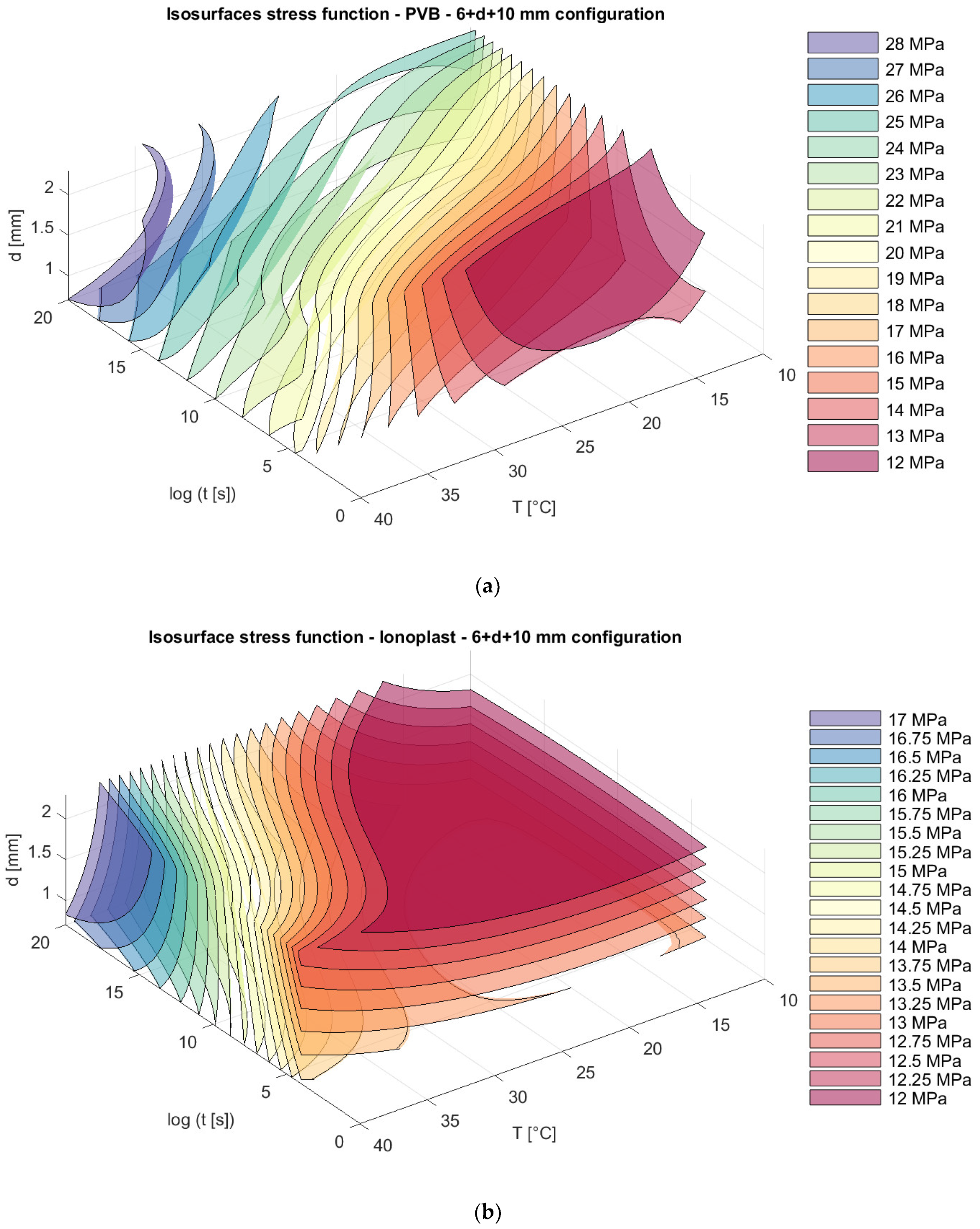
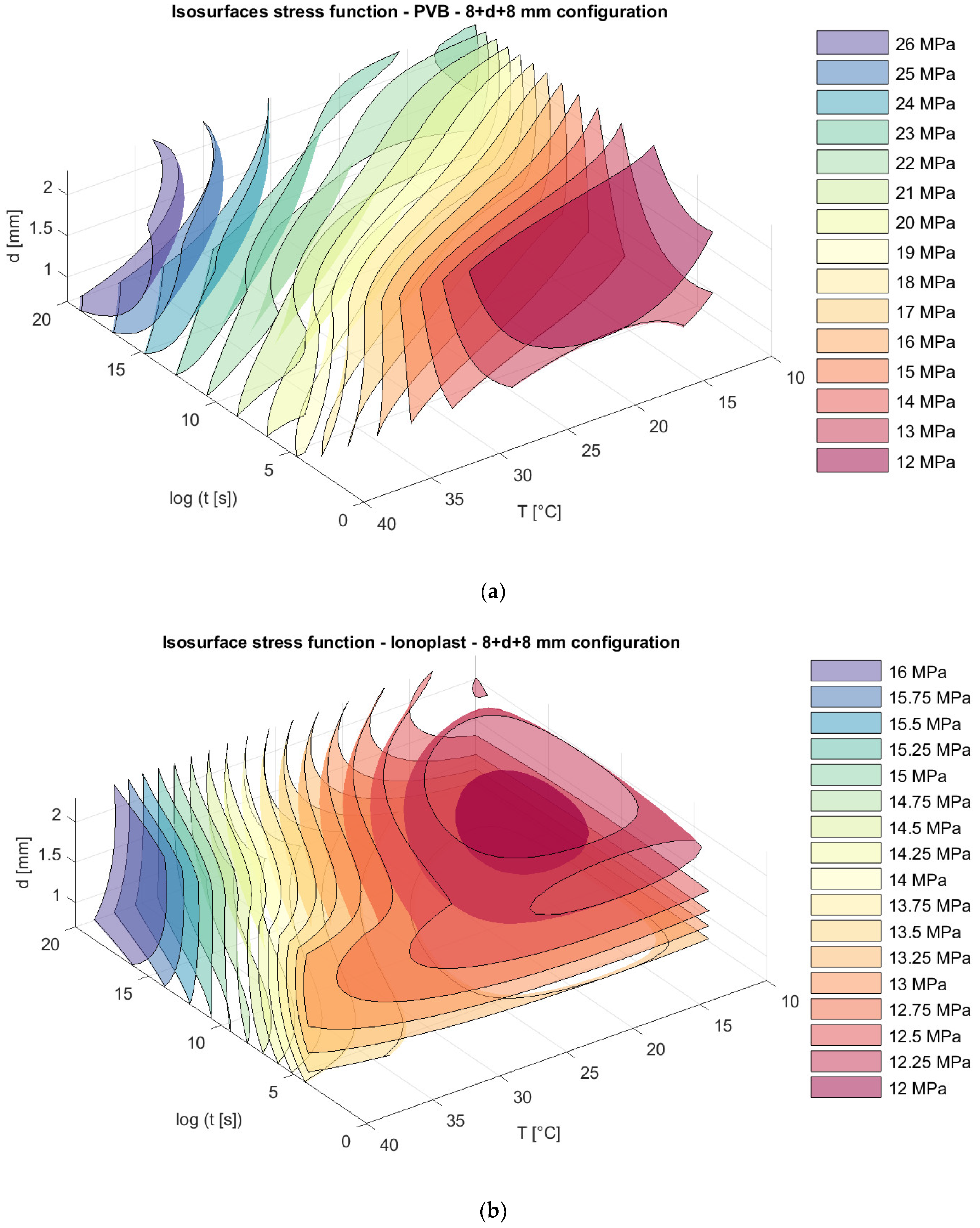
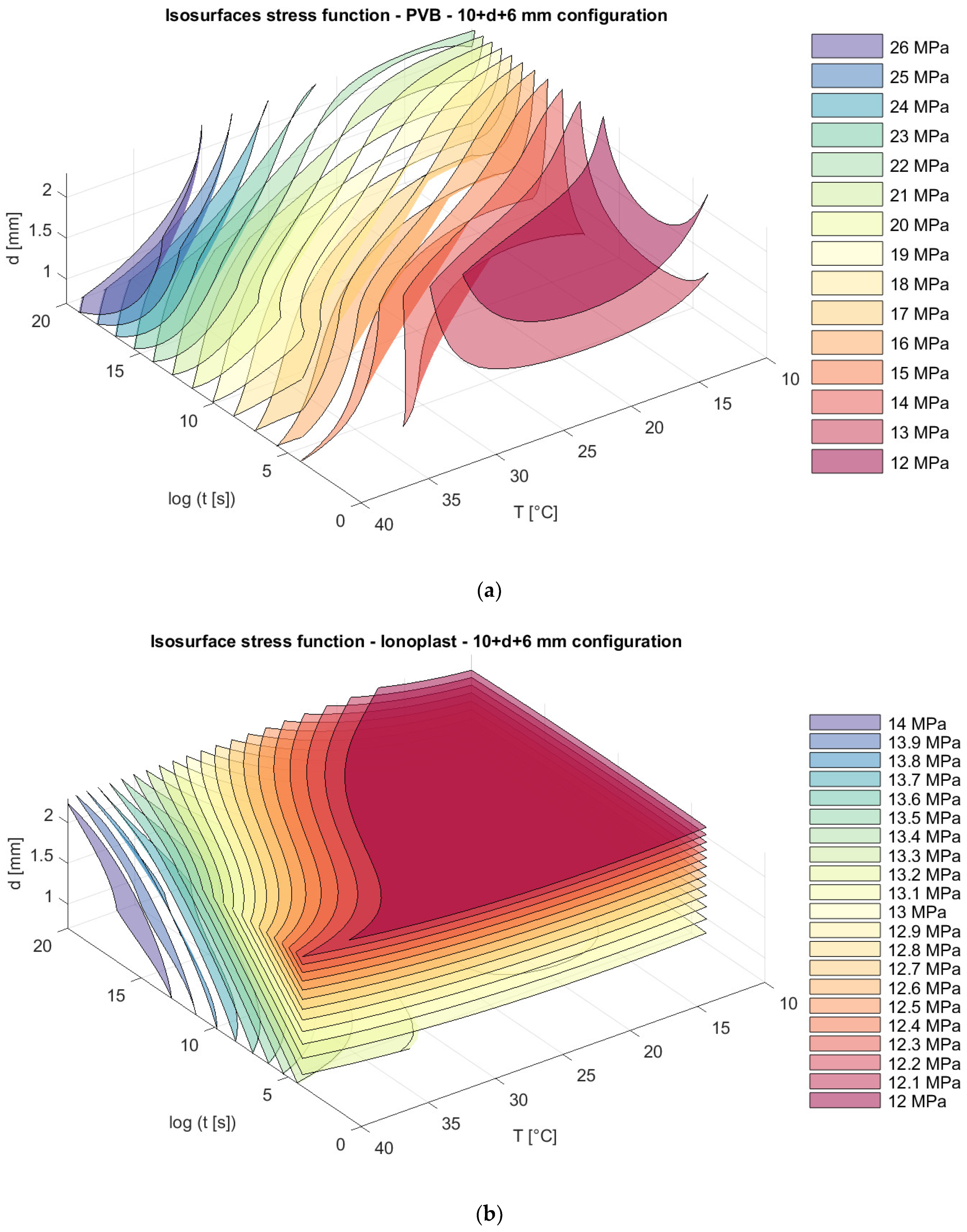
| Properties | Middle Value | Interval |
|---|---|---|
| Glass density | ρ = 2500 kg/m3 | 2250–2750 kg/m3 |
| Young’s modulus | E = 70,000 MPa | 63,000–77,000 MPa |
| Poisson number | µ = 0.23 | 0.20–0.25 |
| Annealed Glass/Float Glass | Heat-Strengthened Glass (HSG) | Thermally Toughened Glass (TTG) |
|---|---|---|
| 45 N/mm2 | 70 N/mm2 | 120 N/mm2 |
| Material | Element Type | Material Model | Contact |
|---|---|---|---|
| Glass | Solid, full integration | Linear elastic (E = 70 GPa; ν = 0.23) | Bonded, no separation |
| Interlayer | Solid, full integration | Load duration and temperature dependent |
| Interlayer Thickness | Glass Thickness (Upper + Bottom Plates) | Interlayer |
|---|---|---|
| 0.76 mm | 8 mm + 8 mm | PVB |
| 0.76 mm | 10 mm + 6 mm | PVB |
| 0.76 mm | 6 mm + 10 mm | PVB |
| 0.89 mm | 8 mm + 8 mm | Ionoplast |
| 0.89 mm | 10 mm + 6 mm | Ionoplast |
| 0.89 mm | 6 mm + 10 mm | Ionoplast |
| 1.52 mm | 8 mm + 8 mm | Ionoplast, PVB |
| 1.52 mm | 10 mm + 6 mm | Ionoplast, PVB |
| 1.52 mm | 6 mm + 10 mm | Ionoplast, PVB |
| 2.28 mm | 8 mm + 8 mm | Ionoplast, PVB |
| 2.28 mm | 10 mm + 6 mm | Ionoplast, PVB |
| 2.28 mm | 6 mm + 10 mm | Ionoplast, PVB |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Galić, M.; Grozdanić, G.; Divić, V.; Marović, P. Parametric Analyses of the Influence of Temperature, Load Duration, and Interlayer Thickness on a Laminated Glass Structure Exposed to Out-of-Plane Loading. Crystals 2022, 12, 838. https://doi.org/10.3390/cryst12060838
Galić M, Grozdanić G, Divić V, Marović P. Parametric Analyses of the Influence of Temperature, Load Duration, and Interlayer Thickness on a Laminated Glass Structure Exposed to Out-of-Plane Loading. Crystals. 2022; 12(6):838. https://doi.org/10.3390/cryst12060838
Chicago/Turabian StyleGalić, Mirela, Gabrijela Grozdanić, Vladimir Divić, and Pavao Marović. 2022. "Parametric Analyses of the Influence of Temperature, Load Duration, and Interlayer Thickness on a Laminated Glass Structure Exposed to Out-of-Plane Loading" Crystals 12, no. 6: 838. https://doi.org/10.3390/cryst12060838
APA StyleGalić, M., Grozdanić, G., Divić, V., & Marović, P. (2022). Parametric Analyses of the Influence of Temperature, Load Duration, and Interlayer Thickness on a Laminated Glass Structure Exposed to Out-of-Plane Loading. Crystals, 12(6), 838. https://doi.org/10.3390/cryst12060838








