Determination of Cooling Rate and Temperature Gradient during Formation of Cathode Spot Craters in a Vacuum Arc
Abstract
1. Introduction
2. Modelling
2.1. Estimation of the Process Parameters
- (i)
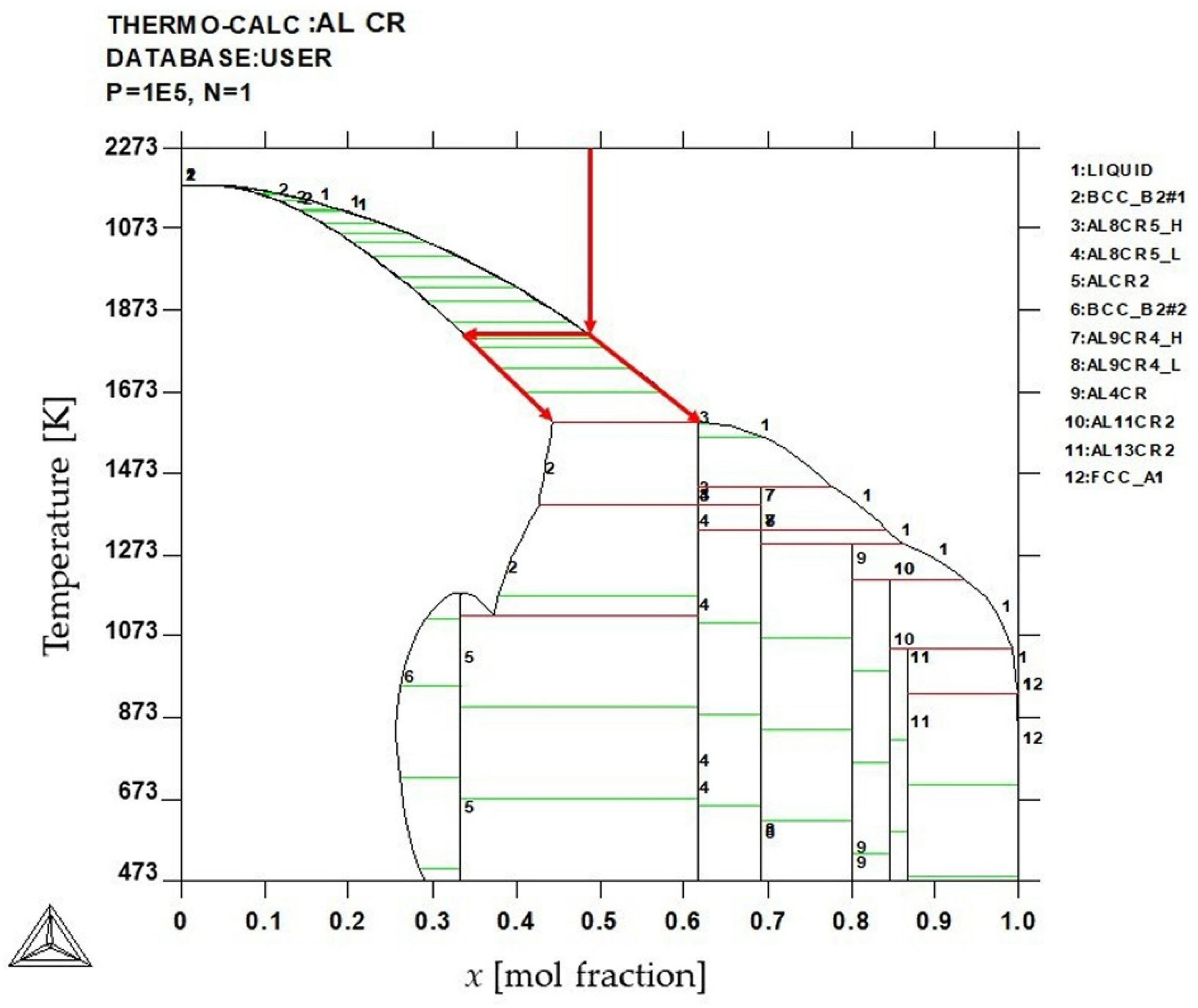
- The Cr-Al phase diagram provides the thermodynamical information relating phases, concentrations, and phase transition temperatures [8];
- (ii)
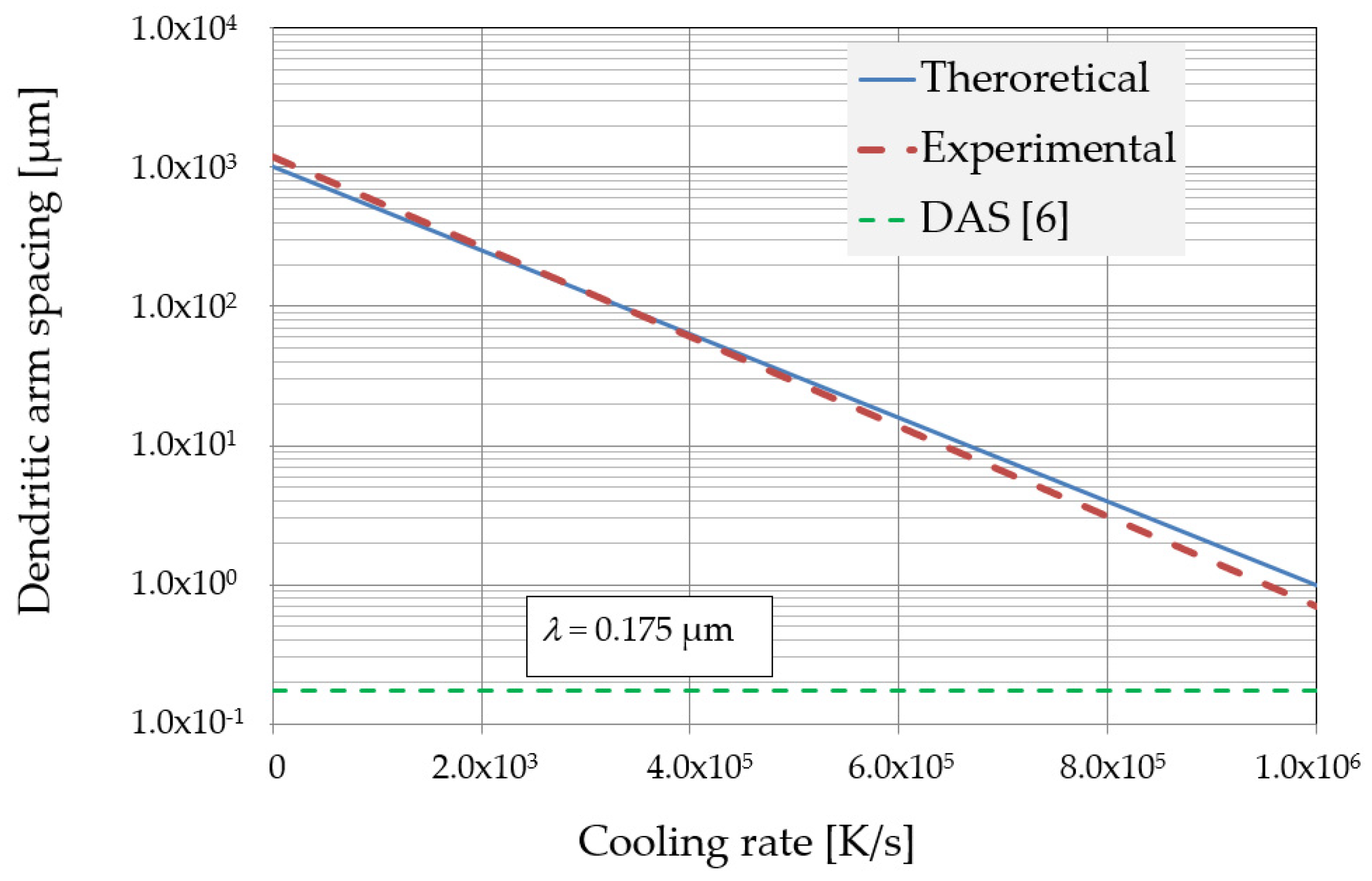
- The expected relationship between the cooling rate and the secondary dendrite arm spacing λ2 was determined based on equations published in [9];
- (iii)
- The criteria for the stability of the solid/liquid (s/l) interface defined whether the diffusion length was smaller than the size of the microstructures and whether a rapid solidification process was present, and the magnitude of the temperature gradient was estimated based on the corresponding equilibrium phase diagram for a Cr-Al cathode shown in Figure 3.
- (i)
- Based on the data published in [12], the expected cooling rate is in the range of > 1 × 106 K/s;
- (ii)
- The Feurer and Wunderlin [9] equations suggest a cooling rate in the range of 1.5 × 107 ≤ ≤ 2.7 × 107 K/s and the expected solidification time is in the order of 6.6 × 10−6 ≤ tf ≤ 1.6 × 10−5 s;
- (iii)
- The solidification velocity V did not reach the absolute velocity where the distribution coefficient k becomes 1;
- (iv)
- The temperature gradient is in the magnitude of GT = 2 × 108 ± 1.5 × 108 K/m or higher.
2.2. Phase Field Simulations
3. Results
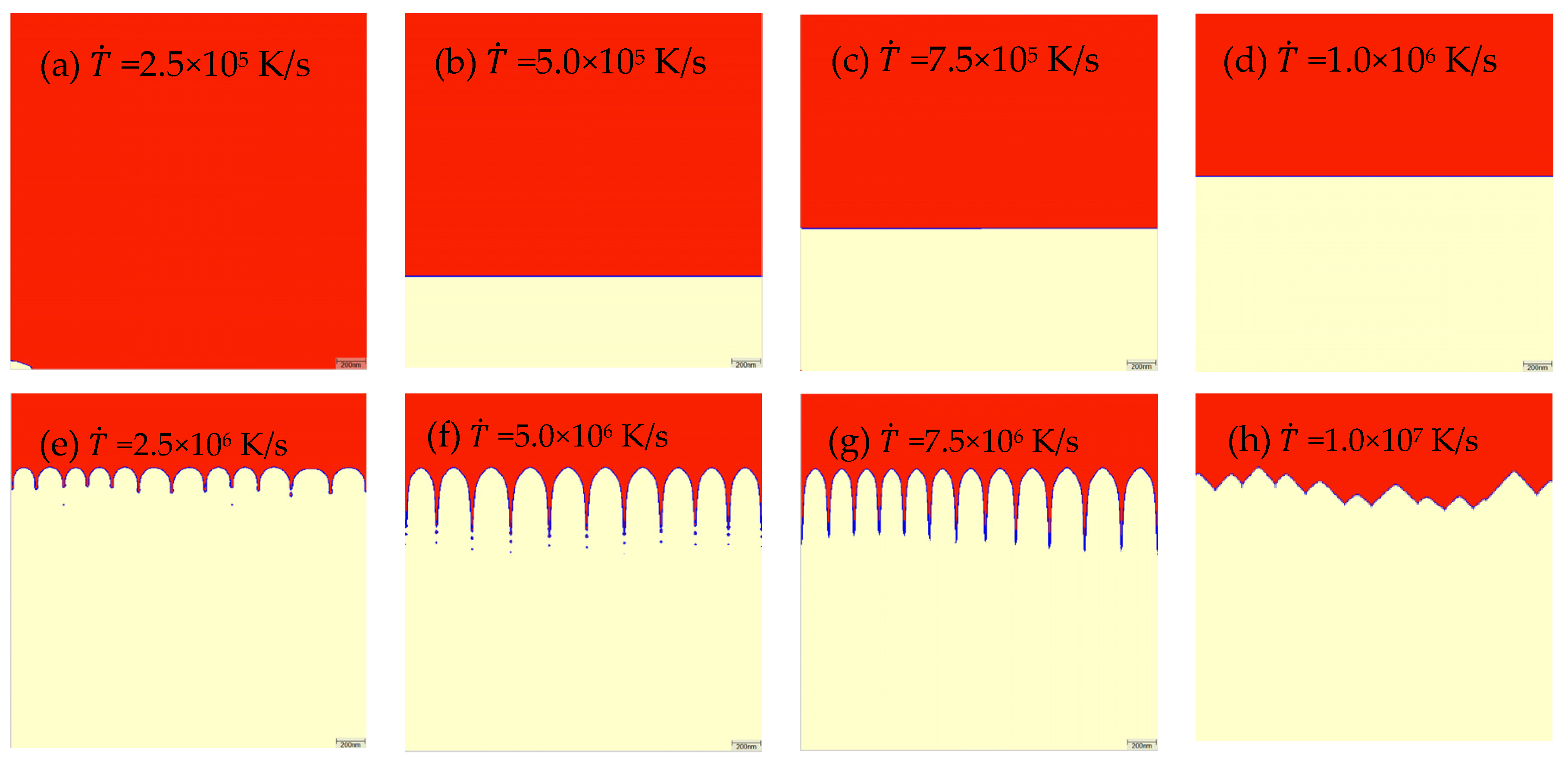
3.1. Predicted Microstructures for GT = 6 × 108 K/m
3.2. Predicted Microstructures for GT = 6 × 107 K/m
3.3. Predicted Microstructures for GT = 6 × 106 K/m
4. Discussion
- (i)
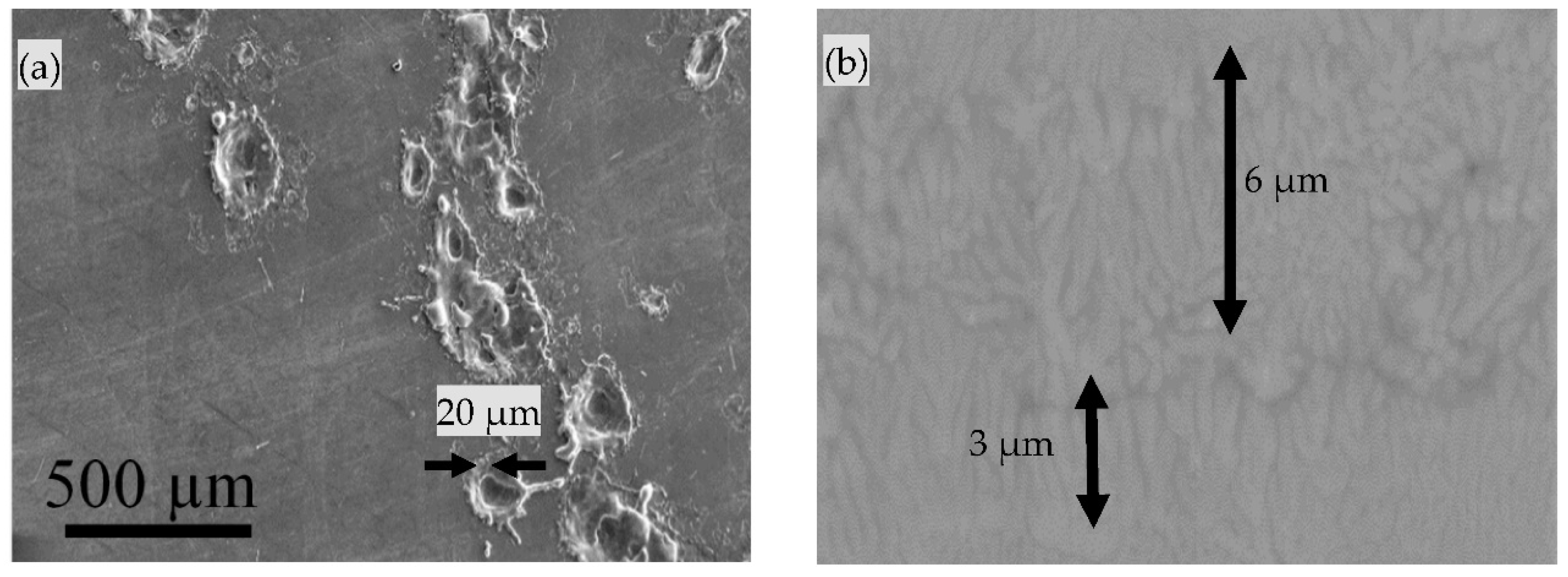
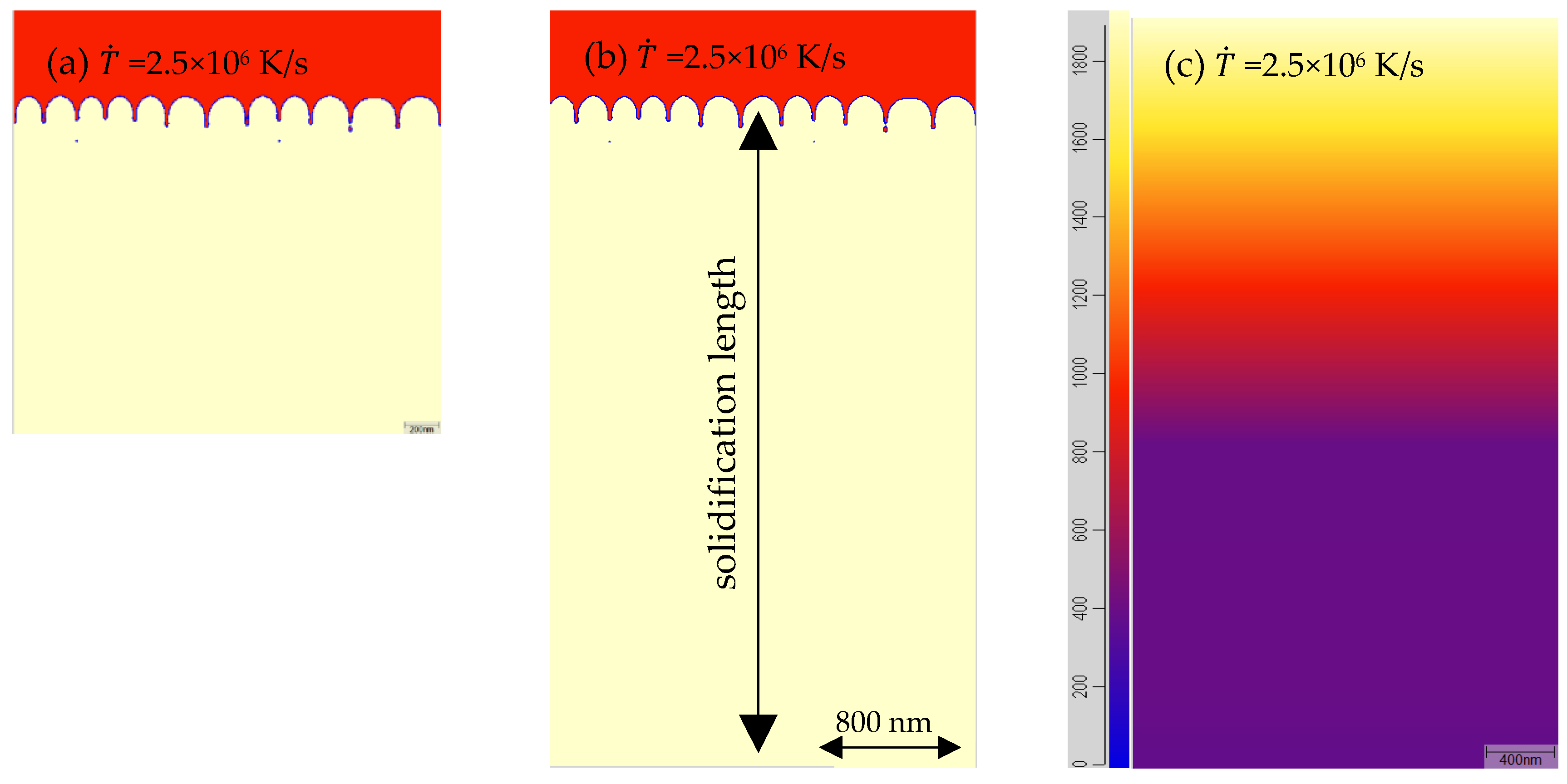
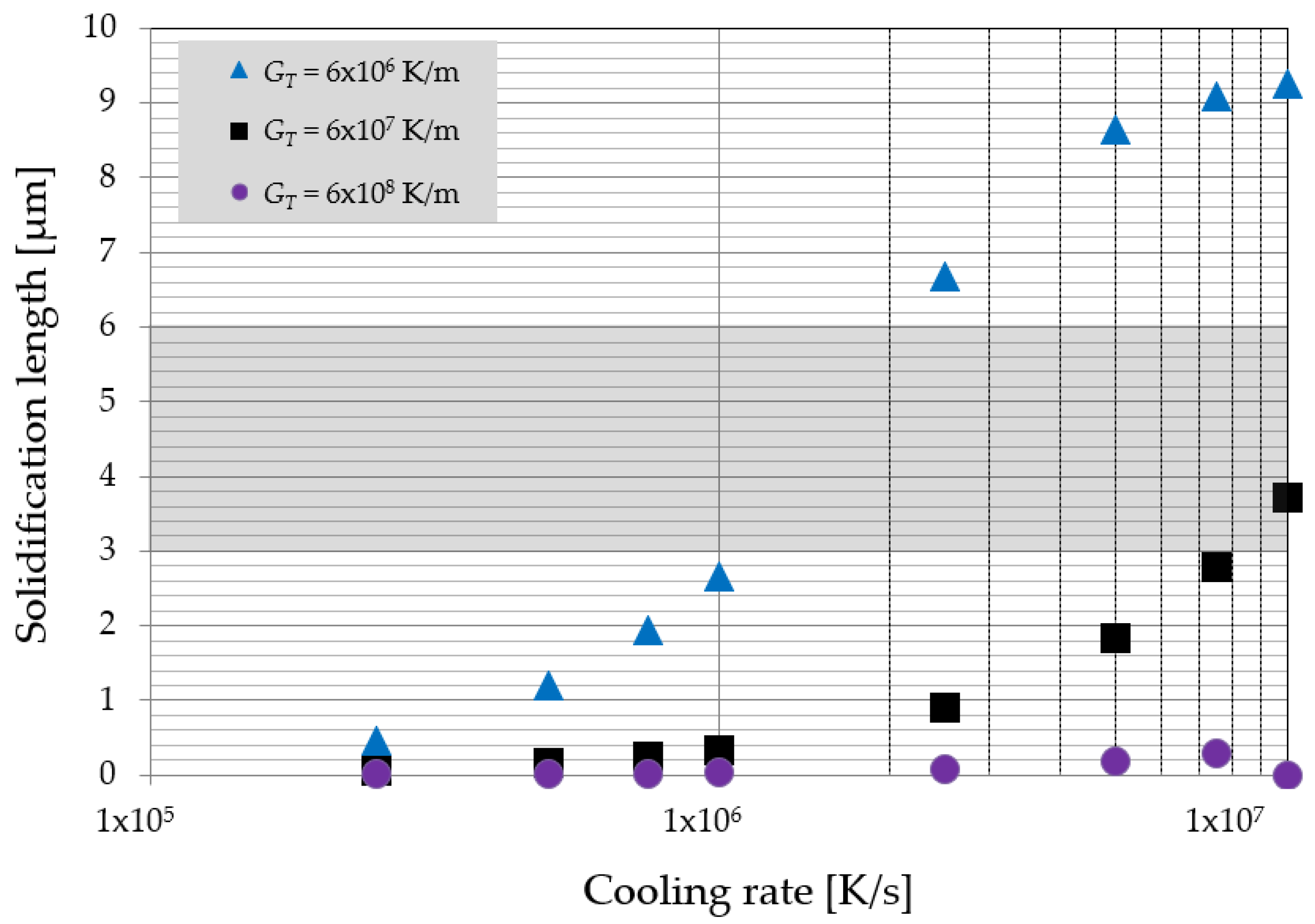
- The solidification length represents the height of the solidified structure measured from the initial grain position to the top s/l interface position. It was determined and investigated for the different selected process conditions. Figure 9 shows the solidification distance for a solidification time of tf = 1.1 × 10−5 s. Note, the solidification time is significantly longer than the expected solidification time of 6.6 × 10−6 ≤ tf ≤ 1.6 × 10−5 s. It can be seen that the minimum solidification length of 3 µm and a maximum solidification length of 6 µm, measured in Figure 1b for the cross-section, was reached for cooling rates of > 1 × 106 K/s and temperature gradients GT ≤ 6 × 107 K/m. The solidification time could not be determined experimentally. However, it can be stated with confidence that the observed structure (Figure 1b) could only result from growth occurring under a cooling rate < 6 × 108 K/m.
- (ii)
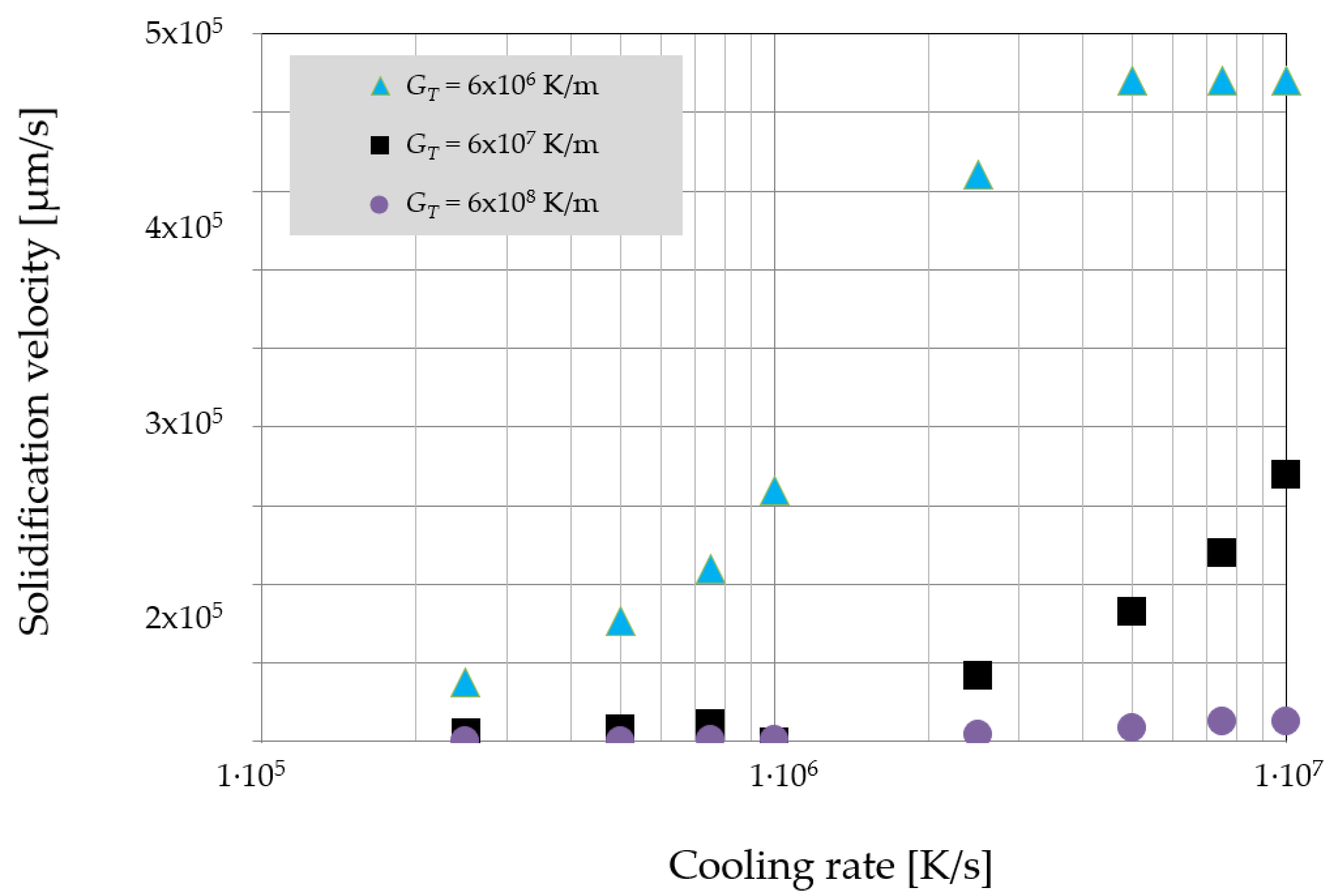
- Regardless of the process parameters selected, the obtained solidification velocities (4.7 × 105 µm/s for GT = 6 × 106 in Figure 10) were an order of magnitude lower than the absolute velocity Va ≥ 2.0 × 106 µm/s (Equation (3)). Therefore, the observed dendritic solidification could not have happened under rapid solidification conditions [10]. In other words, the solidification phenomena in the present cathode spot crater is not concerned by solute trapping, which decreases solute partitioning and alters equilibrium solidification velocity-undercooling relationships.
- (iii)
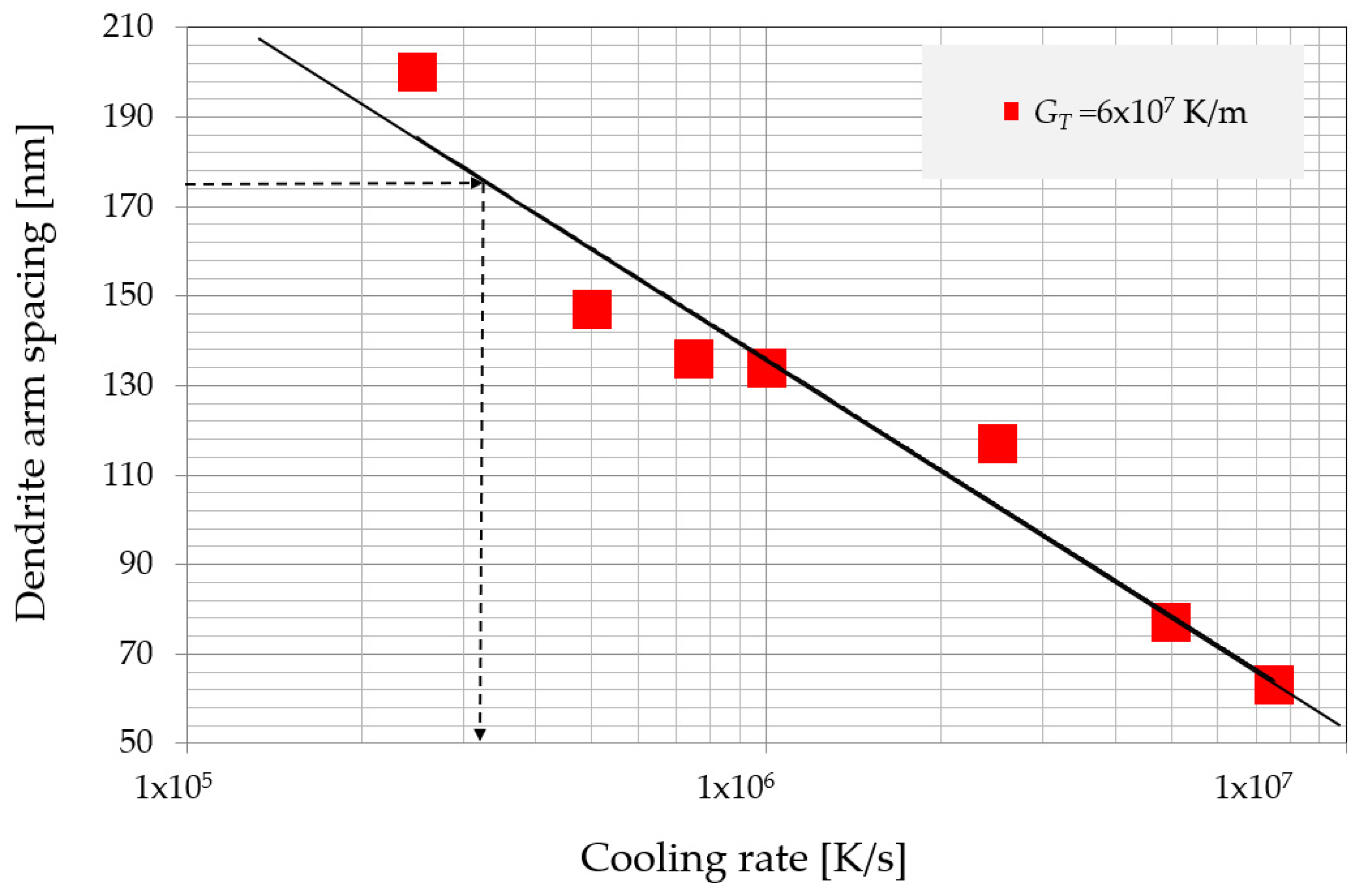
- The growth of secondary arms is triggered by perturbation of the s/l interface at the close vicinity to the dendrite tips. These arms undergo a process from cell-like to dendritic growth and some were eliminated due to competition with their neighbors. Later, a ripening process causes the secondary arms to change with time into coarser, less branched, and more widely-spaced ones. Furthermore, it is possible that the cooling rate during the numerical investigations to a planer front at steady-state conditions and the experimentally obtained dendrites were in a transition status. Due to their time-dependence, the dendritic side arm distances were evaluated at different times to determine an average secondary DAS (Figure 11). The regressing line suggests that a cooling rate of about = 3 × 105 K/s for a temperature gradient GT = 6 × 107 K/m was necessary to obtain the secondary arm spacing of λ2 = 1.75 × 10−7 m observed experimentally.
5. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Anders, A. Cathodic Arcs: From Fractal Spots to Energetic Condensation; Springer: New York, NY, USA, 2008. [Google Scholar]
- Mitterer, C. PVD and CVD Hard Coatings, in Comprehensive Hard Materials; Sarin, V.K., Llanes, L., Mari, D., Eds.; Elsevier: Amsterdam, The Netherlands, 2014; Volume 2, pp. 449–467. [Google Scholar] [CrossRef]
- Franz, R. Insights into surface modification and erosion of multi-element arc cathodes using a novel multilayer cathode design. J. Appl. Phys. 2020, 127, 113301. [Google Scholar] [CrossRef]
- Pohler, M.; Franz, R.; Ramm, J.; Polcik, P.; Mitterer, C. Cathodic arc deposition of (Al, Cr)2O3: Macroparticles and cathode surface modifications. Surf. Coat. Technol. 2011, 206, 1454–1460. [Google Scholar] [CrossRef]
- Franz, R.; Mendez Martin, F.; Polcik, P. Erosion behavior of composite Al-Cr cathodes in cathodic arc plasmas in inert and reactive atmospheres. J. Vac. Sci. Technol. A 2016, 34, 021304. [Google Scholar] [CrossRef]
- Golizadeh, M.; Mendez Martin, F.; Wuster, S.; Mogeritsch, J.P.; Kharicha, A.; Kolozsvari, S.; Mitterer, C.; Franz, R. Rapid solidification and metastable formation during surface modification of composite Al-Cr cathodes exposed to cathodic arc plasma. J. Mater. Sci. Technol. 2021, 94, 147–163. [Google Scholar] [CrossRef]
- Available Online: The Microstructure Evolution Simulation Software. Available online: https://micress.rwth-aachen.de (accessed on 17 October 2022).
- Available Online: Generate Materials Data with Thermo-Calc. Available online: https://thermocalc.com/ (accessed on 17 October 2022).
- Feurer, U.; Wunderlin, R. DGM Fachber; Wunderlin: Frankfurt, Germany, 1977; Volume 38. [Google Scholar]
- Kurz, W.; Fischer, D.J. Fundamentals of Solidification; Trans Tech Publications Ltd.: Wollerau, Switzerland, 1998. [Google Scholar]
- European Commission and Technical Research. Definition of Thermochemical and Thermo-Physical Properties to Provide a Database for the Development of New Light Alloys; COST European Cooperation in the Field of Scientific: Brussels, Belgium, 1998; Volume 2. [Google Scholar]
- Salas, G.B.; Ramirez, J.V.; Noguez, M.E.A.; Robert, T.N. Dendrite arm spacing—local solidification time relationship: An experimental model for a 70-30 brass and comparison with some theoretical models. Scr. Metall. Mater. 1995, 32, 295–299. [Google Scholar] [CrossRef]
- Lijun, W.; Xiao, Z.; Jiagang, L.; Ming, L.; Shenli, J. Study of cathode-spot crater and droplet formation in a vacuum arc. J. Phys. D Appl. Phys. 2021, 54, 215202. [Google Scholar]
- Santos, D.F.N.; Lisnyak, M.; Almeida, N.A.; Benilova, L.G.; Benilov, M.S. Numerical investigation of AC arc ignition on cold electrodes in atmospheric-pressure argon. J. Phys. D Appl. Phys. 2021, 54, 195202. [Google Scholar] [CrossRef]
- Huo, J.; Ronzello, J.A.; Rontey, A.; Wang, Y.; Jacobs, L.; Sommerer, T.; Cao, Y. Development of an arc root model for studying the electrode vaporization and its influence on arc dynamics. AIP Adv. 2020, 10, 085324. [Google Scholar] [CrossRef]
- Karimi-Sibaki, E.; Kharicha, A.; Wu, M.; Ludwig, A.; Bohacek, J. A Parametric Study of the Vacuum Arc Remelting (VAR) Process: Effects of Arc Radius, Side-Arcing, and Gas Cooling. Metall. Mater. Trans. B 2020, 51, 222–235. [Google Scholar] [CrossRef]
- Kaufmann, H.T.C.; Silva, C.; Benilov, M.S. Numerical simulation of the initial stage of unipolar arcing in fusion-relevant conditions. Plasma Phys. Control. Fusion 2019, 61, 095001. [Google Scholar] [CrossRef]



| Name | Symbol | Value | Unit |
|---|---|---|---|
| interfacial solid–liquid (s/l) energy | σ | 1.0 × 10−9 | J/m2 |
| entropy of fusion | Δ∑φ | 1.1 × 106 | J/m3 K |
| Gibbs–Thompson coefficient | Γ | 9.1 × 10−7 | m∙K |
| diffusion in the liquid | DL | 1.0 × 10−9 | m2/s |
| diffusion in the solid | DS | 1.0 × 10−12 | m2/s |
| concentration in the liquid | CL | 64.0 | at.% Al |
| concentration in the solid | CS | 35 | at.% Al |
| initial concentration in the liquid | Co | 50.0 | at.% |
| liquidus temperature | TL | 1773.0 | K |
| solidus temperature | TS | 1593.0 | K |
| slope of liquidus line | mL | −12.9 | K/at.% Al |
| distribution coefficient | k | 0.55 |
| Numerical Parameters | |||
|---|---|---|---|
| domain | |||
| dimension | x | 500 | cells |
| dimension (gradient direction) | z | 500 | cells |
| cell size | Δx | 0.005 | µm |
| Physical Parameters | |||
| diffusion coefficient | DL | 1.0 × 10−9 | m2/s |
| surface energy | σs/l | 1.0 × 10−5 | J/cm2 |
| temperature gradient | GT | variable | K/cm |
| initial concentration | C | 50 | at.% |
| entropy of fusion | SfL,α | 1.1 | J/cm3∙K |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mogeritsch, J.P.; Franz, R.; Golizadeh, M.; Mitterer, C.; Kharicha, A. Determination of Cooling Rate and Temperature Gradient during Formation of Cathode Spot Craters in a Vacuum Arc. Crystals 2022, 12, 1486. https://doi.org/10.3390/cryst12101486
Mogeritsch JP, Franz R, Golizadeh M, Mitterer C, Kharicha A. Determination of Cooling Rate and Temperature Gradient during Formation of Cathode Spot Craters in a Vacuum Arc. Crystals. 2022; 12(10):1486. https://doi.org/10.3390/cryst12101486
Chicago/Turabian StyleMogeritsch, Johann Peter, Robert Franz, Mehran Golizadeh, Christian Mitterer, and Abdellah Kharicha. 2022. "Determination of Cooling Rate and Temperature Gradient during Formation of Cathode Spot Craters in a Vacuum Arc" Crystals 12, no. 10: 1486. https://doi.org/10.3390/cryst12101486
APA StyleMogeritsch, J. P., Franz, R., Golizadeh, M., Mitterer, C., & Kharicha, A. (2022). Determination of Cooling Rate and Temperature Gradient during Formation of Cathode Spot Craters in a Vacuum Arc. Crystals, 12(10), 1486. https://doi.org/10.3390/cryst12101486








