The Third-Order Elastic Constants and Mechanical Properties of 30° Partial Dislocation in Germanium: A Study from the First-Principles Calculations and the Improved Peierls–Nabarro Model
Abstract
:1. Introduction
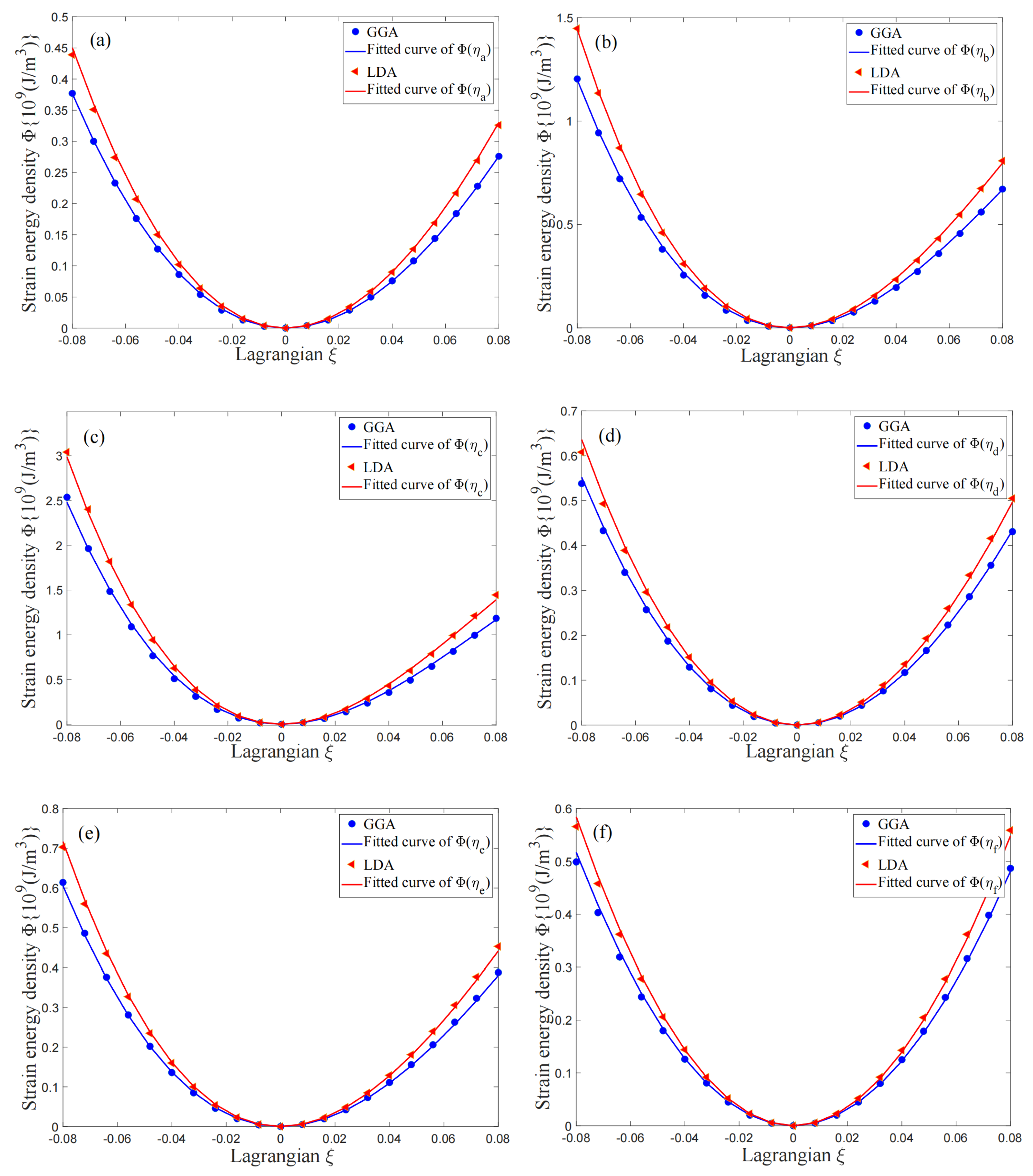
2. Computational Methods and Results
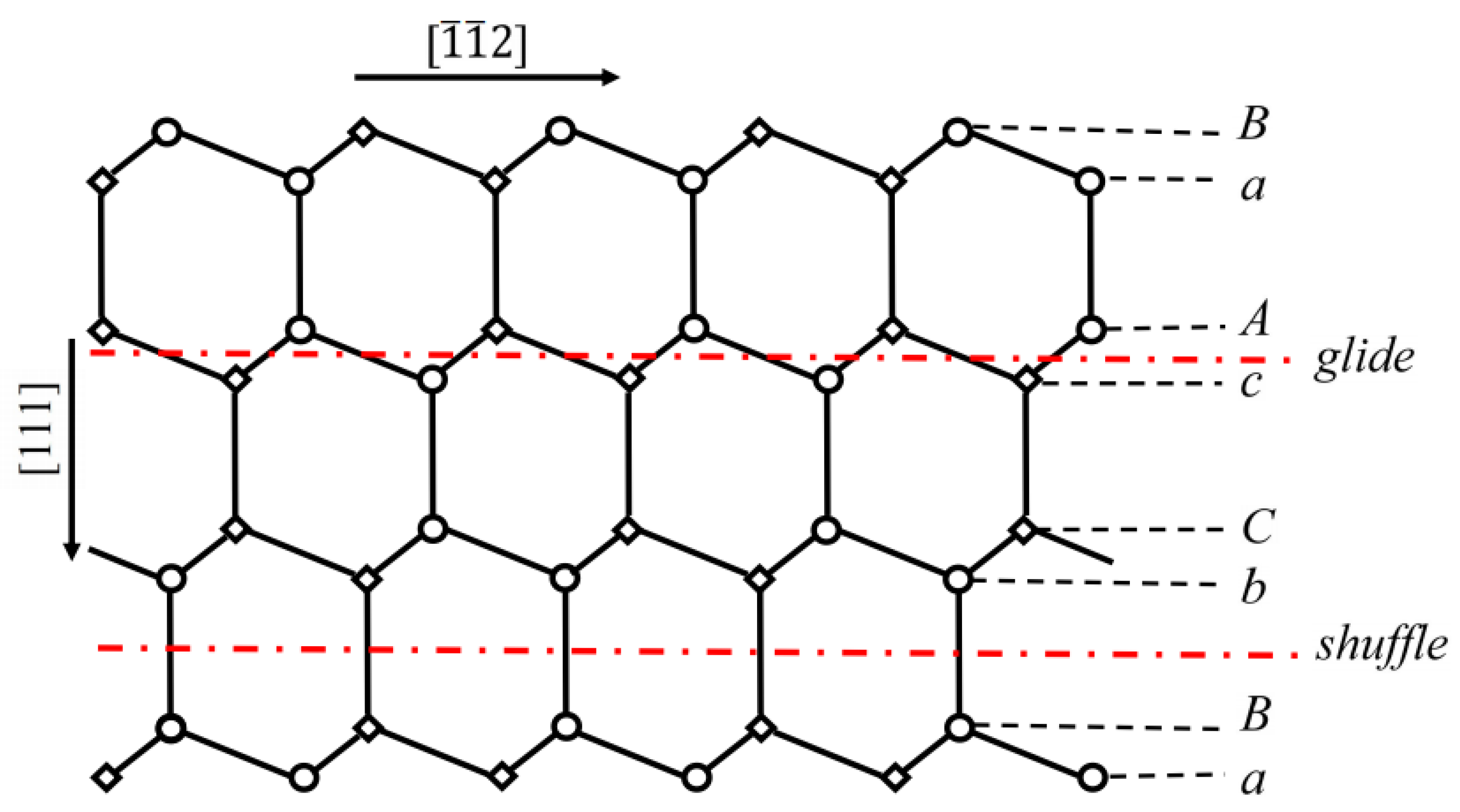
3. Mechanical Properties of Partial Dislocation in Germanium
4. Summary and Discussion
5. Conclusions
- (1)
- The lattice and elastic constants calculated by LDA method are in better agreement with the experimental results.
- (2)
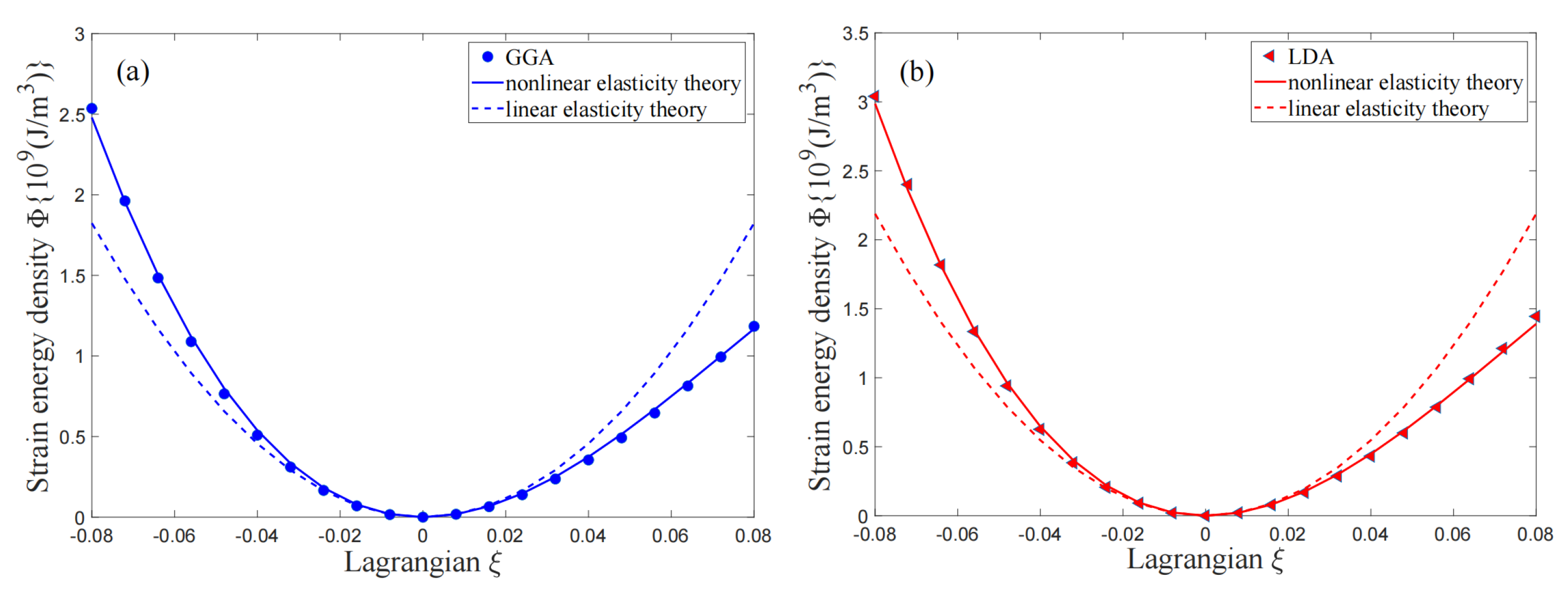
- When the Lagrangian strain is larger than 2% but lower than 8%, the nonlinear effects cannot be neglected and the third-order effects should be considered.
- (3)
- If the ISFE is very low relative to the USFE, the original γ—surface can be directly used to study the dislocation structure and Peierls stress.
- (4)
- For the narrow dislocations in covalent materials, the discrete effect and the contribution of strain energy to the total energy of dislocation cannot be neglected.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Birch, F. Finite Elastic Strain of Cubic Crystals. Phys. Rev. 1947, 71, 809–824. [Google Scholar] [CrossRef]
- Murnaghan, F. Finite Deformation of an Elastic Solid; Wiley: New York, NY, USA, 1951. [Google Scholar]
- Thurston, R.N.; Brugger, K. Third-Order Elastic Constants and the Velocity of Small Amplitude Elastic Waves in Homogeneously Stressed Media. Phys. Rev. 1964, 133, A1604–A1610. [Google Scholar] [CrossRef]
- Brugger, K. Thermodynamic Definition of Higher Order Elastic Coefficients. Phys. Rev. 1964, 133, A1611–A1612. [Google Scholar] [CrossRef]
- Bhagavantam, S. Crystal Symmetry and Physical Properties; Academic: New York, NY, USA, 1966. [Google Scholar]
- Sfyris, D.; Dragatogiannis, D.A.; Charitidis, C. Nonlinear elastic constitutive modeling of α-Ge. Int. J. Non-liner Mech. 2021, 134, 103737. [Google Scholar] [CrossRef]
- Mohabuth, M.; Khanna, A.; Hughes, J.; Vidlera, J.; Kotousova, A.; Ng, C.T. On the determination of the third-order elastic constants of homogeneous isotropic materials utilising Rayleigh waves. Ultrasonics 2019, 96, 96–103. [Google Scholar] [CrossRef] [Green Version]
- Łopuszyński, M.; Majewski, J.A. Ab initio calculations of third-order elastic constants and related properties for selected semiconductors. Phys. Rev. B 2007, 76, 045202. [Google Scholar] [CrossRef] [Green Version]
- Hiki, Y. Higher Order Elastic Constants Of Solids. Annu. Rev. Mater. Sci. 1981, 11, 51–73. [Google Scholar] [CrossRef]
- Johal, A.S.; Dunstan, D.J. Reappraisal of experimental values of third-order elastic constants of some cubic semiconductors and metals. Phys. Rev. B 2006, 73, 024106. [Google Scholar] [CrossRef]
- McSkimin, H.J.; Andreatch, P. Measurement of Third-Order Moduli of Silicon and Germanium. J. App. Phys. 1964, 35, 3312–3319. [Google Scholar] [CrossRef]
- Bogardus, E.H. Third-Order Elastic Constants of Ge, MgO, and Fused SiO2. J. App. Phys. 1965, 36, 2504–2513. [Google Scholar] [CrossRef]
- Abe, Y.; Imai, K. Anharmonic Properties of Ultrasounds in Diamond-Type Crystals and Quartz Plate under an Intense Exicitation. Jpn. J. Appl. Phys. 1986, 25, 67–69. [Google Scholar] [CrossRef] [Green Version]
- Souadkia, M.; Bennecerand, B.; Kalarasse, F. Elastic, vibrational and thermodynamic properties of α-Sn based group IV semiconductors and GeC under pressure. J. Phys. Chem. Solids 2013, 74, 1615–1625. [Google Scholar] [CrossRef]
- Fukumoto, A. First-principles pseudopotential calculations of the elastic properties of diamond, Si, and Ge. Phys. Rev. B 1990, 42, 7462–7469. [Google Scholar] [CrossRef] [PubMed]
- Bhatti, S.S.; Singh, D.P. Third-Order Elastic Constants of Pure and Doped Ge, Si and InSb, GaAs from Keating’s Theory. Acta Acust. United Acust. 1983, 53, 262. [Google Scholar]
- Alexander, H.; Haasen, P. Solid State Physics; Seitz, F., Turnbull, D., Eds.; Academic: New York, NY, USA, 1968; Volume 22, pp. 27–158. [Google Scholar]
- Joos, B.; Ren, Q.; Duesbery, M.S. Peierls-Nabarro model of dislocations in silicon with generalized stacking-fault restoring forces. Phys. Rev. B 1994, 50, 5890–5898. [Google Scholar] [CrossRef]
- Ren, Q.; Joos, B.; Duesbery, M.S. Test of the Peierls-Nabarro model for dislocations in silicon. Phys. Rev. B 1995, 52, 13223–13228. [Google Scholar] [CrossRef]
- Edagawa, K.; Koizumi, H.; Kamimura, Y.; Suzuki, T. Temperature dependence of the flow stress of III–V compounds. Phil. Mag. A 2000, 80, 2591–2608. [Google Scholar] [CrossRef]
- Hirth, J.P.; Lothe, J. Theory of Dislocations, 2nd ed.; Wiley: New York, NY, USA, 1982; p. 373. [Google Scholar]
- Wang, S.F.; Zhang, H.L.; Wu, X.Z.; Liu, R.P. Theoretical calculation of the dislocation width and Peierls barrier and stress for semiconductor silicon. J. Phys. Condens. Matter 2010, 22, 055801. [Google Scholar] [CrossRef]
- Zhang, H.L. Calculation of shuffle 60° dislocation width and Peierls barrier and stress for semiconductors silicon and germanium. Eur. Phys. J. B 2011, 81, 179–183. [Google Scholar] [CrossRef]
- Wang, S.F.; Liu, R.P.; Wu, X.Z. The discrete correction of the core structure for the <100>{010} edge dislocation in bcc Fe. J. Phys. Condens. Matter 2008, 20, 485207. [Google Scholar] [CrossRef]
- Wu, X.Z.; Wang, S.F. On the properties of <100>{110} dissociated superdislocation in B2 structure YAg and YCu: Core structure and Peierls stress. Front. Mater. Sci. China 2009, 3, 205–211. [Google Scholar] [CrossRef]
- Every, A.G.; McCurdy, A.K. Second and higher order elastic constants. In Landolt-B’ornstein Numerical Data and Functional Relationships in Science and Technology; New Series III/29a; Springer: Berlin, Germany, 1992. [Google Scholar]
- Born, M.; Huang, K. Dynamical Theory of Crystal Lattices; Oxford University Press: London, UK, 1956. [Google Scholar]
- Le Page, Y.; Saxe, P. Symmetry-general least-squares extraction of elastic coefficients from ab initio total energy calculations. Phys. Rev. B 2001, 63, 174103. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for open-shell transition metals. Phys. Rev. B 1993, 48, 13115–13118. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmuller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmuller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [Green Version]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Bouderba, H.; Djaballah, Y.; Belgacem-Bouzida, A.; Beddiaf, R. Temperature and pressure effects on phase stabilities in theCa–Ge system from first-principles calculations and Debye-Gruneisen model. Intermetallics 2012, 28, 108–119. [Google Scholar] [CrossRef]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Rinehart and Winston: New York, NY, USA, 1975. [Google Scholar]
- Martienssen, W.; Warlimont, H. Springer Handbook of Condensed Matter and Materials Data; Springer: Berlin, Germany, 2005. [Google Scholar]
- Adachi, S. Properties of Group−IV, III−V and II−VI Semiconductors; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Wang, S.F. Dislocation solution in slowly varying approximation. Phys. Lett. A 2003, 313, 408–411. [Google Scholar] [CrossRef]
- Christian, J.W.; Vitek, V. Dislocations and stacking faults. Rep. Prog. Phys. 1970, 1, 307–411. [Google Scholar] [CrossRef]
- Kamimura, Y.; Edagawa, K.; Iskandarov, A.M.; Osawa, M.; Umeno, Y.; Takeuchi, S. Peierls stresses estimated via the Peierls-Nabarro model using ab-initio γ-surface and their comparison with experiments. Acta Mater. 2018, 148, 355–362. [Google Scholar] [CrossRef]
- Wang, S.F. Dislocation energy and Peierls stress: A rigorous calculation from the lattice theory. Chin. Phys. 2006, 15, 1301–1309. [Google Scholar]

| Present Calculations | Previous Calculations | Experiments | |
|---|---|---|---|
| 5.785 a, 5.648 b | 5.57 c, 5.763 e | 5.66 f, 5.65 i | |
| 102 a, 122 b | 129.92 c, 103.1 e | 129 g, 128.35 j | |
| 44 a, 53 b | 47.98 c, 36.8 e | 48.3 g, 48.23 j | |
| 52 a, 59 b | 67.17 c, 53.1 e | 67.1 g, 66.66 j | |
| −589 a, −705 b | −681 d | −710 ± 6 h, −716 ± 20 j, −714 k | |
| −322 a, −396 b | −363 d | −389 ± 3 h, −403 ± 10 j, −388 k | |
| −20 a, −19 b | −9 d | −18 ± 6 h, −18 ± 30 j, −34 k | |
| −35 a, −37 b | 9 d | −23 ± 16 h, −53 ± 5 j, −9 k | |
| −244 a, −303 b | −323 d | −292 ± 8 h, −315 ± 5 j, −303 k | |
| −35 a, −35 b | −45 d | −53 ± 7 h, −47 ± 10 j, −48 k |
| GGA | LDA | ||
|---|---|---|---|
| 39.5 | 0.269 | 44.5 | 0.286 |
| GGA | Original | 0.0700 | −0.73 | 0.30 | 0.18 | 0.24 | 17.4 | 1.6 |
| Effective | 0.0685 | −0.73 | 0.30 | 0.18 | 0.24 | 16.9 | 1.4 | |
| LDA | Original | 0.0795 | −0.74 | 0.31 | 0.18 | 0.24 | 20.1 | 1.6 |
| Effective | 0.0775 | −0.74 | 0.31 | 0.19 | 0.25 | 19.3 | 1.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Lu, D.; Sun, Y.; Fu, Y.; Tong, L. The Third-Order Elastic Constants and Mechanical Properties of 30° Partial Dislocation in Germanium: A Study from the First-Principles Calculations and the Improved Peierls–Nabarro Model. Crystals 2022, 12, 4. https://doi.org/10.3390/cryst12010004
Zhang H, Lu D, Sun Y, Fu Y, Tong L. The Third-Order Elastic Constants and Mechanical Properties of 30° Partial Dislocation in Germanium: A Study from the First-Principles Calculations and the Improved Peierls–Nabarro Model. Crystals. 2022; 12(1):4. https://doi.org/10.3390/cryst12010004
Chicago/Turabian StyleZhang, Huili, Defang Lu, Yu Sun, Yunchang Fu, and Lumei Tong. 2022. "The Third-Order Elastic Constants and Mechanical Properties of 30° Partial Dislocation in Germanium: A Study from the First-Principles Calculations and the Improved Peierls–Nabarro Model" Crystals 12, no. 1: 4. https://doi.org/10.3390/cryst12010004
APA StyleZhang, H., Lu, D., Sun, Y., Fu, Y., & Tong, L. (2022). The Third-Order Elastic Constants and Mechanical Properties of 30° Partial Dislocation in Germanium: A Study from the First-Principles Calculations and the Improved Peierls–Nabarro Model. Crystals, 12(1), 4. https://doi.org/10.3390/cryst12010004






