Theoretical Investigation of the EPR G-Factor for the Axial Symmetry Ce³⁺ Center in the BaWO₄ Single Crystal

Abstract

The parameters g-factor ($g_{\left|\right|}$ and $g_{\perp }$) together with the local structure of the Ce³⁺ center in BaWO4 single crystal (scheelite structure crystals) were theoretically investigated using a complete diagonalization procedure of energy matrix (CDM method). The intrinsic parameters were calculated. It is shown that the experimental and the calculated values of the g-factors are in good agreement. The angular distortion has also been calculated. It was found that the polar angles of the impurity–ligand bonding are smaller than in BaWO₄ single crystal $\left(\mathrm{\Delta }\theta \approx {1.0}^{°} \right)$ . The validity of the results and the changing in the local environment of the impurity–cerium ion is also discussed.

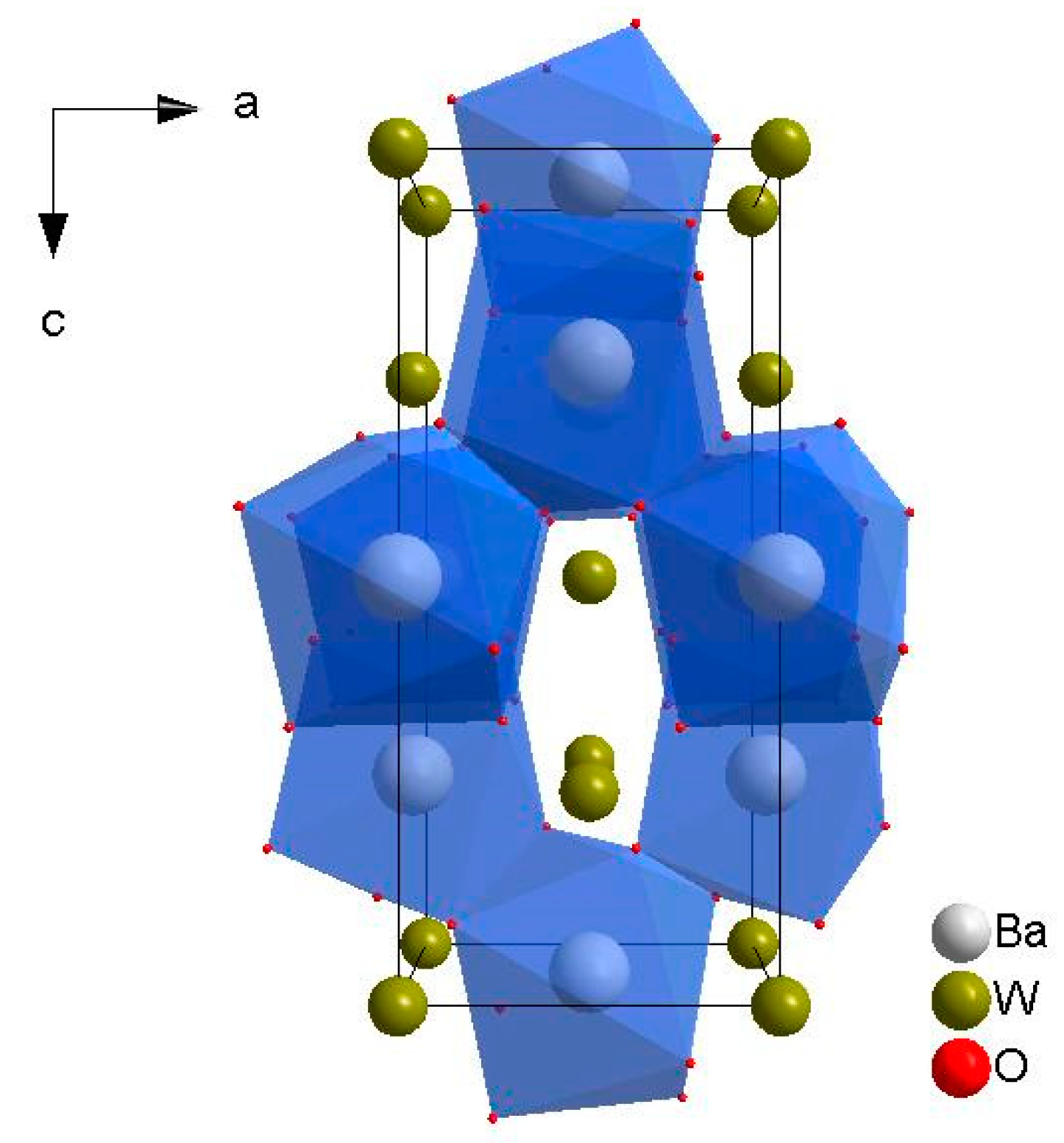
1. Introduction

The scheelite structure crystals, tungstate AWO₄ (A = Ba, Sr, Ca, Pb) doped with trivalent rare-earth ions (Re³⁺), have received much interest thanks to their unusual properties such as luminescence, nonlinear optical activity, or scintillation [1,2,3,4,5,6] and for their application. The barium tungstate (BaWO₄, BWO) is a very interesting inorganic optical material. Its potential applications include stimulated Raman scattering [7], scintillators and X-ray phosphor [8]. The blue and green PL emissions of BaWO₄ are widely discussed for their importance in future optical applications [9,10,11]. The barium tungstate crystals (BaWO₄) can also be used as a material for designing all solid-state lasers, especially for a wide variety of pump pulse durations in Raman laser pulses [12,13]. The barium tungstate (BaWO₄) single crystals and nanocrystals have recently become the subject of intense scientific research [10,14]. More generally, many tungstate crystals like BaWO₄, PbWO₄, CaWO₄, or SrWO₄ are the subject of intense research because of their possible applications in optical devices such as lasers, or scintillators. Doping of tungstate crystals with rare earth elements (RE³⁺) like cerium (Ce³⁺), ytterbium (Yb³⁺), erbium (Er³⁺), etc., is a method of increasing their optical activity and their future applications.

The wolframite and scheelite structures are common structure types for ABO₄ compounds [15]. The BaWO₄ crystal crystallizes in tetragonal space group with C⁶_4h (I4₁/a) [16]. Lattice parameters of BWO are: a = b = 5.6148 Å, c = 12.721 Å [15,16]. Both Ba²⁺ and W⁶⁺ sites have S₄ point symmetry. The Ba²⁺ ion is coordinated by eight O^2- ions in the form of dodecahedron [BaO₈] made of two rotated, interpenetrated tetrahedrons. The distance Ba–O is equal to 2.7857 Å and 2.8310 Å, respectively, for two tetrahedrons. Two tetrahedrons [BaO₄] make up a dodecahedron [BaO₈]. Apart from dodecahedrons [BaO₈], [WO₄] tetrahedrons are an important part in the unit cell of the BaWO₄. The [WO₄] tetrahedron has an almost regular shape only slightly distorted along the c (S₄) axis. The distance between the W and the O ions is equal to 1.8230 Å [15]. Dodecahedrons [BaO₈] are connected by their edges. Each O atom belongs to two dodecahedrons [BaO₈] and one tetrahedron [WO₄]. Figure 1 shows the unit cell structure of BaWO₄ viewed along the b axis with marked dodecahedrons [BaO₈] (blue). One can see that the unit cell of the BaWO₄ has the biggest volume among ABO₄ compounds (V = 401.0 ų) [15].

The present paper is a continuation of our research on the local structure of the BaWO₄ single crystals doped and co-doped with ions of various elements. There are a few papers that have been investigating a local structure of BaWO₄ single crystals doped and co-doped with, Ce, Na, Pr using EPR method [17,18,19]. Five paramagnetic centers with axial symmetry and about ten centers with low symmetry (C₂) were found [17,18]. In the next step, we were investigated the connection between the g—shift and the environment of the Ce³⁺ centers with axial symmetry for four BaWO₄ doped with Ce (cerium) and co-doped with Na (sodium) with different concentrations [20]. We also determined dislocations of Ce³⁺ ions. We were used a simplified method proposed by D. J. Newman [21]. Structural information can be obtained from spin–Hamiltonian parameters (and/or g-parameters) using a superposition model (SPM) [22,23] or perturbation methods (PM) up to second-order [24]. Previously, we used a simplified Newman model, a g-shift model [20]. In this paper, we choose to use superposition model (SPM) to obtain structural information on trivalent cerium ion (Ce³⁺) and its surroundings. Our investigations were focused on the most intense paramagnetic centers with axial symmetry presented in all four BaWO₄ single crystals doped with Ce and co-doped with Na [17,18,19]. Obtained results will be compared with our previous results and other investigations.

We have found only one paper focused on rare-earth centers, this is Er³⁺ centers, in BaWO₄ single crystals [25] and one other about similar, tetragonal Ce³⁺ centers in YPO₄ and LuPO₄ crystals [26]. However, one can find that there are several papers about: (a) Ce³⁺ and Yb³⁺ in garnets [27,28,29], (b) Ce³⁺, Yb³⁺ in YF₃ crystal and Ce³⁺ in fluoride ligands [30,31], (c) Yb³⁺ in CaWO₄ crystal [32], (d) Er³⁺ in CaWO₄ and SrWO₄ crystals [33], Er³⁺ in zircon-type compounds [34] or Er³⁺ in PbMoO₄ and SrMoO₄ [35], Er³⁺ at the Th⁴⁺ site in ThGeO₄ [36], (e) Nb³⁺, Yb³⁺ in double tungstate’s and molybdates [37], (f) color centers in BaWO₄ [38,39] and others [40,41]. All these papers are focused on analysis of spin–Hamiltonian parameters for the rare-earth ion surrounded by oxygen’s [ReO₈] or fluoride’s dodecahedrons [ReF₈]. It is interesting to compare our results with the results from these papers.

It is assumed that dopant ions such as rare earth ions (Re³⁺ like Ce³⁺, Er³⁺, or Yb³⁺) substitute in place of barium ions (Ba²⁺) [24,25,26,27,28,29,30]. Hence, two Re_Ba substitutions gives two excess positive charges, which are compensated by one the barium vacancy (V_Ba). The associated vacancies (V_Ba) do not necessarily affect the surroundings of the dopant ion, dodecahedron [ReO₈]. In our previous articles, we described five paramagnetic centers (Ce³⁺) with axial symmetry detected in the EPR spectra of four BWO monocrystals doped with cerium and/or co-doped with sodium: (1) BaWO₄: 0.5% at. Ce, (2) BaWO₄: 1.0% at. Ce, (3) BaWO₄: 0.5% at. Ce, 1.0% at. Na and (4) BaWO₄: 1.0% at. Ce, 2.0% at. Na [17,18,19,20]. One of these centers is the strongest and occurs in all four monocrystals. It is g-factors are as follows: $g_{\left|\right|}=1.506\left(1\right)$, $g_{\perp }=2.712\left(2\right)$. We will focus our analysis and calculations on this center with axial symmetry.

2. Calculations

Rare-earth ions (like Ce³⁺) substitute Ba²⁺ site in the elementary cell of the barium tungstate (BaWO₄) [17,18,19,20,25,26,38]. The local symmetry of the Ce_Ba site (Ba²⁺ place occupied by Ce³⁺) is S₄ (tetragonal symmetry). However, the D_2d symmetry approximation is often taken for many other rare-earth impurity ions (e.g., Yb³⁺, Er³⁺) in many scheelite-type oxygen and fluoride compounds because of the small distortion [25,26,38,39]. We will apply the D_2d symmetry approximation here. Cerium ion (4f¹ electronic configuration) in tetragonal symmetry has ²F_5/2 ground state and ²F_7/2 exited state. The crystal field with D_2d symmetry splits the ground and exited states into three and four doublets, respectively. The Ce³⁺ ion has an effective spin S = ½, because only the lowest doublet is populated. The effective spin–Hamiltonian for Ce³⁺ ion in an external magnetic fields can be written as [42]:

$$\widehat{H}={\widehat{H}}_f+{\widehat{H}}_{CF}+{\widehat{H}}_{SO}+{\widehat{H}}_{CF}+{\widehat{H}}_Z$$

(1)

where ${\widehat{H}}_f$ is free ion term, ${\widehat{H}}_{SO}=\xi \left(\widehat{L}\mathrm{·}\widehat{S}\right)$ denotes spin–orbit interaction term, with $\xi$—the spin-orbit coupling parameter, ${\widehat{H}}_{CF}$—crystal field term, and ${\widehat{H}}_Z$ is Zeeman term. The Zeeman term is usually expressed as:

$${\widehat{H}}_Z=g_j{\mu }_B\widehat{J}·\overrightarrow{H}$$

(2)

where $\widehat{J}$ is total angular momentum in the ^2S+1L_J manifold. Therefore, we obtain 14 × 14 energy matrix and the energy levels (e.g., eigenvalues) can be calculated after diagonalization of spin–Hamiltonian matrices (1). Usually, the spin–Hamiltonian parameters for 4f¹ ion are calculated using so-called complete diagonalization method (CDM), where the Zeeman term is not included to spin–Hamiltonian [26]. In this paper, the Zeeman term ${\widehat{H}}_Z$ is added to spin–Hamiltonian and next the full spin–Hamiltonian is subject of complete diagonalization method (CDM) according H.G. Liu et al. [26]. Thus, no perturbation formula is needed. We get only g-factor parameters obtained from EPR measurements for Ce³⁺ in BaWO₄ [17,18,19,20]. The g-factor can be obtained using CDM method using the following equations:

$$g_{\left|\right|}=\frac{E_{\left|\right|}}{{\mu }_B{H}_{\left|\right|}}=\frac{E_Z}{{\mu }_B{H}_Z},g_{\perp }=\frac{E_{\perp }}{{\mu }_B{H}_{\perp }}=\frac{E_X}{{\mu }_B{H}_X}$$

(3)

$E_Z$ and $E_X$ are the Zeeman splitting between two the lowest energy levels (doublet) obtained by complete diagonalization the energy matrix from Equation (1), where the magnetic field is directed along the Z and the X axes, respectively. The crystal field interaction term ${\widehat{H}}_{CF}$ for Ce³⁺ ion (4f¹) in tetragonal symmetry (D_2d) in terms of Stevens operator equivalent can be given as follows [22,42]:

$${\widehat{H}}_{CF}=B_2^0{O}_2^0+B_4^0{O}_4^0+B_4^4{O}_4^4+B_6^0{O}_6^0+B_6^4{O}_6^4$$

(4)

$B_k^{q }$ there are the crystal field parameters, $k=2, 4, 6, \left|q\right| \le k$. According the superposition model (SPM), the crystal field parameters $B_k^q$ can be calculated as follows [21,22,23]:

$$B_k^q={\displaystyle \sum }_{i=1}^n{\overline{A}}_k\left(R_0\right){\left(\raisebox{1ex}{$R_0$}\!\left/ \!\raisebox{-1ex}{$R_i$}\right.\right)}^{t_k}{K}_k^q\left({\theta }_i,{\phi }_i\right)$$

(5)

the summation is only over the nearest neighbor ligands. In the case of the dodecahedron [CeO₈] n = 8. The parameters $t_k$ and ${\overline{A}}_k\left(R_0\right)$ are the power law exponents and the intrinsic parameters, respectively. $R_0$, called the reference distance, is often taken as a usual distance between the metal ion and the ligands. The parameters $K_k^q\left({\theta }_i, {\phi }_i\right)$ are the geometric coordination factors [23,24,26,43]. The Ce³⁺ ion is surrounded by eight O²⁻ ions, and only these oxygens O²⁻ ligands were included in the SPM model [20,28]. The dodecahedron [BaO₈] is made in the form of two interpenetrated and rotated tetrahedrons. Figure 2 shows fragments of the BWO cell structure: a schematic view of the dodecahedron [BaO₈] consisting of two rotated tetrahedrons with marked crystallographic axis Z (Z || c) and two polar angles ${\theta }_i$.

Therefore, we have two sets of the structural parameters: the distance $R_i^H$ and the polar angles ${\theta }_i$, and the azimuthal angle $s {\phi }_i$, where $i=1, 2$ means the first and the second tetrahedron, respectively. These polar and azimuthal angles are angles between the metal–ligand distance $R_i^H$ and Z (fourfold) axis and X axis of the crystal, respectively [25,44]. The values for the structural parameters $R_1^H\approx 0.2778$ [nm], ${\theta }_1\approx 69.05°$, ${\phi }_1\approx -35.16°$, and $R_2^H\approx 0.2738$ [nm], ${\theta }_2\approx 143°$, ${\phi }_2\approx -24.41°$, were obtained for the Ba²⁺ ion in the first and the second tetrahedron, respectively (see Figure 2) [44]. The ionic radii and the charge of the impurity rare-earth ion (like Ce³⁺) usually are different form host, e.g., barium ion (Ba²⁺). However, the local lattice relaxation arising from the size mismatch can be sufficiently good approximated from the equation [25,26]:

$$R_j\approx R_j^H+\frac{r_I-r_H}{2}$$

(6)

where $r_I$ and $r_H$ are the ionic radii of the impurity and the host ion, respectively. We assumed that $r_I\approx 0.101$ [nm] and $r_H\approx 0.135$ [nm] for Ce³⁺ and Ba²⁺ ions (both 6 coordination), respectively [43]. Now, one can estimate the distances $R_i, i=1, 2$ for Ce³⁺ ion in both two tetrahedrons in BaWO₄ single crystals according to Equation (6). The impurity–oxygens distances are changed. However, it is usually assumed that the polar angels ${\theta }_i$ and the azimuthal angles ${\phi }_i$ are the same as in the host crystal [25,44]. The structural data that were used in the calculations have been gathered in

Table 1. The structural data for Ce³⁺ ion in the barium site Ba²⁺ in the barium tungstate (BaWO₄) [44].

	${\mathit{R}}_{\mathit{i}} \left[\mathbf{nm}\right]$	${\mathit{R}}_{\mathit{i}}^{\mathit{H}}\left[\mathbf{nm}\right]$	${\mathit{\theta }}_{\mathit{i}}\left[0\right]$	${\mathit{\phi }}_{\mathit{i}}\left[0\right]$
i = 1	0.2608	0.2778	69.05	−35.16
i = 2	0.2568	0.2738	143.00	−24.41

.

$R_0$ (the reference distance) is taken as the average distance $R_0\approx \overline{R}=0.2588$ [nm]. The power law exponents were established as $t_2\approx 5$, $t_4\approx 6$, $t_6\approx 10$ [22,23,26,28,40,41]. We also have to estimate the value of the spin–orbit coupling parameters. H. Ramanantoanina et al. calculated the value of the spin–orbit coupling in the Ce³⁺ ion embedded in the Cs₂NaYCl₆ crystal host ${\xi }_{4f}\approx 624.1$ [cm⁻¹] [45]. Jun Wen et al. in the study of the Ce³⁺ ion doped in the various fluoride compounds estimated the value of ${\xi }_{4f}\approx 614.9$ [cm⁻¹] [46]. H.G. Liu et al. in the investigation of the tetragonal Ce³⁺ centers in the YPO₄ and LuPO₄ crystals calculated the spin–orbit coupling parameter as $\xi \approx 608$ [cm⁻¹] and $\xi \approx 604$ [cm⁻¹] for YPO₄ and LuPO₄ crystals, respectively [26]. We decided to take the average of the two last spin–orbit coupling parameters, because YPO₄ and LuPO₄ crystals have similar zircon-type structure and the Ce³⁺ ion is surrounding by oxygens ligands, oxygens dodecahedron [CeO₈] [26]. Therefore, we assumed that the spin–orbit coupling parameter (spin–orbit term in spin–Hamiltonian) is equal to $\xi \approx 606$ [cm⁻¹].

In first step, by substituting all these parameters into Equations (1)–(6), we can estimate the intrinsic parameters ${\overline{A}}_2\left(R_0\right), {\overline{A}}_4\left(R_0\right), {\overline{A}}_6\left(R_0\right)$ by fitting the EPR g-factors calculated using the new CDM method to the experimental values. Experimental and calculated values of the g-factor were calculated and have been gathered in

Table 2. The EPR g factors for the axial Ce³⁺ center in BaWO₄ single crystal.

	${\mathit{g}}_{\left|\right|}$	${\mathit{g}}_{\perp }$	$\mathbf{\Delta }\mathit{g}={\mathit{g}}_{\left|\right|}-{\mathit{g}}_{\perp }$
Exp. [18,19,20]	1.506 (1)	2.712 (2)	−1.206
Calc. a	1.506 (1)	2.730 (20)	−1.224
Calc. b	1.506 (1)	2.725 (15)	−1.219

^a Calculated using structural polar angles ${\theta }_i$ in the host. ^b Calculated using the local polar angles ${\theta }_i^{\prime }$ due to the angular distortion.

, in the first and the second row. The differences between parallel and the perpendicular g-factor ($\mathrm{\Delta }g=g_{\left|\right|}-g_{\perp }$) have also been presented.

The calculated intrinsic parameters for Ce³⁺ center in BaWO₄ single crystal get values:

$${\overline{A}}_2\left(R_0\right)\approx 475 \left[{\mathrm{cm}}^{-1}\left], {\overline{A}}_4\left(R_0\right)\approx 225 \right[{\mathrm{cm}}^{-1}\left], {\overline{A}}_6\left(R_0\right)\approx 2 \right[{\mathrm{cm}}^{-1}\right]$$

(7)

Table 2 shows that the calculated and observed g factor for Ce³⁺ center in BaWO₄ single crystal are in quite a good agreement with each other, especially in the values of g parallel $g_{\left|\right|, calc}\approx g_{\left|\right|, exp}$. In the next step, we tried to enhancing the fit, by varying the polar angles ${\theta }_i$. The host metal–ligand polar angles ${\theta }_i$ (host–ligands) were replaced by polar angles ${\theta }_i^{\prime }$ (impurity–ligands) with small distortion: ${\theta }_i^{\prime }\approx {\theta }_i+\mathrm{\Delta }\theta$, $\mathrm{\Delta }\theta$ there is the local angular distortion. We obtained the angular distortion:

$$\mathrm{\Delta }\theta \approx {1.0}^{°}$$

(8)

It means that after decreasing the polar angles by one degree, we obtained the better fit to the experimental g-factors (Table 2, third row). The change is not so large, but visible. The difference in percentage between calculated and experimental values for the g perpendicular drop from 0.66% to 0.48% (see Table 2).

3. Discussion

The calculated g factors for above values of intrinsic parameters ${\overline{A}}_2\left(R_0\right), {\overline{A}}_4\left(R_0\right), {\overline{A}}_6\left(R_0\right)$ show good agreement with experimental values (see Table 2). However, the calculation based on the local distortion ${\theta }_i^{\prime }$ of the polar angels gives a slightly better fit to observed values of the g-factors. Hence, it is justifiable to say that the g-factor for tetragonal Ce³⁺ center in BaWO₄ single crystal is quite well explained with additional information about the local structure of the Ce³⁺ ion in dodecahedron [CeO₈]. There are several points that need further discussion.

First, the calculated intrinsic parameters fulfills inequality ${\overline{A}}_2\left(R_0\right) \gg {\overline{A}}_4\left(R_0\right)\gg {\overline{A}}_6\left(R_0\right)$, where ${\overline{A}}_2\left(R_0\right)$ is order 600 [cm⁻¹], and ${\overline{A}}_6\left(R_0\right)$ has a value of a few [cm⁻¹]. Wu Shao-Yi et al. set the values of intrinsic parameters ${\overline{A}}_2\left(R_0\right)\approx 400, {\overline{A}}_4\left(R_0\right)\approx 50, {\overline{A}}_6\left(R_0\right)\approx 17 \left[{\mathrm{cm}}^{-1}\right]$ for Er³⁺ centers in BaWO₄, CaWO₄, and SrWO₄ crystals [25,33]. H.G. Liu, W.C. Zheng and W.L. Feng calculated the intrinsic parameters ${\overline{A}}_2\left(R_0\right)\approx 290, {\overline{A}}_4\left(R_0\right)\approx 48, {\overline{A}}_6\left(R_0\right)\approx 45 \left[{\mathrm{cm}}^{-1}\right]$ from optical spectra for LuPO₄: Ce, and YPO₄: Ce single crystals with zircon structure [26]. We did not found other data regarding cerium ion, but there are many papers on rare-earth impurities, like Er³⁺, Yb³⁺, and Nd³⁺ in different oxide and fluoride crystals. The values of the ${\overline{A}}_2\left(R_0\right)$ (second rank) reach one thousand and more inverse centimeters (even ${\overline{A}}_2\left(R_0\right)\approx 1200 \left[{\mathrm{cm}}^{-1}\right]$ [37]), while sixth rank intrinsic parameter reach a few inverse centimeters (${\overline{A}}_6\left(R_0\right)\approx 3 \left[{\mathrm{cm}}^{-1}\right])$ [37,40,41]. Therefore, the calculated values of intrinsic parameters (rank second, fourth and sixth) should be considered as reasonable and contained within the range of data.

The values of g-factors ($g_{\left|\right|}$ and $g_{\perp }$) for Ce³⁺ in BaWO₄ single crystal, calculated and experimental are in good agreement (Table 2). For g parallel ($g_{\left|\right|}$), the fit is almost perfect. One can observe a small difference in the case of g perpendicular ($g_{\perp }$, about 0.66 %). This difference decreases with the variation of the host metal–ligand polar angles ${\theta }_i, i=1, 2$ with small distortion. The calculated angular distortion $\mathrm{\Delta }\theta \approx 1.0$° decrease a difference between experimental and calculated g perpendicular ($g_{\perp }$) to about 0.48 %. The calculated angular distortion for Ce³⁺ in BaWO₄ agrees with angular distortion for other rare-earth ions. For example: angular distortion calculated for Er³⁺ in BaWO₄ ($\mathrm{\Delta }\theta \approx {1.5}^{°}$) [25], Ce³⁺ in Y₃Al₅O₁₂ and Lu₃Al₅O₁₂ garnets ($\mathrm{\Delta }\theta \le {1.0}^{°}$) [27], Yb³⁺ in Na₃Sc₂V₃O₁₂ garnet ($\mathrm{\Delta }\theta \le {1.0}^{°}$) [29], Er³⁺ in PbMoO₄ and SrMoO₄ ($\mathrm{\Delta }\theta \approx {1.26}^{°},{1.05}^{°}$, respectively) [35]. It should be noticed that the angular distortion for Ce³⁺ in BaWO₄ were calculated previously using a simplified SPM model ($\mathrm{\Delta }\theta \approx {4.0}^{°}$) [20], from which follows that even a simplified SPM model can give a quite good angular distortion result useful for structural analysis. Our calculation shows that angular distortion of polar angles ${\theta }_i, i=1, 2$ gives only a partial approximation to experimental values. The better approximation will be obtained by changing the azimuthal angles (${\phi }_i, i=1, 2$), too. It means that oxygen ligands are not only coming slightly closer to a fourfold axis (Z or c axis), but oxygen tetrahedrons are also twisted (see Figure 2). Further calculations and structural analysis are required for further comprehension.

It should be pointed out that calculated intrinsic parameters were obtained only from the fitting procedure using SPM method to experimental EPR g-factors ($g_{\left|\right|}$ and $g_{\perp }$). These results should be considered as a first approximation and confirmed by fitting to results of the optical measurements. Unfortunately, we did not find the results of optical measurement of barium tungstate doped with cerium (BaWO₄: Ce³⁺). If optical bands of BaWO₄: Ce³⁺ will be recorded, our results could be a useful starting point. Our results could also be a useful help to structural analysis a local surroundings of the rare-earth ion (Ce³⁺), which substitute a Ba²⁺ site in oxygens dodecahedron [BaO₈]. It may be important in further application of BaWO₄: Ce³⁺ single crystals, for example in optical devices.