The Depth-Dependent Mechanical Behavior of Anisotropic Native and Cross-Linked HheG Enzyme Crystals
Abstract
:1. Introduction
2. Materials and Methods
2.1. Crystallization and Cross-Linking
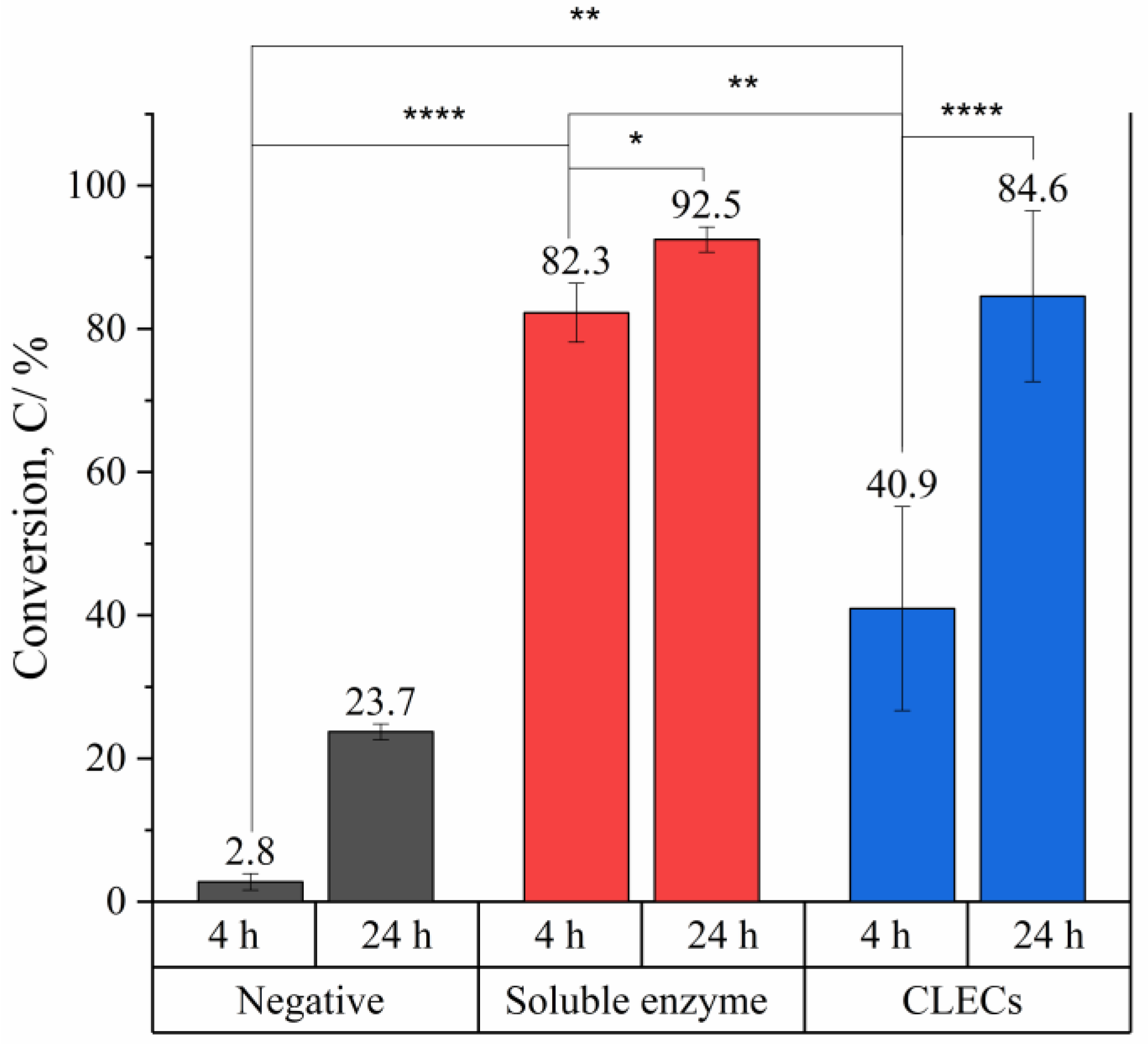
2.2. Bioconversion of Cyclohexene Oxide Using HheG CLECs
2.3. Mechanical Analysis on Single Protein Crystals
3. Results and Discussion
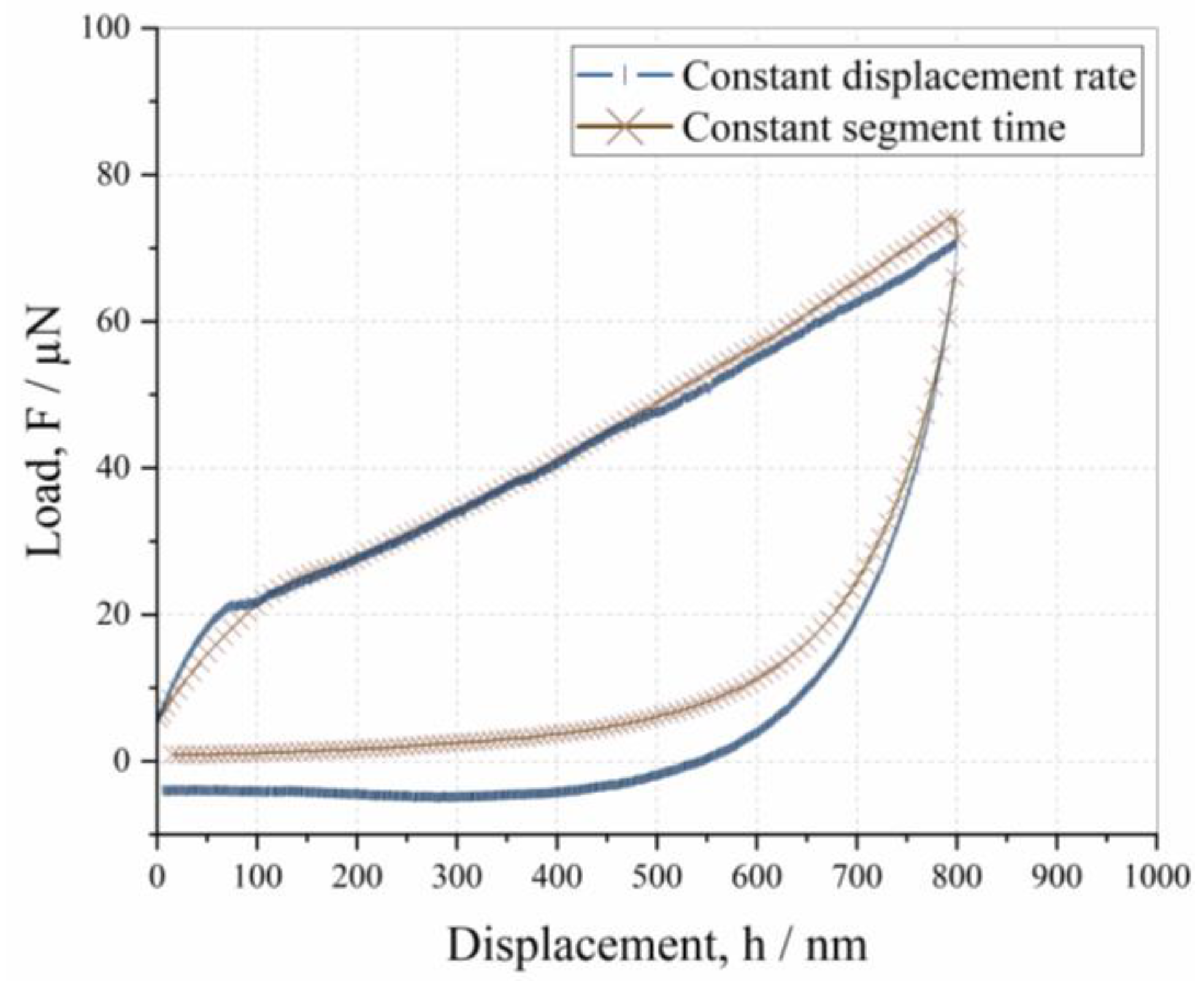
3.1. Influence of Displacement Rate on Depth-Dependent Mechanical Response
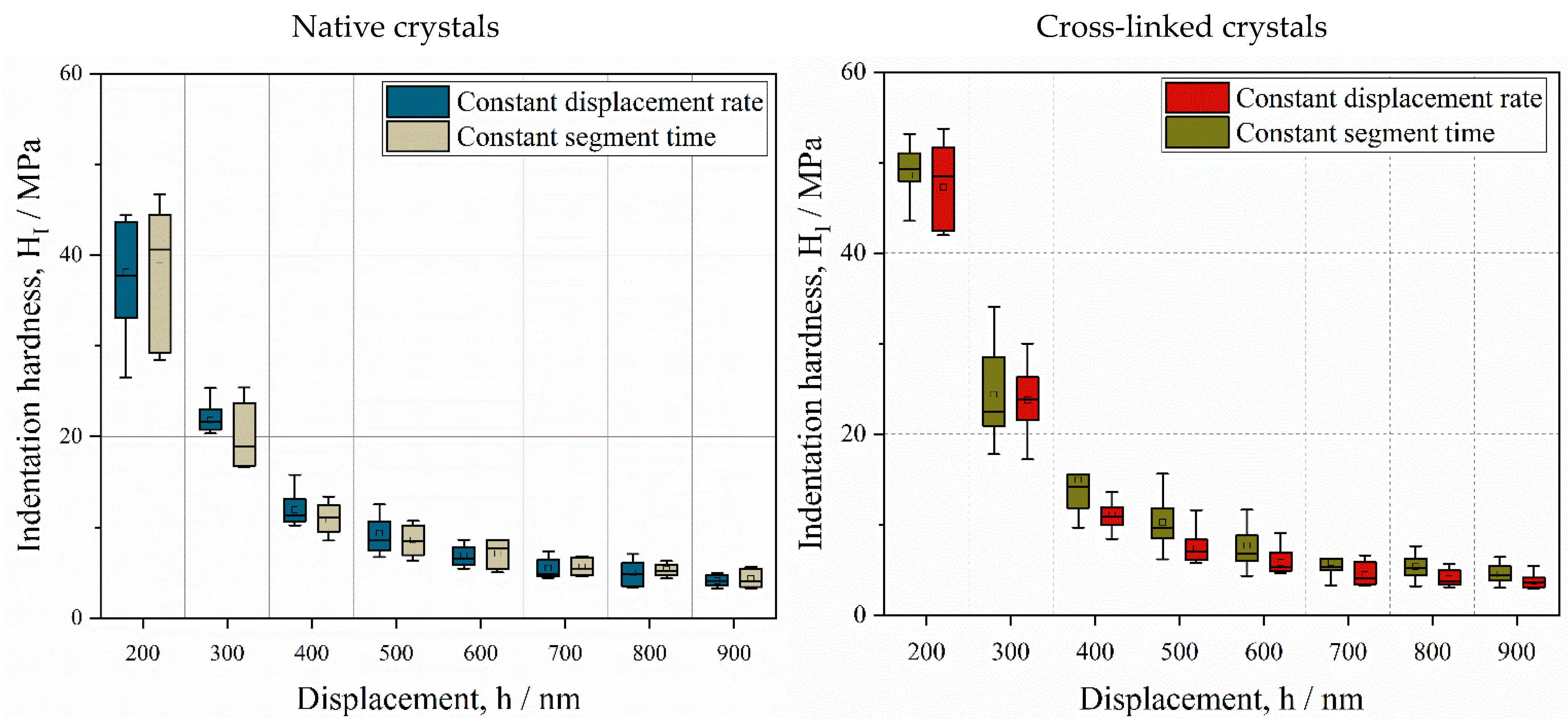
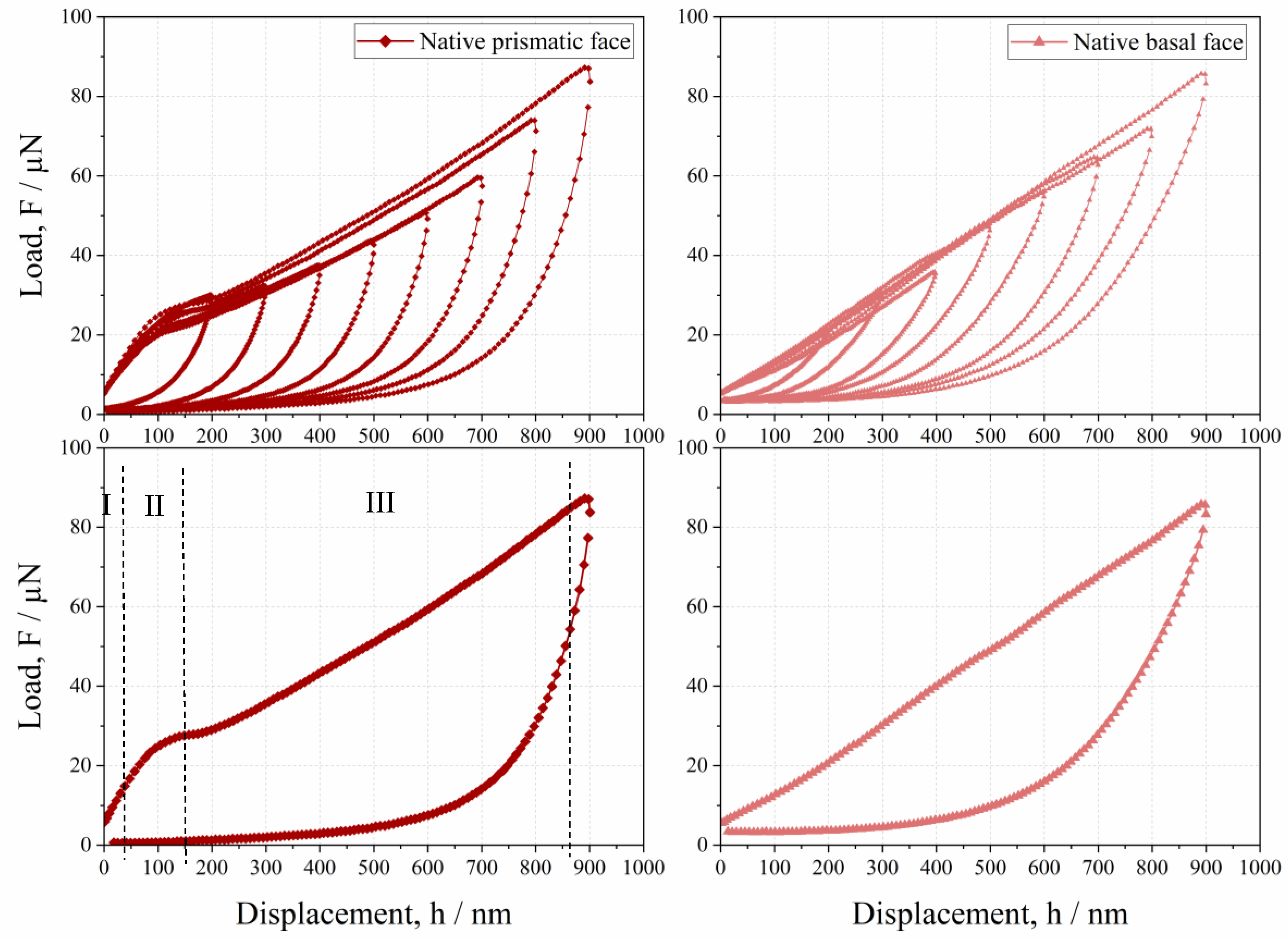
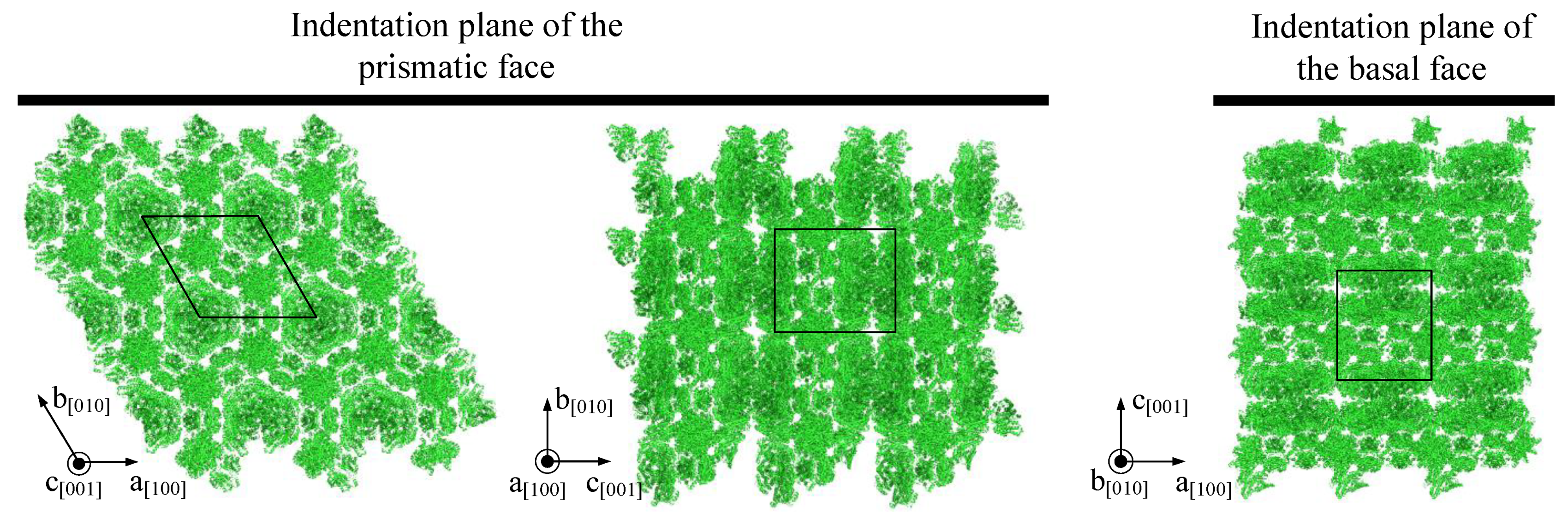
3.2. Anisotropic Behavior of Native HheG Crystals
3.3. Mechanical and Catalytic Properties of Cross-Linked HheG Crystals
4. Conclusions
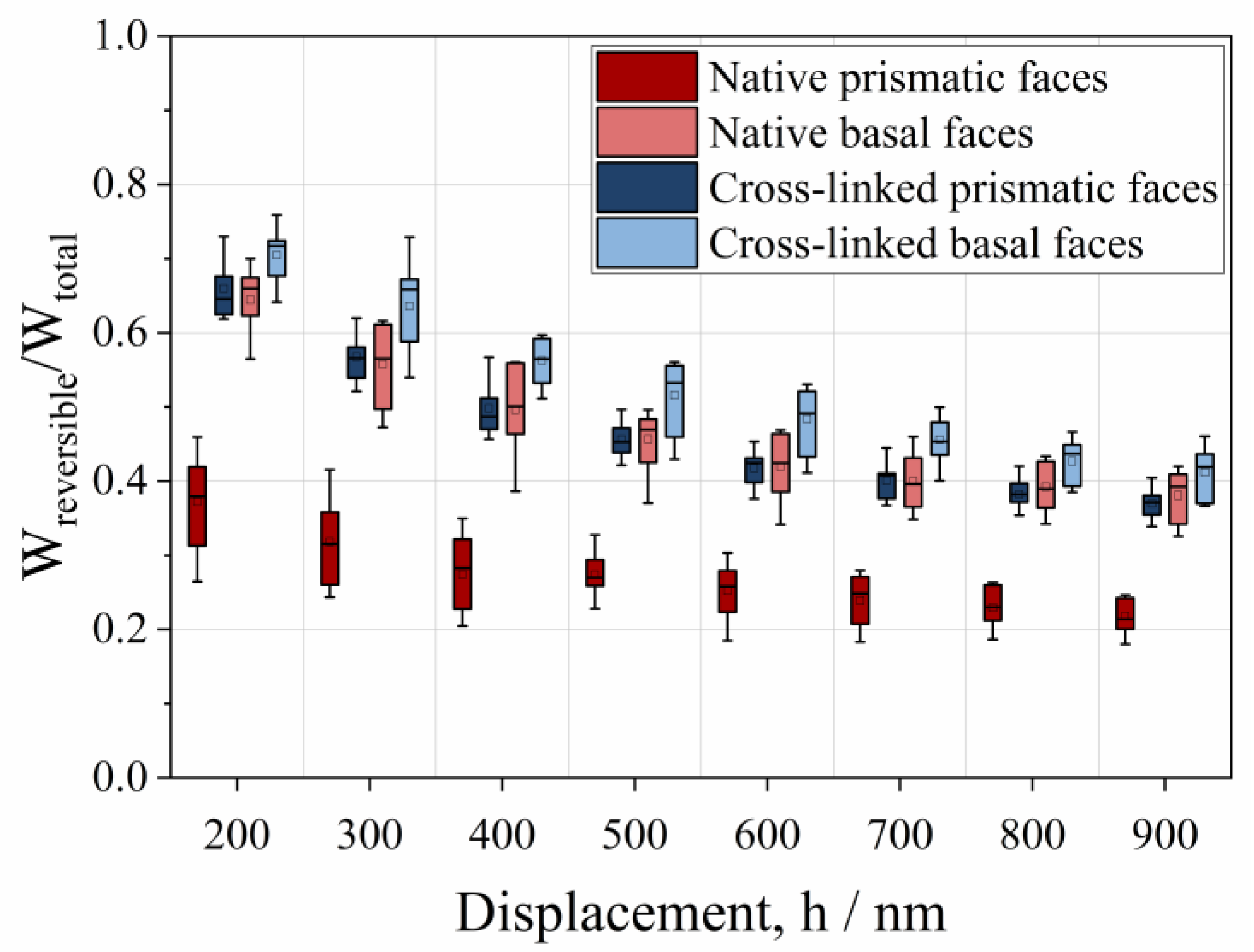
- The mechanical properties of native and cross-linked enzyme crystals, such as hardness and the fractions of elastic and plastic energy, decrease with an increase in penetration depth, meaning that they exhibit an indentation size effect (ISE).
- The mechanical properties are not dependent on the indentation rate, but the tendency toward adhesion increases at a slower displacement rate.
- Native crystals show anisotropic mechanical behaviors, where the basal face is more elastic than the prismatic one.
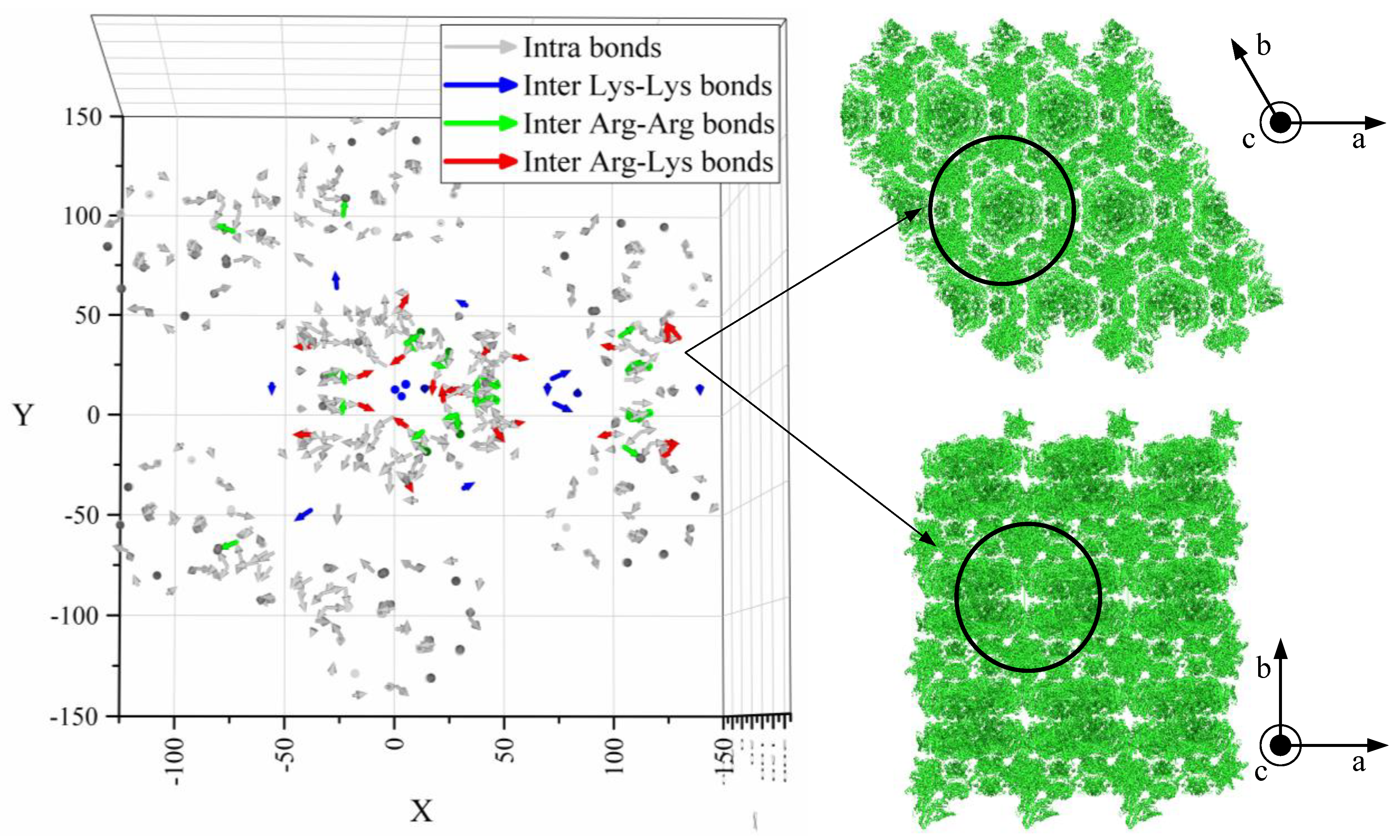
- The lower resistance of prismatic faces against plastic deformation seems to be caused by anisotropic crystallographic planes and intermolecular water.
- Cross-linking increases the fraction of the reversible indentation energy of prismatic and basal faces by approximately 68% and 8%, respectively.
- HheG CLECs cross-linked with glutaraldehyde showed high catalytic activity under a conversion time of 24 h. Due to diffusion limitations, a longer reaction time was required to complete the reaction compared to that when using a soluble enzyme.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Schallmey, A.; Schallmey, M. Recent advances on halohydrin dehalogenases-from enzyme identification to novel biocatalytic applications. Appl. Microbiol. Biotechnol. 2016, 100, 7827–7839. [Google Scholar] [CrossRef] [Green Version]
- Hasnaoui-Dijoux, G.; Majerić Elenkov, M.; Lutje Spelberg, J.H.; Hauer, B.; Janssen, D.B. Catalytic promiscuity of halohydrin dehalogenase and its application in enantioselective epoxide ring opening. ChemBioChem 2008, 9, 1048–1051. [Google Scholar] [CrossRef] [PubMed]
- Koopmeiners, J.; Halmschlag, B.; Schallmey, M.; Schallmey, A. Biochemical and biocatalytic characterization of 17 novel halohydrin dehalogenases. Appl. Microbiol. Biotechnol. 2016, 100, 7517–7527. [Google Scholar] [CrossRef] [PubMed]
- Koopmeiners, J.; Diederich, C.; Solarczek, J.; Voß, H.; Mayer, J.; Blankenfeldt, W.; Schallmey, A. HheG, a Halohydrin Dehalogenase with Activity on Cyclic Epoxides. ACS Catal. 2017, 7, 6877–6886. [Google Scholar] [CrossRef]
- Calderini, E.; Wessel, J.; Süss, P.; Schrepfer, P.; Wardenga, R.; Schallmey, A. Selective Ring-Opening of Di-Substituted Epoxides Catalysed by Halohydrin Dehalogenases. ChemCatChem 2019, 11, 2099–2106. [Google Scholar] [CrossRef]
- An, M.; Liu, W.; Zhou, X.; Ma, R.; Wang, H.; Cui, B.; Han, W.; Wan, N.; Chen, Y. Highly α-position regioselective ring-opening of epoxides catalyzed by halohydrin dehalogenase from Ilumatobacter coccineus: A biocatalytic approach to 2-azido-2-aryl-1-ols. RSC Adv. 2019, 9, 16418–16422. [Google Scholar] [CrossRef] [Green Version]
- Sternberg, J.A.; Geffken, D.; Adams, J.B.; Pstages, R.; Sternberg, C.G.; Campbell, C.L.; Moberg, W.K. Famoxadone: The discovery and optimisation of a new agricultural fungicide. Pest. Manag. Sci. 2001, 57, 143–152. [Google Scholar] [CrossRef]
- Swaney, S.M.; Aoki, H.; Ganoza, M.C.; Shinabarger, D.L. The oxazolidinone linezolid inhibits initiation of protein synthesis in bacteria. Antimicrob. Agents Chemother. 1998, 42, 3251–3255. [Google Scholar] [CrossRef] [Green Version]
- Heravi, M.M.; Zadsirjan, V.; Farajpour, B. Applications of oxazolidinones as chiral auxiliaries in the asymmetric alkylation reaction applied to total synthesis. RSC Adv. 2016, 6, 30498–30551. [Google Scholar] [CrossRef]
- Tischer, W.; Kasche, V. Immobilized enzymes: Crystals or carriers? Trends Biotechnol. 1999, 17, 326–335. [Google Scholar] [CrossRef]
- Guo, S.; Akhremitchev, B.B. Investigation of mechanical properties of insulin crystals by atomic force microscopy. Langmuir 2008, 24, 880–887. [Google Scholar] [CrossRef]
- Tait, S.; White, E.T.; Litster, J.D. Mechanical Characterization of Protein Crystals. Part. Part. Syst. Charact. 2008, 25, 266–276. [Google Scholar] [CrossRef]
- Koizumi, H.; Kawamoto, H.; Tachibana, M.; Kojima, K. Effect of intracrystalline water on micro-Vickers hardness in tetragonal hen egg-white lysozyme single crystals. J. Phys. D Appl. Phys. 2008, 41, 74019. [Google Scholar] [CrossRef]
- Tachibana, M.; Kobayashi, Y.; Shimazu, T.; Ataka, M.; Kojima, K. Growth and mechanical properties of lysozyme crystals. J. Cryst. Growth 1999, 198–199, 661–664. [Google Scholar] [CrossRef]
- Kishi, T.; Suzuki, R.; Shigemoto, C.; Murata, H.; Kojima, K.; Tachibana, M. Microindentation Hardness of Protein Crystals under Controlled Relative Humidity. Crystals 2017, 7, 339. [Google Scholar] [CrossRef] [Green Version]
- Zamiri, A.; De, S. Modeling the mechanical response of tetragonal lysozyme crystals. Langmuir 2010, 26, 4251–4257. [Google Scholar] [CrossRef] [Green Version]
- Koizumi, H.; Tachibana, M.; Kawamoto, H.; Kojima, K. Temperature dependence of microhardness of tetragonal hen-egg-white lysozyme single crystals. Philos. Mag. 2004, 84, 2961–2968. [Google Scholar] [CrossRef]
- Rupp, B. Biomolecular Crystallography: Principles, Practice, and Application to Structural Biology; Garland Science: New York, NY, USA, 2010; ISBN 978-0815340812. [Google Scholar]
- Yan, E.-K.; Lu, Q.-Q.; Zhang, C.-Y.; Liu, Y.-L.; He, J.; Chen, D.; Wang, B.; Zhou, R.-B.; Wu, P.; Yin, D.-C. Preparation of cross-linked hen-egg white lysozyme crystals free of cracks. Sci. Rep. 2016, 6, 34770. [Google Scholar] [CrossRef] [Green Version]
- Zelinski, T.; Waldmann, H. Cross-Linked Enzyme Crystals (CLECs): Efficient and Stable Biocatalysts for Preparative Organic Chemistry. Angew. Chem. Int. Ed. Engl. 1997, 36, 722–724. [Google Scholar] [CrossRef]
- St Clair, N.L.; Navia, M.A. Cross-linked enzyme crystals as robust biocatalysts. J. Am. Chem. Soc. 1992, 114, 7314–7316. [Google Scholar] [CrossRef]
- Quiocho, F.A.; Richards, F.M. Intermolecular Cross Linking of a Protein in the Crystalline State: Carboxypeptidase-A. Proc. Natl. Acad. Sci. USA 1964, 52, 833–839. [Google Scholar] [CrossRef] [Green Version]
- Govardhan, C.P. Crosslinking of enzymes for improved stability and performance. Curr. Opin. Biotechnol. 1999, 10, 331–335. [Google Scholar] [CrossRef]
- Jegan Roy, J.; Emilia Abraham, T. Strategies in making cross-linked enzyme crystals. Chem. Rev. 2004, 104, 3705–3722. [Google Scholar] [CrossRef]
- Yan, E.-K.; Cao, H.-L.; Zhang, C.-Y.; Lu, Q.-Q.; Ye, Y.-J.; He, J.; Huang, L.-J.; Yin, D.-C. Cross-linked protein crystals by glutaraldehyde and their applications. RSC Adv. 2015, 5, 26163–26174. [Google Scholar] [CrossRef]
- Barbosa, O.; Ortiz, C.; Berenguer-Murcia, Á.; Torres, R.; Rodrigues, R.C.; Fernandez-Lafuente, R. Glutaraldehyde in bio-catalysts design: A useful crosslinker and a versatile tool in enzyme immobilization. RSC Adv. 2014, 4, 1583–1600. [Google Scholar] [CrossRef] [Green Version]
- Morozov, V.N.; Morozova, T.Y. Viscoelastic properties of protein crystals: Triclinic crystals of hen egg white lysozyme in different conditions. Biopolymers 1981, 20, 451–467. [Google Scholar] [CrossRef]
- Lee, T.S.; Turner, M.K.; Lye, G.J. Mechanical stability of immobilized biocatalysts (CLECs) in dilute agitated suspensions. Biotechnol. Prog. 2002, 18, 43–50. [Google Scholar] [CrossRef]
- Kubiak, M.; Solarczek, J.; Kampen, I.; Schallmey, A.; Kwade, A.; Schilde, C. Micromechanics of Anisotropic Cross-Linked Enzyme Crystals. Cryst. Growth Des. 2018, 18, 5885–5895. [Google Scholar] [CrossRef]
- Kubiak, M.; Mayer, J.; Kampen, I.; Schilde, C.; Biedendieck, R. Structure-Properties Correlation of Cross-Linked Penicillin G Acylase Crystals. Crystals 2021, 11, 451. [Google Scholar] [CrossRef]
- Kubiak, M.; Storm, K.-F.; Kampen, I.; Schilde, C. Relationship between Cross-Linking Reaction Time and Anisotropic Mechanical Behavior of Enzyme Crystals. Cryst. Growth Des. 2019, 19, 4453–4464. [Google Scholar] [CrossRef]
- Margolin, A.L. Novel crystalline catalysts. Trends Biotechnol. 1996, 14, 223–230. [Google Scholar] [CrossRef]
- Oyen, M.L.; Cook, R.F. A practical guide for analysis of nanoindentation data. J. Mech. Behav. Biomed. Mater. 2009, 2, 396–407. [Google Scholar] [CrossRef] [PubMed]
- Lusty, C.J. A gentle vapor-diffusion technique for cross-linking of protein crystals for cryocrystallography. J. Appl. Crystallogr. 1999, 32, 106–112. [Google Scholar] [CrossRef] [Green Version]
- López-Jaramillo, F.J.; Moraleda, A.B.; González-Ramírez, L.A.; Carazo, A.; García-Ruiz, J.M. Soaking: The effect of osmotic shock on tetragonal lysozyme crystals. Acta Crystallogr. Sect. D Struct. Biol. 2002, 58, 209–214. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Monsan, P.; Puzo, G.; Mazarguil, H. Étude du mécanisme d’établissement des liaisons glutaraldéhyde-protéines. Biochimie 1976, 57, 1281–1292. [Google Scholar] [CrossRef]
- Solarczek, J.; Klünemann, T.; Brandt, F.; Schrepfer, P.; Wolter, M.; Jacob, C.R.; Blankenfeldt, W.; Schallmey, A. Position 123 of halohydrin dehalogenase HheG plays an important role in stability, activity, and enantioselectivity. Sci. Rep. 2019, 9, 5106. [Google Scholar] [CrossRef] [PubMed]
- Oliver, W.C.; Pharr, G.M. Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 2004, 19, 3–20. [Google Scholar] [CrossRef]
- Schilde, C.; Burmeister, C.F.; Kwade, A. Measurement and simulation of micromechanical properties of nanostructured aggregates via nanoindentation and DEM-simulation. Powder Technol. 2014, 259, 1–13. [Google Scholar] [CrossRef]
- Morozov, V.N.; Morozova, T.Y.; Myachin, E.G.; Kachalova, G.S. Metastable state of a protein crystal. Acta Crystallogr. B Struct. Sci. 1985, 41, 202–205. [Google Scholar] [CrossRef]
- Nix, W.D.; Gao, H. Indentation size effects in crystalline materials: A law for strain gradient plasticity. J. Mech. Phys. Solids 1998, 46, 411–425. [Google Scholar] [CrossRef]
- Suzuki, R.; Shigemoto, C.; Abe, M.; Kojima, K.; Tachibana, M. Analysis of slip systems in protein crystals with a triclinic form using a phenomenological macro-bond method. CrystEngComm 2021, 23, 3753–3760. [Google Scholar] [CrossRef]
- Suzuki, R.; Kishi, T.; Tsukashima, S.; Tachibana, M.; Wako, K.; Kojima, K. Hardness and slip systems of orthorhombic hen egg-white lysozyme crystals. Philos. Mag. 2016, 96, 2930–2942. [Google Scholar] [CrossRef]
- Aibara, S.; Suzuki, A.; Kidera, A.; Shibata, K.; Yamane, T.; DeLucas, L.J.; Hirose, M. The Crystal Structure of the Orthorhombic Form of Hen Egg White Lysozyme at 1.5 Angstroms Resolution. 2004. Available online: https://www.rcsb.org/structure/1VDQ (accessed on 22 June 2021).
- Swadener, J.G.; George, E.P.; Pharr, G.M. The correlation of the indentation size effect measured with indenters of various shapes. J. Mech. Phys. Solids 2002, 50, 681–694. [Google Scholar] [CrossRef]
- Kucharski, S.; Woźniacka, S. Size Effect in Single Crystal Copper Examined with Spherical Indenters. Metall. Mater. Trans. A 2019, 50, 2139–2154. [Google Scholar] [CrossRef] [Green Version]
- Raut, D.; Kiran, M.S.R.N.; Mishra, M.K.; Asiri, A.M.; Ramamurty, U. On the loading rate sensitivity of plastic deformation in molecular crystals. CrystEngComm 2016, 18, 3551–3555. [Google Scholar] [CrossRef]
- Buehler, M.J.; Ackbarow, T. Fracture mechanics of protein materials. Mater. Today 2007, 10, 46–58. [Google Scholar] [CrossRef]
- Labonte, D.; Lenz, A.-K.; Oyen, M.L. On the relationship between indenation hardness and modulus, and the damage resistance of biological materials. Acta Biomater. 2017, 57, 373–383. [Google Scholar] [CrossRef]
- Edwards, A.J.; Mackenzie, C.F.; Spackman, P.R.; Jayatilaka, D.; Spackman, M.A. Intermolecular interactions in molecular crystals: What’s in a name? Faraday Discuss. 2017, 203, 93–112. [Google Scholar] [CrossRef] [Green Version]
- Wang, C.; Sun, C.C. The landscape of mechanical properties of molecular crystals. CrystEngComm 2020, 22, 1149–1153. [Google Scholar] [CrossRef]
- Reddy, C.M.; Rama Krishna, G.; Ghosh, S. Mechanical properties of molecular crystals—applications to crystal engineering. CrystEngComm 2010, 12, 2296. [Google Scholar] [CrossRef]
- Dunitz, J.D. Weak Interactions in Molecular Crystals. In Implications of Molecular and Materials Structure for New Technologies; Howard, J.A.K., Allen, F.H., Shields, G.P., Eds.; Springer: Dordrecht, The Netherlands, 1999; pp. 175–184. ISBN 978-0-7923-5817-6. [Google Scholar]
- Buehler, M.J. Atomistic modeling of elasticity, plasticity and fracture of protein crystals. MRS Proc. 2005, 898, 1003. [Google Scholar] [CrossRef]
- Taylor, G.I. The mechanism of plastic deformation of crystals. Part I.—Theoretical: Geoffrey Ingram Taylor. Proc. R. Soc. Lond. A 1934, 145, 362–387. [Google Scholar] [CrossRef]
- Taylor, G.I. The mechanism of plastic deformation of crystals. Part II.—Comparison with observations. Proc. R. Soc. Lond. A 1934, 145, 388–404. [Google Scholar] [CrossRef]
- Suzuki, R.; Tachibana, M.; Koizumi, H.; Kojima, K. Direct observation of stress-induced dislocations in protein crystals by synchrotron X-ray topography. Acta Mater. 2018, 156, 479–485. [Google Scholar] [CrossRef]
- Xia, W.; Dehm, G.; Brinckmann, S. Insight into indentation-induced plastic flow in austenitic stainless steel. J. Mater. Sci. 2020, 55, 9095–9108. [Google Scholar] [CrossRef]
- Nanev, C.N. Brittleness of protein crystals. Cryst. Res. Technol. 2012, 47, 922–927. [Google Scholar] [CrossRef]
- Margolin, A.L.; Navia, M.A. Protein Crystals as Novel Catalytic Materials. Angew. Chem. Int. Ed. Engl. 2001, 40, 2204–2222. [Google Scholar] [CrossRef]
- DeSantis, G.; Jones, J.B. Chemical modification of enzymes for enhanced functionality. Curr. Opin. Biotechnol. 1999, 10, 324–330. [Google Scholar] [CrossRef]
- Wong, S.S.; Wong, L.-J.C. Chemical crosslinking and the stabilization of proteins and enzymes. Enzym. Microb. Technol. 1992, 14, 866–874. [Google Scholar] [CrossRef]
- Makinen, M.W.; Fink, A.L. Reactivity and cryoenzymology of enzymes in the crystalline state. Annu. Rev. Biophys. Bioeng. 1977, 6, 301–343. [Google Scholar] [CrossRef]
- Westbrook, E.M.; Sigler, P.B. Enzymatic function in crystals of delta 5-3-ketosteroid isomerase. Catalytic activity and binding of competitive inhibitors. J. Biol. Chem. 1984, 259, 9090–9095. [Google Scholar] [CrossRef]
- Kasvinsky, P.J.; Madsen, N.B. Activity of glycogen phosphorylase in the crystalline state. J. Biol. Chem. 1976, 251, 6852–6859. [Google Scholar] [CrossRef]
- Spilburg, C.A.; Bethune, J.L.; Valee, B.L. Kinetic properties of crystalline enzymes. Carboxypeptidase A. Biochemistry 1977, 16, 1142–1150. [Google Scholar] [CrossRef]
- Alter, G.M.; Leussing, D.L.; Neurath, H.; Vallee, B.L. Kinetic properties of carboxypeptidase B in solutions and crystals. Biochemistry 1977, 16, 3663–3668. [Google Scholar] [CrossRef]
- Migneault, I.; Dartiguenave, C.; Bertrand, M.J.; Waldron, K.C. Glutaraldehyde: Behavior in aqueous solution, reaction with proteins, and application to enzyme crosslinking. BioTechniques 2004, 37, 790–802. [Google Scholar] [CrossRef]


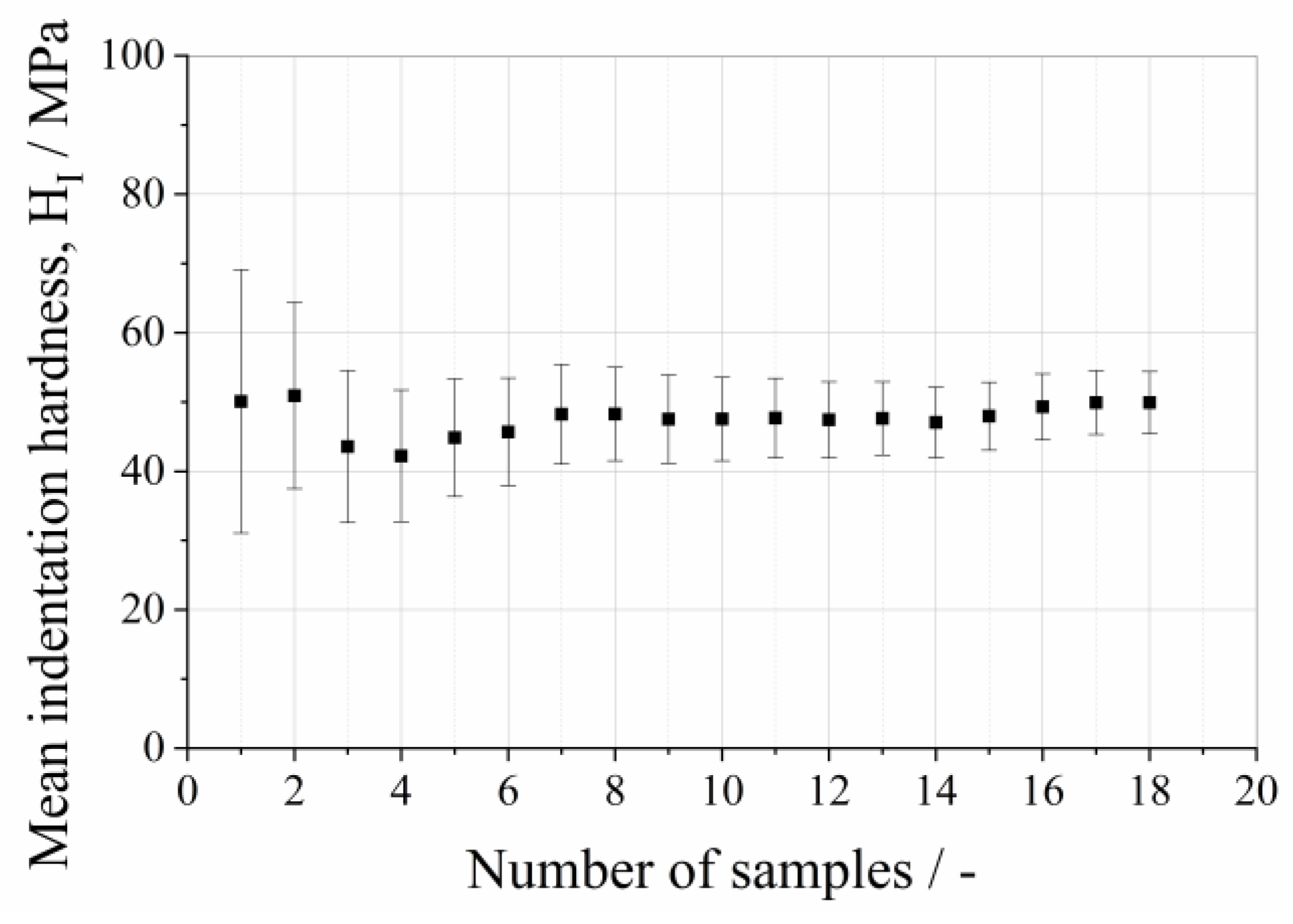



| Enzyme Crystal | Specifics | Measuring Method | Hardness/MPa | Literature |
|---|---|---|---|---|
| Lysozyme | Native, wet triclinic crystals | Micro-Vickers hardness | 25–28 | [42] |
| Lysozyme | Native, wet orthorombic crystals | Micro-Vickers hardness | 6–10 | [43] |
| Lysozyme | Native, wet tetragonal crystals | Nanoindenter | 15 | [12] |
| Lyzozyme | Cross-linked tetragonal crystals in liquid | AFM Nanoindentation | 11 | [29] |
| HheG | Cross-linked prismatic crystal faces | AFM Nanoindentation | 8 | [31] |
| Crystal Face | Arg–Arg | Arg–Lys | Lys–Lys | Sum |
|---|---|---|---|---|
| Surf 1 | 271.77 | 142 | 145.4 | 559.17 |
| Surf 2 | 231.63 | 137.81 | 107.04 | 476.48 |
| Surf 3 | 230.52 | 140.01 | 105.47 | 476.00 |
| Surf 4 | 230.49 | 141.84 | 107.97 | 480.30 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kubiak, M.; Staar, M.; Kampen, I.; Schallmey, A.; Schilde, C. The Depth-Dependent Mechanical Behavior of Anisotropic Native and Cross-Linked HheG Enzyme Crystals. Crystals 2021, 11, 718. https://doi.org/10.3390/cryst11070718
Kubiak M, Staar M, Kampen I, Schallmey A, Schilde C. The Depth-Dependent Mechanical Behavior of Anisotropic Native and Cross-Linked HheG Enzyme Crystals. Crystals. 2021; 11(7):718. https://doi.org/10.3390/cryst11070718
Chicago/Turabian StyleKubiak, Marta, Marcel Staar, Ingo Kampen, Anett Schallmey, and Carsten Schilde. 2021. "The Depth-Dependent Mechanical Behavior of Anisotropic Native and Cross-Linked HheG Enzyme Crystals" Crystals 11, no. 7: 718. https://doi.org/10.3390/cryst11070718
APA StyleKubiak, M., Staar, M., Kampen, I., Schallmey, A., & Schilde, C. (2021). The Depth-Dependent Mechanical Behavior of Anisotropic Native and Cross-Linked HheG Enzyme Crystals. Crystals, 11(7), 718. https://doi.org/10.3390/cryst11070718







