Modeling and Reconstruction Strategy for Compton Scattering Tomography with Scintillation Crystals
Abstract
1. Introduction
- Good energy resolution;
- High density, high atomic number which enhances the probability for photoelectric absorption;
- No intrinsic activity in the measurement range (355 keV, 1332 keV) which would create a disturbance in the measured distribution;
- Small size and compactness.
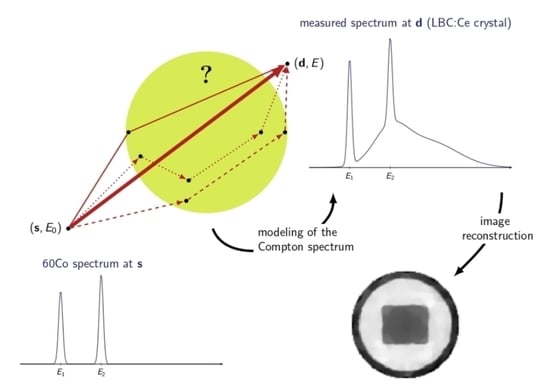
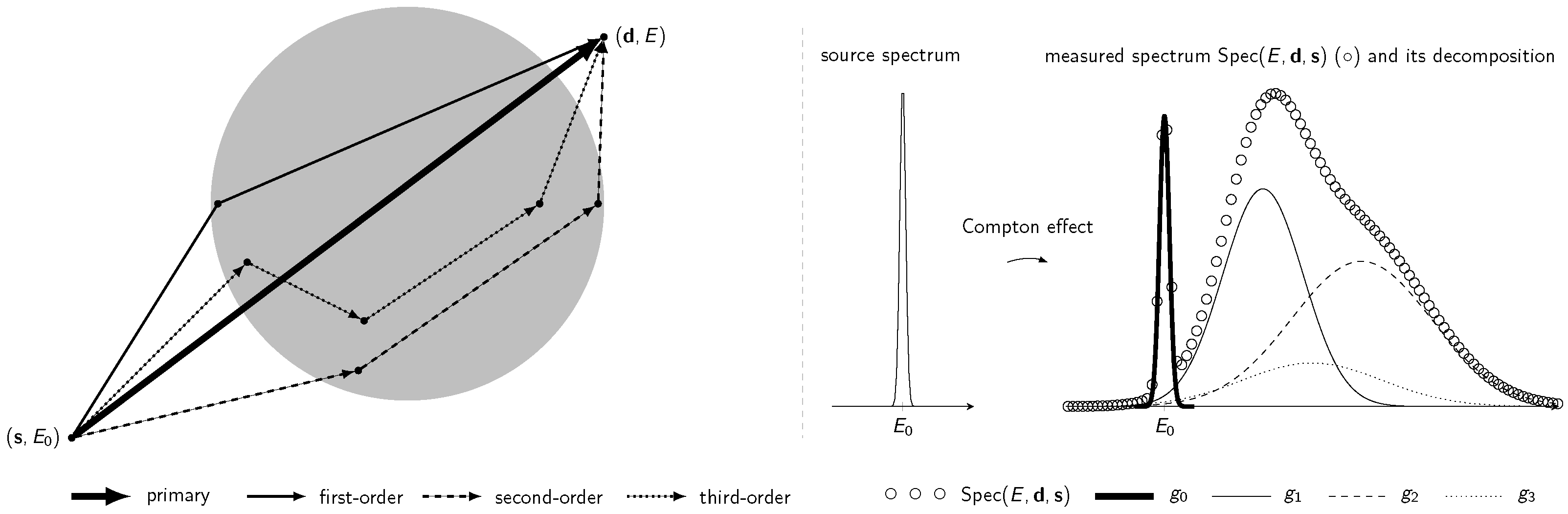
2. Modeling and Processing of the Compton Scattered Data
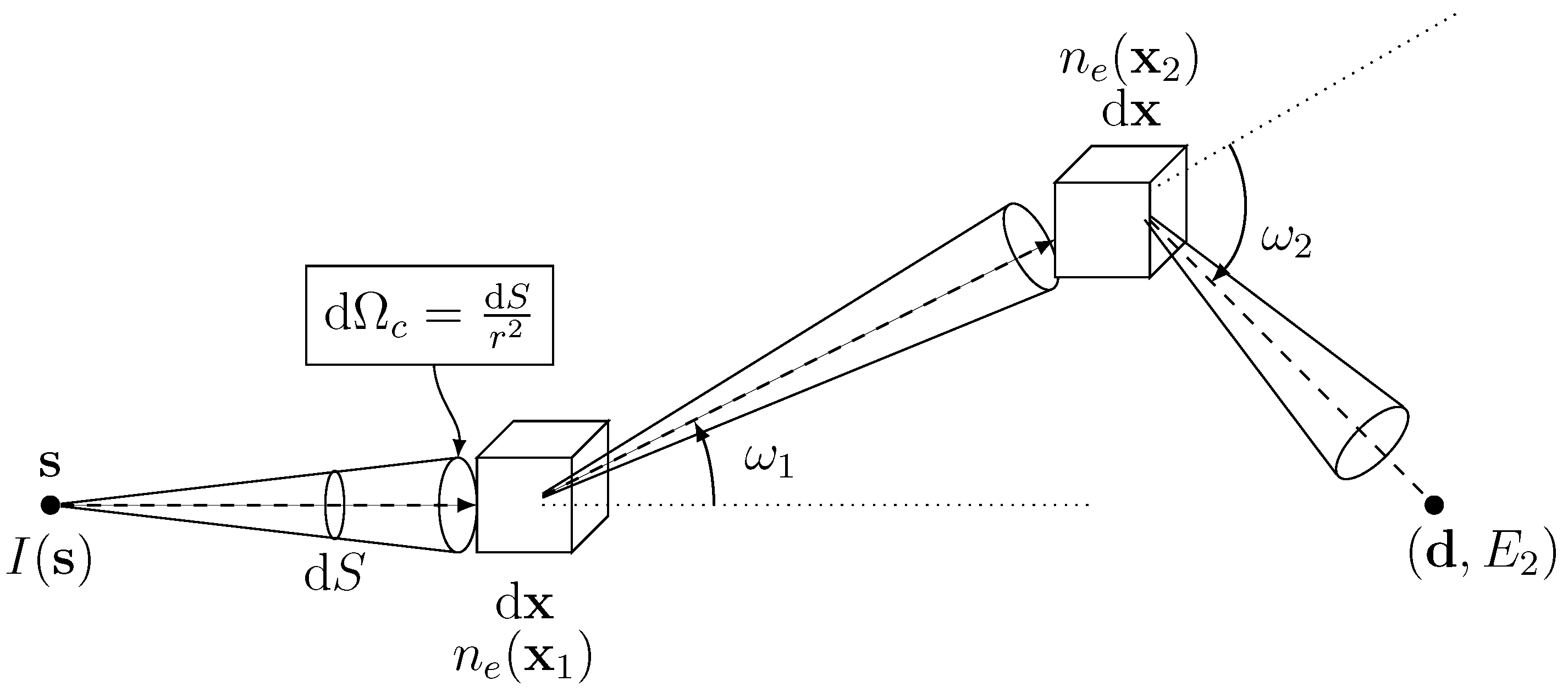
2.1. The Scattered Flux
- The photon beam is emitted by the point source in a differential solid angle with an energy ;
- Photons may be absorbed or scattered along the path following the Beer–Lambert law (Equation (1)) leading to the attenuation of the beam by the weight ;
- A part of the beam may collide with an electron at position which belongs to a differential volume ;
- Due to Compton scattering, the photon is scattered by an angle within a solid angle which follows the Klein–Nishina probability ([40]);
- Again the scattered beam is attenuated by a weight due to absorption or scattering of higher order;
- The attenuated and scattered beam finally reaches the point detector at .
2.2. Extension to Polychromatic -ray Sources
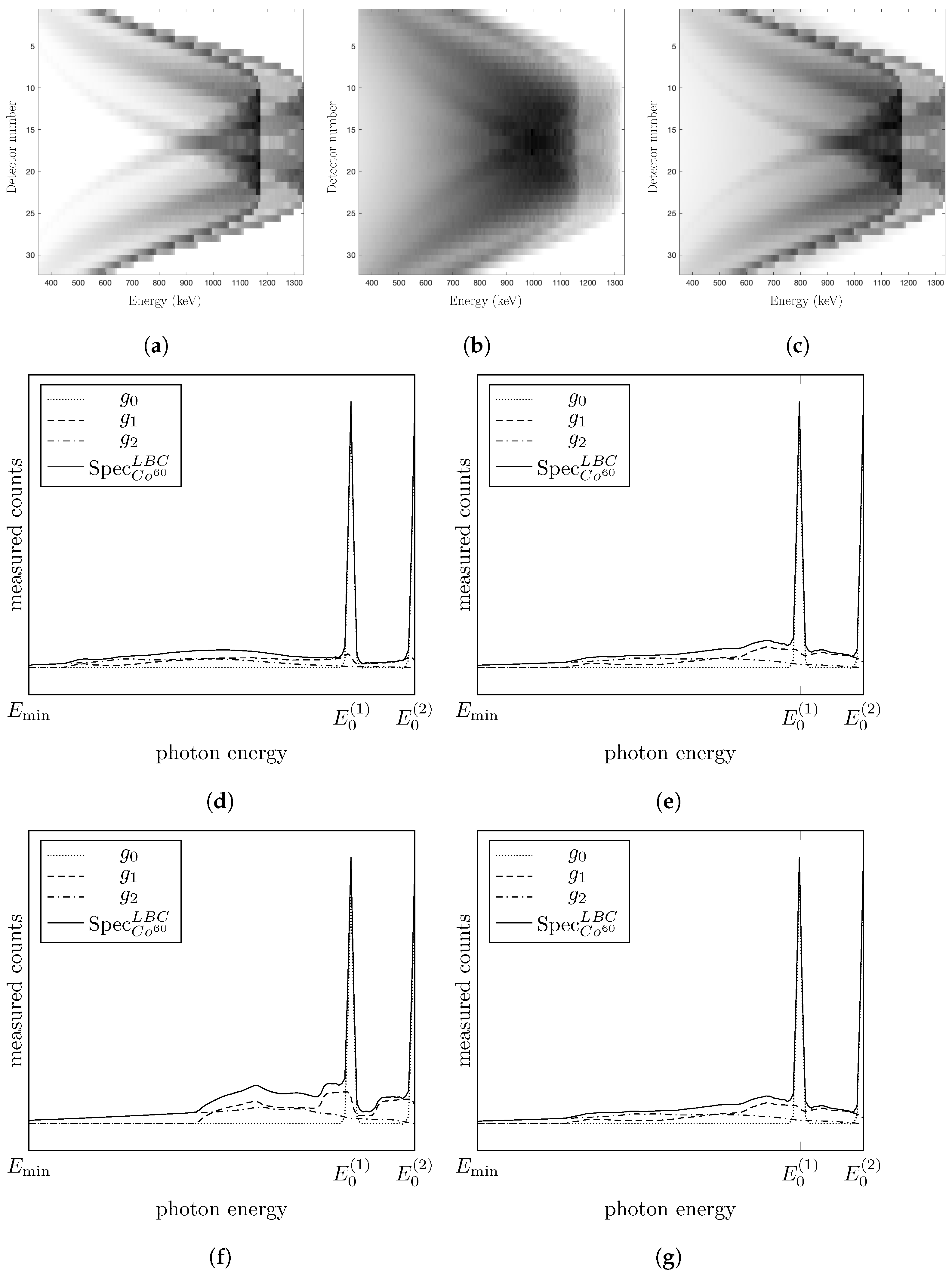
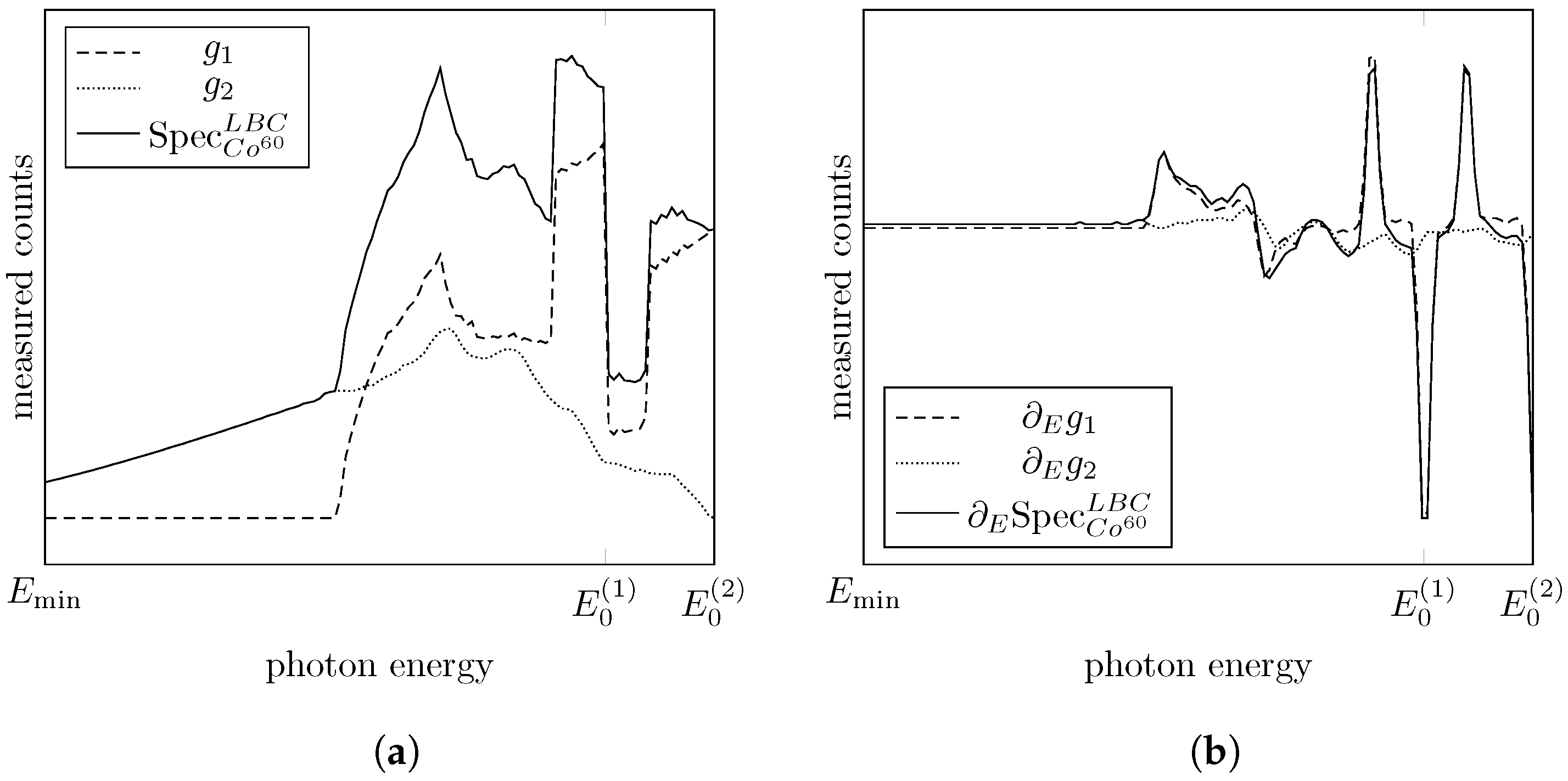
2.3. Modeling the Impact of the Scintillation Crystals
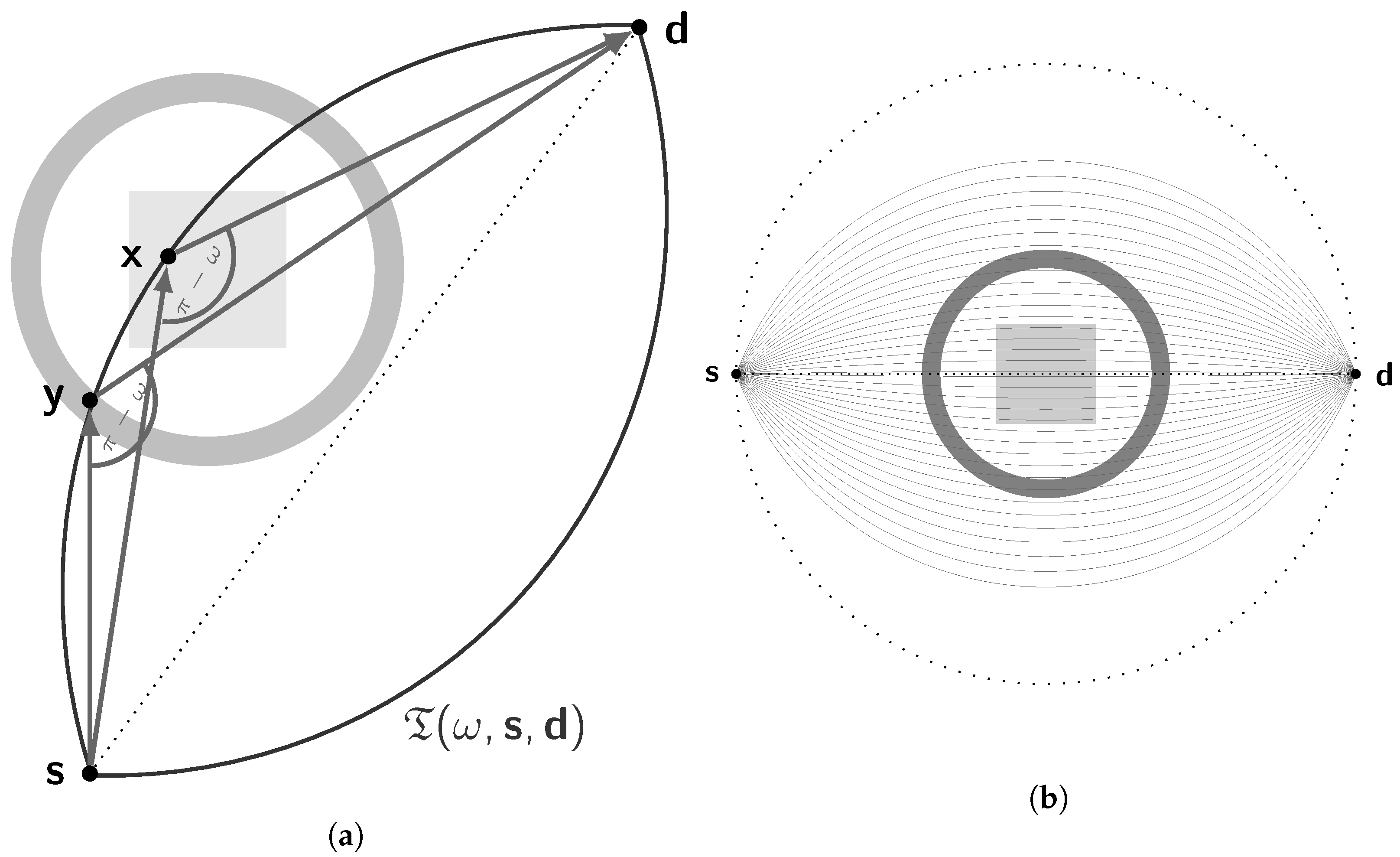
2.4. A General Approach for the Reconstruction of the Electron Density
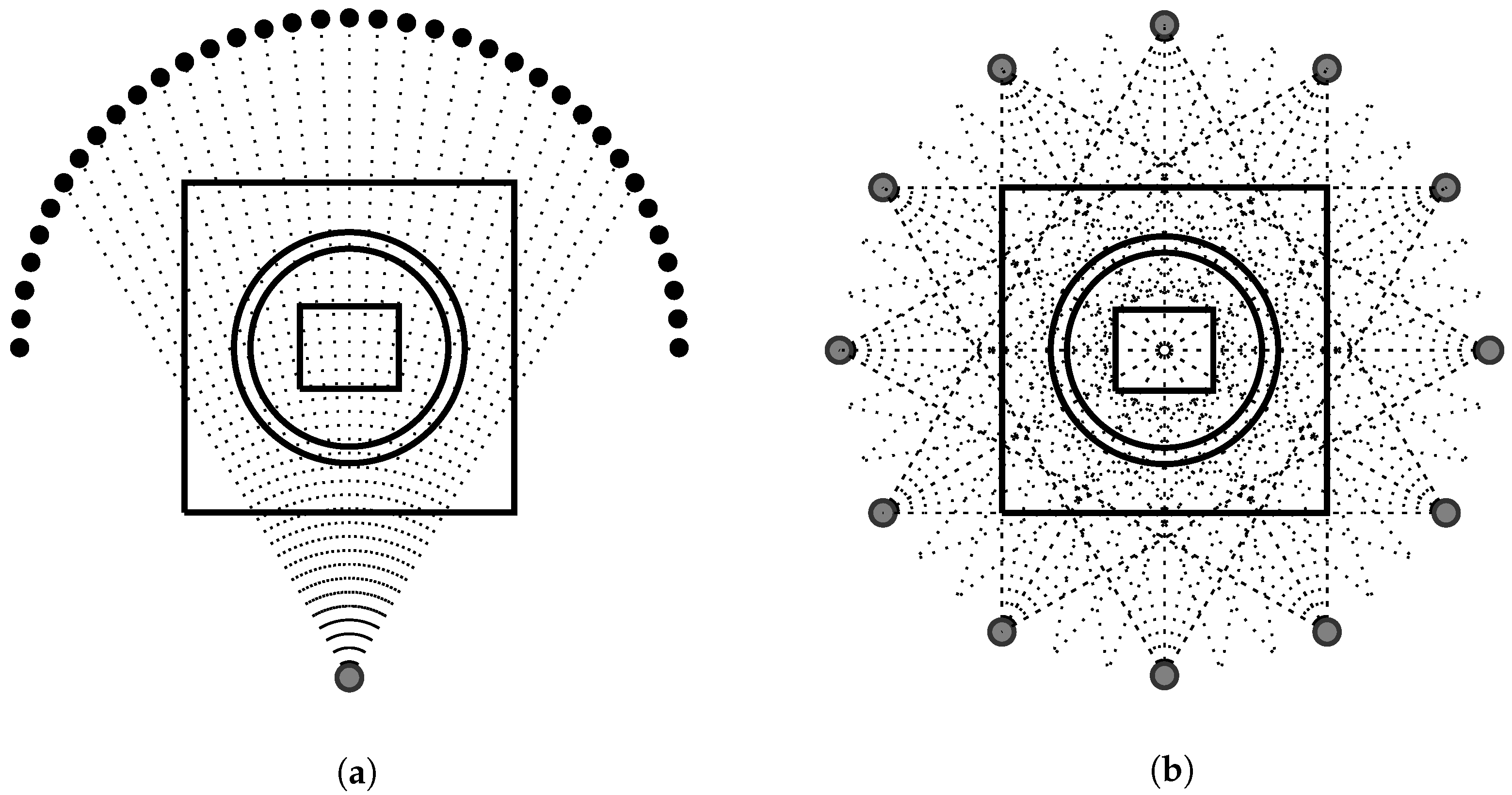
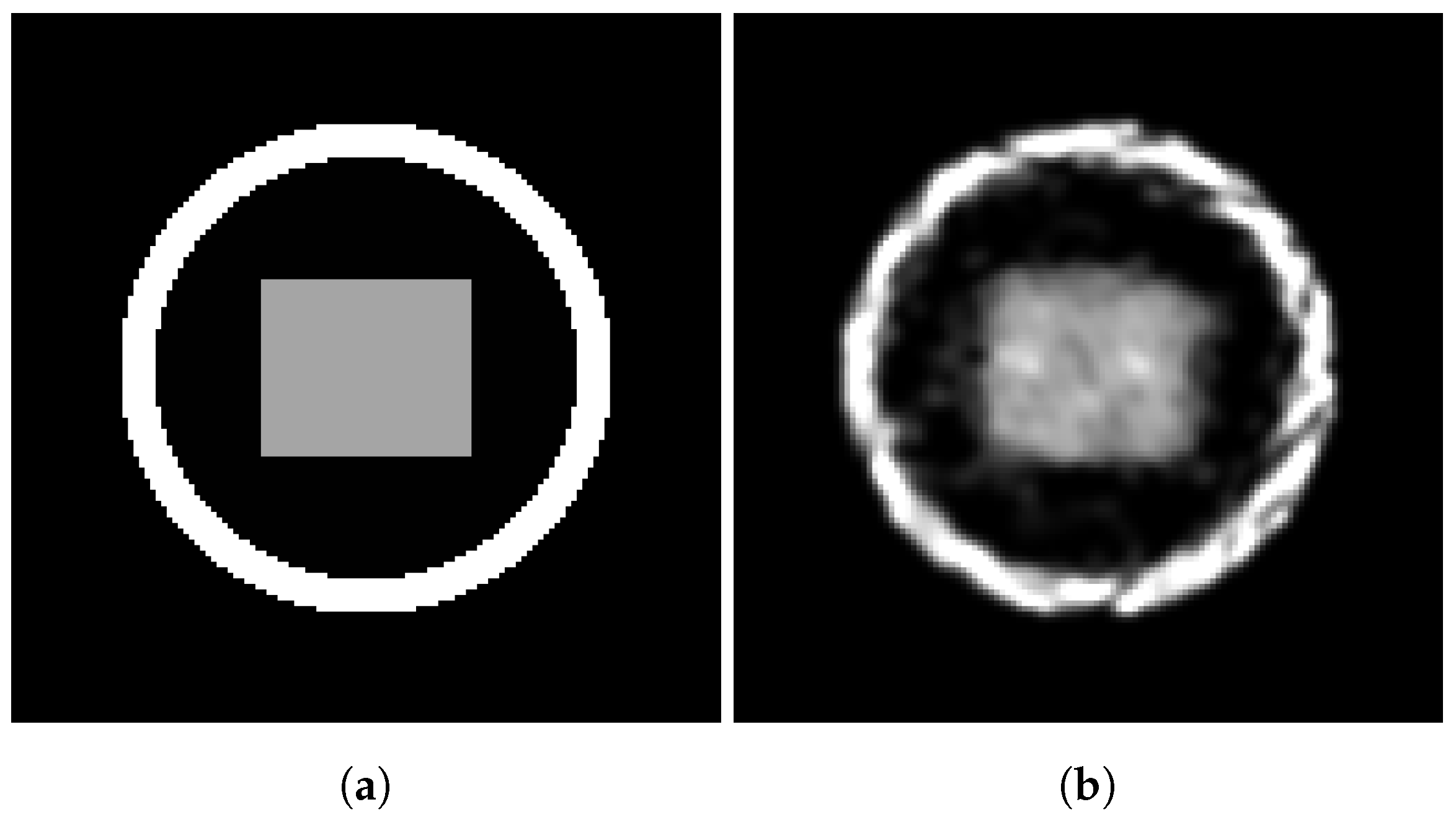
3. A CT-CST Scanner
3.1. Ballistic Data
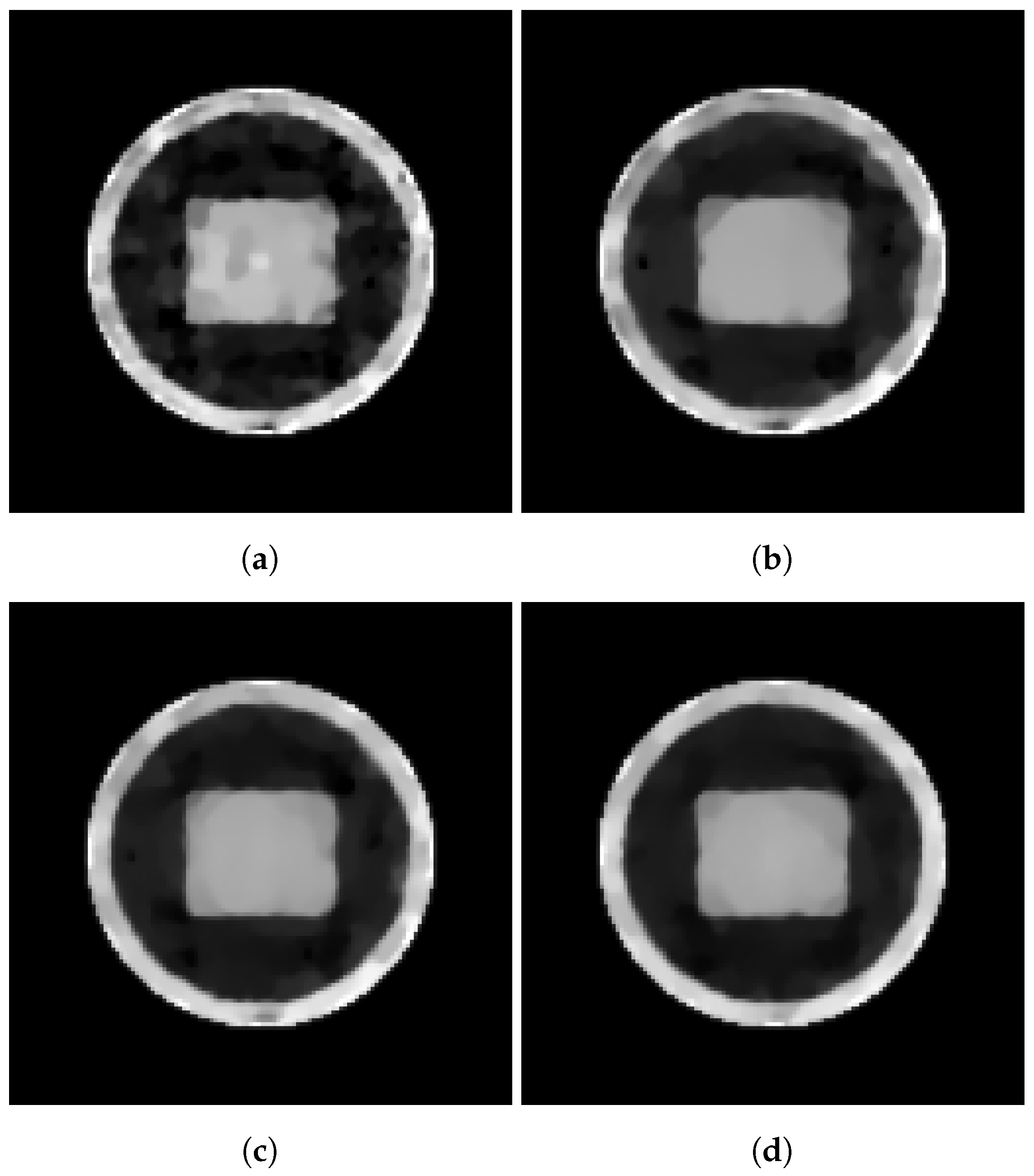
3.2. Results Based on the Compton Scattered Spectrum
4. Conclusions and Discussion
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CeBr3 | Cerium Bromide |
| CCD | Charge-coupled device |
| CST | Compton scattering tomography |
| CT | Computerized tomography |
| LBC:Ce | Lanthanum BromoChloride (La(BrCl)3:Ce) |
| MSE | Mean square error |
| PSNR | Peak signal-to-noise ratio |
References
- Natterer, F. The Mathematics of Computerized Tomography; Classics in Mathematics; Society for Industrial and Applied Mathematics: New York, NY, USA, 2001. [Google Scholar]
- Natterer, F.; Wübbeling, F. Mathematical Methods in Image Reconstruction; Classics in Mathematics; Society for Industrial and Applied Mathematics: New York, NY, USA, 2001. [Google Scholar]
- Hounsfield, G. Computerized transverse axial scanning (tomography). I. Description of system. Br. J. Radiol. 1973, 46, 1016–1022. [Google Scholar] [CrossRef] [PubMed]
- Alvarez, R.; Macovski, A. Energy-selective reconstructions in X-ray computerized tomography. Phys. Med. Biol. 1976, 21, 733–744. [Google Scholar] [CrossRef]
- Primak, A.; Giraldo, J.R.; Liu, X.; Yu, L.; McCollough, C. Improved dual-energy material discrimination for dual-source CT by means of additional spectral filtration. Med. Phys. 2009, 36, 1359–1369. [Google Scholar] [CrossRef]
- Shefer, E.; Altman, A.; Behling, R.; Goshen, R.; Gregorian, L.; Roterman, Y.; Uman, I.; Wainer, N.; Yagil, Y.; Zarchin, O. State of the Art of CT Detectors and Sources: A Literature Review. Curr. Radiol. Rep. 2013, 1, 76–91. [Google Scholar] [CrossRef]
- Tracey, B.; Miller, E. Stabilizing dual-energy X-ray computed tomography reconstructions using patch-based regularization. Inverse Probl. 2015, 31, 05004. [Google Scholar] [CrossRef]
- McCollough, C.; Leng, S.; Lifeng, Y.; Fletcher, J. Dual- and Multi-Energy CT: Principles, Technical Approaches, and Clinical Applications. Radiology 2015, 276, 637–653. [Google Scholar] [CrossRef] [PubMed]
- Goo, H.; Goo, J. Dual-Energy CT: New Horizon in Medical Imaging. Korean J. Radiol. 2017, 18, 555–569. [Google Scholar] [CrossRef]
- Fredenberg, E. Spectral and dual-energy X-ray imaging for medical applications. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2018, 878, 74–87. [Google Scholar] [CrossRef]
- Tian, Y.; Shimazoe, K.; Yan, X.; Ueda, O.; Ishikura, T.; Fujiwara, T.; Uesaka, M.; Ohno, M.; Tomita, H.; Yoshihara, Y.; et al. High energy X-ray photon counting imaging using linear accelerator and silicon strip detectors. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2016, 830, 251–255. [Google Scholar] [CrossRef]
- Izumi, S.; Kamata, S.; Satoh, K.; Miyai, H. High energy X-ray computed tomography for industrial applications. IEEE Trans. Nucl. Sci. 1993, 40, 158–161. [Google Scholar] [CrossRef]
- Toyokawa, H.; Kanada, H.; Kaihori, T.; Koike, M.; Yamada, K. Application of high-energy photon CT system with laser-Compton scattering to non-destructive test. IEEE Trans. Nucl. Sci. 2008, 55, 3571–3578. [Google Scholar] [CrossRef]
- Fraile, L.M.; Mach, H.; Vedia, V.; Olaizola, B.; Paziy, V.; Picado, E.; Udías, J.M. Fast timing study of a CeBr3 crystal: Time resolution below 120 ps at 60Co energies. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2013, 701, 235–242. [Google Scholar] [CrossRef]
- Otaka, Y.; Shimazoe, K.; Mitsuya, Y.; Uenomachi, M.; Seng, F.W.; Kamada, K.; Yoshikawa, A.; Sakuragi, S.; Binder, T.; Takahashi, H. Performance Evaluation of Liquinert-Processed CeBr3 Crystals Coupled With a Multipixel Photon Counter. IEEE Trans. Nucl. Sci. 2020, 67, 988–993. [Google Scholar] [CrossRef]
- Quarati, F.; Dorenbos, P.; van der Biezen, J.; Owens, A.; Selle, M.; Parthier, L.; Schotanus, P. Scintillation and detection characteristics of high-sensitivity CeBr 3 gamma-ray spectrometers. Nucl. Instrum. Methods Phys. Res. A 2013, 729, 596–604. [Google Scholar] [CrossRef]
- Hellma Materials GmbH. Radiation Detection Crystals. 2014. Available online: https://www.hellma.com/en/crystalline-materials/radiation-detection-crystals/ (accessed on 26 May 2021).
- Hellma Materials GmbH, Scionix Holland BV and Gonitec. Scintillation Properties of High-Resolution La(BrxCl1-x)3:Ce and High-Sensitivity CeBr3. 2017. Available online: https://indico.cern.ch/event/388511/contributions/2612029/attachments/1522152/2378455/SCINT2017_211_Poster.pdf (accessed on 26 May 2021).
- Stonestrom, J.P.; Alvarez, R.E.; Macovski, A. A framework for spectral artifact corrections in X-ray CT. IEEE Trans. Biomed. Eng. 1981, 28, 128–141. [Google Scholar] [CrossRef]
- Compton, A.H. A quantum theory of the scattering of X-rays by light elements. Phys. Rev. 1923, 21, 483–502. [Google Scholar] [CrossRef]
- Lale, P.G. The Examination of Internal Tissues, using Gamma-ray Scatter with a Possible Extension to Megavoltage Radiography. Phys. Med. Biol. 1959, 4, 159–167. [Google Scholar] [CrossRef]
- Norton, S.J. Compton scattering tomography. J. Appl. Phys. 1994, 76, 2007–2015. [Google Scholar] [CrossRef]
- Cesareo, R.; Borlino, C.C.; Brunetti, A.; Golosio, B.; Castellano, A. A simple scanner for Compton tomography. Nucl. Instrum. Methods Phys. Res. A 2002, 487, 188–192. [Google Scholar] [CrossRef]
- Adejumo, O.O.; Balogun, F.A.; Egbedokun, G.G.O. Developing a Compton scattering tomography system for soil studies: Theory. J. Sustain. Dev. Environ. Prot. 2011, 1, 73–81. [Google Scholar]
- Anghaie, S.; Humphries, L.L.; Diaz, N.J. Material characterization and flaw detection, sizing, and location by the differential gamma scattering spectroscopy technique. Part1: Development of theoretical basis. Nucl. Technol. 1990, 91, 361–375. [Google Scholar] [CrossRef]
- Balogun, F.A.; Cruvinel, P.E. Compton scattering tomography in soil compaction study. Nucl. Instrum. Methods Phys. Res. A 2003, 505, 502–507. [Google Scholar] [CrossRef]
- Brunetti, A.; Cesareo, R.; Golosio, B.; Luciano, P.; Ruggero, A. Cork quality estimation by using Compton tomography. Nucl. Instrum. Methods Phys. Res. B 2002, 196, 161–168. [Google Scholar] [CrossRef]
- Clarke, R.L.; Dyk, G.V. A new method for measurement of bone mineral content using both transmitted and scattered beams of gamma-rays. Phys. Med. Biol. 1973, 18, 532–539. [Google Scholar] [CrossRef] [PubMed]
- Evans, B.L.; Martin, J.B.; Burggraf, L.W.; Roggemann, M.C. Nondestructive inspection using Compton scatter tomography. IEEE Trans. Nucl. Sci. 1998, 45, 950–956. [Google Scholar] [CrossRef]
- Farmer, F.T.; Collins, M.P. A new approach to the determination of anatomical cross-sections of the body by Compton scattering of gamma-rays. Phys. Med. Biol. 1971, 16, 577–586. [Google Scholar] [CrossRef]
- Gorshkov, V.A.; Kroening, M.; Anosov, Y.V.; Dorjgochoo, O. X-ray scattering tomography. Nondestruct. Test. Eval. 2005, 20, 147–157. [Google Scholar] [CrossRef]
- Harding, G.; Harding, E. Compton scatter imaging: A tool for historical exploitation. Appl. Radiat. Isot. 2010, 68, 993–1005. [Google Scholar] [CrossRef] [PubMed]
- Meneley, D.A.; Hussein, E.M.A.; Banerjee, S. On the solution of the inverse problem of radiation scattering imaging. Nucl. Sci. Eng. 1986, 92, 341–349. [Google Scholar]
- Arendtsz, N.V.; Hussein, E.M.A. Energy-spectral Compton scatter Imaging—Part 1: Theory and mathematics. IEEE Trans. Nucl. Sci. 1995, 42, 2155–2165. [Google Scholar] [CrossRef]
- Guzzardi, R.; Licitra, G. A critical review of Compton imaging. CRC Crit. Rev. Biomed. Imaging 1988, 15, 237–268. [Google Scholar]
- Rigaud, G. Compton Scattering Tomography: Feature Reconstruction and Rotation-Free Modality. SIAM J. Imaging Sci. 2017, 10, 2217–2249. [Google Scholar] [CrossRef]
- Gurov, Y.B.; Egorov, N.Y.; Ponomarev, D.V.; Rozov, S.V.; Sandukovsky, V.G.; Shakhov, K.V.; Yakushev, E.A.; Zhivun, V.M. Study of characteristics of CdZnTe detector. J. Instrum. 2019, 14, P11002. [Google Scholar] [CrossRef]
- Negm, H.; Ohgaki, H.; Izuru, D.; Hayakawa, T.; Zen, H.; Kii, T.; Masuda, K.; Hori, T.; Hajima, R.; Shizuma, T.; et al. Reaction-yield dependence of the (γ, γ’) reaction of 238U on the target thickness. J. Nucl. Sci. Technol. 2014, 1–10. [Google Scholar] [CrossRef]
- Knoll, G.F. Radiation Detection and Measurement; John Wiley & Sons, Inc.: New York, NY, USA, 1989. [Google Scholar]
- Klein, O.; Nishina, Y. Über die Streuung von Strahlung durch freie Elektronen nach der neuen relativistischen Quantendynamik von Dirac. Z. Phys. 1929, 52, 853–869. [Google Scholar] [CrossRef]
- Rigaud, G. 3D Compton scattering imaging with multiple scattering: Analysis by FIO and contour reconstruction. Inverse Probl. 2021. [Google Scholar] [CrossRef]
- Kuger, L.; Rigaud, G. Joint fan-beam CT and Compton scattering tomography: Analysis and image reconstruction. arXiv 2020, arXiv:2008.06699. [Google Scholar]
- Rudin, L.; Osher, S.; Fatemi, E. Nonlinear total variation based noise removal algorithms. Physica D 1992, 46, 259–268. [Google Scholar] [CrossRef]


| LBC:Ce | CeBr3 | |
|---|---|---|
| Emission Wavelength [nm] | 380 | 380 |
| Energy resolution at 662 keV [% FWHM] | 2.8 | 3.8 |
| Light Yield [photons/MeV] | 75,000 | 60,000 |
| Decay Time [ns] | <25 | 19 |
| Density [g/cm3] | ≈5 | 5.1 |
| Atomic Number (effective) | 44 … 45.2 | 45.9 |
| Intrinsic Activity [Bq/cm3] in range [1550 keV, 2250 keV] | ≈1.5 | <0.002 |
| hygroscopic | yes | yes |
| Annulus | Rectangle | Crystal | |
|---|---|---|---|
| Aluminium | Polyethylene | LBC:Ce | |
| Surface | 53 cm outer/45 cm inner radius | ||
| Thickness | 2 cm | 2 cm | 5 cm |
| 150 cm | 100 cm | 75 cm | 60 cm | |
|---|---|---|---|---|
| PSNR | 13.69 dB | 18.02 dB | 19.16 dB | 19.62 dB |
| MSE | 4.28% | 1.58% | 1.21% | 1.09% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuger, L.; Rigaud, G. Modeling and Reconstruction Strategy for Compton Scattering Tomography with Scintillation Crystals. Crystals 2021, 11, 641. https://doi.org/10.3390/cryst11060641
Kuger L, Rigaud G. Modeling and Reconstruction Strategy for Compton Scattering Tomography with Scintillation Crystals. Crystals. 2021; 11(6):641. https://doi.org/10.3390/cryst11060641
Chicago/Turabian StyleKuger, Lorenz, and Gael Rigaud. 2021. "Modeling and Reconstruction Strategy for Compton Scattering Tomography with Scintillation Crystals" Crystals 11, no. 6: 641. https://doi.org/10.3390/cryst11060641
APA StyleKuger, L., & Rigaud, G. (2021). Modeling and Reconstruction Strategy for Compton Scattering Tomography with Scintillation Crystals. Crystals, 11(6), 641. https://doi.org/10.3390/cryst11060641