Exploring the Regimes of Particle Behavior upon Impact via the Discrete Element Method
Abstract
1. Introduction
2. Materials and Methods
2.1. DEM Model with Adhesion
2.2. Agglomerate Preparation
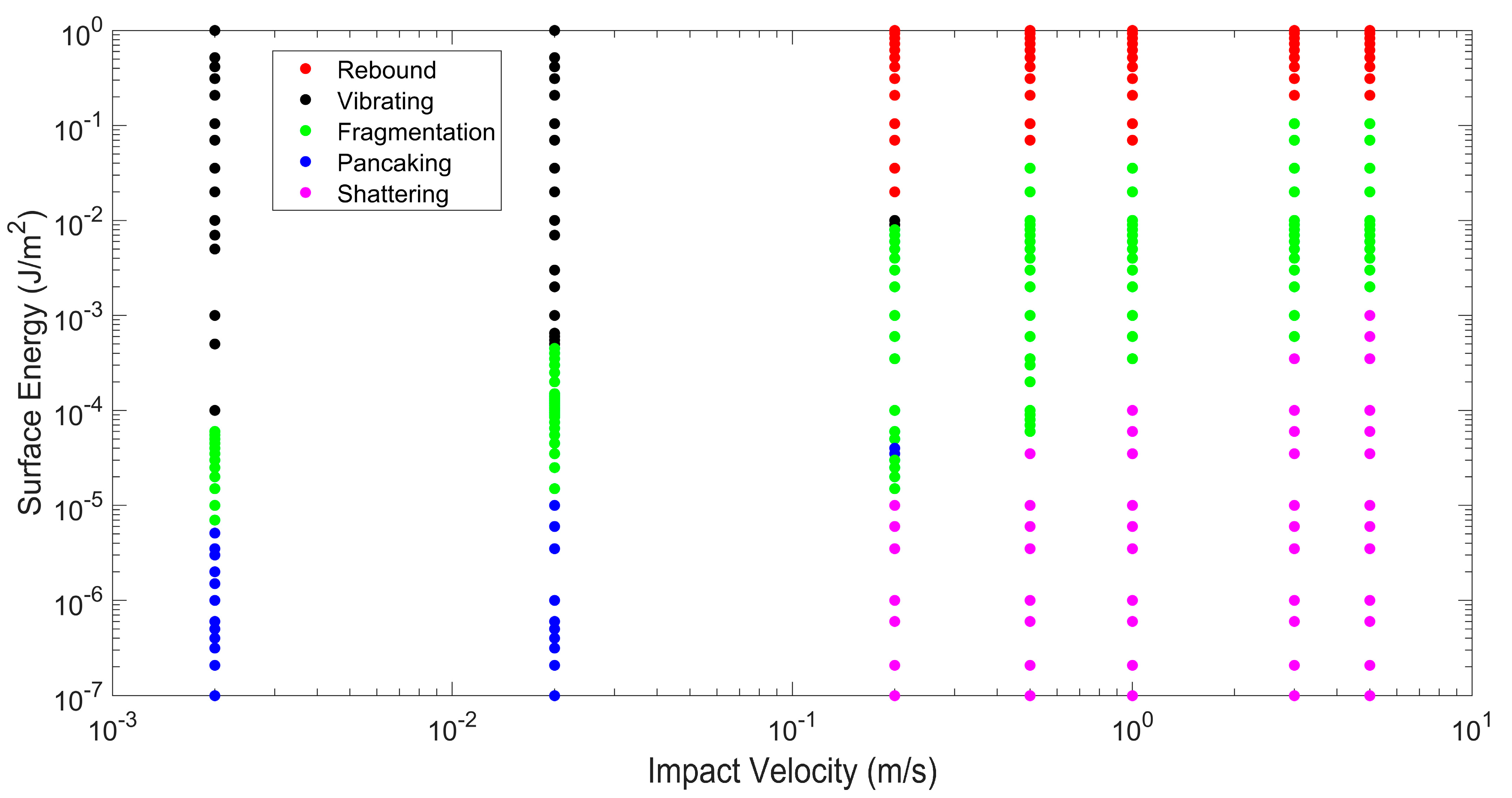
3. Results and Discussion
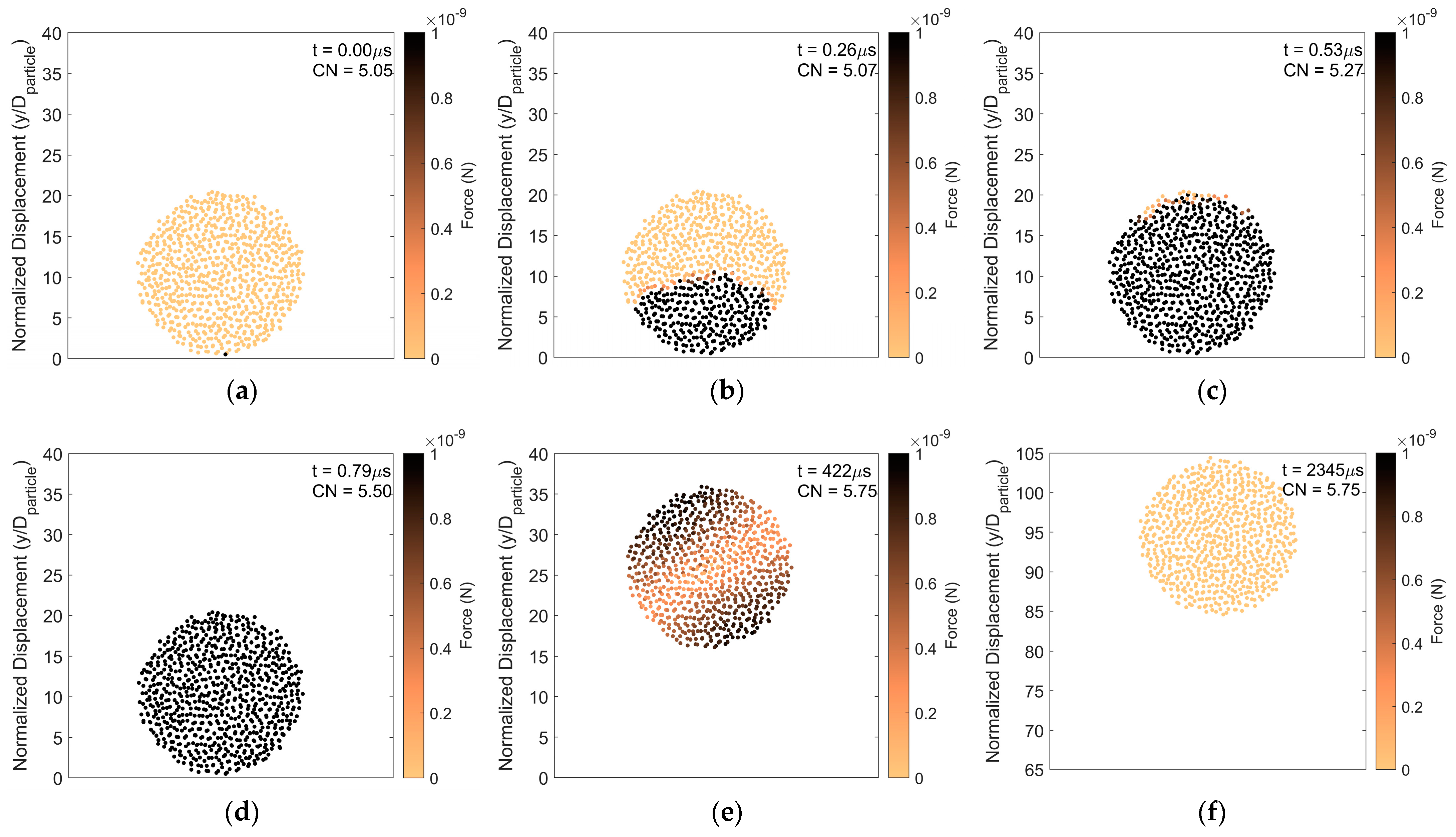
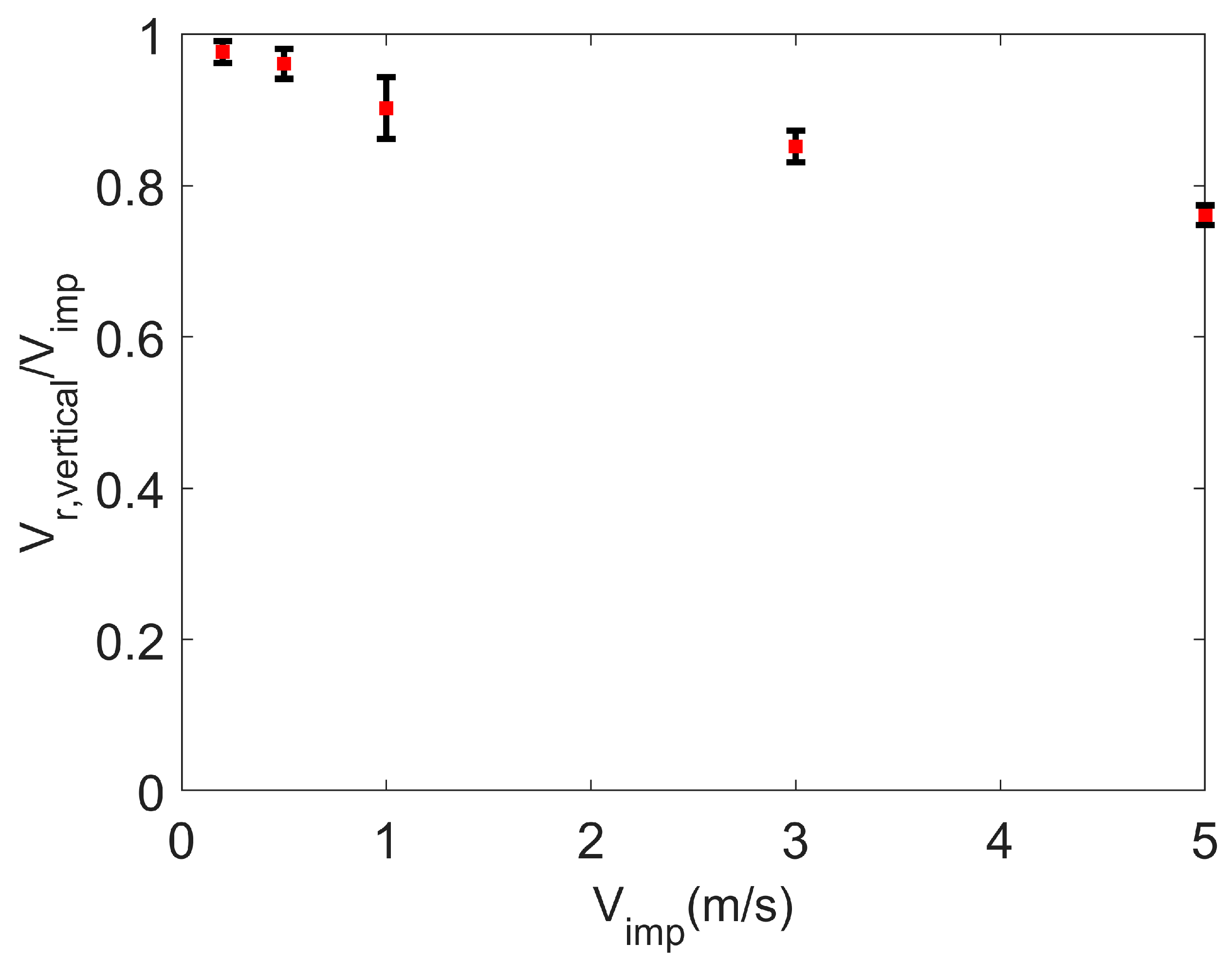
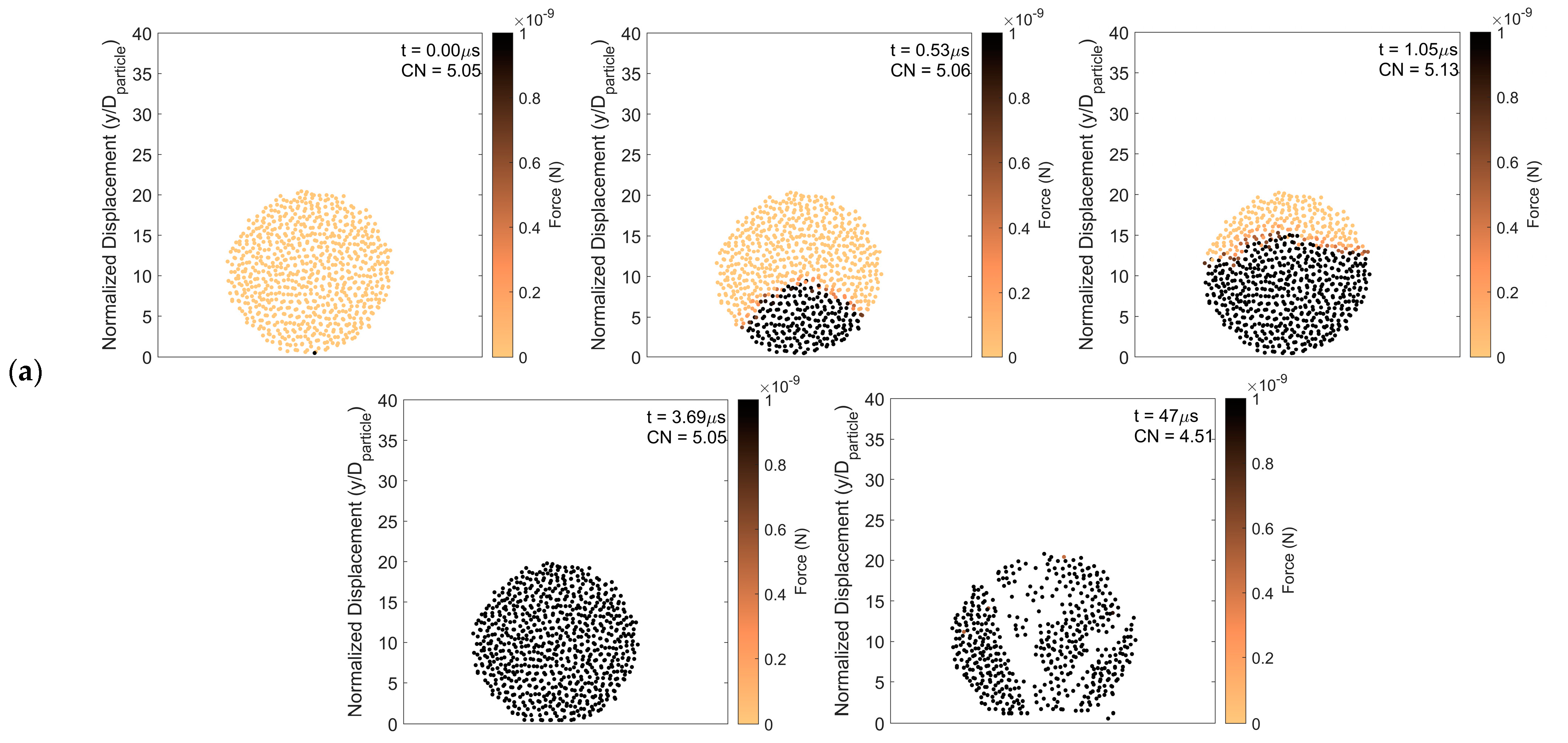
3.1. Rebound Regime
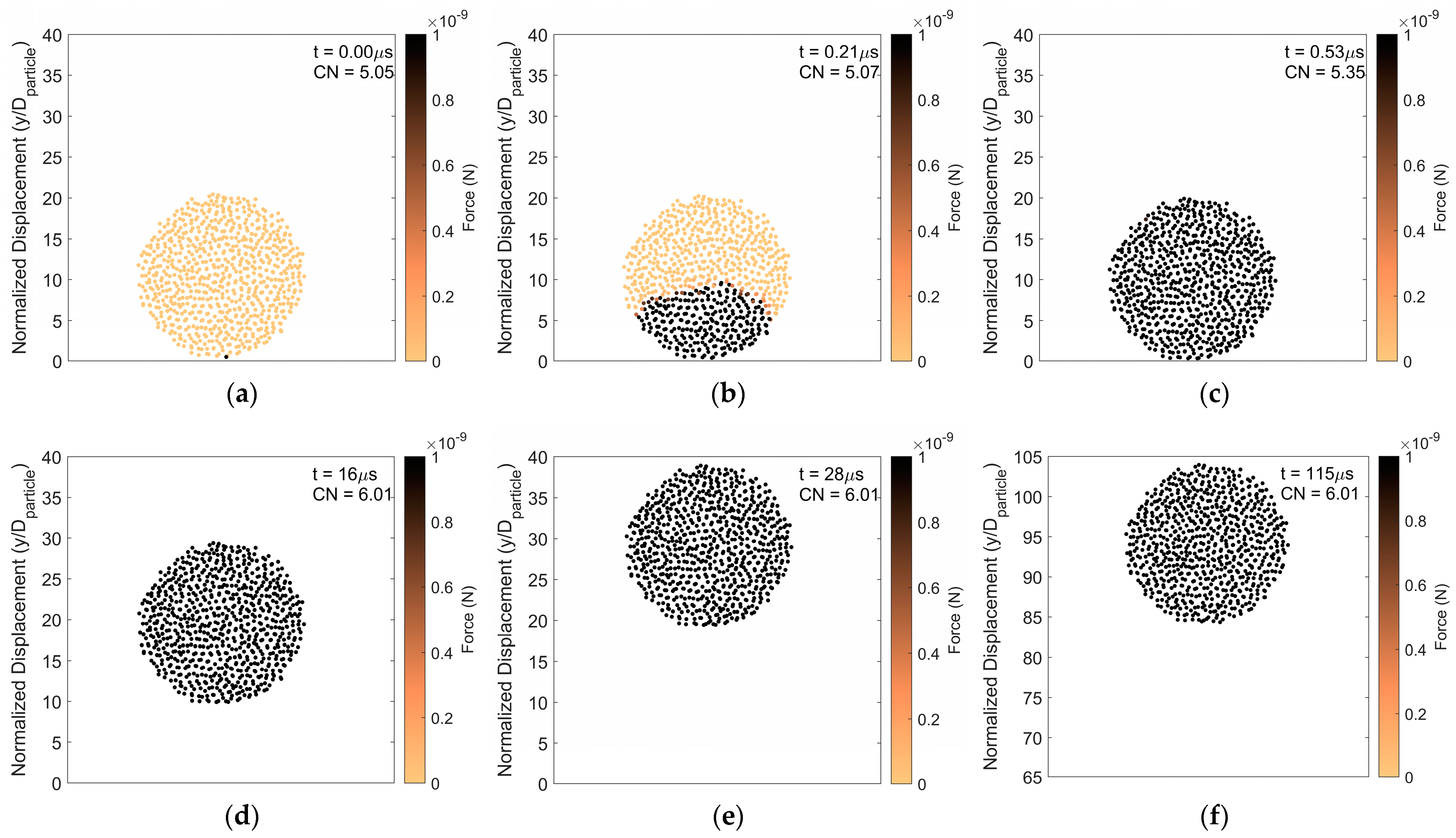
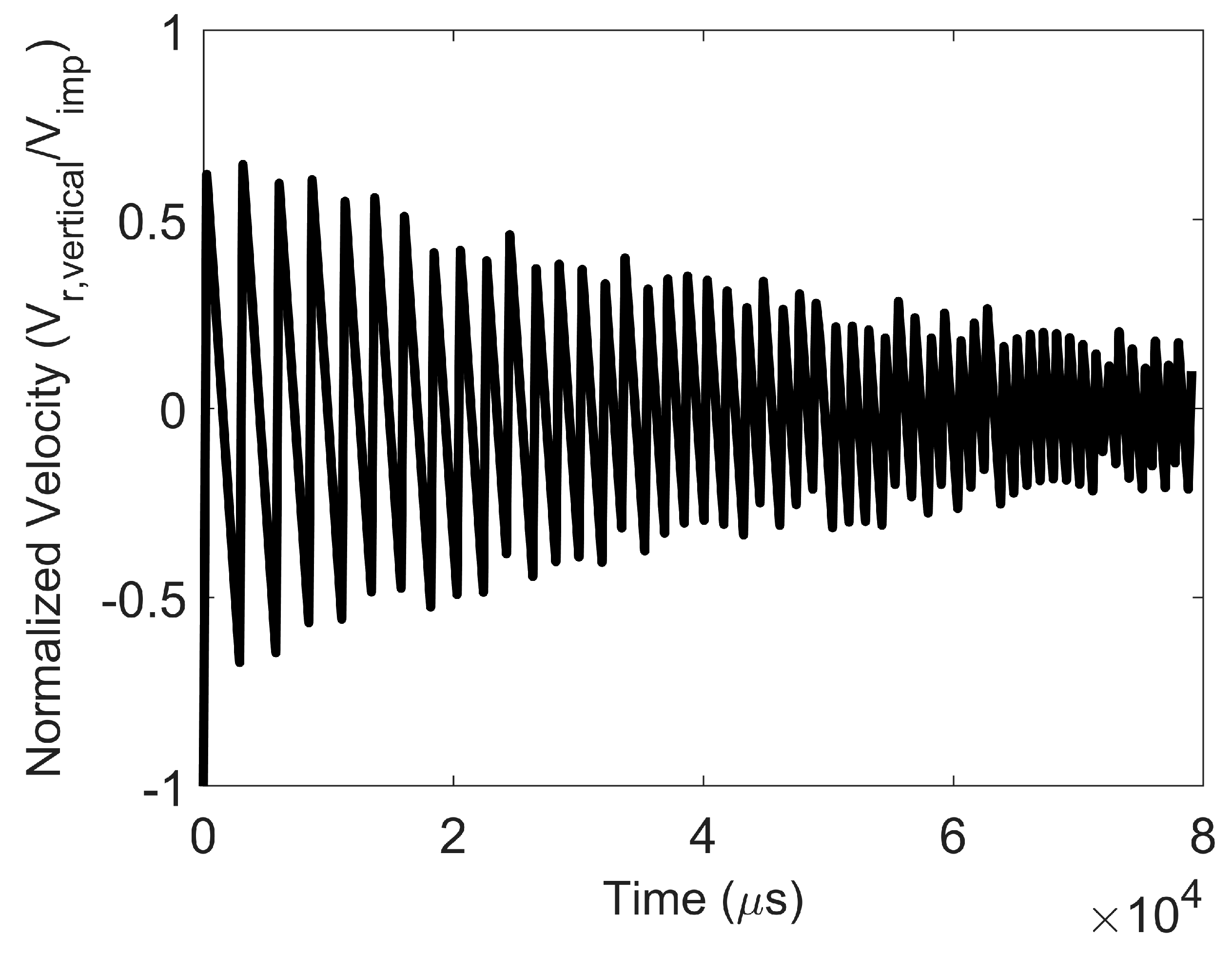
3.2. Vibrating Regime
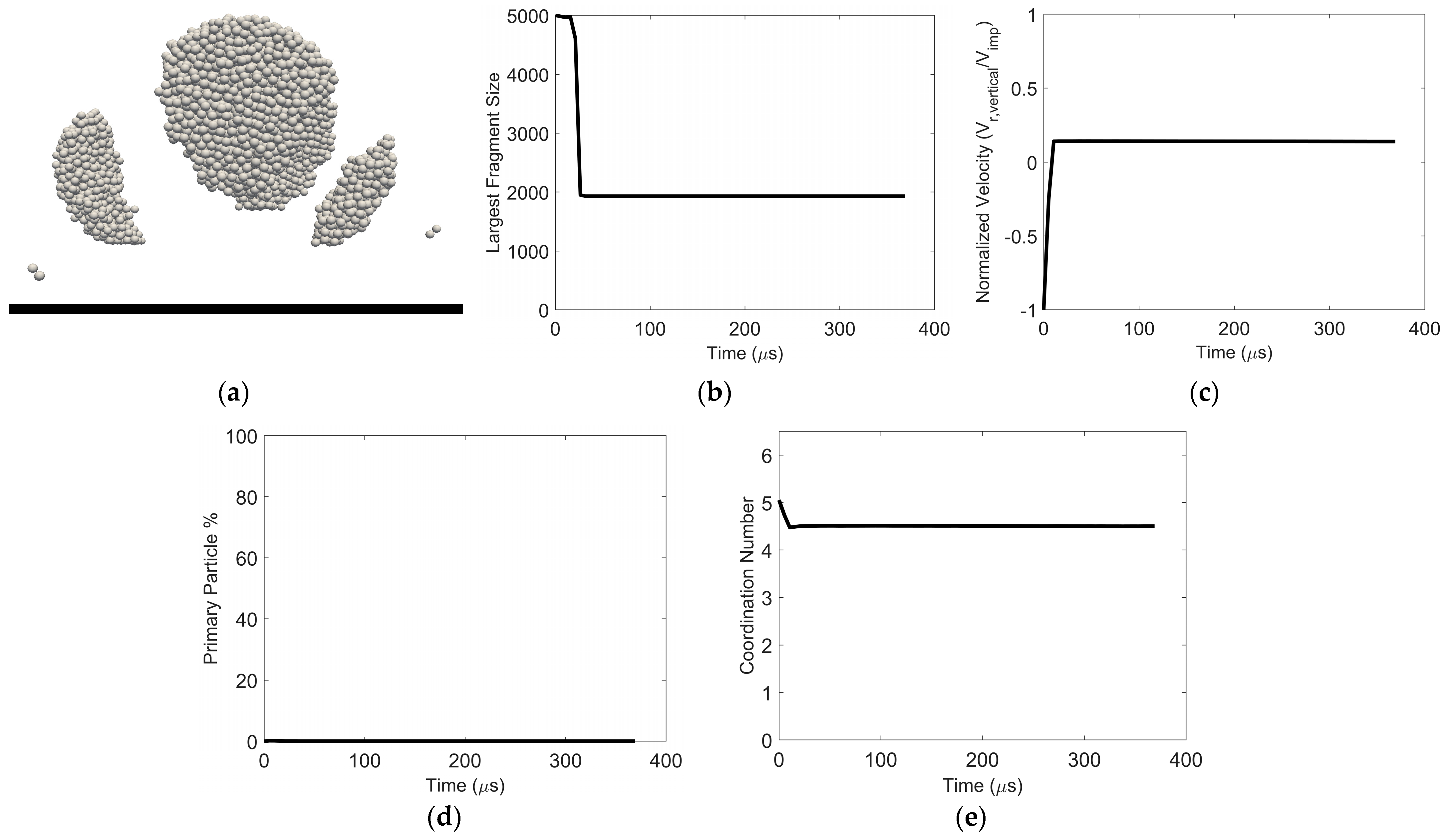
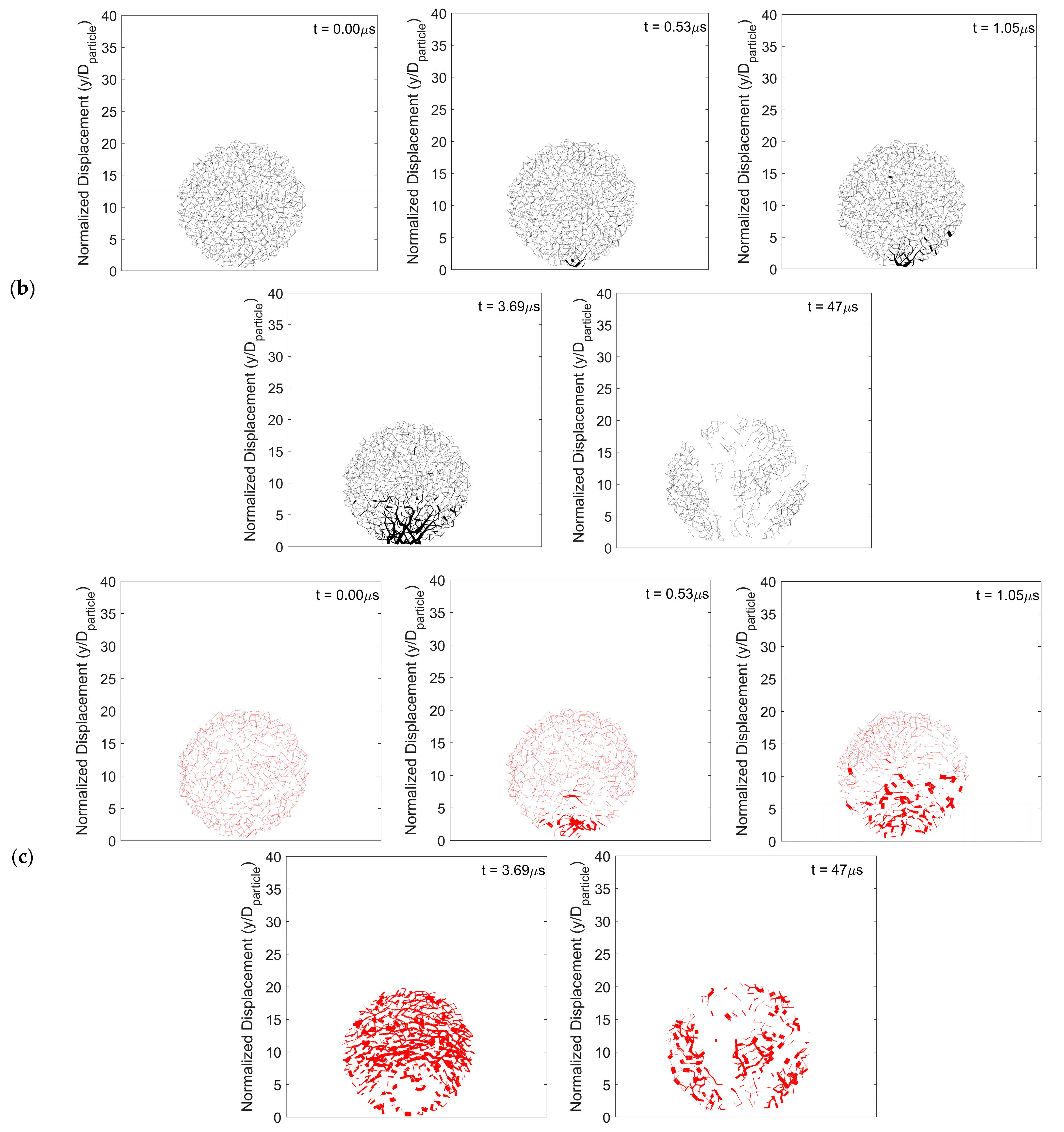
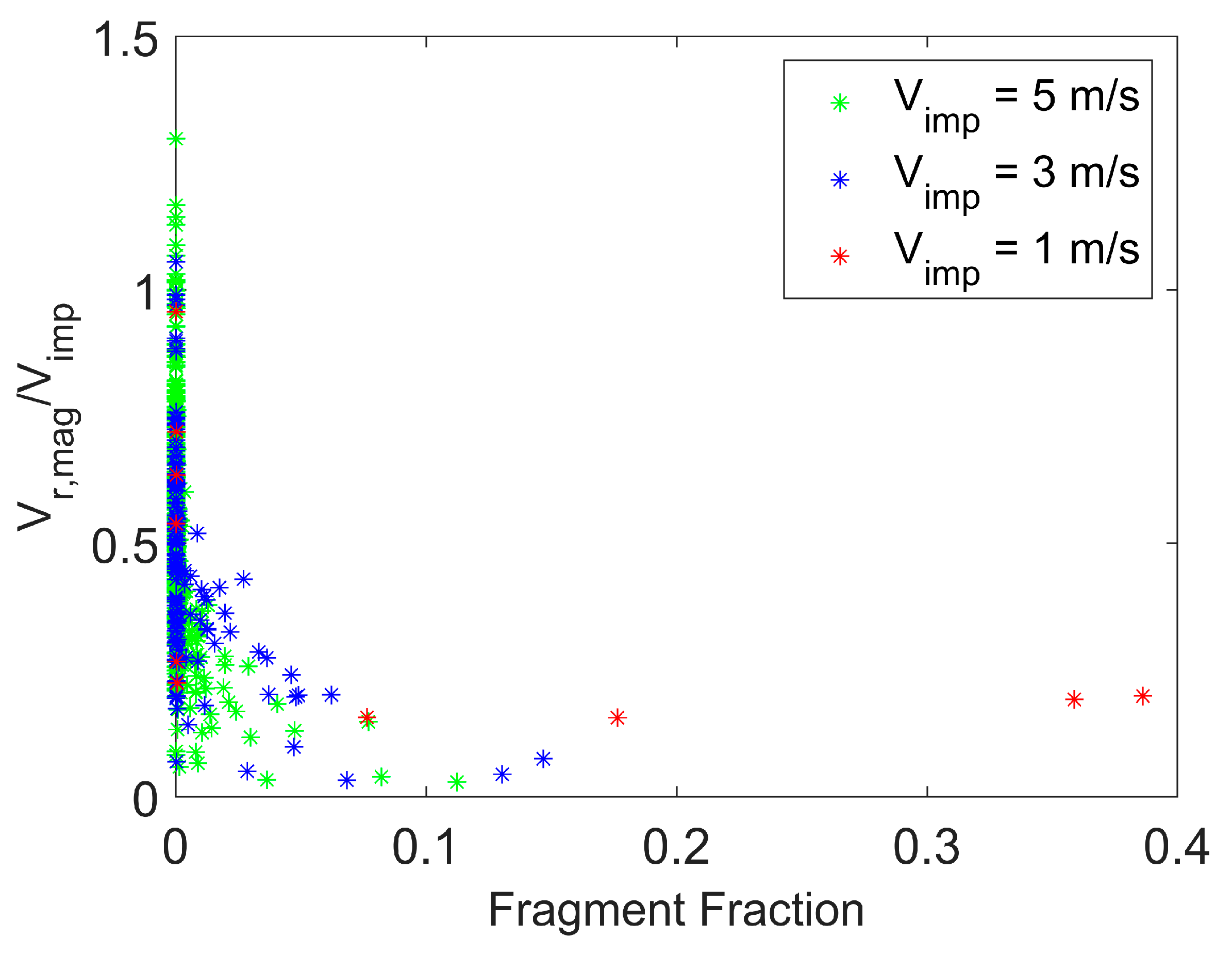
3.3. Fragmentation Regime
3.4. Pancaking Regime
3.5. Shattering Regime
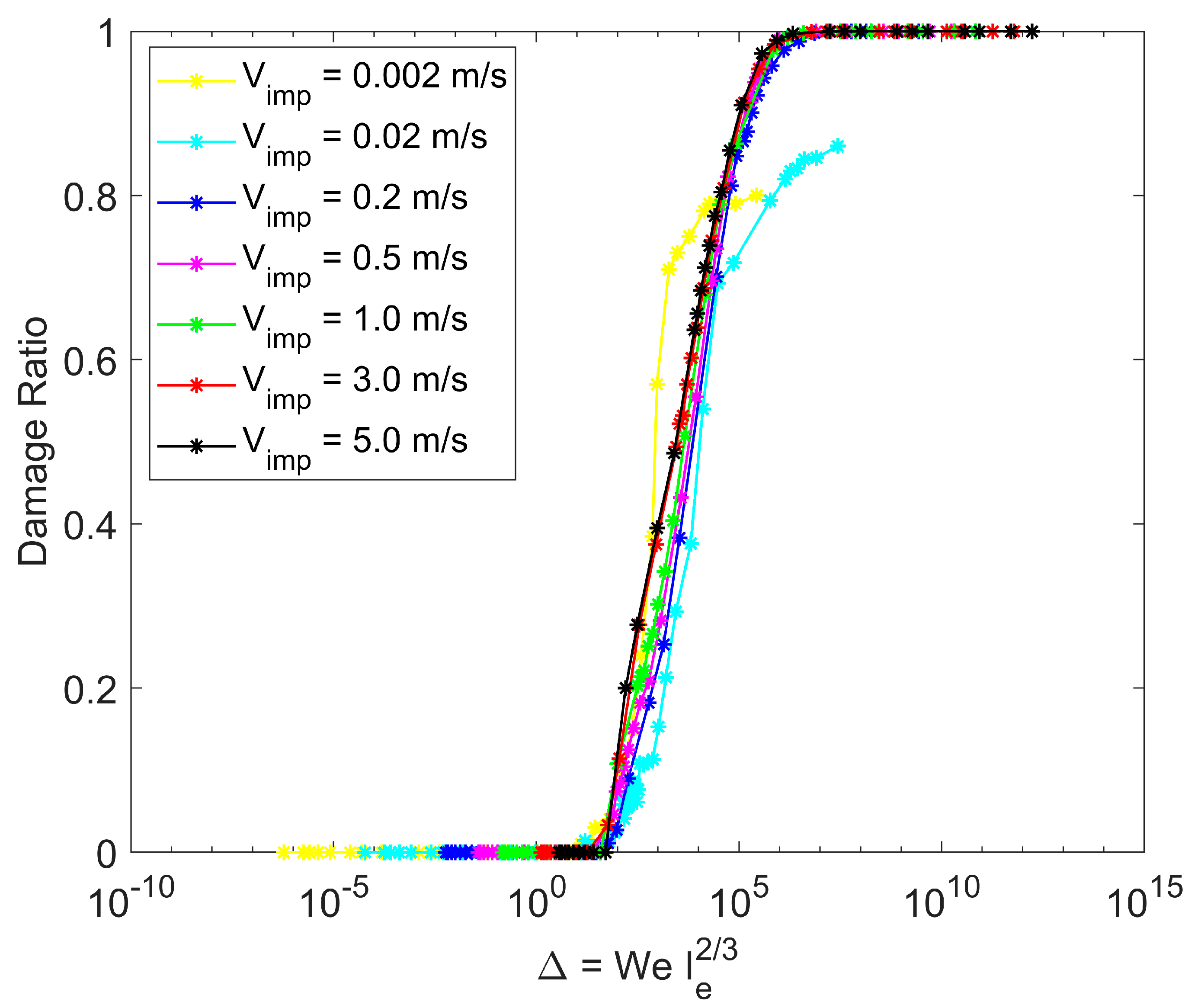
3.6. Comparison with Mechanistic Model
4. Conclusions
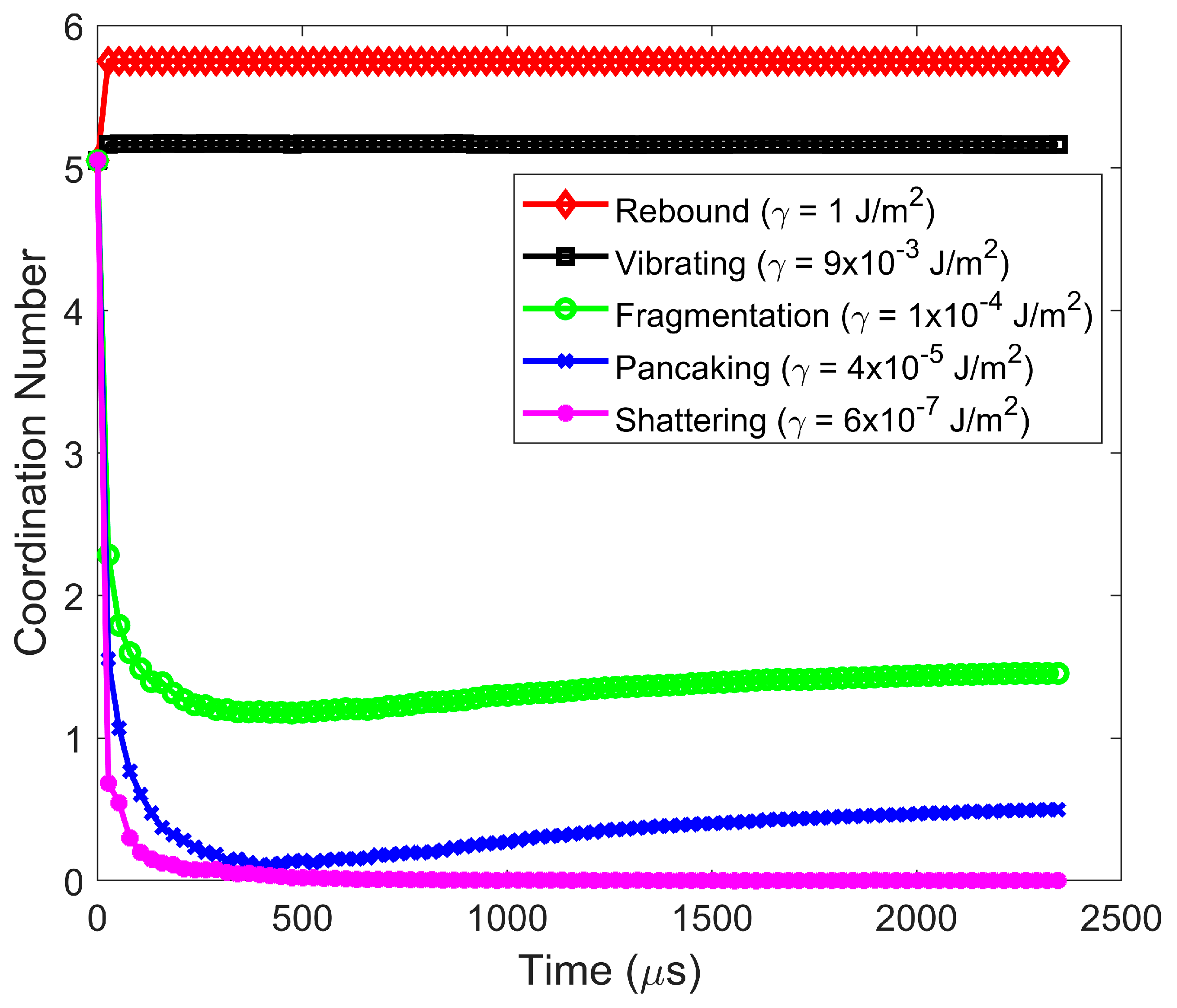
- Rebound regime: Increasing particle agglomerate compaction and coordination number and decreasing coefficient of restitution with increasing impact velocity.
- Vibration regime: Rapid decay of rebound velocity with time with minimal change in particle agglomerate packing and coordination number.
- Fragmentation regime: Smaller fragments have a larger rebound velocity than larger fragments and the smallest fragments can have rebound velocities which exceed the initial impact velocity. The rebound velocity of the smallest fragments can also be directed towards the wall once again due to collisions between fragments.
- Pancaking regime: Frequent collisions between fragments at or near the wall lead to an increase in the size of some of the fragments with time.
- Shattering regime: The trajectory of the resulting primary particles is radially directed along the plate from the point of impact at lower impact velocities but has a significant vertical component as the impact velocity is increased.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Weinhart, T.; Lechman, J.; Poschel, T. Fragmentation and abrasion in granular matter systems. Comput. Part. Mech. 2021, 8, 1003–1004. [Google Scholar] [CrossRef]
- Mueller, R.; Kleinebudde, P. Abrasion of tablets during scale-up. Eur. J. Pharm. Biopharm. 2007, 67, 458–463. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, T.; Assadi, H.; Gartner, F.; Richter, H.; Stoltenhoff, T.; Kreye, H.; Klassen, T. From particle acceleration to impact and bonding in cold spraying. J. Therm. Spray Technol. 2009, 18, 794–808. [Google Scholar] [CrossRef]
- Assadi, H.; Kreye, H.; Gartner, F.; Klassen, T. Cold spraying—A materials perspective. Acta Mater. 2016, 116, 382–407. [Google Scholar] [CrossRef]
- Zhang, F.; Frost, D.L.; Thibault, P.A.; Murray, S.B. Explosive dispersal of solid particles. Shock. Waves 2001, 10, 431–443. [Google Scholar] [CrossRef]
- Frost, D.L.; Ornthanalai, C.; Zarei, Z. Particle momentum effects from detonation of heterogeneous explosives. J. Appl. Phys. 2007, 101, 113529. [Google Scholar] [CrossRef]
- Hare, C.; Bonakdar, T.; Ghadiri, M. Impact breakage of pharmaceutical tablets. Int. J. Pharm. 2018, 536, 370–376. [Google Scholar] [CrossRef] [PubMed]
- Subero, J.; Ghadiri, M. Breakage patterns of agglomerates. Powder Technol. 2001, 120, 232–243. [Google Scholar] [CrossRef]
- Ali, M.; Bonakdar, T.; Ghadiri, M.; Tinke, A. Particle Breakage in a Scirocco Disperser. Powder Technol. 2015, 285, 138–145. [Google Scholar] [CrossRef]
- Salman, A.D.; Biggs, C.A.; Fu, J.; Angyal, I.; Szabo, M.; Hounslow, M.J. An experimental investigation of particle fragmentation using single particle impact studies. Powder Technol. 2002, 128, 36–46. [Google Scholar] [CrossRef]
- Salman, A.D.; Reynolds, G.K.; Fu, J.S.; Cheong, Y.S.; Biggs, C.A.; Adams, M.J.; Gorham, D.A.; Lukenics, J.; Hounslow, M.J. Descriptive classification of the impact failure modes of spherical particles. Powder Technol. 2004, 143, 19–30. [Google Scholar] [CrossRef]
- Olaleye, B.; Wu, C.-Y.; Liu, L. Impact breakage of single pharmaceutical tablets in an air gun. Int. J. Pharm. 2021, 597, 120273. [Google Scholar] [CrossRef] [PubMed]
- Hassani-Gangaraj, M.; Veysset, D.; Nelson, K.A.; Schuh, C.A. Melt-driven erosion in microparticle impact. Nat. Commun. 2018, 9, 5077. [Google Scholar] [CrossRef] [PubMed]
- Wu, C.-Y.; Ruddy, O.; Bentham, A.; Hancock, B.; Best, S.; Elliott, J. Modelling the mechanical behavior of pharmaceutical powders during compaction. Powder Technol. 2005, 152, 107–117. [Google Scholar] [CrossRef]
- Sinha, T.; Bharadwaj, B.; Curtis, J.; Hancock, B.; Wassgren, C. Finite element analysis of pharmaceutical tablet compaction using a density dependent material plasticity model. Powder Technol. 2010, 202, 46–54. [Google Scholar] [CrossRef]
- Partheniadis, I.; Terzi, V.; Nikolakakis, I. Finite element analysis and modeling in pharmaceutical tableting. Pharmaceutics 2022, 14, 673. [Google Scholar] [CrossRef]
- Bunsell, A.; Gorbatikh, L.; Morton, H.; Pimenta, S.; Sinclair, I.; Spearing, M.; Swolfs, Y.; Thionnet, A. Benchmarking of strength models for unidirectional composites under longitudinal tension. Compos. Part A 2018, 111, 138–150. [Google Scholar] [CrossRef]
- Li, H.; Shi, G. A dynamic material model for rock materials under conditions of high confining pressures and high strain rates. Int. J. Impact Eng. 2016, 89, 38–48. [Google Scholar] [CrossRef]
- Barton, N.R.; Bernier, J.V.; Becker, R.; Arsenlis, A.; Cavallo, R.; Marian, J.; Rhee, M.; Park, H.-S.; Remington, B.A.; Olson, R.T. A multiscale strength model for extreme loading conditions. J. Appl. Phys. 2011, 109, 073501. [Google Scholar] [CrossRef]
- Ge, R.; Ghadiri, M.; Bonakdar, T.; Hapgood, K. 3D printed agglomerates for granule breakage tests. Powder Technol. 2017, 306, 103–112. [Google Scholar] [CrossRef]
- Ge, R.; Ghadiri, M.; Bonakdar, T.; Zhou, Z.; Larson, I.; Hapgood, K. Experimental study of the deformation and breakage of 3D printed agglomerates: Effects of packing density and inter-particle bond strength. Powder Technol. 2018, 340, 299–310. [Google Scholar] [CrossRef]
- Ge, R.; Wang, L.; Zhou, Z. DEM analysis of compression breakage of 3D printed agglomerates with different structures. Powder Technol. 2019, 356, 1045–1058. [Google Scholar] [CrossRef]
- Ge, R.; Ghadiri, M.; Bonakdar, T.; Zheng, Q.; Zhou, Z.; Larson, L.; Hapgood, K. Deformation of 3D printed agglomerates: Multiscale experimental tests and DEM simulation. Chem. Eng. Sci. 2020, 217, 115526. [Google Scholar] [CrossRef]
- Dosta, M.; Dale, S.; Antonyuk, S.; Wassgren, C.; Heinrich, S.; Litster, J.D. Numerical and experimental analysis of influence of granule microstructure on its compression breakage. Powder Technol. 2016, 299, 87–97. [Google Scholar] [CrossRef]
- Quist, J.; Evertsson, C.M. Cone crusher modelling and simulation using DEM. Miner. Eng. 2016, 85, 92–105. [Google Scholar] [CrossRef]
- Scholtes, L.; Donze, F.-V. A DEM model for soft and hard rocks: Role of grain interlocking on strength. J. Mech. Phys. Solids 2013, 61, 352–369. [Google Scholar] [CrossRef]
- Liu, G.Y.; Xu, W.J.; Sun, Q.C.; Govender, N. Study on the particle breakage of ballast based on a GPU accelerated discrete element method. Geosci. Front. 2020, 11, 461–471. [Google Scholar] [CrossRef]
- Gimenez, C.; Finke, B.; Nowak, C.; Schilde, C.; Kwade, A. Structural and mechanical characterization of lithium-ion battery electrodes via DEM simulations. Adv. Powder Technol. 2018, 29, 2312–2321. [Google Scholar] [CrossRef]
- Brown, N.J.; Chen, J.-F.; Ooi, J.Y. A bond model for DEM simulation of cementitious materials and deformable structures. Granul. Matter 2014, 16, 299–311. [Google Scholar] [CrossRef]
- Potyondy, D.O.; Cundall, P.A. A bonded-particle model for rock. Int. J. Rock Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Antonyuk, S.; Palis, S.; Heinrich, S. Breakage behavior of agglomerates and crystals by static loading and impact. Powder Technol. 2011, 206, 88–98. [Google Scholar] [CrossRef]
- Cabiscol, R.; Finke, J.; Kwade, A. A bi-directional DEM-PBM coupling to evaluate chipping and abrasion of pharmaceutical tablets. Adv. Powder Technol. 2021, 32, 2839–2855. [Google Scholar] [CrossRef]
- Yang, J.; Bello, L.; Buettner, K.; Guo, Y.; Wassgren, C.; Curtis, J.S. Breakage of wet flexible fiber agglomerates impacting a plane. AIChE J. 2019, 65, e16626. [Google Scholar] [CrossRef]
- Subero, J.; Ning, Z.; Ghadiri, M.; Thornton, C. Effect of interface energy on the impact strength of agglomerates. Powder Technol. 1999, 105, 66–73. [Google Scholar] [CrossRef]
- Moreno, R.; Ghadiri, M.; Antony, S.J. Effect of the impact angle on the breakage of agglomerates: A numerical study using DEM. Powder Technol. 2003, 130, 132–137. [Google Scholar] [CrossRef]
- Tong, Z.B.; Yang, R.Y.; Yu, A.B.; Adi, S.; Chan, H.K. Numerical modelling of the breakage of loose agglomerates of fine particles. Powder Technol. 2009, 196, 213–221. [Google Scholar] [CrossRef]
- Tamadondar, M.R.; de Martin, L.; Rasmuson, A. Agglomerate breakage and adhesion upon impact with complex-shaped particles. AIChE J. 2019, 65, e16581. [Google Scholar] [CrossRef]
- Johnson, K.L.; Kendall, K.; Roberts, A.D. Surface energy and the contact of elastic solids. Proc. R. Soc. Lond. A 1971, 324, 301–313. [Google Scholar]
- Johnson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Mindlin, R.D.; Deresiewicz, H. Elastic spheres in contact under varying oblique force. J. Appl. Mech. 1953, 20, 327–344. [Google Scholar] [CrossRef]
- Savkoor, A.R.; Briggs, G.A.D. The effect of tangential force on the contact of elastic solids in adhesion. Proc. R. Soc. Lond. A. 1977, 356, 103–114. [Google Scholar]
- Thornton, C.; Yin, K.K. Impact of elastic spheres with and without adhesion. Powder Technol. 1991, 65, 153–166. [Google Scholar] [CrossRef]
- Zhang, L. The Behaviour of Granular Material in Pure Shear, Direct Shear and Simple Shear. Ph.D. Thesis, Aston University, Birmingham, UK, 2003. [Google Scholar]
- Phani, K.K.; Sanyal, D. The relations between the shear modulus, the bulk modulus and Young’s modulus for porous isotropic ceramic materials. Mater. Sci. Eng. A 2008, 490, 305–312. [Google Scholar] [CrossRef]
- Guo, Y.; Wassgren, C.; Ketterhagen, W.; Hancock, B.; James, B.; Curtis, J. A numerical study of granular shear flows of rod-like particles using the discrete element method. J. Fluid Mech. 2012, 713, 1–26. [Google Scholar] [CrossRef]
- Moreno-Atanasio, R.; Ghadiri, M. Mechanistic analysis and computer simulation of impact breakage of agglomerates: Effect of surface energy. Chem. Eng. Sci. 2006, 61, 2476–2481. [Google Scholar] [CrossRef]
- Hua, X.; Curtis, J.; Guo, Y.; Hancock, B.; Ketterhagen, W.; Wassgren, C. The internal loads, moments, and stresses in rod-like particles in a low-speed vertical axis mixer. Chem. Eng. Sci. 2015, 134, 581–598. [Google Scholar] [CrossRef]



| Critical normal displacement | Johnson [39] | |
| Pull-off force | Ri, radius of constituent particle i | Johnson [39] |
| Critical tangential contact force | Thornton and Yin [42] | |
| Effective radius | Johnson [39] Thornton and Yin [42] | |
| Effective Young’s modulus | νi, Poisson’s ratio of particle i Ei, Young’s modulus of particle i | Johnson [39] Thornton and Yin [42] |
| Shear modulus | Gi, shear modulus of particle i | Phani and Sanyal [44] |
| Effective shear modulus | Johnson [39] Thornton and Yin [42] | |
| Contact damping coefficient | e, coefficient of normal restitution | Guo et al. [45] |
| Effective mass | mi, mass of constituent particle i | Guo et al. [45] |
| Normal contact stiffness | Thornton and Yin [42] | |
| Tangential contact stiffness | Thornton and Yin [42] |
| Parameter | Value |
|---|---|
| Number of constituent particles | 5000 |
| 5 μm | |
| Agglomerate diameter | 105 μm |
| Constituent particle density, ρ | 1490 kg/m3 |
| Young’s modulus, E | 1 × 108 N/m2 |
| Poisson’s ratio, ν | 0.29 |
| Particle-particle friction coefficient | 0.3 |
| Particle-wall friction coefficient | 0.3 |
| Particle-particle coefficient of restitution | 0.95 |
| Particle-wall coefficient of restitution | 0.90 |
| Impact velocity, Vimp | 0.002–5 m/s |
| Constituent particle surface energy, γ | 1 × 10−7–1 J/m2 |
| Wall surface energy | 0 J/m2 |
| Time step | 1.3 × 10−8 s for Vimp = 0.002 m/s, 0.02 m/s 5.3 × 10−9 s for Vimp = 0.2 m/s and 0.5 m/s 1.1 × 10−9 s for Vimp = 1 m/s, 3 m/s, 5 m/s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, C.; Curtis, J.; Salari, K. Exploring the Regimes of Particle Behavior upon Impact via the Discrete Element Method. Pharmaceutics 2024, 16, 727. https://doi.org/10.3390/pharmaceutics16060727
Nguyen C, Curtis J, Salari K. Exploring the Regimes of Particle Behavior upon Impact via the Discrete Element Method. Pharmaceutics. 2024; 16(6):727. https://doi.org/10.3390/pharmaceutics16060727
Chicago/Turabian StyleNguyen, Chanh, Jennifer Curtis, and Kambiz Salari. 2024. "Exploring the Regimes of Particle Behavior upon Impact via the Discrete Element Method" Pharmaceutics 16, no. 6: 727. https://doi.org/10.3390/pharmaceutics16060727
APA StyleNguyen, C., Curtis, J., & Salari, K. (2024). Exploring the Regimes of Particle Behavior upon Impact via the Discrete Element Method. Pharmaceutics, 16(6), 727. https://doi.org/10.3390/pharmaceutics16060727







