Nearest Neighborhood Characteristics of a Tropical Mixed Broadleaved Forest Stand
Abstract
:1. Introduction
2. Materials and Methods
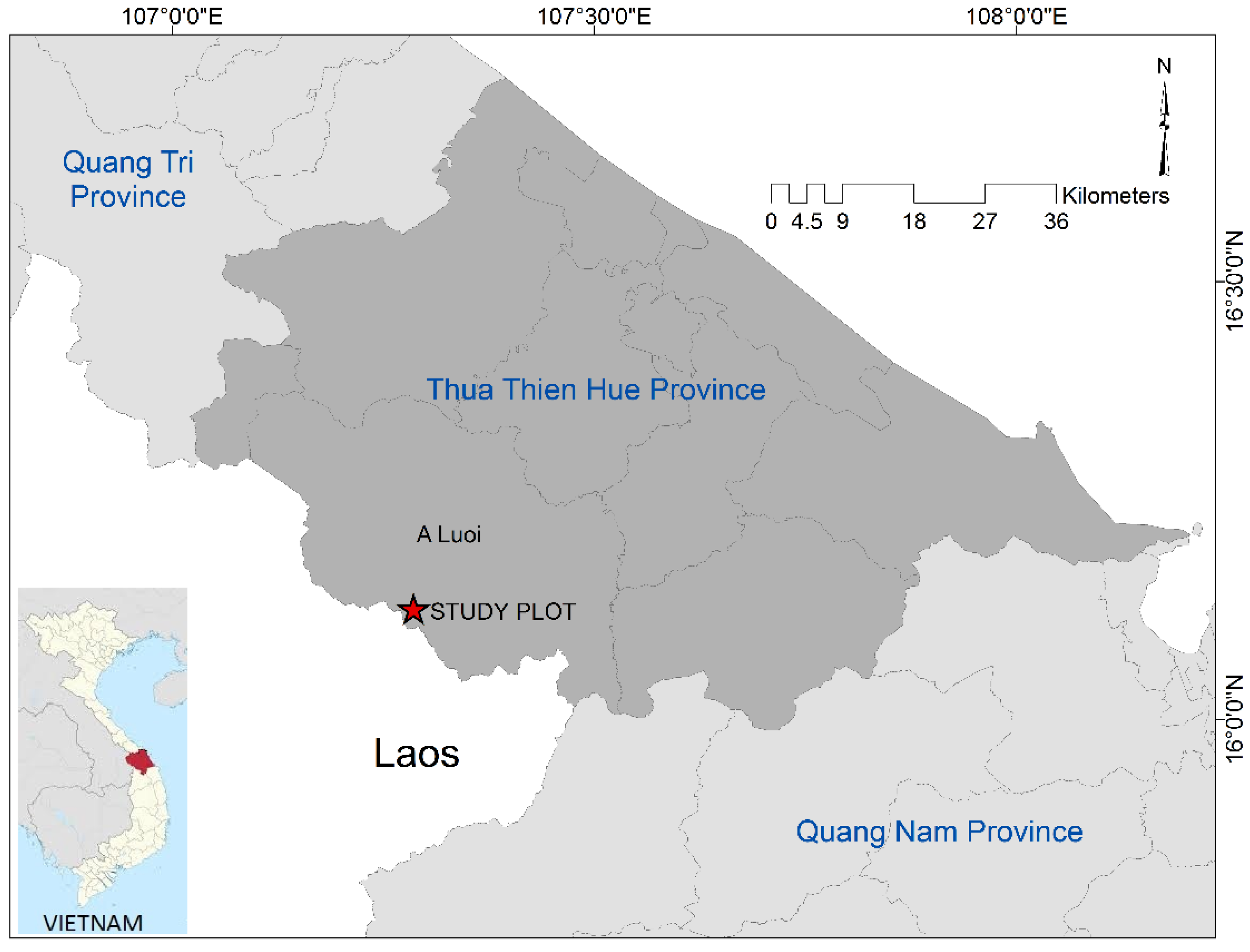
2.1. Study Site and Data Collection
2.2. Data Analysis
2.2.1. Analysis 1—Intraspecific Patterns
2.2.2. Analysis 2—Overall Interspecific Association Patterns
3. Results
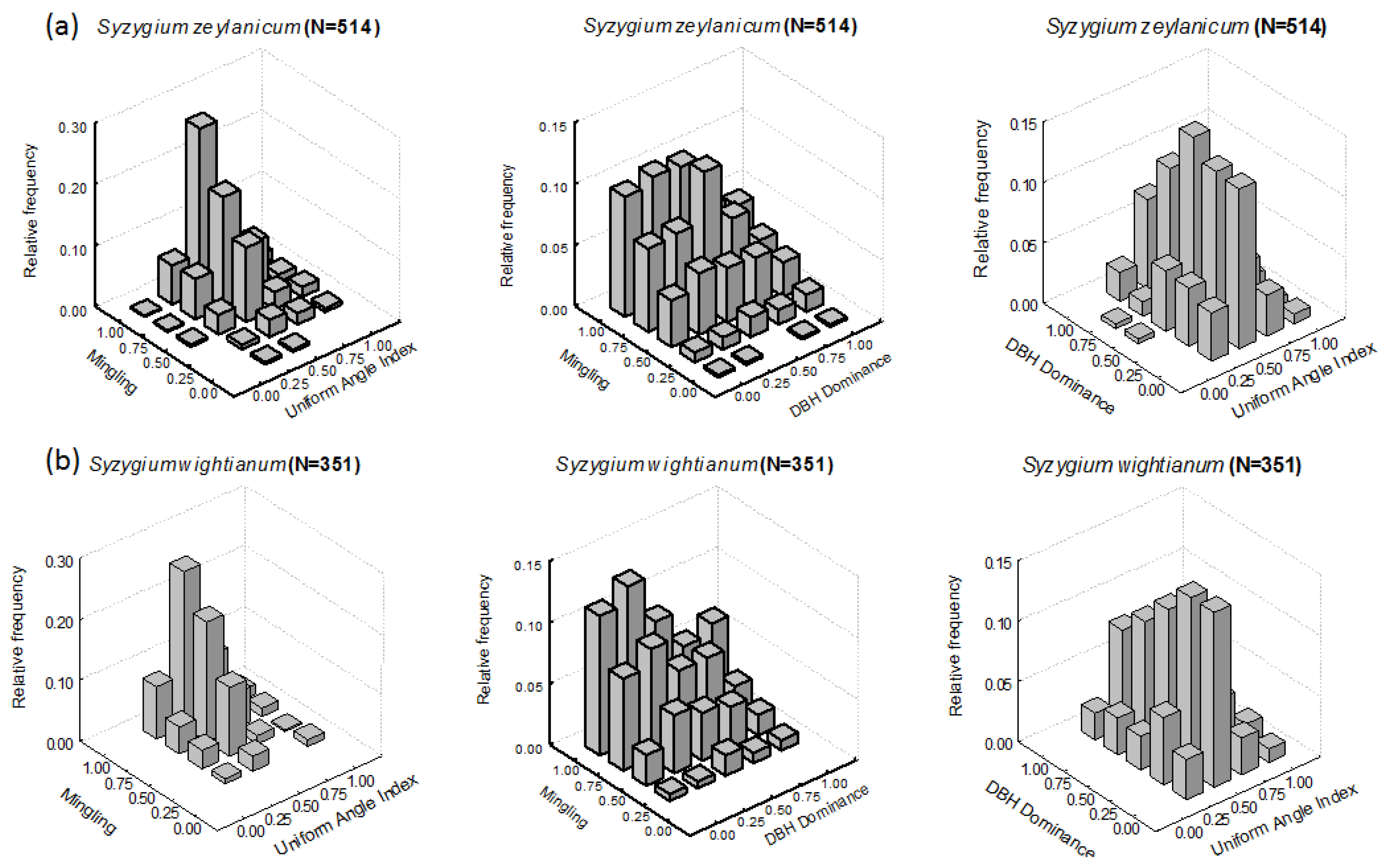
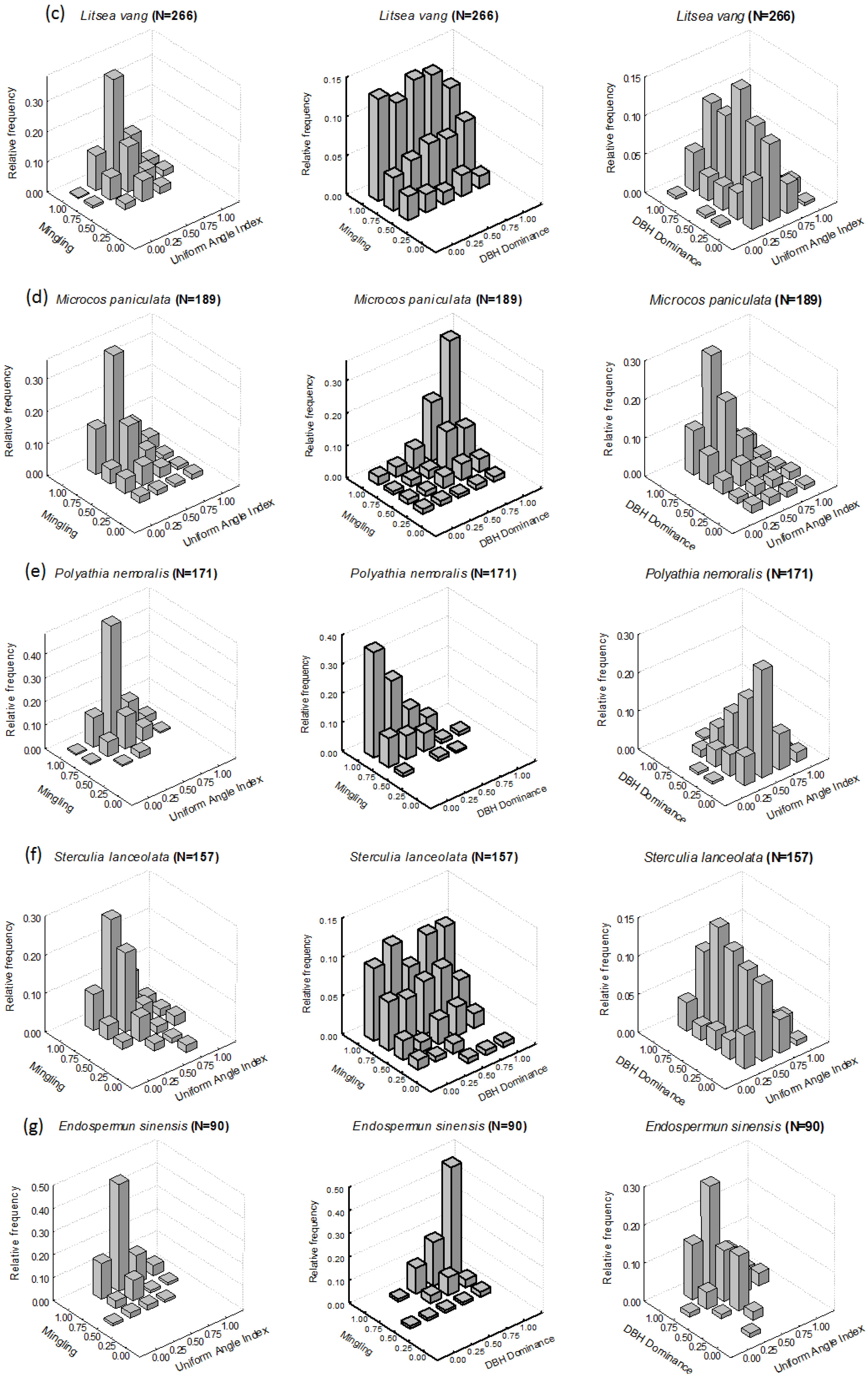
3.1. Intraspecific Patterns
3.1.1. M-W Bivariate Distribution
3.1.2. M-U Bivariate Distribution
3.1.3. U-W Bivariate Distribution
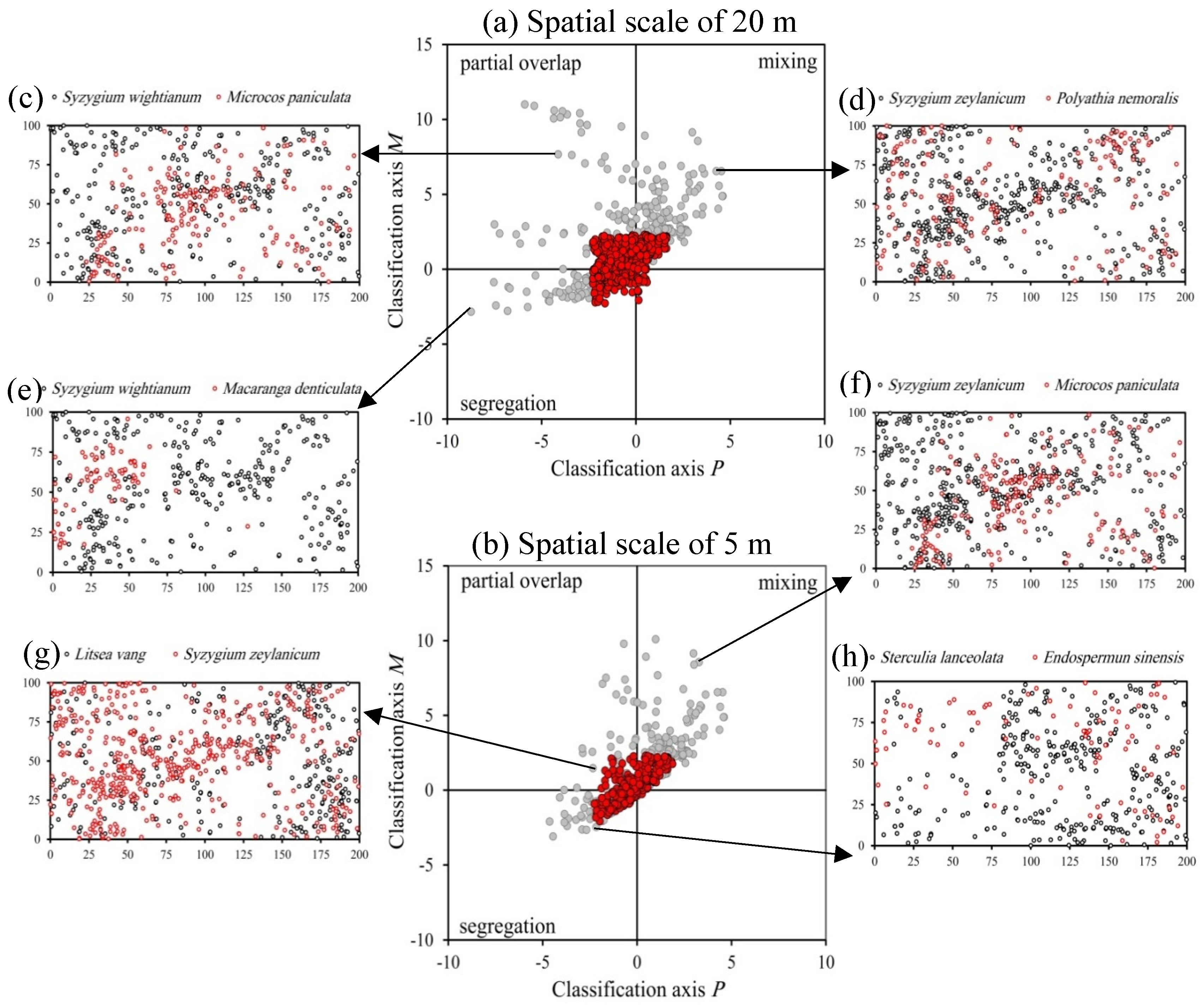
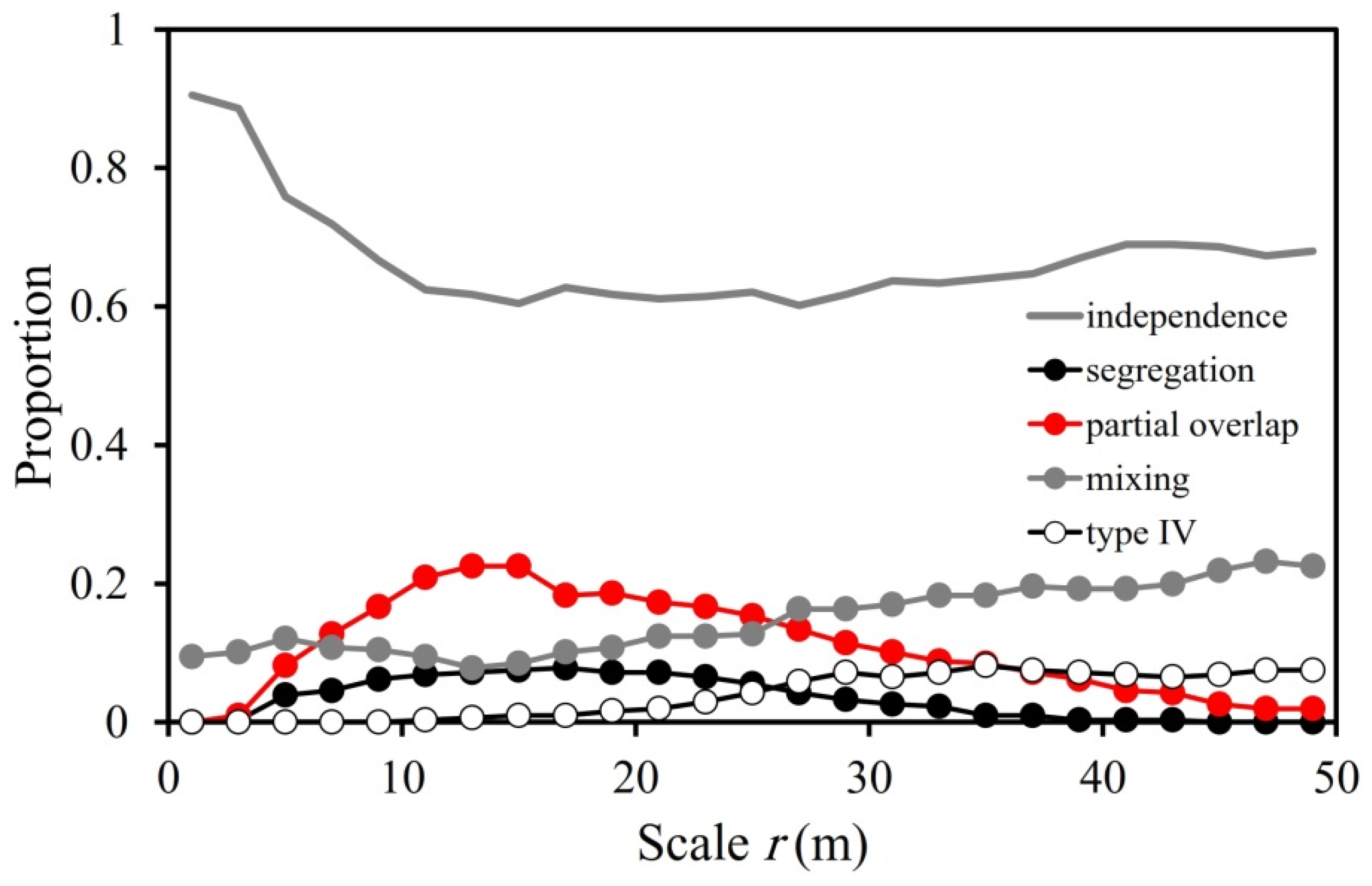
3.2. Overall Interspecific Association Patterns
4. Discussion
4.1. Intraspecific Patterns
4.2. Overall Interspecific Association Patterns
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ricklefs, R.; Miller, G. Ecology; W.H. Freeman: New York, NY, USA, 1990. [Google Scholar]
- Legendre, P.; Fortin, M.J. Spatial pattern and ecological analysis. Vegetatio 1989, 80, 107–138. [Google Scholar] [CrossRef]
- Wiegand, T.; Huth, A.; Getzin, S.; Wang, X.; Hao, Z.; Gunatilleke, C.S.; Gunatilleke, I.N. Testing the independent species’ arrangement assertion made by theories of stochastic geometry of biodiversity. Proc. R. Soc. B 2012. [Google Scholar] [CrossRef] [PubMed]
- Genet, A.; Pothier, D. Modeling tree spatial distributions after partial harvesting in uneven-aged boreal forests using inhomogeneous point processes. For. Ecol. Manag. 2013, 305, 158–166. [Google Scholar] [CrossRef]
- Wright, J.S. Plant diversity in tropical forests: A review of mechanisms of species coexistence. Oecologia 2002, 130, 1–14. [Google Scholar] [CrossRef] [PubMed]
- Stoll, P.; Prati, D. Intraspecific aggregation alters competitive interactions in experimental plant communities. Ecology 2001, 82, 319–327. [Google Scholar] [CrossRef]
- Monzeglio, U.; Stoll, P. Spatial patterns and species performances in experimental plant communities. Oecologia 2005, 145, 619–628. [Google Scholar] [CrossRef] [PubMed]
- Tilman, D. Competition and biodiversity in spatially structured habitats. Ecology 1994, 75, 2–16. [Google Scholar] [CrossRef]
- Stoll, P.; Weiner, J. A neighborhood view of interactions among individual plants. In The Geometry of Ecological Interactions: Simplifying Spatial Complexity; Cambridge University Press: Cambridge, UK, 2000; pp. 11–27. [Google Scholar]
- Getzin, S.; Wiegand, T.; Hubbell, S.P. Stochastically driven adult–recruit associations of tree species on Barro Colorado Island. Proc. Biol. Soc. 2014, 281, 20140922. [Google Scholar] [CrossRef] [PubMed]
- Janzen, D.H. Herbivores and the number of tree species in tropical forests. Am. Nat. 1970, 104, 501–528. [Google Scholar] [CrossRef]
- Connell, J. On the role of natural enemies in preventing competitive exclusion in some marine animals and in rain forest trees. In Dynamics of Population; Den Boer, P.J., Gradwell, G.R., Eds.; Pudoc: Wageningen, The Netherlands, 1971. [Google Scholar]
- Wiegand, T.; Gunatilleke, S.; Gunatilleke, N. Species associations in a heterogeneous Sri Lankan dipterocarp forest. Am. Nat. 2007, 170, E77–E95. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Liang, M.; Etienne, R.S.; Wang, Y.; Staehelin, C.; Yu, S. Experimental evidence for a phylogenetic Janzen-Connell effect in a subtropical forest. Ecol. Lett. 2012, 15, 111–118. [Google Scholar] [CrossRef] [PubMed]
- Chacón-Labella, J.; Cruz, M.; Escudero, A. Evidence for a stochastic geometry of biodiversity: The effects of species abundance, richness and intraspecific clustering. J. Ecol. 2017, 105, 382–390. [Google Scholar] [CrossRef]
- Wang, Q.; Bao, D.; Guo, Y.; Lu, J.; Lu, Z.; Xu, Y.; Zhang, K.; Liu, H.; Meng, H.; Jiang, M. Species associations in a species-rich subtropical forest were not well-explained by stochastic geometry of biodiversity. PLoS ONE 2014, 9, e97300. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Wiegand, T.; Kraft, N.J.; Swenson, N.G.; Davies, S.J.; Hao, Z.; Howe, R.W.; Lin, Y.; Ma, K.; Mi, X.; et al. Stochastic dilution effects weaken deterministic effects of niche-based processes in species rich forests. Ecology 2016, 97, 347–360. [Google Scholar] [CrossRef] [PubMed]
- McGill, B.J. Towards a unification of unified theories of biodiversity. Ecol. Lett. 2010, 13, 627–642. [Google Scholar] [CrossRef] [PubMed]
- Corral-Rivas, J.J.; Wehenkel, C.; Castellanos-Bocaz, H.A.; Vargas-Larreta, B.; Diéguez-Aranda, U. A permutation test of spatial randomness: Application to nearest neighbour indices in forest stands. J. For. Res. 2010, 15, 218–225. [Google Scholar] [CrossRef]
- Clark, P.J.; Evans, F.C. Distance to nearest neighbor as a measure of spatial relationships in populations. Ecology 1954, 35, 445–453. [Google Scholar] [CrossRef]
- Pielou, E. Mathematical Ecology, 2nd ed.; Wiley: Hoboken, NJ, USA, 1977. [Google Scholar]
- Diggle, P.J. Statistical Analysis of Spatial and Spatio-Temporal Point Patterns; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Ripley, B.D. Modelling spatial patterns. J. R. Stat. Soc. Ser. B. Methodol. 1977, 39, 172–212. [Google Scholar]
- Von Gadow, K.; Hui, G.; Albert, M. Das Winkelmaß-ein Strukturparameter zur Beschreibung der Individualverteilung in Waldbeständen. Centralblatt für das Gesamte Forstwesen 1998, 115, 1–9. [Google Scholar]
- Aguirre, O.; Hui, G.; von Gadow, K.; Jiménez, J. An analysis of spatial forest structure using neighbourhood-based variables. For. Ecol. Manag. 2003, 183, 137–145. [Google Scholar] [CrossRef]
- Hui, G.; Zhao, X.; Zhao, Z.; von Gadow, K. Evaluating tree species spatial diversity based on neighborhood relationships. For. Sci. 2011, 57, 292–300. [Google Scholar]
- Von Gadow, K.; Hui, G. Characterizing forest spatial structure and diversity. In Sustainable Forestry in Temperate Regions; Materiały Konferencyjne IUFRO: Lund, Sweden, 2002; pp. 20–30. [Google Scholar]
- Van Laar, A.; Akça, A. Forest Mensuration; Springer Science & Business Media: Dordrecht, The Netherlands, 2007; Volume 13. [Google Scholar]
- Von Gadow, K.; Zhang, C.Y.; Wehenkel, C.; Pommerening, A.; Corral-Rivas, J.; Korol, M.; Myklush, S.; Hui, G.Y.; Kiviste, A.; Zhao, X.H. Forest structure and diversity. In Continuous Cover Forestry; Springer: Berlin, Germany, 2012; pp. 29–83. [Google Scholar]
- Pommerening, A.; Gonçalves, A.C.; Rodríguez-Soalleiro, R. Species mingling and diameter differentiation as second-order characteristics. Allgemeine Forst Und Jagdzeitung 2011, 182, 115–129. [Google Scholar]
- Wiegand, T.; Moloney, K.A. Handbook of Spatial Point-Pattern Analysis in Ecology; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Pommerening, A.; Stoyan, D. Edge-correction needs in estimating indices of spatial forest structure. Can. J. For. Res. 2006, 36, 1723–1739. [Google Scholar] [CrossRef]
- Li, Y.; Hui, G.; Zhao, Z.; Hu, Y.; Ye, S. Spatial structural characteristics of three hardwood species in Korean pine broad-leaved forest—Validating the bivariate distribution of structural parameters from the point of tree population. For. Ecol. Manag. 2014, 314, 17–25. [Google Scholar] [CrossRef]
- Hubbell, S.P.; Foster, R.B. Biology, chance, and history and the structure of tropical rain forest tree communities. Community Ecol. 1986, 314–329. [Google Scholar]
- Hubbell, S.P. Neutral theory and the evolution of ecological equivalence. Ecology 2006, 87, 1387–1398. [Google Scholar] [CrossRef]
- Nguyen, H.H.; Uria-Diez, J.; Wiegand, K. Spatial distribution and association patterns in a tropical evergreen broad-leaved forest of north-central Vietnam. J. Veg. Sci. 2016, 27, 318–327. [Google Scholar] [CrossRef]
- Zhang, J.; Chen, L.; Guo, Q.; Nie, D.; Bai, X.; Jiang, Y. Research on changes of dominant tree population distribution patterns during developmental processes of a climax forest community. Acta Phytoecol. Sin. 1999, 23, 256–268. [Google Scholar]
- Raventós, J.; Wiegand, T.; Luis, M.D. Evidence for the spatial segregation hypothesis: A test with nine-year survivorship data in a Mediterranean shrubland. Ecology 2010, 91, 2110–2120. [Google Scholar] [CrossRef] [PubMed]

| Association Type | P(r) | M(r) | Description |
|---|---|---|---|
| Segregation | <0 | <0 | Individuals of species j occur consistently less around individuals of species i within neighbourhoods with spatial scale r than expected under independence |
| Partial overlap | <0 | ≥0 | Individuals of species j occur on average more often within neighbourhoods of individuals of species i than expected but a notable proportion of individuals of species i have less individuals of species j at their neighbourhood than expected |
| Mixing | ≥0 | ≥0 | Individuals of species j occur consistently more around individuals of species i within neighbourhoods with radius r than expected |
| Type IV | ≥0 | <0 | Individuals of species i are highly clustered and few individuals of species j are close to the species i clusters |
| No | Species | N | DBH (Mean ± SD) (cm) | IVI (%) | Mean | ||
|---|---|---|---|---|---|---|---|
| M | U | W | |||||
| 1 | Syzygium zeylanicum (L.) DC. * | 514 | 10.05 ± 8.01 | 10.44 | 0.77 | 0.44 | 0.51 |
| 2 | Syzygium wightianum Wall. ex Wight & Arn * | 351 | 9.54 ± 7.18 | 7.35 | 0.80 | 0.42 | 0.52 |
| 3 | Litsea vang Lecomte * | 266 | 12.30 ± 9.85 | 7.04 | 0.87 | 0.50 | 0.51 |
| 4 | Microcos paniculata L. * | 189 | 21.37 ± 6.93 | 7.07 | 0.86 | 0.81 | 0.50 |
| 5 | Polyalthia nemoralis Aug. DC. * | 171 | 5.61 ± 2.58 | 3.39 | 0.92 | 0.23 | 0.51 |
| 6 | Sterculia lanceolata Cav.* | 157 | 11.94 ± 6.84 | 4.22 | 0.80 | 0.49 | 0.54 |
| 7 | Diospyros eriantha Champ. ex Benth. | 112 | 5.33 ± 2.05 | 2.69 | 0.93 | 0.23 | 0.51 |
| 8 | Endospermun sinensis Benth. * | 90 | 25.26 ± 10.13 | 4.76 | 0.94 | 0.82 | 0.49 |
| 9 | Aphanamixis polystachya (Wall.) R.Parker | 81 | 14.79 ± 11.35 | 1.95 | 0.92 | 0.55 | 0.52 |
| 10 | Ardisia lindleyana D.Dietr. | 76 | 14.23 ± 5.90 | 3.18 | 0.81 | 0.67 | 0.55 |
| 11 | Macaranga denticulata (Blume) Müll.Arg. | 79 | 3.78 ± 0.72 | 1.70 | 0.85 | 0.14 | 0.53 |
| 12 | Schefflera octophylla (Lour.) Harms | 71 | 14.43 ± 11.37 | 2.67 | 0.92 | 0.55 | 0.48 |
| 13 | Nephelium melliferum Gagnep. | 64 | 19.53 ± 14.52 | 4.31 | 0.94 | 0.71 | 0.49 |
| 14 | Quercus platicalyx Hickel & A. Camus | 63 | 29.96 ± 12.68 | 3.32 | 0.91 | 0.84 | 0.48 |
| 15 | Dillenia scabrella (D.Don) Roxb. ex Wall. | 62 | 14.32 ± 11.58 | 1.74 | 0.89 | 0.48 | 0.51 |
| 16 | Adina pilulifera (Lam.) Franch. ex Drake | 61 | 8.9 ± 4.93 | 2.46 | 0.89 | 0.42 | 0.57 |
| 17 | Archidendron balansae (Oliv.) I.C.Nielsen | 54 | 12.35 ± 6.76 | 1.98 | 0.93 | 0.51 | 0.52 |
| 18 | Ormosia balansae Drake | 50 | 29.71 ± 16.42 | 3.53 | 0.95 | 0.86 | 0.49 |
| 19 | 62 others | 643 | 13.76 ± 10.94 | 26.11 | |||
| All trees | 3154 | 12.53 | 100 | ||||
| Spatial Scales | Types of Association | |||
|---|---|---|---|---|
| Segregation (%) | Partial Overlap (%) | Mixing (%) | Type IV (%) | |
| Up to 5 m | 1.4 | 2.5 | 11.5 | 0.0 |
| Up to 20 m | 5.5 | 14.6 | 10.3 | 0.5 |
| Up to 50 m | 3.5 | 10.8 | 15.0 | 4.0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, H.H.; Erfanifard, Y.; Petritan, I.C. Nearest Neighborhood Characteristics of a Tropical Mixed Broadleaved Forest Stand. Forests 2018, 9, 33. https://doi.org/10.3390/f9010033
Nguyen HH, Erfanifard Y, Petritan IC. Nearest Neighborhood Characteristics of a Tropical Mixed Broadleaved Forest Stand. Forests. 2018; 9(1):33. https://doi.org/10.3390/f9010033
Chicago/Turabian StyleNguyen, Hong Hai, Yousef Erfanifard, and Ion Catalin Petritan. 2018. "Nearest Neighborhood Characteristics of a Tropical Mixed Broadleaved Forest Stand" Forests 9, no. 1: 33. https://doi.org/10.3390/f9010033
APA StyleNguyen, H. H., Erfanifard, Y., & Petritan, I. C. (2018). Nearest Neighborhood Characteristics of a Tropical Mixed Broadleaved Forest Stand. Forests, 9(1), 33. https://doi.org/10.3390/f9010033






