Investigating the Potential of Transparent Parallel-Arranged Micro-Perforated Panels (MPPs) as Sound Absorbers in Classrooms
Abstract
1. Introduction
2. Materials and Methods
2.1. Theoretical Background
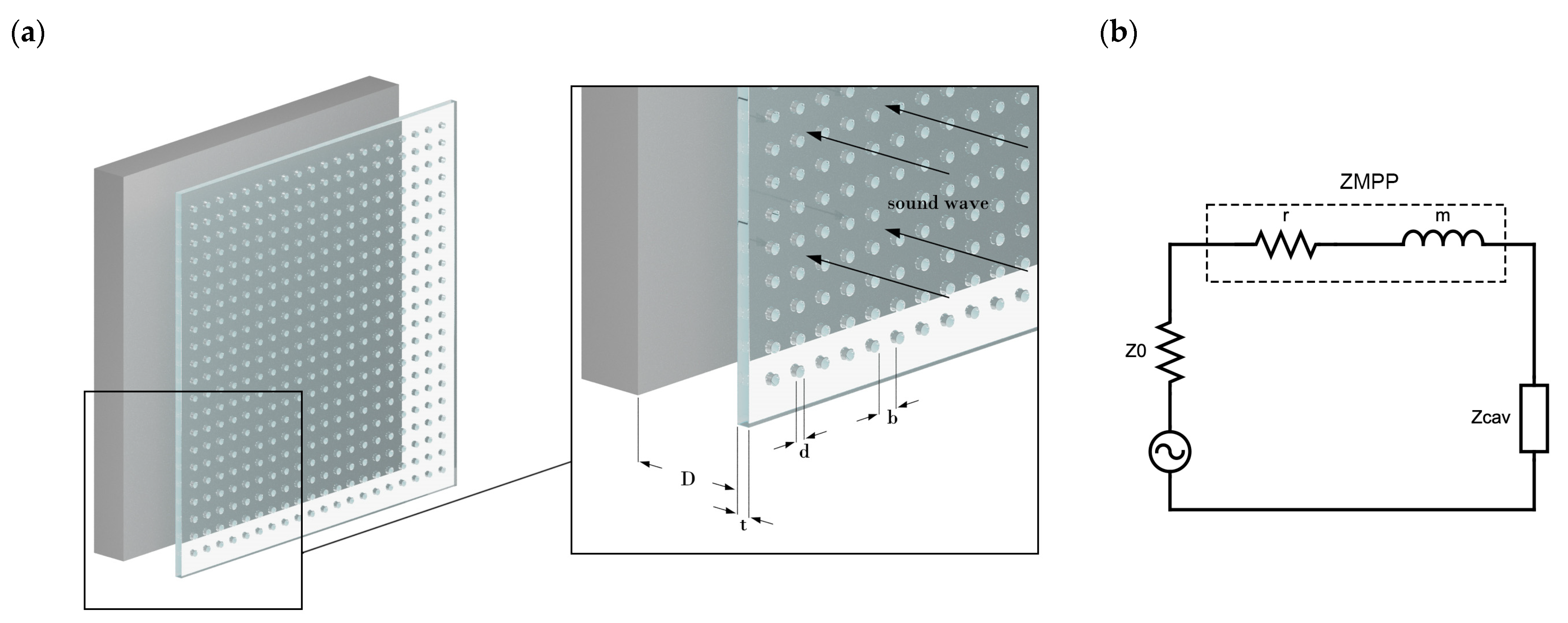
2.1.1. Acoustic Impedance of MPPs According to ECM
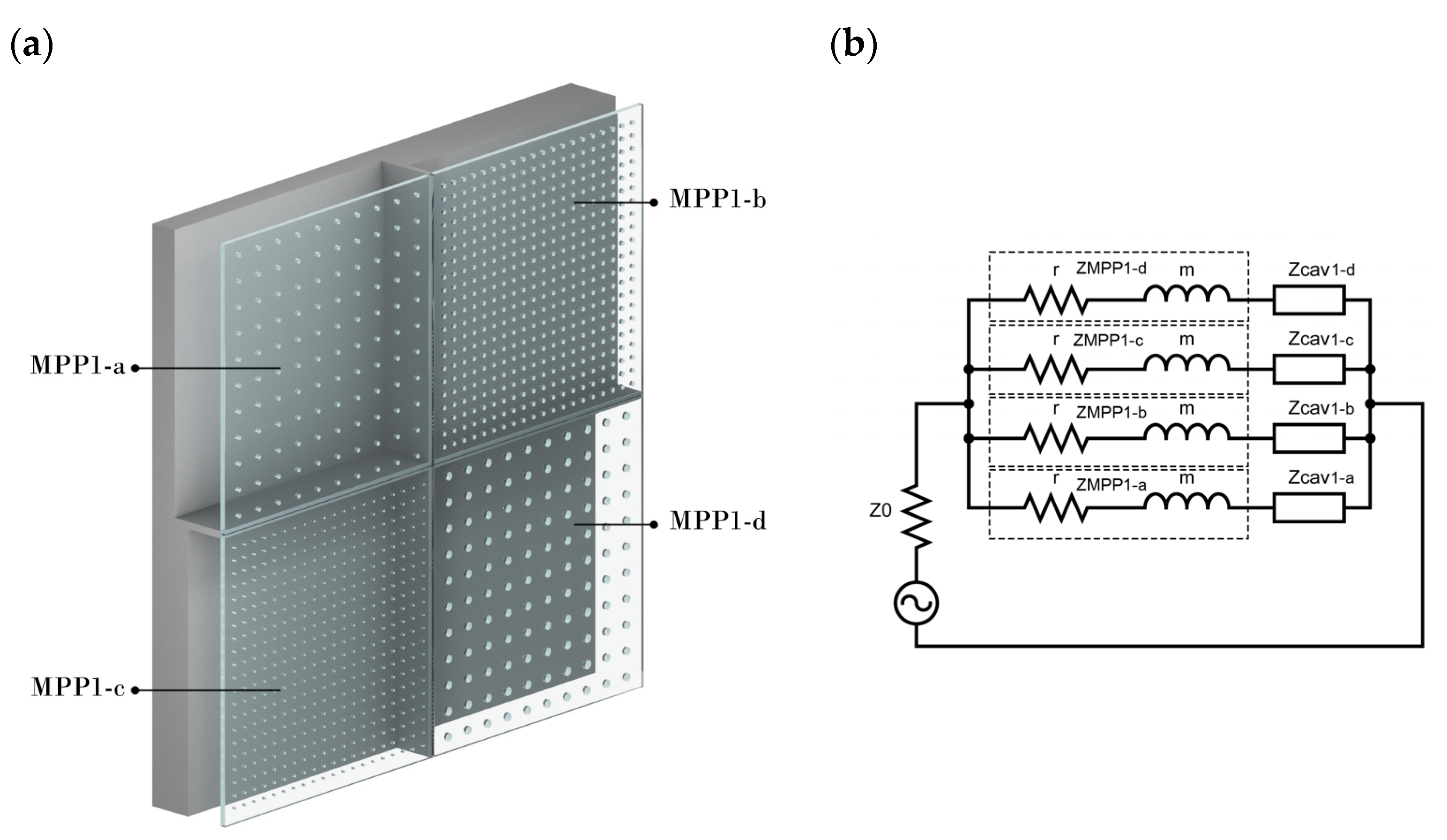
2.1.2. Parallel-Arranged MPPs (Prototype 1)
2.1.3. Double-Layer Parallel-Arranged MPPs (Prototype 2)
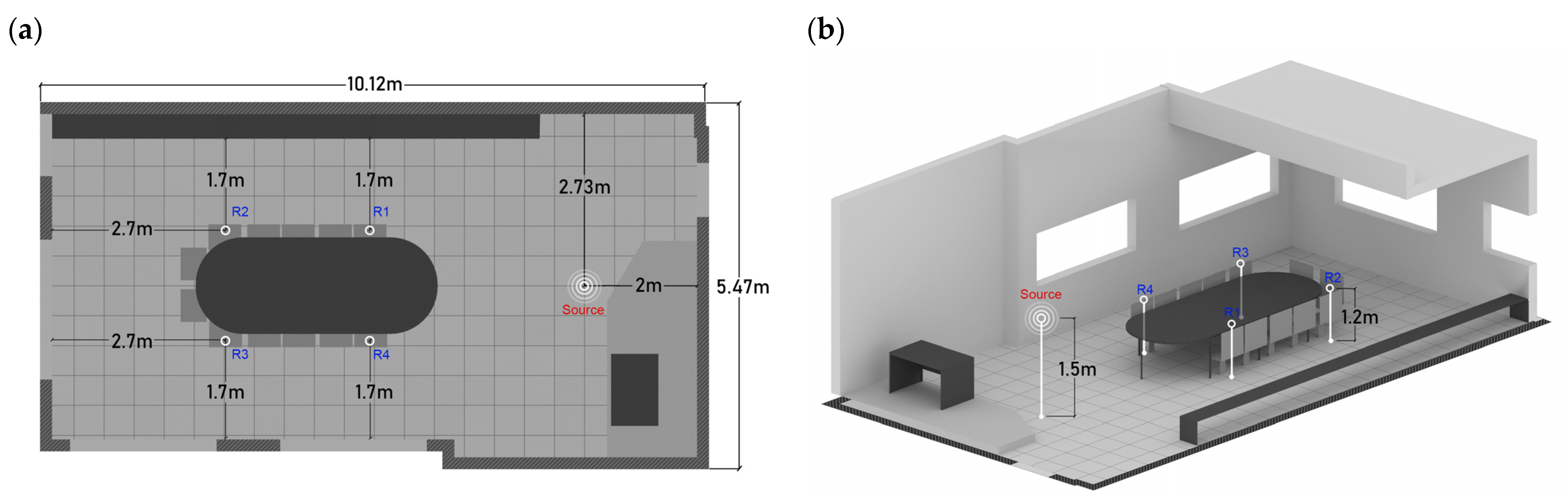
2.2. Experimental Setup
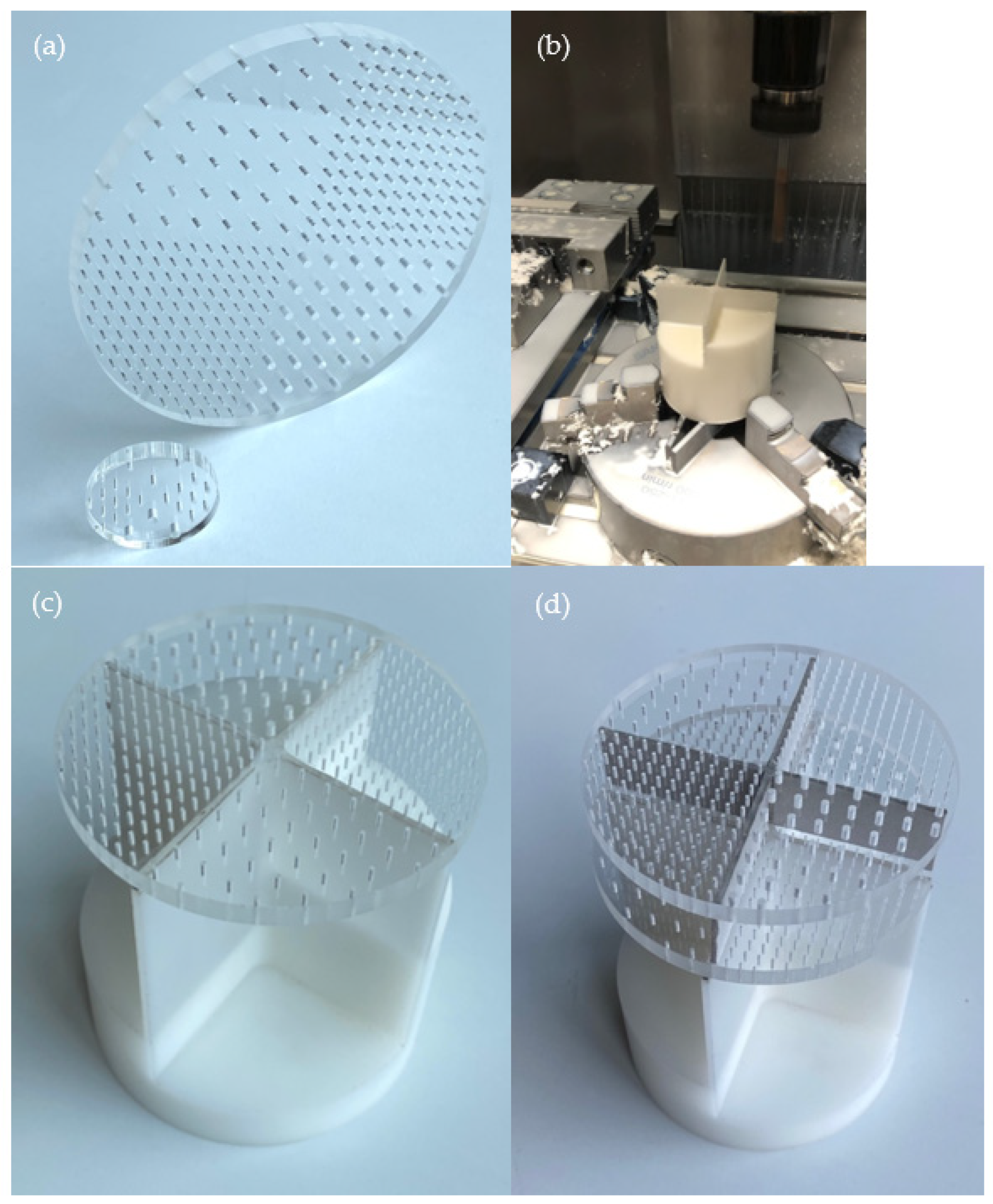
2.2.1. Production of Samples
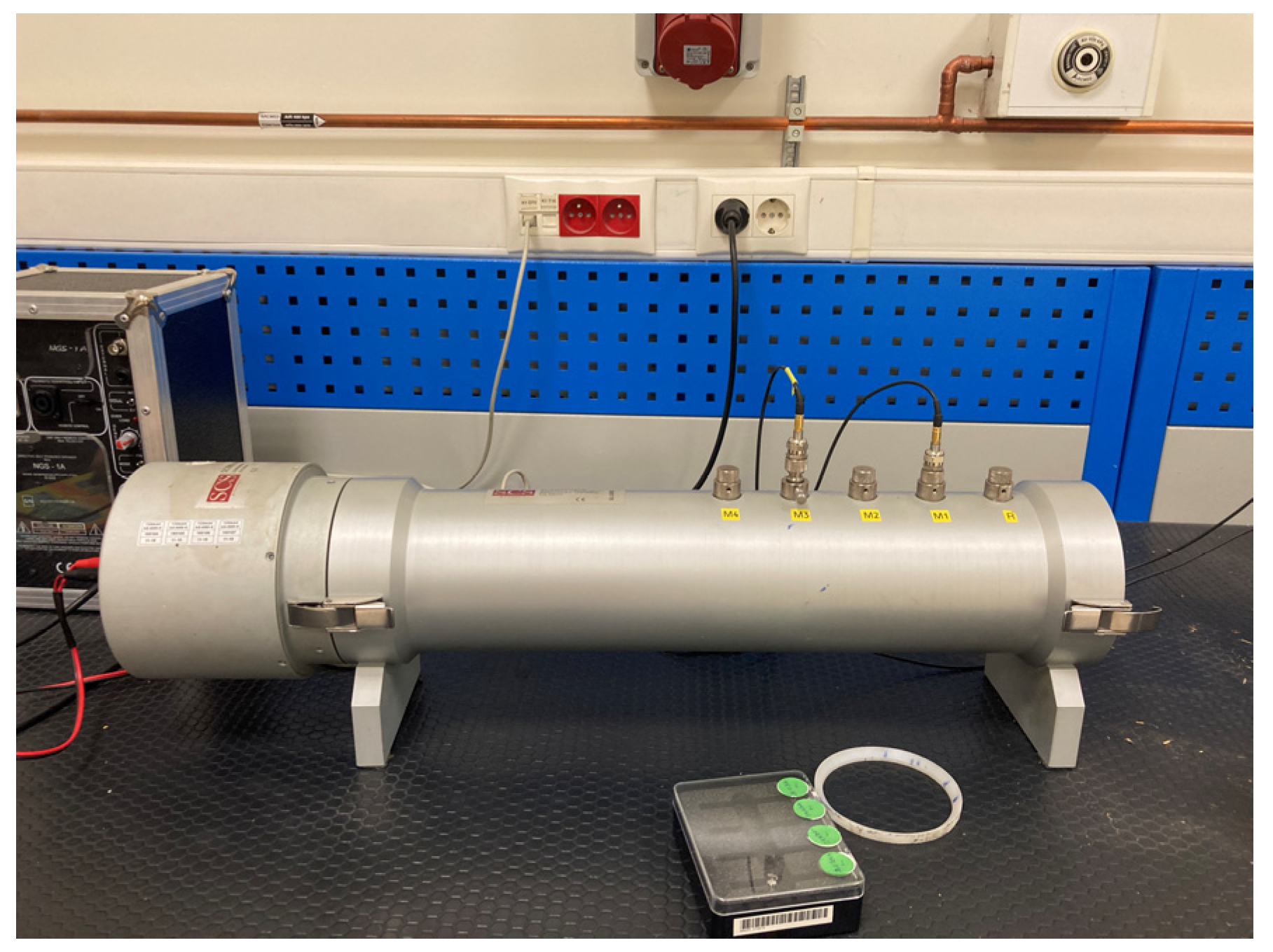
2.2.2. Assessment of Absorption Coefficient
2.3. Case Study
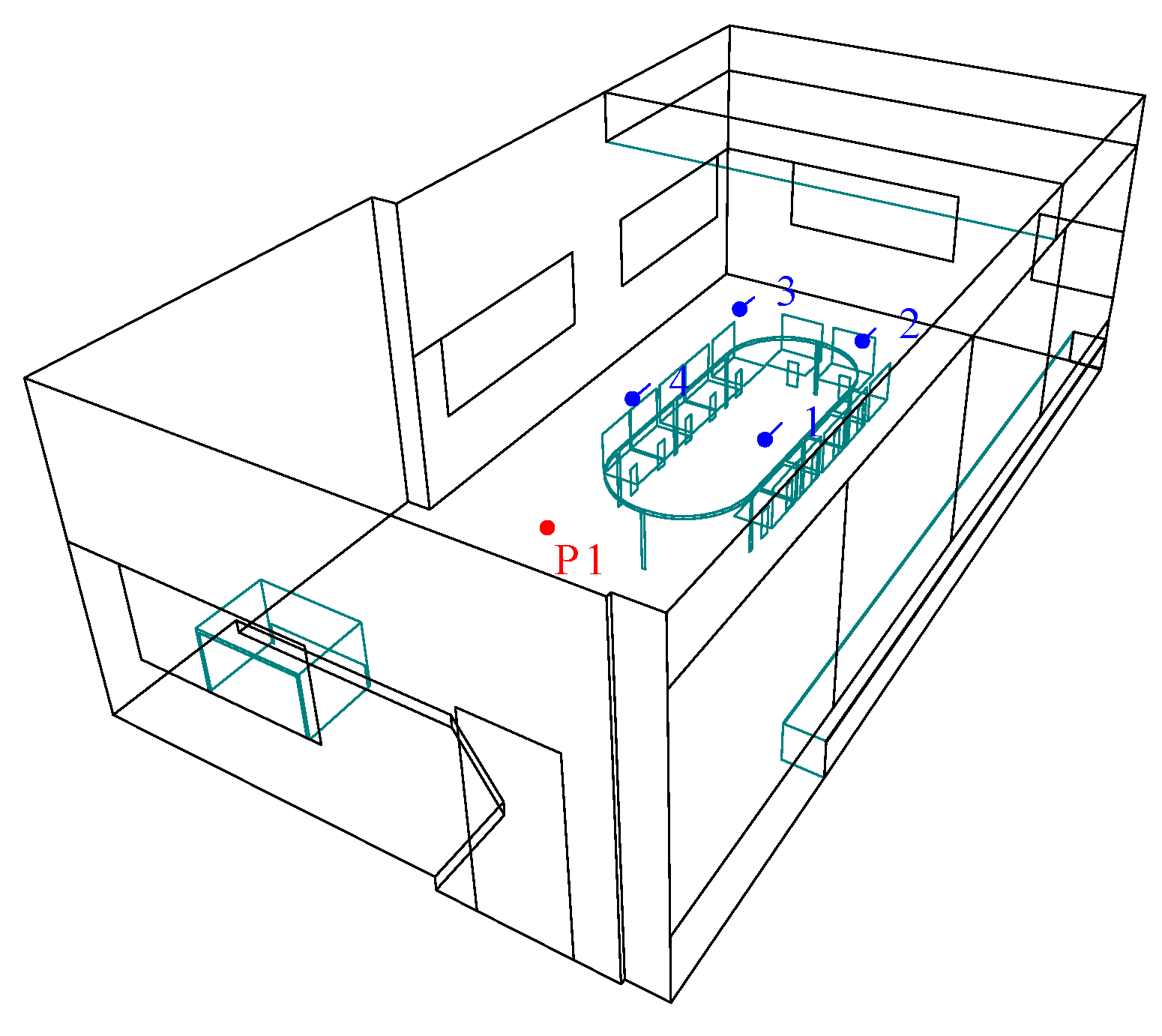
2.3.1. In Situ Measurements
2.3.2. Numerical Simulations and Calibration of Models
3. Results
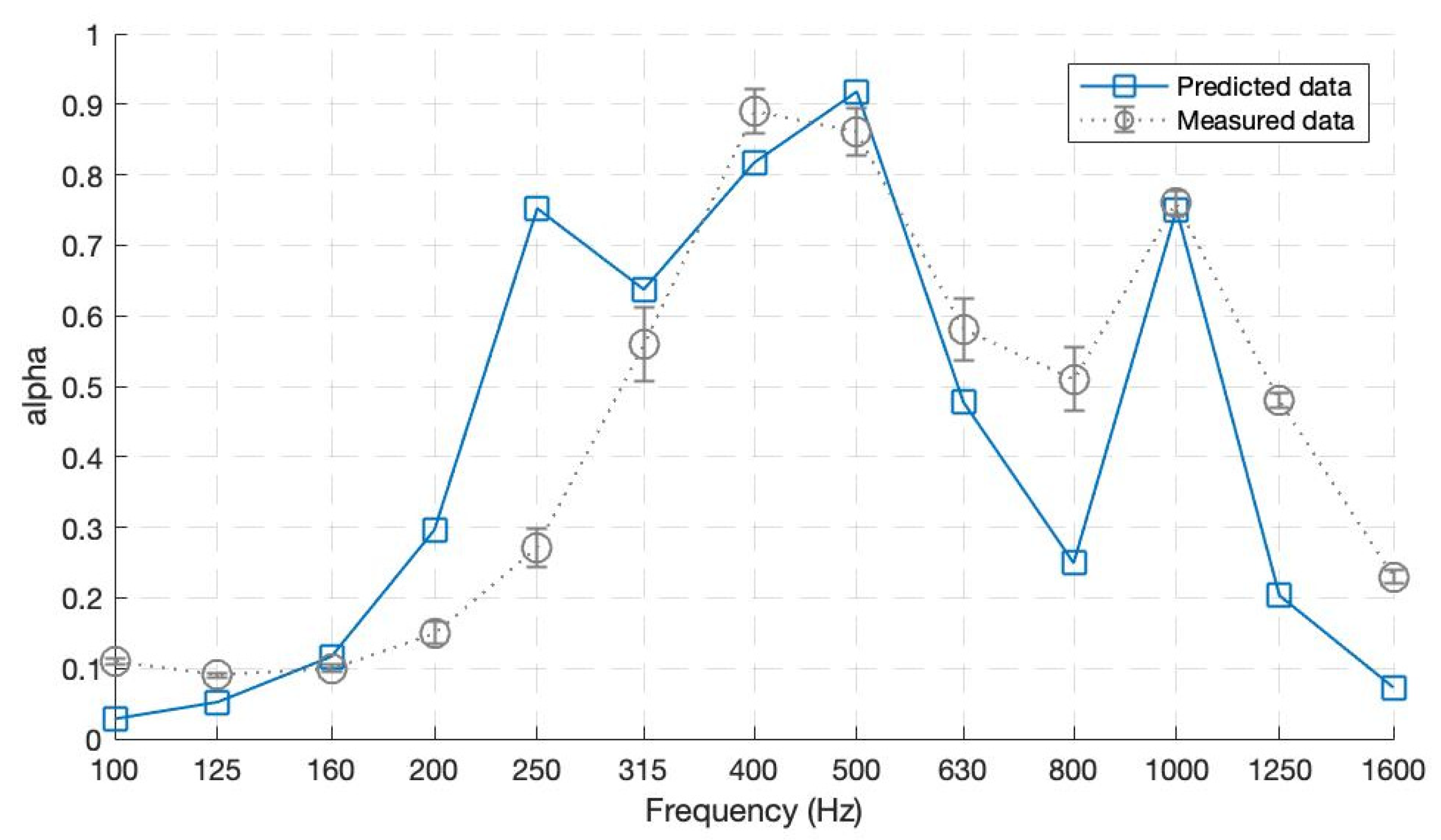
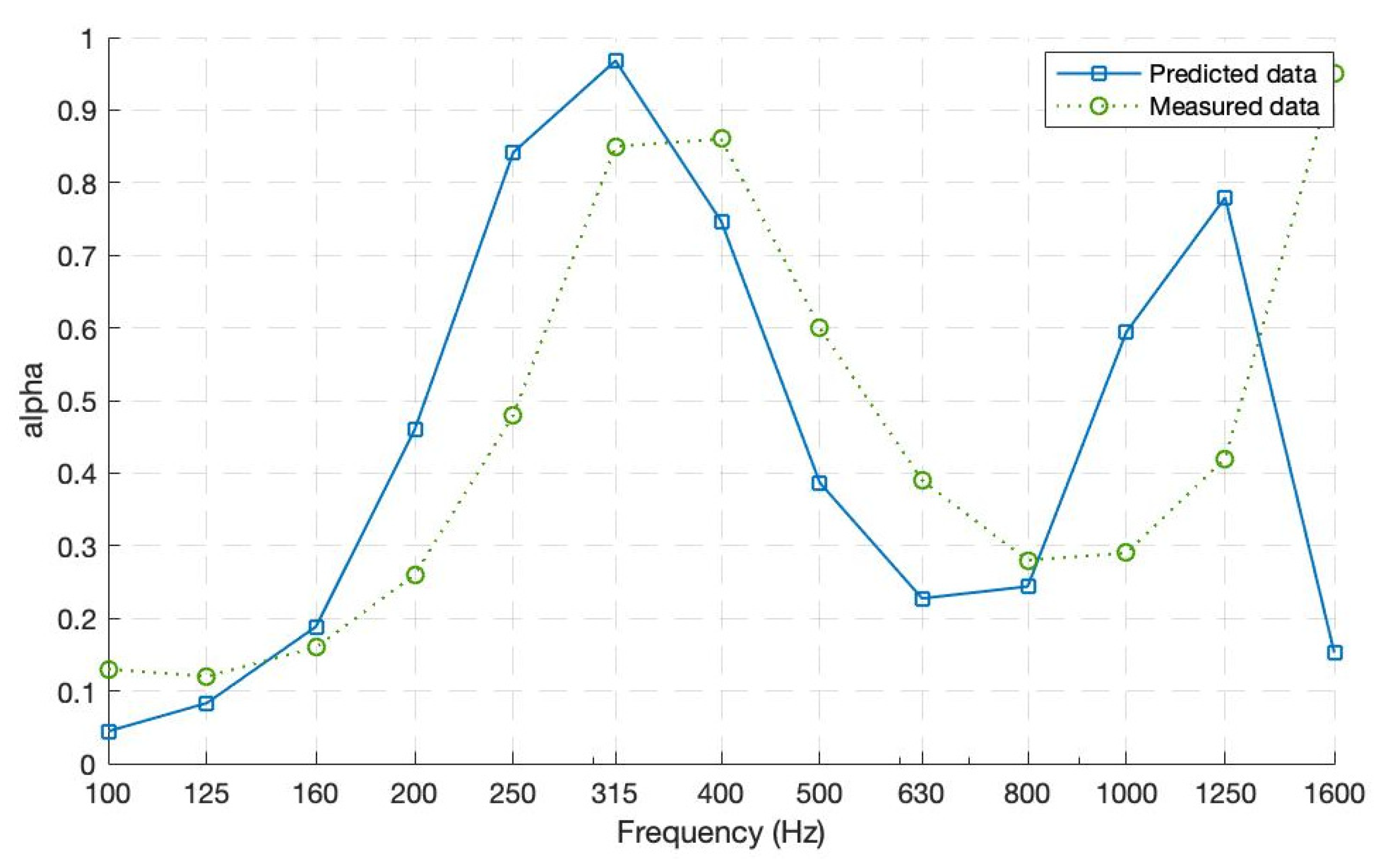
3.1. Impedance Tube Results
- Prototype 1—One-layer parallel-arranged MPPs
- Prototype 2—Double-layer parallel-arranged MPPs
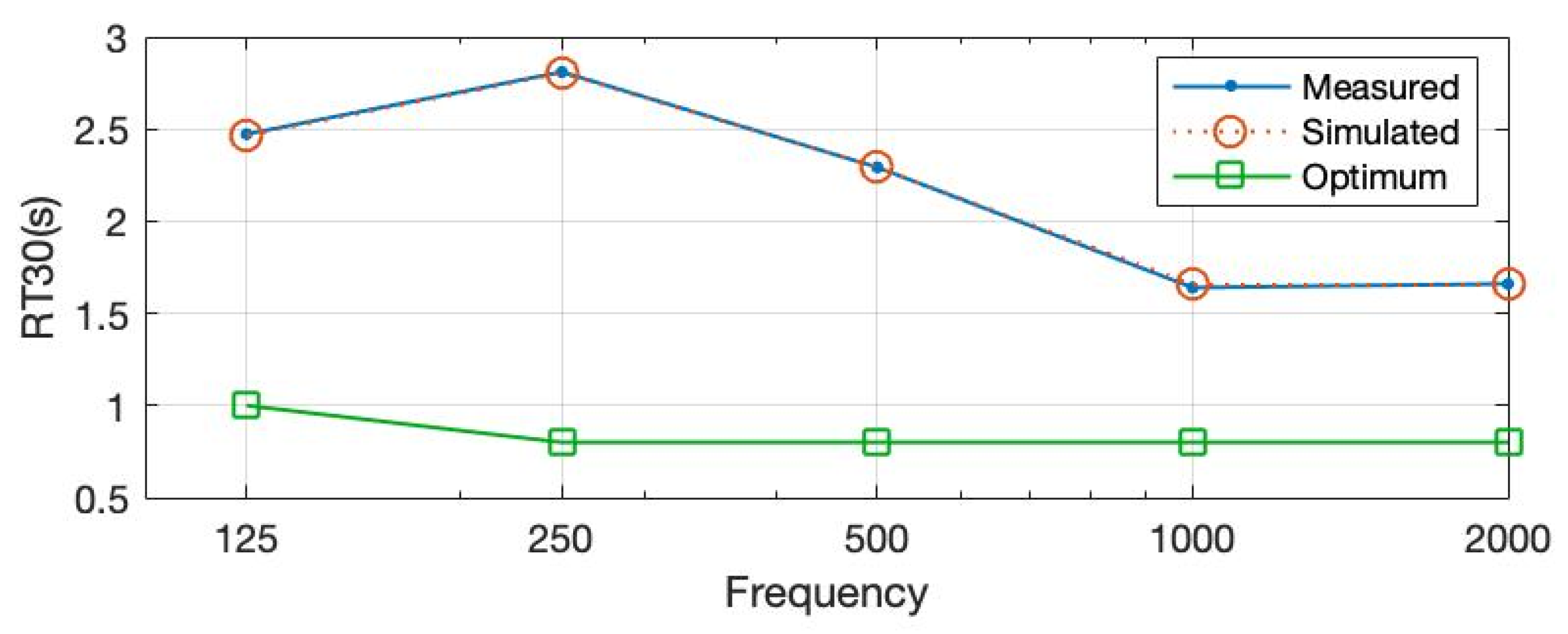
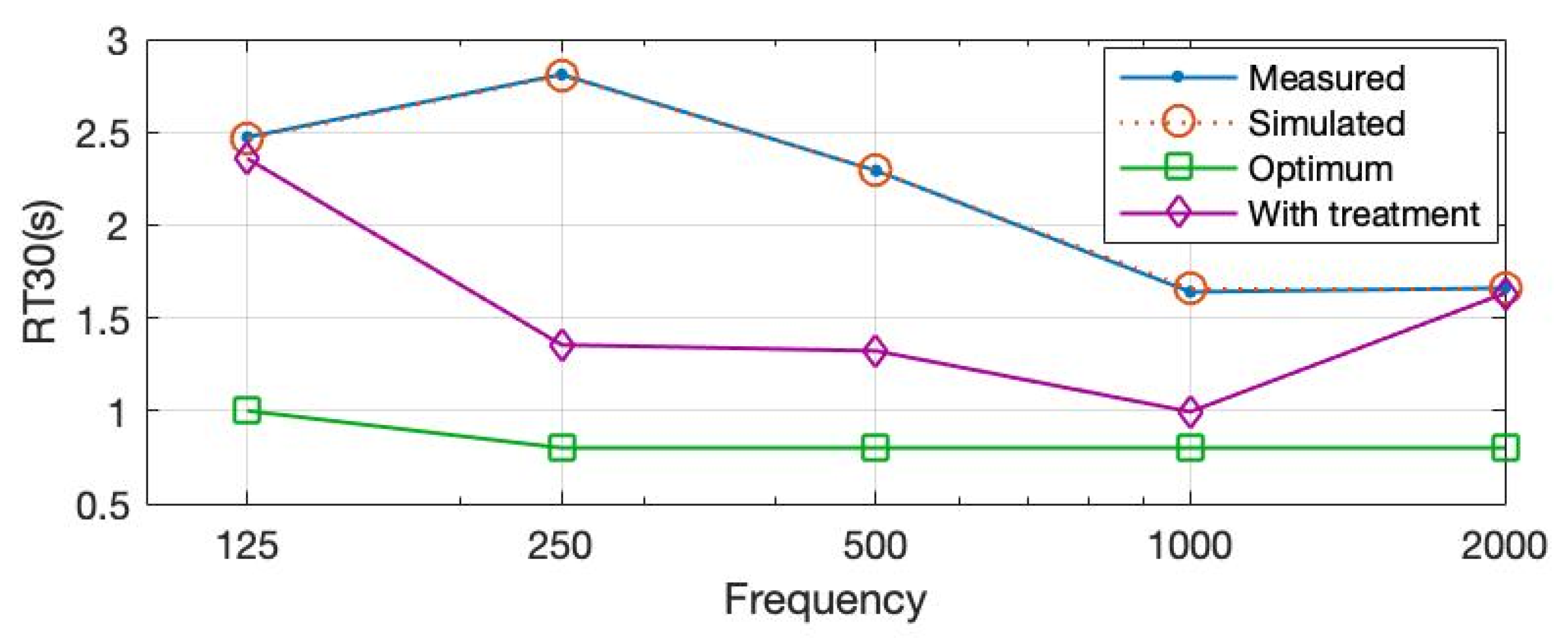
3.2. Geometrical Acoustics Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fuchs, H. Applied Acoustics: Concepts, Absorbers, and Silencers for Acoustical Comfort and Noise Control: Alternative Solutions—Innovative Tools—Practical Examples; Springer: Berlin/Heidelberg, Germany, 2013; Volume 9783642293672, ISBN 9783642293672. [Google Scholar]
- Cowan, J.P. Handbook of Environmental Acoustics; Wiley: New York, NY, USA, 1994. [Google Scholar]
- Antoniadou, P.; Papadopoulos, A.M. Occupants’ Thermal Comfort: State of the Art and the Prospects of Personalized Assessment in Office Buildings. Energy Build. 2017, 153, 136–149. [Google Scholar] [CrossRef]
- Puglisi, G.E.; Warzybok, A.; Astolfi, A.; Kollmeier, B. Effect of Reverberation and Noise Type on Speech Intelligibility in Real Complex Acoustic Scenarios. Build. Environ. 2021, 204, 108137. [Google Scholar] [CrossRef]
- Çankaya Topak, S.; Yılmazer, S. A Comparative Study on Indoor Soundscape Assessment via a Mixed Method: A Case of the High School Environment. Appl. Acoust. 2022, 189, 108554. [Google Scholar] [CrossRef]
- Jablonska, J. Architectural Acoustics and Speech Legibility in University Environment—Case Study. Appl. Acoust. 2021, 177, 107917. [Google Scholar] [CrossRef]
- Yang, D.; Mak, C.M. Effects of Acoustical Descriptors on Speech Intelligibility in Hong Kong Classrooms. Appl. Acoust. 2021, 171, 107678. [Google Scholar] [CrossRef]
- Sticca, F.; Goetz, T.; Bieg, M.; Hall, N.C.; Eberle, F.; Haag, L. Examining the Accuracy of Students’ Self-Reported Academic Grades from a Correlational and a Discrepancy Perspective: Evidence from a Longitudinal Study. PLoS ONE 2017, 12, e0187367. [Google Scholar] [CrossRef]
- Bottalico, P.; Murgia, S.; Puglisi, G.E.; Astolfi, A.; Kirk, K.I. Effect of Masks on Speech Intelligibility in Auralized Classrooms). J. Acoust. Soc. Am. 2020, 148, 2878. [Google Scholar] [CrossRef]
- Valente, D.L.; Plevinsky, H.M.; Franco, J.M. Experimental Investigation of the Effects of the Acoustical Conditions in a Simulated Classroom on Speech Recognition and Learning in Children. J. Acoust. Soc. Am. 2012, 131, 232. [Google Scholar] [CrossRef]
- Klatte, M.; Hellbruelr’, J.; Seidel, J.; Leistner, P. Effects of Classroom Acoustics on Performance and Well-Being in Elementary School Children: A Field Study. Environ. Behav. 2017, 42, 659–692. [Google Scholar] [CrossRef]
- Shield, B.M.; Dockrell, J.E. The Effects of Environmental and Classroom Noise on the Academic Attainments of Primary School Children. J. Acoust. Soc. Am. 2008, 123, 133. [Google Scholar] [CrossRef]
- Klatte, M.; Meis, M.; Sukowski, H.; Schick, A. Noise and Health. Noise Health 2007, 9, 64. [Google Scholar] [CrossRef] [PubMed]
- Szalma, J.L.; Hancock, P.A. Noise Effects on Human Performance: A Meta-Analytic Synthesis. Psychol. Bull. 2011, 137, 682–707. [Google Scholar] [CrossRef] [PubMed]
- Minelli, G.; Puglisi, G.E.; Astolfi, A. Acoustical Parameters for Learning in Classroom: A Review. Build. Environ. 2022, 208, 108582. [Google Scholar] [CrossRef]
- Daniels, R.; Bodkin, A. Acoustic Design of Schools: Performance Standards. Build. Bull. 2015, 93, 1–43. [Google Scholar]
- ANSI S12.60-2002—Acoustical Performance Criteria, Design Requirements, and Guidelines for Schools. Available online: https://webstore.ansi.org/Standards/ASA/ansis12602002 (accessed on 10 October 2022).
- UNI 11532-2:2020—Caratteristiche Acustiche Interne Di Ambienti Confinati—Metodi Di Progettazione e Tecniche Di Valutazione—Parte 2: Settore Scolastico. Available online: https://store.uni.com/uni-11532-2-2020 (accessed on 10 October 2022).
- Programma van Eisen Frisse Scholen; The Netherlands. 2015. Available online: https://www.rvo.nl/sites/default/files/2016/01/Programma%20van%20Eisen%20Frisse%20Scholen%20-%20September%202015%20v3.pdf (accessed on 10 October 2022).
- Çevresel Gürültünün Değerlendirilmesi ve Yönetimi Yönetmeliği. 2022. Available online: https://www.mevzuat.gov.tr/mevzuat?MevzuatNo=14012&MevzuatTur=7&MevzuatTertip=5 (accessed on 10 October 2022).
- Astolfi, A.; Bottalico, P.; Barbato, G. Subjective and Objective Speech Intelligibility Investigations in Primary School Classrooms. J. Acoust. Soc. Am. 2012, 131, 247. [Google Scholar] [CrossRef]
- Astolfi, A.; Carullo, A.; Pavese, L.; Puglisi, G.E. Duration of Voicing and Silence Periods of Continuous Speech in Different Acoustic Environments. J. Acoust. Soc. Am. 2015, 137, 565. [Google Scholar] [CrossRef]
- Zhang, D.; Tenpierik, M.; Bluyssen, P.M. Individual Control as a New Way to Improve Classroom Acoustics: A Simulation-Based Study. Appl. Acoust. 2021, 179, 108066. [Google Scholar] [CrossRef]
- Cox, T.J.; D’Antonio, P. Acoustic Absorbers and Diffusers: Theory, Design and Application, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2017; ISBN 9781498740999. [Google Scholar]
- Schmitz, A. Raumakustik—Planungsgrundlagen und Sanierungsvorschläge. In Lärmminderung in Schulen; Hessisches Landesamt für Umwelt und Geologie: Weisbaden, Germany, 2007; Volume 4, pp. 31–51. [Google Scholar]
- Kumar, S.; Lee, H. The Present and Future Role of Acoustic Metamaterials for Architectural and Urban Noise Mitigations. Acoustics 2019, 1, 35. [Google Scholar] [CrossRef]
- Qiu, X. Principles of Sound Absorbers. In Acoustic Textiles; Springer: Singapore, 2016; pp. 43–72. [Google Scholar]
- Yang, T.; Hu, L.; Xiong, X.; Petrů, M.; Noman, M.T.; Mishra, R.; Militký, J. Sound absorption properties of natural fibers: A review. Sustainability 2020, 12, 8477. [Google Scholar] [CrossRef]
- Arenas, J.P.; Sakagami, K. Sustainable Acoustic Materials. Sustainability 2020, 12, 6540. [Google Scholar] [CrossRef]
- Tang, X.; Yan, X. Acoustic energy absorption properties of fibrous materials: A review. Compos. Part A Appl. Sci. Manuf. 2017, 101, 360–380. [Google Scholar] [CrossRef]
- Maa, D. Theory and Design of Microperforated Panel Sound-Absorbing Constructions. Scient. Sin. 1975, 18, 55–71. [Google Scholar]
- Adams, T. Sound Materials: A Compendium of Sound Absorbing Materials for Architecture and Design; Frame Publishers: Amsterdam, The Netherlands, 2016; ISBN 9492311011. [Google Scholar]
- Maa, D. Potential of Microperforated Panel Absorber. J. Acoust. Soc. Am. 1988, 104, 2861–2866. [Google Scholar] [CrossRef]
- Bucciarelli, F.; Malfense Fierro, G.P.; Meo, M. A Multilayer Microperforated Panel Prototype for Broadband Sound Absorption at Low Frequencies. Appl. Acoust. 2019, 146, 134–144. [Google Scholar] [CrossRef]
- Cobo, P.; de la Colina, C.; Roibás-Millán, E.; Chimeno, M.; Simón, F. A Wideband Triple-Layer Microperforated Panel Sound Absorber. Compos. Struct. 2019, 226. [Google Scholar] [CrossRef]
- Yang, W.; Bai, X.; Zhu, W.; Kiran, R.; An, J.; Chua, C.K.; Zhou, K. 3D Printing of Polymeric Multi-Layer Micro-Perforated Panels for Tunable Wideband Sound Absorption. Polymers 2020, 12, 360. [Google Scholar] [CrossRef]
- Sakagami, K.; Nakamori, T.; Morimoto, M.; Yairi, M. Double-Leaf Microperforated Panel Space Absorbers: A Revised Theory and Detailed Analysis. Appl. Acoust. 2009, 70, 703–709. [Google Scholar] [CrossRef]
- Li, X.; Wu, Q.; Kang, L.; Liu, B. Design of Multiple Parallel-Arranged Perforated Panel Absorbers for Low Frequency Sound Absorption. Materials 2019, 12, 2099. [Google Scholar] [CrossRef]
- Wang, C.; Huang, L. On the Acoustic Properties of Parallel Arrangement of Multiple Micro-Perforated Panel Absorbers with Different Cavity Depths. J. Acoust. Soc. Am. 2011, 130, 208. [Google Scholar] [CrossRef]
- Mosa, A.I.; Putra, A.; Ramlan, R.; Prasetiyo, I.; Esraa, A.A. Theoretical Model of Absorption Coefficient of an Inhomogeneous MPP Absorber with Multi-Cavity Depths. Appl. Acoust. 2019, 146, 409–419. [Google Scholar] [CrossRef]
- Prasetiyo, I.; Sihar, I.; Sudarsono, A.S. Realization of a Thin and Broadband Microperforated Panel (MPP) Sound Absorber. Appl. Acoust. 2021, 183, 108295. [Google Scholar] [CrossRef]
- Gai, X.L.; Xing, T.; Li, X.H.; Zhang, B.; Wang, F.; Cai, Z.N.; Han, Y. Sound Absorption of Microperforated Panel with L Shape Division Cavity Structure. Appl. Acoust. 2017, 122, 41–50. [Google Scholar] [CrossRef]
- Rafique, F.; Wu, J.H.; Liu, C.R.; Ma, F. Low-Frequency Sound Absorption of an Inhomogeneous Micro-Perforated Panel with J-Shaped Cavities of Different Depths. Acoust. Aust. 2022, 50, 203–214. [Google Scholar] [CrossRef]
- Boccaccio, M.; Bucciarelli, F.; Fierro, G.P.M.; Meo, M. Microperforated Panel and Deep Subwavelength Archimedean-Inspired Spiral Cavities for Multi-Tonal and Broadband Sound Absorption. Appl. Acoust. 2021, 176, 107901. [Google Scholar] [CrossRef]
- Wang, S.; Li, F. A Broadband Sound Absorber of Hybrid-Arranged Perforated Panels with Perforated Partitions. Appl. Acoust. 2022, 188, 108547. [Google Scholar] [CrossRef]
- Guo, J.; Fang, Y.; Qu, R.; Liu, Q.; Zhang, X. An Extra-Broadband Compact Sound-Absorbing Structure Composing of Double-Layer Resonator with Multiple Perforations. J. Acoust. Soc. Am. 2021, 150, 1370–1380. [Google Scholar] [CrossRef]
- Mosa, A.I.; Putra, A.; Ramlan, R.; Esraa, A.A. Wideband Sound Absorption of a Double-Layer Microperforated Panel with Inhomogeneous Perforation. Appl. Acoust. 2020, 161, 107167. [Google Scholar] [CrossRef]
- Qian, Y.J.; Zhang, J.; Sun, N.; Kong, D.Y.; Zhang, X.X. Pilot Study on Wideband Sound Absorber Obtained by Adopting a Serial-Parallel Coupling Manner. Appl. Acoust. 2017, 124, 48–51. [Google Scholar] [CrossRef]
- Rafique, F.; Wu, J.H.; Waqas, M.; Lushuai, X.; Ma, F. A Thin Double-Layer Multiple Parallel-Arranged Inhomogeneous Microperforated Panel Absorber for Wideband Low-Frequency Sound Absorption. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 31. [Google Scholar] [CrossRef]
- Mosa, A.I.; Putra, A.; Ramlan, R.; Esraa, A.A. Absorption Coefficient of a Double-Layer Inhomogeneous Micro-Perforated Panel Backed with Multiple Cavity Depths. Acoust. Aust. 2020, 48, 69–78. [Google Scholar] [CrossRef]
- ISO. ISO 10534-2:1998. Acoustics—Determination of Sound Absorption Coefficient and Impedance in Impedance Tubes—Part 2: Transfer-Function Method. Available online: https://www.iso.org/standard/22851.html (accessed on 17 February 2022).
- Fuchs, H.V.; Zha, X. Acrylic-Glass Sound Absorbers in the Plenum of the Deutscher Bundestag. Appl. Acoust. 1997, 51, 211–217. [Google Scholar] [CrossRef]
- Zhang, P. Advanced Industrial Control Technology; Elsevier: Amsterdam, The Netherlands, 2010. [Google Scholar] [CrossRef]
- Yilmazer, S.; Ozdeniz, M.B. The Effect of Moisture Content on Sound Absorption of Expanded Perlite Plates. Build. Environ. 2005, 40, 311–318. [Google Scholar] [CrossRef]
- ISO 3382-2:2008(En), Acoustics—Measurement of Room Acoustic Parameters—Part 2: Reverberation Time in Ordinary Rooms. Available online: https://www.iso.org/obp/ui/#iso:std:iso:3382:-2:ed-1:v1:en (accessed on 17 February 2021).
- Vorländer, M. Auralization: Fundamentals of Acoustics, Modelling, Simulation, Algorithms and Acoustic Virtual Reality; Springer: Berlin/Heidelberg, Germany, 2008; ISBN 3540488308. [Google Scholar]
- Astolfi, A.; Corrado, V.; Griginis, A. Comparison between Measured and Calculated Parameters for the Acoustical Characterization of Small Classrooms. Appl. Acoust. 2008, 69, 966–976. [Google Scholar] [CrossRef]
- Houtgast, T.; Steeneken, H.J.M.; Plomp, R. Predicting Speech Intelligibility in Rooms from the Modulation Transfer Function. In Past, Present and Future of the Speech Transmission Index; TNO Human Factors: Soesterberg, The Netherlands, 2002. [Google Scholar]
- Lu, C.H.; Chen, W.; Zhu, Y.W.; Du, S.Z.; Liu, Z.E. Comparison Analysis and Optimization of Composite Micro-Perforated Absorbers in Sound Absorption Bandwidth. Acoust. Aust. 2018, 46, 305–315. [Google Scholar] [CrossRef]
- Xiang, N.; Fackler, C.J.; Hou, Y.; Schmitt, A.A.J. Bayesian Design of Broadband Multilayered Microperforated Panel Absorbers. J. Acoust. Soc. Am. 2022, 151, 3094. [Google Scholar] [CrossRef]
- Kusaka, M.; Sakagami, K.; Okuzono, T. A Basic Study on the Absorption Properties and Their Prediction of Heterogeneous Micro-Perforated Panels: A Case Study of Micro-Perforated Panels with Heterogeneous Hole Size and Perforation Ratio. Acoustics 2021, 3, 31. [Google Scholar] [CrossRef]
- Krushynska, A.O. Between Science and Art: Thin Sound Absorbers Inspired by Slavic Ornaments. Front. Mater. 2019, 6, 182. [Google Scholar] [CrossRef]
- Lee, H.P.; Kumar, S.; Aow, J.W. Proof-of-Concept Design for Mpp Acoustic Absorbers with Elements of Art. Designs 2021, 5, 72. [Google Scholar] [CrossRef]
- Sakagami, K.; Kusaka, M.; Okuzono, T. A Basic Study on the Design of Dotted-Art Heterogeneous MPP Sound Absorbers. Acoustics 2022, 4, 37. [Google Scholar] [CrossRef]
- Dzhambov, A.M. Long-Term Noise Exposure and the Risk for Type 2 Diabetes: A Meta-Analysis. Noise Health 2015, 17, 23–33. [Google Scholar] [CrossRef]
- Stansfeld, S.A.; Matheson, M.P. Noise Pollution: Non-Auditory Effects on Health. Br. Med. Bull. 2003, 68, 243–257. [Google Scholar] [CrossRef] [PubMed]
- Bateni, H.; Vaizasatya, A.; Blaschak, M.J. The Effect of 80 DB Environmental Noise on Control of Posture in Healthy Young Adults. Hum. Factors Ergon. Manuf. 2013, 23, 213–221. [Google Scholar] [CrossRef]



| MPP1 | ||||
|---|---|---|---|---|
| d (mm) | t (mm) | p (%) | D (mm) | |
| MPP1-a | 1 | 6 | 1.6 | 80 |
| MPP1-b | 0.7 | 6 | 2.8 | 60 |
| MPP1-c | 1 | 6 | 3.14 | 40 |
| MPP1-d | 2 | 6 | 6.4 | 20 |
| MPP1 | MPP2 | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| d (mm) | t (mm) | p (%) | D1 (mm) | d (mm) | t (mm) | p (%) | D2 (mm) | ||
| MPP1-a | 1 | 6 | 4.9 | 20 | MPP2-a | 1 | 6 | 1.6 | 60 |
| MPP1-b | 1 | 6 | 1.6 | 20 | MPP2-b | 0.7 | 6 | 2.8 | 20 |
| MPP1-c | 2 | 6 | 6.4 | 20 | MPP2-c | 1 | 6 | 4.9 | 80 |
| MPP1-d | 0.7 | 6 | 2.8 | 20 | MPP2-d | 2 | 6 | 6.4 | 40 |
| Surfaces | Materials | 125 Hz | 250 Hz | 500 Hz | 1000 Hz | 2000 Hz |
|---|---|---|---|---|---|---|
| Floor | Ceramic tile | 0.01 | 0.01 | 0.02 | 0.02 | 0.02 |
| Thin carpet on underlay | 0.03 | 0.09 | 0.3 | 0.54 | 0.5 | |
| Wall | Plaster on concrete | 0.03 | 0.03 | 0.02 | 0.03 | 0.04 |
| Ceiling | Plaster on concrete | 0.03 | 0.03 | 0.02 | 0.03 | 0.04 |
| Windows | Ordinary window glass | 0.35 | 0.25 | 0.18 | 0.12 | 0.07 |
| Door | Wooden door | 0.14 | 0.1 | 0.06 | 0.08 | 0.1 |
| Furniture | Wooden desks | 0.14 | 0.07 | 0.21 | 0.25 | 0.15 |
| Plastic/wooden chairs | 0.06 | 0.1 | 0.2 | 0.4 | 0.35 |
| Prototype 1 | 125 Hz | 250 Hz | 500 Hz | 1000 Hz | 2000 Hz |
|---|---|---|---|---|---|
| One-layer parallel-arranged MPPs with different cavities | 0.05 | 0.76 | 0.92 | 0.75 | 0.04 |
| STI ranges | 0.00–0.30 | 0.30–0.45 | 0.45–60 | 0.60–0.75 | 0.75–1.00 |
| Evaluation | bad | data | fair | good | excellent |
| STI | R1 | R2 | R3 | R4 |
|---|---|---|---|---|
| Before MPP treatment | 0.48 | 0.5 | 0.48 | 0.49 |
| After MPP treatment | 0.55 | 0.55 | 0.55 | 0.55 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fasllija, E.; Yilmazer, S. Investigating the Potential of Transparent Parallel-Arranged Micro-Perforated Panels (MPPs) as Sound Absorbers in Classrooms. Int. J. Environ. Res. Public Health 2023, 20, 1445. https://doi.org/10.3390/ijerph20021445
Fasllija E, Yilmazer S. Investigating the Potential of Transparent Parallel-Arranged Micro-Perforated Panels (MPPs) as Sound Absorbers in Classrooms. International Journal of Environmental Research and Public Health. 2023; 20(2):1445. https://doi.org/10.3390/ijerph20021445
Chicago/Turabian StyleFasllija, Ela, and Semiha Yilmazer. 2023. "Investigating the Potential of Transparent Parallel-Arranged Micro-Perforated Panels (MPPs) as Sound Absorbers in Classrooms" International Journal of Environmental Research and Public Health 20, no. 2: 1445. https://doi.org/10.3390/ijerph20021445
APA StyleFasllija, E., & Yilmazer, S. (2023). Investigating the Potential of Transparent Parallel-Arranged Micro-Perforated Panels (MPPs) as Sound Absorbers in Classrooms. International Journal of Environmental Research and Public Health, 20(2), 1445. https://doi.org/10.3390/ijerph20021445







