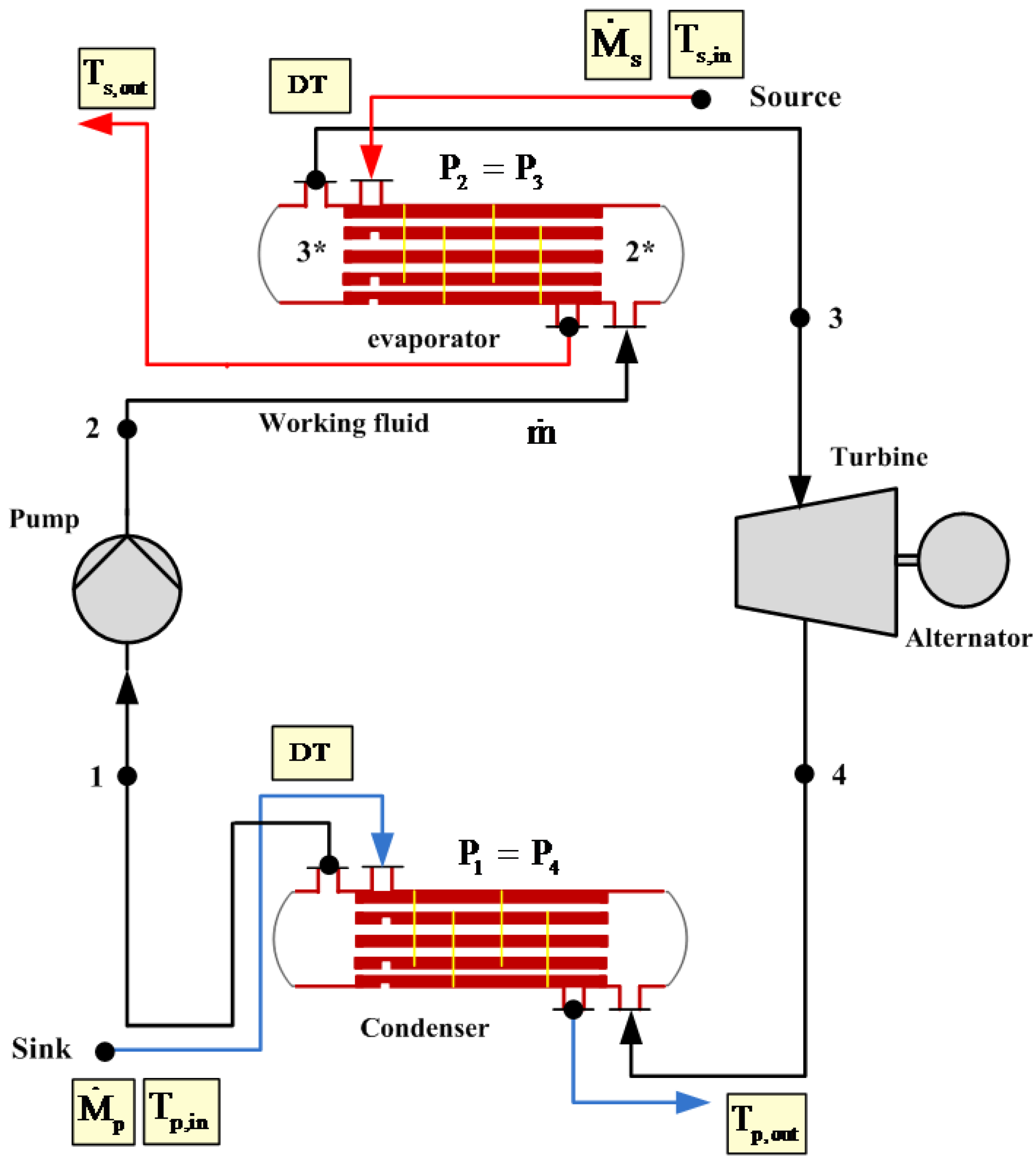
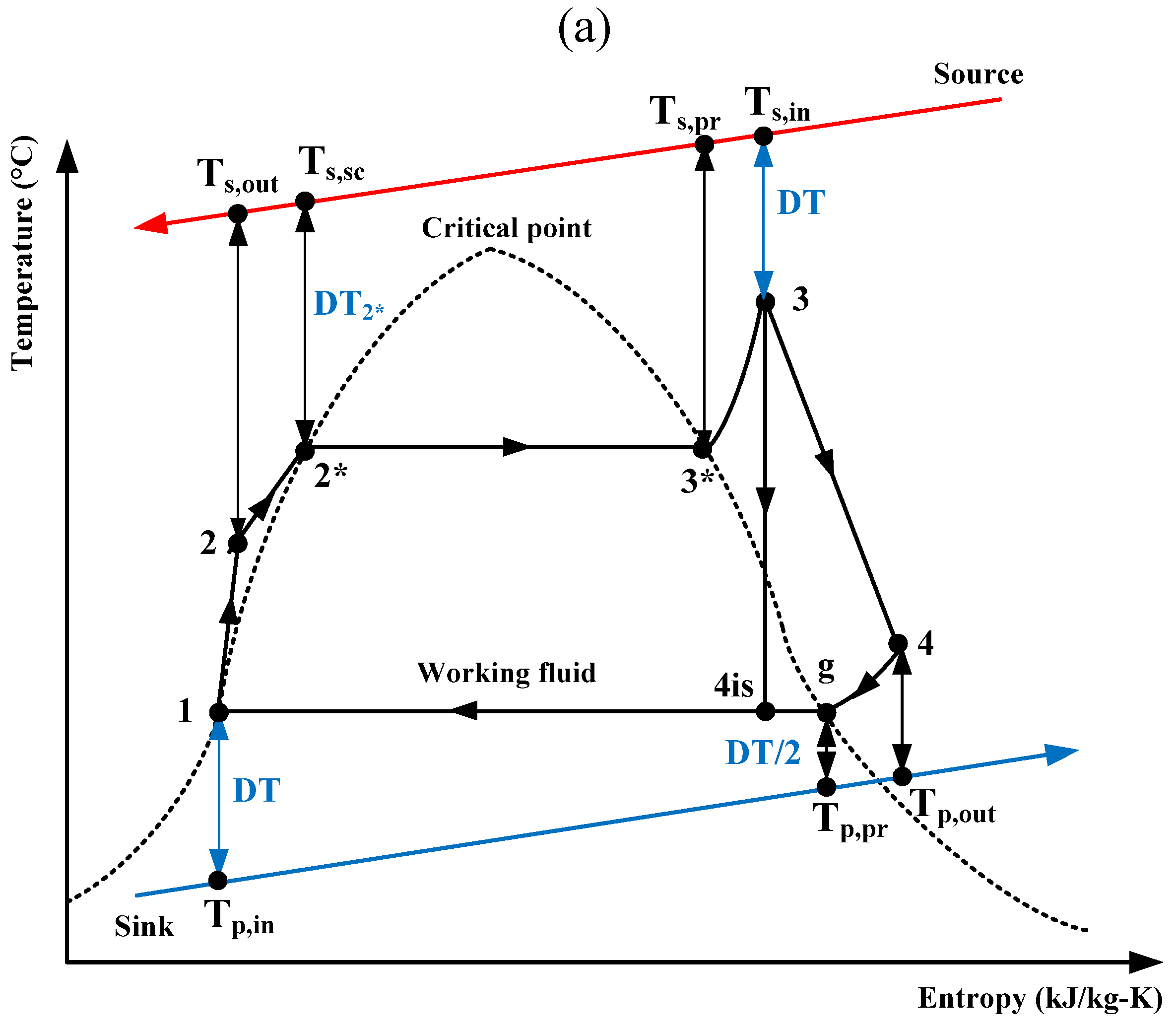
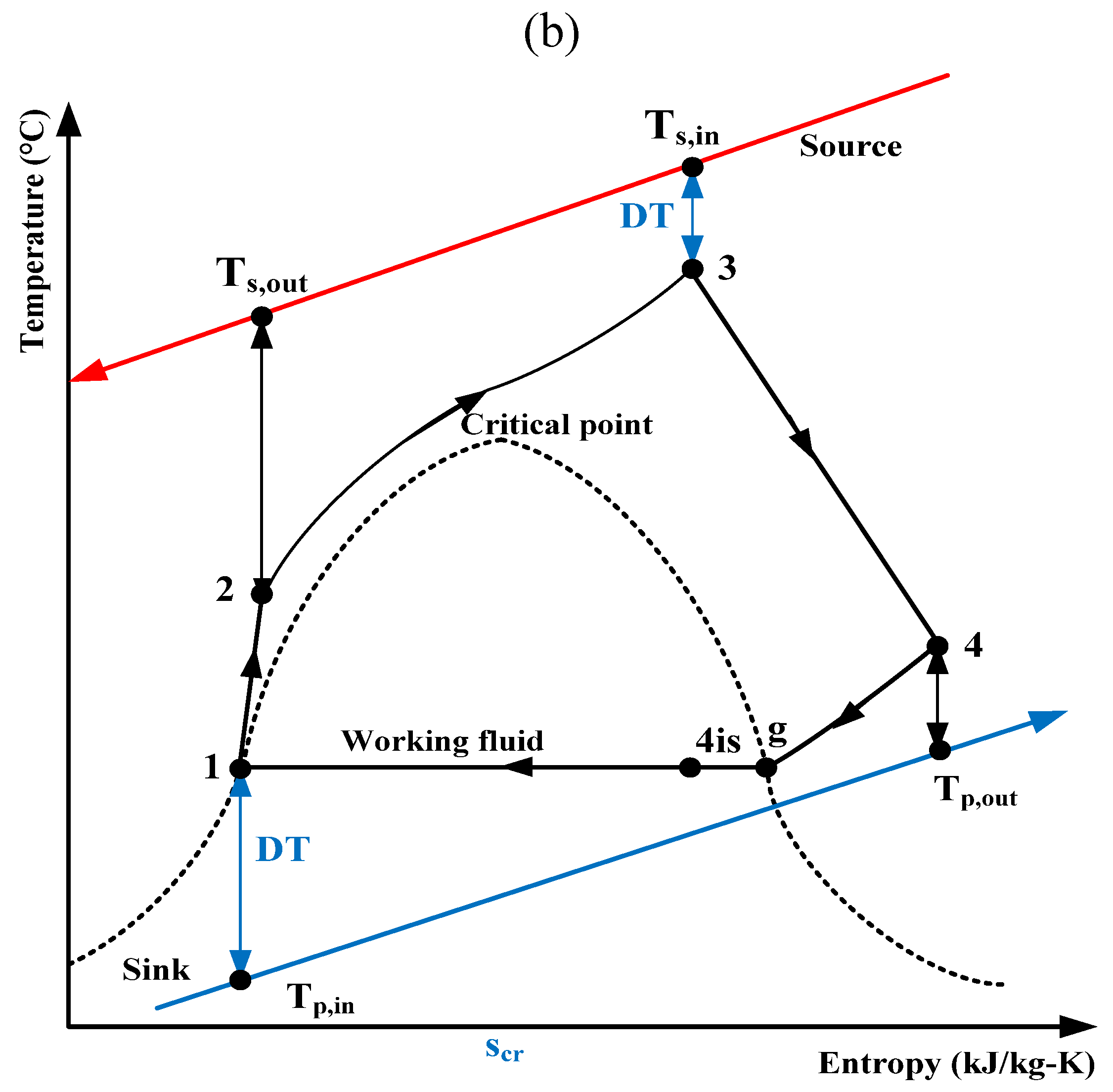
The results presented here have been calculated for the previously mentioned two working fluids with the four fixed variables (
= 314.5 kg/s, T
p,in = 10 °C, ηT = ηP = 0.8), three different source temperatures (T
s,in = 100, 165 and 230 °C) and three different values of the non-dimensional net power output α which is obtained by dividing (
) by the following reference power:
is evaluated by considering a Carnot process operating between the inlet temperatures of the heat source and sink. The product of the mass flowrate, the specific heat and the temperature difference is higher than the actual heat extracted from the heat source while the Carnot efficiency is higher than that of the actual cycle using the specified heat source and sink. Therefore the values of (α) are considerably lower than one (Khennich and Galanis [
7], Cayer et al. [
9]). In the present study the adopted values of (α) are 0.04, 0.08 and 0.12. The range of values for the temperature difference DT and the evaporation pressure P
ev has been specified in
Section 3.
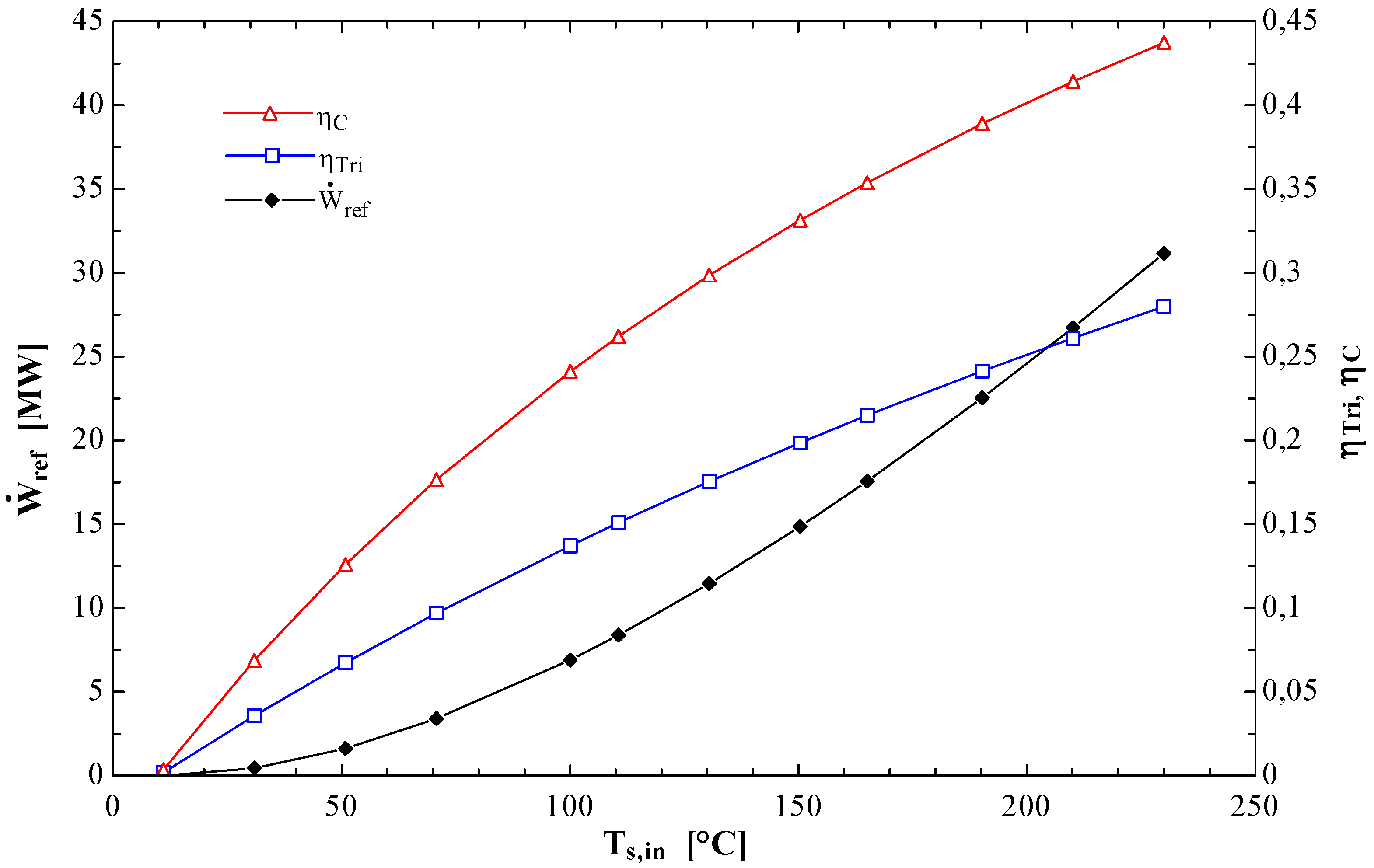
Figure 4 shows the effect of the inlet source temperature on the reference power as well as on the Carnot (η
C) and triangular (η
Tri) cycle efficiencies. The latter, which is considered to be a more realistic upper limit than the Carnot efficiency for trans-critical cycles is calculated from the following expression (Schuster
et al. [
14]):
Figure 4.
Effect of Ts,in on the reference power, the Carnot efficiency and the triangular efficiency.
The results of
Figure 4 show that these three quantities which are independent of the working fluid increase non-linearly with T
s,in. All three are equal to zero when T
s,in is equal to T
p,in; as T
s,in increases the reference power tends to infinity while the Carnot and triangular efficiencies approach unity. A comparison of the cycle’s thermal efficiency calculated from Equation (12) with the two values obtained from Equations (16a) and (16b) would give a clear indication of the thermodynamic performance of the system under study. However, since the thermal efficiency can be interpreted as the ratio of revenues (which are proportional to the net power output) to running costs (which are to a large extent proportional to the rate of heat input) it is not a very important performance criterion for systems which use a free source of heat (waste or solar or geothermal heat). In such cases an economic analysis is required to select an optimum design as pointed out by Lakew and Bolland [
6]. Such an analysis requires the specification of several details (for example: the diameter of the tubes and the shell in a tube and shell heat exchanger, see [
9]) and lacks the generality aimed for in the present study. Instead we introduce the following non-dimensional parameter which combines the total thermal conductance of the heat exchangers and the net power output:
The total thermal conductance of the heat exchangers, UA
t, is considered to represent the initial cost of the system. It is of course true that this cost depends on other variables, e.g., the operating pressures and the turbine size SP. However, it is shown later that, for a fixed combination of source temperature and net power output, SP does not depend much on the design criterion while the opposite is true for UA
t. Therefore the initial cost will depend more on the latter variable. On the other hand the denominator of F [Equation (17)] determines the revenues generated by the system. In view of the above discussion we believe that this parameter is more appropriate than the thermal efficiency for the comparison of systems using free sources of heat since low values of F likely correspond to a smaller initial cost per kW or a higher output (higher revenues) for a given investment. It should also be noted that the ratio of total heat transfer area A to the net power output (Ẇ
T − Ẇ
P) was used as an optimization criterion in the study by Madhawa Hettiarachchi
et al. [
12]. That ratio lacks the generality of the parameter F used in the present study since it is dimensional and its calculation requires the specification of additional information regarding the heat exchangers. Specifically the type of heat exchanger (plate, shell and tubes,
etc.), the size of its parts as well as correlations for the calculation of heat transfer coefficients are the minimum requirements for the evaluation of U as illustrated in [
12] and [
19].
5.1. Results for R134a
Table 2 shows the optimization results for R134a and a source temperature of 100 °C. Part 2.1 presents the maximum thermal efficiency, the operating conditions (P
ev,opt and DT
opt) for which this maximum efficiency is attained as well as the corresponding values of several other parameters. We note that in this case the optimum evaporation pressure is equal to the highest value compatible with the imposed constraints and that the optimum temperature difference DT is equal to the lower limit of the adopted range. As expected the maximum efficiency of this subcritical cycle is lower than the corresponding Carnot (η
C = 24.1%) and triangular (η
Tri = 13.7%) efficiencies. Its value and the operating conditions for which it is attained are independent of the non-dimensional power output. On the other hand the non-dimensional exergy destruction, the total thermal conductance, the turbine size parameter and the working fluid mass flowrate increase monotonically with the non-dimensional power output. In fact
increases linearly with α while the rate of increase of β and SP decreases as (α) increases and that of UA
t increases with (α). For all the values of (α) the working fluid is superheated at the turbine outlet (x
4 > 1) while the evaporator pinch occurs at the inlet of the source and is therefore always equal to DT
opt. For these conditions the value of F [Equation (17)] increases with the non-dimensional power output indicating that the initial cost per kW likely decreases as the net power output decreases.
Part 2.2 of
Table 2 shows that the minimum non-dimensional total exergy destruction is obtained for the same operating conditions (P
ev,opt and DT
opt) which maximize the thermal efficiency. Therefore the values of all the other calculated variables in Parts 2.1 and 2.2 are identical. This result establishes the fact that for this source temperature it is possible to simultaneously maximize the thermal efficiency and minimize the total exergy destruction.
Part 2.3 of
Table 2 presents the minimum total thermal conductance of the heat exchangers, the operating conditions (P
ev,opt and DT
opt) for which this thermal conductance is attained as well as the corresponding values of other calculated parameters. For a given value of (α) the minimum thermal conductance is approximately 45% lower than the one corresponding to maximum thermal efficiency and minimum exergy destruction. However, this important reduction of the heat exchangers’ conductance is accompanied by a significant deterioration of the other calculated parameters. Thus, the thermal efficiency decreases by approximately 30%, the total exergy destruction increases by approximately 60%, the working fluid mass flowrate and turbine size increase by approximately 75% and 10% respectively. These results establish a fact observed throughout this study,
i.e., that for a given combination of T
s,in and (α) (or equivalently a fixed net power output) the influence of different design scenarios on the variation of SP is rather small compared to that of UA
t. It therefore justifies the assertion that the numerator of Equation (17) determines to a considerable degree the initial cost of the system. The results of Part 2.3 show that the values of F obtained by minimizing UA
t are approximately half of the corresponding values in Parts 2.1 and 2.2 of the Table indicating that the minimization of UA
t leads to systems requiring a smaller initial investment for a given power output.
Table 2.
Optimization of the ORC with R134a for Ts,in = 100 °C (sub-critical), DT between 5 and 25 °C (ηC = 24.1%, ηTri = 13.7%, = 6897 kW).
Table 2.
Optimization of the ORC with R134a for Ts,in = 100 °C (sub-critical), DT between 5 and 25 °C (ηC = 24.1%, ηTri = 13.7%, = 6897 kW).
| [2.1] α | ηth,max | Pev,opt | DTopt | β | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 11.26 | 2365.0 | 5.00 | 7.64 | 662.3 | 0.0502 | 10.376 | 1.122 | 5.00 | 216.1 |
| 0.08 | 11.26 | 2365.0 | 5.00 | 14.19 | 1351.5 | 0.0710 | 20.753 | 1.122 | 5.00 | 220.4 |
| 0.12 | 11.26 | 2365.0 | 5.00 | 19.61 | 2087.3 | 0.0870 | 31.129 | 1.122 | 5.00 | 227.0 |
| [2.2] α | βmin | Pev,opt | DTopt | ηth | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 7.64 | 2365.0 | 5.00 | 11.26 | 662.3 | 0.0502 | 10.376 | 1.122 | 5.00 | 216.1 |
| 0.08 | 14.19 | 2365.0 | 5.00 | 11.26 | 1351.5 | 0.0710 | 20.753 | 1.122 | 5.00 | 220.4 |
| 0.12 | 19.61 | 2365.0 | 5.00 | 11.26 | 2087.3 | 0.0870 | 31.129 | 1.122 | 5.00 | 227.0 |
| [2.3] α | UAt,min | Pev,opt | DTopt | β | ηth | SP | | x4 | Pinchev | F |
| | (kW/K) | (kPa) | (°C) | % | % | (m) | (kg/s) | | (°C) | |
| 0.04 | 341.0 | 2365.0 | 21.69 | 12.09 | 8.07 | 0.0559 | 18.101 | 1.012 | 18.09 | 111.2 |
| 0.08 | 749.0 | 2320.9 | 18.80 | 20.54 | 8.55 | 0.0773 | 32.504 | 1.042 | 12.71 | 122.2 |
| 0.12 | 1268.3 | 2205.5 | 15.91 | 26.93 | 8.84 | 0.0945 | 44.678 | 1.079 | 8.57 | 137.9 |
| [2.4] α | SPmin | Pev,opt | DTopt | β | ηth | UAt | | x4 | Pinchev | F |
| | (m) | (kPa) | (°C) | % | % | (kW/K) | (kg/s) | | (°C) | |
| 0.04 | 0.0502 | 2365.0 | 5.00 | 7.64 | 11.26 | 662.3 | 10.376 | 1.122 | 5.00 | 216.1 |
| 0.08 | 0.0710 | 2365.0 | 5.00 | 14.19 | 11.26 | 1351.5 | 20.753 | 1.122 | 5.00 | 220.4 |
| 0.12 | 0.0870 | 2365.0 | 5.00 | 19.61 | 11.26 | 2087.3 | 31.129 | 1.122 | 5.00 | 227.0 |
Finally, Part 2.4 of
Table 2 shows that the minimum turbine size is obtained for the same operating conditions (P
ev,opt and DT
opt) which maximize the thermal efficiency and minimize the non-dimensional total exergy destruction. Therefore the values of all the other calculated variables in Parts 2.1, 2.2 and 2.4 are identical. This result is important since it establishes the fact that for this source temperature it is possible to simultaneously maximize the thermal efficiency and minimize the total exergy destruction as well as the turbine size. The corresponding combination of P
ev = 2365 kPa and DT = 5 °C constitutes an interesting design condition for T
s,in = 100 °C. However such a system is likely quite costly since the corresponding total thermal conductance is approximately twice the minimum value given in Part 2.3 of
Table 2.
Analogous results for a trans-critical cycle with a source temperature of 165 °C are presented in
Table 3. They show that in this case the operating conditions which maximize the thermal efficiency, minimize the total exergy destruction and minimize the turbine size are not identical. The difference between the conditions which maximize the thermal efficiency and those which minimize the exergy destruction are small (same optimum DT, small differences in optimum P
ev). On the other hand the conditions minimizing the turbine size are significantly different (smaller optimum evaporation pressures and higher values of DT). However, for a given value of (α) (or equivalently a fixed net power output) the maximum value of SP in the four parts of
Table 3 is within 5% of its minimum while the corresponding maximum for UA
t is 249% of its minimum. The values of F for the conditions minimizing UA
t (Part 3.3) are even lower than those obtained for T
s,in = 100 °C (see Part 2.3 of
Table 2) indicating that the initial cost per kW of such optimum systems probably decreases as the source temperature increases. The uncertainty in this last statement is due to two factors. First, the initial cost of the system increases with the area A of the heat exchangers rather than their thermal conductance UA. Second, the value of F does not take into account the higher cost resulting from the increase of the evaporation pressure corresponding to that of the heat source temperature (
c.f. Table 2 and
Table 3).
Table 3.
Optimization of the ORC cycle with R134a for Ts,in = 165 °C (trans-critical), DT between 5 and 25 °C (ηC = 35.4%, ηTri = 21.5%, = 17565 kW).
Table 3.
Optimization of the ORC cycle with R134a for Ts,in = 165 °C (trans-critical), DT between 5 and 25 °C (ηC = 35.4%, ηTri = 21.5%, = 17565 kW).
| [3.1] α | ηth,max | Pev,opt | DTopt | β | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 15.94 | 7120.9 | 5.00 | 7.96 | 1044.7 | 0.0568 | 16.882 | 1.176 | 5.00 | 230.5 |
| 0.08 | 15.94 | 7120.9 | 5.00 | 14.93 | 2115.3 | 0.0803 | 33.764 | 1.176 | 5.00 | 233.3 |
| 0.12 | 15.94 | 7120.9 | 5.00 | 20.87 | 3222.2 | 0.0984 | 50.646 | 1.176 | 5.00 | 236.9 |
| [3.2] α | βmin | Pev,opt | DTopt | ηth | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 7.96 | 7081.6 | 5.00 | 15.94 | 1042.9 | 0.0568 | 16.849 | 1.178 | 5.00 | 230.1 |
| 0.08 | 14.93 | 7078.6 | 5.00 | 15.94 | 2111.3 | 0.0803 | 33.694 | 1.178 | 5.00 | 232.9 |
| 0.12 | 20.87 | 7075.0 | 5.00 | 15.94 | 3215.5 | 0.0984 | 50.531 | 1.178 | 5.00 | 236.5 |
| [3.3] α | UAt,min | Pev,opt | DTopt | β | ηth | SP | | x4 | Pinchev | F |
| | (kW/K) | (kPa) | (°C) | % | % | (m) | (kg/s) | | (°C) | |
| 0.04 | 390.6 | 4968.1 | 25.00 | 11.84 | 11.84 | 0.0589 | 25.936 | 1.199 | 25.00 | 86.2 |
| 0.08 | 814.5 | 4968.1 | 25.00 | 21.86 | 11.84 | 0.0833 | 51.871 | 1.199 | 25.00 | 89.8 |
| 0.12 | 1295.0 | 4968.1 | 25.00 | 29.87 | 11.84 | 0.1019 | 77.863 | 1.197 | 25.00 | 95.2 |
| [3.4] α | SPmin | Pev,opt | DTopt | β | ηth | UAt | | x4 | Pinchev | F |
| | (m) | (kPa) | (°C) | % | % | (kW/K) | (kg/s) | | (°C) | |
| 0.04 | 0.0565 | 6428.5 | 10.47 | 8.76 | 14.88 | 600.5 | 18.678 | 1.183 | 10.47 | 132.5 |
| 0.08 | 0.0800 | 6428.7 | 10.47 | 16.39 | 14.88 | 1225.4 | 37.358 | 1.183 | 10.47 | 135.2 |
| 0.12 | 0.0979 | 6429.1 | 10.47 | 22.81 | 14.88 | 1886.1 | 56.033 | 1.183 | 10.47 | 138.7 |
Analogous results have been calculated for a trans-critical cycle with a source temperature of 230 °C. They confirm the trends discussed above but are not presented because R134a may not be stable at the corresponding high temperatures and pressures.
By comparing these results the following comments can be formulated:
The maximum thermal efficiency (Part 1 of the Tables) is independent of the net power output and increases with Ts,in. The corresponding optimum evaporation pressure also increases with Ts,in while the corresponding optimum value of DT is always equal to the smallest value in the chosen range.
The minimum non-dimensional exergy losses (Part 2 of the Tables), the minimum total thermal conductance of the heat exchangers (Part 3 of the Tables) and the minimum turbine size (Part 4 of the Tables) increase with the net power output and with Ts,in.
The conditions (Pev and DT) which minimize the non-dimensional exergy losses are essentially the same as those which maximize the thermal efficiency.
The proposed performance indicator F (which provides a first approximation of the initial cost per unit net output) increases with the net power output. For any given source temperature the lowest values of F are obtained for the conditions which minimize UAt. These lowest values of F decrease as Ts,in increases.
At the exit from the turbine the working fluid is always superheated. In the case of the trans-critical cycle with Ts,in = 230 °C, for which the superheating is most important, the use of regeneration may be advisable.
For the conditions maximizing the thermal efficiency (Part 1 of the Tables) and those minimizing the non-dimensional exergy losses (Part 2 of the Tables), the total thermal conductance of the heat exchangers (Part 3 of the Tables) and the turbine size (Part 4 of the Tables) the evaporator pinch is equal to the corresponding optimum value of DT (with the exception of Part 3 of
Table 2) and occurs at the inlet of the heat source in most cases.
5.2. Results for R141b
Table 4,
Table 5,
Table 6 and
Table 7 show analogous results for this second working fluid which has a considerably higher critical temperature than R134a (see
Table 1). Thus
Table 4 and
Table 5 for T
s,in equal to 100 and 165 °C respectively correspond to a sub-critical cycle. In the case of T
s,in = 230 °C sub-critical and trans-critical results are presented in
Table 6 and
Table 7 respectively; the latter were obtained by increasing the range of DT to 30–50 °C. By comparing the results in these Tables the following comments can be formulated:
The maximum thermal efficiency (Part 1 of
Table 4,
Table 5,
Table 6 and
Table 7 ) is independent of the net power output and increases with T
s,in. For T
s,in = 230 °C the trans-critical cycle has a better thermal efficiency than the sub-critical one. The corresponding optimum evaporation pressure also increases with T
s,in and is highest for the trans-critical cycle with T
s,in = 230 °C; the corresponding optimum value of DT is always equal to the smallest value in the chosen range.
The minimum non-dimensional exergy losses (Part 2 of
Table 4,
Table 5,
Table 6 and
Table 7) increase with the net power output and with T
s,in. The lowest minimum exergy losses are obtained for T
s,in = 165 °C. In the case with T
s,in = 230 °C the trans-critical cycle has a lower β
min than the sub-critical cycle.
The conditions (Pev and DT) which minimize the non-dimensional exergy losses are essentially the same as those which maximize the thermal efficiency.
The minimum total thermal conductance of the heat exchangers (Part 3 of
Table 4,
Table 5,
Table 6 and
Table 7) increases with the non-dimensional power output. When α = 0.04 UA
t is lowest for T
s,in = 100 °C followed by the sub-critical cycle with T
s,in = 230 °C. When α = 0.12 it is lowest for the sub-critical cycle with T
s,in = 230 °C followed by the cycle with T
s,in = 165 °C.
The minimum turbine size (Part 4 of
Table 4,
Table 5,
Table 6 and
Table 7) increases with the non-dimensional power output. The smallest values of SP
min are obtained for the sub-critical cycle with T
s,in = 230 °C.
At the exit from the turbine the fluid is always superheated.
For any of the four cycles under consideration the working fluid mass flowrate is lowest for the conditions which maximize ηth and minimize β; it is highest for the conditions minimizing UAt and increases with the net power output.
For the conditions maximizing ηth and minimizing β the pinch in the evaporator is equal to the smallest value of DT in the chosen range and occurs at the inlet of the source (except for the sub-critical cycle with Ts,in = 230 °C and α = 0.12). For the conditions minimizing UAt and SP the evaporator pinch has a value higher than the smallest value of DT.
The conditions minimizing UAt give turbines which are not much bigger than the corresponding smallest one. On the other hand the conditions which minimize SP give very high values of UAt compared to their minimum values. It is therefore justified to base a preliminary design on the conditions which minimize UAt and to use the proposed performance criterion F [see Equation (17)] as an indicator of the specific cost of the system.
The value of F increases with the non-dimensional power output and its lowest values are obtained for the conditions which minimize UA
t as in the case of R134a. For any given combination of T
s,in and (α) the lowest values of F and the corresponding evaporation pressure are smaller in the case of R141b suggesting a lower initial cost per unit output for systems using this working fluid. The subcritical cycle with T
s,in = 230 °C designed for the operating conditions which minimize UA
t has the lowest values of F among all the cases included in
Table 2,
Table 3,
Table 4,
Table 5,
Table 6 and
Table 7.
Table 4.
Optimization of the ORC cycle with R141b for Ts,in = 100 °C (sub-critical), DT between 5 and 25 °C (ηC = 24.1%, ηTri = 13.7%, = 6897 kW).
Table 4.
Optimization of the ORC cycle with R141b for Ts,in = 100 °C (sub-critical), DT between 5 and 25 °C (ηC = 24.1%, ηTri = 13.7%, = 6897 kW).
| [4.1] α | ηth,max | Pev,opt | DTopt | β | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 12.18 | 371.3 | 5.00 | 6.56 | 612.7 | 0.1220 | 7.822 | 1.100 | 5.00 | 199.9 |
| 0.08 | 12.18 | 371.3 | 5.00 | 12.19 | 1255.4 | 0.1725 | 15.644 | 1.100 | 5.00 | 204.8 |
| 0.12 | 12.10 | 366.0 | 5.00 | 17.08 | 1958.8 | 0.2124 | 23.609 | 1.101 | 5.00 | 213.0 |
| [4.2] α | βmin | Pev,opt | DTopt | ηth | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 6.56 | 371.3 | 5.00 | 12.18 | 612.7 | 0.1220 | 7.822 | 1.100 | 5.00 | 199.9 |
| 0.08 | 12.19 | 371.3 | 5.00 | 12.18 | 1255.4 | 0.1725 | 15.644 | 1.100 | 5.00 | 204.8 |
| 0.12 | 17.08 | 366.0 | 5.00 | 12.10 | 1958.8 | 0.2124 | 23.609 | 1.101 | 5.00 | 213.0 |
| [4.3] α | UAt,min | Pev,opt | DTopt | β | ηth | SP | | x4 | Pinchev | F |
| | (kW/K) | (kPa) | (°C) | % | % | (m) | (kg/s) | | (°C) | |
| 0.04 | 315.3 | 371.3 | 21.29 | 10.14 | 9.02 | 0.1224 | 11.920 | 1.043 | 17.39 | 102.9 |
| 0.08 | 702.5 | 359.2 | 18.57 | 17.83 | 9.35 | 0.1746 | 22.489 | 1.056 | 11.60 | 114.6 |
| 0.12 | 1217.1 | 331.1 | 15.43 | 24.28 | 9.48 | 0.2211 | 32.404 | 1.076 | 7.46 | 132.4 |
| [4.4] α | SPmin | Pev,opt | DTopt | β | ηth | UAt | x4 | | Pinchev | F |
| | (m) | (kPa) | (°C) | % | % | (kW/K) | (kg/s) | | (°C) | |
| 0.04 | 0.1205 | 371.3 | 12.86 | 8.03 | 10.65 | 351.9 | 9.459 | 1.074 | 12.86 | 114.8 |
| 0.08 | 0.1704 | 371.3 | 12.86 | 14.83 | 10.65 | 749.7 | 18.918 | 1.074 | 12.47 | 122.3 |
| 0.12 | 0.2101 | 366.0 | 12.51 | 20.47 | 10.64 | 1260.6 | 28.330 | 1.076 | 6.69 | 137.1 |
Table 5.
Optimization of the ORC cycle with R141b for Ts,in = 165 °C (sub-critical), DT between 5 and 25 °C (ηC = 35.4%, ηTri = 21.5%, = 17565 kW).
Table 5.
Optimization of the ORC cycle with R141b for Ts,in = 165 °C (sub-critical), DT between 5 and 25 °C (ηC = 35.4%, ηTri = 21.5%, = 17565 kW).
| [5.1] α | ηth,max | Pev,opt | DTopt | β | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 19.06 | 1503.0 | 5.00 | 5.58 | 866.2 | 0.1271 | 11.108 | 1.162 | 5.00 | 191.1 |
| 0.08 | 19.06 | 1503.0 | 5.00 | 10.48 | 1778.7 | 0.1798 | 22.217 | 1.162 | 5.00 | 196.2 |
| 0.12 | 19.06 | 1503.0 | 5.00 | 14.67 | 2793.8 | 0.2202 | 33.325 | 1.162 | 5.00 | 205.4 |
| [5.2] α | βmin | Pev,opt | DTopt | ηth | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 5.58 | 1503.0 | 5.00 | 19.06 | 866.2 | 0.1271 | 11.108 | 1.162 | 5.00 | 191.1 |
| 0.08 | 10.48 | 1503.0 | 5.00 | 19.06 | 1778.7 | 0.1798 | 22.217 | 1.162 | 5.00 | 196.2 |
| 0.12 | 14.67 | 1503.0 | 5.00 | 19.06 | 2793.8 | 0.2202 | 33.325 | 1.162 | 5.00 | 205.4 |
| [5.3] α | UAt,min | Pev,opt | DTopt | β | ηth | SP | | x4 | Pinchev | F |
| | (kW/K) | (kPa) | (°C) | % | % | (m) | (kg/s) | | (°C) | |
| 0.04 | 331.0 | 1132.4 | 25.00 | 8.40 | 14.70 | 0.1182 | 16.208 | 1.133 | 25.00 | 73.0 |
| 0.08 | 703.3 | 1063.0 | 25.00 | 16.21 | 14.39 | 0.1701 | 32.958 | 1.142 | 24.35 | 77.6 |
| 0.12 | 1151.0 | 979.1 | 25.00 | 23.47 | 13.97 | 0.2134 | 50.630 | 1.154 | 17.11 | 84.6 |
| [5.4] α | SPmin | Pev,opt | DTopt | β | ηth | UAt | | x4 | Pinchev | F |
| | (m) | (kPa) | (°C) | % | % | (kW/K) | (kg/s) | | (°C) | |
| 0.04 | 0.1107 | 1503.0 | 25.00 | 7.29 | 15.94 | 352.1 | 15.379 | 1.085 | 17.77 | 114.9 |
| 0.08 | 0.1566 | 1503.0 | 25.00 | 13.59 | 15.94 | 786.2 | 30.758 | 1.085 | 10.54 | 128.2 |
| 0.12 | 0.1918 | 1503.0 | 25.00 | 18.86 | 15.94 | 1499.3 | 46.136 | 1.085 | 3.31 | 163.0 |
Table 6.
Optimization of the ORC cycle with R141b for Ts,in = 230 °C (trans-critical), DT between 5 and 25 °C (ηC = 43.7%, ηTri = 28.0%, = 31158 kW).
Table 6.
Optimization of the ORC cycle with R141b for Ts,in = 230 °C (trans-critical), DT between 5 and 25 °C (ηC = 43.7%, ηTri = 28.0%, = 31158 kW).
| [6.1] α | ηth,max | Pev,opt | DTopt | β | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 22.62 | 4383.3 | 5.00 | 5.93 | 1182.5 | 0.1408 | 15.528 | 1.187 | 5.00 | 208.7 |
| 0.08 | 22.62 | 4383.3 | 5.00 | 11.21 | 2402.0 | 0.1991 | 31.055 | 1.187 | 5.00 | 212.0 |
| 0.12 | 22.62 | 4383.3 | 5.00 | 15.81 | 3679.8 | 0.2439 | 46.583 | 1.187 | 5.00 | 216.5 |
| [6.2] α | βmin | Pev,opt | DTopt | ηth | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 5.93 | 4365.3 | 5.00 | 22.62 | 1180.8 | 0.1408 | 15.507 | 1.189 | 5.00 | 208.4 |
| 0.08 | 11.21 | 4364.3 | 5.00 | 22.62 | 2398.4 | 0.1991 | 31.012 | 1.189 | 5.00 | 211.7 |
| 0.12 | 15.81 | 4362.1 | 5.00 | 22.62 | 3673.8 | 0.2436 | 46.514 | 1.189 | 5.00 | 216.2 |
| [6.3] α | UAt,min | Pev,opt | DTopt | β | ηth | SP | | x4 | Pinchev | F |
| | (kW/K) | (kPa) | (°C) | % | % | (m) | (kg/s) | | (°C) | |
| 0.04 | 423.2 | 4250.0 | 25.00 | 8.02 | 18.69 | 0.1269 | 23.739 | 1.028 | 25.00 | 74.7 |
| 0.08 | 878.7 | 4250.0 | 25.00 | 15.08 | 18.69 | 0.1795 | 47.478 | 1.028 | 22.68 | 77.6 |
| 0.12 | 1384.5 | 4250.0 | 25.00 | 21.11 | 18.69 | 0.2198 | 71.216 | 1.028 | 18.57 | 81.5 |
| [6.4] α | SPmin | Pev,opt | DTopt | β | ηth | UAt | | x4 | Pinchev | F |
| | (m) | (kPa) | (°C) | % | % | (kW/K) | (kg/s) | | (°C) | |
| 0.04 | 0.1253 | 4250.0 | 22.65 | 7.50 | 19.44 | 438.8 | 21.547 | 1.074 | 22.65 | 77.5 |
| 0.08 | 0.1772 | 4250.0 | 22.65 | 14.12 | 19.44 | 912.7 | 43.094 | 1.074 | 19.67 | 80.6 |
| 0.12 | 0.2171 | 4250.0 | 22.65 | 19.80 | 19.44 | 1443.2 | 64.642 | 1.074 | 15.72 | 84.9 |
Table 7.
Optimization of the ORC cycle with R141b for Ts,in = 230 °C (sub-critical), DT between 30 and 50 °C (ηC = 43.7%, ηTri = 28.0%, = 31158 kW).
Table 7.
Optimization of the ORC cycle with R141b for Ts,in = 230 °C (sub-critical), DT between 30 and 50 °C (ηC = 43.7%, ηTri = 28.0%, = 31158 kW).
| [7.1] α | ηth,max | Pev,opt | DTopt | β | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 18.20 | 2913.0 | 30.00 | 8.14 | 354.5 | 0.1175 | 21.230 | 1.201 | 30.00 | 62.6 |
| 0.08 | 18.20 | 2913.0 | 30.00 | 15.27 | 742.0 | 0.1661 | 42.461 | 1.201 | 30.00 | 65.5 |
| 0.12 | 18.20 | 2913.0 | 30.00 | 21.31 | 1185.9 | 0.2034 | 63.691 | 1.201 | 23.24 | 69.8 |
| [7.2] α | βmin | Pev,opt | DTopt | ηth | UAt | SP | | x4 | Pinchev | F |
| | % | (kPa) | (°C) | % | (kW/K) | (m) | (kg/s) | | (°C) | |
| 0.04 | 8.14 | 2913.0 | 30.00 | 18.20 | 354.5 | 0.1175 | 21.230 | 1.201 | 30.00 | 62.6 |
| 0.08 | 15.27 | 2913.0 | 30.00 | 18.20 | 742.0 | 0.1661 | 42.461 | 1.201 | 30.00 | 65.5 |
| 0.12 | 21.31 | 2913.0 | 30.00 | 18.20 | 1185.9 | 0.2034 | 63.691 | 1.201 | 23.24 | 69.8 |
| [7.3] α | UAt,min | Pev,opt | DTopt | β | ηth | SP | | x4 | Pinchev | F |
| | (kW/K) | (kPa) | (°C) | % | % | (m) | (kg/s) | | (°C) | |
| 0.04 | 321.3 | 2511.1 | 48.67 | 10.44 | 15.11 | 0.1109 | 29.086 | 1.149 | 47.47 | 56.7 |
| 0.08 | 687.6 | 2445.4 | 44.59 | 18.69 | 15.58 | 0.1593 | 54.111 | 1.179 | 37.22 | 60.7 |
| 0.12 | 1124.4 | 2349.6 | 39.64 | 24.90 | 16.11 | 0.1999 | 74.840 | 1.213 | 27.80 | 66.2 |
| [7.4] α | SPmin | Pev,opt | DTopt | β | ηth | UAt | | x4 | Pinchev | F |
| | (m) | (kPa) | (°C) | % | % | (kW/K) | (kg/s) | | (°C) | |
| 0.04 | 0.1094 | 2867.3 | 50.00 | 10.29 | 15.28 | 324.2 | 30.302 | 1.092 | 40.85 | 57.2 |
| 0.08 | 0.1547 | 2867.3 | 50.00 | 19.12 | 15.28 | 700.2 | 60.605 | 1.092 | 30.68 | 61.8 |
| 0.12 | 0.1894 | 2867.3 | 50.00 | 26.36 | 15.28 | 1178.4 | 90.907 | 1.092 | 20.51 | 69.3 |