Three-Dimensional Unsteady Axisymmetric Viscous Beltrami Vortex Solutions to the Navier–Stokes Equations
Abstract
:1. Introduction
2. Dynamical and Constraint Equations for the Axisymmetric Beltrami Vortex
3. Consistency of Dynamical and Constraint Equations
4. Vortex Solutions
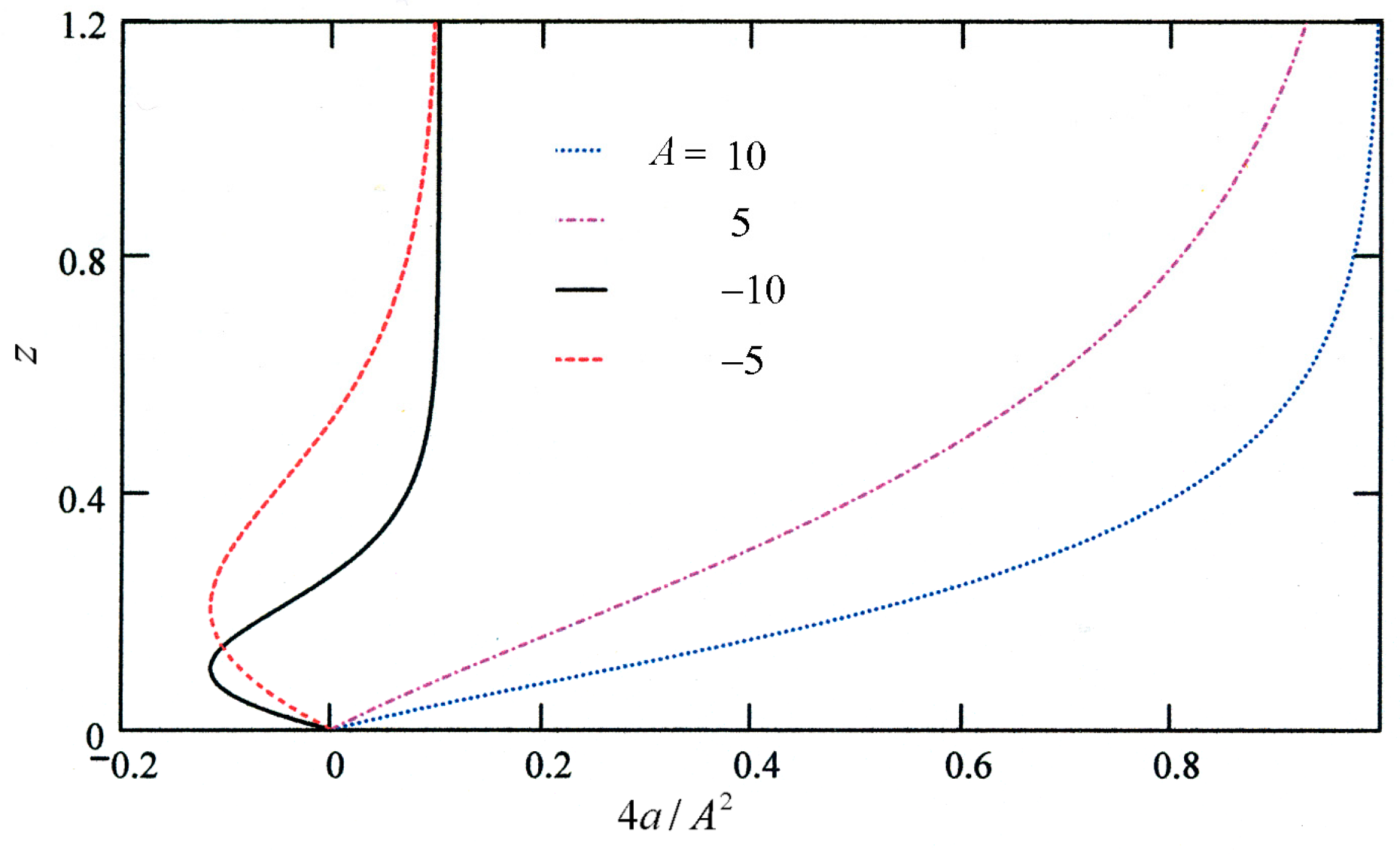
4.1. Constant c
4.2. Nonconstant c
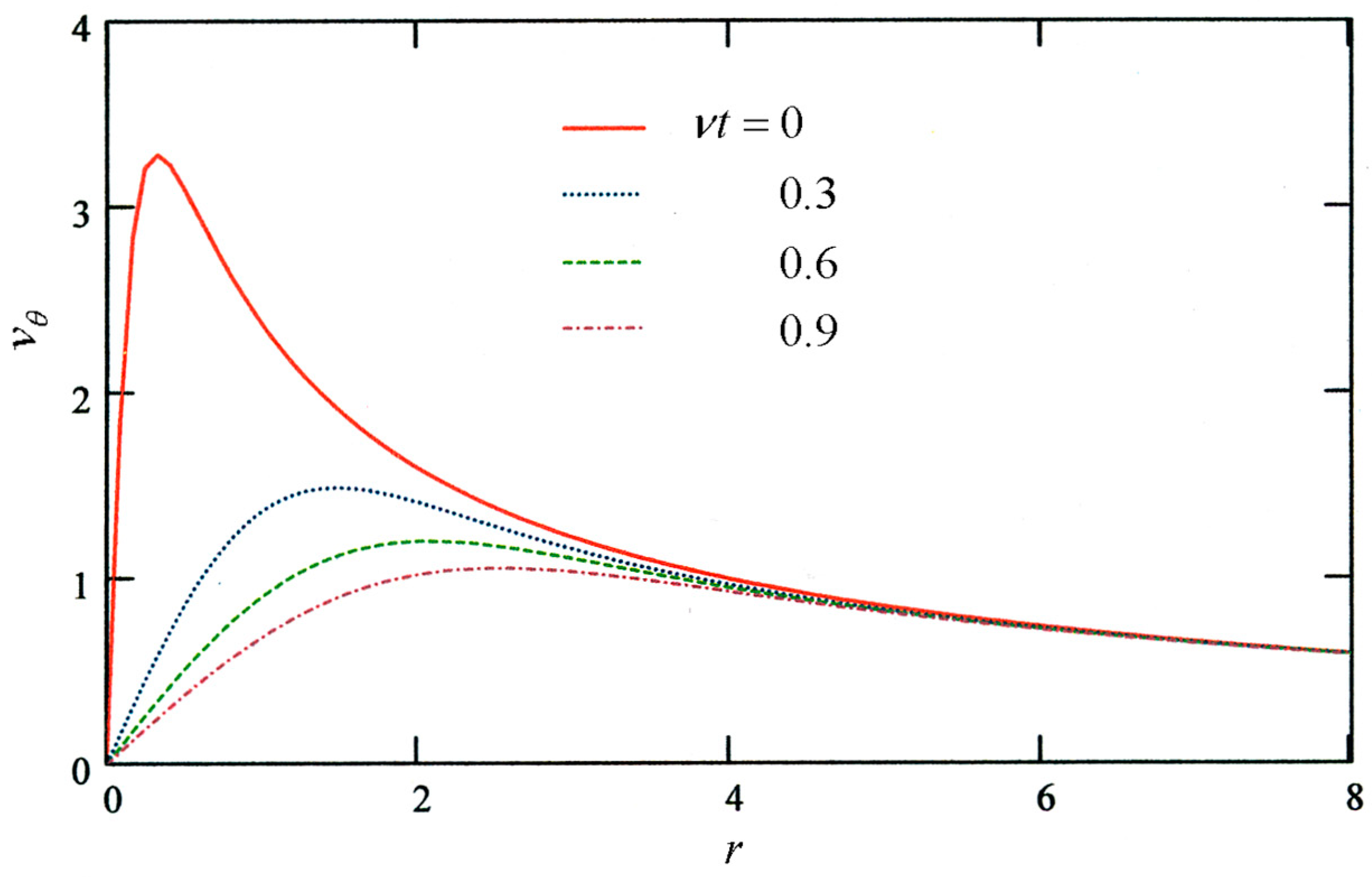
4.3. Time Dependence
5. Conclusions and Discussion
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Consistency of Constraints with the Navier–Stokes Equations (1) and (3)
Appendix B. Variable c Generally Implies Time-Dependent External Force
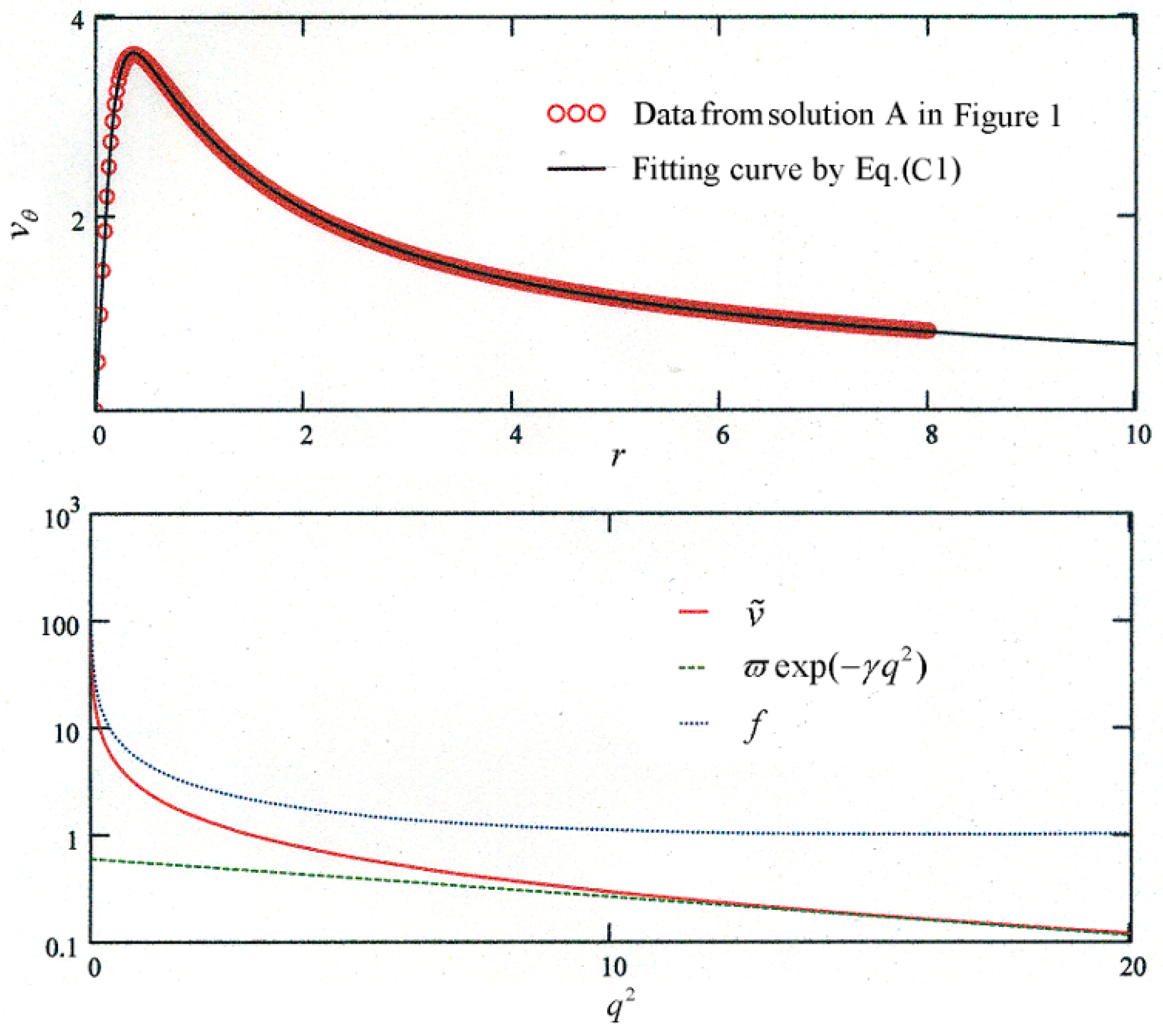
Appendix C. Extracting the Gaussian Factor and the Spectrum Function from the Solution (i) in Figure 1
References
- Burgers, J.M. A Mathematical Model Illustrating the Theory of Turbulence. In Advances in Applied Mechanics; Von Mises, R., Von Kármán, T., Eds.; Elsevier: Amsterdam, The Netherlands, 1948; Volume 1, pp. 171–199. [Google Scholar]
- Rott, N. On the Viscous Core of a Line Vortex. J. Appl. Math. Phys. (ZAMP) 1958, 9, 543–553. [Google Scholar] [CrossRef]
- Sullivan, R.D. A Two-Cell Vortex Solution of the Navier-Stokes Equations. J. Aerosp. Sci. 1959, 26, 767–768. [Google Scholar] [CrossRef]
- Bellamy-Knights, P.G. An Unsteady Two-Cell Vortex Solution of the Navier—Stokes Equations. J. Fluid Mech. 1970, 41, 673–687. [Google Scholar] [CrossRef]
- Bellamy-Knights, P.G. Unsteady Multicellular Viscous Vortices. J. Fluid Mech. 1971, 50, 1–16. [Google Scholar] [CrossRef]
- Craik, A.D.D. Exact Vortex Solutions of the Navier–Stokes Equations with Axisymmetric Strain and Suction or Injection. J. Fluid Mech. 2009, 626, 291–306. [Google Scholar] [CrossRef]
- Weinbaum, S.; O’Brien, V. Exact Navier-Stokes Solutions Including Swirl and Cross Flow. Phys. Fluids 1967, 10, 1438–1447. [Google Scholar] [CrossRef]
- Gibbon, J.D.; Fokas, A.S.; Doering, C.R. Dynamically Stretched Vortices as Solutions of the 3D Navier–Stokes Equations. Phys. D Nonlinear Phenom. 1999, 132, 497–510. [Google Scholar] [CrossRef] [Green Version]
- Takahashi, K. Three-Dimensional Unsteady Axisymmetric Vortex Solutions to the Bellamy-Knights Equation and the Distribution of Boundary Conditions. AIP Adv. 2022, 12, 085324. [Google Scholar] [CrossRef]
- Drazin, P.G.; Riley, N. The Navier-Stokes Equations: A Classification of Flows and Exact Solutions; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Trkal, V. A Note on the Hydrodynamics of Viscous Fluids. Czechoslov. J. Phys. 1994, 44, 97–106. [Google Scholar] [CrossRef]
- Gromeka, I.S. Some Cases of the Motion of an Incompressible Fluid; Collected Works; Academy of Sciences: Moscow, USSR, 1952. (In Russian) [Google Scholar]
- Wood, V.T.; Brown, R.A. Simulated Tornadic Vortex Signatures of Tornado-Like Vortices Having One- and Two-Celled Structures. J. Appl. Meteorol. Climatol. 2011, 50, 2338–2342. [Google Scholar] [CrossRef]
- Baker, C. Some Musings on Tornado Vortex Models. Available online: https://profchrisbaker.com/2020/06/30/some-musings-on-tornado-vortex-models/ (accessed on 30 July 2023).
- Barber, T.A.; Majdalani, J. On the Beltramian Motion of the Bidirectional Vortex in a Conical Cyclone. J. Fluid Mech. 2017, 828, 708–732. [Google Scholar] [CrossRef] [Green Version]
- Maicke, B.; Majdalani, J. On the Compressible Bidirectional Vortex. Part 1: A Bragg-Hawthorne Stream Function Formulation. In Proceedings of the 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Nashville, TN, USA, 9–12 January 2012; Aerospace Sciences Meetings. American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- Williams, L.L.; Majdalani, J. Exact Beltramian Solutions for Hemispherically Bounded Cyclonic Flowfields. Phys. Fluids 2021, 33, 093601. [Google Scholar] [CrossRef]
- Arnold, V.I.; Arnold, V.I. Sur La Topologie des Écoulements Stationnaires des Fluides Parfaits; Givental, A.B., Khesin, B.A., Varchenko, A.N., Vassiliev, V.A., Viro, O.Y., Eds.; Springer: Berlin/Heidelberg, Germany, 1965; pp. 15–18. [Google Scholar]
- Dombre, T.; Frisch, U.; Greene, J.M.; Hénon, M.; Mehr, A.; Soward, A.M. Chaotic Streamlines in the ABC Flows. J. Fluid Mech. 1986, 167, 353. [Google Scholar] [CrossRef]
- Bouya, I.; Dormy, E. Revisiting the ABC Flow Dynamo. Phys. Fluids 2013, 25, 037103. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, P.; Roy, A.; Chakraborty, S. Topology and Transport in Generalized Helical Flows. Phys. Fluids 2021, 33, 117106. [Google Scholar] [CrossRef]
- Bělík, P.; Su, X.; Dokken, D.P.; Scholz, K.; Shvartsman, M.M. On the Axisymmetric Steady Incompressible Beltrami Flows. OJFD 2020, 10, 208–238. [Google Scholar] [CrossRef]
- Yi, T. Some General Solutions for Linear Bragg–Hawthorne Equation. Phys. Fluids 2021, 33, 077113. [Google Scholar] [CrossRef]
- Viúdez, A. Multipolar Spherical and Cylindrical Vortices. J. Fluid Mech. 2022, 936, A13. [Google Scholar] [CrossRef]
- Chandrasekhar, S. On force-free magnetic fields. Proc. Natl. Acad. Sci. USA 1956, 42, 1–5. [Google Scholar] [CrossRef]
- Chandrasekhar, S.; Woltjer, L. On force-free magnetic fields. Proc. Natl. Acad. Sci. USA 1958, 44, 285–289. [Google Scholar] [CrossRef]
- Pelz, R.B.; Yakhot, V.; Orszag, S.A.; Shtilman, L.; Levich, E. Velocity-Vorticity Patterns in Turbulent Flow. Phys. Rev. Lett. 1985, 54, 2505–2508. [Google Scholar] [CrossRef] [PubMed]
- Golubkin, V.N.; Sizykh, G.B. Some General Properties of Plane-Parallel Viscous Flows. Fluid Dyn. 1987, 22, 479–481. [Google Scholar] [CrossRef]
- Freedman, M.H. A Note on Topology and Magnetic Energy in Incompressible Perfectly Conducting Fluids. J. Fluid Mech. 1988, 194, 549–551. [Google Scholar] [CrossRef]
- Moffatt, H.K. Helicity and Singular Structures in Fluid Dynamics. Proc. Natl. Acad. Sci. USA 2014, 111, 3663–3670. [Google Scholar] [CrossRef] [PubMed]
- Davies-Jones, R. Can a Descending Rain Curtain in a Supercell Instigate Tornadogenesis Barotropically? J. Atmos. Sci. 2008, 65, 2469–2497. [Google Scholar] [CrossRef] [Green Version]
- Yoshida, Z.; Mahajan, S.M.; Ohsaki, S.; Iqbal, M.; Shatashvili, N. Beltrami Fields in Plasmas: High-Confinement Mode Boundary Layers and High Beta Equilibria. Phys. Plasmas 2001, 8, 2125–2131. [Google Scholar] [CrossRef]
- Bhattacharjee, C. Beltrami–Bernoulli Equilibria in Weakly Rotating Self-Gravitating Fluid. J. Plasma Phys. 2022, 88, 175880101. [Google Scholar] [CrossRef]
- Morgulis, A.; Yudovich, V.I.; Zaslavsky, G.M. Compressible Helical Flows. Comm. Pure Appl. Math. 1995, 48, 571–582. [Google Scholar] [CrossRef]
- Berker, R. Integration des Équations du Mouvement d’un Fluide Visqueux Incompressible; Handbuch der Physik, Bd VIII/2, Encyclopedia of Physics; Springer: Berlin, Germany, 1963. [Google Scholar]
- Ershkov, S.V. Non-Stationary Helical Flows for Incompressible 3D Navier–Stokes Equations. Appl. Math. Comput. 2016, 274, 611–614. [Google Scholar] [CrossRef]
- Dierkes, D.; Cheviakov, A.; Oberlack, M. New Similarity Reductions and Exact Solutions for Helically Symmetric Viscous Flows. Phys. Fluids 2020, 32, 053604. [Google Scholar] [CrossRef]
- Wang, C.Y. Exact Solutions of the Unsteady Navier-Stokes Equations. Appl. Mech. Rev. 1989, 42, S269–S282. [Google Scholar] [CrossRef]
- Joseph, S.P. Polynomial Solutions and Other Exact Solutions of Axisymmetric Generalized Beltrami Flows. Acta Mech. 2018, 229, 2737–2750. [Google Scholar] [CrossRef]
- Wang, C.Y. Exact Solutions of the Steady-State Navier-Stokes Equations. Annu. Rev. Fluid Mech. 1991, 23, 159–177. [Google Scholar] [CrossRef]
- Dyck, N.J.; Straatman, A.G. Exact Solutions to the Three-Dimensional Navier–Stokes Equations Using the Extended Beltrami Method. J. Appl. Mech. 2020, 87, 011004. [Google Scholar] [CrossRef]
- Holm, D.D.; Kerr, R. Transient Vortex Events in the Initial Value Problem for Turbulence. Phys. Rev. Lett. 2002, 88, 244501. [Google Scholar] [CrossRef] [Green Version]
- Choi, Y.; Kim, B.-G.; Lee, C. Alignment of Velocity and Vorticity and the Intermittent Distribution of Helicity in Isotropic Turbulence. Phys. Rev. E 2009, 80, 017301. [Google Scholar] [CrossRef]
- Jacobitz, F.G.; Schneider, K.; Bos, W.J.T.; Farge, M. On Helical Multiscale Characterization of Homogeneous Turbulence. J. Turbul. 2012, 13, N35. [Google Scholar] [CrossRef] [Green Version]
- Constantin, P.; Majda, A. The Beltrami Spectrum for Incompressible Fluid Flows. Commun. Math. Phys. 1988, 115, 435–456. [Google Scholar] [CrossRef]
- Ershkov, S.V.; Shamin, R.V.; Giniyatullin, A.R. On a New Type of Non-Stationary Helical Flows for Incompressible 3D Navier-Stokes Equations. J. King Saud Univ. Sci. 2020, 32, 459–467. [Google Scholar] [CrossRef]
- Hicks, W.M. Researches in Vortex Motion. Part III. On Spiral or Gyrostatic Vortex Aggregates. [Abstract]. Proc. R. Soc. Lond. 1897, 62, 332–338. [Google Scholar]
- Bragg, S.L.; Hawthorne, W.R. Some Exact Solutions of the Flow Through Annular Cascade Actuator Discs. J. Aeronaut. Sci. 1950, 17, 243–249. [Google Scholar] [CrossRef]
- Gledzer, E.B.; Makarov, A.L. A Class of Steady Axisymmetric Incompressible Flows. Fluid Dyn. 1991, 25, 832–838. [Google Scholar] [CrossRef]
- Taylor, G.I. LXXV. On the Decay of Vortices in a Viscous Fluid. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1923, 46, 671–674. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Takahashi, K. Three-Dimensional Unsteady Axisymmetric Viscous Beltrami Vortex Solutions to the Navier–Stokes Equations. J 2023, 6, 460-476. https://doi.org/10.3390/j6030030
Takahashi K. Three-Dimensional Unsteady Axisymmetric Viscous Beltrami Vortex Solutions to the Navier–Stokes Equations. J. 2023; 6(3):460-476. https://doi.org/10.3390/j6030030
Chicago/Turabian StyleTakahashi, Koichi. 2023. "Three-Dimensional Unsteady Axisymmetric Viscous Beltrami Vortex Solutions to the Navier–Stokes Equations" J 6, no. 3: 460-476. https://doi.org/10.3390/j6030030





