A Model for the Generalised Dispersion of Synovial Fluids on Nutritional Transport with Joint Impacts of Electric and Magnetic Field
Abstract
:1. Introduction
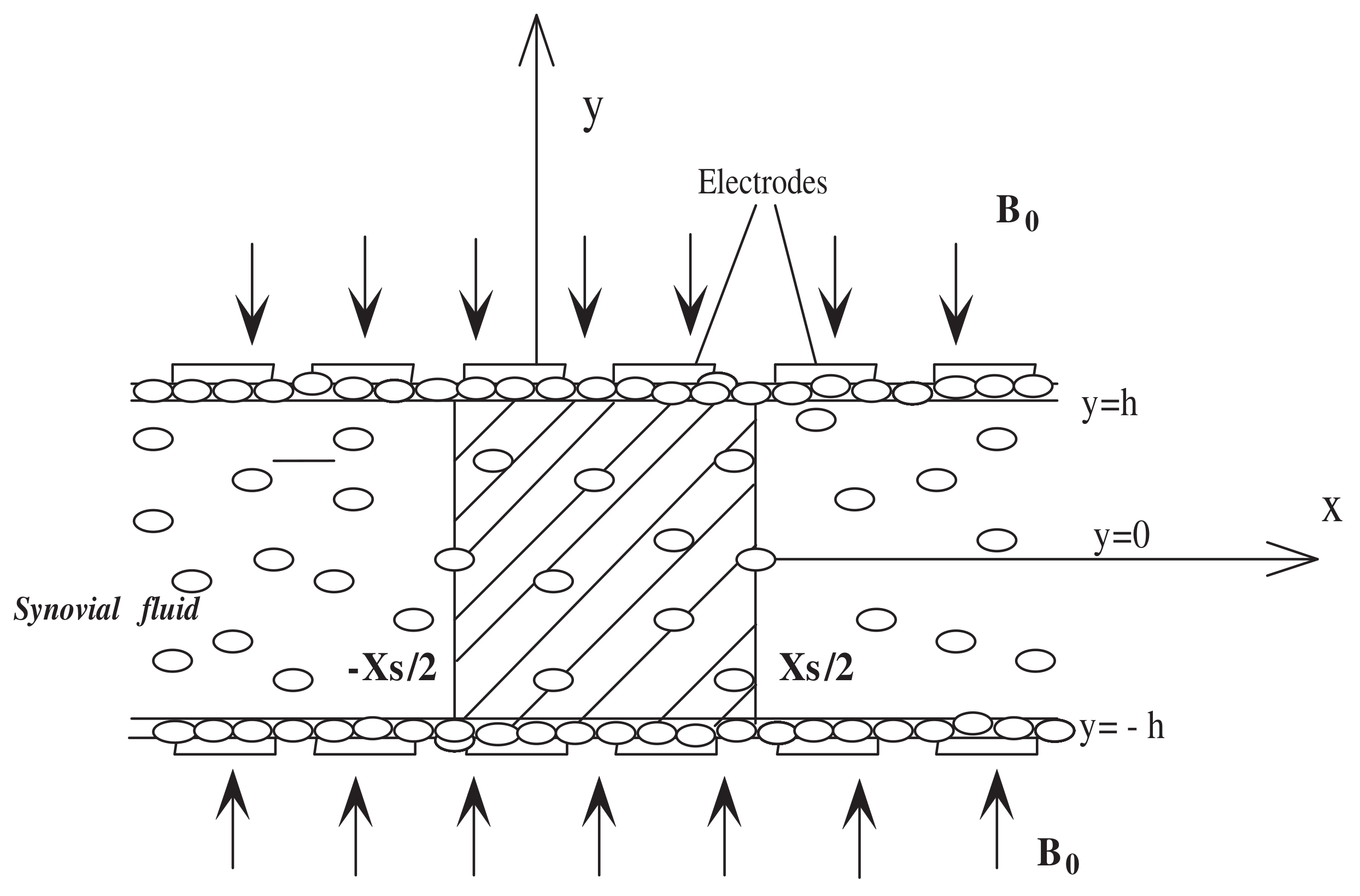
2. Formulation of the Problem
- A 2D, electrically conducting, viscous and incompressible synovial fluid is considered.
- Flow of fluid is laminar and steady.
- A constant magnetic field of strength is applied in the transverse direction.
3. Method of Solution
3.1. Velocity Distribution
3.2. Generalized Dispersion Model (GDM)
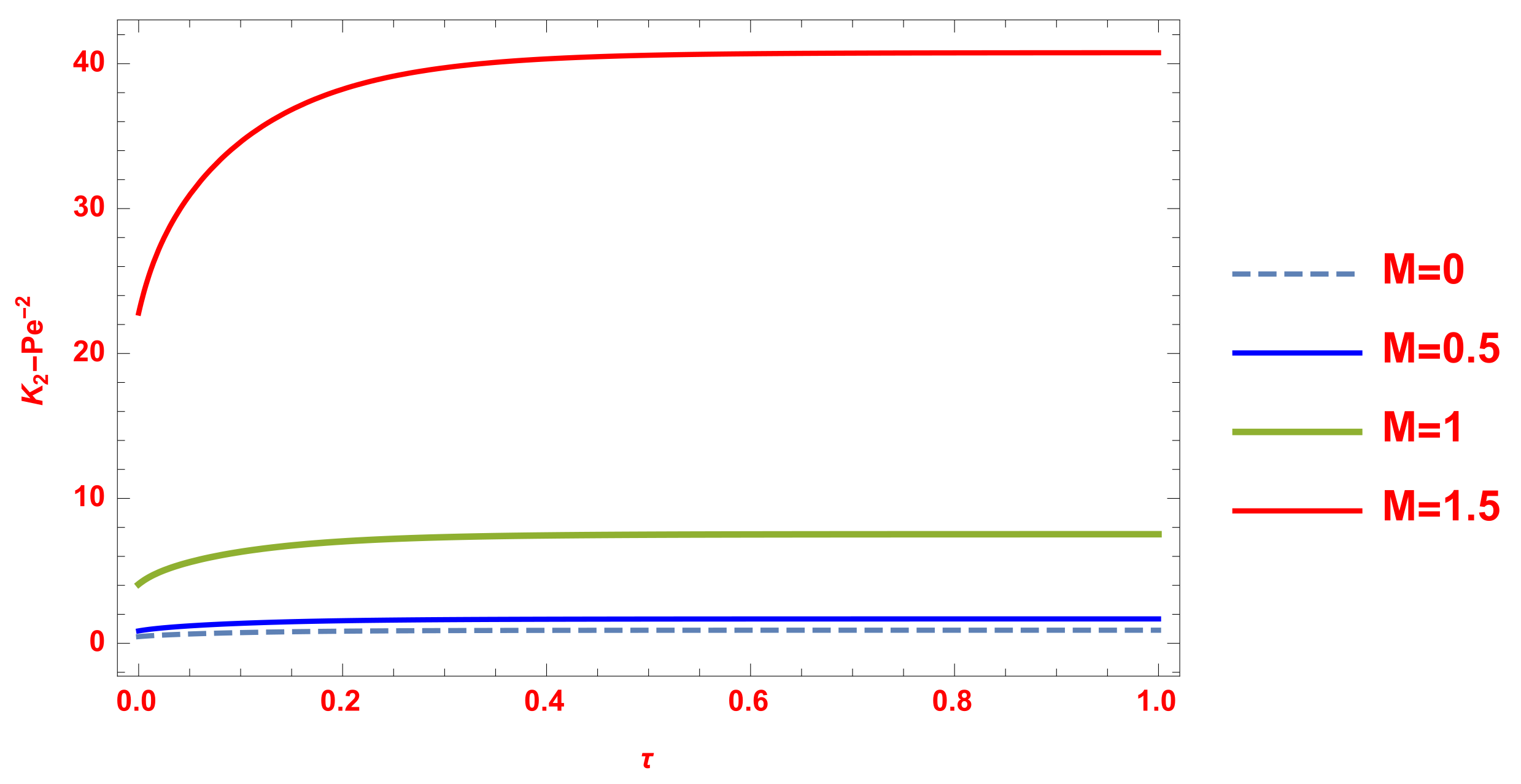
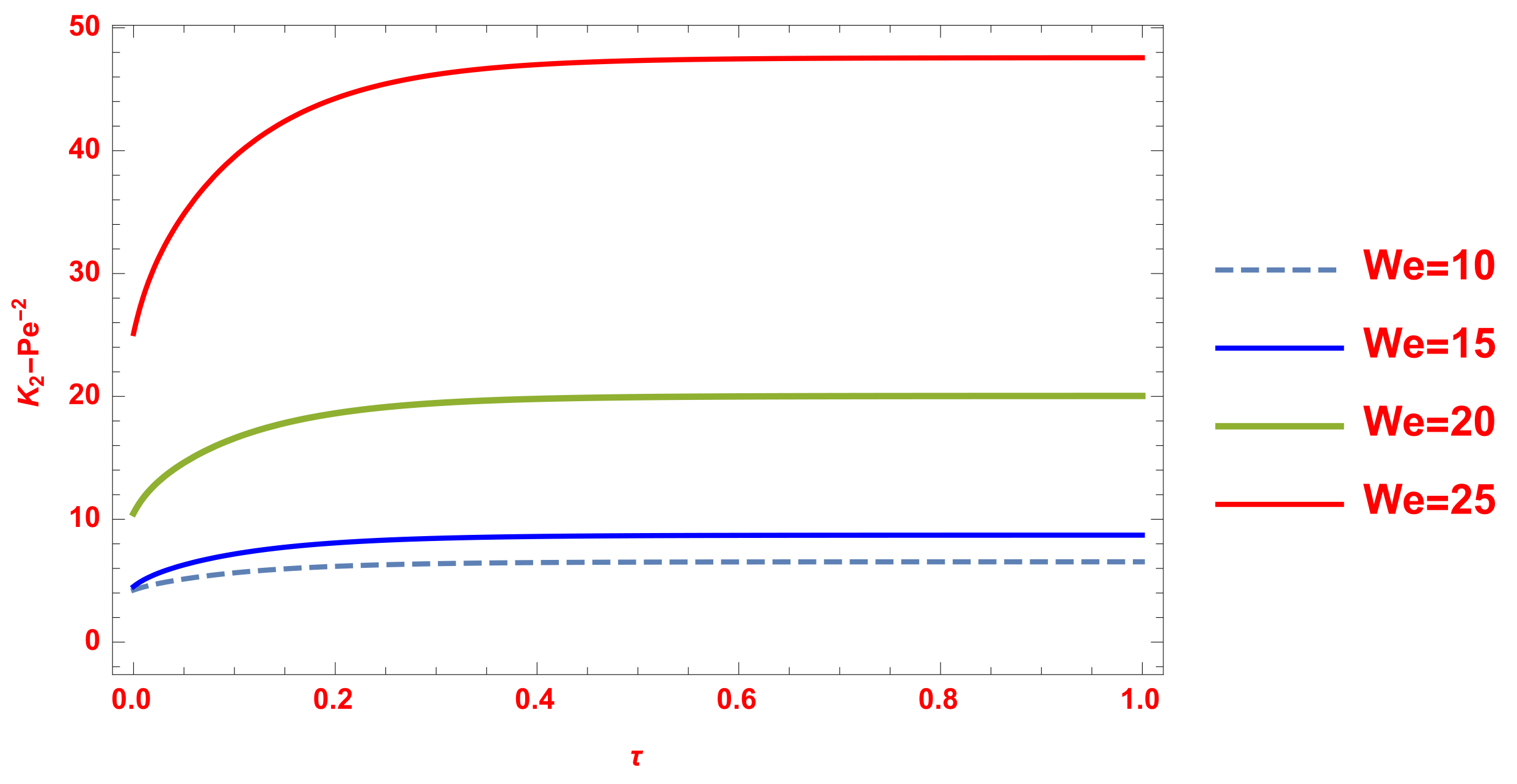
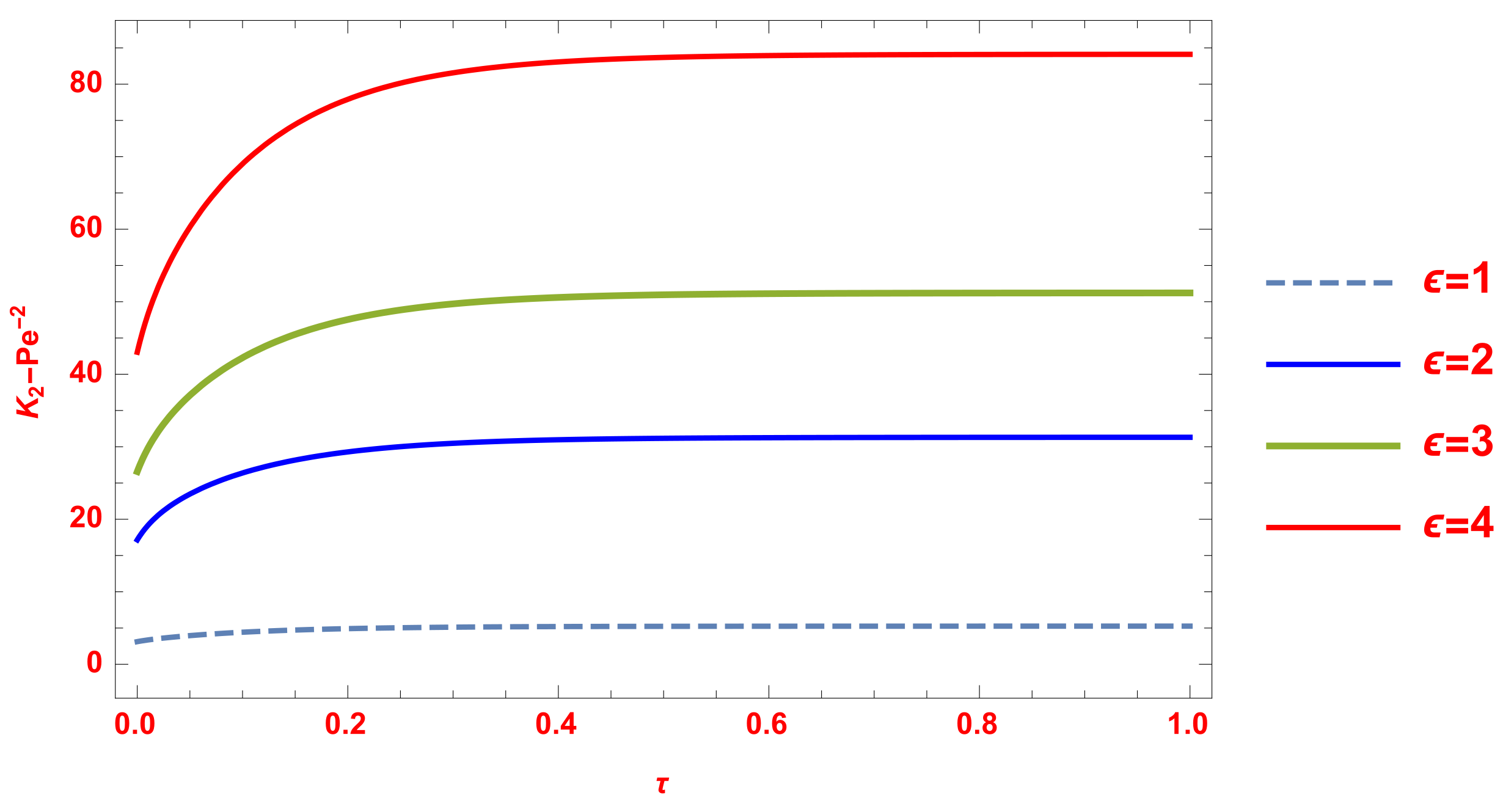
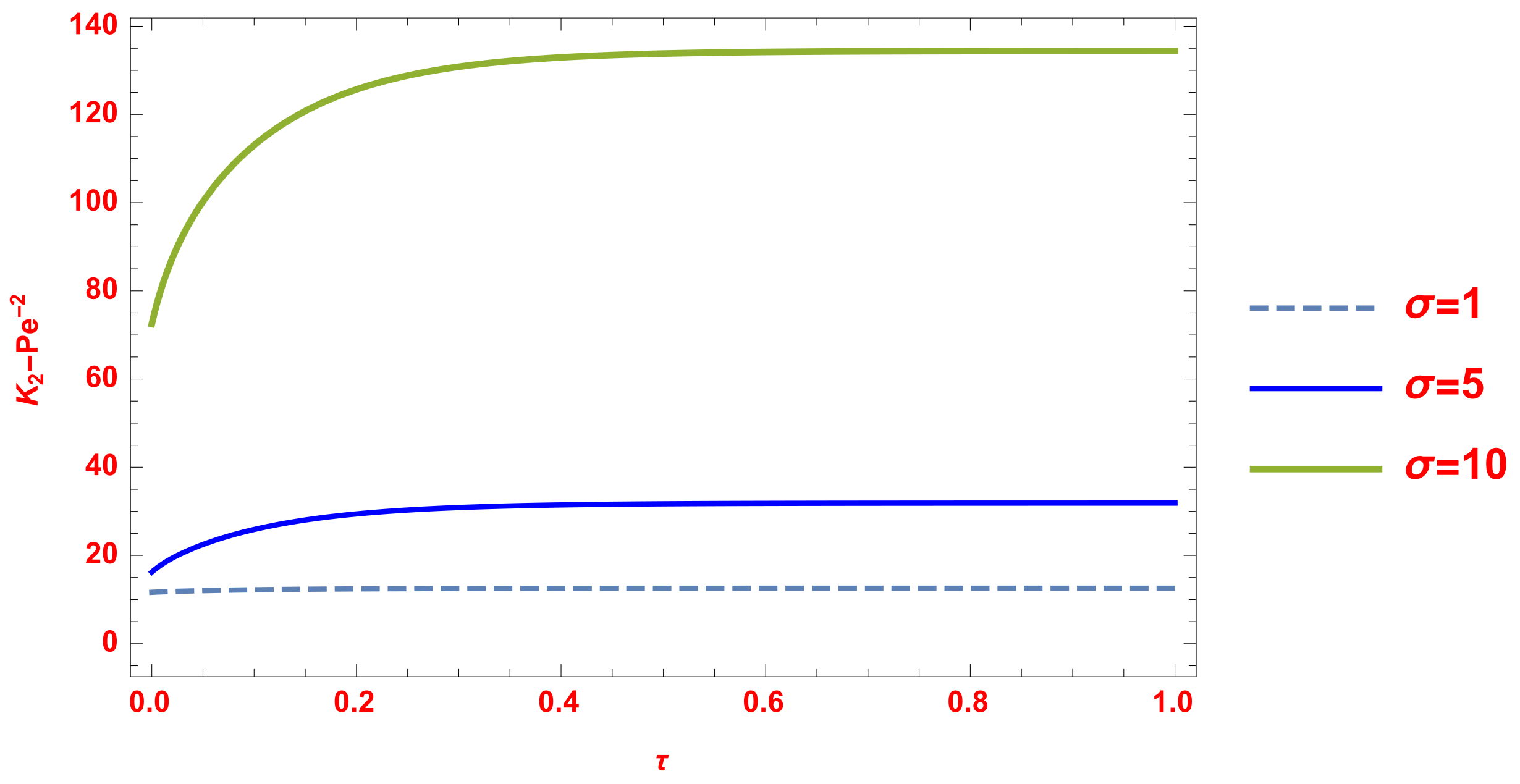
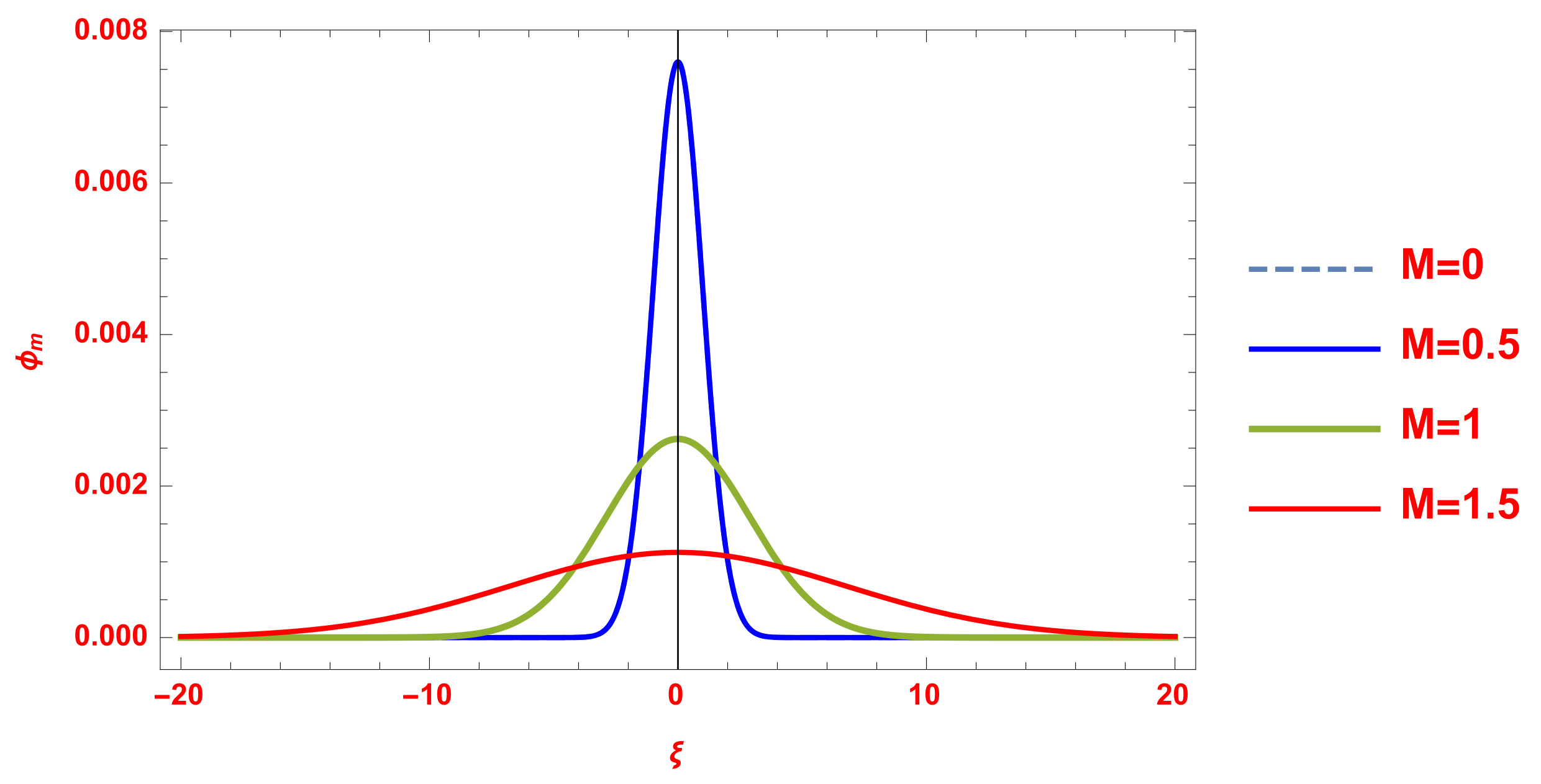
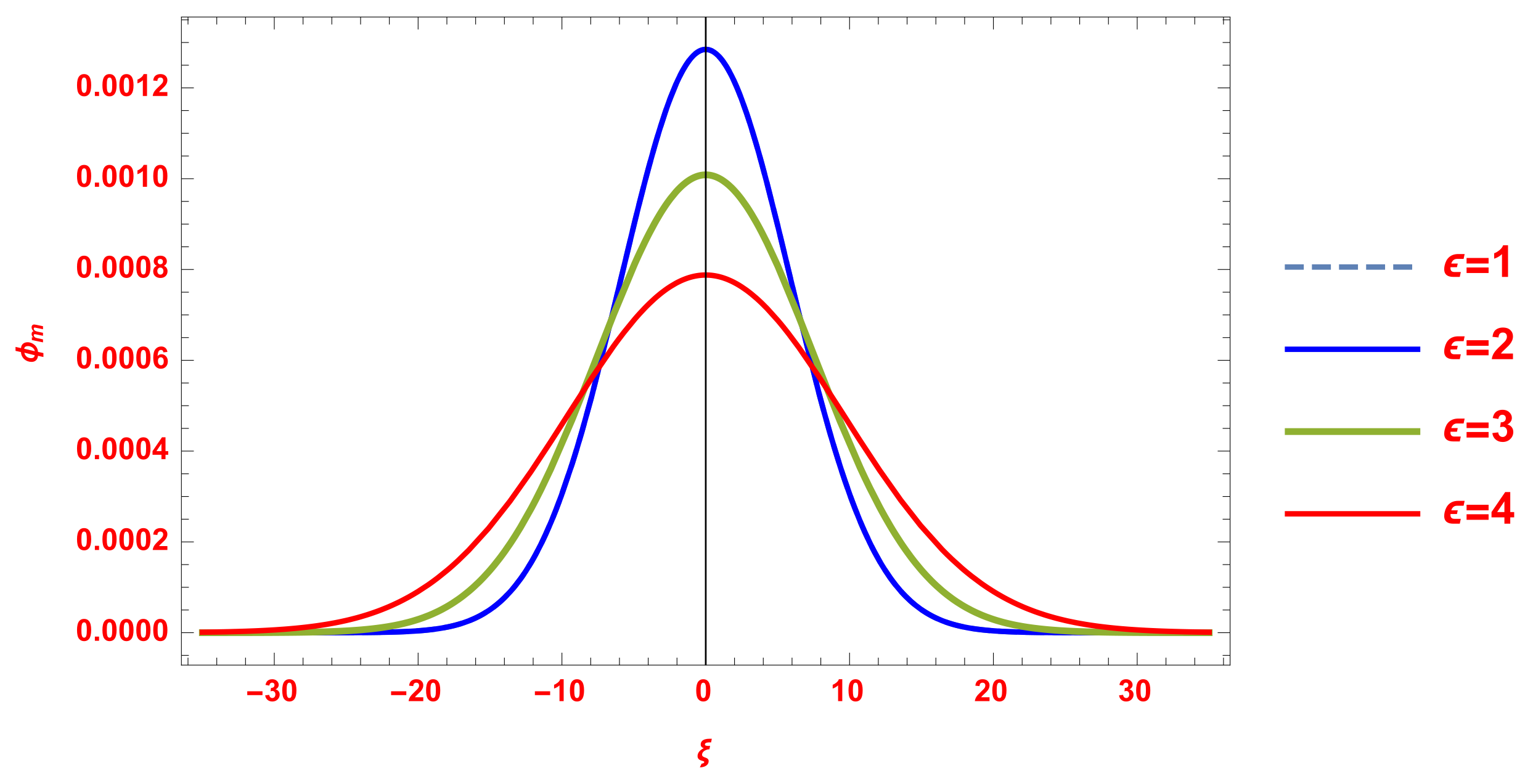
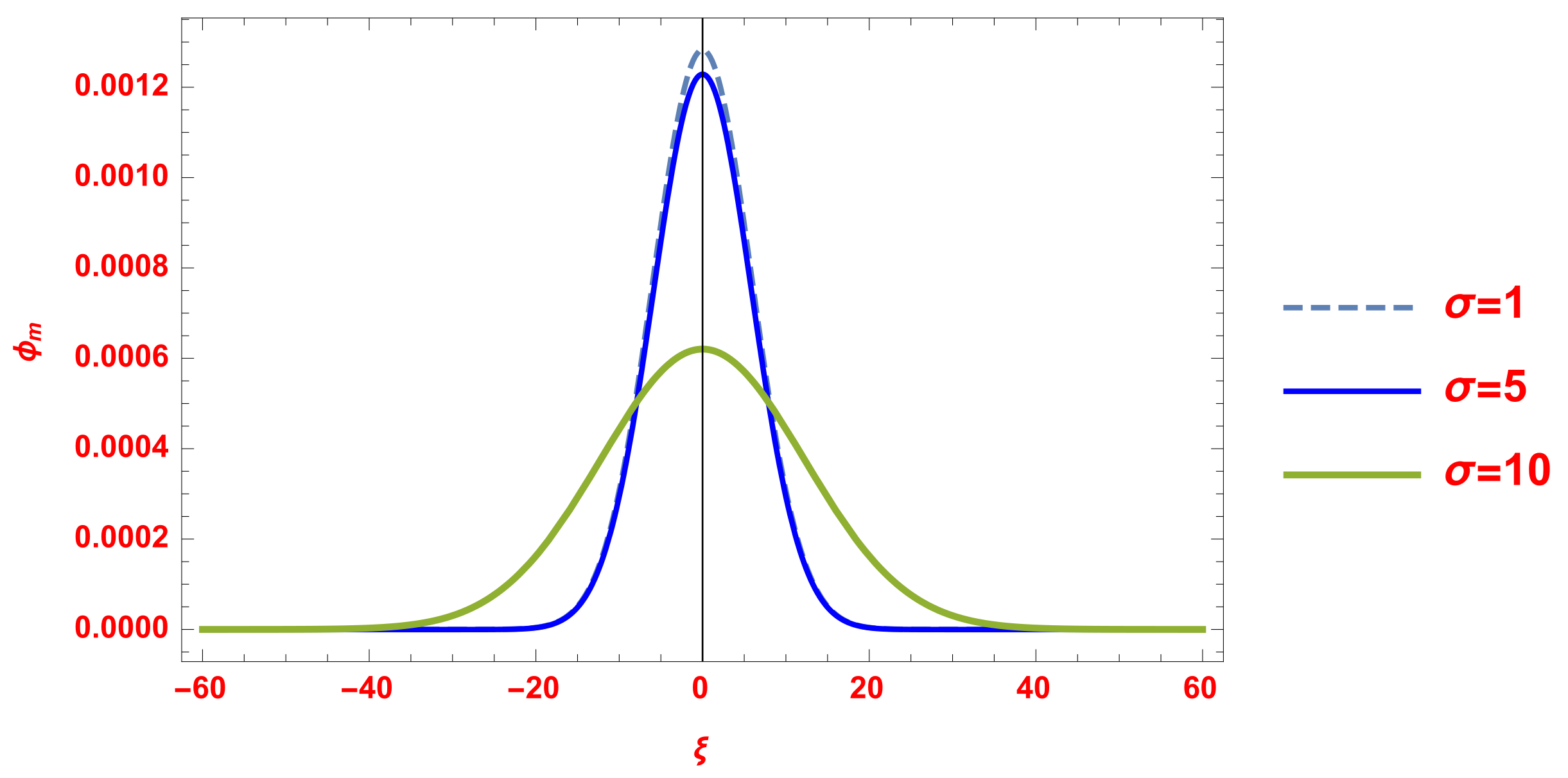
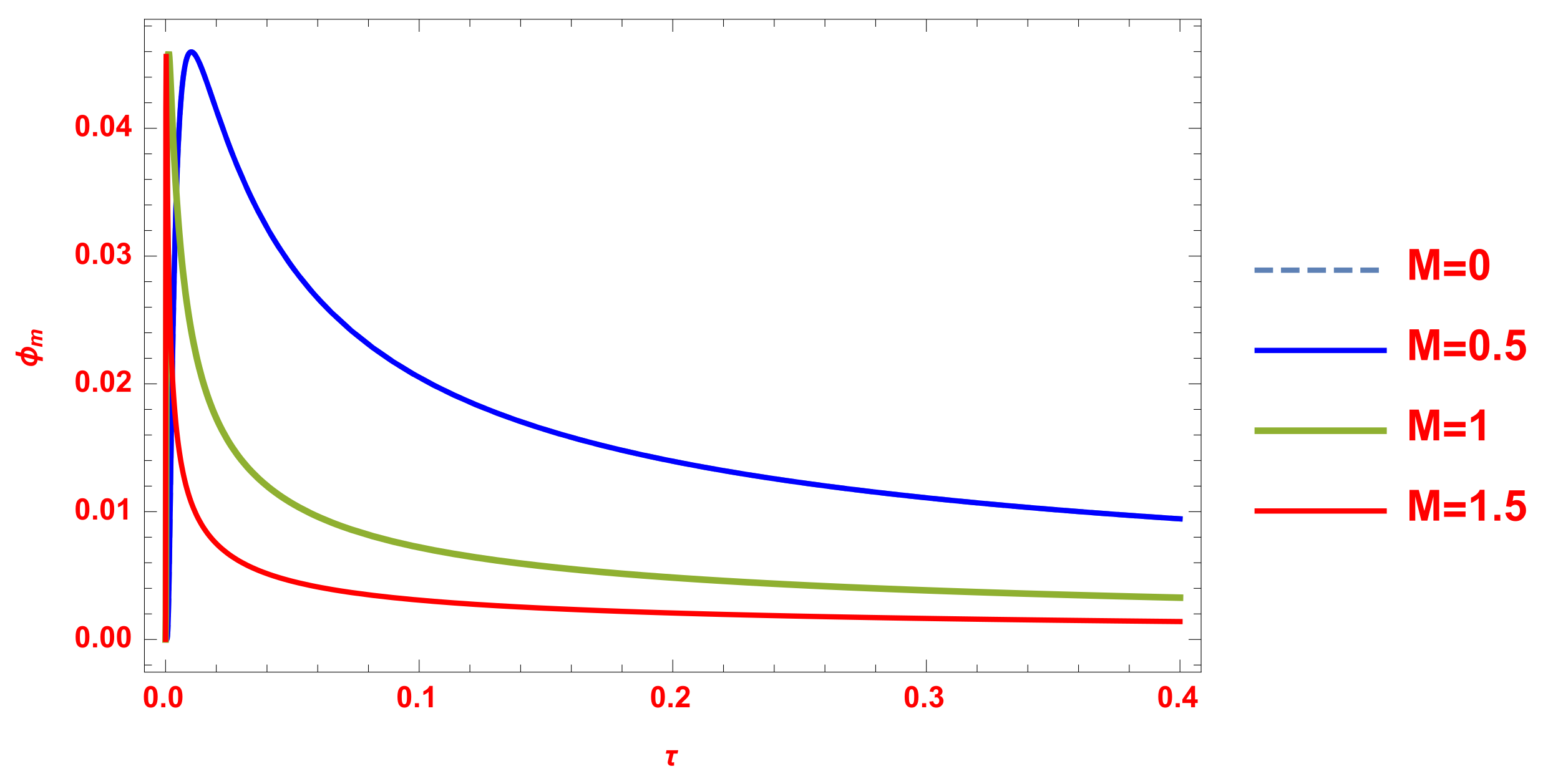
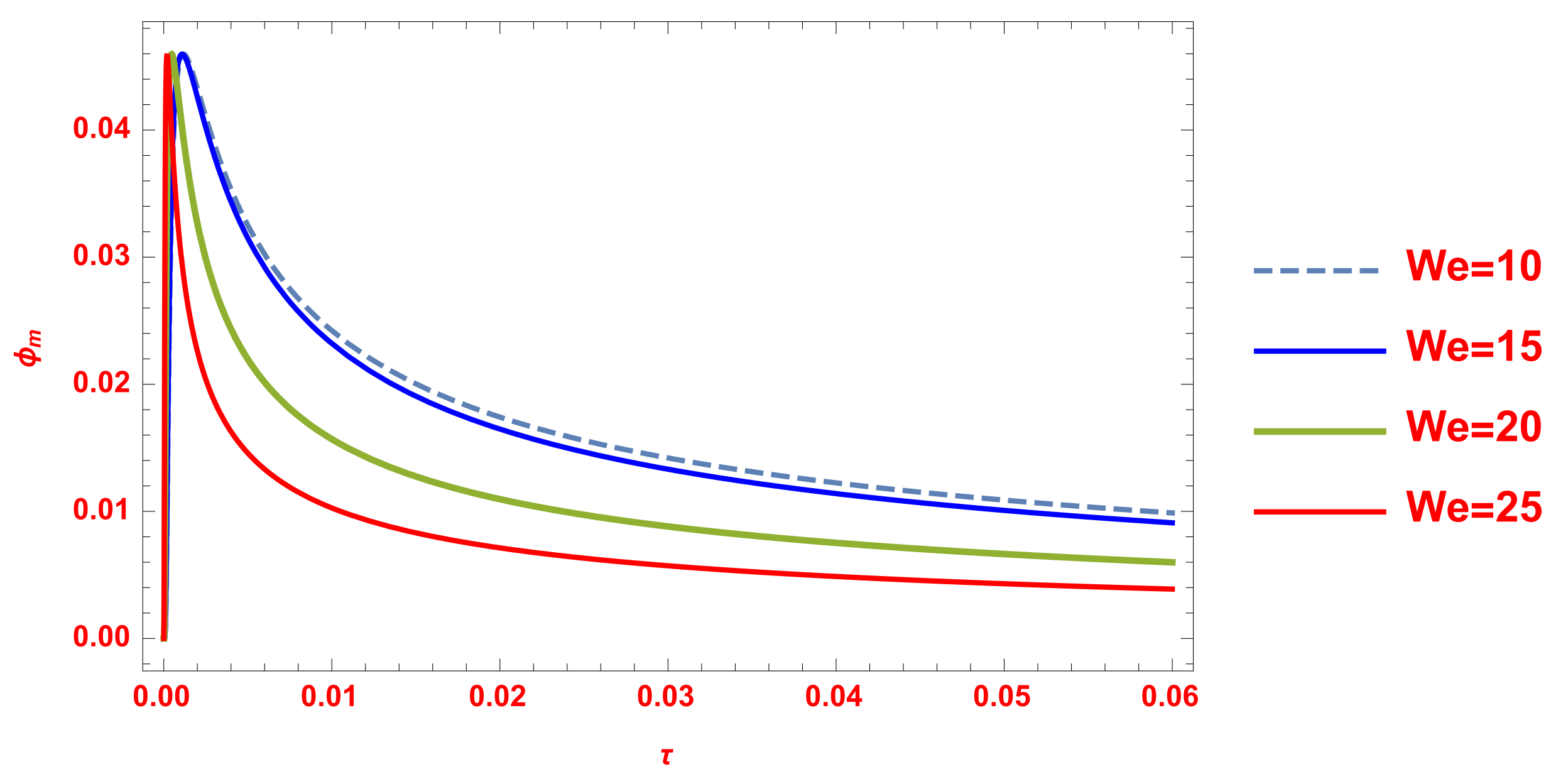
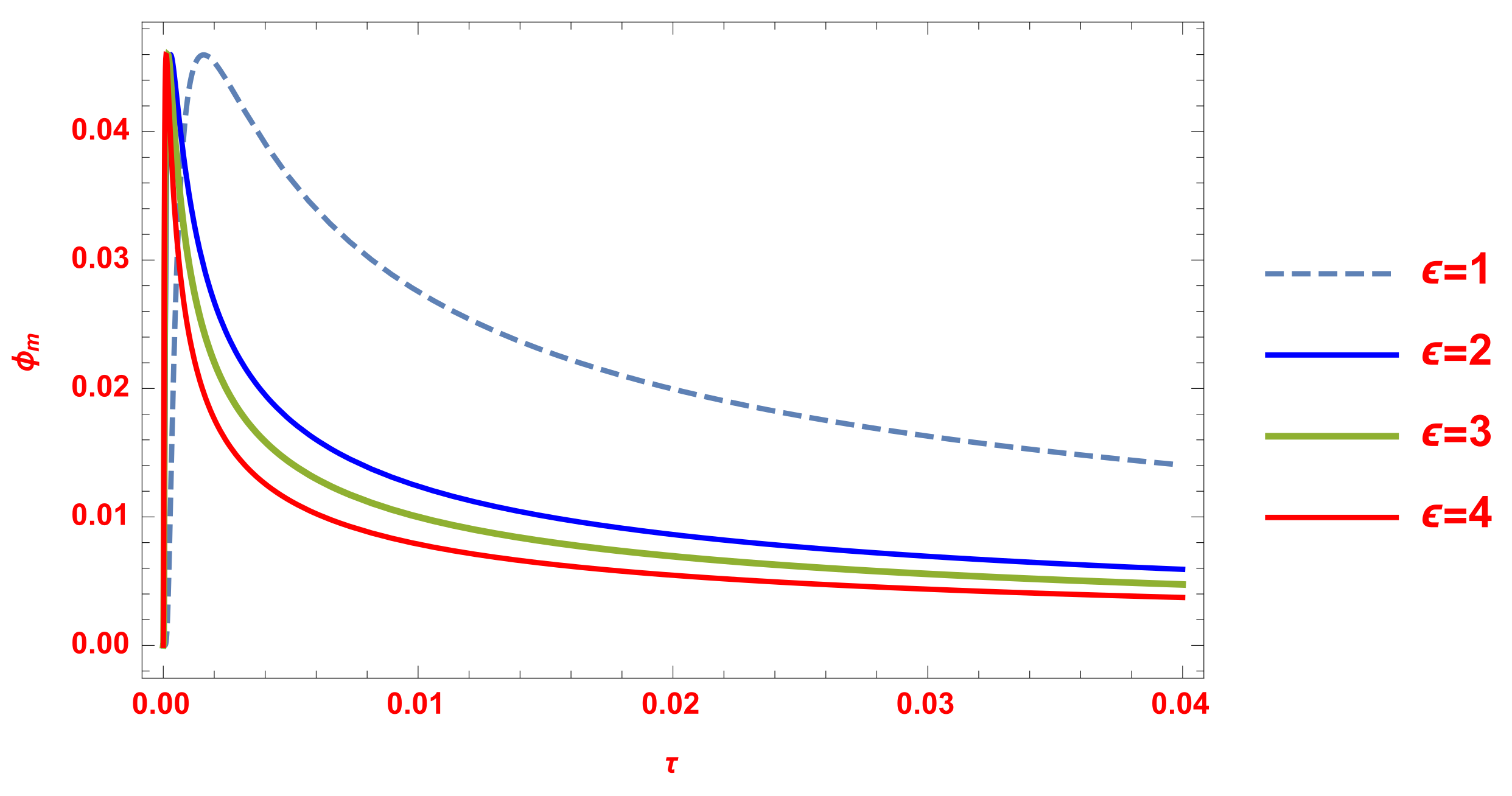
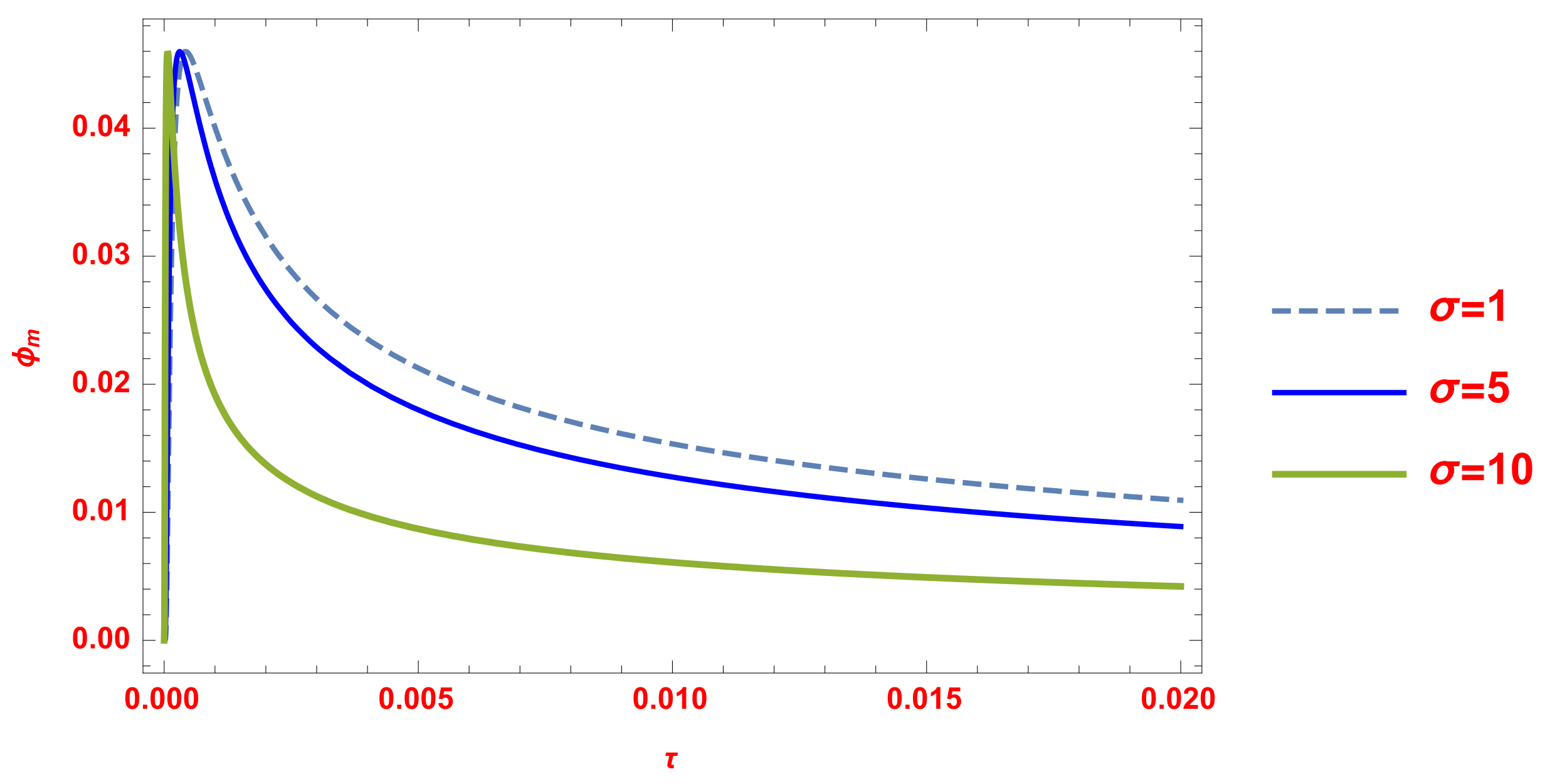
4. Results and Discussion
5. Conclusions
- Dispersion is accelerated by electromagnetic fields and other physical factors.
- In contrast to electromagnetic fields and other physical factors, the mean concentration drops as axial distance and time increase.
- Cells in the centre receive more nutrients than those in the periphery.
- The dispersion mechanism formula is used by orthopaedic surgeons to assess how well joints function.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Horizontal and normal components of the fluid velocity | |
| Cartesian coordinates | |
| Pressure | |
| average velocity | |
| Magnetic induction | |
| k | Permeability of porous medium |
| x component of electric field | |
| Species concentration | |
| Initial species concentration | |
| Diffusion coefficient | |
| Dispersion coefficient | |
| M | Hartmann number |
| Electric number | |
| Reynolds number | |
| Peclet number | |
| Darcy number | |
| Greek Symbols | |
| Dynamic viscosity | |
| Kinematic viscosity | |
| Slip parameter | |
| Electrical conductivity | |
| Porous parameter | |
| Viscoelastic parameter | |
| Concentration | |
| Mean concentration | |
| Dimensionless charge density | |
| Dimensionless time | |
| Dimensionless axial distance | |
References
- Alshehri, M.; Sharma, S.K. Computational model for the generalised dispersion of synovial fluid. Int. J. Adv. Comput. Sci. Appl. 2017, 8, 134–138. [Google Scholar] [CrossRef] [Green Version]
- Zahn, M.; Shenton, K.E. Magnetic Fluids Bibliography. IEEE Trans. 1980, 16, 387–415. [Google Scholar] [CrossRef]
- Akbar, N.S.; Nadeem, S.; Noor, N.F.M. Free convective MHD peristaltic flow of a Jeffrey nanofluid with convective surface boundary condition: A biomedicine–Nano model. Curr. Nanosci. 2014, 10, 432–440. [Google Scholar] [CrossRef]
- Faghiri, S.; Akbari, S.; Shafii, M.B.; Hosseinzadeh, K. Hydrothermal analysis of non-Newtonian fluid flow (blood) through the circular tube under prescribed non-uniform wall heat flux. Theor. Appl. Mech. Lett. 2022, 12, 100360. [Google Scholar] [CrossRef]
- Gulzar, M.M.; Aslam, A.; Waqas, M.; Javed, M.A.; Hosseinzadeh, K. A nonlinear mathematical analysis for magneto-hyperbolic-tangent liquid featuring simultaneous aspects of magnetic field, heat source and thermal stratification. Appl. Nanosci. 2020, 10, 4513–4518. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Shah, Z.; Baleanu, D.; Sherif, E.S.M. Impact of magnetic field on boundary-layer flow of Sisko liquid comprising nanomaterials migration through radially shrinking/stretching surface with zero mass flux. J. Mater. Res. Technol. 2020, 9, 3699–3709. [Google Scholar] [CrossRef]
- Attar, M.A.; Roshani, M.; Hosseinzadeh, K.; Ganji, D.D. Analytical solution of fractional differential equations by Akbari–Ganji’s method. Partial. Differ. Equations Appl. Math. 2022, 6, 100450. [Google Scholar] [CrossRef]
- Hossain, M.S.; Fayz-Al-Asad, M.; Mallik, M.S.I.; Yavuz, M.; Alim, M.A.; Khairul Basher, K.M. Numerical Study of the Effect of a Heated Cylinder on Natural Convection in a Square Cavity in the Presence of a Magnetic Field. Math. Comput. Appl. 2022, 27, 58. [Google Scholar] [CrossRef]
- Bhuvaneswari, M.; Sivasankaran, S.; Kim, Y.J. Magnetoconvection in a square enclosure with sinusoidal temperature distributions on both side walls. Numer. Heat Transf. Part A Appl. 2011, 59, 167–184. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Malleswaran, A.; Bhuvaneswari, M.; Balan, P. Hydro-magnetic mixed convection in a lid-driven cavity with partially thermally active walls. Sci. Iran. 2017, 24, 153–163. [Google Scholar] [CrossRef]
- Narrein, K.; Sivanandam, S.; Ganesan, P. Influence of transverse magnetic field on microchannel heat sink performance. J. Appl. Fluid Mech. 2016, 9, 3159–3166. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Narrein, K. Influence of geometry and magnetic field on convective flow of nanofluids in trapezoidal microchannel heat sink. Iran. J. Sci. Technol. Trans. Mech. Eng. 2020, 44, 373–382. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Bhuvaneswari, M.; Alzahrani, A.K. Numerical study on influence of magnetic field and discrete heating on free convection in a porous container. Sci. Iran. 2022. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Lee, J.; Bhuvaneswari, M. Effect of a partition on hydro-magnetic convection in an enclosure. Arab. J. Sci. Eng. 2011, 36, 1393–1406. [Google Scholar] [CrossRef]
- Bindhu, R.; Sai SundaraKrishnan, G.; Sivasankaran, S.; Bhuvaneswari, M. Magneto-convection of water near its maximum density in a cavity with partially thermally active walls. Energy Environ. 2019, 30, 833–853. [Google Scholar] [CrossRef]
- Bhuvaneswari, M.; Sivasankaran, S.; Karthikeyan, S.; Rajan, S. Stratification and Cross Diffusion Effects on Magneto-Convection Stagnation-Point Flow in a Porous Medium with Chemical Reaction, Radiation, and Slip Effects. In Applied Mathematics and Scientific Computing; Birkhäuser: Cham, Switzerland, 2019; pp. 245–253. [Google Scholar] [CrossRef]
- Rashad, A.M.; Sivasankaran, S.; Mansour, M.A.; Bhuvaneswari, M. Magneto-convection of nanofluids in a lid-driven trapezoidal cavity with internal heat generation and discrete heating. Numer. Heat Transf. Part A Appl. 2017, 71, 1223–1234. [Google Scholar] [CrossRef]
- Niranjan, H.; Sivasankaran, S.; Bhuvaneswari, M. Analytical and numerical study on magnetoconvection stagnation-point flow in a porous medium with chemical reaction, radiation, and slip effects. Math. Probl. Eng. 2016, 2016, 4017076. [Google Scholar] [CrossRef] [Green Version]
- Akbar, N.S.; Maraj, E.N.; Noor, N.F.M.; Habib, M.B. Exact solutions of an unsteady thermal conductive pressure driven peristaltic transport with temperature-dependent nanofluid viscosity. Case Stud. Therm. Eng. 2022, 35, 102124. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Khan, I.; Nisar, K.S. Insight into the dynamics of transient blood conveying gold nanoparticles when entropy generation and Lorentz force are significant. Int. Commun. Heat Mass Transf. 2021, 127, 105415. [Google Scholar] [CrossRef]
- Kune, R.; Naik, H.S.; Reddy, B.S.; Chesneau, C. Role of Nanoparticles and Heat Source/Sink on MHD Flow of Cu-H2O Nanofluid Flow Past a Vertical Plate with Soret and Dufour Effects. Math. Comput. Appl. 2022, 27, 102. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Ananthan, S.S.; Abdul Hakeem, A.K. Mixed convection in a lid-driven cavity with sinusoidal boundary temperature at the bottom wall in the presence of magnetic field. Sci. Iran. 2016, 23, 1027–1036. [Google Scholar] [CrossRef] [Green Version]
- Sivasankaran, S.; Malleswaran, A.; Lee, J.; Sundar, P. Hydro-magnetic combined convection in a lid-driven cavity with sinusoidal boundary conditions on both sidewalls. Int. J. Heat Mass Transf. 2011, 54, 512–525. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Bhuvaneswari, M.; Kim, Y.J.; Ho, C.J.; Pan, K.L. Numerical study on magneto-convection of cold water in an open cavity with variable fluid properties. Int. J. Heat Fluid Flow 2011, 32, 932–942. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Bhuvaneswari, M. Effect of thermally active zones and direction of magnetic field on hydromagnetic convection in an enclosure. Therm. Sci. 2011, 15, 367–382. [Google Scholar] [CrossRef]
- Tandon, P.N.; Chaurasia, A.; Jain, V.K.; Gupta, T. Application of magnetic fields to synovial joints. Comput. Math. Appl. 1991, 22, 33–45. [Google Scholar] [CrossRef] [Green Version]
- Rudraiah, N.; Kasiviswanathan, S.R.; Kaloni, P.N. Generalized dispersion in a synovial fluid of human joints. Biorheology 1991, 28, 207–219. [Google Scholar] [CrossRef]
- Gill, W.N.; Sankarasubramanian, R. Exact analysis of unsteady convective diffusion. Proc. R. Soc. Lond. A Math. Phys. Sci. 1970, 316, 341–350. [Google Scholar] [CrossRef]
- Ng, C.O.; Rudraiah, N.; Naiaraj, C.; Nagaraj, H.N. Electrohydrodynamic dispersion of macromolecular components in nanostructured biological bearing. J. Energy Heat Mass Transf. 2005, 27, 39–64. [Google Scholar]
- Khan, A.A.; Farooq, A.; Vafai, K. Impact of induced magnetic field on synovial fluid with peristaltic flow in an asymmetric channel. J. Magn. Magn. Mater. 2018, 446, 54–67. [Google Scholar] [CrossRef]
- Nagaraj, C.; Dinesh, P.A.; Kalavathi, G.K. Combined effects of electric field and magnetic field on electro hydrodynamic dispersion of macromolecular components in biological bearing. Defect Diffus. Forum 2018, 388, 361–377. [Google Scholar] [CrossRef]
- Redeker, J.I.; Schmitt, B.; Grigull, N.P.; Braun, C.; Büttner, A.; Jansson, V.; Mayer-Wagner, S. Effect of electromagnetic fields on human osteoarthritic and non-osteoarthritic chondrocytes. BMC Complement. Altern. Med. 2017, 17, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Racine, J. Effects of electromagnetic fields on articular cells and osteoarthritis. Orthop. Proc. 2018, 100 (Supp. 15), 72. [Google Scholar] [CrossRef]
- Ramakrishnan, K.; Swetha, N.N. Influence of porous parameter and thickness of the porous plate on the flow of synovial fluid in Human Joints. Int. J. Pure Appl. Math. 2018, 119, 221–229. [Google Scholar]
- Beretta, G.; Mastorgio, A.F.; Pedrali, L.; Saponaro, S.; Sezenna, E. The effects of electric, magnetic and electromagnetic fields on microorganisms in the perspective of bioremediation. Rev. Environ. Sci. Bio/Technol. 2019, 18, 29–75. [Google Scholar] [CrossRef] [Green Version]
- VijayaKumar, R.; Ratchagar, N.P. Mathematical Modeling of Synovial Joints with Chemical Reaction. J. Phys. Conf. Ser. 2021, 1724, 012051. [Google Scholar] [CrossRef]
- Tandon, P.N.; Nirmala, P.; Pal, T.S.; Agarwal, R. Rheological study of lubricant gelling in synovial joints during articulation. Appl. Math. Model. 1988, 12, 72–77. [Google Scholar] [CrossRef]
- Bali, R.; Shukla, A.K. Rheological effects of synovial fluid on nutritional transport. Tribol. Lett. 2001, 9, 233–239. [Google Scholar] [CrossRef]
- Beavers, G.S.; Joseph, D.D. Boundary conditions at a naturally permeable wall. J. Fluid Mech. 1967, 30, 197–207. [Google Scholar] [CrossRef]
- Rao K., S. Introduction to Partial Differential Equations, 3rd ed.; Prentice-Hall of India: New Delhi, India, 2010. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kumar, B.R.; Vijayakumar, R.; Rani, A.J. A Model for the Generalised Dispersion of Synovial Fluids on Nutritional Transport with Joint Impacts of Electric and Magnetic Field. Math. Comput. Appl. 2023, 28, 3. https://doi.org/10.3390/mca28010003
Kumar BR, Vijayakumar R, Rani AJ. A Model for the Generalised Dispersion of Synovial Fluids on Nutritional Transport with Joint Impacts of Electric and Magnetic Field. Mathematical and Computational Applications. 2023; 28(1):3. https://doi.org/10.3390/mca28010003
Chicago/Turabian StyleKumar, B. Rushi, R. Vijayakumar, and A. Jancy Rani. 2023. "A Model for the Generalised Dispersion of Synovial Fluids on Nutritional Transport with Joint Impacts of Electric and Magnetic Field" Mathematical and Computational Applications 28, no. 1: 3. https://doi.org/10.3390/mca28010003





