Low-Energy Elastic Electron Scattering from Helium Atoms
Abstract
:1. Introduction
2. Numerical Methods
2.1. Relativistic Polarized Orbital Approach
2.2. Nonrelativistic B-Spline R-Matrix with Pseudostates
3. Results and Discussion
3.1. Scattering Phase
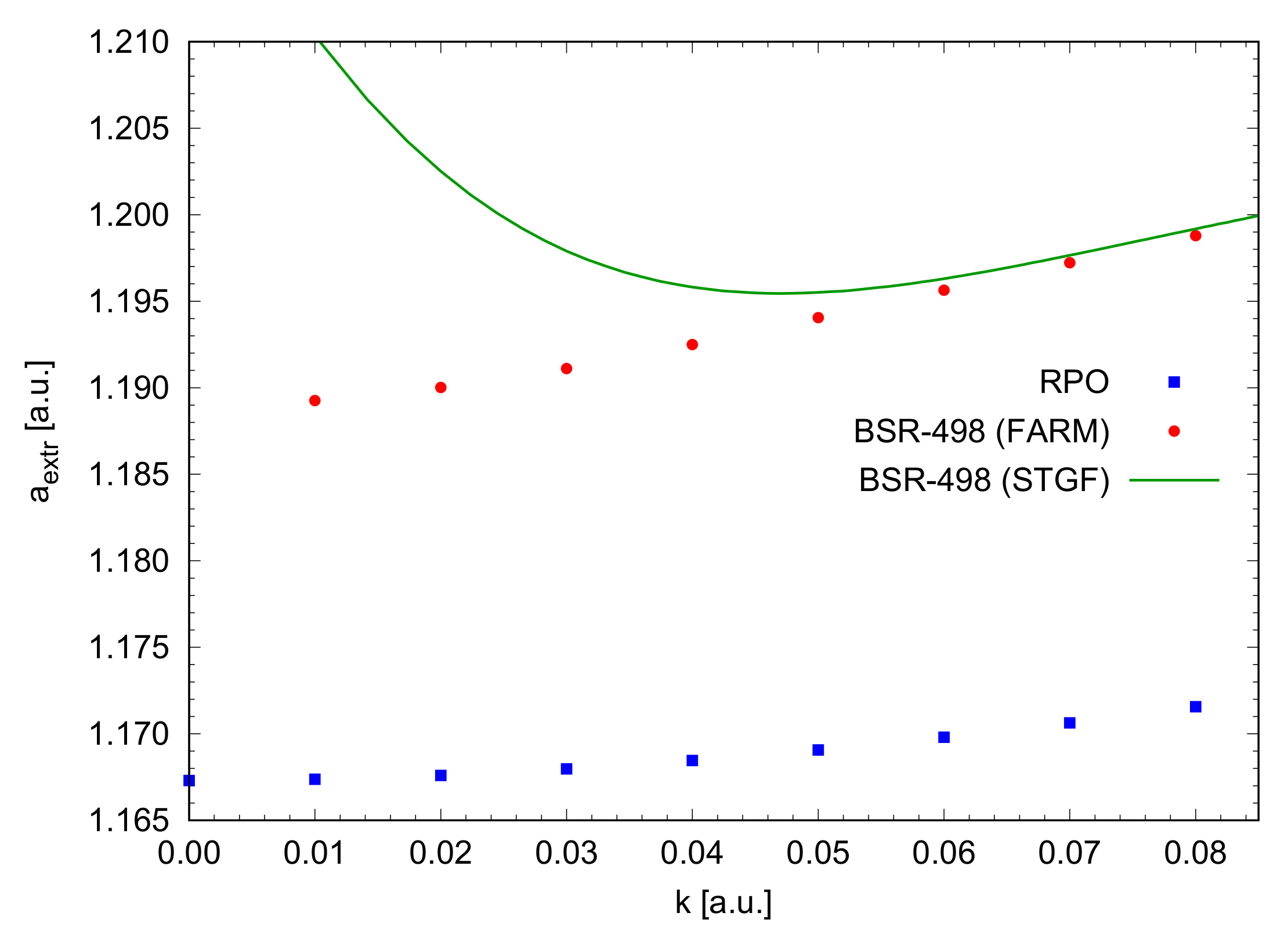
3.2. Scattering Length
3.3. Cross Sections

4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| RPO | Relativistic Polarized Orbital |
| BSR | B-Spline R-matrix |
References
- Wan, J.Y.; Wu, M.S.; Zhang, J.Y.; Yan, Z.C. Confined variational calculations of low-energy electron-helium scattering. Phys. Rev. A 2021, 103, 042814. [Google Scholar] [CrossRef]
- Atomic Spectra Database. Available online: https://www.nist.gov/pml/atomic-spectra-database (accessed on 12 September 2021).
- Brunger, M.J.; Buckman, S.J. Electron–molecule scattering cross-sections. I. Experimental techniques and data for diatomic molecules. Phys. Rep. 2002, 357, 215–458. [Google Scholar] [CrossRef]
- Gopalan, A.; Bömmels, J.; Götte, S.; Landwehr, A.; Franz, K.; Ruf, M.W.; Hotop, H.; Bartschat, K. A novel electron scattering apparatus combining a laser photoelectron source and a triply differentially pumped supersonic beam target: Characterization and results for the He−(1s2s2) resonance. Eur. Phys. J. D 2002, 22, 17–29. [Google Scholar] [CrossRef]
- Zatsarinny, O.; Bartschat, K. The B-spline R-matrix method for atomic processes: Application to atomic structure, electron collisions, and photoionization. J. Phys. B At. Mol. Opt. Phys. 2013, 46, 112001. [Google Scholar] [CrossRef]
- Zatsarinny, O. BSR: B-Spline Atomic R-Matrix Codes. Comp. Phys. Commun. 2006, 174, 273. [Google Scholar] [CrossRef]
- Oleg Zatsarinny’s GitHub Repository. Available online: https://github.com/zatsaroi (accessed on 12 September 2021).
- Atomic, Molecular, and Optical Sciences Gateway. Available online: https://ampgateway.org (accessed on 12 September 2021).
- Nesbet, R.K. Variational calculations of accurate e--He cross sections below 19 eV. Phys. Rev. A 1979, 20, 58–70. [Google Scholar] [CrossRef]
- Fursa, D.V.; Bray, I. Calculation of electron-helium scattering. Phys. Rev. A 1995, 52, 1279. [Google Scholar] [CrossRef] [PubMed]
- Hudson, E.T.; Bartschat, K.; Scott, M.P.; Burke, P.G.; Burke, V.M. Electron scattering from helium atoms. Phase shifts, resonance parameters and total cross sections. J. Phys. B At. Mol. Opt. Phys. 1996, 29, 5513. [Google Scholar] [CrossRef]
- Chen, S.; McEachran, R.P.; Stauffer, A.D. Ab initio optical potentials for elastic electron and positron scattering from the heavy noble gases. J. Phys. B At. Mol. Opt. Phys. 2008, 41, 025201. [Google Scholar] [CrossRef]
- McEachran, R.P.; Blanco, F.; García, G.; Brunger, M.J. A Relativistic Complex Optical Potential Calculation for Electron–Beryllium Scattering: Recommended Cross Sections. J. Phys. Chem. Ref. Data 2018, 47, 033103. [Google Scholar] [CrossRef]
- McEachran, R.P.; Blanco, F.; García, G.; Stokes, P.W.; White, R.D.; Brunger, M.J. Integral Cross Sections for Electron–Magnesium Scattering Over a Broad Energy Range (0–5000 eV). J. Phys. Chem. Ref. Data 2018, 47, 043104. [Google Scholar] [CrossRef]
- Grant, I.; McKenzie, B.; Norrington, P.; Mayers, D.; Pyper, N. An atomic multiconfigurational Dirac-Fock package. Comput. Phys. Commun. 1980, 21, 207–231. [Google Scholar] [CrossRef]
- Hibbert, A. Energies and Oscillator Strengths Using Configuration Interaction Wavefunctions. In Computational Atomc Physics; Bartschat, K., Ed.; Springer: Heidelberg, Germany; New York, NY, USA, 1996; Chapter 3; pp. 27–64. [Google Scholar]
- McEachran, R.P.; Morgan, D.L.; Ryman, A.G.; Stauffer, A.D. Positron scattering from noble gases: Corrected results for helium. J. Phys. B At. Mol. Phys. 1978, 11, 951–953. [Google Scholar] [CrossRef]
- McEachran, R.P.; Stauffer, A.D. Dynamic distortion effects in electron-atom scattering. J. Phys. B At. Mol. Opt. Phys. 1990, 23, 4605–4614. [Google Scholar] [CrossRef]
- McEachran, R.P.; Stauffer, A.D. Relativistic Effects in Low-Energy Electron–Argon Scattering. Aust. J. Phys. 1997, 50, 511–5244. [Google Scholar] [CrossRef] [Green Version]
- Puchalski, M.; Szalewicz, K.; Lesiuk, M.; Jeziorski, B. QED calculation of the dipole polarizability of helium atom. Phys. Rev. A 2020, 101, 022505. [Google Scholar] [CrossRef] [Green Version]
- Wang, Y.S.; Kar, S.; Ho, Y.K. Dynamic Multipole Polarizabilities of Helium and Screened-Helium Atoms. Atoms 2020, 8, 90. [Google Scholar] [CrossRef]
- Zatsarinny, O.; Bartschat, K. Nonperturbative B-spline R-matrix-with-pseudostates calculations for electron-impact ionization of helium. Phys. Rev. A 2012, 85, 062709. [Google Scholar] [CrossRef]
- Pekeris, C.L. 11S and 23S States of Helium. Phys. Rev. 1959, 115, 1216–1221. [Google Scholar] [CrossRef]
- Bartschat, K.; Hudson, E.T.; Scott, M.P.; Burke, P.G.; Burke, V.M. Electron-atom scattering at low and intermediate energies using a pseudo-state/R-matrix basis. J. Phys. B At. Mol. Phys. 1996, 29, 115. [Google Scholar] [CrossRef]
- Berrington, K.A.; Eissner, W.; Norrington, P.H. RMATRIX-I: Belfast Atomic R-Matrix Codes. Comput. Phys. Commun. 1995, 92, 290. [Google Scholar] [CrossRef]
- O’Malley, T.F.; Spruch, L.; Rosenberg, L. Modification of Effective-Range Theory in the Presence of a Long-Range (r−4) Potential. J. Math. Phys. 1961, 2, 491. [Google Scholar] [CrossRef]
- Fernández-Menchero, L.; Conroy, A.; Ballance, C.; Badnell, N.; Mitnik, D.; Gorczyca, T.; Seaton, M. PSTGF: Time-independent R-matrix atomic electron-impact code. Comput. Phys. Commun. 2020, 256, 107489. [Google Scholar] [CrossRef]
- Burke, V.; Noble, C. Farm—A flexible asymptotic R-matrix package. Comput. Phys. Commun. 1995, 85, 471–500. [Google Scholar] [CrossRef]
- Ali, M.K.; Fraser, P.A. The contribution of long-range forces to low-energy phaseshifts. J. Phys. B At. Mol. Phys. 1977, 10, 3091–3104. [Google Scholar] [CrossRef]
- Alves, L.L.; Bartschat, K.; Biagi, S.F.; Bordage, M.C.; Pitchford, L.C.; Ferreira, C.M.; Hagelaar, G.J.M.; Morgan, W.L.; Pancheshnyi, S.; Phelps, A.V.; et al. Comparisons of sets of electron–neutral scattering cross sections and swarm parameters in noble gases: II. Helium and neon. J. Phys. D Appl. Phys. 2013, 46, 334002. [Google Scholar] [CrossRef]
- LXCat Database. Available online: https://nl.lxcat.net (accessed on 14 September 2021).
| k | Nesbet [9] | RMPS [11] | Wan et al. [1] | Present RPO | Present BSR-498 |
|---|---|---|---|---|---|
| 0.1 | −0.1282 | −0.1260 | −0.1281 | −0.1262 | −0.1286 |
| 0.2 | −0.2655 | −0.2607 | −0.2650 | −0.2614 | −0.2560 |
| 0.3 | −0.4021 | −0.3979 | −0.4030 | −0.3978 | −0.4043 |
| 0.4 | −0.5388 | −0.5380 | −0.5319 | −0.5398 | |
| 0.5 | −0.6684 | −0.6617 | −0.6678 | −0.6609 | −0.6699 |
| 0.6 | −0.7930 | −0.7848 | −0.7907 | −0.7835 | −0.7932 |
| 0.7 | −0.9067 | −0.9062 | −0.8990 | −0.9088 | |
| 0.8 | −1.0155 | −1.0085 | −1.103 | −1.0069 | −1.0162 |
| 0.9 | −1.1163 | −1.1077 | −1.112 | −1.1071 | −1.1151 |
| 1.0 | −1.2056 | −1.202 | −1.1998 | −1.2055 | |
| 1.1 | −1.2848 | −1.2789 | −1.291 | −1.2854 | −1.2863 |
| k | Nesbet [9] | RMPS [11] | Wan et al. [1] | Present RPO | Present BSR-498 |
|---|---|---|---|---|---|
| 0.1 | 0.00308 | 0.0026 | 0.003029 | 0.00304 | 0.00305 |
| 0.2 | 0.01311 | 0.0129 | 0.01269 | 0.01264 | 0.01261 |
| 0.3 | 0.03063 | 0.0306 | 0.02964 | 0.02944 | 0.02945 |
| 0.4 | 0.05519 | 0.05390 | 0.05348 | 0.05352 | |
| 0.5 | 0.08605 | 0.0880 | 0.08449 | 0.08380 | 0.08386 |
| 0.6 | 0.1209 | 0.1244 | 0.1209 | 0.11856 | 0.11865 |
| 0.7 | 0.1588 | 0.1588 | 0.15535 | 0.15556 | |
| 0.8 | 0.1960 | 0.2000 | 0.1960 | 0.19175 | 0.19211 |
| 0.9 | 0.2305 | 0.2349 | 0.2305 | 0.22576 | 0.22657 |
| 1.0 | 0.2626 | 0.2626 | 0.25608 | 0.25772 | |
| 1.1 | 0.2932 | 0.2934 | 0.2932 | 0.28211 | 0.28524 |
| k | Nesbet [9] | Wan et al. [1] | Present RPO | Present BSR-498 |
|---|---|---|---|---|
| 0.1 | 0.0004 | 0.0004693 | 0.00042 | 0.00079 |
| 0.2 | 0.0017 | 0.001658 | 0.00165 | 0.00178 |
| 0.3 | 0.0037 | 0.003658 | 0.00367 | 0.00366 |
| 0.4 | 0.0066 | 0.006497 | 0.00650 | 0.00651 |
| 0.5 | 0.0104 | 0.01006 | 0.01017 | 0.01014 |
| 0.6 | 0.0149 | 0.01448 | 0.01471 | 0.01462 |
| 0.7 | 0.0203 | 0.01970 | 0.02010 | 0.02002 |
| 0.8 | 0.0265 | 0.02567 | 0.02630 | 0.02629 |
| 0.9 | 0.0335 | 0.0323 | 0.03318 | 0.03326 |
| 1.0 | 0.0414 | 0.0396 | 0.04060 | 0.04112 |
| 1.1 | 0.0501 | 0.0450 | 0.04837 | 0.04969 |
| k | Nesbet [9] | Wan et al. [1] | Present RPO | Present BSR-498 |
|---|---|---|---|---|
| 0.01 | −0.01181 | −0.01224 | ||
| 0.02 | −0.02386 | −0.02430 | ||
| 0.03 | −0.03612 | −0.03679 | ||
| 0.04 | −0.04926 | −0.04919 | −0.04858 | −0.04949 |
| 0.05 | −0.06207 | −0.06219 | −0.06119 | −0.06236 |
| 0.06 | −0.07504 | −0.07516 | −0.07396 | −0.07539 |
| 0.07 | −0.08815 | −0.08824 | −0.08686 | −0.08855 |
| 0.08 | −0.09987 | −0.10183 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
McEachran, R.P.; Hamilton, K.R.; Bartschat, K. Low-Energy Elastic Electron Scattering from Helium Atoms. Atoms 2021, 9, 82. https://doi.org/10.3390/atoms9040082
McEachran RP, Hamilton KR, Bartschat K. Low-Energy Elastic Electron Scattering from Helium Atoms. Atoms. 2021; 9(4):82. https://doi.org/10.3390/atoms9040082
Chicago/Turabian StyleMcEachran, Robert P., Kathryn R. Hamilton, and Klaus Bartschat. 2021. "Low-Energy Elastic Electron Scattering from Helium Atoms" Atoms 9, no. 4: 82. https://doi.org/10.3390/atoms9040082






