Signatures of Quantum Chaos of Rydberg-Dressed Bosons in a Triple-Well Potential
Abstract
:1. Introduction
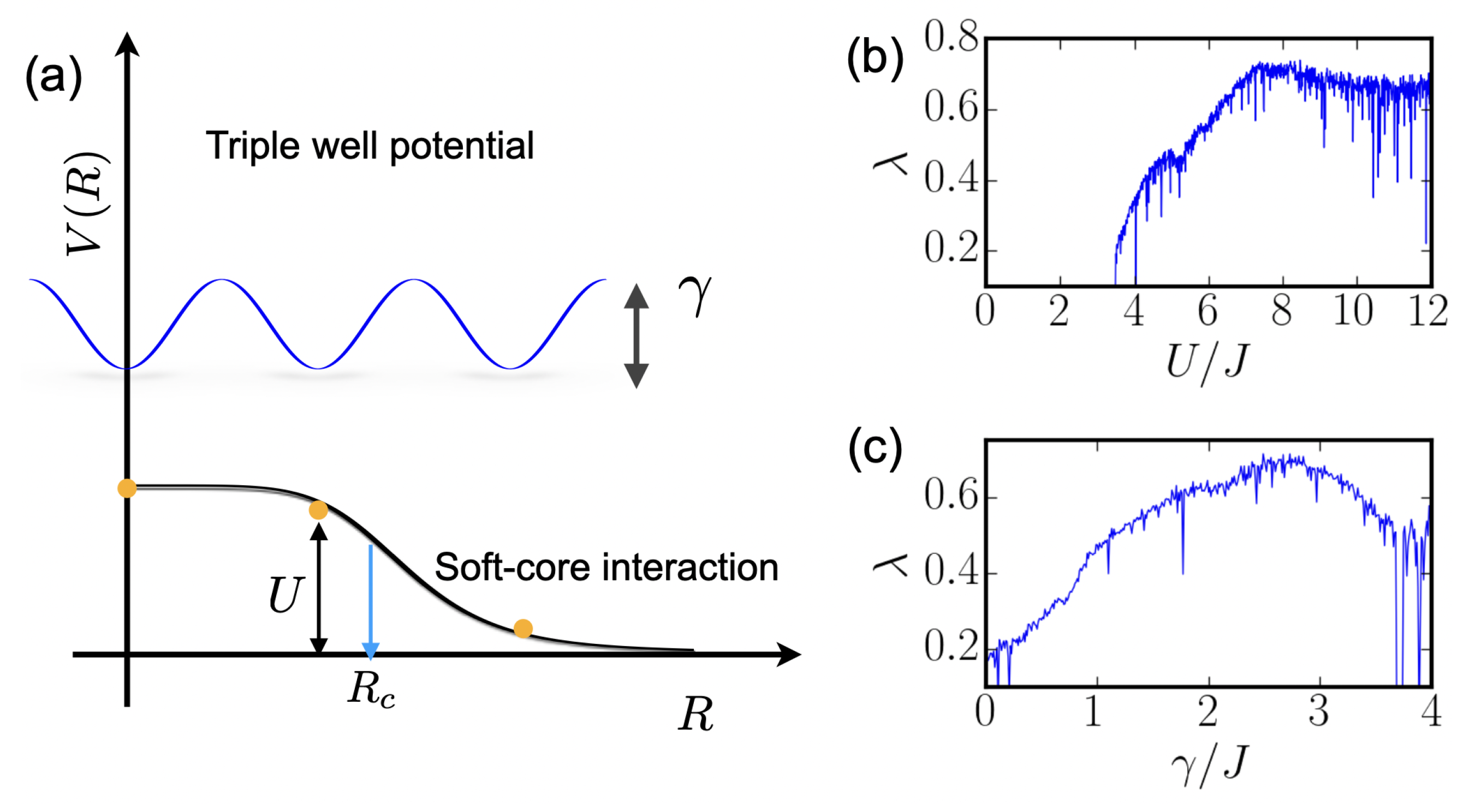
2. Model
3. Results
3.1. Level Statistics
3.2. Entanglement Entropy
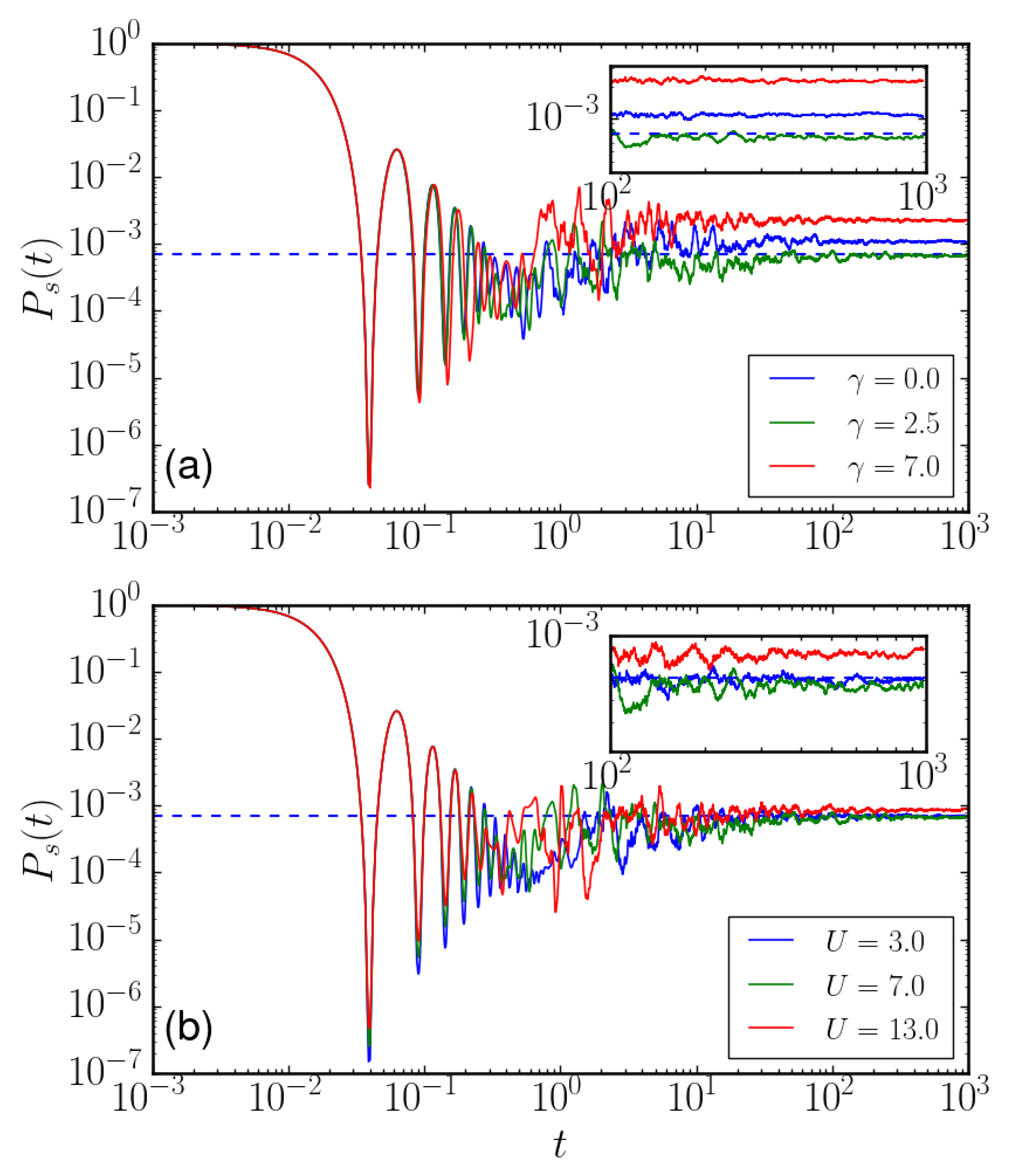
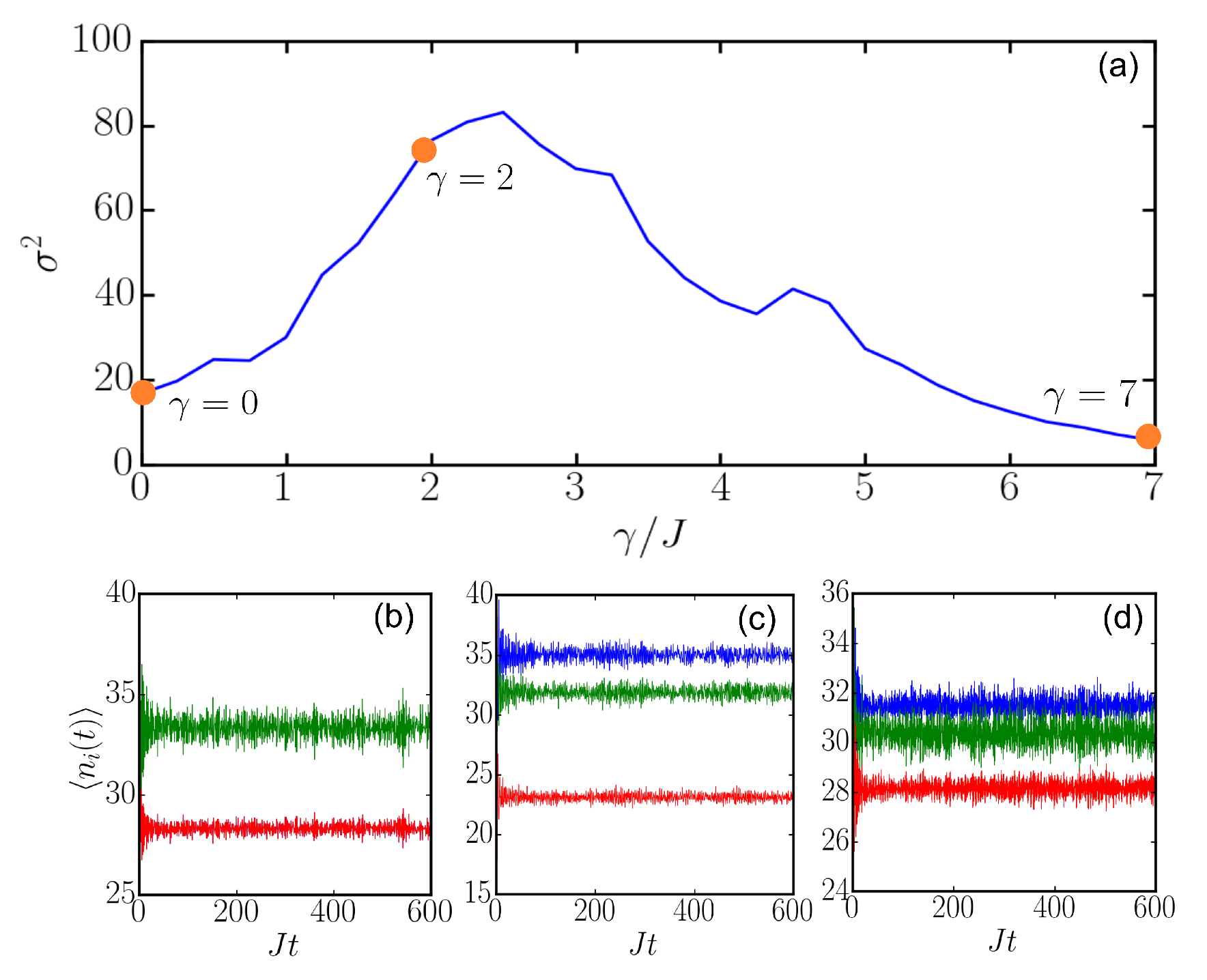
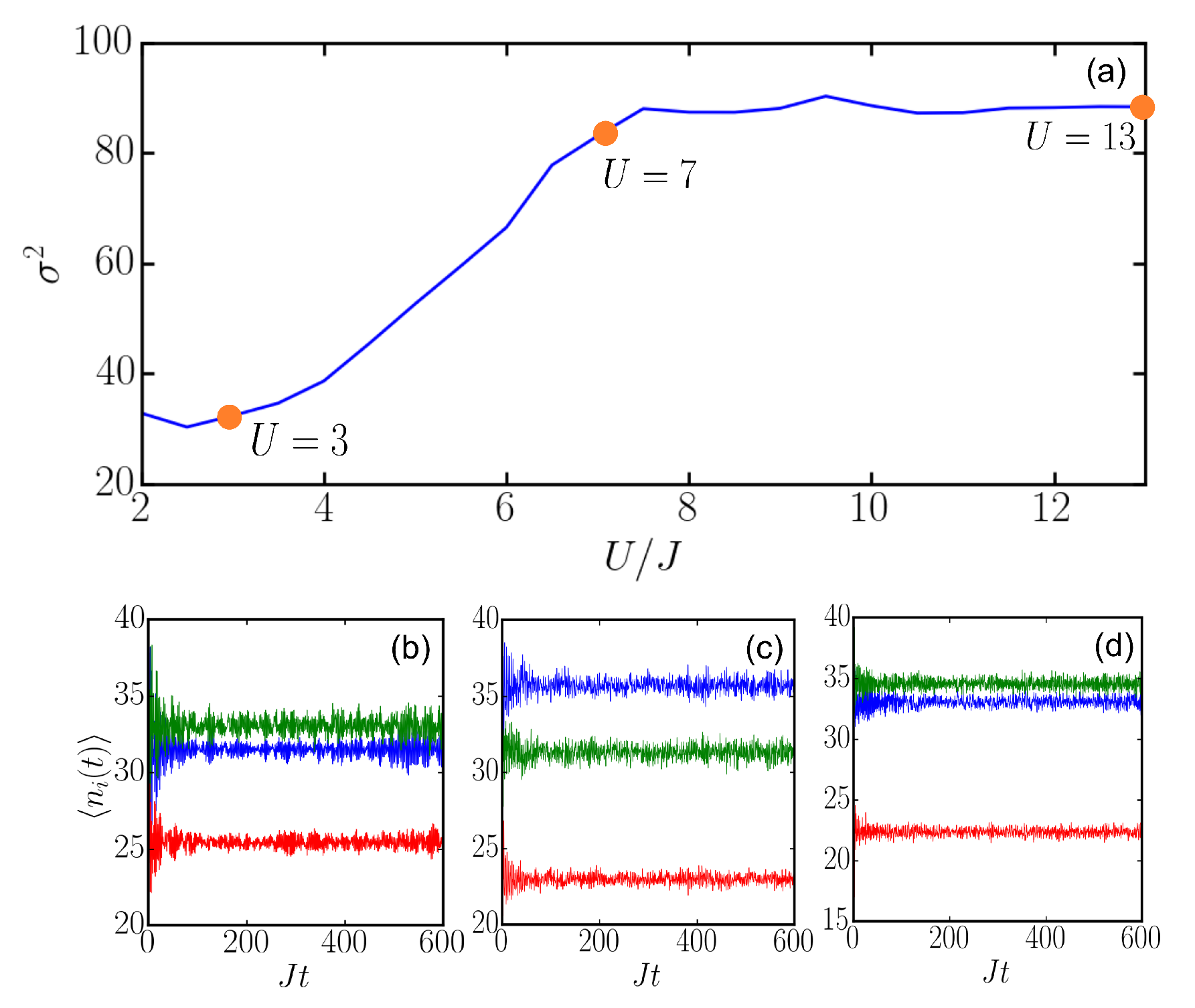
3.3. Survival Probability and Variance of Populations
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Saturation Values of
References
- Bloch, I.; Dalibard, J.; Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 2008, 80, 885. [Google Scholar] [CrossRef]
- Góral, K.; Santos, L.; Lewenstein, M. Quantum Phases of Dipolar Bosons in Optical Lattices. Phys. Rev. Lett. 2002, 88, 170406. [Google Scholar] [CrossRef]
- Trefzger, C.; Menotti, C.; Capogrosso-Sansone, B.; Lewenstein, M. Ultracold dipolar gases in optical lattices. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 193001. [Google Scholar] [CrossRef]
- Rossini, D.; Fazio, R. Phase diagram of the extended Bose–Hubbard model. New J. Phys. 2012, 14, 065012. [Google Scholar] [CrossRef]
- Ejima, S.; Lange, F.; Fehske, H. Spectral and entanglement properties of the bosonic Haldane insulator. Phys. Rev. Lett. 2014, 113, 020401. [Google Scholar] [CrossRef]
- Xiong, B.; Fischer, U.R. Interaction-induced coherence among polar bosons stored in triple-well potentials. Phys. Rev. A 2013, 88, 063608. [Google Scholar] [CrossRef]
- Chomaz, L.; Ferrier-Barbut, I.; Ferlaino, F.; Laburthe-Tolra, B.; Lev, B.L.; Pfau, T. Dipolar Physics: A Review of Experiments with Magnetic Quantum Gases. Rep. Prog. Phys. 2022, 86, 026401. [Google Scholar] [CrossRef]
- Saffman, M.; Walker, T.G.; Mølmer, K. Quantum Information with Rydberg Atoms. Rev. Mod. Phys. 2010, 82, 2313. [Google Scholar] [CrossRef]
- Bouchoule, I.; Molmer, K. Spin squeezing of atoms by the dipole interaction in virtually excited Rydberg states. Phys. Rev. A 2002, 65, 041803. [Google Scholar] [CrossRef]
- Henkel, N.; Nath, R.; Pohl, T. Three-Dimensional Roton Excitations and Supersolid Formation in Rydberg-Excited Bose-Einstein Condensates. Phys. Rev. Lett. 2010, 104, 195302. [Google Scholar] [CrossRef]
- Honer, J.; Weimer, H.; Pfau, T.; Büchler, H.P. Collective many-body interaction in rydberg dressed atoms. Phys. Rev. Lett. 2010, 105, 160404. [Google Scholar] [CrossRef] [PubMed]
- Pupillo, G.; Micheli, A.; Boninsegni, M.; Lesanovsky, I.; Zoller, P. Strongly correlated gases of rydberg-dressed atoms: Quantum and classical dynamics. Phys. Rev. Lett. 2010, 104, 223002. [Google Scholar] [CrossRef] [PubMed]
- Johnson, J.E.; Rolston, S.L. Interactions between Rydberg-dressed atoms. Phys. Rev. A 2010, 82, 033412. [Google Scholar] [CrossRef]
- Li, W.; Hamadeh, L.; Lesanovsky, I. Probing the interaction between Rydberg-dressed atoms through interference. Phys. Rev. A 2012, 85, 053615. [Google Scholar] [CrossRef]
- Xiong, B.; Jen, H.H.; Wang, D.W. Topological superfluid by blockade effects in a Rydberg-dressed Fermi gas. Phys. Rev. A 2014, 90, 013631. [Google Scholar] [CrossRef]
- Hsueh, C.H.; Wang, C.W.; Wu, W.C. Vortex structures in a rotating Rydberg-dressed Bose-Einstein condensate with the Lee-Huang-Yang correction. Phys. Rev. A 2020, 102, 063307. [Google Scholar] [CrossRef]
- Maucher, F.; Henkel, N.; Saffman, M.; Królikowski, W.; Skupin, S.; Pohl, T. Rydberg-induced solitons: Three-dimensional self-trapping of matter waves. Phys. Rev. Lett. 2011, 106, 170401. [Google Scholar] [CrossRef]
- Cinti, F.; MacRì, T.; Lechner, W.; Pupillo, G.; Pohl, T. Defect-induced supersolidity with soft-core bosons. Nat. Commun. 2014, 5, 4235. [Google Scholar] [CrossRef]
- Mukherjee, R.; Ates, C.; Li, W.; Wüster, S. Phase-Imprinting of Bose-Einstein Condensates with Rydberg Impurities. Phys. Rev. Lett. 2015, 115, 040401. [Google Scholar] [CrossRef]
- Hsueh, C.H.; Tsai, Y.C.; Wu, W.C. Excitations of one-dimensional supersolids with optical lattices. Phys. Rev. A 2016, 93, 063605. [Google Scholar] [CrossRef]
- McCormack, G.; Nath, R.; Li, W. Dynamical excitation of maxon and roton modes in a Rydberg-Dressed Bose-Einstein Condensate. Phys. Rev. A 2020, 102, 023319. [Google Scholar] [CrossRef]
- Li, Y.; Cai, H.; Wang, D.w.; Li, L.; Yuan, J.; Li, W. Many-Body Chiral Edge Currents and Sliding Phases of Atomic Spin Waves in Momentum-Space Lattice. Phys. Rev. Lett. 2020, 124, 140401. [Google Scholar] [CrossRef]
- Zhou, Y.; Nath, R.; Wu, H.; Lesanovsky, I.; Li, W. Multipolar Fermi-surface Deformation in a Rydberg-dressed Fermi Gas with Long-Range Anisotropic Interactions. Phys. Rev. A 2021, 104, L061302. [Google Scholar] [CrossRef]
- Lauer, A.; Muth, D.; Fleischhauer, M. Transport-induced melting of crystals of Rydberg dressed atoms in a one-dimensional lattice. New J. Phys. 2012, 14, 095009. [Google Scholar] [CrossRef]
- Lan, Z.; Minar, J.; Levi, E.; Li, W.; Lesanovsky, I. Emergent Devil’s Staircase without Particle-Hole Symmetry in Rydberg Quantum Gases with Competing Attractive and Repulsive Interactions. Phys. Rev. Lett. 2015, 115, 203001. [Google Scholar] [CrossRef] [PubMed]
- Angelone, A.; Mezzacapo, F.; Pupillo, G. Superglass Phase of Interaction-Blockaded Gases on a Triangular Lattice. Phys. Rev. Lett. 2016, 116, 135303. [Google Scholar] [CrossRef]
- Chougale, Y.; Nath, R. Ab initio calculation of Hubbard parameters for Rydberg-dressed atoms in a one-dimensional optical lattice. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 144005. [Google Scholar] [CrossRef]
- Li, Y.; Geißler, A.; Hofstetter, W.; Li, W. Supersolidity of lattice bosons immersed in strongly correlated Rydberg dressed atoms. Phys. Rev. A 2018, 97, 023619. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, Y.; Nath, R.; Li, W. Quench dynamics of Rydberg-dressed bosons on two-dimensional square lattices. Phys. Rev. A 2020, 101, 013427. [Google Scholar] [CrossRef]
- Barbier, M.; Geißler, A.; Hofstetter, W. Decay-dephasing-induced steady states in bosonic Rydberg-excited quantum gases in an optical lattice. Phys. Rev. A 2019, 99, 033602. [Google Scholar] [CrossRef]
- McCormack, G.; Nath, R.; Li, W. Nonlinear dynamics of Rydberg-dressed Bose-Einstein condensates in a triple-well potential. Phys. Rev. A 2020, 102, 063329. [Google Scholar] [CrossRef]
- Jau, Y.Y.; Hankin, A.M.; Keating, T.; Deutsch, I.H.; Biedermann, G.W. Entangling atomic spins with a Rydberg-dressed spin-flip blockade. Nat. Phys. 2016, 12, 3487. [Google Scholar] [CrossRef]
- Zeiher, J.; Van Bijnen, R.; Schauß, P.; Hild, S.; Choi, J.Y.; Pohl, T.; Bloch, I.; Gross, C. Many-body interferometry of a Rydberg-dressed spin lattice. Nat. Phys. 2016, 12, 3835. [Google Scholar] [CrossRef]
- Zeiher, J.; Choi, J.Y.; Rubio-Abadal, A.; Pohl, T.; Van Bijnen, R.; Bloch, I.; Gross, C. Coherent many-body spin dynamics in a long-range interacting Ising chain. Phys. Rev. X 2017, 7, 041063. [Google Scholar] [CrossRef]
- Guardado-Sanchez, E.; Spar, B.M.; Schauss, P.; Belyansky, R.; Young, J.T.; Bienias, P.; Gorshkov, A.V.; Iadecola, T.; Bakr, W.S. Quench Dynamics of a Fermi Gas with Strong Nonlocal Interactions. Phys. Rev. X 2021, 11, 021036. [Google Scholar] [CrossRef]
- Borish, V.; Marković, O.; Hines, J.A.; Rajagopal, S.V.; Schleier-Smith, M. Transverse-Field Ising Dynamics in a Rydberg-Dressed Atomic Gas. Phys. Rev. Lett. 2020, 124, 063601. [Google Scholar] [CrossRef]
- McCormack, G.; Nath, R.; Li, W. Hyperchaos in a Bose-Hubbard chain with Rydberg-Dressed interactions. Photonics 2021, 8, 554. [Google Scholar] [CrossRef]
- de la Cruz, J.; Lerma-Hernández, S.; Hirsch, J.G. Quantum chaos in a system with high degree of symmetries. Phys. Rev. E 2020, 102, 032208. [Google Scholar] [CrossRef]
- Choy, T.; Haldane, F. Failure of Bethe-Ansatz solutions of generalisations of the Hubbard chain to arbitrary permutation symmetry. Phys. Lett. A 1982, 90, 83–84. [Google Scholar] [CrossRef]
- Kolovsky, A.R.; Buchleitner, A. Quantum chaos in the Bose-Hubbard model. Europhys. Lett. (EPL) 2004, 68, 632–638. [Google Scholar] [CrossRef]
- Oelkers, N.; Links, J. Ground-state properties of the attractive one-dimensional Bose-Hubbard model. Phys. Rev. B 2007, 75, 115119. [Google Scholar] [CrossRef]
- Nakerst, G.; Haque, M. Eigenstate thermalization scaling in approaching the classical limit. Phys. Rev. E 2021, 103, 042109. [Google Scholar] [CrossRef] [PubMed]
- Wittmann W., K.; Castro, E.R.; Foerster, A.; Santos, L.F. Interacting bosons in a triple well: Preface of many-body quantum chaos. Phys. Rev. E 2022, 105, 034204. [Google Scholar] [CrossRef]
- Kollath, C.; Roux, G.; Biroli, G.; Läuchli, A.M. Statistical properties of the spectrum of the extended Bose–Hubbard model. J. Stat. Mech. Theory Exp. 2010, 2010, P08011. [Google Scholar] [CrossRef]
- Pethick, C.J.; Smith, H. Microscopic theory of the Bose gas. In Bose–Einstein Condensation in Dilute Gases; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar] [CrossRef]
- Haake, F. Quantum signatures of chaos. In Quantum Coherence in Mesoscopic Systems; Springer: Cham, Switzerland, 1991; pp. 583–595. [Google Scholar]
- Berry, M.V.; Tabor, M. Level clustering in the regular spectrum. Proc. R. Soc. Lond. A Math. Phys. Sci. 1977, 356, 375–394. [Google Scholar]
- Chirikov, B.V.; Shepelyansky, D.L. Shnirelman Peak in Level Spacing Statistics. Phys. Rev. Lett. 1995, 74, 518–521. [Google Scholar] [CrossRef] [PubMed]
- Pandey, A.; Ramaswamy, R. Level spacings for harmonic-oscillator systems. Phys. Rev. A 1991, 43, 4237–4243. [Google Scholar] [CrossRef]
- Guhr, T.; Müller-Groeling, A.; Weidenmüller, H.A. Random-matrix theories in quantum physics: Common concepts. Phys. Rep. 1998, 299, 189–425. [Google Scholar] [CrossRef]
- Castro, E.R.; Chávez-Carlos, J.; Roditi, I.; Santos, L.F.; Hirsch, J.G. Quantum-Classical Correspondence of a System of Interacting Bosons in a Triple-Well Potential. Quantum 2021, 5, 563. [Google Scholar] [CrossRef]
- Bohigas, O.; Giannoni, M.J.; Schmit, C. Characterization of Chaotic Quantum Spectra and Universality of Level Fluctuation Laws. Phys. Rev. Lett. 1984, 52, 1–4. [Google Scholar] [CrossRef]
- Wigner, E.P. Characteristic Vectors of Bordered Matrices With Infinite Dimensions. Ann. Math. 1955, 62, 548–564. [Google Scholar] [CrossRef]
- Brody, T.A.; Flores, J.; French, J.B.; Mello, P.A.; Pandey, A.; Wong, S.S.M. Random-matrix physics: Spectrum and strength fluctuations. Rev. Mod. Phys. 1981, 53, 385–479. [Google Scholar] [CrossRef]
- von Neumann, J. Mathematische Grundlagen der Quantenmechanik; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar] [CrossRef]
- Schiulaz, M.; Torres-Herrera, E.J.; Santos, L.F. Thouless and relaxation time scales in many-body quantum systems. Phys. Rev. B 2019, 99, 174313. [Google Scholar] [CrossRef]
- Faiez, D.; Šafránek, D. How much entanglement can be created in a closed system. Phys. Rev. B 2020, 101, 060401. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, T.; Collins, M.; Nath, R.; Li, W. Signatures of Quantum Chaos of Rydberg-Dressed Bosons in a Triple-Well Potential. Atoms 2023, 11, 89. https://doi.org/10.3390/atoms11060089
Yan T, Collins M, Nath R, Li W. Signatures of Quantum Chaos of Rydberg-Dressed Bosons in a Triple-Well Potential. Atoms. 2023; 11(6):89. https://doi.org/10.3390/atoms11060089
Chicago/Turabian StyleYan, Tianyi, Matthew Collins, Rejish Nath, and Weibin Li. 2023. "Signatures of Quantum Chaos of Rydberg-Dressed Bosons in a Triple-Well Potential" Atoms 11, no. 6: 89. https://doi.org/10.3390/atoms11060089





