Constitutive Modeling of Mechanical Behaviors of Carbon-Based CNTs and GSs, and Their Sensing Applications as Nanomechanical Resonators: A Review
Abstract
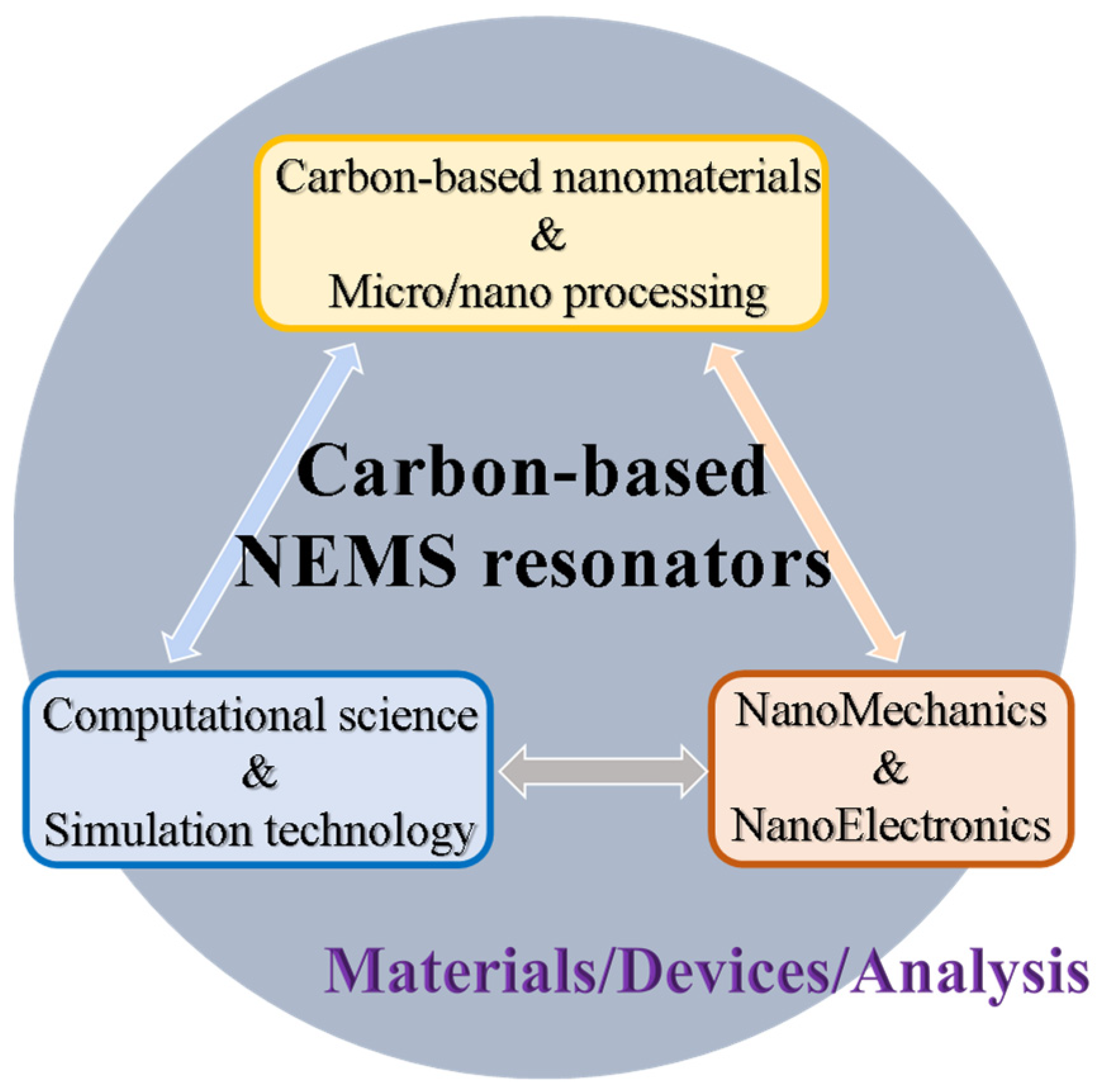
:1. Introduction
2. Mechanical Properties, Vibration, and Buckling of CNTs and GSs
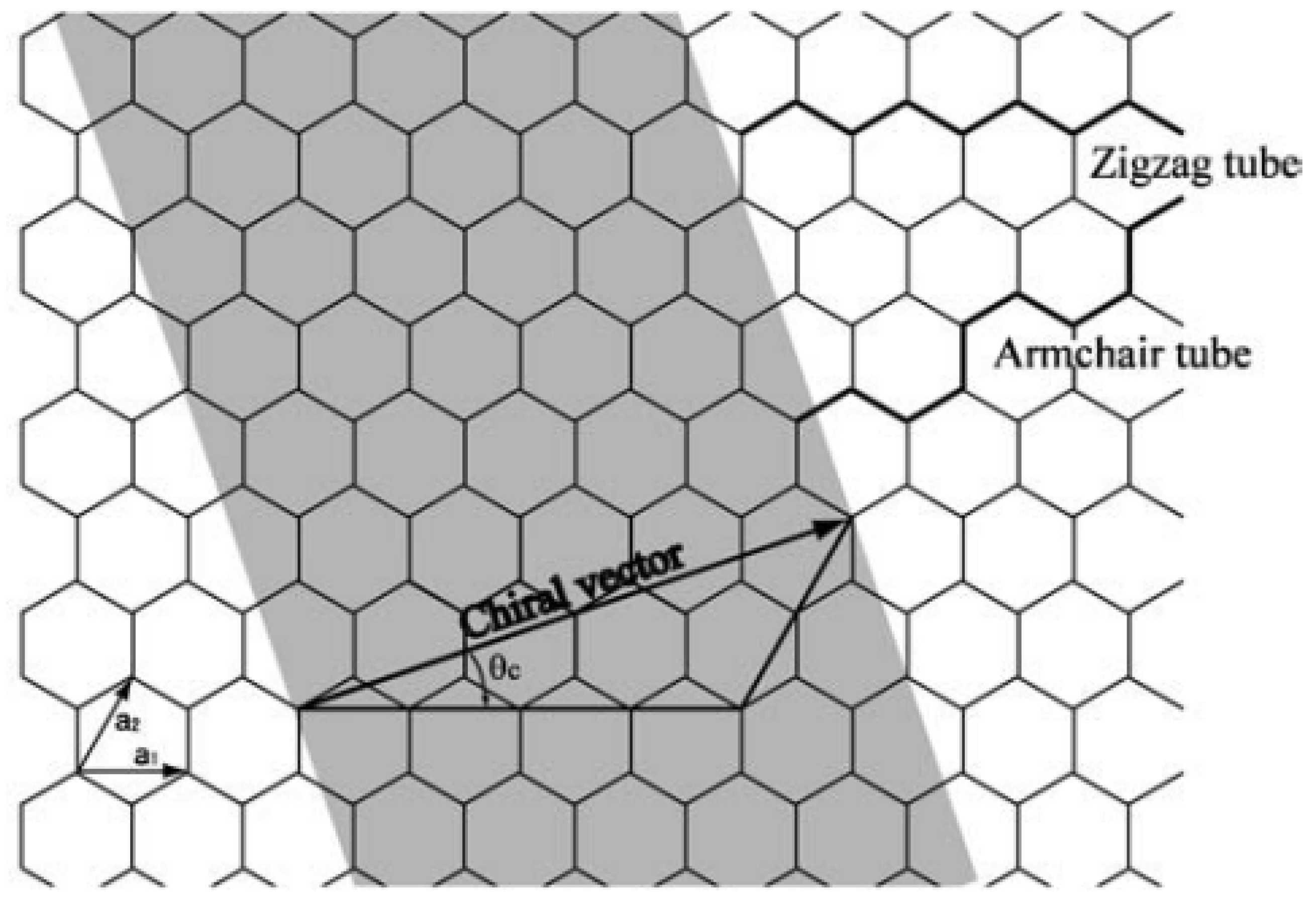
2.1. Structure, Modeling, and Properties of CNTs
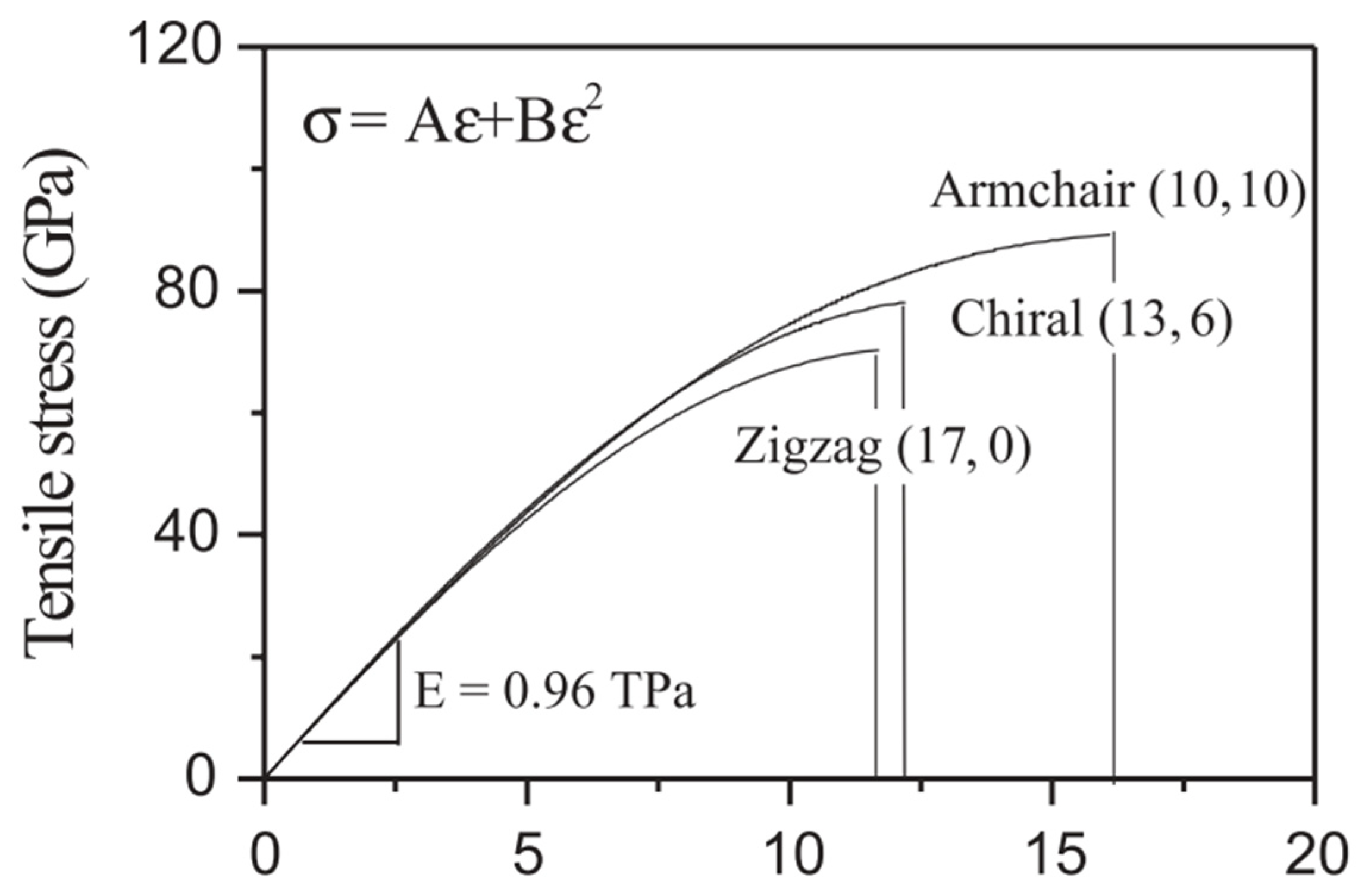
2.1.1. Measurements of Tensile Properties and Analytical Modeling
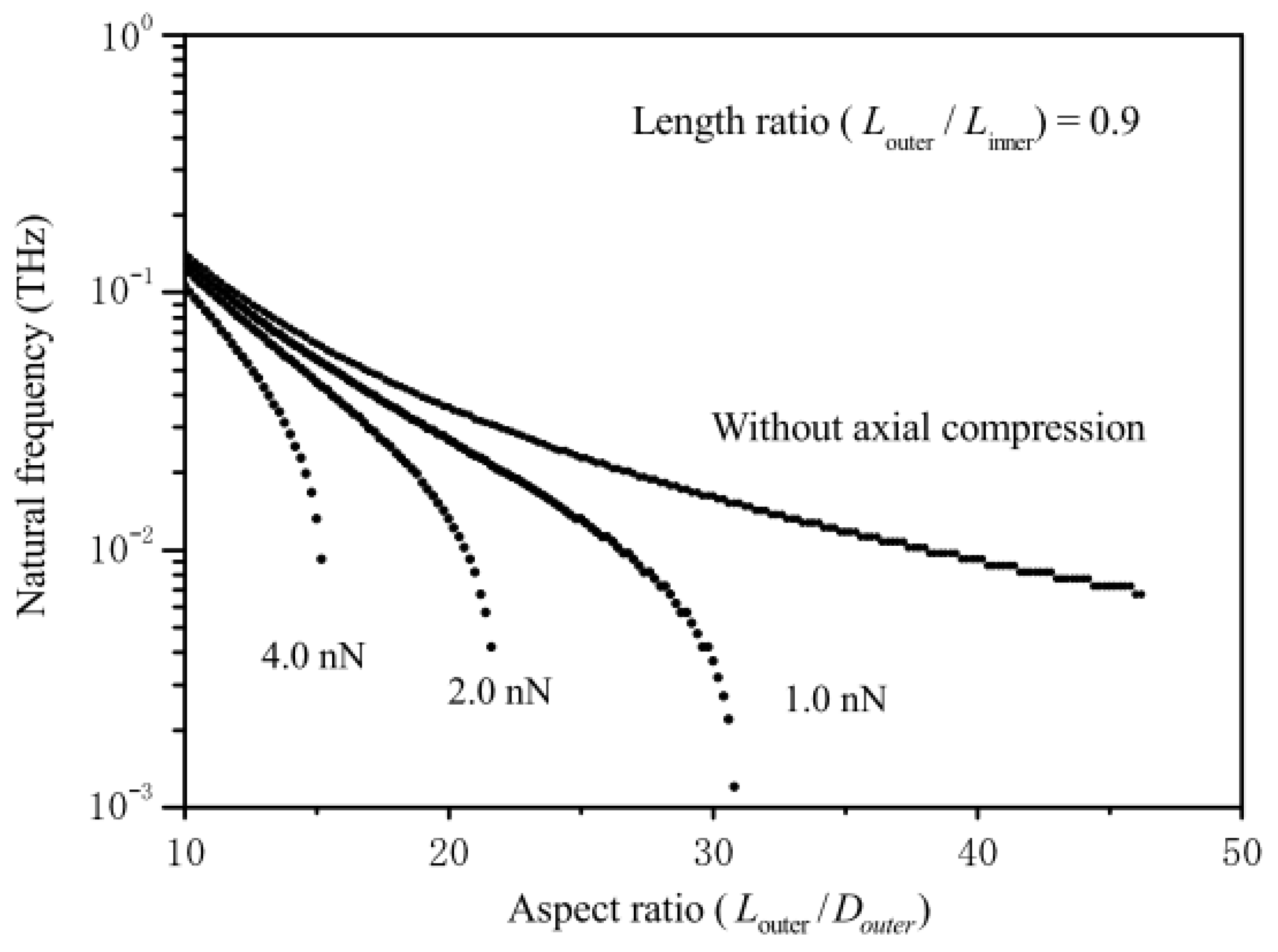
2.1.2. Vibrations, Buckling, and Modeling
- For the Bernoulli–Euler beam theory,
- For the Timoshenko theory beam theory,where denotes the transverse deflection of the CNT beam, represents the slope, and P denotes the internal axial compressive loading.
2.2. Mechanical Properties, Vibration, and Buckling Analysis of GSs
2.2.1. The Tensile and Bending Mechanical Properties
2.2.2. Analysis Modeling, Buckling Instability, and Vibration Properties
3. Analytical Modeling and Application of CNTs and GSs as Nanosensor Materials
3.1. CNTs and GSs Used for Nanomass Sensing Applications
3.2. Modeling and Analysis of CNT- and GS-Based Nanomass Sensor
3.2.1. Modeling and Differential Equation for CNT Beams
3.2.2. Modeling and Differential Equation for GS Plate
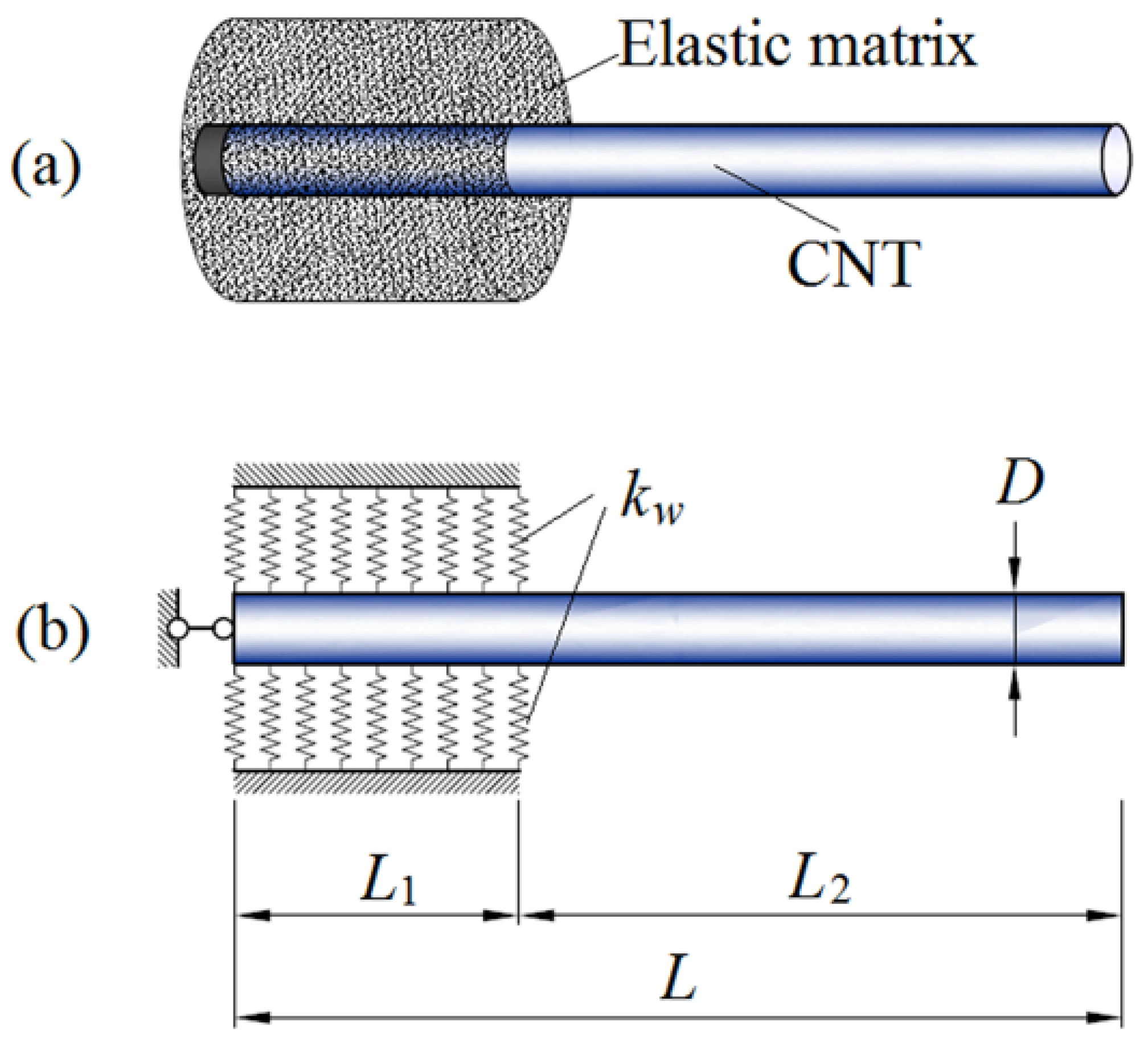
3.3. Modeling and Analysis of Nanoforce Sensor Based on CNTs
4. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ruoff, R.S.; Qian, D.; Liu, W.K. Mechanical properties of carbon nanotubes: Theoretical predictions and experimental measurements. Comptes Rendus Phys. 2003, 4, 993–1008. [Google Scholar] [CrossRef]
- Maiti, D.; Tong, X.M.; Mou, X.Z.; Yang, K. Carbon-based nanomaterials for biomedical applications: A recent study. Front. Pharmacol. 2019, 9, 1401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tomioka, Y.; Natsuki, T.; Shi, J.X.; Lei, X.W. Theoretical evaluation of impact characteristics of wavy graphene sheets with disclinations formed by origami and Kirigami. Nanomaterials 2022, 12, 436. [Google Scholar] [CrossRef]
- Qin, Y.; Zhang, Y.; Qi, N.; Wang, Q.Z.; Zhang, X.J.; Li, Y. Preparation of graphene aerogel with high mechanical stability and microwave absorption ability via combining surface support of metallic-CNTs and interfacial cross-linking by magnetic nanoparticles. ACS Appl. Mater. Interfaces 2019, 11, 10409–10417. [Google Scholar] [CrossRef]
- Hung, N.T.; Van Truong, D.; Van Thanh, V.; Saito, R. Intrinsic strength and failure behaviors of ultra-small single-walled carbon nanotubes. Comput. Mater. Sci. 2016, 114, 167–171. [Google Scholar] [CrossRef] [Green Version]
- Shokrieh, M.M.; Rafiee, R. Prediction of Young’s modulus of graphene sheets and carbon nanotubes using nanoscale continuum mechanics approach. Mater. Des. 2010, 31, 790–795. [Google Scholar] [CrossRef]
- Castillo-Henríquez, L.; Brenes-Acuña, M.; Castro-Rojas, A.; Cordero-Salmerón, R.; Lopretti-Correa, M.; Vega-Baudrit, J.R. Biosensors for the detection of bacterial and viral clinical pathogens. Sensors 2020, 20, 6926. [Google Scholar] [CrossRef]
- Barsan, M.M.; Ghica, M.E.; Brett, C.M.A. Electrochemical sensors and biosensors based on redox polymer/carbon nanotube modified electrodes: A review. Anal. Cim. Acta 2015, 881, 1–23. [Google Scholar] [CrossRef]
- Yuan, W.J.; Shi, G.Q. Graphene-based gas sensors. J. Mater. Chem. A 2013, 1, 10078–10091. [Google Scholar] [CrossRef]
- Loghin, F.; Abdellah, A.; Falco, A.; Becherer, M.; Lugli, P.; Rivadeneyra, A. Time stability of carbon nanotube gas sensors. Measurement 2019, 136, 323–325. [Google Scholar] [CrossRef]
- Moser, J.; Guttinger, J.; Eichler, A.; Esplandiu, M.J.; Liu, D.E.; Dykman, M.I.; Bachtold, A. Ultrasensitive force detection with a nanotube mechanical resonator. Nat. Nanotechnol. 2013, 8, 493–496. [Google Scholar] [CrossRef] [PubMed]
- Singh, K.; Sharma, S.; Singh, B.; Gupta, M.; Tripathi, C.C. Fabrication of graphene, graphite and multi wall carbon nano tube based thin films and their potential application as strain sensor. Thin Solid Film. 2022, 761, 139540. [Google Scholar] [CrossRef]
- Mirfakhrai, T.; Oh, J.; Kozlov, M.E.; Fang, S.L.; Zhang, M.; Baughman, R.H.; Madden, J.D.W. Mechanoelectrical force sensors using twisted yarns of carbon nanotubes. IEEE ASME Trans. Mechatron. 2011, 16, 90–97. [Google Scholar] [CrossRef]
- Wang, J.; Musameh, M. Carbon nanotube screen-printed electrochemical sensors. Analyst 2004, 129, 1–2. [Google Scholar] [CrossRef] [PubMed]
- Chen, K.; Gao, W.; Emaminejad, S.; Kiriya, D.; Ota, H.; Nyein, H.Y.Y.; Takei, K.; Javey, A. Printed carbon nanotube electronics and sensor systems. Adv. Mater. 2016, 28, 4397–4414. [Google Scholar] [CrossRef]
- Kauth, C.; Pastre, M.; Kayal, M. A novel approach to high-speed high-resolution on-chip mass sensing. Microelectron. J. 2014, 45, 1648–1655. [Google Scholar] [CrossRef]
- Mirakhory, M.; Khatibi, M.M.; Sadeghzadeh, S. Nanoparticle mass detection by single-layer triangular graphene sheets, the extraordinary geometry for detection of nanoparticle. J. Nanopart. Res. 2020, 22, 159. [Google Scholar] [CrossRef]
- Crica, L.E.; Dennison, T.J.; Guerini, E.A.; Kostarelos, K. A method for the measurement of mass and number of graphene oxide sheets in suspension based on non-spherical approximations. 2D Mater. 2021, 8, 035044. [Google Scholar] [CrossRef]
- Iijima, S. Helical microtubles of graphitic carbon. Nature 1991, 354, 56–58. [Google Scholar] [CrossRef]
- Qian, D.; Wagner, G.J.; Liu, W.K.; Yu, M.F.; Ruoff, R.S. Mechanics of carbon nanotubes. Appl. Mech. Rev. 2002, 55, 495–533. [Google Scholar] [CrossRef]
- Lau, K.T.; Hui, D. The revolutionary creation of new advanced materials: Carbon nanotube composites. Compos. Part B 2002, 33, 263–277. [Google Scholar] [CrossRef]
- Li, Y.; Wang, S.; He, E.; Wang, Q. The effect of sliding velocity on the tribological properties of polymer/carbon nanotube composites. Carbon 2016, 106, 106–109. [Google Scholar] [CrossRef]
- Sun, R.; Li, L.; Feng, C.; Kitipornchai, S.; Yang, J. Tensile behavior of polymer nanocomposite reinforced with graphene containing defects. Eur. Polym. J. 2018, 98, 475–482. [Google Scholar] [CrossRef] [Green Version]
- Natsuki, T.; Endo, M. Stress simulation of carbon nanotubes in tension and compression. Carbon 2004, 42, 2137–2151. [Google Scholar] [CrossRef]
- Qian, D.; Dickey, E.C.; Andrews, R.; Rantell, T. Load transfer and deformation mechanisms in carbon nanotube-polystyrene composites. Appl. Phys. Lett. 2000, 76, 2868–2870. [Google Scholar] [CrossRef] [Green Version]
- Schadler, L.S.; Giannaris, S.C.; Ajayan, P.M. Load transfer in carbon nanotube epoxy composites. Appl. Phys. Lett. 1998, 73, 3842–3844. [Google Scholar] [CrossRef]
- Wanger, H.D.; Lourie, O.; Feldman, Y.; Tenne, R. Stress-induced fragmentation of multiwall carbon nanotubes in a polymer matrix. Appl. Phys. Lett. 1998, 72, 188–190. [Google Scholar]
- Treacy, M.M.J.; Ebbesen, T.W.; Gibson, J.M. Exceptionally high young’s modulus observed for individual nanotubes. Nature 1996, 381, 678–680. [Google Scholar] [CrossRef]
- Wong, E.W.; Sheehan, P.E.; Lieber, C.M. Nanobeam mechanics: Elasticity, strength, and toughness of nanorods and nanotubes. Science 1997, 277, 1971–1975. [Google Scholar] [CrossRef]
- Krishnan, A.; Dujardin, E.; Ebbesen, T.W.; Yianilos, P.N.; Treacy, M.M.J. Young’s modulus of single-walled nanotubes. Phys. Rev. B 1998, 58, 14013–14019. [Google Scholar] [CrossRef] [Green Version]
- Natsuki, T.; Natsuki, J. Measurement of the elastic modulus of nanowires based on resonant frequency and boundary condition effects. Phys. E Low Dimens. Syst. Nanostruct. 2019, 105, 207–211. [Google Scholar] [CrossRef]
- Zhang, Y.Q.; Liu, G.R.; Xie, X.Y. Free transverse vibration of double-walled carbon nanotubes using a theory of nonlocal elasticity. Phys. Rev. B 2005, 71, 195404. [Google Scholar] [CrossRef]
- Yu, M.F.; Lourie, O.; Dyer, M.J.; Moloni, K.; Kelly, T.F.; Ruoff, R.S. Strength and breaking mechanism of multi-walled carbon nanotubes under tensile load. Science 2000, 287, 637–640. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jiang, H.; Jiang, L.; Posner, J.D.; Vogt, B.D. Atomistic-based continuum constitutive relation for microtubules: Elastic modulus prediction. Comput. Mech. 2008, 42, 607–618. [Google Scholar] [CrossRef]
- Natsuki, T.; Tantrakarn, K.; Endo, M. Prediction of elastic properties for single-walled carbon nanotubes. Carbon 2004, 42, 39–45. [Google Scholar] [CrossRef]
- Lei, X.; Natsuki, T.; Shi, J.; Ni, Q.Q. Analysis of carbon nanotubes on the mechanical properties at atomic scale. J. Nanomater. 2011, 2011, 805313. [Google Scholar] [CrossRef] [Green Version]
- Natsuki, T.; Endo, M. Structural dependence of nonlinear elastic properties for carbon nanotube using a continuum analysis. Appl. Phys. A 2005, 80, 1463–1468. [Google Scholar] [CrossRef]
- Jacob, M.; Werni Shaker, A. Meguid, Atomistic-based continuum modeling of the nonlinear behavior of carbon nanotubes. Acta Mech. 2010, 212, 167–179. [Google Scholar]
- Al-Kharusi, M.S.M.; Alzebdeh, K.; Pervez, T. An atomistic-based continuum modeling for evaluation of effective elastic properties of single-walled carbon nanotubes. J. Nanomater. 2016, 2016, 8641954. [Google Scholar] [CrossRef] [Green Version]
- Barnard, A.W.; Zhang, M.; Wiederhecker, G.S.; Lipson, M.; McEuen, P.L. Real-time vibrations of a carbon nanotube. Nature 2019, 566, 89–93. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Li, T. Ultrafast nano-oscillators based on interlayer-bridged carbon nanoscrolls. Nanoscale Res. Lett. 2011, 6, 470. [Google Scholar] [CrossRef] [Green Version]
- Willick, K.; Tang, X.; Baugh, J. Probing the non-linear transient response of a carbon nanotube mechanical oscillator. Appl. Phys. Lett. 2017, 111, 223108. [Google Scholar] [CrossRef] [Green Version]
- Sazonova, V.; Yaish, Y.; Ustenel, H.; Roundy, D.; Arias, T.A.; McEuen, P.A. Tunable carbon nanotube electromechanical oscillator. Nature 2004, 431, 284–287. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hu, W.; Song, M.; Yin, T.; Wei, B.; Deng, Z. Energy dissipation of damping cantilevered single-walled carbon nanotube oscillator. Nonlinear Dyn. 2018, 91, 767–776. [Google Scholar] [CrossRef]
- Elishakoff, I.; Pentaras, D. Natural frequencies of carbon nanotubes based on simplified Bresse-Timoshenko theory. J. Comp. Theor. Nanosci. 2009, 6, 1527–1531. [Google Scholar] [CrossRef]
- Hutchinson, J.R. Shear coefficients for Timoshenko beam theory. J. Appl. Mech. (ASME) 2001, 68, 87–92. [Google Scholar] [CrossRef]
- Yoon, J.; Ru, C.Q.; Mioduchowski, A. Timoshenko-beam effects on transverse wave propagation in carbon nanotubes. Compos. Part B Eng. 2004, 35, 87–93. [Google Scholar] [CrossRef]
- Eringen, A.C. Nonlocal polar elastic continua. Int. J. Eng. Sci. 1972, 10, 1–16. [Google Scholar] [CrossRef]
- Eringen, A.C. Interaction of a dislocation with a crack. J. Appl. Phys. 1983, 54, 6811. [Google Scholar] [CrossRef]
- Noureddine, M.; Mohamed, L.; Al-Douri, Y.; Djillali, B.; Mokhtar, B. Effect of chiral angle and chiral index on the vibration of single-walled carbon nanotubes using nonlocal Euler-Bernoulli beam model. Comput. Condens. Matter 2002, 30, 300655. [Google Scholar] [CrossRef]
- Wang, Q.; Zhou, G.Y.; Lin, K.C. Scale effect on wave propagation of double-walled carbon nanotubes. Int. J. Solids Struct. 2006, 43, 6071–6084. [Google Scholar] [CrossRef] [Green Version]
- Lu, P.; Lee, H.P.; Lu, C.; Zhang, P.Q. Application of nonlocal beam models for carbon nanotubes. Int. J. Solids Struct. 2007, 44, 2589–5300. [Google Scholar] [CrossRef] [Green Version]
- Lei, X.; Natsuki, T.; Shi, J. Ni, Surface effects on the vibrational frequency of double-walled carbon nanotubes using the nonlocal Timoshenko beam model. Compos. Part B Eng. 2012, 43, 64–69. [Google Scholar] [CrossRef]
- Boumia, L.; Zidour, M.; Benzair, A.; Tounsi, A. A Timoshenko beam model for vibration analysis of chiral single-walled carbon nanotubes. Phys. E Low Dimens. Syst. Nanostruct. 2014, 59, 186–191. [Google Scholar] [CrossRef]
- Natsuki, T.; Ni, Q.Q.; Endo, M. Analysis of the vibration characteristics of double-walled carbon nanotubes. Carbon 2008, 46, 1570–1573. [Google Scholar] [CrossRef] [Green Version]
- de Borbon, F.; Ambrosini, D. On the influence of van der Waals coefficient on the transverse vibration of double walled carbon nanotubes. Comput. Mater. Sci. 2012, 65, 504–508. [Google Scholar] [CrossRef]
- Girifalco, L.A.; Lad, R.A. Energy of cohesion, compressibility, and the potential energy functions of the graphite system. J. Chem. Phys. 1956, 25, 693. [Google Scholar] [CrossRef]
- Ru, C.Q. Column buckling of multiwalled carbon nanotubes with interlayer radial displacements. Phys. Rev. B 2000, 62, 16962. [Google Scholar] [CrossRef]
- Saito, R.; Matsuo, R.; Kimura, T.; Dresselhaus, G.; Dresselhaus, M.S. Anomalous potential barrier of double-wall carbon nanotube. Chem. Phys. Lett. 2001, 348, 187–193. [Google Scholar] [CrossRef]
- He, X.Q.; Kitipornchai, S.; Liew, K.M. Buckling analysis of multi-walled carbon nanotubes: A continuum model accounting for van der Waals interaction. J. Mech. Phys. Solids 2005, 53, 303–326. [Google Scholar] [CrossRef]
- Kumar, D.; Heinrich, C.; Waasa, A.M. Buckling analysis of carbon nanotubes modeled using nonlocal continuum theories. J. Appl. Phys. 2008, 103, 073521. [Google Scholar] [CrossRef] [Green Version]
- Wang, Q.; Shindo, Y. Nonlocal continuum models for carbon nanotubes subjected to static loading. J. Mech. Mater. Struct. 2006, 1, 663–680. [Google Scholar] [CrossRef]
- Narendar, S.; Gopalakrishnan, S. Critical buckling temperature of single-walled carbon nanotubes embedded in a one-parameter elastic medium based on nonlocal continuum mechanics. Phys. E Low Dimens. Syst. Nanostruct. 2011, 43, 1185–1191. [Google Scholar] [CrossRef]
- Hosseini-Hashemi, S.h.; Ilkhani, M.R. Nonlocal modeling for dynamic stability of spinning nanotube under axial load. Meccanica 2017, 52, 1107–1121. [Google Scholar] [CrossRef]
- Heireche, H.; Tounsi, A.; Benzair, A.; Mechab, I. Sound wave propagation in single-walled carbon nanotubes with initial axial stress. J. Appl. Phys. 2008, 104, 014301. [Google Scholar] [CrossRef]
- Murmu, T.; Pradhan, S.C. Buckling analysis of a single-walled carbon nanotube embedded in an elastic medium based on nonlocal elasticity and Timoshenko beam theory and using DQM. Phys. E Low Dimens. Syst. Nanostruct. 2009, 41, 1232–1239. [Google Scholar] [CrossRef]
- Natsuki, T.; Ni, Q.Q.; Elishakoff, I. Influence of the axial compression on the natural frequency of AFM probes using double-walled carbon nanotubes with different wall lengths. Appl. Phys. A 2013, 110, 1–7. [Google Scholar] [CrossRef]
- Elishakoff, I.; Bucas, S. Buckling of a clamped-free double-walled carbon nanotube by the Bubnov-Galerkin method. J. Appl. Mech. 2013, 80, 011004. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [Green Version]
- Wan, S.J.; Li, Y.C.; Mu, J.K.; Aliev, A.E.; Fang, S.L.; Kotov, N.A.; Jiang, L.; Cheng, Q.F.; Baughman, R.H. Sequentially bridged graphene sheets with high strength, toughness, and electrical conductivity. Proc. Natl. Acad. Sci. USA 2018, 115, 5359–5364. [Google Scholar] [CrossRef] [Green Version]
- Cai, C.L.; Wang, T.; Qu, G.W.; Feng, Z.Q. High thermal conductivity of graphene and structure defects: Prospects for thermal applications in graphene sheets. Chin. Chem. Lett. 2021, 32, 1293–1298. [Google Scholar] [CrossRef]
- Sierra-Chi, C.A.; Aguilar-Bolados, H.; Lopez-Manchado, M.A.; Verdejo, R.; Cauich-Rodriguez, J.V.; Aviles, F. Flexural electromechanical properties of multilayer graphene sheet /carbon nanotube/vinyl ester hybrid nanocomposites. Compos. Sci. Technol. 2020, 194, 108164. [Google Scholar] [CrossRef]
- Tang, L.H.; Wang, Y.; Li, Y.M.; Feng, H.B.; Lu, J.; Li, J.H. Preparation, structure, and electrochemical properties of reduced graphene sheet films. Adv. Funct. Mater. 2009, 19, 2782–2789. [Google Scholar] [CrossRef]
- Garcia-Sanchez, D.; van der Zande, A.M.; San Paulo, A.; Lassagne, B.; McEuen, P.L.; Bachtold, A. Imaging mechanical vibrations in suspended graphene sheets. Nano Lett. 2008, 8, 1399–1403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Frank, I.W.; Tanenbaum, D.M.; van der Zande, A.M.; McEuen, P.L. Mechanical properties of suspended graphene sheets. J. Vac. Sci. Technol. B 2007, 25, 2558–2561. [Google Scholar] [CrossRef] [Green Version]
- Lee, C.; Wei, X.D.; Kysar, G.W.; Hone, J. Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef]
- Gao, Y.W.; Hao, P. Mechanical properties of monolayer graphene under tensile and compressive loading. Phys. E Low Dimens. Syst. Nanostruct. 2009, 41, 1561–1566. [Google Scholar] [CrossRef]
- Palaiologos, A.; Grigoriadis., K.; Zavos, A.; Nikolakopoulo, P.G. A dynamic and tribological simulation of a monolayer graphene sheet for a carbon atom-graphene contact. Lubr. Sci. 2019, 31, 194–209. [Google Scholar] [CrossRef]
- Shi, J.X.; Natsuki, T.; Lei, X.W.; Ni, Q.Q. Equivalent Young’s modulus and thickness of graphene sheets for the continuum mechanical models. Appl. Phys. Lett. 2014, 104, 223101. [Google Scholar] [CrossRef]
- Natsuki, T.; Natsuki, J. Prediction of mechanical properties for hexagonal boron nitride nanosheets using molecular mechanics model. Appl. Phys. A 2017, 123, 283. [Google Scholar] [CrossRef]
- Madani, S.H.; Sabour, M.H.; Fadaee, M. Molecular dynamics simulation of vibrational behavior of annular graphene sheet: Identification of nonlocal parameter. J. Mol. Graph. Model. 2018, 79, 264–272. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J. Mechanical deformations of carbon nanorings: A study by molecular dynamics and nonlocal continuum mechanics. Meccanica 2019, 54, 2281–2293. [Google Scholar] [CrossRef]
- Sahmani, S.; Fattah, A.M. Development an efficient calibrated nonlocal plate model for nonlinear axial instability of zirconia nanosheets using molecular dynamics simulation. J. Mol. Graph. Model. 2017, 75, 20–31. [Google Scholar] [CrossRef]
- Pour, M.A.; Golmakani1, M.E.; Malikan, M. Thermal buckling analysis of circular bilayer graphene sheets resting on an elastic matrix based on nonlocal continuum mechanics. J. Appl. Comput. Mech. 2021, 7, 1862–1877. [Google Scholar]
- Ghannadpour, S.A.M.; Moradi, F.; Tornabene, F. Exact analytical solution to the problem of relative post-buckling stiffness of thin nonlocal graphene sheet. Thin Wall. Struct. 2020, 151, 106712. [Google Scholar] [CrossRef]
- Wu, C.P.; Li, W.C. Asymptotic nonlocal elasticity theory for the buckling analysis of embedded single-layered nanoplates/graphene sheets under biaxial compression. Phys. E Low Dimens. Syst. Nanostruct. 2017, 89, 160–169. [Google Scholar] [CrossRef]
- Shahsavari, D.; Behrouz, K.; Li, L. Damped vibration of a graphene sheet using a higher-order nonlocal strain-gradient Kirchhoff plate model. Comptes Rendus Mécanique 2018, 346, 1216–1232. [Google Scholar] [CrossRef]
- Shen, L.; Shen, H.S.; Zhang, C.L. Nonlocal plate model for nonlinear vibration of single layer graphene sheets. Comput. Mater. Sci. 2010, 48, 680–685. [Google Scholar] [CrossRef]
- Ansari, R.; Sahmani, S.; Arash, B. Nonlocal plate model for free vibrations of single-layered graphene sheets. Phys. Lett. A 2010, 375, 53–62. [Google Scholar] [CrossRef]
- Pradhan, S.C.; Phadika, J.K. Small scale effect on vibration of embedded multilayered graphene sheets based on nonlocal continuum model. Phys. Lett. A 2009, 373, 1062–1069. [Google Scholar] [CrossRef]
- Wang, K.F.; Wang, B.L.; ·Zeng, S. Small scale effect on the pull-in instability and vibration of graphene sheets. Microsyst. Technol. 2017, 23, 2033–2041. [Google Scholar] [CrossRef]
- Pradhan, S.C.; Murmu, T. Small scale effect on the buckling analysis of single-layered graphene sheet embedded in an elastic medium based on nonlocal plate theory. Phys. E Low Dimens. Syst. Nanostruct. 2010, 42, 1293–1301. [Google Scholar] [CrossRef]
- Murmu, T.; McCarthy, M.A.; Adhikari, S. In-plane magnetic field affected transverse vibration of embedded single-layer graphene sheets using equivalent nonlocal elasticity approach. Compos. Struct. 2013, 96, 57–63. [Google Scholar] [CrossRef]
- Narendar, S.; Gopalakrishnan, S. Strong nonlocalization induced by small scale parameter on terahertzflexural wave dispersion characteristics of a monolayer graphene. Phys. E Low Dimens. Syst. Nanostruct. 2010, 43, 423–430. [Google Scholar] [CrossRef]
- Golmakani, M.E.; Sadraee Far, M.N. Buckling analysis of biaxially compressed double-layered graphene sheets with various boundary conditions based on nonlocal elasticity theory. Microsyst. Technol. 2017, 23, 2145–2161. [Google Scholar] [CrossRef]
- He, X.Q.; Wang, J.B.; Liu, B.; Liew, K.M. Analysis of nonlinear forced vibration of multi-layered graphene sheets. Comput. Mater. Sci. 2012, 61, 194–199. [Google Scholar] [CrossRef]
- Kitipornchai, S.; He, X.Q.; Liew, K.M. Continuum model for the vibration of multilayered graphene sheets. Phys. Rev. B 2005, 72, 075443. [Google Scholar] [CrossRef] [Green Version]
- Jensen, K.; Kim, K.; Zettl, A. An atomic-resolution nanomechanical mass sensor. Nat. Nanotechnol. 2008, 3, 533–537. [Google Scholar] [CrossRef] [PubMed]
- Aydogdu, M.; Filiz, S. Modeling carbon nanotube-based mass sensors using axial vibration and nonlocal elasticity. Phys. E Low Dimens. Syst. Nanostruct. 2011, 43, 1229–1234. [Google Scholar] [CrossRef]
- Lassagne, B.; Bachtold, A. Carbon nanotube electromechanical resonator for ultrasensitive mass/force sensing. Comptes Rendus Phys. 2010, 11, 355–361. [Google Scholar] [CrossRef]
- Natsuki, T. Carbon nanotube-based nanomechanical sensor: Theoretical analysis of mechanical and vibrational properties. Electronics 2017, 6, 56. [Google Scholar] [CrossRef] [Green Version]
- Shi, J.X.; Lei, X.W.; Natsuki, T. Review on carbon nanomaterials-based nano-mass and nano-force sensors by theoretical analysis of vibration behavior. Sensors 2021, 21, 1907. [Google Scholar] [CrossRef]
- Lassagne, B.; Garcia-Sanchez, D.; Aguasca, A.; Bachtold, A. Ultrasensitive mass sensing with a nanotube electromechanical resonator. Nano Lett. 2008, 8, 3735–3738. [Google Scholar] [CrossRef] [PubMed]
- Li, J.J.; Zhu, K.D. Weighing a single atom using a coupled plasmon-carbon nanotube system. Sci. Technol. Adv. Mater. 2012, 13, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Bunch, J.S.; van der Zande, A.M.; Verbridge, S.S.; Frank, I.W.; Tanenbaum, D.M.; Parpia, J.M.; Craighead, H.G.; McEuen, P.L. Electromechanical resonators from graphene sheets. Science 2007, 315, 490–493. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sharma, A.; Varshney, U.; Lu, Y. Electronic applications of graphene mechanical resonators. IET Circuits Devices Syst. 2015, 9, 413–419. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.W. Mass detection using carbon nanotube-based nanomechanical resonators. Appl. Phys. Lett. 2004, 84, 5246–5248. [Google Scholar] [CrossRef] [Green Version]
- Lee, H.L.; Hsu, J.H.; Chang, W.J. Frequency shift of carbon-nanotube-based mass sensor using nonlocal elasticity theory. Nanoscale Res. Lett. 2010, 5, 1774–1778. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shen, Z.B.; Deng, B.; Li, X.F.; Tang, G.J. Vibration of double-walled carbon nanotube-based mass sensor via nonlocal Timoshenko beam theory. J. Nanotechnol. Eng. 2012, 2, 031003. [Google Scholar] [CrossRef]
- Shen, Z.B.; Sheng, L.P.; Li, X.F.; Tang, G.J. Nonlocal timoshenko beam theory for vibration of carbon nanotube-based biosensor. Phys. E Low Dimens. Syst. Nanostruct. 2012, 44, 1169–1175. [Google Scholar] [CrossRef]
- Mehrez, S.; Karati, S.A.; DolatAbadi, P.T.; Shah, S.N.R.; Azam, S.; Khorami, M.; Assilzadeh, H. Nonlocal dynamic modeling of mass sensors consisting of graphene sheets based on strain gradient theory. Adv. Nano Res. 2020, 9, 221–235. [Google Scholar]
- Jiang, R.W.; Shen, Z.B.; Tang, G.J. Vibration analysis of a single-layered graphene sheet-based mass sensor using the Galerkin strip distributed transfer function method. Acta Mech. 2016, 227, 2899–2910. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.-W. A structural mechanics approach for the analysis of carbon nanotubes. Int. J. Solids Struct. 2003, 40, 2487–2499. [Google Scholar] [CrossRef]
- Natsuki, T.; Matsuyama, N.; Shi, J.X.; Ni, Q.Q. Vibration analysis of nanomechanical mass sensor using carbon nanotubes under axial tensile load. Appl. Phys. A 2014, 116, 1001–1007. [Google Scholar] [CrossRef]
- Natsuki, T.; Shi, J.X.; Ni, Q.Q. Vibration analysis of nanomechanical mass sensor using double-layered graphene sheets resonators. J. Appl. Phys. 2013, 114, 094307. [Google Scholar] [CrossRef]
- Shi, J.; Natsuki, T.; Lei, X.W.; Ni, Q.Q. Buckling instability of carbon nanotube Atomic Force Microscope probe clamped in an elastic medium. J. Nanotechnol. Eng. Med. 2012, 3, 02903. [Google Scholar] [CrossRef]
- Lei, X.W.; Natsuki, T.; Shi, J.X.; Ni, Q.Q. An atomic-resolution nanomechanical mass sensor based on circular monolayer graphene sheet: Theoretical analysis of vibrational properties. J. Appl. Phys. 2013, 113, 154313. [Google Scholar] [CrossRef]
- Shen, Z.B.; Tang, H.L.; Li, D.K.; Tang, G.J. Vibration of single-layered graphene sheet-based nanomechanical sensor via nonlocal Kirchhoff plate theory. Comput. Mater. Sci. 2012, 61, 200–205. [Google Scholar] [CrossRef]
- Patel, A.M.; Joshi, A.M. Characterizing the nonlinear behaviour of double walled carbon nanotube based nano mass sensor. Microsyst. Technol. 2017, 23, 1879–1889. [Google Scholar] [CrossRef]
- Natsuki, T.; Matsuyama, N.; Ni, Q.Q. Vibration analysis of carbon nanotube-based resonator using nonlocal elasticity theory. Appl. Phys. A 2015, 120, 1309–1313. [Google Scholar] [CrossRef]
- Zhou, S.M.; Sheng, L.P.; Shen, Z.B. Transverse vibration of circular graphene sheet-based mass sensor via nonlocal Kirchhoff plate theory. Comput. Mater. Sci. 2014, 86, 73–78. [Google Scholar] [CrossRef]
- Natsuki, T. Theoretical analysis of vibration frequency of graphene sheet used as nanomechanical mass sensor. Electronics 2015, 4, 723–738. [Google Scholar] [CrossRef] [Green Version]
- Natsuki, T.; Yiwada, A.; Natsuki, J. Influence of temperature on vibrational frequency of graphene sheet used as nano-scale sensing. C-J. Carbon Res. 2017, 3, 4. [Google Scholar] [CrossRef] [Green Version]
- Menacer, F.; Dibi, Z.; Kadri, A.; Djeffal, F. A new smart nanoforce sensor based on suspended gate SOIMOSFET using carbon nanotube. Measurement 2018, 125, 232–242. [Google Scholar] [CrossRef]
- Menacer, F.; Kadri, A.; Dibi, Z. Modeling of a smart nano force sensor using finite elements and neural networks. Int. J. Autom. Comput. 2020, 17, 279–291. [Google Scholar] [CrossRef]
- Natsuki, T.; Ni, Q.Q.; Endo, M. Stability analysis of double-walled carbon nanotubes as AFM probes based on a continuum model. Carbon 2011, 49, 2532–2537. [Google Scholar] [CrossRef]
- Natsuki, T.; Urakam, K. Analysis of vibration frequency of carbon nanotubes used as nano-force sensors considering clamped boundary condition. Electronics 2019, 8, 1082. [Google Scholar] [CrossRef] [Green Version]


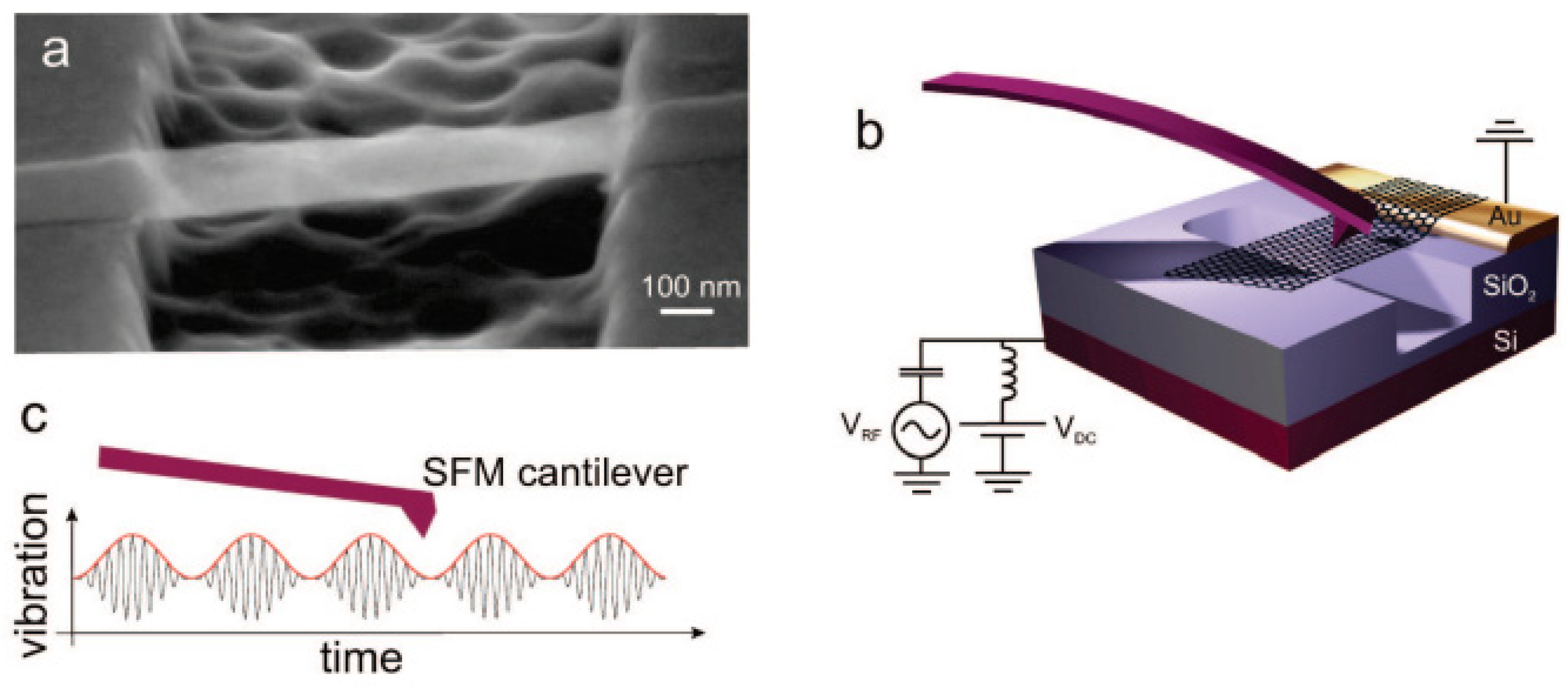
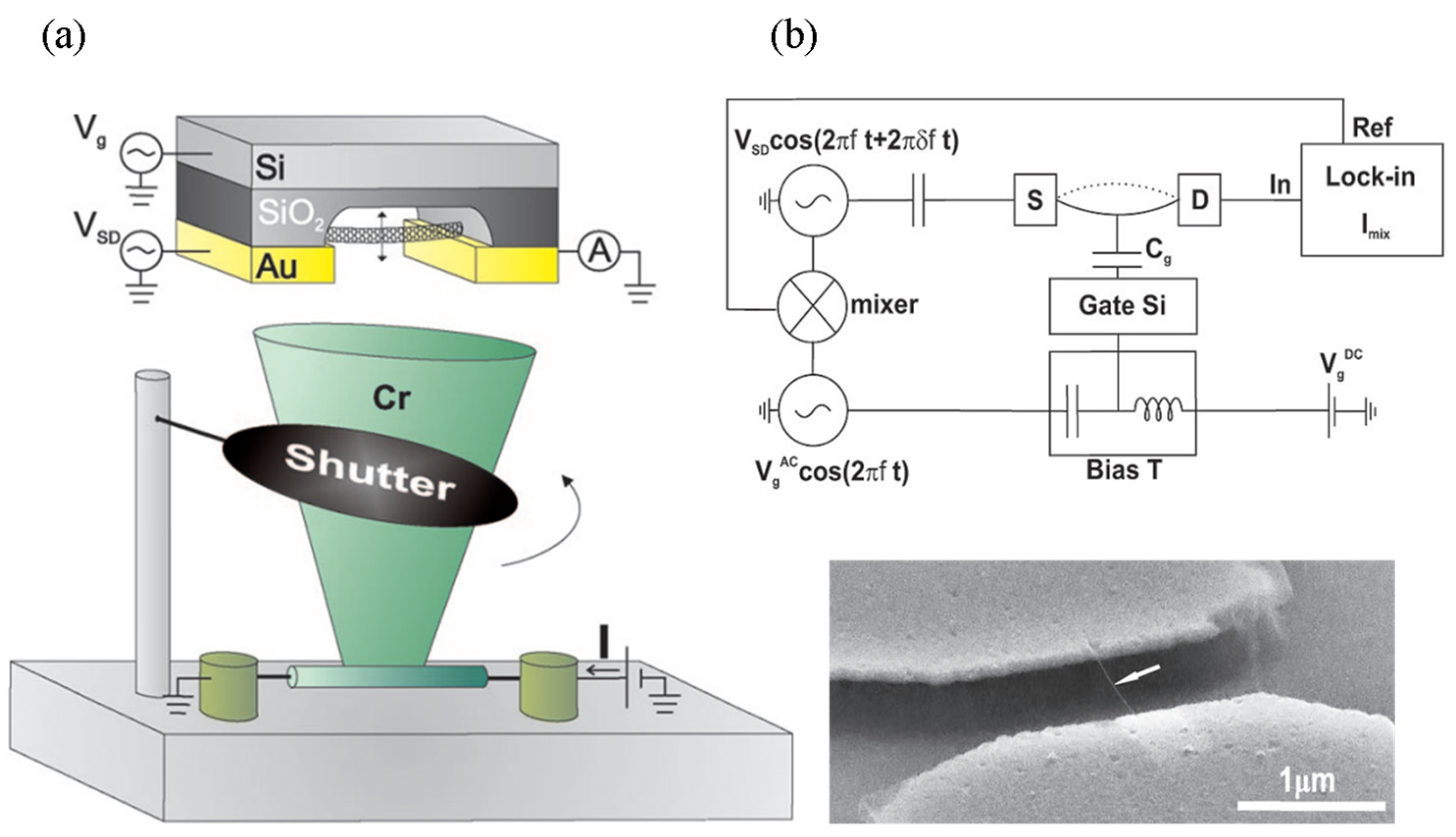
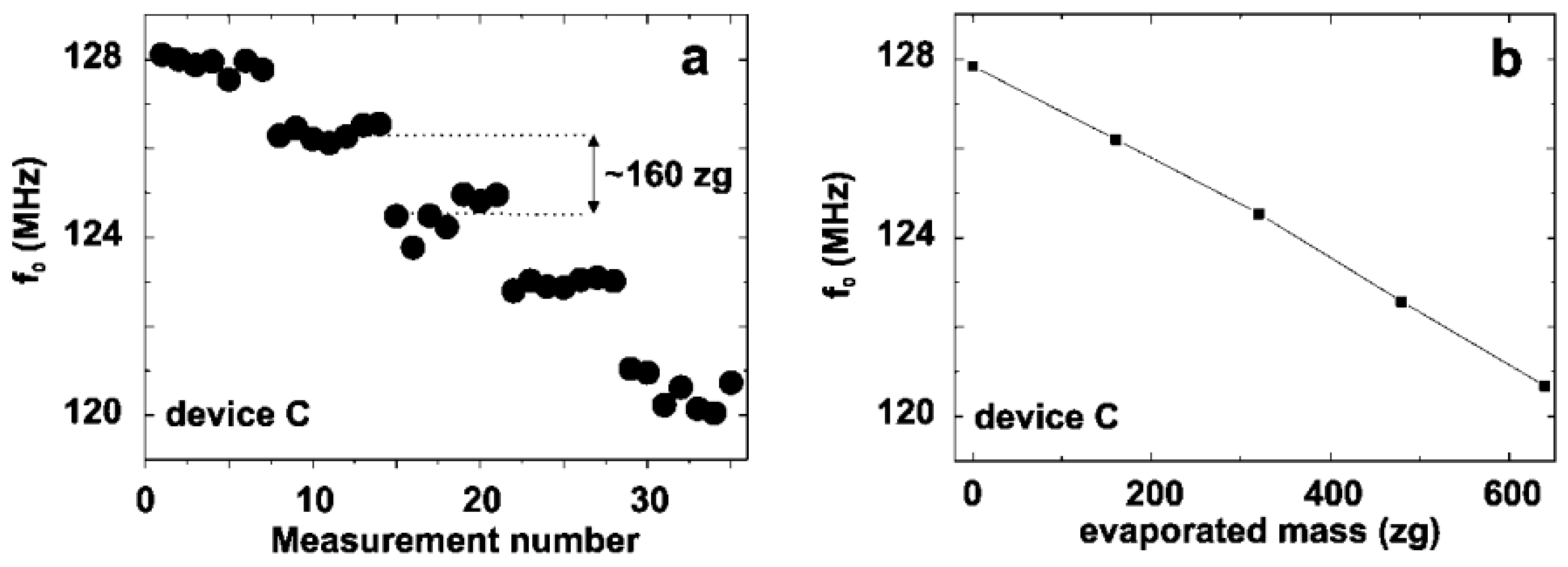
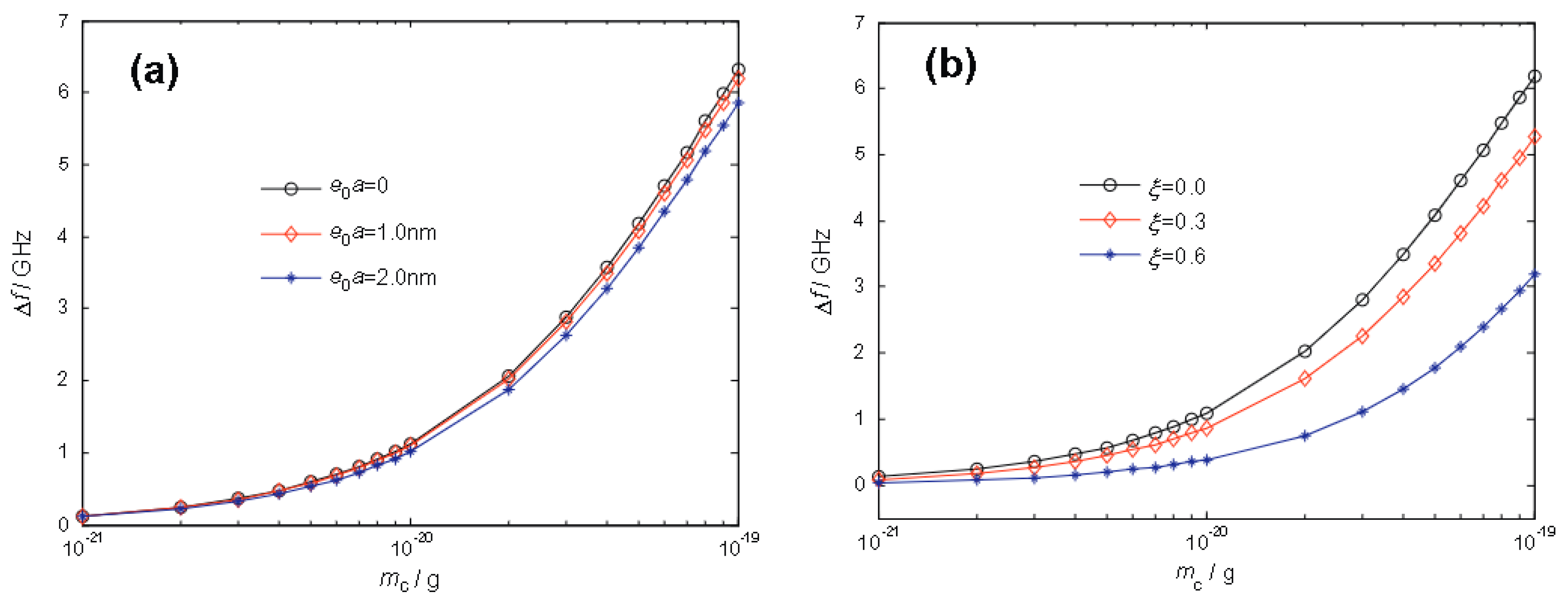

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Natsuki, T.; Natsuki, J. Constitutive Modeling of Mechanical Behaviors of Carbon-Based CNTs and GSs, and Their Sensing Applications as Nanomechanical Resonators: A Review. Nanomaterials 2023, 13, 1834. https://doi.org/10.3390/nano13121834
Natsuki T, Natsuki J. Constitutive Modeling of Mechanical Behaviors of Carbon-Based CNTs and GSs, and Their Sensing Applications as Nanomechanical Resonators: A Review. Nanomaterials. 2023; 13(12):1834. https://doi.org/10.3390/nano13121834
Chicago/Turabian StyleNatsuki, Toshiaki, and Jun Natsuki. 2023. "Constitutive Modeling of Mechanical Behaviors of Carbon-Based CNTs and GSs, and Their Sensing Applications as Nanomechanical Resonators: A Review" Nanomaterials 13, no. 12: 1834. https://doi.org/10.3390/nano13121834
APA StyleNatsuki, T., & Natsuki, J. (2023). Constitutive Modeling of Mechanical Behaviors of Carbon-Based CNTs and GSs, and Their Sensing Applications as Nanomechanical Resonators: A Review. Nanomaterials, 13(12), 1834. https://doi.org/10.3390/nano13121834





