Characteristics of Partially Coherent Circular Flattened Gaussian Vortex Beams in Turbulent Biological Tissues

Abstract

The characteristics of partially coherent circular flattened Gaussian vortex beams in turbulent biological tissues are investigated, and the analytical formula for the cross-spectral density of this beam is derived. According to the cross-spectral density matrix, the average intensity and degree of polarization can be obtained. By numerical simulation, the distributions of the normalized average intensity and degree of polarization of partially coherent circular flattened Gaussian vortex beams are demonstrated on the research plane of turbulent biological tissues. The effects of the two beam parameters, the topological charge, the two transverse coherent lengths, and the structural constant of biological turbulence on the normalized average intensity and degree of polarization are analyzed. This study is of great significance for the potential application of partially coherent circular flattened Gaussian vortex beams in medical imaging and medical diagnosis.

1. Introduction

Since the intensity distribution of a flat-topped beam is almost uniform, the flat-topped beam has the potential for application in some special fields. So far, research on the propagation factor, kurtosis parameter, focal shift, fractional Fourier transform, spectral change, and laser coupling of the flat-topped beam has been reported [1,2,3,4,5,6,7]. The properties of a flat-topped beam passing through a misaligned optical system, an apertured optical system, and an unapertured optical system have been extensively studied [8,9,10,11,12,13,14]. The average intensity, scintillation index, and Stokes parameters of the flat-topped beam have also been demonstrated in the turbulent atmosphere and ocean [15,16,17,18,19,20,21,22,23,24]. It was found that an efficient optical system could be proposed to generate flat-topped beams [25], while complex amplitude modulation only requires a phase spatial light modulator [26]. Many different mathematical models have been proposed to describe the properties of the flat-topped beam [27,28,29,30], with one of the successful models being the circular flattened Gaussian beam [31,32]. The advantage of this beam is that its intensity distribution varies continuously from the maximum value to zero. The characteristics of circular flattened Gaussian beams passing through an apertured and unapertured optical system in the turbulent atmosphere have been investigated [33,34]. Under the non-approximate condition, the vector structure properties of a circular flattened Gaussian beam have been revealed [35].

It should be noted that fully coherent optical beams are just theoretical models since all practical optical beams are only partially coherent [36]. Accordingly, the partially coherent circular flattened Gaussian beam has been proposed [37]. Moreover, the circular flattened Gaussian beam can be extended to the circular flattened Gaussian vortex beam. Therefore, the object of this paper is a partially coherent circular flattened Gaussian vortex beam. In addition to propagating in free space, nonlinear medium, uniaxial crystals, and turbulent atmosphere, optical beams can also propagate in turbulent biological tissues, which is of even more importance for researchers of agriculture and forestry. Many applications, such as, optical imaging and medical diagnosis, involve the propagation of optical beams in turbulent biological tissues [38,39]. The difference in the initial distribution of the optical beams propagating in a turbulent biological tissue will affect the quality of optical imaging and thus the accuracy of the medical diagnosis. Therefore, various types of beams, such as an anisotropic electromagnetic beam, stochastic electromagnetic vortex beam, Gaussian–Schell beam, Laguerre–Gaussian–Schell beam, Bessel–Gaussian–Schell beam, and optical spherical wave, are reported to propagate in turbulent biological tissues [40,41,42,43]. However, as far as we know, there are no updated reports on partially coherent circular flattened Gaussian vortex beam propagating in turbulent biological tissues. If the partially coherent circular flattened Gaussian vortex beam can continue to maintain the advantages of the flat-topped intensity distribution in turbulent biological tissues, a similar light intensity distribution can be achieved in the largest possible illumination region, which is the purpose of this paper. In the rest of this paper, the properties of partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues are investigated.

2. Partially Coherent Circular Flattened Gaussian Vortex Beams Propagating in a Turbulent Biological Tissue

Because of the symmetry of the studied beam, a cylindrical coordinates system is an optimal choice. The longitudinal axis namely z-axis is the propagating axis; z = 0 is the initial plane, and (ρ₀, θ₀) are the transverse coordinates in the initial plane. The cross-spectral density matrix is used to describe the second-order coherence and the polarized characteristics of partially coherent optical beams. The 2 × 2 matrix of cross-spectral density for a partially coherent circular flattened Gaussian vortex beam in the initial plane z = 0 is described by [44]

$$\overleftrightarrow{W}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)=\left[\begin{array}{cc}{W}_{xx}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)& W_{xy}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)\\ W_{yx}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)& W_{yy}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)\end{array}\right],$$

(1)

with $W_{xx}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)$ and $W_{yy}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)$ being given by

$$W_{jj}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)=I_{j0}{\displaystyle \sum _{n=1}^N{b}_n\exp \left(-\frac{n{\rho }_{01}^2}{w_0^2}\right)}{\displaystyle \sum _{n^{\prime }=1}^N{b}_{n^{\prime }}\exp \left(-\frac{n^{\prime }{\rho }_{02}^2}{w_0^2}\right)}\exp [im({\theta }_{01}-{\theta }_{02})]\times \exp \left[-\frac{{\rho }_{01}^2+{\rho }_{02}^2-2{\rho }_{01}{\rho }_{02}\cos ({\theta }_{01}-{\theta }_{02})}{{\delta }_{jj}^2}\right]$$

(2)

where j = x or y. (x, y) are the transverse coordinates of the Cartesian coordinates system, $I_{j0}={\left|E_{j0}^{}\right|}^2$ and E_j0 are the characteristic amplitude, $b_n={(-1)}^{n-1}N!/(Nn!(N-n)!)$ is the weight coefficient, N is an integer and is assumed to be greater than or equal to 2, $w_0/\sqrt{n}$ is the Gaussian waist, m is the topological charge, δ_jj is the transverse coherent length in the j-direction. A Schell model source is employed in Equation (2). Here, the simplest case is considered, namely, W_xy(ρ₀₁, ρ₀₂, θ₀₁, θ₀₂, 0) = W_yx(ρ₀₁, ρ₀₂, θ₀₁, θ₀₂, 0) = 0, which means that the two orthogonal components are irrelevant in the initial plane. The intensity in the initial plane is given by W_xx(ρ₀, ρ₀, θ₀, θ₀, 0) + W_yy(ρ₀, ρ₀, θ₀, θ₀, 0). Therefore, the intensity in the initial plane is determined by the parameters N and w₀, as shown in Figure 1. w₀ = 2 μm in Figure 1a and N = 2 in Figure 1b. Though the flatness of the partially coherent circular flattened Gaussian vortex beam depends on the two parameters N and w₀, the role of the parameter N is more obvious than that of the parameter w₀.

By using the extended Huygens–Fresnel integral formula, the cross-spectral density of the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues can be expressed as [41,45]:

$$\begin{gathered} W_{jj}^{}(\rho ,\theta ,\rho ,\theta ,z)=\frac{k^2}{4{\pi }^2{z}^2}{\displaystyle {\int }_0^{\infty }{\displaystyle {\int }_0^{\infty }{\displaystyle {\int }_0^{2\pi }{\displaystyle {\int }_0^{2\pi }{W}_{jj}^{}({\rho }_{01},{\rho }_{02},{\theta }_{01},{\theta }_{02},0)}}}}<\exp [\psi ({\rho }_{01},{\theta }_{01},\rho ,\theta )+{\psi }^{\ast }({\rho }_{02},{\theta }_{02},\rho ,\theta )]> \\ \times \exp \left\{\frac{ik}{2z}[{\rho }_{02}^2-{\rho }_{01}^2-2{\rho }_{02}\rho \cos ({\theta }_{02}-\theta )+2{\rho }_{01}\rho \cos ({\theta }_{01}-\theta )]\right\}{\rho }_{01}{\rho }_{02}d{\rho }_{01}d{\rho }_{02}d{\theta }_{01}d{\theta }_{02}, \end{gathered}$$

(3)

with $<\exp [\psi ({\rho }_{01},{\theta }_{01},\rho ,\theta )+{\psi }^{\ast }({\rho }_{02},{\theta }_{02},\rho ,\theta )]>$ being given by

$$<\exp [\psi ({\rho }_{01},{\theta }_{01},\rho ,\theta )+{\psi }^{\ast }({\rho }_{02},{\theta }_{02},\rho ,\theta )]>=\exp \left[-\frac{{\rho }_{01}^2+{\rho }_{02}^2-2{\rho }_{01}{\rho }_{02}\cos ({\theta }_{01}-{\theta }_{02})}{{\sigma }_0^2}\right],$$

(4)

where k = 2π/λ. λ is the wavelength of the incident beam. The asterisk denotes the complex conjugation, $\left|{\sigma }_0\right|=0.22{(C_n^2{k}^{2}z)}^{-1/2}$, the parameter $C_n^2$ is the structural constant of the refraction index in the biological tissue. The spherical wave lateral coherence length in turbulent atmosphere is characterized by σ₀ = (0.545$C_n^2$k²z)^−3/5. Moreover, the order of magnitude of $C_n^2$ in turbulent biological tissues is generally 1–10² m⁻¹, while the order of magnitude of $C_n^2$ in turbulent atmosphere is generally 10⁻¹⁵–10⁻¹³ m^−2/3.

In order to perform the integral in Equation (3), the following mathematical formulae are used [46]

$$\exp \left[\frac{ik\rho {\rho }_{01}}{z}\cos (\theta -{\theta }_{01})\right]={\displaystyle \sum _{l=-\infty }^{\infty }{i}^l}{J}_l\left(\frac{k\rho {\rho }_{01}}{z}\right)\exp [il(\theta -{\theta }_{01})],$$

(5)

$${\displaystyle {\int }_0^{2\pi }\exp [-im{\theta }_{01}+2c{\rho }_{01}{\rho }_{02}\cos ({\theta }_{01}-{\theta }_{02})]}d{\theta }_{01}=2\pi \exp (-im{\theta }_{02})I_m(2c{\rho }_{01}{\rho }_{02}),$$

(6)

$$J_l(x)=\frac{i^l}{2\pi }{\displaystyle {\int }_0^{2\pi }\exp (-ix\cos \phi -il\phi )}d\phi ,$$

(7)

where J_l(⋅) and I_m(⋅) are lth-order Bessel and mth-order modified Bessel functions of the first kind, respectively. The cross-spectral density of the partially coherent circular flattened Gaussian vortex beam propagating in turbulent biological tissues can be rewritten as:

$$W_{jj}^{}(\rho ,\theta ,\rho ,\theta ,z)=\frac{k^2{I}_{j0}}{z^2}{\displaystyle {\int }_0^{\infty }{\displaystyle {\int }_0^{\infty }{\displaystyle \sum _{n=1}^N{\displaystyle \sum _{n^{\prime }=1}^N{\displaystyle \sum _{l=-\infty }^{\infty }{b}_n{b}_{n^{\prime }}}}}{J}_l\left(\frac{k\rho {\rho }_{01}}{z}\right)}}{J}_l\left(\frac{k\rho {\rho }_{02}}{z}\right)\exp (-{\alpha }_1{\rho }_{01}^2-{\alpha }_2{\rho }_{02}^2)I_{l-m}(2{\alpha }_3{\rho }_{01}{\rho }_{02}){\rho }_{01}{\rho }_{02}d{\rho }_{01}d{\rho }_{02},$$

(8)

with the auxiliary parameters α₁, α₂, and α₃ being defined by:

$${\alpha }_1=\frac{n}{w_0^2}+\frac{1}{{\sigma }_0^2}+\frac{1}{{\delta }_{jj}^2}+\frac{ik}{2z},$$

(9)

$${\alpha }_2=\frac{n^{\prime }}{w_0^2}+\frac{1}{{\sigma }_0^2}+\frac{1}{{\delta }_{jj}^2}-\frac{ik}{2z},$$

(10)

$${\alpha }_3=\frac{1}{{\sigma }_0^2}+\frac{1}{{\delta }_{jj}^2}.$$

(11)

To obtain the analytical outcome of Equation (8), I_l−m(⋅) is first expanded as:

$$I_{l-m}(2{\alpha }_3{\rho }_{01}{\rho }_{02})={\displaystyle \sum _{s=0}^{\infty }\frac{{({\alpha }_3{\rho }_{01}{\rho }_{02})}^{2s+l-m}}{s!\Gamma (s+l-m)}},$$

(12)

Then, we recall the following integral formula [46]:

$${\displaystyle {\int }_0^{\infty }{t}^{m-1}\exp (-\alpha t^2)J_l(\beta t)dt}=\frac{{\alpha }^{-m/2}}{2l!}\Gamma \left(\frac{l+m}{2}\right){\left(\frac{{\beta }^2}{4\alpha }\right)}^{l/2}\exp \left(-\frac{{\beta }^2}{4\alpha }\right){}_1{F}_1\left(\frac{l-m}{2}+1;l+1;\frac{{\beta }^2}{4\alpha }\right),$$

(13)

where Γ(.) and ₁F₁(.; .; .) are Gamma and Kummer functions, respectively. The cross-spectral density of the partially coherent circular flattened Gaussian vortex beam propagating in turbulent biological tissues can be analytically expressed as:

$$\begin{gathered} W_{jj}^{}(\rho ,\theta ,\rho ,\theta ,z)=\frac{k^2{I}_{j0}}{4z^2}{\displaystyle \sum _{n=1}^N{\displaystyle \sum _{n^{\prime }=1}^N{\displaystyle \sum _{l=-\infty }^{\infty }{\displaystyle \sum _{s=0}^{\infty }\frac{b_n{b}_{n^{\prime }}{\Gamma }^2(p_1){({\alpha }_1{\alpha }_2)}^{-p_1}{\alpha }_3^{2s+l-m}}{{(l!)}^{2}s!\Gamma (s+l-m)}}}}}{\left(\frac{k^2{\rho }^2}{4z^2}\right)}^l \\ \times \exp \left(-\frac{k^2{\rho }^2}{4{\alpha }_1{z}^2}--\frac{k^2{\rho }^2}{4{\alpha }_2{z}^2}\right){}_1{F}_1\left(p_2;l+1;\frac{k^2{\rho }^2}{4{\alpha }_1{z}^2}\right){}_1{F}_1\left(p_2;l+1;\frac{k^2{\rho }^2}{4{\alpha }_2{z}^2}\right), \end{gathered}$$

(14)

where the auxiliary parameters p₁ and p₂ are given by:

$$p_1=l+s-0.5m+1,$$

(15)

$$p_2=-s+0.5m.$$

(16)

Apparently, W_xy(ρ, ρ, θ, θ, z) and W_yx(ρ, ρ, θ, θ, z) are equal to zero. The matrix of the cross-spectral density for the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues $\overleftrightarrow{W}(\rho ,\rho ,\theta ,\theta ,z)$ is given by:

$$\overleftrightarrow{W}(\rho ,\rho ,\theta ,\theta ,z)=\left[\begin{array}{cc}{W}_{xx}^{}(\rho ,\rho ,\theta ,\theta ,z)& W_{xy}^{}(\rho ,\rho ,\theta ,\theta ,z)\\ W_{yx}^{}(\rho ,\rho ,\theta ,\theta ,z)& W_{yy}^{}(\rho ,\rho ,\theta ,\theta ,z)\end{array}\right],$$

(17)

The average intensity and the degree of polarization for the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues turn out to be [44]:

$$I(\rho ,\theta ,z)=\mathrm{Tr}\overleftrightarrow{W}(\rho ,\rho ,\theta ,\theta ,z)=W_{xx}^{}(\rho ,\rho ,\theta ,\theta ,z)+W_{yy}^{}(\rho ,\rho ,\theta ,\theta ,z),$$

(18)

$$P(\rho ,\theta ,z)={\left\{1-\frac{4\det \overleftrightarrow{W}(\rho ,\rho ,\theta ,\theta ,z)}{{[\mathrm{Tr}\overleftrightarrow{W}(\rho ,\rho ,\theta ,\theta ,z)]}^2}\right\}}^{1/2}=\frac{\left|W_{xx}^{}(\rho ,\rho ,\theta ,\theta ,z)-W_{yy}^{}(\rho ,\rho ,\theta ,\theta ,z)\right|}{W_{xx}^{}(\rho ,\rho ,\theta ,\theta ,z)+W_{yy}^{}(\rho ,\rho ,\theta ,\theta ,z)},$$

(19)

where Tr and det mean the trace and the determinant, respectively. The degree of polarization of the partially coherent circular flattened Gaussian vortex beam in the initial plane yields:

$$P^{(0)}=\frac{\left|I_{x0}-I_{y0}\right|}{I_{x0}+I_{y0}}.$$

(20)

3. Numerical Simulation and Results

On the basis of the analytical expressions obtained in Section 2, the characteristics of the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues are simulated numerically. In this paper, numerical simulation was carried out using Mathematica 10.0 software; λ was set to be 0.6328 μm in the following calculations. Since the average intensity of a partially coherent circular flattened Gaussian vortex beam propagating in turbulent biological tissues is determined by w₀, N, m, δ_xx, δ_yy, and C_n², it is necessary to understand the effects of these parameters. 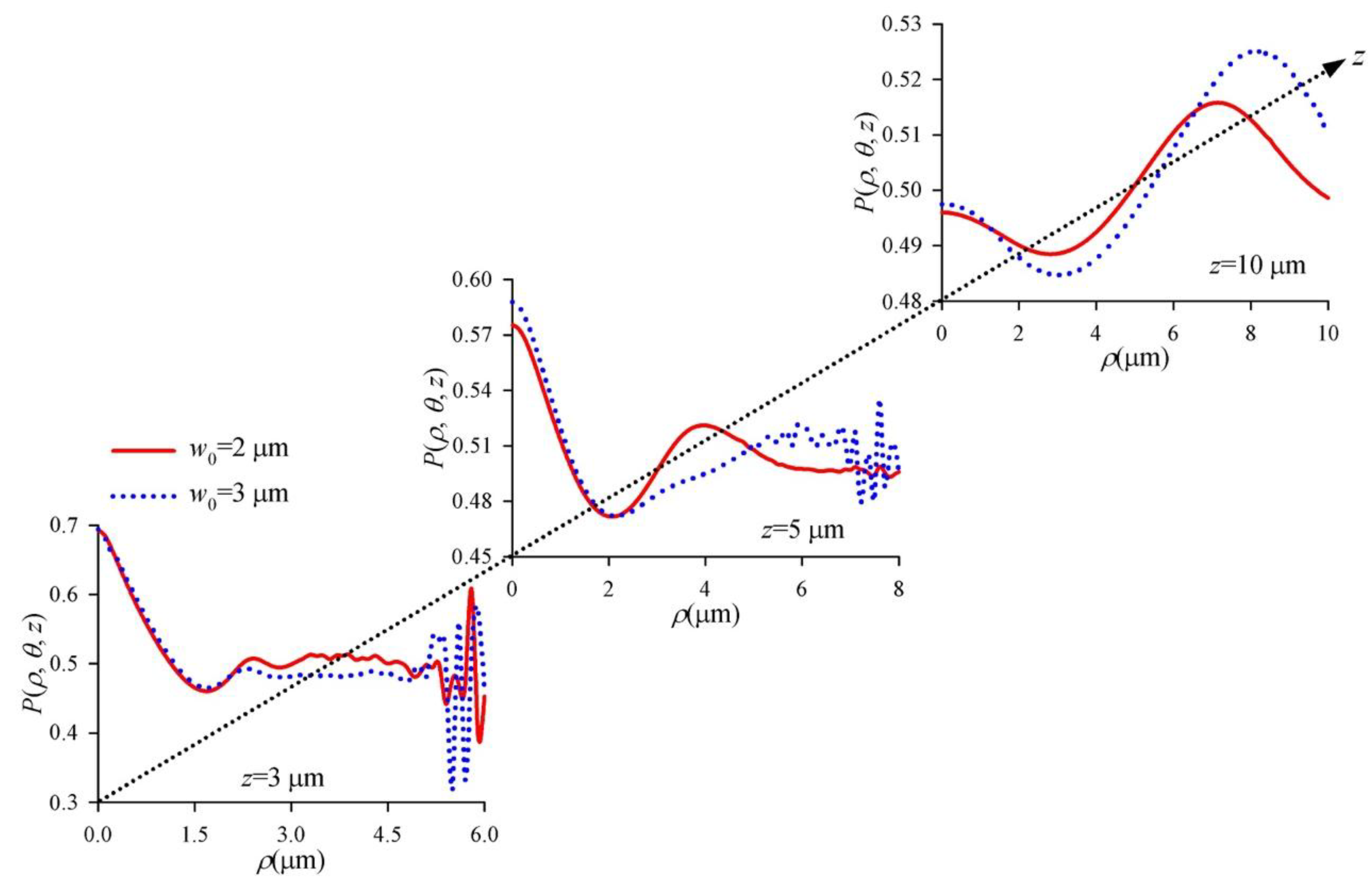Figure 2 shows the normalized average intensity distribution of partially coherent circular flattened Gaussian vortex beam with different w₀ values on the selected study plane in the turbulent biological tissue. The investigation planes were selected as z = 0, 3 μm, 5 μm, and 10 μm, respectively. Other parameters in Figure 2 were set to N = 2, m = 3, δ_xx = 2 μm, δ_yy = 4 μm, P⁽⁰⁾ = 0.5, and C_n² = 10⁻⁴ μm⁻¹. In the initial plane z = 0, the partially coherent circular flattened Gaussian vortex beam is a solid beam with the maximum normalized average intensity on the longitudinal axis. In the investigation plane of the turbulent biological tissue near the initial plane, the partially coherent circular flattened Gaussian vortex beam is a dark-hollow beam. When the axial propagating distance in the turbulent biological tissue augments, the valley value in the distribution of on-axis normalized average intensity gradually increases and eventually vanishes. When z = 10 μm, the flat-topped average intensity distribution is reproduced. When the waist size of the fundamental Gaussian part w₀ increases, the divergence of the partially coherent circular flattened Gaussian vortex beam in the turbulent biological tissue decreases.

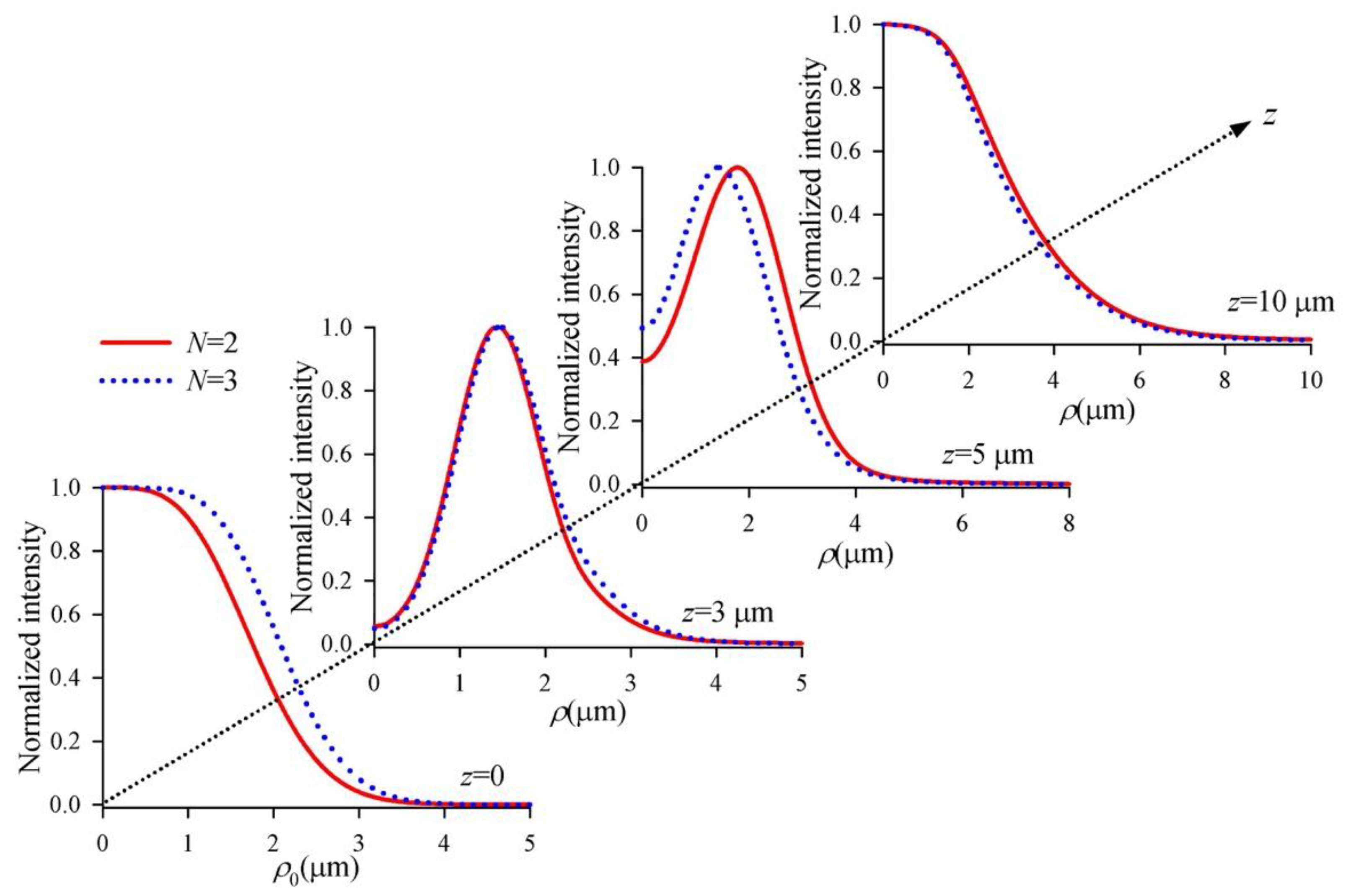
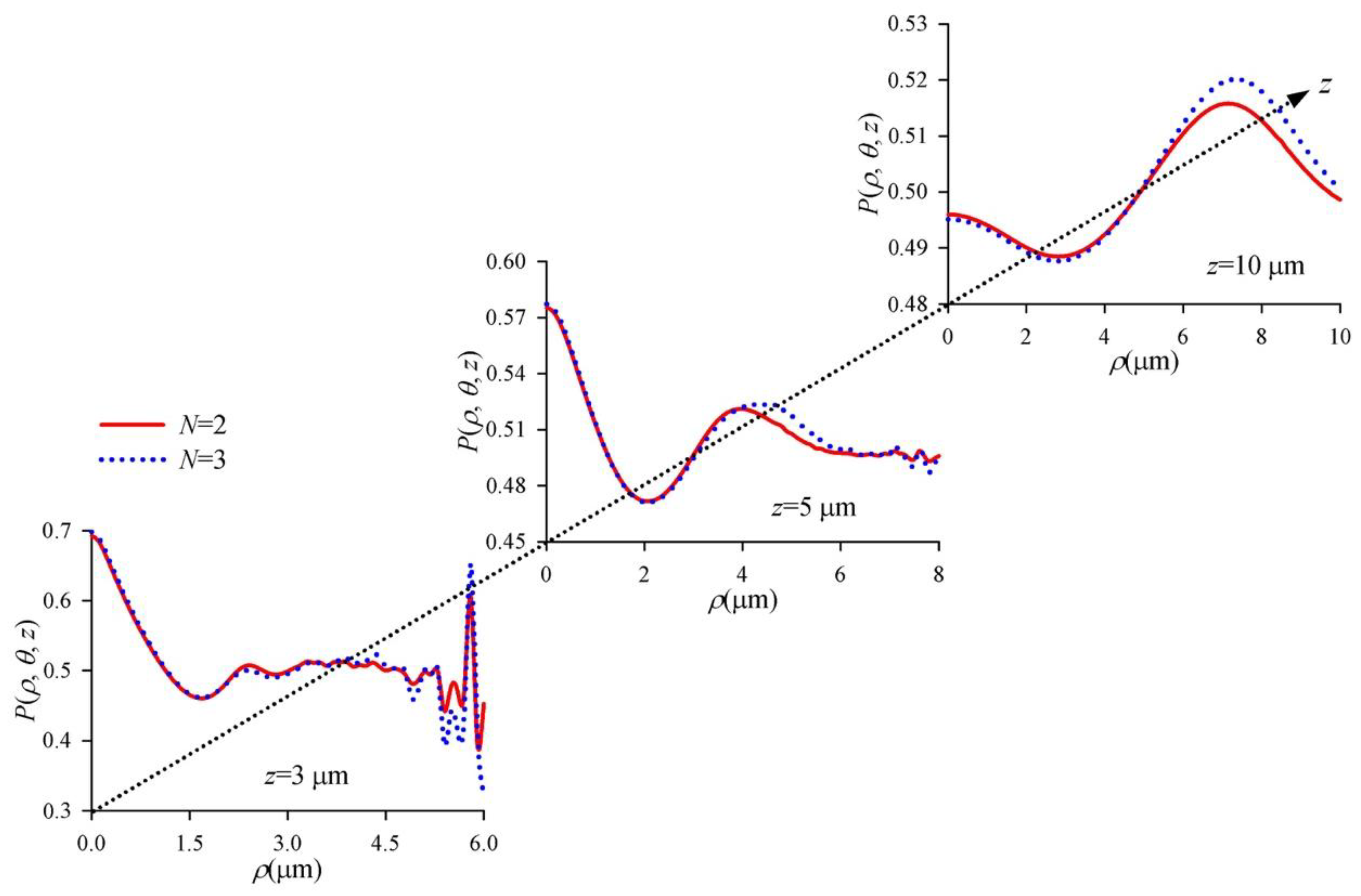
The effect of the parameter N on the distribution of normalized average intensity in the selected investigation plane of the turbulent biological tissue is demonstrated in Figure 3, where w₀ = 2 μm, and the other parameters are the same as those in Figure 2. With the increase of the parameter N, the beam size of the partially coherent circular flattened Gaussian vortex beam in the initial plane becomes bigger, and the flat-topped length in the initial plane becomes longer. With the increase of the parameter N, the valley value in the on-axis normalized average intensity also accelerates upward, while the divergence of the partially coherent circular flattened Gaussian vortex beam in the turbulent biological tissue decreases. This results in a beam size, in the case of N = 3, being smaller than in the case of N = 2 in the investigation plane z = 5 μm. 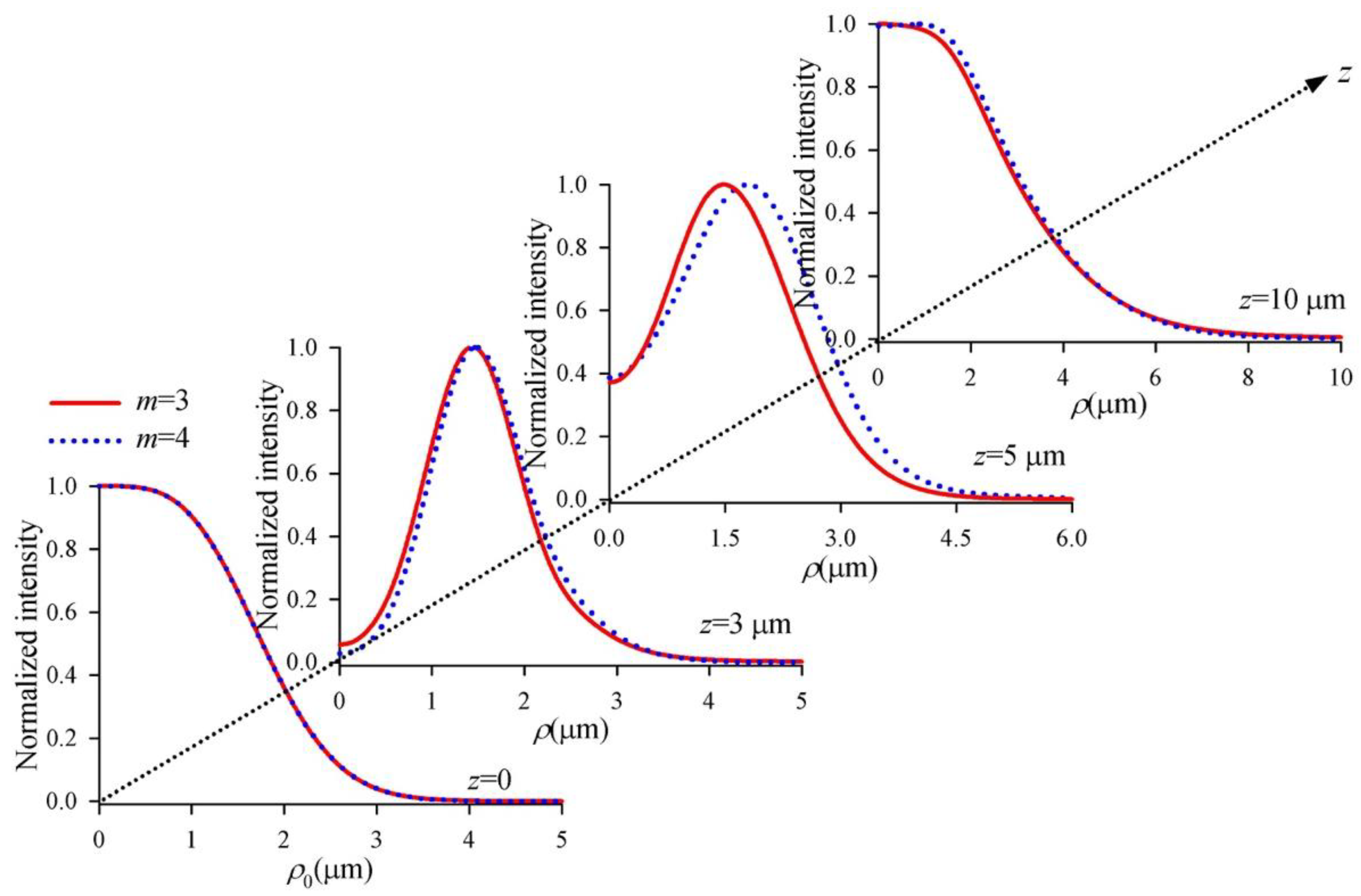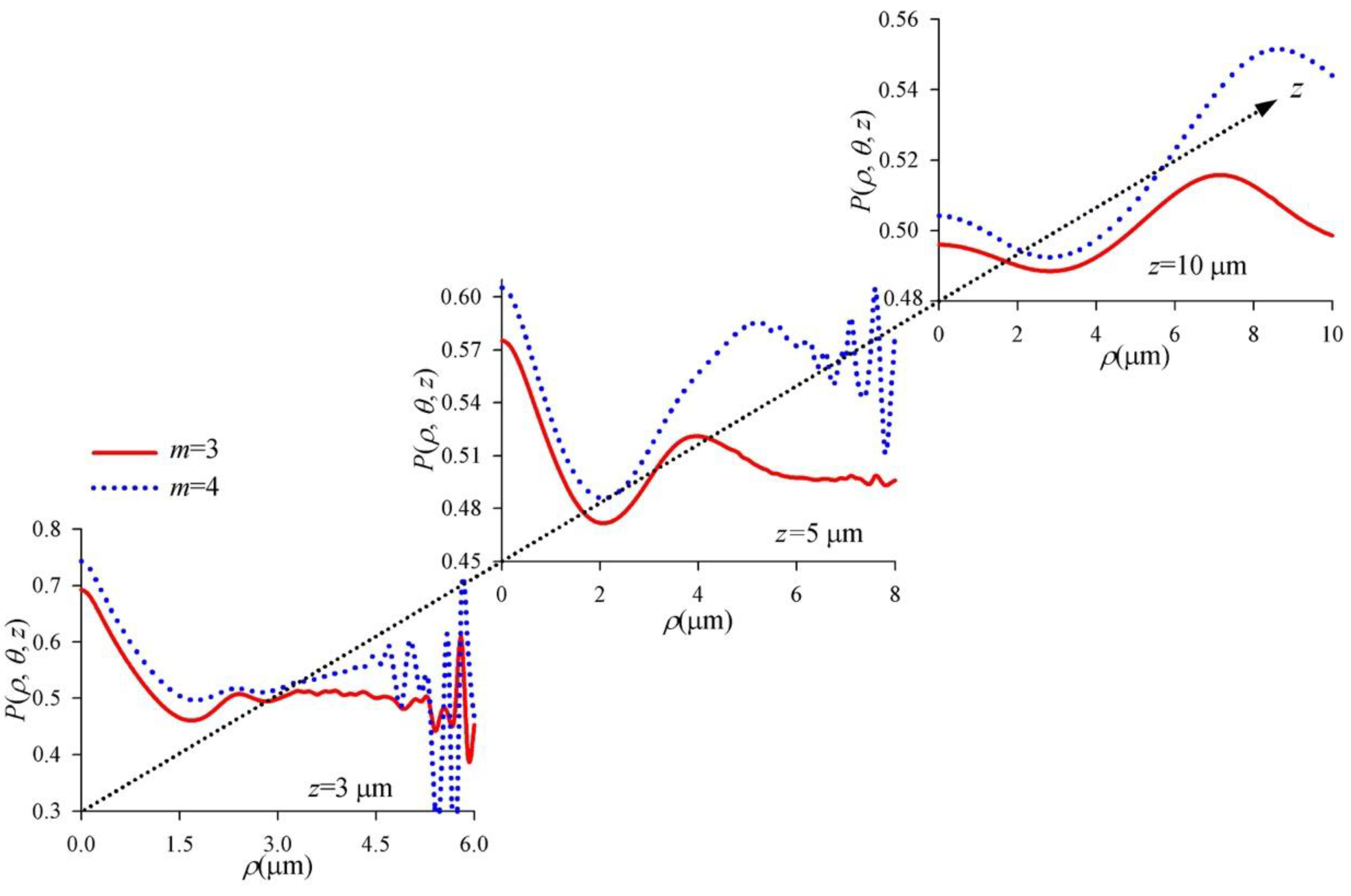The flat-topped average intensity distribution is also reproduced in the investigation plane z = 10 μm. In the investigation plane of the turbulent biological tissue, the effect of the topological charge m on the distribution of normalized average intensity is shown in Figure 4, where w₀ = 2 μm. The other parameters in Figure 4 are the same as in Figure 2. In the research plane of the turbulent biological tissue, the beam size, in the case of m = 4, is larger than that in the case of m = 3. When the topological charge m increases, the divergence of the partially coherent circular flattened Gaussian vortex beam in the turbulent biological tissue is more pronounced, and the flat-topped length in the study plane z = 10 μm also increases.

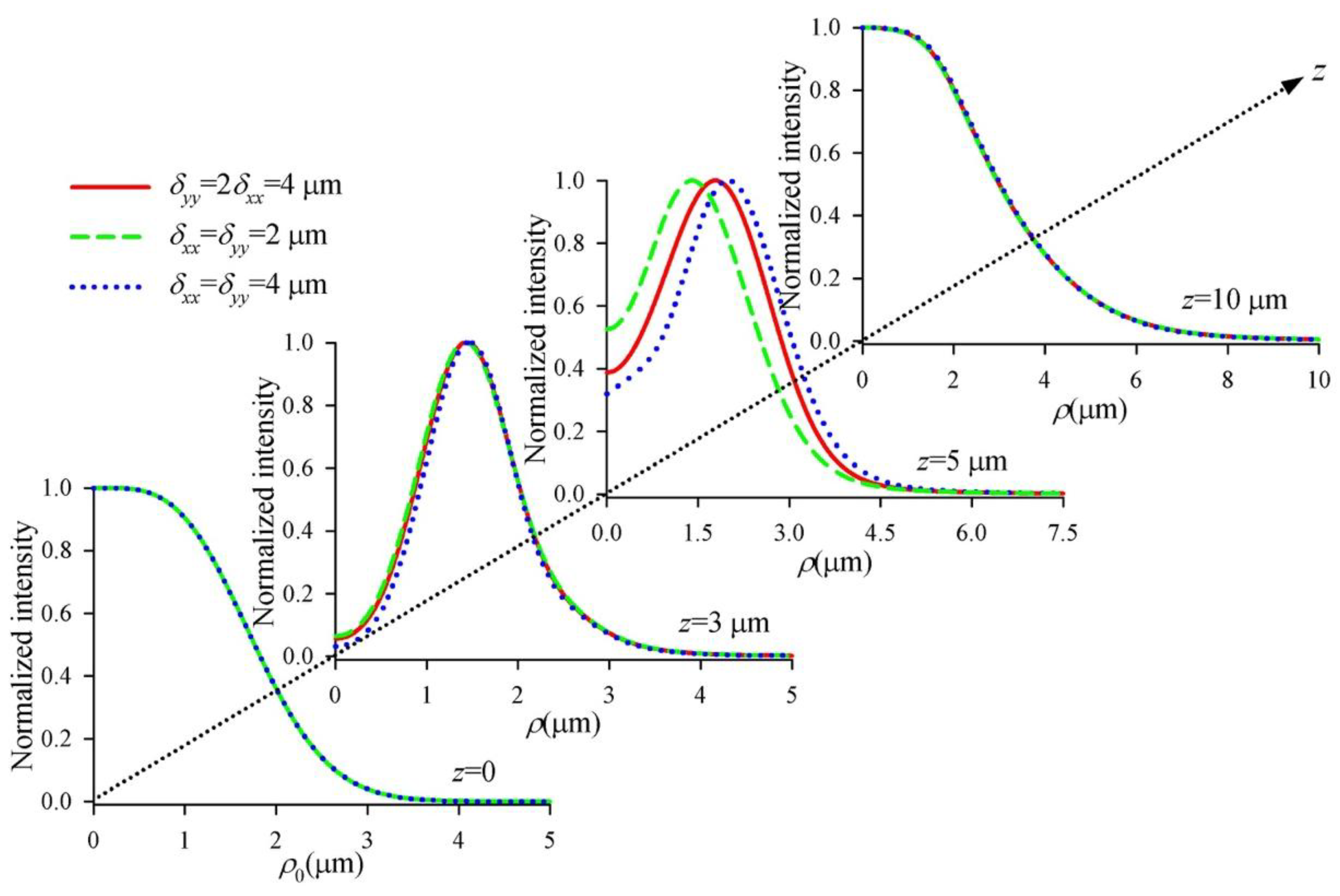
Figure 5 shows the normalized average intensity distribution of a partially coherent circular flattened Gaussian vortex beam with different transverse coherent lengths in the study plane of turbulent biological tissue, where w₀ = 2 μm. The other parameters in Figure 5 are the same as in Figure 2. In the study plane z = 3 μm, the beam size of the partially coherent circular flattened Gaussian vortex beam slightly increases with the increase of the transverse coherent length. In the investigation plane z = 5 μm, the beam size of this partially coherent circular flattened Gaussian vortex beam still increases with the increase of the transverse coherent length. 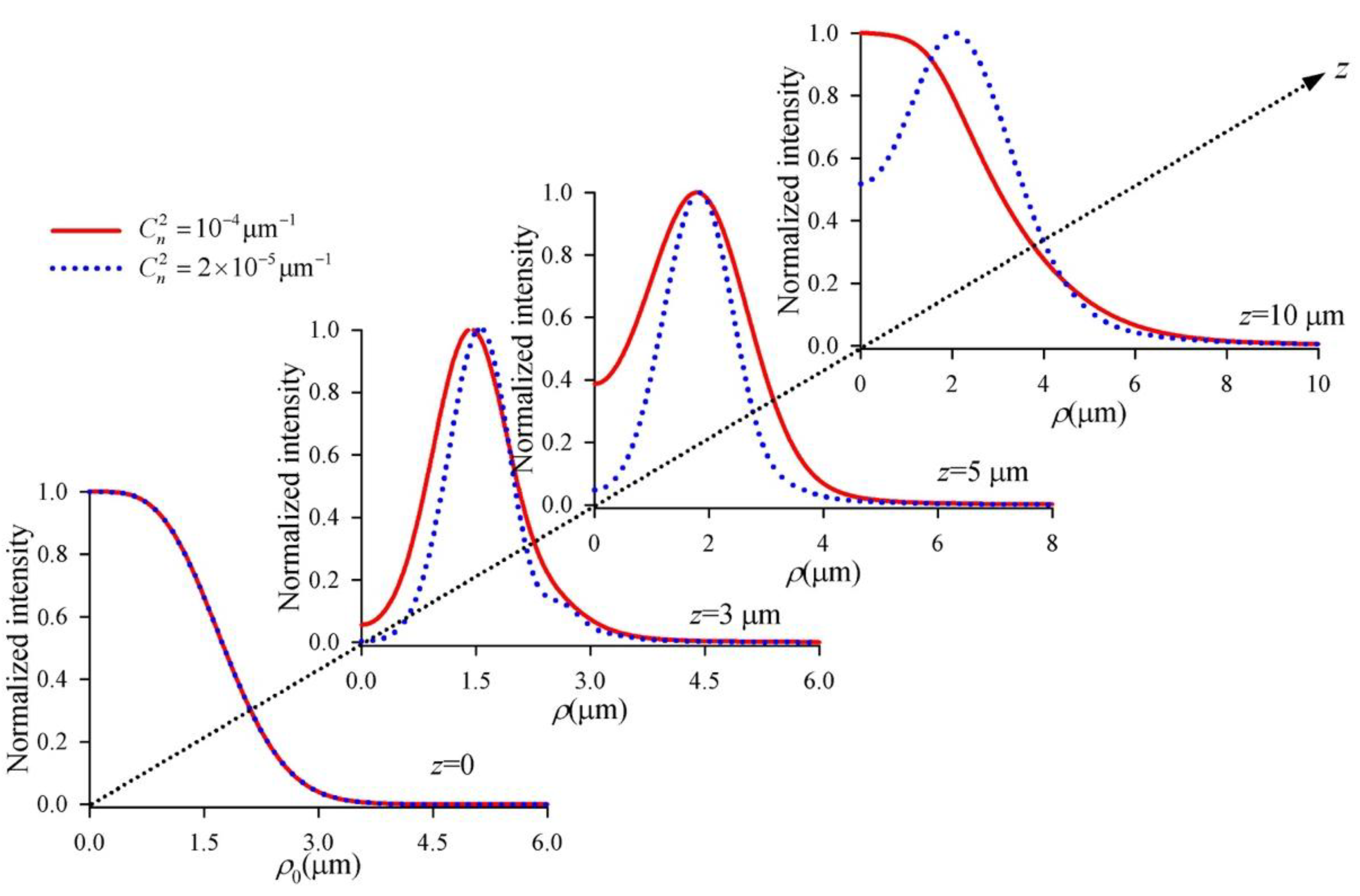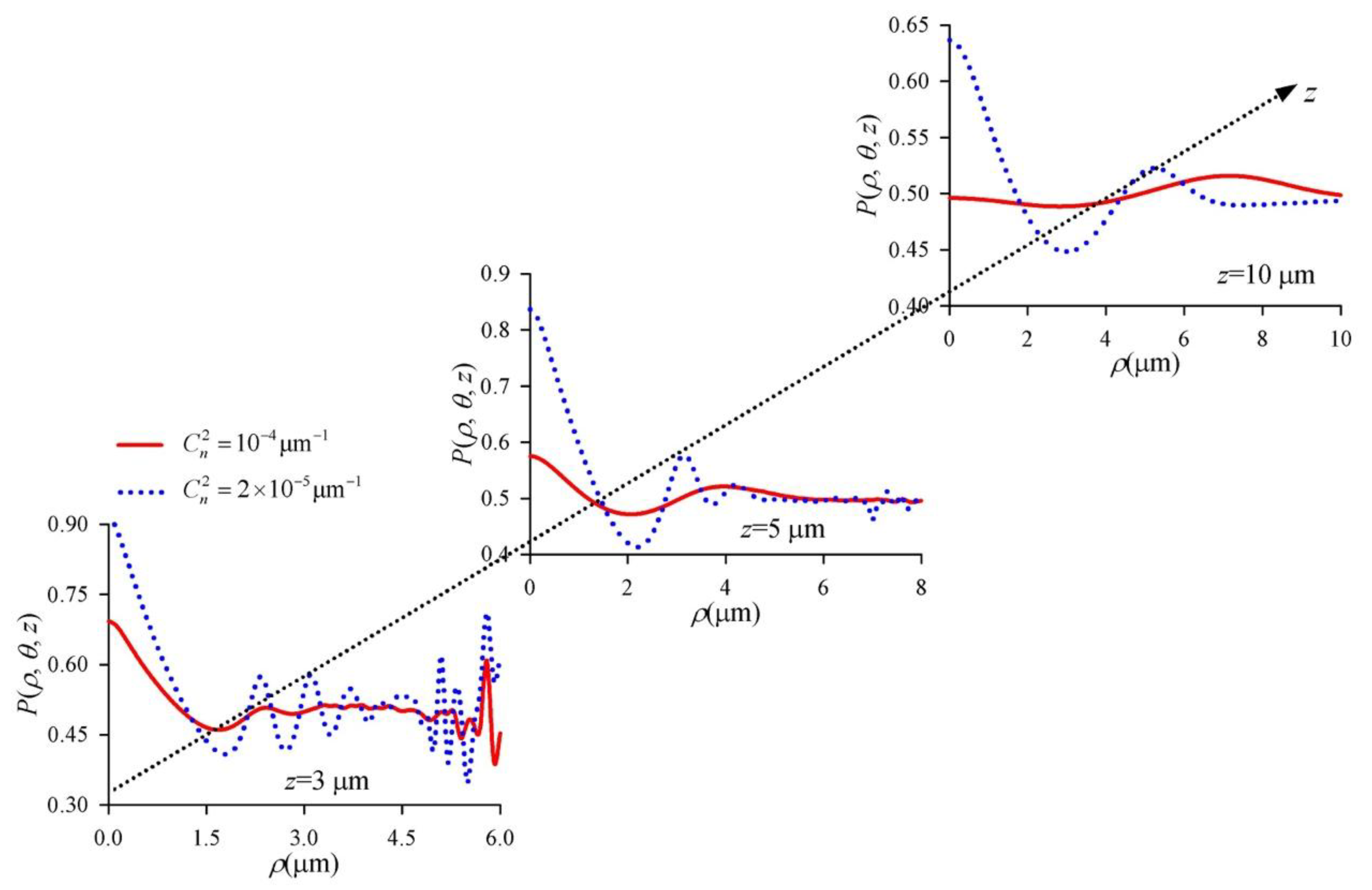However, when the investigation plane is sufficiently far away, such as z = 10 μm, the normalized average intensity distribution of this beam is almost independent of the two transverse coherent lengths. The normalized average intensity distribution of a partially coherent circular flattened Gaussian vortex beam in the biological tissue study plane with different structural constants is shown in Figure 6, where w₀ = 2 μm. The other parameters in Figure 6 are the same as in Figure 2. As the turbulence decreases, the propagation distance in the reserved dark region is extended, and the beam size in the turbulent biological tissue is also reduced. Moreover, the axial propagation distance required to reproduce the flat-topped average intensity distribution lengthens with the decrease of the structural constant.

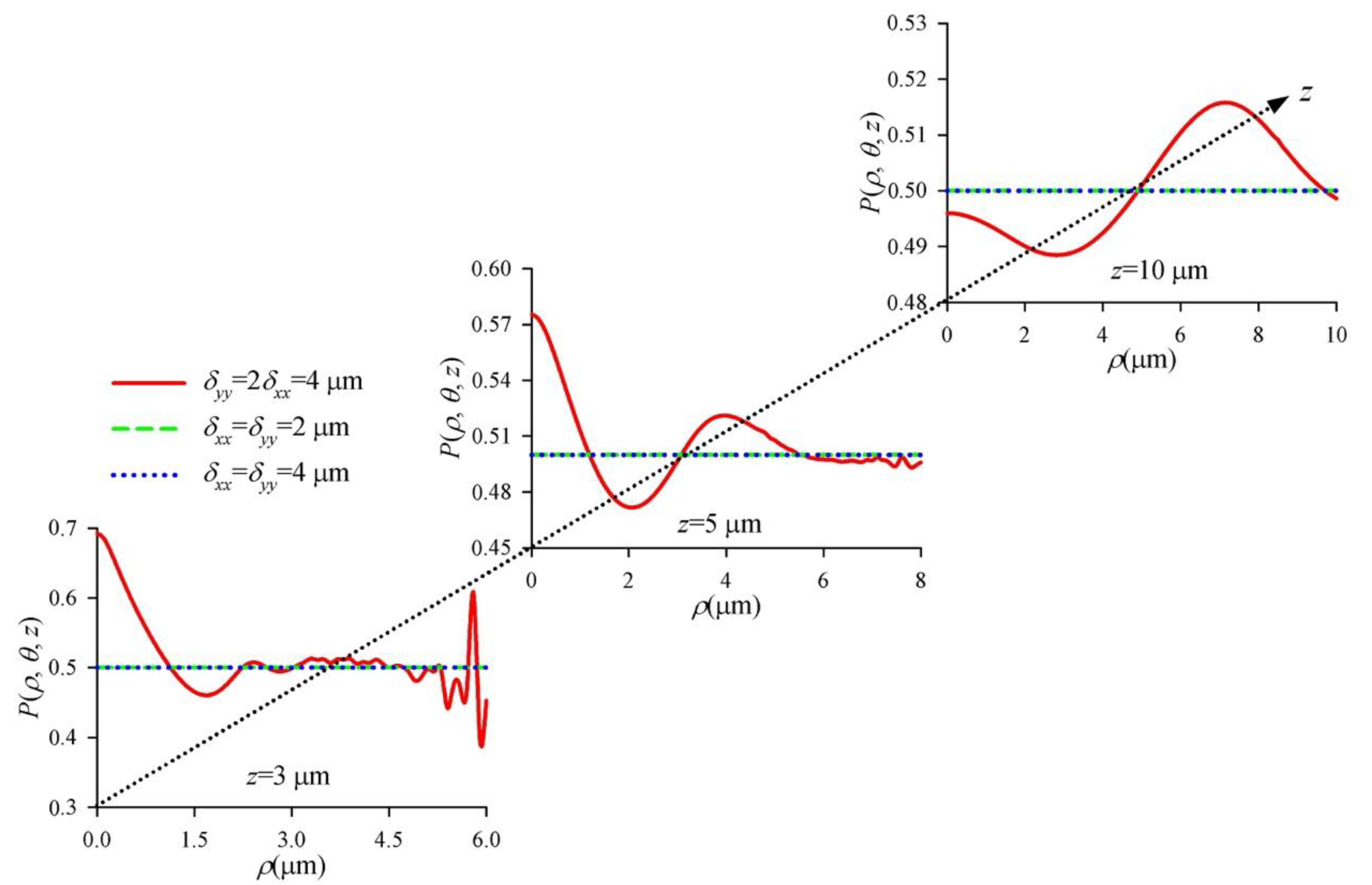
The degree of polarization distribution of a partially coherent circular flattened Gaussian vortex beam in different study planes of the turbulent biological tissue is demonstrated in Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11. Except for different subjects, the parameters in Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11 are equivalent to those in Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6, respectively. Though the degree of polarization is the same in every point of the initial plane, the degree of polarization of a partially coherent circular flattened Gaussian vortex beam in the turbulent biological tissue is usually variable. Only when the two transverse coherent lengths are identical, the degree of polarization of the partially coherent circular flattened Gaussian vortex beam in the turbulent biological tissue is equal to P⁽⁰⁾ and remains unchanged, as shown in Figure 10. In Figure 7, as the value of radial coordinate ρ increases, the degree of polarization first decreases, then increases, and finally decreases again. In the investigation planes z = 3 μm and z = 5 μm, the edge of the degree of polarization may fluctuate, and the fluctuation increases with the increase of the waist size of the fundamental Gaussian part w₀. The reason is as follows. Although W_xx(ρ, ρ, θ, θ, z) and W_yy(ρ, ρ, θ, θ, z) are very small for large ρ and w₀, the variations of W_xx(ρ, ρ, θ, θ, z) and W_yy(ρ, ρ, θ, θ, z) are not synchronous, which results in a highly oscillating behavior of the degree of polarization for large ρ and w₀. Upon propagation in the turbulent biological tissue, the range of change for the degree of polarization narrows. When the flat-topped average intensity is reproduced, the variation range of the degree of polarization is relatively small. When the waist size of the fundamental Gaussian part w₀ increases, the range of change for the degree of polarization in the initial plane z = 10 μm also increases. When the radial coordinate ρ is small, the degree of polarization is insensitive to the parameter N. As the parameter N changes, the curve of the degree of polarization versus the radial coordinate ρ changes little, as shown in Figure 8. When the topological charge m increases, the degree of polarization in the points, except for the edge of the initial plane z = 3 μm, augments, as shown in Figure 9. With the decrease of the turbulence level, the degree of polarization varies more widely and fluctuates more frequently, as shown in Figure 11. In this case, the flat-topped average intensity was been reproduced.

When only the degree of polarization in the initial plane is changed, the normalized average intensity distribution in the selected investigation plane of the turbulent biological tissue will not change, as shown in Figure 12a. In this case, the curve shape of the degree of polarization with respect to the radial coordinates remains basically unvaried, as shown in Figure 12b. Therefore, the conclusions drawn from Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10 and Figure 11 are universal. These seemingly similar diagrams are designed to analyze the effect of each parameter on the average intensity and the degree of polarization. Another advantage of these seemingly similar diagrams is that they facilitate the comparative analysis of the differences between them. Only such an exhaustive analysis can lead to universal conclusions.

4. Conclusions

The propagating feature of a partially coherent circular flattened Gaussian vortex beam was investigated in a turbulent biological tissue. The analytical expression for the cross-spectral density of the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues was presented. As a result, the average intensity and the degree of polarization were obtained by employing the cross-spectral density matrix. By numerical simulation, the distributions of normalized average intensity and degree of polarization for a partially coherent circular flattened Gaussian vortex beam were demonstrated in a selected study plane of a turbulent biological tissue.

The effects of the two beam parameters, the topological charge, the two transverse coherent lengths, and the structural constant on the distribution of normalized average intensity in the turbulent biological tissue were examined. When the waist size of the fundamental Gaussian part w₀ or the parameter N increase, the divergence of the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues decreases. When the topological charge m or the structural constant C_n² decrease, the divergence of the partially coherent circular flattened Gaussian vortex beam in turbulent biological tissues also decreases. When the selected investigation plane is sufficiently far away, the distribution of normalized average intensity is almost independent of the two transversal coherent lengths. Otherwise, the beam size increases with the increase of the two transverse coherent lengths.

When the two transverse coherent lengths are not the same, the degree of polarization for a partially coherent circular flattened Gaussian vortex beam in a turbulent biological tissue is variable. Upon propagation in a turbulent biological tissue, the range of change for the degree of polarization narrows. With the increase of the waist size of the fundamental Gaussian part w₀, the range of change for the degree of polarization also increases. The curve of the degree of polarization hardly varies with the parameter N. When the topological charge m increases, the degree of polarization increases. As the structural constant C_n² decreases, the variation range for the degree of polarization becomes wider.

When propagating in a turbulent biological tissue, a partially coherent circular flattened Gaussian vortex beam has the advantage of rapidly reproducing the flat-topped average intensity distribution. The axial propagation distance required to reproduce the flat-topped average intensity distribution is very small (it was only 0.1 mm in our calculation). When the flat-topped average intensity is reproduced, the degree of polarization distribution is stable, and the variation range of the degree of polarization is also small. The results obtained here are of great significance for the potential application of a partially coherent circular flattened Gaussian vortex beam in medical imaging and medical diagnosis.