An Enhanced Flume Testing Procedure for the Study of Rill Erosion
Abstract
1. Introduction
2. Materials and Methods
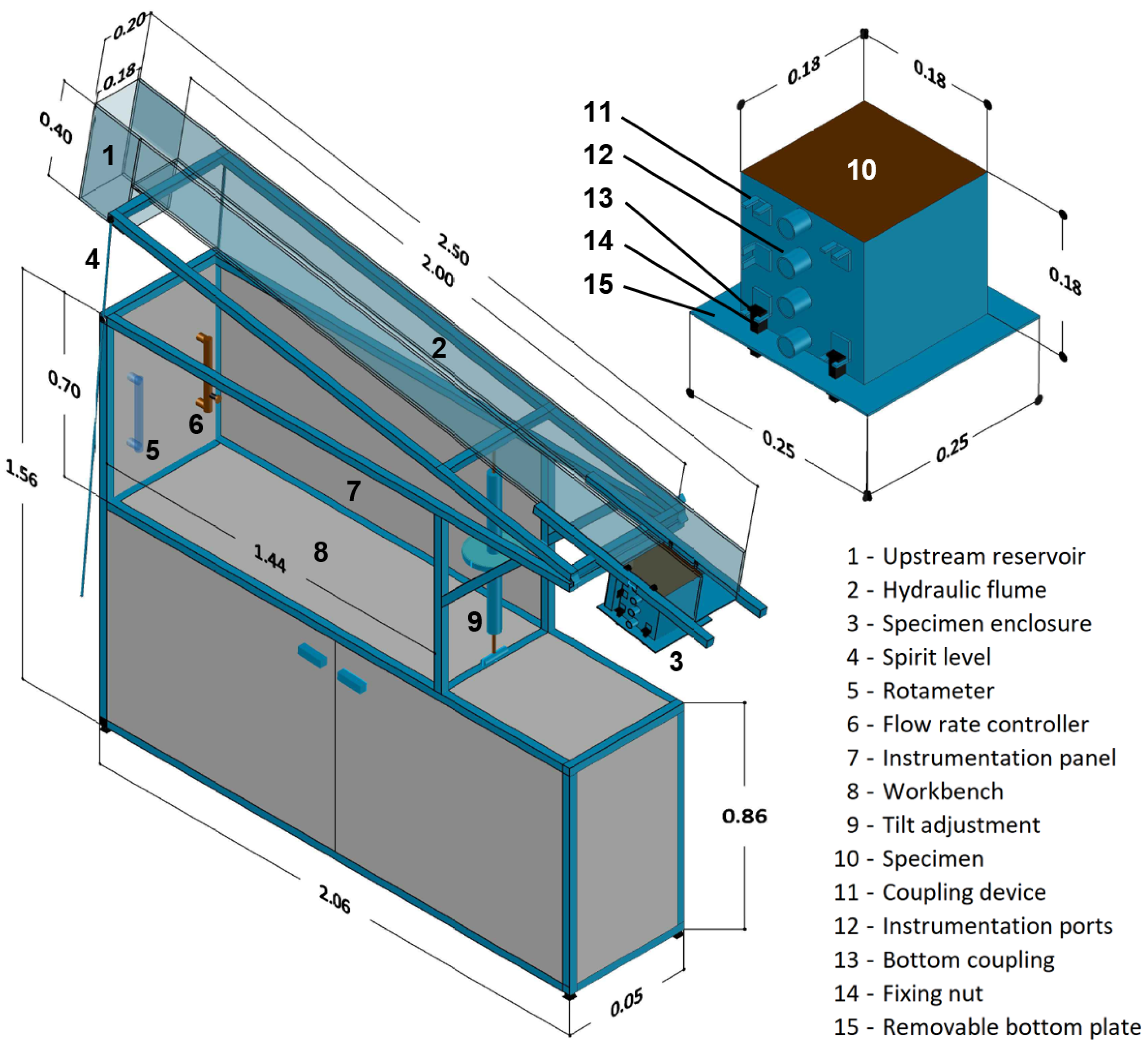
2.1. General Apparatus Characteristics and Hydraulic Design
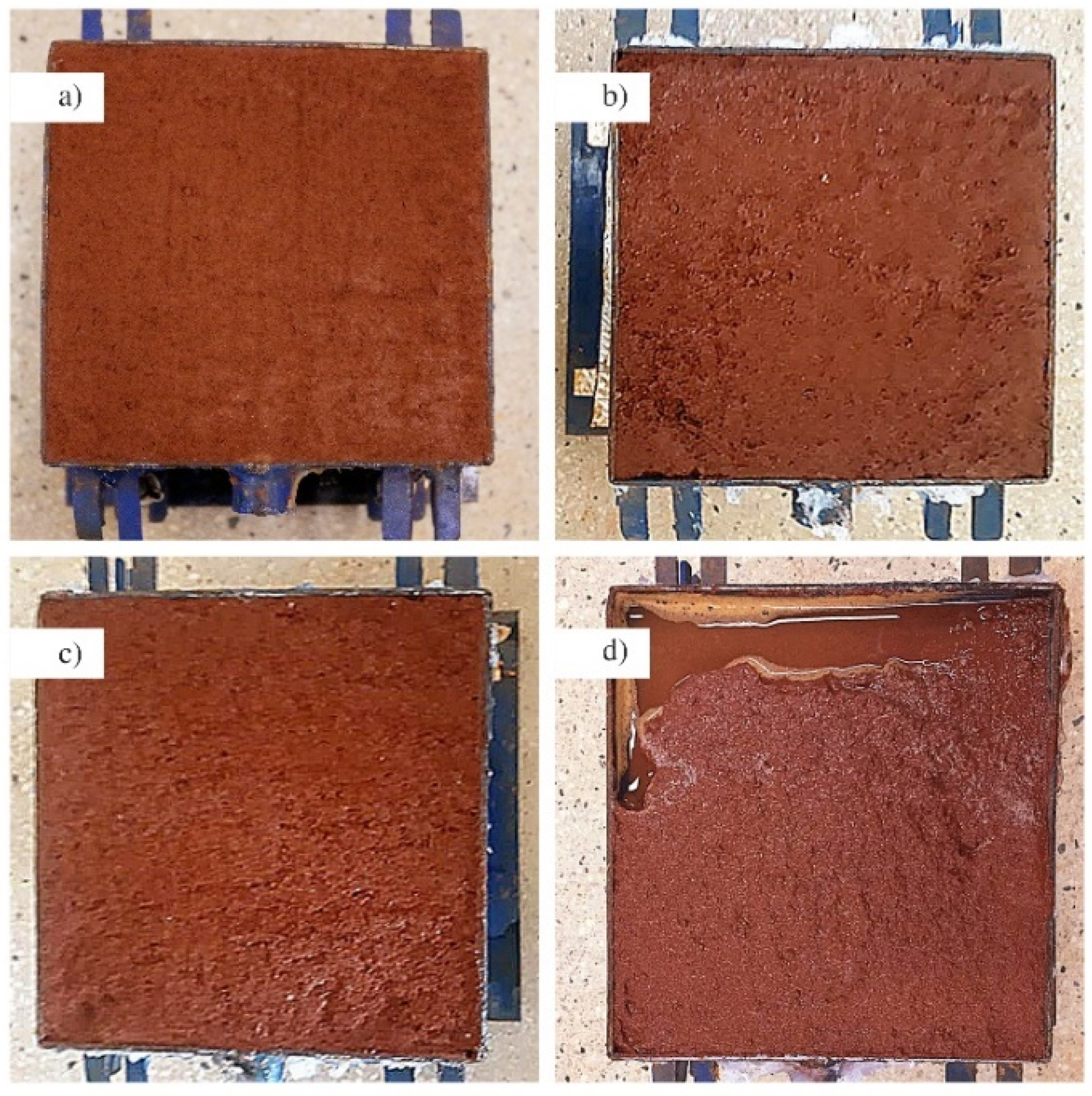
2.2. Soil Characteristics and Specimen Preparation for Flume Tests
2.3. Flume Testing Procedure
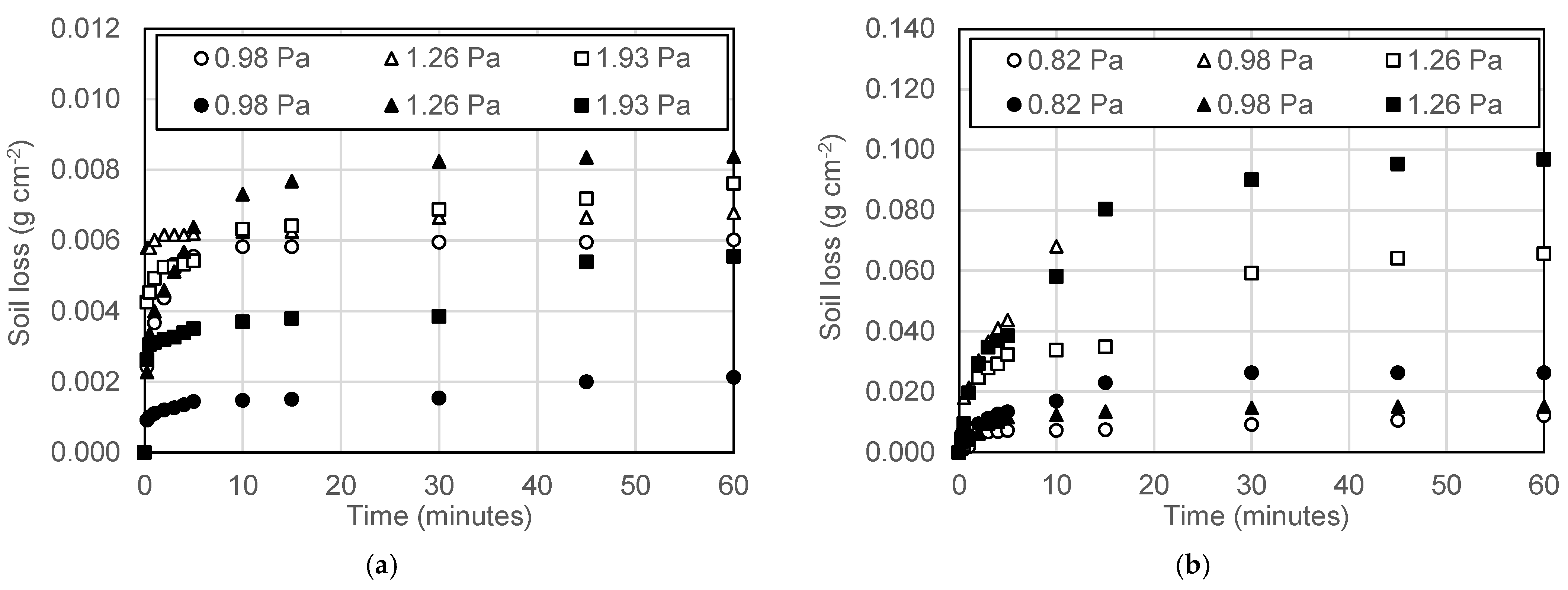
2.4. Flume Testing Program
- Out of the 31 specimens, a total of 16 specimens were compacted at a void ratio of 1.5. The remaining 15 specimens were compacted with a void ratio of 1.0;
- Out of the 31 specimens, 12 were used to assess the repeatability of the tests performed with the new apparatus. This was accomplished by testing six pairs of specimens under identical flow conditions;
- Five different flow conditions were used to analyze the influence of the hydraulic shear stress on the erosion curves.
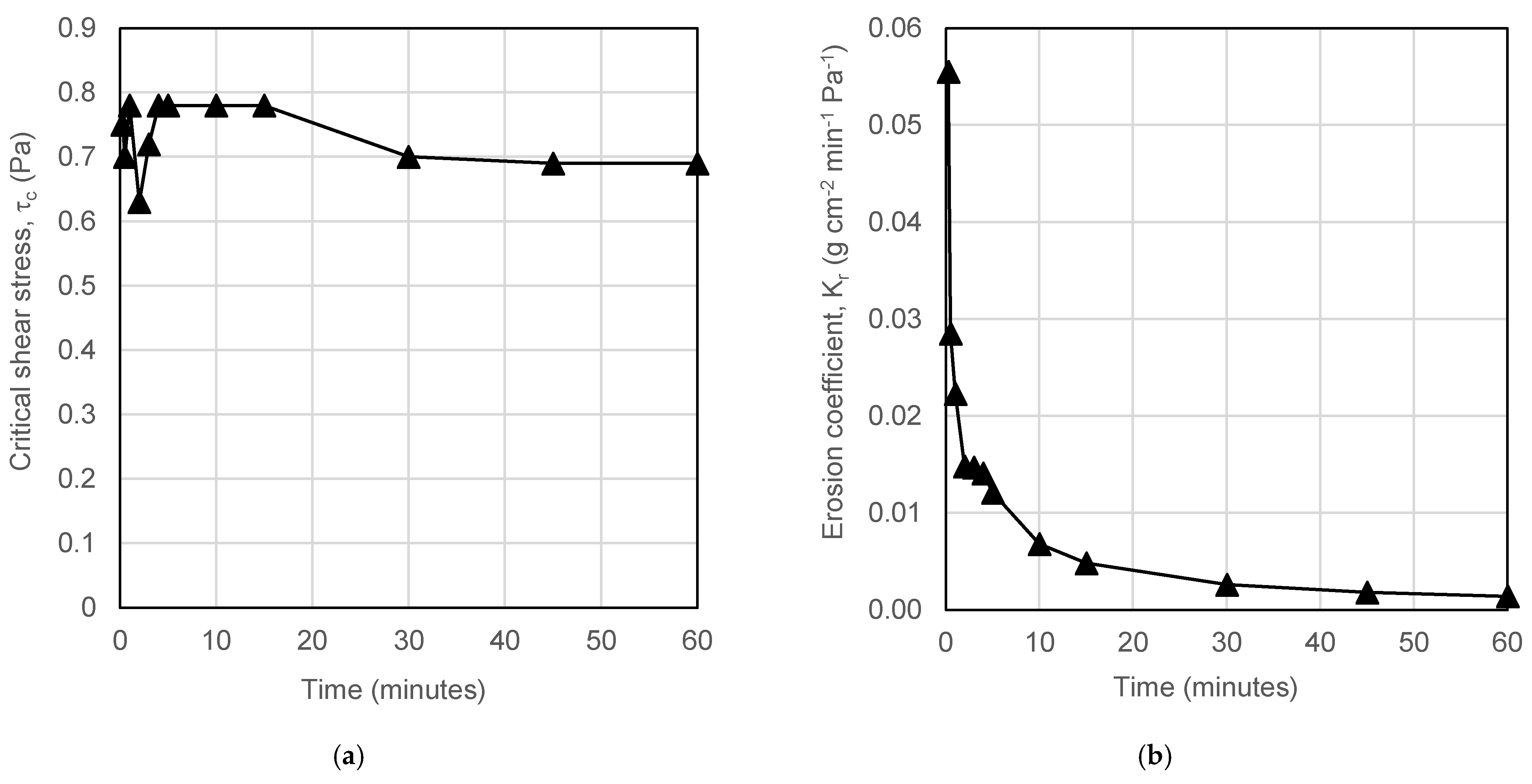
3. Results and Discussion
4. Final Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Julian, J.P.; Torres, R. Hydraulic Erosion of Cohesive Riverbanks. Geomorphology 2006, 76, 193–206. [Google Scholar] [CrossRef]
- Meng, X.M.; Jia, Y.G.; Shan, H.X.; Yang, Z.N.; Zheng, J.W. An Experimental Study on Erodibility of Intertidal Sediments in the Yellow River Delta. Int. J. Sediment Res. 2012, 27, 240–249. [Google Scholar] [CrossRef]
- Rinaldi, M.; Mengoni, B.; Luppi, L.; Darby, S.E.; Mosselman, E. Numerical Simulation of Hydrodynamics and Bank Erosion in a River Bend. Water Resour. Res. 2008, 44. [Google Scholar] [CrossRef]
- Kimiaghalam, N.; Goharrokhi, M.; Clark, S.P.; Ahmari, H. A Comprehensive Fluvial Geomorphology Study of Riverbank Erosion on the Red River in Winnipeg, Manitoba, Canada. J. Hydrol. 2015, 529, 1488–1498. [Google Scholar] [CrossRef]
- Foster, G.R. Modeling the Erosion Process. In Hydrologic Modeling of Small Watersheds, 1st ed.; Haan, C.T., Johnson, H.P., Brakensiek, D.L., Eds.; American Society of Agricultural Engineers: St. Joseph, MI, USA, 1982; pp. 297–380. [Google Scholar]
- Baigorria, G.A.; Romero, C.C. Assessment of Erosion Hotspots in a Watershed: Integrating the WEPP Model and GIS in a Case Study in the Peruvian Andes. Environ. Model. Softw. 2007, 22, 1175–1183. [Google Scholar] [CrossRef]
- Pandey, A.; Chowdary, V.M.; Mal, B.C.; Billib, M. Runoff and Sediment Yield Modeling from a Small Agricultural Watershed in India Using the WEPP Model. J. Hydrol. 2008, 348, 305–319. [Google Scholar] [CrossRef]
- Dey, S. Fluvial Hydrodynamics—Hydrodynamic and Sediment Transport Phenomena, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2014; 687p. [Google Scholar]
- Shen, N.; Wang, Z.; Wang, S. Flume Experiment to Verify WEPP Rill Erosion Equation Performances Using Loess Material. J. Soils Sediments 2016, 16, 2275–2285. [Google Scholar] [CrossRef]
- Pandey, A.; Himanshu, S.K.; Mishra, S.K.; Singh, V.P. Physically Based Soil Erosion and Sediment Yield Models Revisited. Catena 2016, 147, 595–620. [Google Scholar] [CrossRef]
- Du Boys, M.P. Le rhone et les rivieres a lit affouillable. Ann. Ponts Chaussés 1879, 18, 141–195. [Google Scholar]
- Partheniades, E. Erosion and deposition of cohesive soils. J. Hydraul. Hydraul. Eng. 1965, 91, 105–138. [Google Scholar]
- Nearing, M.A.; Deer-Ascough, L.; Laflen, J.M. Sensitivity Analysis of the WEPP Hillslope Profile Erosion Model. Trans. ASAE 1990, 33, 839–849. [Google Scholar] [CrossRef]
- Zhang, X.C.; Nearing, M.A.; Risse, L.M.; McGregor, K.C. Evaluation of WEPP Runoff and Soil Loss Predictions Using Natural Runoff Plot Data. Trans. ASAE 1996, 39, 855–863. [Google Scholar] [CrossRef]
- Bastos, C.A.B. Estudo Geotécnico Sobre a Erodibilidade de Solos Residuais não Saturados. Ph.D. Thesis, Universidade Federal do Rio Grande do Sul, Porto Alegre, Brazil, 1999. [Google Scholar]
- Kimiaghalam, N.; Clark, S.P.; Ahmari, H. An Experimental Study on the Effects of Physical, Mechanical, and Electrochemical Properties of Natural Cohesive Soils on Critical Shear Stress and Erosion Rate. Int. J. Sediment Res. 2016, 31, 1–15. [Google Scholar] [CrossRef]
- Nearing, M.A.; West, L.T.; Brown, L.C. A Consolidation Model for Estimating Changes in Rill Erodibility. Trans. ASAE 1988, 31, 0696–0700. [Google Scholar] [CrossRef]
- Sanford, L.P.; Maa, J.P.Y. A Unified Erosion Formulation for Fine Sediments. Mar. Geol. 2001, 179, 9–23. [Google Scholar] [CrossRef]
- Khanal, A.; Klavon, K.R.; Fox, G.A.; Daly, E.R. Comparison of Linear and Nonlinear Models for Cohesive Sediment Detachment: Rill Erosion, Hole Erosion Test, and Streambank Erosion Studies. J. Hydraul. Eng. 2016, 142, 04016026. [Google Scholar] [CrossRef]
- Wardinski, K.M.; Guertault, L.; Fox, G.A.; Castro-Bolinaga, C.F. Suitability of a Linear Model for Predicting Cohesive Soil Detachment during Jet Erosion Tests. J. Hydrol. Eng. 2018, 23, 06018004. [Google Scholar] [CrossRef]
- Gao, X.; Wang, Q.; Ma, G. Experimental Investigation on the Erosion Threshold and Rate of Gravel and Silty Clay Mixtures. Trans. ASABE 2019, 62, 867–875. [Google Scholar] [CrossRef]
- Khanal, A.; Fox, G.A.; Guertault, L. Soil Moisture Impacts Linear and Nonlinear Erodibility Parameters from Jet Erosion Tests. Trans. ASABE 2020, 63, 1123–1131. [Google Scholar] [CrossRef]
- Lane, L.J.; Foster, G.R.; Nicks, A.D. Use of fundamental erosion mechanics in erosion prediction. Am. Soc. Agric. Eng. 1987, 11. Available online: https://agris.fao.org/agris-search/search.do?recordID=US8843443 (accessed on 20 September 2021).
- Lim, S.S.; Khalili, N. An improved rotating cylinder test design for laboratory measurement of erosion in clayey soils. Geotech. Test. J. 2018, 32, 1–7. [Google Scholar] [CrossRef]
- Lyle, W.M.; Smerdon, E.T. Relation of Compaction and Other Soil Properties to Erosion Resistance of Soils. Trans. ASAE 1965, 8, 419–422. [Google Scholar] [CrossRef]
- Zhang, H.Y.; Li, M.; Wells, R.R.; Liu, Q.J. Effect of Soil Water Content on Soil Detachment Capacity for Coarse- and Fine-Grained Soils. Soil Sci. Soc. Am. J. 2019, 83, 697–706. [Google Scholar] [CrossRef]
- Nearing, M.A.; Parker, S.C. Detachment of Soil by Flowing Water Under Turbulent and Laminar Conditions. Soil Sci. Soc. Am. J. 1994, 58, 1612. [Google Scholar] [CrossRef]
- Debnath, K.; Nikora, V.; Aberle, J.; Westrich, B.; Muste, M. Erosion of Cohesive Sediments: Resuspension, Bed Load, and Erosion Patterns from Field Experiments. J. Hydraul. Eng. 2007, 133, 508–520. [Google Scholar] [CrossRef]
- Tolhurst, T.J.; Black, K.S.; Paterson, D.M.; Mitchener, H.J.; Termaat, G.R.; Shayler, S.A. A Comparison and Measurement Standardisation of Four in Situ Devices for Determining the Erosion Shear Stress of Intertidal Sediments. Cont. Shelf Res. 2000, 20, 1397–1418. [Google Scholar] [CrossRef]
- Ghebreiyessus, Y.T.; Gantzer, C.J.; Alberts, E.E.; Lentz, R.W. Soil Erosion by Concentrated Flow: Shear Stress and Bulk Density. Trans. ASAE 1994, 37, 1791–1797. [Google Scholar] [CrossRef]
- Kamphuis, J.W.; Gaskin, P.N.; Hoogendoorn, E. Erosion Tests on Four Intact Ontario Clays. Can. Geotech. J. 1990, 27, 692–696. [Google Scholar] [CrossRef]
- Zhu, J.C.; Gantzer, C.J.; Peyton, R.L.; Alberts, E.E.; Anderson, S.H. Simulated Small-Channel Bed Scour and Head Cut Erosion Rates Compared. Soil Sci. Soc. Am. J. 1995, 59, 211–218. [Google Scholar] [CrossRef]
- Cantalice, J.R.B.; Cassol, E.A.; Reichert, J.M.; Borges, A.L.O. Hidráulica do escoamento e transporte de sedimentos em sulcos em solo franco-argilo-arenoso. Rev. Bras. Ciênc. Solo 2005, 29, 597–607. [Google Scholar] [CrossRef]
- Shepard, D.M.; Bloomquist, D.; Henderson, M.; Kerr, K.; Trammell, M.; Marin, J.; Slagle, P. Design and Construction of Apparatus for Measuring Rate of Water Erosion of Sediments, 1st ed.; The National Academies of Science, Engineering, Medicine: Washington, DC, USA, 2005; 66p. [Google Scholar]
- Ni, S.; Zhang, D.; Wen, H.; Cai, C.; Wilson, G.V.; Wang, J. Erosion Processes and Features for a Coarse-Textured Soil with Different Horizons: A Laboratory Simulation. J. Soils Sediments 2020, 20, 2997–3012. [Google Scholar] [CrossRef]
- Smerdon, E.T.; Beasley, R.P. Critical Tractive Forces in Cohesive Soils. J. Agric. Eng. 1961, 42, 26–29. [Google Scholar]
- Shaikh, A.; Abt, S.R. Erosion Rate of Compacted NA-Montmorillonite Soils. J. Geotech. Eng. 1998, 114, 10. [Google Scholar] [CrossRef]
- Ting, F.C.K.; Briaud, J.-L.; Chen, H.C.; Gudavalli, R.; Perugu, S.; Wei, G. Flume Tests for Scour in Clay at Circular Piers. J. Hydraul. Eng. 2001, 127, 969–978. [Google Scholar] [CrossRef]
- Ravisangar, V.; Dennett, K.E.; Sturm, T.W.; Amirtharajah, A. Effect of Sediment PH on Resuspension of Kaolinite Sediments. J. Environ. Eng. 2001, 127, 531–538. [Google Scholar] [CrossRef]
- Barry, K.M.; Thieke, R.J.; Mehta, A.J. Quasi-Hydrodynamic Lubrication Effect of Clay Particles on Sand Grain Erosion. Estuar. Coast. Shelf Sci. 2006, 67, 161–169. [Google Scholar] [CrossRef]
- Koetz, M.; Pruski, F.F.; Mehl, H.U.; Silva, D.D.; Marques, E.A.G. Métodos Para a Determinação da Erodibilidade e da Tensão Crítica de Cisalhamento do Solo em Estradas não Pavimentadas. Rev. Eng. Agric. 2009, 17, 110–119. [Google Scholar] [CrossRef]
- Almeida, J.G.R.; Romão, P.A.; Mascarenha, M.M.A.; Sales, M.M. Erodibilidade de solos tropicais não saturados nos municípios de Senador Canedo e Bonfinópolis (GO). Geociências 2015, 34, 441–451. [Google Scholar]
- Wang, Y.-C.; Sturm, T.W. Effects of Physical Properties on Erosional and Yield Strengths of Fine-Grained Sediments. J. Hydraul. Eng. 2016, 142, 04016049. [Google Scholar] [CrossRef]
- Munson, B.R.; Rothmayer, A.P.; Okiishi, T.H.; Huebsch, W.W. Fundamentals of Fluid Mechanics, 7th ed.; Wiley: Hoboken, NJ, USA, 2012; p. 792. [Google Scholar]
- Vatankhah, A.R. Explicit Solutions for Critical and Normal Depths in Trapezoidal and Parabolic Open Channels. Ain Shams Eng. J. 2013, 4, 17–23. [Google Scholar] [CrossRef][Green Version]
- Martins, J.R.R.A.; Sturdza, P.; Alonso, J.J. The Complex-Step Derivative Approximation. ACM Trans. Math. Softw. 2003, 29, 245–262. [Google Scholar] [CrossRef]
- Christopher, B.R.; Holtz, R.D. Geotextile Engineering Manual—Report FHWA-TS-86/203, 1st ed.; The National Academies of Science, Engineering, Medicine: Washington, DC, USA, 1985; p. 1044. [Google Scholar]
- Nascimento, R.O.; Oliveira, L.M.; Mascarenha, M.M.A.; Angelim, R.R.; Oliveira, R.B.; Sales, M.M.; Luz, M.P. Uso de solução de cal para mitigação de processos erosivos em um solo da UHE de Itumbiara. Geociências 2019, 38, 279–295. [Google Scholar] [CrossRef]
- Basso, L. Estudo da Erodibilidade de Solos e Rochas Sedimentares de Uma Voçoroca na Cidade de São Francisco de Assis—RS. Master’s Thesis, Universidade Federal de Santa Maria, Santa Maria, Brazil, 2013. (In Portuguese). [Google Scholar]
- Grimaldi, S.; Nardi, F.; Piscopia, R.; Petroselli, A.; Apollonio, C. Continuous Hydrologic Modelling for Design Simulation in Small and Ungauged Basins: A Step Forward and Some Tests for its Practical Use. J. Hydrol. 2021, 595, 125664. [Google Scholar] [CrossRef]
- Apollonio, C.; Petroselli, A.; Tauro, F.; Cecconi, M.; Biscarini, C.; Zarotti, C.; Grimaldi, S. Hillslope Erosion Mitigation: An Experimental Proof of a Nature-Based Solution. Sustainability 2021, 13, 6058. [Google Scholar] [CrossRef]
- Stanchi, S.; Zecca, O.; Hudek, C.; Pintaldi, E.; Viglietti, D.; D’Amico, M.E.; Colombo, N.; Goslino, D.; Letey, M.; Freppaz, M. Effect of Soil Management on Erosion in Mountain Vineyards (N-W Italy). Sustainability 2021, 13, 1991. [Google Scholar] [CrossRef]
- Mohamadi, M.A.; Kavian, A. Effects of Rainfall Patterns on Runoff and Soil Erosion in Field Plots. Int. Soil Water Conserv. Res. 2015, 3, 273–281. [Google Scholar] [CrossRef]
- Mendes, T.A.; Pereira, S.A.d.S.; Rebolledo, J.F.R.; Gitirana, G.d.F.N., Jr.; Melo, M.T.d.S.; Luz, M.P.d. Development of a Rainfall and Runoff Simulator for Performing Hydrological and Geotechnical Tests. Sustainability 2021, 13, 3060. [Google Scholar] [CrossRef]
- Mendes, T.A.; Alves, R.D.; Gitirana, G.d.F.N., Jr.; Pereira, S.A.d.S.; Rebolledo, J.F.R.; da Luz, M.P. Evaluation of Rainfall Interception by Vegetation Using a Rainfall Simulator. Sustainability 2021, 13, 5082. [Google Scholar] [CrossRef]


| Void Ratio | Test | τh (Pa) | Dc(×10−2) (g cm−2 mim−1) | (g cm−2) | RMSE (×10−4) | R2 |
|---|---|---|---|---|---|---|
| 1.000 | 1 | 0.98 | 0.81 | 0.14 | 0.73 | 0.931 |
| 2 | 0.98 | 1.25 | 0.60 | 2.52 | 0.950 | |
| 3 | 1.26 | 1.00 | 0.70 | 4.46 | 0.940 | |
| 4 | 1.26 | 2.85 | 0.62 | 0.66 | 0.937 | |
| 5 | 1.93 | 4.03 | 0.35 | 1.14 | 0.972 | |
| 6 | 1.93 | 5.55 | 0.55 | 1.20 | 0.984 | |
| 1.500 | 7 | 0.82 | 0.89 | 2.01 | 6.27 | 0.993 |
| 8 | 0.82 | 0.52 | 0.97 | 8.78 | 0.912 | |
| 9 | 0.98 | 0.83 | 1.48 | 9.24 | 0.942 | |
| 10 | 0.98 | 4.00 | 5.43 | 15.9 | 0.999 | |
| 11 | 1.26 | 2.85 | 5.54 | 12.5 | 0.998 | |
| 12 | 1.26 | 2.20 | 4.65 | 17.6 | 0.997 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
de Oliveira, V.N.; Gitirana, G.d.F.N., Jr.; dos Anjos Mascarenha, M.M.; Sales, M.M.; Varrone, L.F.R.; da Luz, M.P. An Enhanced Flume Testing Procedure for the Study of Rill Erosion. Water 2021, 13, 2956. https://doi.org/10.3390/w13212956
de Oliveira VN, Gitirana GdFN Jr., dos Anjos Mascarenha MM, Sales MM, Varrone LFR, da Luz MP. An Enhanced Flume Testing Procedure for the Study of Rill Erosion. Water. 2021; 13(21):2956. https://doi.org/10.3390/w13212956
Chicago/Turabian Stylede Oliveira, Vinícius Naves, Gilson de F. N. Gitirana, Jr., Marcia Maria dos Anjos Mascarenha, Mauricio Martines Sales, Luiz Felipe Ramos Varrone, and Marta Pereira da Luz. 2021. "An Enhanced Flume Testing Procedure for the Study of Rill Erosion" Water 13, no. 21: 2956. https://doi.org/10.3390/w13212956
APA Stylede Oliveira, V. N., Gitirana, G. d. F. N., Jr., dos Anjos Mascarenha, M. M., Sales, M. M., Varrone, L. F. R., & da Luz, M. P. (2021). An Enhanced Flume Testing Procedure for the Study of Rill Erosion. Water, 13(21), 2956. https://doi.org/10.3390/w13212956







