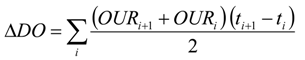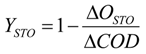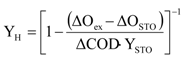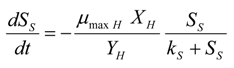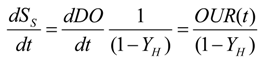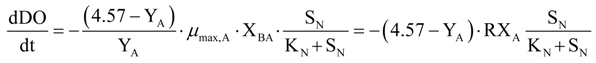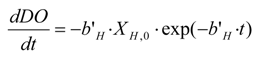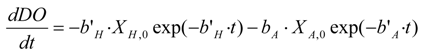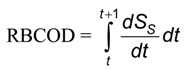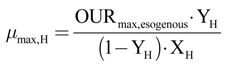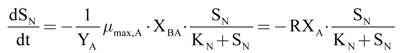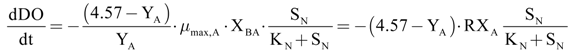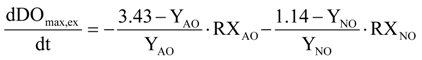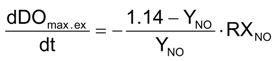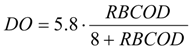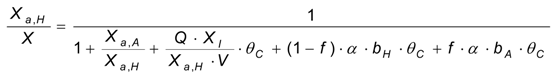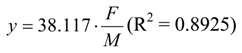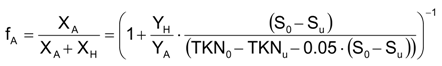1. Introduction
The determination of kinetic and stoichiometric parameters, as well as sewage sludge and water inflow characterization has assumed a fundamental role in the last decade for optimized planning [
1], modelling [
2,
3], design [
4], management [
5] and upgrading [
6,
7,
8] of the biological stage in a wastewater treatment plant. However, the history of respirometry is older than a decade: An important review of the popularity of respirometry explained by its historical impact and its sensitivity and robustness has been available since 15 years ago [
9]. This demonstrates that respirometry has a farther origin, evolving in parallel with the development of process modeling. Indeed, in 1983, the International Association on Water Quality organized a task group to promote the development and application of practical models for the design and operation of biological wastewater treatment plants [
10]. The result of the work was presented in 1987: Today, the resulting model is named Activated Sludge Model No 1 (ASM1); many basic concepts were adapted from an earlier model called the University of Cape Town (UCT) model [
10], demonstrating that the sector evolved significantly since early in the 1980s.
Stoichiometric parameters can often assume values that are variable in a restricted range [
11]. On the contrary, some parameters, such as growth and cellular decay velocities, can significantly change from one plant to another plant, particularly for urban-industrial mixed wastewater [
11]. In this context, respirometry becomes an essential technique suitable for determining both the biodegradability and inhibitory effects on bacterial biomass [
12].
The present research has been developed with the aim of both optimizing and upgrading the performance of an analyzed plant, which is negatively affected by both an insufficient treatment municipal capacity and plant undersizing or malfunctioning together with an incomplete collection system (sewage network). Respirometric techniques have been selected to measure data useful not only for the present case study, but also as a contribution to updating the knowledge of the kinetic sector. The application of respirometry is definitely not new. However, reproducing specific assessments on the subject could be doubly useful. The first important and useful aspect was purely scientific and consisted of checking, or better, updating, which researchers have not done in recent times, but with a different and likely slurry, with plants that have undergone several upgrades over the course of time. The second aspect is related to the aim of the research performed, which was to make a contribution toward verifying the reliability of the values, commonly used for a long time, for some kinetic parameters.
2. Materials and Methods
The experimentation presented in this paper concerns a series of tests carried out in a major wastewater treatment plant (WWTP) located in Italy (Olgiate-Olona Municipality, Varese Province), with particular reference to the biological stage (oxidation and nitrification) operation. This plant has operated since 2002 and is composed of gridding, sand-oil removal, primary sedimentation, oxidation in an activated sludge reactor, nitrification, secondary sedimentation and disinfection (by the sludge line with pre-thickening, anaerobic digestion and dehydration). In 2007, two original treatment lines were upgraded with a new, third one. At present, the plant serves seven municipalities with a total population equivalent of 175,000 inhabitants and a significant contribution from industrial activities (around 30% of the total inflow) [
13,
14]. The plant operates by means of a typical modified Ludzack–Ettinger pre-denitrification process [
15,
16,
17,
18], and some layout modifications are under study, also considering the application of new technologies in the future [
19].
The analyzed kinetic parameters, regarding both heterotrophic and autotrophic biomass, were: Cellular yield coefficients, decay rate, maximum growth rate and the substrate removal rate.
Biomass was examined through the application of selected respirometric tests [
12,
19,
20].
Activated sludge and wastewater were collected at the full-scale plant: The sludge and wastewater were sampled, respectively, into an oxidation-nitrification tank and at the outlet of the primary settling tank. Both wastewaters and sludge have been characterized as a function of total suspended solids (TSS), COD and nitrogen content following a procedure described by Dircks
et al. [
21]. COD has been measured in the filtered sample after the use of a 0.45-μm membrane.
Before each test, the sludge was added into a 1-L reactor and aerated until the dissolved oxygen (DO) concentration approached the saturation value to achieve endogenous respiration conditions. The reactor was located in a lab room, where the temperature was maintained at a controlled value of 18°C. All tests were carried out by adding a volume of 0.5 L of sludge. The solution used as the substrate was then added in a known volume after the sludge aeration stoppage. At the start of operation, the sludge concentration was operated in the range of 2.0–3.5 g SST L−1.
DO data were recorded until a concentration close to 2 mg L−1 was reached. Each test was repeated 5–6 times. DO was measured with an Oxi 330 equipped with a CellOx 325.
In order to analyze the heterotrophic bacteria kinetics of growth, sodium acetate was used as a carbonaceous substrate, adding an equivalent mass of 2 g COD L−1 or an equivalent rate of 10 mgCOD L−1 of sludge. Considering that one of the aims of the research was to make a contribution to verify the reliability of values commonly used for long time for some kinetic parameters, thus, for a comparison with the present situation, it was reasonable to use sodium acetate, even if the respirometric sector is evolving towards additional methods.
Then, 50 mL of such a solution was added into the reactor. Low values of substrate and biomass concentrations (F/M (food to microorganism)) were maintained during the test, approximately 0.05 mg COD mg−1 VSS (volatile suspended solids). Allylthiourea (ATU, 10–20 mg ATU L−1) was added into the sludge in order to inhibit autotrophic bacteria. Ammonium chloride (NH4Cl) and sodium nitrite (NaNO2) were used as the nitrogen substrate (for the study of the kinetics of autotrophic bacteria).
The recorded data of the oxygen uptake rate (OUR) were plotted as a function of time. Substrate consumption, operated by biomass, was represented by a primary curve segment, which is characterized by a higher slope; on the contrary, the final segment, characterized by a lower slope, represents DO consumption under endogenous conditions. Total DO consumption (
ΔDO) has been determined by calculating the area defined by the measured oxygen consumption curve using Equation (1):
The observed heterotrophic cellular yield coefficient,
YH,obs, can be defined by means of Equation (2) defined by Gujer
et al. [
22]:
where
YSTO is the yield of ready biodegradable substrate (
SS) and
YH corresponds to the heterotrophic cellular yield of the biomass produced (
XH) by the degradation of an internal storage product unit.
The conversion yield of ready biodegradable substrate and heterotrophic cellular yield have been determined by calculating
YSTO and
YH with Equations (3) and (4), as defined by Karahan-Gül
et al. [
23]:
where
ΔCOD is the organic load,
ΔOex is the oxygen consumption due to the added substrate and
ΔOSTO is the surface between the respirogram curve and the line connecting OUR and respirogram inflexion points.
ΔOex is calculated as the difference between the area subtended by the total respirogram graph and the integral of the endogenous respiration curve.
The internal storage polymers produced by substrate removal are calculated with Equation (5):
In order to describe the trends of DO and RBCOD (readily biodegradable) consumption rates, it is necessary to write ordinary differential Equations (6) and (7) (independent variable: time):
The relationship between the two equations is described by Equation (8):
Activated sludge models proposed by IWA [
22] describe the nitrification process as a function of ammonia conversion directly to nitric nitrogen, without considering the intermediate nitrite state [
24]. Usually, this assumption can be considered acceptable in modeling, the conversion being very fast. A different approach is required whenever it is necessary to achieve very low limits for nitrites or there is: (a) an excessive accumulation of nitrites in the system; (b) an insufficient oxidation volume or (c) a limited nitrification capacity.
Equation (9) describes the oxidation of ammonia to nitrite; Equation (10) represents the corresponding DO consumption.
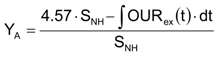
The autotrophic biomass yield coefficient,
YA, has been calculated from the experimental respirogram using Equation (11) [
12]:
while the heterotrophic cellular decay rate in endogenous respiration,
bH, can be expressed with Equation (12) [
25]:
The parameter,
bH, is connected with
b’H , introduced to describe all the processes responsible for energy consumption and biomass reduction and not associated with cellular growth (decay, lysis,
etc.) [
22]. The cellular decay rate,
b’H, is represented by the slope of the line that interpolates the experimental points plotted on a semi-logarithmic graph of OUR
max,ex [
12].
The autotroph cellular decay rate,
bA, has been determined by arranging the experimental results registered on the respirogram. The result obtained at test temperature T* has been referenced to a temperature of 20 °C using Equation (13) where α is equal to 1.07:
For each respirometric test, the DO consumption velocity corresponding to the absence of the exogenous substrate has been determined by means of the following equation:
The active heterotrophic biomass concentration has been computed using the least-squares method applied to the difference between the theoretical and experimental curves. The concentration has been assumed to be reasonably constant (XH,0 = XH), because of the limited mass of the added substrate.
The autotrophic biomass concentration,
XA, has been determined through the use of Equation (15) and minimizing the sum of squared differences between experimental and theoretical curves:
The autotrophic biomass content,
fA, has been determined according to Equation (16) [
9]:
The Michaelis–Menten curve expressing the relation between the consumption rate of a rapidly biodegradable substrate,
S(t), and its concentration within the activated sludge sample (
SS) has been used to determine the
μmax,H and
ks values [
24,
26]. The substrate removal rate as a function of time has been calculated from the experimental respirogram using Equation (17):
The concentration of rapidly biodegradable substrate,
S(t), has been calculated from the amount of sodium acetate added at the beginning by subtracting the correspondent quantity removed during each time interval. The Michaelis–Menten curve has been drawn by considering the RBCOD consumption rate data as a function of the corresponding
S(t) values.
μmax,H and
ks parameters have been calculated by minimizing the sum of the squares of the differences between the experimental and theoretical curves. Equation (18) has been used to calculate the maximum growth rate from experimental data [
9]:
The experimental data interpolation following a Michaelis–Menten curve is calculated by the use of Equations (19) and (20):
The autotroph maximum growth rate (µmax,A) has been calculated, with Equation (21), as a function of the DO consumption maximum velocity, due to the oxidation of the added ammoniacal nitrogen.
The experimental results regarding the parameters describing the conversion kinetics of ammoniacal nitrogen in nitrates have been corrected with the aim of considering a possible temperature influence. According to this feature, Equation (22) has been used (with α = 1.07):
Taking into account the hypothesis that the quantity of the added substrate during the tests was high enough to reach the maximum conversion rate, the increase of the oxygen uptake has been considered proportional to both maximum growth and biomass concentration. The parameters,
RXAO and
RXNO, represent the product between the maximum growth rate and active biomass concentration, respectively, for ammonium (Equation (23)) and nitrite oxidizing bacteria (Equation (24)).
Equations (25) and (26) have been used for the conversion from ammonia to nitrate and from nitrite to nitrate:
3. Results and Discussion
The more recent modeling approach [
22] classifies COD as a function of the organic substrate biodegradability by determining the following fractions: Particulate organic matter as the sum of inert particulates, slowly biodegradable substrates, internal storage polymer, heterotrophic and autotrophic biomass and soluble organic matter as the sum of the soluble inert compounds and rapidly biodegradable organic substrates. The use of filtration in order to characterize COD implies the consequence of a significant fraction of slowly biodegradable substrate passes into the filtrate, increasing the soluble content. In such a way, RBCOD increases, with respect to the total amount, up to 40% [
22]. With respect to this concern, the results of influent wastewater COD gave a total COD equal to 316 mg COD L
−1 and an RBCOD equal to 126 mg COD L
−1, corresponding to a ratio between these two values equal to 40%. The sampled sludge was characterized by a 2–3 g TSS L
−1 content, according to the data reported in the technical literature [
1,
4,
23].
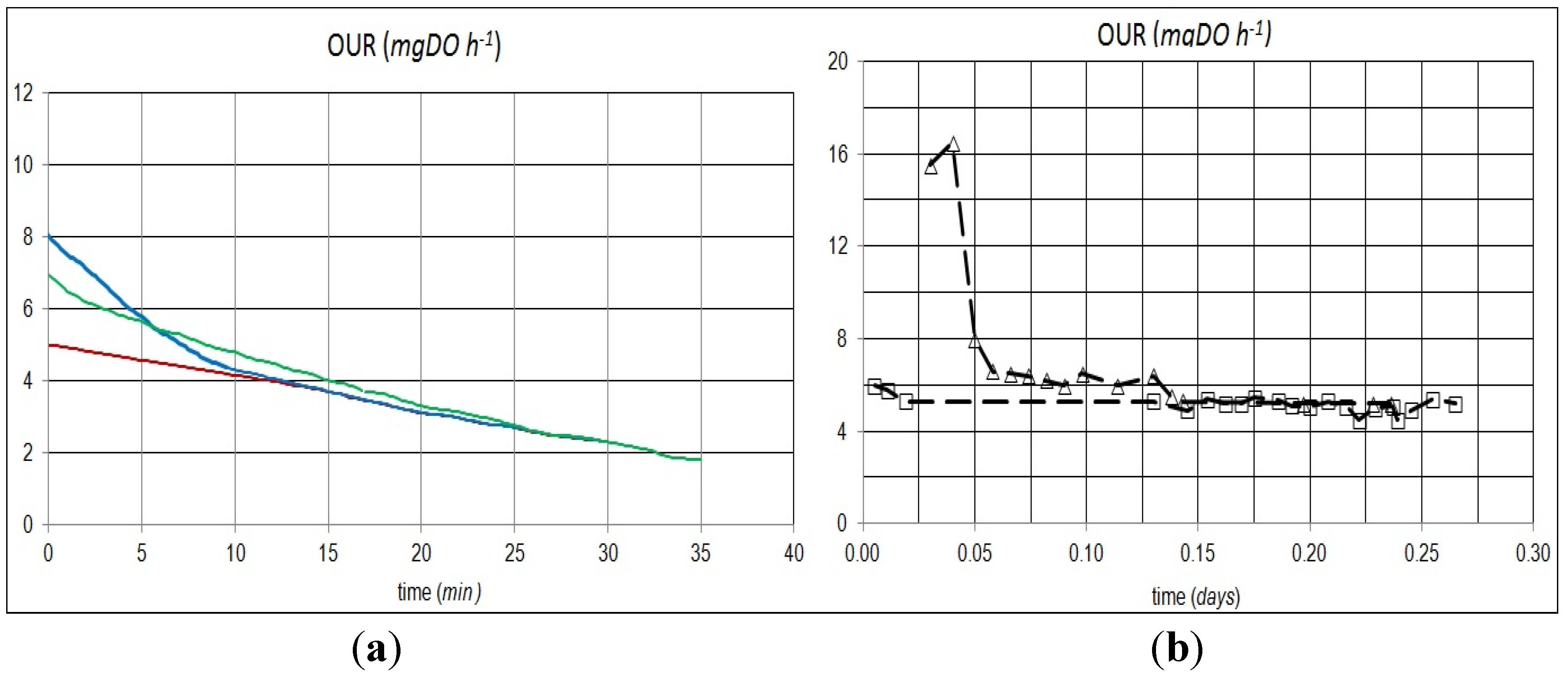
Experimental DO values (
Figure 1a), measured during the test carried out by adding 10 mg L
−1 of COD equivalent of sodium acetate and 50 mL of wastewater and providing endogenous respiration, were interpolated by a linear function with a good reproducibility (R
2 > 0.98). The slope of the interpolation lines representing the experimental data of DO uptake are, respectively, 0.394 and 0.173 mg DO h
−1, and the slopes of the endogenous processes were, respectively, 0.105, 0.107 and 0.094 mg DO h
−1.
The interpolation of all data collected during this last phase made possible the determination of the DO consumption with respect to the RBCOD addition. The absence of a clear change in slope can be probably justified by the limited presence of rapidly hydrolysable substrates with different oxidation rates. All data can be correlated each other with a linear and a Monod-type relationship, as indicated by Equations (27) and (28):
In the absence of an exogenous substrate, the trend of the respirogram represents a decay that can be described by a decreasing exponential function. Under these conditions, substrate addition causes an immediate increase in the oxygen consumption rate, which returns to values corresponding to endogenous conditions only when the feed (substrate) has been completely removed (
Figure 1b).
The trend of OUR values, obtained upon RBCOD addition, shows two different phases. During the first period (high OUR), sodium acetate removal occurs, and it is probable that RBCOD conversion takes place in the internal storage products [
19]. The second phase, in endogenous conditions after the complete removal of RBCOD, shows a different oxygen consumption rate because of the internal polymers used for the production of the new cellular biomass [
22].
The sequence of the presence and absence of an external substrate, in a “feast/famine” way, causes different microorganism reactions to occur (such as substrate adsorption and accumulation or storage [
24]). Variable environmental conditions, such as changes in the influent wastewater quality, which can affect nutrient availability, favor microorganisms that are able to store the substrate very quickly and use it a second time for a more balanced growth [
19]. In a modeling approach, this process is represented according to the hypothesis that all RBCOD is transformed into an internal product and, then, assimilated by heterotrophic bacteria [
22].
Figure 1.
Respirometric test curves: (a) the experimental dissolved oxygen (DO) concentration during the addition of 10 mg L−1 of COD equivalent of sodium acetate (blue curve), the addition of 50 mL of wastewater (green curve) and endogenous activity (brown curve); (b) the oxygen uptake rate in the degradation of an external substrate (triangles) and endogenous respiration (squares). (F/M (food to microorganism) = 0.01 mg COD mg−1 VSS (volatile suspended solids). OUR, oxygen uptake rate.
Figure 1.
Respirometric test curves: (a) the experimental dissolved oxygen (DO) concentration during the addition of 10 mg L−1 of COD equivalent of sodium acetate (blue curve), the addition of 50 mL of wastewater (green curve) and endogenous activity (brown curve); (b) the oxygen uptake rate in the degradation of an external substrate (triangles) and endogenous respiration (squares). (F/M (food to microorganism) = 0.01 mg COD mg−1 VSS (volatile suspended solids). OUR, oxygen uptake rate.
The cellular decay rate (heterotrophic biomass) has been measured through an interpolation of all the data reported in
Table 1 (
YH,OSS = 0.65 and
fP = 8%) (
Table 2 shows the nomenclature). The parameters were evaluated as
b’H = 0.1349 day
−1,
bH = 0.34 day
−1,
bA = 0.0561 day
−1 and
XH,0 = 865 mg COD L
−1. The literature reports heterotrophic cellular decay rate values of 0.06–0.15 day
−1 [
25] and b
H values (for industrial wastewater) in the range of 0.10–0.24 day
−1 (b’
H = 0.05–0.10 day
−1) [
12].
Moreover, respirometric test data concerning cellular yield coefficients are reported in
Table 1. The results agree well with the data from the literature [
19,
24,
27]. Active heterotrophic biomass concentration data are reported in the same
Table 1; values expressed as volatile solids were calculated by a conversion factor of COD/VSS = 1.48. The obtained values were lower than the volatile suspended solids observed in the laboratory via gravimetric measurements and in the range of 1750–2100 mg VSS L
−1.
The active heterotrophic biomass concentration, determined from the respirometric tests, presents a variable percentage in the range of 30%–37% of the total amount of organic matter in the activated ludge sample. The same result can be determined using a mass balance of the total organic matter and the active biomass and couching the ratio:
Table 1.
Heterotrophic biomass characterization.
Concerning the maximum growth rate, the observed data seem to highlight the slow kinetics of organic matter removal. In fact, the maximum growth rates of heterotrophic bacteria that have been found in the literature are in the range of 3.0–13.2 day
−1 [
25].
The substrate removal rate (
vmax,H), progressively increased as the ratio (F/M) increased, following a linear relation:
The technical literature does not provide univocal indications regarding the values of the F/M ratio to be adopted for respirometric tests. Many authors give importance to other parameters, because their values can provide useful indications for management at the real scale [
28].
Short tests conducted with small feeding variations, with low organic load, give generally good results compared to tests carried out with high values of organic load, which can cause the internal storage of polymers, and determine the increase of the microorganisms mass, making the interpretation of the experimental results difficult. During the respirometric tests, the highest values for organic matter removal do not correspond to the highest concentrations of RBCOD. This phenomenon can be explained by the hypothesis that the microorganisms are accustomed to the use of a different substrate and need time to acclimate according to different feeding conditions. A similar study showed that it is possible to obtain an immediate reaction from the microorganisms by repeatedly adding the substrate to the sludge [
28].
Cellular decay (autotrophic biomass) results lie in the range reported in the literature:
bA = 0.03–0.15 [
29]. Concerning the autotrophic biomass decay rate, values were arranged with a linear relation:
Utilizing data from six tests (time from zero to 240 hours and OURMAX,EXOGENOUS from 28.28 down to 16.45 mg DO L−1 h−1), the measured cellular decay was - = 0.0561 day−1 at a temperature of 26.5 °C and 0.0361 day−1 at 20 °C (estimated).
Concerning the yield coefficient, three respirometric tests have showed values of Y
A equal to 0.23–0.27 mg COD mg
−1 N; such results are very close to the typical value (0.24) proposed in the technical literature [
19].
The obtained result could have been characterized with a certain level of uncertainty, because the determination of autotrophic and heterotrophic biomass concentration has been performed as the same procedure. Autotrophic bacteria have been quantified as a fraction of the total amount of biomass in activated sludge (fA = 0.082), and the corresponding average value resulted as 77 mg COD L−1.
Concerning the maximum growth rate and semi-saturation constant, the experimental respirogram has been worked out for drawing the Michaelis–Menten curve and the related ammoniacal nitrogen removal rate as a function of concentration (
Figure 2a). We obtained similar results adopting the two different procedures utilized for data elaboration, confirming the opportunity to adopt both methods for the analysis of nitrification kinetics. The maximum growth rate of nitrifying biomass
(μmaxA) is in the range of 0.2–0.9 day
−1 [
29,
30], while the maximum conversion rate of ammoniacal ammonium in nitrates (
vmax,A) is generally assumed equal to 120–150 mg N g
−1 VSS h
−1.
Table 2.
Nomenclature.
| Symbol | Definition |
|---|
| DO | Dissolved oxygen (mg L−1) |
| ΔDO | Dissolved oxygen consumption (mg L−1) |
| OURi + 1 | Oxygen uptake rate at time i+1 (mg DO/L/h) |
| OURi | Oxygen uptake rate at time i (mg DO/L/h) |
| t I + 1 | time i +1 (minutes) |
| ti | time i (minutes) |
| YH,obs | Observed heterotrophic cellular yield coefficient (mgXH/mgSs) |
| YSTO | Yield of stored product per unit of Ss (mgXSTO/mgSs) |
| YH | Heterotrophic cellular yield (mgXH/mgXSTO) |
| ΔOSTO | Surface between the respirogram curve and the line connecting the OUR point and the respirogram inflexion point (mg DO L−1) |
| ΔOex | Oxygen consumption due to the added substrate (mg DO L−1) |
| ΔCOD | Organic load of the ready biodegradable substrate (mg COD L−1) |
| XSTO | Cell internal storage polymers (mg COD L−1) |
| RBCOD | Ready biodegradable substrate (mg COD L−1) |
| µmaxH | Maximum heterotrophic growth rate (day−1) |
| XH | Heterotrophic biomass (mg COD L−1) |
| XH,0 | Heterotrophic biomass at time t = 0 (mg COD L−1) |
| Xa,H | Active heterotrophic biomass concentration (mg COD L−1) |
| X | Total amount of organic matter in the activated sludge sample (mg COD L−1) |
| XL | Inert organic fraction in the liquid influent (mg COD L−1) |
| θC | Sludge age (day) |
| Ks | Semi-saturation constant (mg COD L−1) |
| SN; SNH | Ammonia concentration (mg N L−1) |
| YA | Autotrophic growth yield (mg COD biomass/mg N) |
| YAO | Ammonium oxidizers growth yield (mg COD biomass/mg N) |
| YNO | Nitrite oxidizers growth yield (mg COD biomass/mg N) |
| µmaxA | Maximum growth rate of nitrifying biomass (day−1) |
| KN; KNH | Semi-saturation constant (mg N L−1) |
| bH | Heterotrophic decay rate coefficient in endogenous respiration (day−1) |
| b’H | Observed heterotrophic decay rate coefficient in endogenous respiration (day−1) |
| fP | Endogen residue (dimensionless) |
| bA | Autotrophs decay rate coefficient (day−1) |
| T* | Test temperature (°C) |
| Α | Correction coefficient for temperature (dimensionless) |
| XA; XBA | Autotrophic biomass concentration (mg N L−1) |
| XA,0 | Autotrophic biomass concentration at time t = 0 (mg COD L−1) |
| Xa,A | Active autotrophic biomass concentration (mg COD L−1) |
| fA | Autotrophic biomass/total amount of biomass in activated sludge (-) |
| XAO | Ammonium oxidizer biomass concentration (mg N L−1) |
| XNO | Nitrite oxidizer biomass concentration (mg N L−1) |
| S0, Su | Ready biodegradable substrate, at time (mg COD L−1) |
| TKN0, TKNu | TKN, at time (mg N L−1) |
| Vmax,H | Maximum substrate removal rate (day−1) |
| Vmax,A | Maximum conversion rate of ammoniacal nitrogen in nitrates (mg N/g VSS/h) |
Figure 2.
Autotrophic biomass tests: (a) comparison of the data by the Michaelis–Menten theory; (b) respirogram obtained with the addition of ammonium chloride (small rhombs) and sodium nitrite (squares) in the same sample.
Figure 2.
Autotrophic biomass tests: (a) comparison of the data by the Michaelis–Menten theory; (b) respirogram obtained with the addition of ammonium chloride (small rhombs) and sodium nitrite (squares) in the same sample.
The respirogram reported in
Figure 2b has been obtained by adding sodium nitrite only when the ammoniacal nitrogen that was previously fed was completely converted into nitric nitrogen, therefore observing endogenous growth conditions.
The maximum growth rate of the nitrification was (
µ max,A)
T=26.5 °C = 0.52 day
−1 at a temperature of 26.5 °C, according to the technical literature [
9,
19]. The following parameters values were assumed for the calculation:
(Vmax,A)T=20 = 82.22 mg N g
−1 VSS h
−1;
(Vmax,A)T=26,5 = 127.05 mg N g
−1 VSS h
−1;
KNH = 1.32 mg N L
−1;
YA = 0.25 mg COD mg
−1 N; and
RXA = 6.61 mg N L
−1 h
−1. All cellular conversion coefficients have been searched for in the technical literature [
19], and they result as being equal to, respectively, 0.14 (
YAO) and 0.09 (
YNO). Moreover, it has been found that
RXAO = 0.98 mg N L
−1 h
−1 and
RXNO = 0.49 mg N L
−1 h
−1.
4. Conclusions
The reduction of pollution originating in surface waters receiving discharges from WWTP is a key objective of water pollution control at a local scale in highly populated areas. The operation of all of the plants operating along a single receiving water body should be implemented at a management network level. In this perspective, a direct measurement of experimental parameters in a full-scale plant may allow for the implementation of a systematic collection of data that can be used in plan design, stage performance control and operation, supported by suitably calibrated modeling.
Furthermore, while, on the one hand, the technical literature contains many indications concerning the values of the kinetic constants (in the mesophilic field), it is also true that their values must be checked periodically, by carrying out studies, like the one presented here.
The present paper investigates the biological parameters in a major municipal WWTP by means of the oxygen uptake rate method. By sampling wastewater and sludge, parameters related to processes promoted by heterotrophic and autotrophic biomass were determined by means of assays based on the measurement of oxygen uptake. The obtained results present good applicability to the design process, considering the specificity of the wastewater to be treated and the environmental problems of the area where the WWTP is located. The kinetics of the biological process of biomass growth and substrate removal were analyzed, and they fit remarkably well with the results of the experimental activity.
Several tests were performed to obtain reliable statistical values.
The values obtained for the kinetic parameters agreed with the literature ranges in this case for the greater part of the kinetic parameters, while they resulted as being out of the ranges in the case of the cellular decay rate (heterotrophic biomass). Furthermore, concerning the maximum growth rate, the observed data seem to highlight the slow kinetics of organic matter removal. Partially, the differences between the obtained results and the data reported in the technical literature can be explained, because the literature does not provide univocal indications regarding the values of the F/M ratio to be adopted for respirometric tests.
Regarding the fitting mode, improvements could be obtained to better define the OUR profile by the assumption of a suitable selecting mode of points to be used for arranging the maximum activity values. Regarding the biological process in the experimental set-up, suitable operation should take into account a more proper concentration of acetate, according to the defined sludge concentration.
The evolution of the work that we are carrying out is the comparison of the results obtained both in the thermophilic field of temperature and in mesophilic conditions. This aspect could have a great deal of importance considering different aspects, such as improving the treatment yield passing from mesophilic to thermophilic conditions, at full scale (with the potential increase of the energetic consumption passing from mesophilic to thermophilic conditions).