Effect of Protoneutron Star Magnetized Envelops in Neutrino Energy Spectra
Abstract
:1. Introduction
2. Neutrino Kinetics
3. Neutrino Dynamics in Magnetized Neutrino Sphere
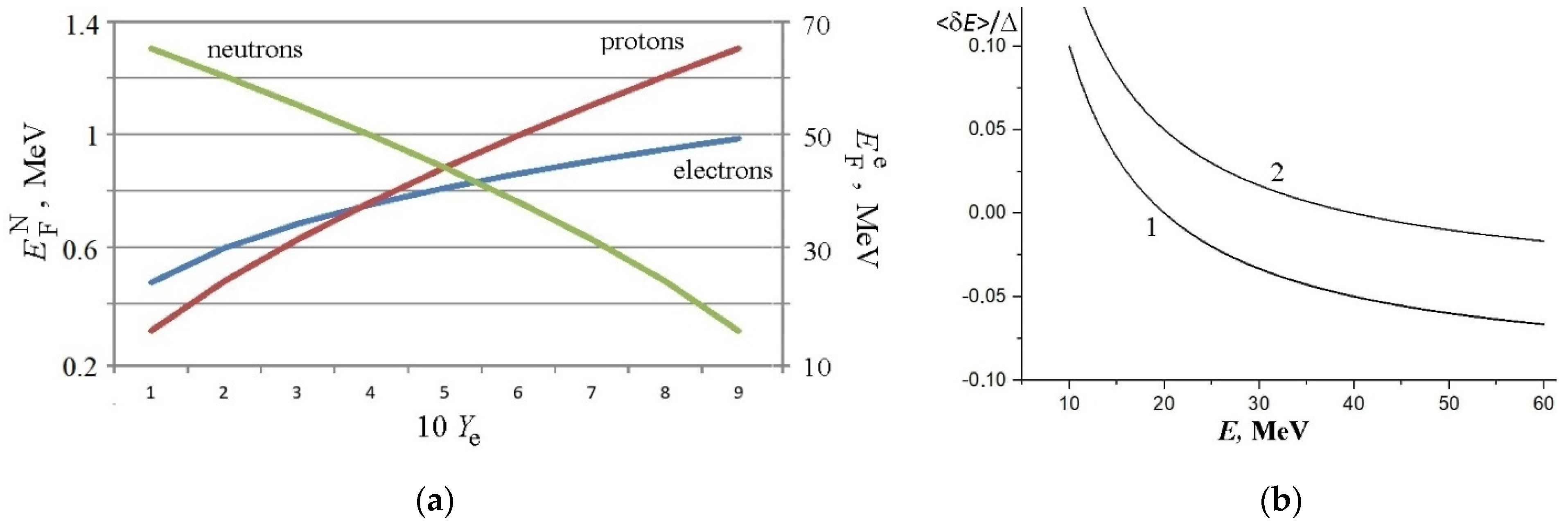
3.1. Neutrino Sphere Properties
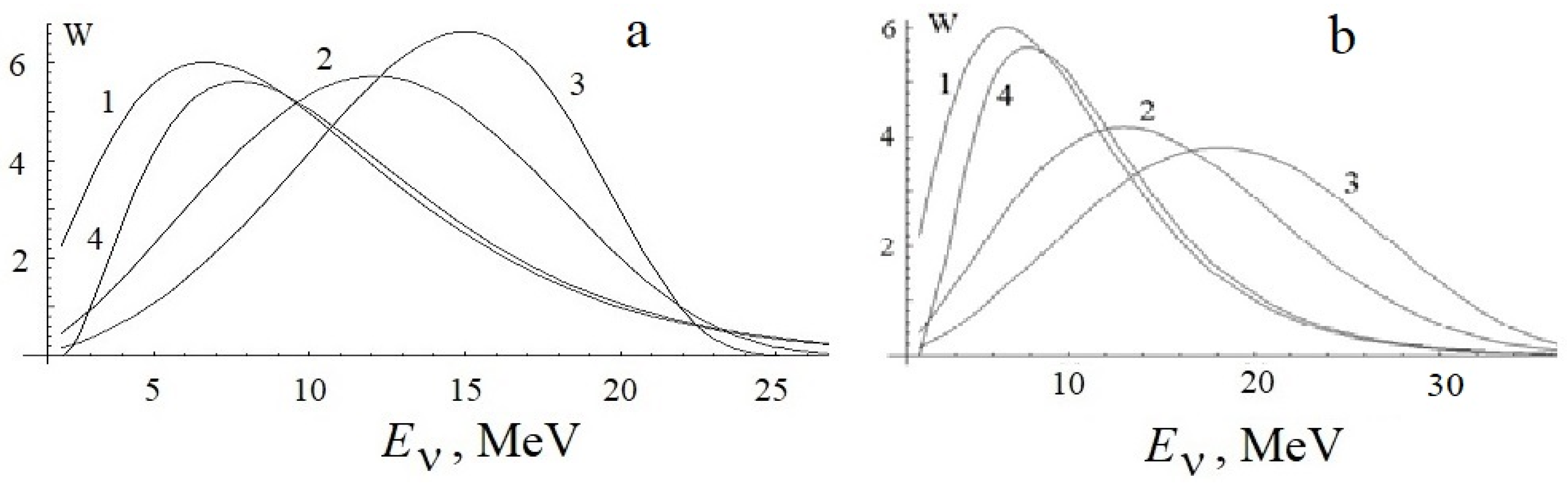
3.2. Energy Transfer in Neutrino Spectra
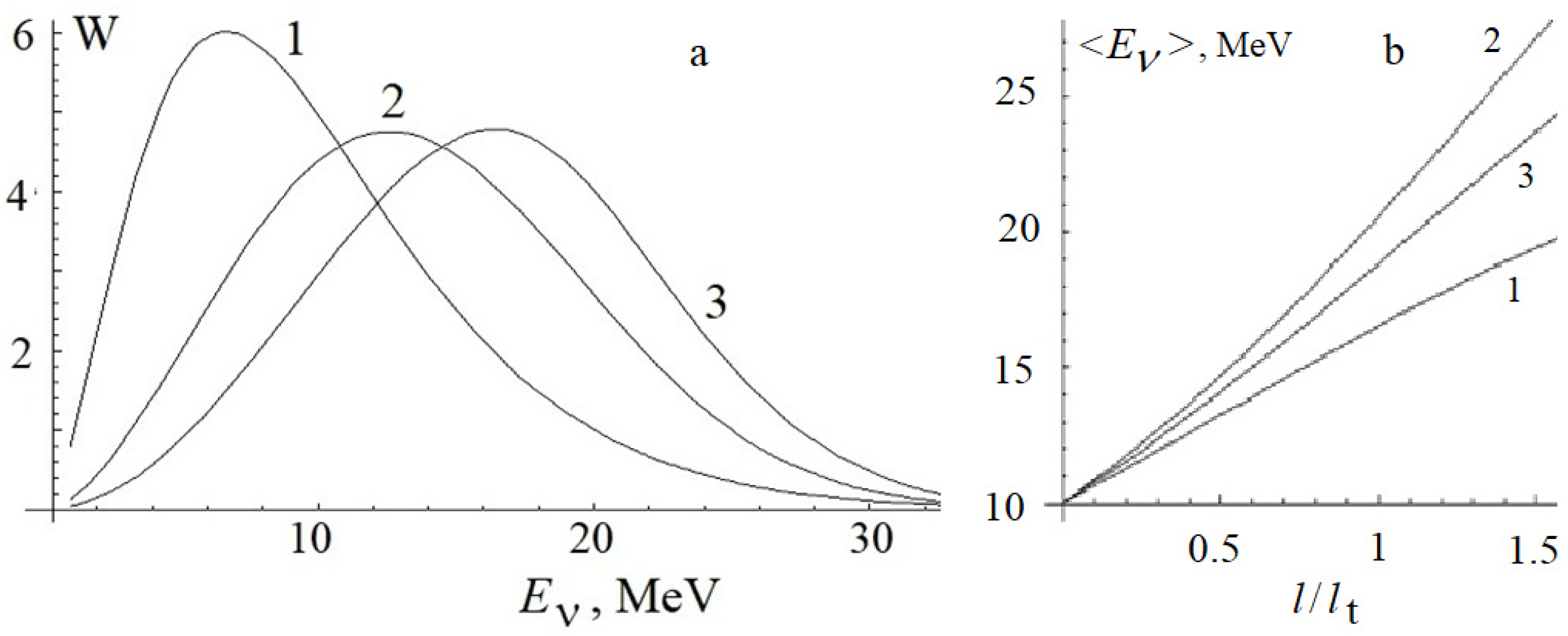
3.3. Fluctuation Effects in Energy Spectra
4. Strengthening of Spectrum Hardness and Large Neutrino Detector Sensitivity
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Mösta, P.; Ott, C.D.; Radice, D.; Roberts, L.; Schnetter, E.; Haas, R. A large-scale dynamo and magnetoturbulence in rapidly rotating core-collapse supernovae. Nature 2015, 528, 376–379. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guilet, J.; Müller, E. Numerical simulations of the magnetorotational instability in protoneutron stars—I. Influence of buoyancy. Mon. Not. R. Astron. Soc. 2015, 450, 2153–2171. [Google Scholar] [CrossRef]
- Bethe, H.A.; Wilson, H.A. Revival of a stalled supernova shock by neutrino heating. Astrophys. J. 1985, 295, 14. [Google Scholar] [CrossRef]
- Bethe, H.A. Supernova mechanisms. Rev. Mod. Phys. 1990, 62, 801. [Google Scholar] [CrossRef]
- Janka, H.-T.; Melson, T.; Summa, T. Physics of core-collapse supernovae in three dimensions: A sneak preview. Annu. Rev. Nucl. Part. Sci. 2016, 66, 341–375. [Google Scholar] [CrossRef] [Green Version]
- Kondratyev, V.N.; Korovina, Y.V. Synthesis of chemical elements in dynamo active supernovae. JETP Lett. 2015, 102, 131. [Google Scholar] [CrossRef]
- Kondratyev, V.N. Properties and composition of magnetized nuclei. Particles 2020, 3, 272–277. [Google Scholar] [CrossRef] [Green Version]
- Kondratyev, V.N. Neutron capture reactions in strong magnetic fields of magnetars. Phys. Rev. C 2004, 69, 038801. [Google Scholar] [CrossRef]
- Kondratyev, V.N. R-Process with magnetized nuclei at dynamo-explosive supernovae and neutron star mergers. Universe 2021, 7, 487. [Google Scholar] [CrossRef]
- Horowitz, C.J. Weak magnetism for antineutrinos in supernovae. Phys. Rev. D 2002, 65, 043001. [Google Scholar] [CrossRef] [Green Version]
- Kondratyev, V.N.; Dzhioev, A.A.; Vdovin, A.I.; Cherubini, S.; Baldo, M. Energy exchange in neutrino nuclear scattering. Phys. Rev. C 2019, 100, 045802. [Google Scholar] [CrossRef]
- Adrián-Martínez, S.; Ageron, M.; Aharonian, F.; Aiello, S.; Albert, A.; Ameli, F.; Anassontzis, E.; Andre, M.; Androulakis, G.; Anghinolfi, M.; et al. Letter of intent for KM3NeT 2.0. J. Phys. G Nucl. Part. Phys. 2016, 43, 084001. [Google Scholar] [CrossRef]
- Avrorin, A.D.; Avrorin, A.V.; Aynutdinov, V.M.; Bannash, R.; Belolaptikov, I.A.; Brudanin, V.B.; Budnev, N.M.; Doroshenko, A.A.; Domogatsky, G.V.; Dvornický, R.; et al. Baikal-GVD. EPJ WoC 2017, 136, 04007. [Google Scholar] [CrossRef] [Green Version]
- The Garching Core-Collapse Supernova Research. Available online: https://wwwmpa.mpa-garching.mpg.de/ccsnarchive/ (accessed on 7 April 2022).
- Aiello, S.; Albert, A.; Garre, S.A.; Aly, Z.; Ambrosone, A.; Ameli, F.; Andre, M.; Androulakis, G.; Anghinolfi, M.; Anguita, M.; et al. The KM3NeT potential for the next core-collapse supernova observation with neutrinos. Eur. Phys. J. C 2021, 81, 445. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kondratyev, V.N.; Lobanovskaya, T.D.; Torekhan, D.B. Effect of Protoneutron Star Magnetized Envelops in Neutrino Energy Spectra. Particles 2022, 5, 128-134. https://doi.org/10.3390/particles5020011
Kondratyev VN, Lobanovskaya TD, Torekhan DB. Effect of Protoneutron Star Magnetized Envelops in Neutrino Energy Spectra. Particles. 2022; 5(2):128-134. https://doi.org/10.3390/particles5020011
Chicago/Turabian StyleKondratyev, Vladimir N., Tamara D. Lobanovskaya, and Dimash B. Torekhan. 2022. "Effect of Protoneutron Star Magnetized Envelops in Neutrino Energy Spectra" Particles 5, no. 2: 128-134. https://doi.org/10.3390/particles5020011
APA StyleKondratyev, V. N., Lobanovskaya, T. D., & Torekhan, D. B. (2022). Effect of Protoneutron Star Magnetized Envelops in Neutrino Energy Spectra. Particles, 5(2), 128-134. https://doi.org/10.3390/particles5020011




