Numerical and Experimental Reconstruction of Temperature Distribution and Soot Concentration Field for Thin-Slice Flames Using a Charge Coupled Device Camera
Abstract
:1. Introduction
2. Reconstruction Model
2.1. Direct Problem
2.2. Inverse Problem
3. Numerical Reconstruction Results and Discussion
3.1. Effects of Wavelength Combinations on Reconstruction Accuracy
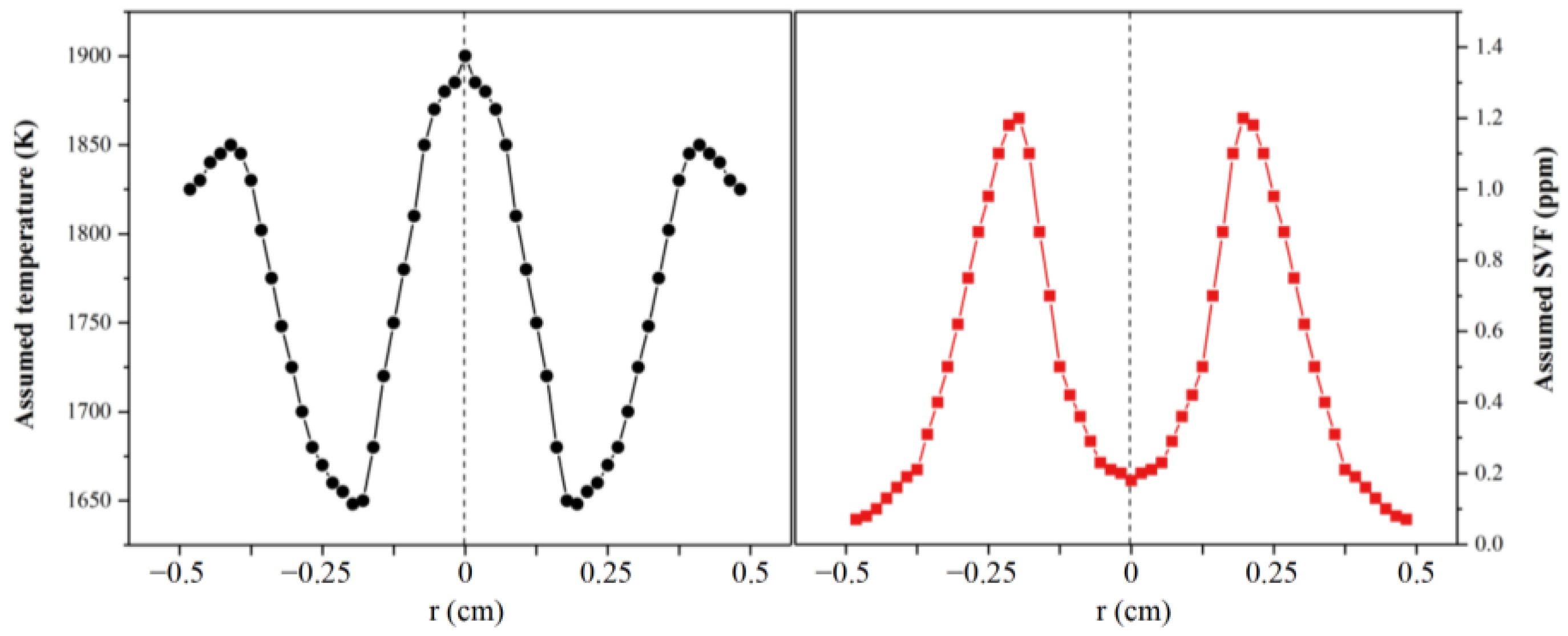
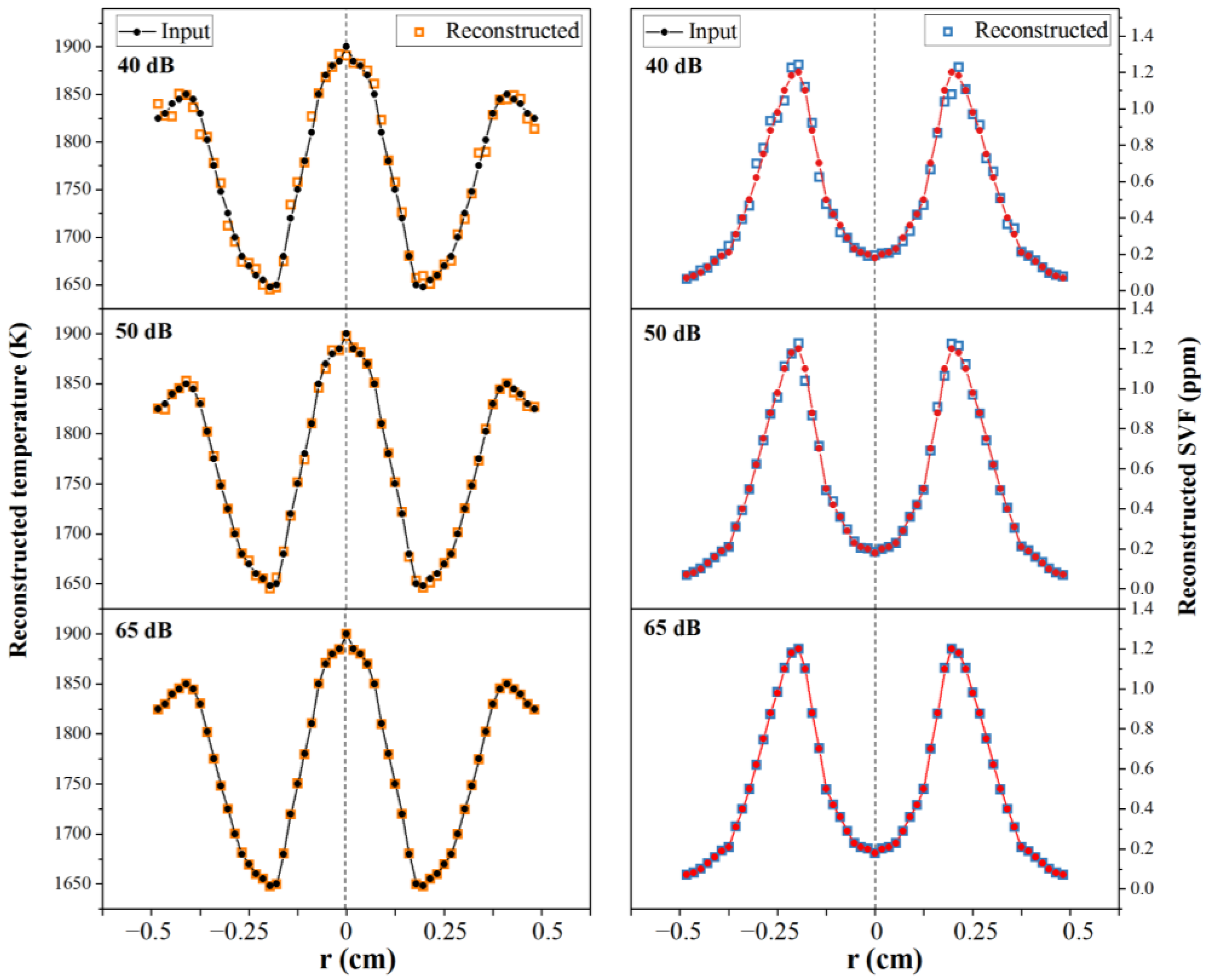
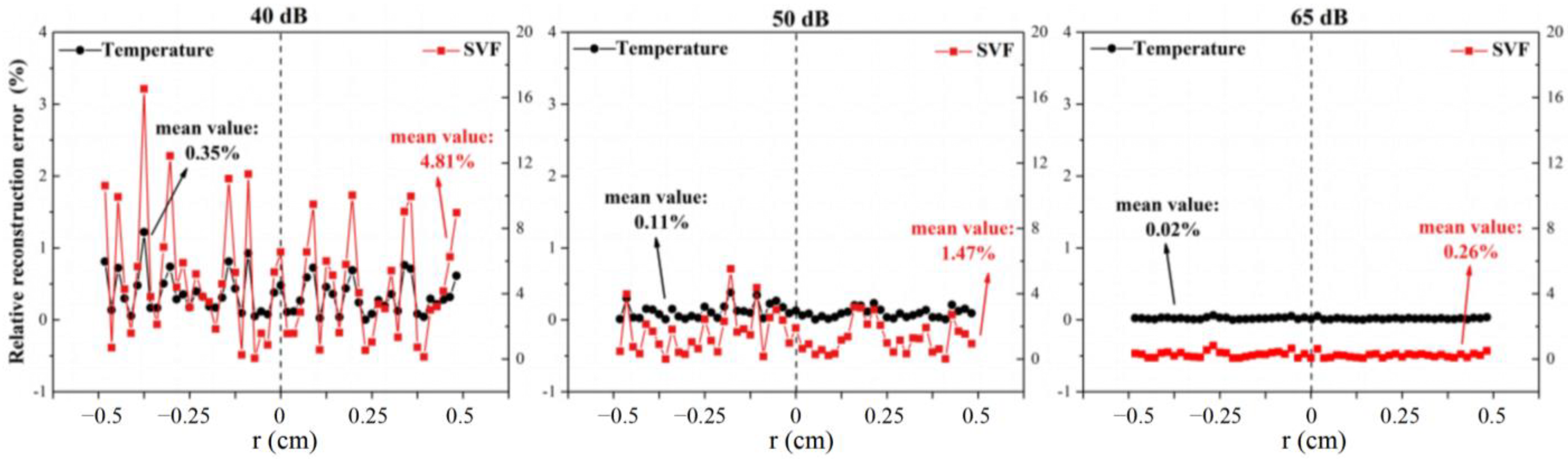
3.2. Effects of Measurement Errors on Reconstruction Accuracy
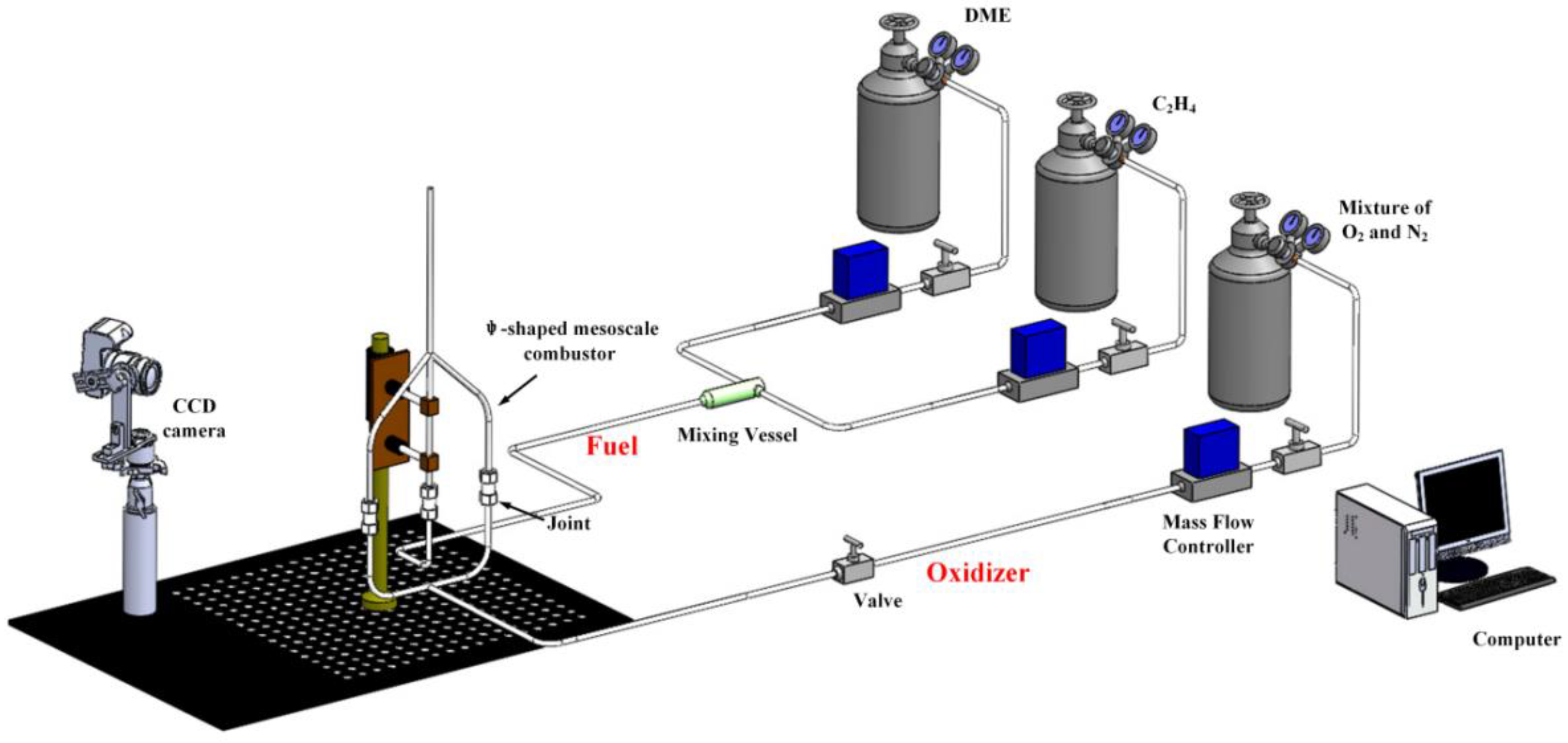
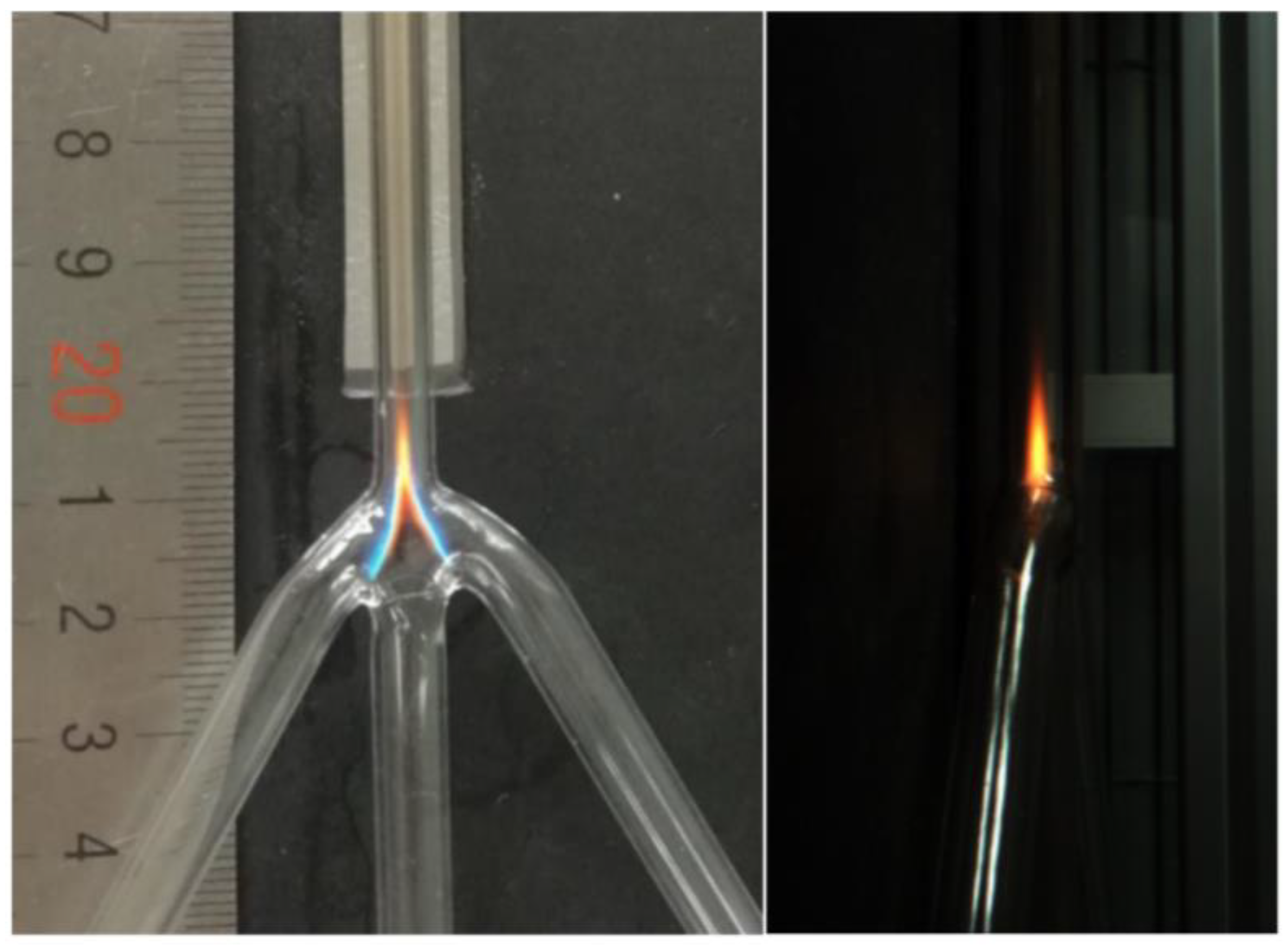
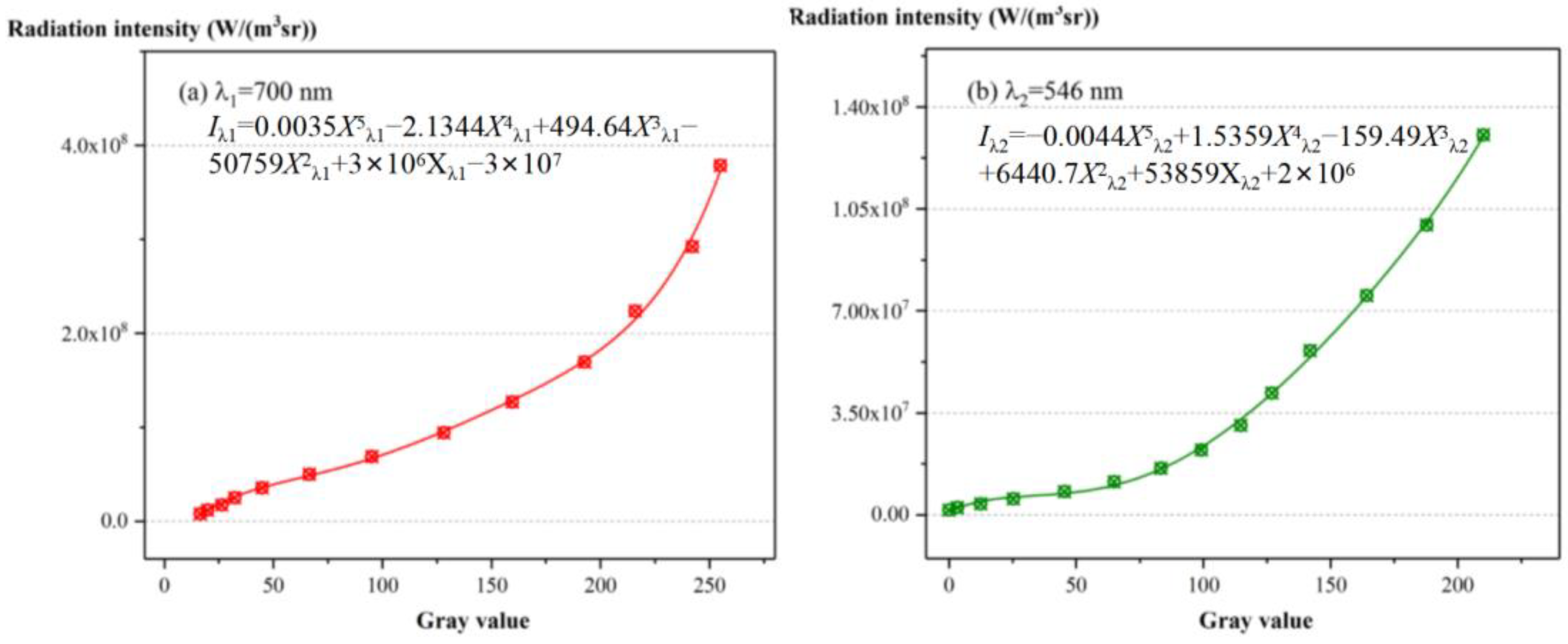
4. Experimental Reconstruction Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Meng, X.; de Jong, W.; Kudra, T. A state-of-the-art review of pulse combustion: Principles, modeling, applications and R&D issues. Renew. Sustain. Energy Rev. 2016, 55, 73–114. [Google Scholar]
- Holme, J.A.; Vondráček, J.; Machala, M.; Lagadic-Gossmann, D.; Vogel, C.F.; Le Ferrec, E.; Sparfel, L.; Øvrevik, J. Lung cancer associated with combustion particles and fine particulate matter (PM2.5)—The roles of polycyclic aromatic hydrocarbons (PAHs) and the aryl hydrocarbon receptor (AhR). Biochem. Pharmacol. 2023, 216, 115801. [Google Scholar] [CrossRef] [PubMed]
- Broumand, M.; Albert-Green, S.; Yun, S.; Hong, Z.; Thomson, M.J. Spray combustion of fast pyrolysis bio-oils: Applications, challenges, and potential solutions. Prog. Energy Combust. Sci. 2020, 79, 100834. [Google Scholar] [CrossRef]
- Ardebili, S.M.S.; Solmaz, H.; Ïpci, D.; Calam, A.; Mostafaei, M. A review on higher alcohol of fusel oil as a renewable fuel for internal combustion engines: Applications, challenges, and global potential. Fuel 2020, 279, 118516. [Google Scholar] [CrossRef]
- Li, T.; Geschwindner, C.; Dreizler, A.; Böhm, B. An experimental study of coal particle group combustion in conventional and oxy-fuel atmospheres using multi-parameter optical diagnostics. Proc. Combust. Inst. 2023, 39, 3259–3269. [Google Scholar] [CrossRef]
- Aliramezani, M.; Koch, C.R.; Shahbakhti, M. Modeling, diagnostics, optimization, and control of internal combustion engines via modern machine learning techniques: A review and future directions. Prog. Energy Combust. Sci. 2022, 88, 100967. [Google Scholar] [CrossRef]
- Kiefer, J.; Ewart, P. Laser diagnostics and minor species detection in combustion using resonant four-wave mixing. Prog. Energy Combust. Sci. 2011, 37, 525–564. [Google Scholar] [CrossRef]
- Zheng, S.; Yang, Y.; Li, X.; Liu, H.; Yan, W.; Sui, R.; Lu, Q. Temperature and emissivity measurements from combustion of pine wood, rice husk and fir wood using flame emission spectrum. Fuel Process. Technol. 2020, 204, 106423. [Google Scholar]
- Tornatore, C.; Marchitto, L.; Valentino, G.; Corcione, F.E.; Merola, S.S. Optical diagnostics of the combustion process in a PFI SI boosted engine fueled with butanol-gasoline blend. Energy 2012, 45, 277–287. [Google Scholar] [CrossRef]
- Kerl, J.; Sponfeldner, T.; Beyrau, F. An external Raman laser for combustion diagnostics. Combust Flame 2011, 158, 1905–1907. [Google Scholar] [CrossRef]
- Aldén, M.; Bood, J.; Li, Z.; Richter, M. Visualization and understanding of combustion processes using spatially and temporally resolved laser diagnostic techniques. Proc. Combust. Inst. 2011, 33, 69–97. [Google Scholar] [CrossRef]
- Zhao, H.; Ladommatos, N. Optical diagnostics for soot and temperature measurement in diesel engines. Prog. Energy Combust. Sci. 1998, 24, 221–255. [Google Scholar] [CrossRef]
- Joo, H.I.; Guider, Ö.L. Soot formation and temperature field structure in co-flow laminar methane-air diffusion flames at pressures from 10 to 60 atm. Proc. Combust. Inst. 2009, 32, 769–775. [Google Scholar] [CrossRef]
- Salinero, J.; Gómez-Barea, A.; Tripiana, M.; Leckner, B. Measurement of char surface temperature in a fluidized bed combustor using pyrometry with digital camera. Chem. Eng. J. 2016, 288, 441–450. [Google Scholar] [CrossRef]
- Govender, D.; Liu, H.; Peng, F.; Cai, W.; Worth, N.A. Tomographic reconstruction of an azimuthally forced flame in an annular chamber. Proc. Combust. Inst. 2023, 39, 1367–1375. [Google Scholar] [CrossRef]
- Kailasanathan, R.K.A.; Zhang, J.; Fang, T.; Roberts, W.L. Effects of diluents on soot surface temperature and volume fraction in diluted ethylene diffusion flames at pressure. Combust. Sci. Technol. 2014, 186, 815–828. [Google Scholar] [CrossRef]
- Hottel, H.C.; Broughton, F.P. Determination of True Temperature and Total Radiation from Luminous Gas Flames. Ind. Eng. Chem. Res. 1932, 4, 166–175. [Google Scholar] [CrossRef]
- Park, H.M.; Lee, W.J. An inverse radiation problem of estimating heat-transfer coefficient in participating media. Chem. Eng. Sci. 2002, 57, 2007–2014. [Google Scholar] [CrossRef]
- Draper, T.S.; Zeltner, D.; Tree, D.R.; Xue, Y.; Tsiava, R. Two-dimensional flame temperature and emissivity measurements of pulverized oxy-coal flames. Appl. Energy 2012, 95, 38–44. [Google Scholar] [CrossRef]
- Liu, F.; Thomson, K.A.; Smallwood, G.J. Soot temperature and volume fraction retrieval from spectrally resolved flame emission measurement in laminar axisymmetric coflow diffusion flames: Effect of self-absorption. Combust Flame 2013, 160, 1693–1705. [Google Scholar] [CrossRef]
- Arana, M.; Pontoni, S.; Sen, I.K. Field measurements of soot volume fractions in laminar partially premixed coflow ethylene/air flames. Combust Flame 2004, 138, 362–372. [Google Scholar] [CrossRef]
- Zheng, S.; Cai, W.; Zhao, C.; Li, D.; Zhang, J.; Lu, Q. On the Measurement of Flame Temperature and Emissivity Based on Multispectral Imaging Technique. Measurement 2022, 196, 111272. [Google Scholar] [CrossRef]
- Liu, D.; Huang, Q.X.; Wang, F.; Chi, Y.; Cen, K.F.; Yan, J.H. Simultaneous measurement of three-dimensional soot temperature and volume fraction fields in axisymmetric or asymmetric small unconfined flames with CCD cameras. J. Heat Transf. 2010, 132, 061202. [Google Scholar] [CrossRef]
- Gross, M.L.; Hedman, T.D.; Son, S.F.; Jackson, T.L.; Beckstead, M.W. Coupling micro and meso-scale combustion models of AP/HTPB propellants. Cobust Flame 2013, 160, 982–992. [Google Scholar] [CrossRef]
- Agarwal, S.; Kumar, V.; Shakher, C. Temperature measurement of wick stabilized micro diffusion flame under the influence of magnetic field using digital holographic interferometry. Opt. Lasers Eng. 2018, 102, 161–169. [Google Scholar] [CrossRef]
- Kim, I.; Kim, J.; Choe, Y.; Ryu, K.; Cha, J.; Ri, J. Effect of vane angle on combustion characteristics of premixed H2/air in swirl micro-combustors with straight vane or twisted vane. Appl. Therm. Eng. 2023, 228, 120528. [Google Scholar] [CrossRef]
- Das, D.D.; Cannella, W.J.; McEnally, C.S.; Mueller, C.J.; Pfefferle, L.D. Two-dimensional soot volume fraction measurements in flames doped with large hydrocarbons. Proc. Combust. Inst. 2017, 36, 871–879. [Google Scholar] [CrossRef]
- Anchukaitis, K.J.; Smerdon, J.E. Progress and uncertainties in global and hemispheric temperature reconstructions of the Common Era. Quat. Sci. Rev. 2022, 286, 107537. [Google Scholar] [CrossRef]
- Braga, N.R.F.; Junqueira, O.C. Configuration entropy in the soft wall AdS/QCD model and the Wien law. Phys. Lett. B 2021, 820, 136485. [Google Scholar] [CrossRef]
- Modest, M.F. Radiative Heat Transfer; Academic Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Chang, H.-C.; Charalampopoulos, T.T. Determination of the wavelength dependence of refractive indices of flame soot. Proc. R. Soc. A Math. Phys. Eng. Sci. 1990, 430, 577–591. [Google Scholar]
- Zhou, H.-C.; Lou, C.; Lu, J. Recent achievements in measurements of soot volume fraction and temperatures in a coflow diffuse Ethylene-air flame by visible image processing. J. Phys. Conf. Ser. 2009, 147, 012086. [Google Scholar] [CrossRef]
- Thériault, J.-F.; Yu, L.; Stephen, P.; Sheng, Y.; Lin, S.-X. Tunable ultraviolet laser induces high signal-to-noise ratio in intrinsic fluorescence titration for protein- ligand interaction studies. Results Phys. 2023, 44, 106120. [Google Scholar] [CrossRef]
- Chen, M.; Liu, D.; Jiang, B. Soot formation and combustion characteristics in confined mesoscale combustors under conventional and oxy-combustion conditions (O2/N2 and O2/CO2). Fuel 2020, 264, 116808. [Google Scholar] [CrossRef]
- Liu, F.; He, X.; Ma, X.; Zhang, Q.; Thomson, M.; Guo, H.; Smallwood, G.; Shuai, S.; Wang, J. An experimental and numerical study of the effects of dimethyl ether addition to fuel on polycyclic aromatic hydrocarbon and soot formation in laminar coflow ethylene/air diffusion flames. Combust Flame 2011, 158, 547–563. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, M.; Chen, Y.; Li, T.; Liu, D. Numerical and Experimental Reconstruction of Temperature Distribution and Soot Concentration Field for Thin-Slice Flames Using a Charge Coupled Device Camera. Fire 2023, 6, 392. https://doi.org/10.3390/fire6100392
Chen M, Chen Y, Li T, Liu D. Numerical and Experimental Reconstruction of Temperature Distribution and Soot Concentration Field for Thin-Slice Flames Using a Charge Coupled Device Camera. Fire. 2023; 6(10):392. https://doi.org/10.3390/fire6100392
Chicago/Turabian StyleChen, Mingfei, Yun Chen, Tianjiao Li, and Dong Liu. 2023. "Numerical and Experimental Reconstruction of Temperature Distribution and Soot Concentration Field for Thin-Slice Flames Using a Charge Coupled Device Camera" Fire 6, no. 10: 392. https://doi.org/10.3390/fire6100392






