Development of a Semi-Empirical Model for Estimating the Efficiency of Thermodynamic Power Cycles

Abstract

Power plants constitute the main sources of electricity production, and the calculation of their efficiency is a critical factor that is needed in energy studies. The efficiency improvement of power plants through the optimization of the cycle is a critical means of reducing fuel consumption and leading to more sustainable designs. The goal of the present work is the development of semi-empirical models for estimating the thermodynamic efficiency of power cycles. The developed model uses only the lower and the high operating temperature levels, which makes it flexible and easily applicable. The final expression is found by using the literature data for different power cycles, named as: organic Rankine cycles, water-steam Rankine cycles, gas turbines, combined cycles and Stirling engines. According to the results, the real operation of the different cases was found to be a bit lower compared to the respective endoreversible cycle. Specifically, the present global model indicates that the thermodynamic efficiency is a function of the temperature ratio (low cycle temperature to high cycle temperature). The suggested equation can be exploited as a quick and accurate tool for calculating the thermodynamic efficiency of power plants by using the operating temperature levels. Moreover, separate equations are provided for all of the examined thermodynamic cycles.

1. Introduction

1.1. Power Plants Efficiency

The global energy demand will increase by 50% between 2020 and 2050 [1], which will also lead to a 25% increase in CO₂ emissions on a yearly basis [2]. The population increase and new lifestyle trends are basic reasons for the present and future energy situation. Power plants produce the majority of the required electricity; therefore, great interest is given to them, with the aim of increasing their performance [3]. Moreover, the incorporation of renewable energies (e.g., solar energy [4]) is vital for reducing the associated CO₂ emissions. The optimization of power plants [5] is also an important weapon for achieving sustainability and leading to suitable units that are ideal for reducing the cost of electricity and simultaneously increasing CO₂ avoidance. Another important option is the optimization of power cycles in order to increase their thermodynamic efficiency, which would reduce fuel consumption and consequently lead to sustainable designs.

1.2. Brief Literature Review

Quick and simplistic methods for the estimation of power plants’ efficiency are an issue that has been examined by various researchers. Theoretical thermodynamic studies have been performed to determine the optimal operating conditions of power plants. Usually, the operating temperature levels of the thermodynamic cycles are used in the examined models because they play a critical role in the efficiency. Specifically, the temperatures commonly incorporated in the calculations are related to the temperatures of the heat sinks that communicate with the power cycles (heat input and heat rejection sinks). Also, there are models that incorporate extra parameters like the capacity and the heat transfer coefficients.

The theoretical maximum limit for the thermodynamic efficiency of the power cycle is determined by the Carnot ideal cycle, using the low cycle temperature (T_low) and the high cycle temperature (T_high), as shown below [6]:

$${\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}=1-\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}$$

(1)

However, the Carnot cycle is the theoretical ideal cycle and cannot be achieved in real life. Therefore, a more realistic approach has been suggested by Curzon and Ahlborn [7], which indicates lower thermodynamic efficiency values. Specifically, this formula takes the real heat transfer from the hot and cold sinks into consideration, but it assumes a Carnot cycle after the heat transfer. Thus, there is an endoreversible cycle, where the efficiency can be found below [7]:

$${\mathsf{\eta }}_{\mathrm{e}\mathrm{n}\mathrm{d}\mathrm{o}\mathrm{r}}=1-\sqrt{\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}}$$

(2)

Through the application of Taylor’s theory, the endoreversible efficiency can be written, as below, by giving the first three factors of the polynomial approximation expression [8]:

$${\mathsf{\eta }}_{\mathrm{e}\mathrm{n}\mathrm{d}\mathrm{o}\mathrm{r}}=1-\sqrt{1-{\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}}\approx \frac{{\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}}{2}+\frac{{{(\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}})}^2}{8}+\frac{{{6\cdot (\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}})}^3}{96}+\dots$$

(3)

Interestingly, the previous formula indicates that the endoreversible efficiency is a bit higher than half of the Carnot efficiency—an interesting result that can be exploited for the cycle efficiency analysis.

Considering the maximum high temperature as the exergetically equivalent temperature, then the cycle efficiency can be written as below, according to Bejan [9]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}=1-\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}\cdot \left(1+\ln \left[\frac{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}\right]\right)$$

(4)

The previous formula also considers the power plant as an obstacle between the high and low heat sinks.

Assuming that a heat engine operates at ecological optimization conditions, the thermodynamic efficiency can be written as below [10,11]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}=1-\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}\cdot \sqrt{\frac{1+\frac{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}}{2}}$$

(5)

A generalized approach uses the irreversibility (i) and leads to the following cycle efficiency, which has been suggested by Novikov [6,12]:

$${\mathsf{\eta }}_{\mathrm{e}\mathrm{n}\mathrm{d}\mathrm{o}\mathrm{r}}=1-\sqrt{\left(1+\mathrm{i}\right)\cdot \frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}}$$

(6)

Another general approach uses the superscript (a) in the temperature ratio, as below [13]. The following expression can approximate any cycle by selecting the suitable value of parameter (a):

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}=1-{\left(\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}\right)}^{\mathrm{a}}$$

(7)

The use of infinite reversible Carnot cycles together leads to the concept of the “poly-cycle”, which presents the following theoretical efficiency [14]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}=1+\frac{1-{\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}}{{\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}}\cdot \ln \left[1-{\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}\right]$$

(8)

The theoretical efficiency of a combined cycle, using endoreversible sub-cycles, is given by also introducing the medium heat exchange temperature (T_m) [15]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h},\mathrm{c}\mathrm{c}}=\left(1-\sqrt{\frac{{\mathrm{T}}_{\mathrm{m}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}}\right)+\left(1-\sqrt{\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{m}}}}\right)\cdot \sqrt{\frac{{\mathrm{T}}_{\mathrm{m}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}}$$

(9)

Therefore, it is clear that there is great interest in the literature for thermodynamic cycle performance with various suggested formulas. Different modeling and approximation strategies lead to different expressions. However, the common point of the summarized modeling is the use of the operating temperatures for the estimation of the thermodynamic efficiencies. This fact indicates that the temperatures are the critical parameters for the efficiency of power plants. Also, many equations correlate the thermodynamic efficiency of the cycle with the maximum lit of the Carnot cycle, which, in practice, means a direct connection with the operating temperature levels.

1.3. The Scope of the Present Work

The previous analysis proves that significant research has been conducted with the aim of estimating power plant efficiency. Numerous researchers have developed theoretical equations that can estimate the cycle efficiency in a realistic way, by applying different “boundary conditions” that simulate the real operation in a proper way. However, the majority of the literature studies are theoretical and do not use practical data (e.g., experimental or simulation results). In this direction, the present work aims to fill this scientific gap by developing a semi-empirical model that estimates power plant efficiency in a proper way using the literature data. This model uses the generalized formula of engine efficiency and also employs the literature data to properly fit the general model to the data. The analysis is performed for different power cycles, and a global model is suggested, which can be applied to every thermodynamic cycle. The final results of the present work can be exploited in the future for the accurate and quick estimation of the power plants’ efficiency—something that is important in optimization procedures, environmental studies, as well as in economic investigations. The use of the developed semi-empirical models can accelerate performance improvement and the design of future power plants for achieving sustainability.

2. Material and Methods

2.1. Basic Mathematical Background

The present work is based on the exploitation of the generalized expression for the thermodynamic efficiency (η_th) of a heat engine. More specifically, the following expression is used [13]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}=1-{\left(\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}\right)}^{\mathrm{a}}$$

(10)

where the low cycle temperature (T_low) and the high cycle temperature (T_high) are used in the previous formula, while parameter (a) determines the cycle’s performance. Specifically, the different values of the parameter (a) can lead to different cases of the usual thermodynamic cycles.

For a = 1, Equation (10) represents the Carnot cycle, as below [6]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}\left(\mathrm{a}=1\right)={\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}=1-\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}$$

(11)

For a = 1/2, Equation (10) indicates the Curzon–Ahlborn equations, which correspond to an endoreversible cycle performance [7]:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}\left(\mathrm{a}=\frac{1}{2}\right)={\mathsf{\eta }}_{\mathrm{e}\mathrm{n}\mathrm{d}\mathrm{o}\mathrm{r}}=1-\sqrt{\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}}$$

(12)

For the case with (a = 0), the cycle has zero efficiency and it cannot produce useful work. Therefore, the present expression can model every possible cycle that has a performance from zero up to the maximum Carnot cycle limit. In this direction, the maximum value for the parameter (a) is determined to be 1 (a ≤ 1) because higher values lead to non-acceptable thermodynamic efficiency values.

In cases where the cycle temperatures and efficiency are known, then superscript (a) can be calculated as below [16]:

$$\mathrm{a}=\frac{\ln \left(1-{\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}\right)}{\ln \left(\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}\right)}$$

(13)

An alternative expression of superscript (a), using the cycle efficiency (η_th) and the respective Carnot cycle efficiency (η_carnot), is given below:

$$\mathrm{a}=\frac{\ln \left(1-{\mathsf{\eta }}_{\mathrm{t}\mathrm{h}}\right)}{\ln \left(1-{\mathsf{\eta }}_{\mathrm{c}\mathrm{a}\mathrm{r}\mathrm{n}\mathrm{o}\mathrm{t}}\right)}$$

(14)

The previous expressions can be used for the proper analysis of the data obtained from the literature studies for the calculation of parameter (a).

2.2. Followed Methodology

In the present work, the literature data have been used in order to summarize the thermodynamic efficiency of different power cycles. In each case, the low cycle temperature (T_low), the high cycle temperature (T_high) and the cycle efficiency (η_th) are extracted, aiming to estimate superscript (a), which describes the cycle behavior according to Equation (10). Linear regression methods are applied to estimate the suitable values of parameter (a) in every case. In this work, data for various cycles have been used, and more specifically, for the organic Rankine cycle (ORC), water-steam Rankine cycle (WS-RC), Stirling engine, combined cycle (CC), air gas turbine (Air-GT) and supercritical carbon dioxide gas turbine (SCO₂-GT). The maximum examined temperature is 1800 K for the air gas turbine, while the minimum is 353 K for ORC. It is useful to state that the data for the ORC were separated into two categories: one for low-temperature ORC (LT-ORC) and one for high-temperature ORC (HT-ORC). The low-temperature ORC presents a maximum cycle temperature of up to 110 °C, while the cases with higher temperatures are included in the high-temperature ORC.

For each case, parameter (a) is calculated, as well as for every team of data that corresponds to a specific power cycle. Moreover, all the data will be examined together to determine the global value of the parameter (a) and the final results will be discussed. Finally, the reported data will be compared with the theoretical curves for the Carnot cycle and the endoreversible cycle.

3. Results and Discussion

The present section is devoted to including the results of the present analysis regarding the performance of the thermodynamic cycle and regression with the model presented in this paper.

Table 1. Literature input data in the present analysis.

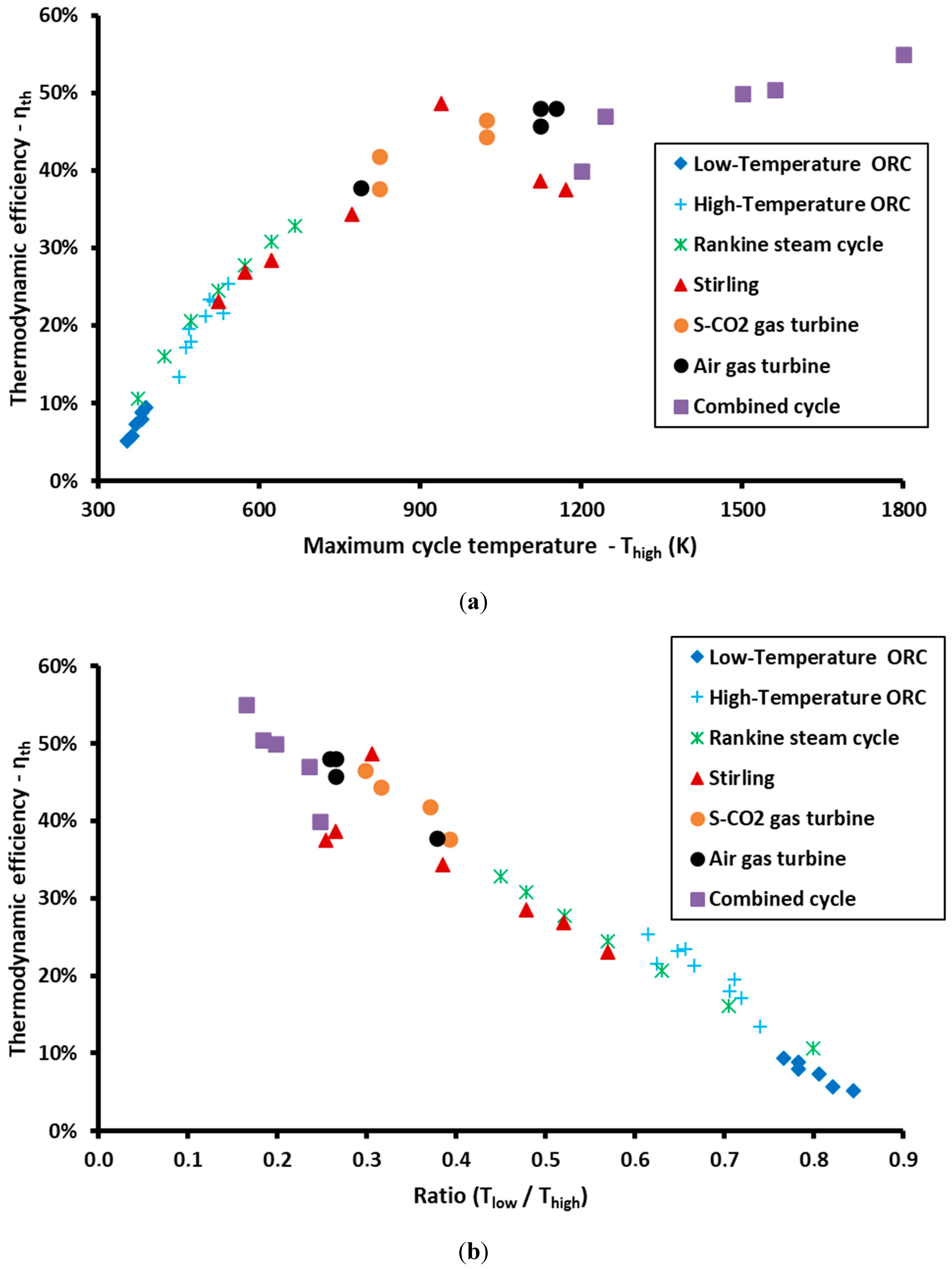
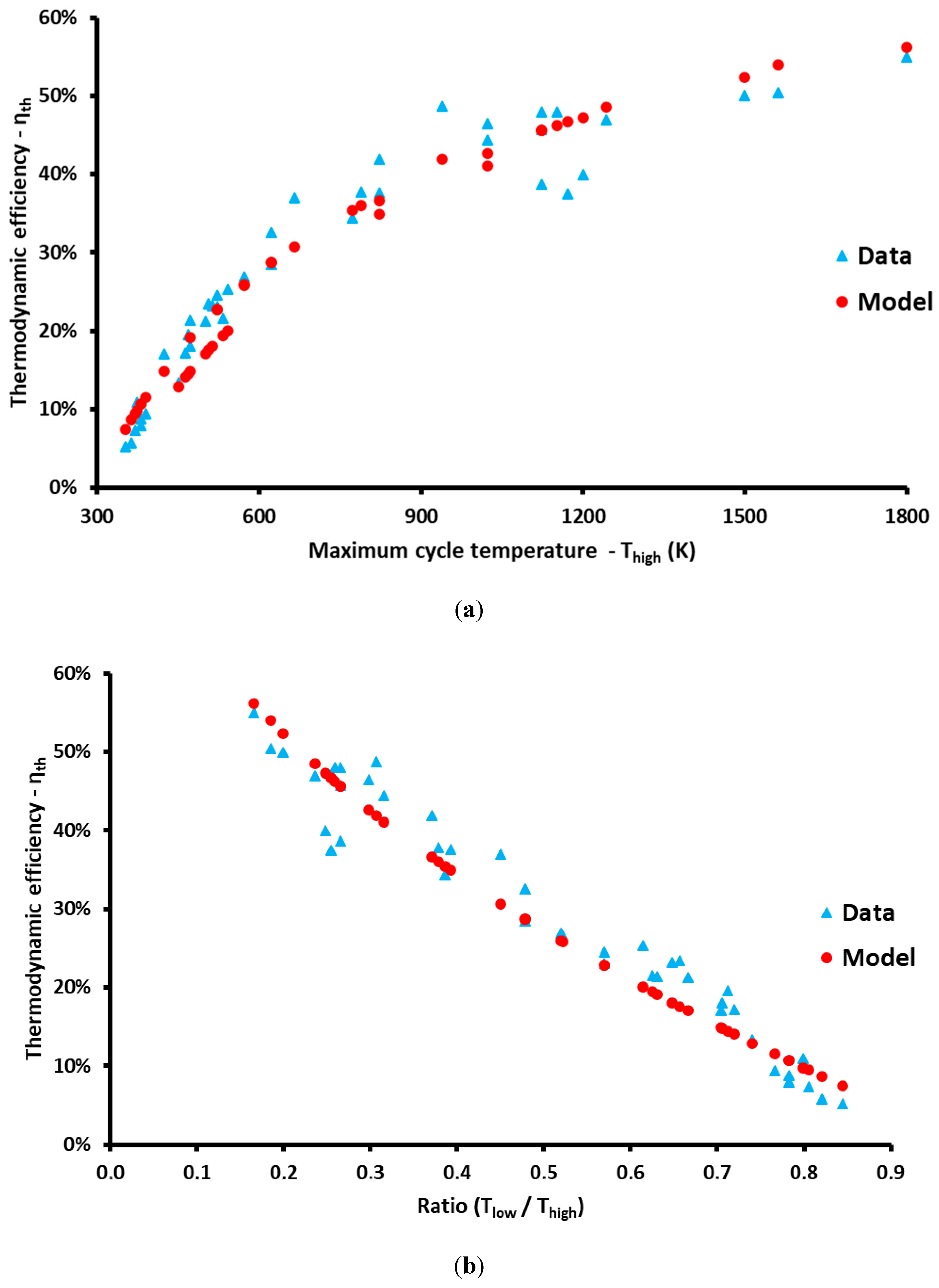
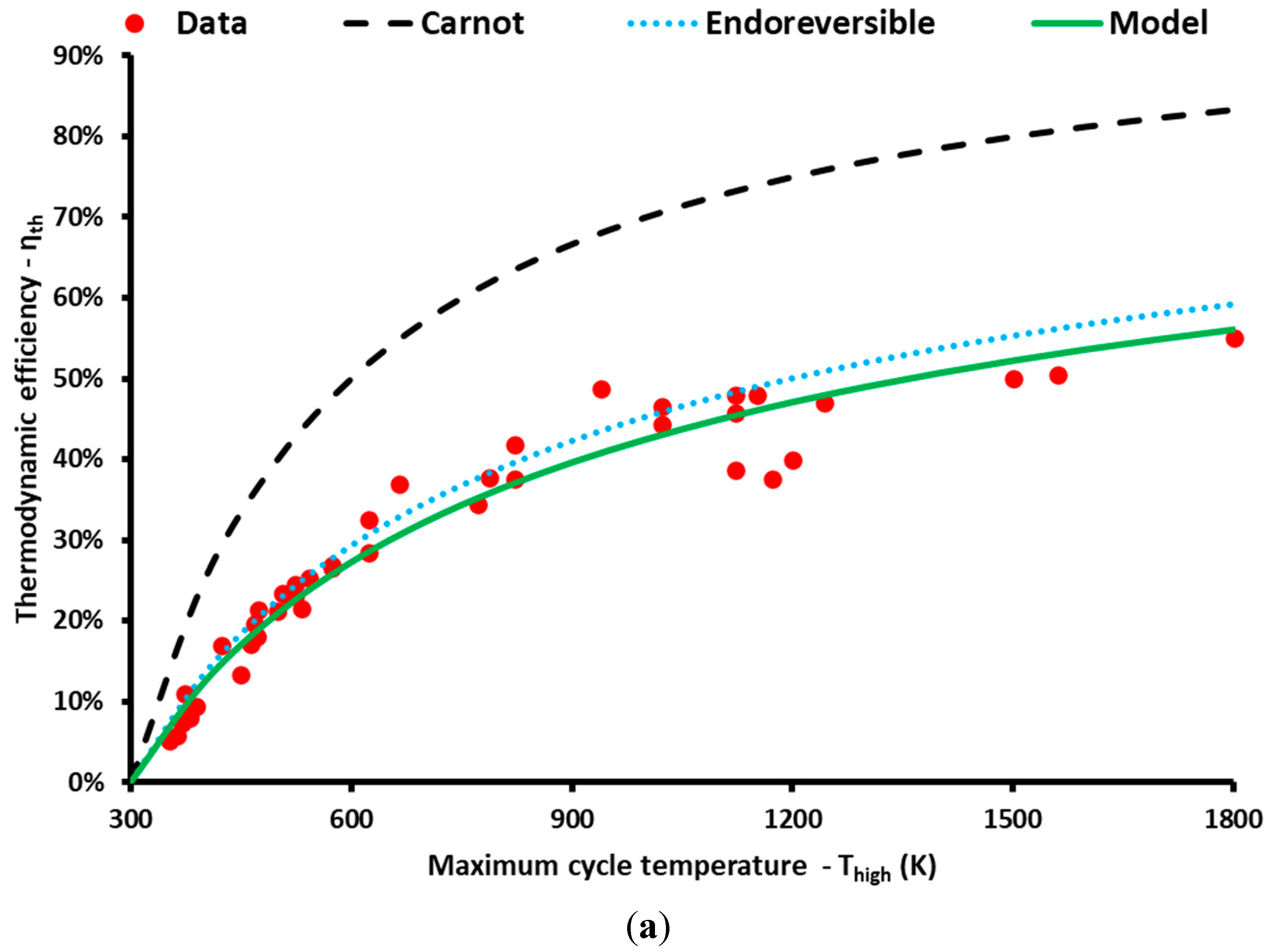
N/A	Thigh (K)	Tlow (K)	ηth	ηcarnot	ηendor	a	Cycle	Type	Ref.
1	389	298	0.0940	0.2339	0.1247	0.3704	LT-ORC	EXPER	[17]
2	381	298	0.0881	0.2178	0.1156	0.3753	LT-ORC	EXPER	[17]
3	370	298	0.0737	0.1946	0.1026	0.3538	LT-ORC	EXPER	[17]
4	363	298	0.0574	0.1791	0.0939	0.2996	LT-ORC	THEOR	[17]
5	353	298	0.0522	0.1558	0.0812	0.3165	LT-ORC	EXPER	[18]
6	381	298	0.0794	0.2178	0.1156	0.3367	LT-ORC	EXPER	[18]
7	542	333	0.2536	0.3856	0.2162	0.6005	HT-ORC	THEOR	[19]
8	507	333	0.2341	0.3432	0.1896	0.6345	HT-ORC	THEOR	[19]
9	514	333	0.2316	0.3521	0.1951	0.6069	HT-ORC	THEOR	[19]
10	533	333	0.2155	0.3752	0.2096	0.5160	HT-ORC	THEOR	[19]
11	500	333	0.2125	0.3340	0.1839	0.5877	HT-ORC	THEOR	[19]
12	468	333	0.1957	0.2885	0.1565	0.6399	HT-ORC	THEOR	[19]
13	472	333	0.1800	0.2945	0.1601	0.5689	HT-ORC	THEOR	[19]
14	463	333	0.1714	0.2808	0.1519	0.5705	HT-ORC	THEOR	[19]
15	450	333	0.1338	0.2600	0.1398	0.4770	HT-ORC	THEOR	[19]
16	623	298	0.3260	0.5217	0.3084	0.5350	WS-RC	THEOR	[20]
17	373	298	0.1092	0.2011	0.1062	0.5151	WS-RC	THEOR	[21]
18	423	298	0.1702	0.2955	0.1607	0.5326	WS-RC	THEOR	[21]
19	473	298	0.2139	0.3700	0.2063	0.5209	WS-RC	THEOR	[21]
20	523	298	0.2451	0.4302	0.2452	0.4999	WS-RC	THEOR	[21]
21	573	299	0.2658	0.4782	0.2776	0.4750	WS-RC	THEOR	[21]
22	666	300	0.3700	0.5495	0.3288	0.5793	WS-RC	EXPER	[22]
23	1123	298	0.3870	0.7346	0.4849	0.3689	Stirling cycle	EXPER	[23]
24	1172	298	0.3750	0.7457	0.4958	0.3432	Stirling cycle	EXPER	[23]
25	773	298	0.3440	0.6145	0.3791	0.4423	Stirling cycle	THEOR	[24]
26	939	288	0.4873	0.6933	0.4462	0.5653	Stirling cycle	THEOR	[25]
27	623	298	0.2850	0.5217	0.3084	0.4549	Stirling cycle	THEOR	[26]
28	573	298	0.2690	0.4799	0.2788	0.4793	Stirling cycle	THEOR	[26]
29	523	298	0.2310	0.4302	0.2452	0.4670	Stirling cycle	THEOR	[26]
30	823	305	0.4190	0.6294	0.3912	0.5470	SCO2-GT	THEOR	[27]
31	1023	305	0.4652	0.7019	0.4540	0.5172	SCO2-GT	THEOR	[27]
32	823	323	0.3765	0.6075	0.3735	0.5051	SCO2-GT	THEOR	[27]
33	1023	323	0.4440	0.6843	0.4381	0.5092	SCO2-GT	THEOR	[27]
34	1123	298	0.4800	0.7346	0.4849	0.4929	Air-GT	EXPER	[28]
35	1123	298	0.4580	0.7346	0.4849	0.4617	Air-GT	EXPER	[28]
36	1152	298	0.4800	0.7413	0.4914	0.4836	Air-GT	EXPER	[28]
37	788	298	0.3780	0.6218	0.3850	0.4883	Air-GT	EXPER	[28]
38	1200	298	0.4000	0.7517	0.5017	0.3667	CC	THEOR	[29]
39	1500	298	0.5000	0.8013	0.5543	0.4289	CC	THEOR	[29]
40	1800	298	0.5500	0.8344	0.5931	0.4440	CC	THEOR	[29]
41	1244	293	0.4700	0.7645	0.5147	0.4391	CC	EXPER	[30]
42	1561	288	0.5047	0.8155	0.5705	0.4157	CC	THEOR	[31]

summarizes the studies included in the present analysis, including the operating temperature levels, the reported thermodynamic efficiency, as well as the calculated efficiency of the respective Carnot cycle and endoreversible cycle. Specifically, there are data from 15 different works, as reported in Table 1. Forty-two different cases are included for seven different cycle types. Also, both experimental and theoretical data were used in the present analysis, as reported in Table 1. For every study, parameter (a) is determined for estimating the cycle efficiency (η_th) according to Equation (10), and the results are reported in Table 1. Moreover, the respective results for the Carnot and the nonreversible cycle are reported. It is clear that the Carnot efficiency is always greater than the reported efficiency, while the endoreversible efficiency is close to the reported efficiency data. Moreover, it is useful to add that the endoreversible efficiency is a bit higher than the respective Carnot efficiency, as was mentioned in the introduction part regarding Equation (3).

Figure 1 depicts the reported data by classifying them according to the power cycle type. Seven different categories were reported and the thermodynamic efficiency was given as a function of the high cycle temperature in Figure 1a and as a function of the temperature ratio (low temperature to high temperature) in Figure 1b.

Table 2. Summary of the parameter (a) for the different cycles by using the logarithmic approximation of the reported results.

Cycle	atotal	R2	amin	amax
Low-Temperature ORC	0.3481	99.44%	0.2996	0.3753
High-Temperature ORC	0.5813	99.37%	0.4770	0.6399
Water-Steam Rankine cycle	0.5295	99.51%	0.4750	0.5793
Stirling cycle	0.4267	96.47%	0.3432	0.5653
S-CO2 gas turbine	0.5189	99.91%	0.5051	0.5470
Air gas turbine	0.4808	99.93%	0.4617	0.4929
Combined cycle	0.4220	99.63%	0.3667	0.4440
TOTAL	0.4594	98.06%	-	-

summarizes the calculated superscripts (a) for the different cycles, and the regression coefficient (R²) is also given for every case. It is very important to highlight that parameter (a) was calculated with a linear regression of the logarithmic factors, according to Equation (10). It is obvious that the reported (R²) values are high; therefore, the regressions are assumed as reliable. Specifically, the values of (R²) ranged from 96.47% up to 99.91%—a fact that verifies the validity of the regression procedures.

More specifically, the following results are reported:

➢

For the LT-ORC, the total (a) is found at 0.3481 while the reported results indicate a variation from 0.2996 up to 0.3753.

➢

For the HT-ORC, the total (a) is found at 0.5813 while the reported results indicate a variation from 0.4770 up to 0.6399.

➢

For the WS-RC, the total (a) is found at 0.5295 while the reported results indicate a variation from 0.4750 up to 0.5793.

➢

For the Stirling cycle, the total (a) is found at 0.4267 while the reported results indicate a variation from 0.3432 up to 0.5653.

➢

For the SCO₂-GT, the total (a) is found at 0.5189 while the reported results indicate a variation from 0.5051 up to 0.5470.

➢

For the Air-GT, the total (a) is found at 0.4808 while the reported results indicate a variation from 0.4617 up to 0.4929.

➢

For the CC, the total (a) is found at 0.4220 while the reported results indicate a variation from 0.3667 up to 0.4440.

The increase in parameter (a) means a higher performance and this renders the cycle close to the respective ideal one (Carnot where a = 1). The highest values of the parameter (a) were found for the HT-ORC, WS-RC and SCO₂-GT, while the next cycles that follow are the Air-GT, Stirling cycle, combined cycle and LT-ORC, respectively. At this point, it is critical to state that value (a) can be variable when a thermodynamic cycle is optimized, but the present results give a general overview of typical cycle cases.

The total set of the 42 reported cases leads to the global value of the parameter (a) of 0.4594, as is given in Table 2. The (R²) is 98.06% for this case, and thus this regression is acceptable. Figure 2 depicts the reported data and the approximated data with the calculated approximation model. Specifically, Figure 2a shows the thermodynamic efficiency results for different values of the high cycle temperature, while in Figure 2b, they are shown as a function of the temperature ratio (low temperature to high temperature). The global approximation model for all the cycles is described by the next equation:

$${\mathsf{\eta }}_{\mathrm{t}\mathrm{h},\mathrm{a}\mathrm{p}\mathrm{p}\mathrm{r}}=1-{\left(\frac{{\mathrm{T}}_{\mathrm{l}\mathrm{o}\mathrm{w}}}{{\mathrm{T}}_{\mathrm{h}\mathrm{i}\mathrm{g}\mathrm{h}}}\right)}^{0.4594}$$

(15)

It is obvious that the real collected data are very close to the data that are produced by the aforementioned approximation model. Thus, there is also a graphical verification of the developed model and it is clear that the developed model gives reasonable results. This note makes it clear that the created model can be used for future calculations of power cycle efficiencies. It constitutes a quick and accurate tool for estimating the efficiency of the power cycle by knowing two critical parameters: the low and the high cycle temperature levels. Moreover, this formula can be used for optimization studies of power cycles (e.g., coupling with solar thermal system).

In the last part of the analysis, Figure 3 depicts the correlation of the reported data and of the developed expression with the curves of the Carnot efficiency and the endoreversible efficiency. Specifically, Figure 3a shows the results for different values of the high cycle temperature, while in Figure 3b, they are shown as a function of the temperature ratio (low temperature to high temperature). It is clear that the Carnot efficiency curves have a significant deviation from the reported results; however, this is reasonable and acceptable. On the other hand, the developed Equation (15) is close to the endoreversible efficiency curve, which indicates that the real operation is close to the endoreversible or the Curzon–Ahlborn cycle. However, the real operation is found to be a bit lower, as is obvious in Figure 3. The reported deviations of the data compared to the curves are explained by the existence of extra variables that influence the results, such as the isentropic efficiency, the working fluid selection, the use of regenerators, etc.

The greatest deviations were reported for high temperatures around 1200 K; however, in these cases, the deviation is also acceptable. On the other hand, the calculation with the suggested model is closer to the data in the low- and high-cycle temperature cases. Additionally, the results of Figure 1 and Figure 2 indicate that all the cycles generally obey the suggested rule, with the results for the Stirling Engine presenting some greater deviations. In this case, the R² is found to be 96.47%, which is the smallest reported value among the cycles; however, it is an acceptable value for the present analysis.

In the future, the developed formula (Equation (15)) can be used as an acceptable and reliable tool for estimating power plant efficiency using only the basic operating temperature levels. Also, the present model can be used for optimization studies, for example, for increasing the cycle performance, for investigating solar-driven cycles, etc.

4. Conclusions

The objective of this investigation is the development of semi-empirical models for estimating the thermodynamic efficiency of power cycles. The developed models only use the lower and higher operating temperature levels. The final expression is found by using the literature data for different power cycles: organic Rankine cycles, water-steam Rankine cycles, gas turbines, combined cycles and Stirling engines. This model can be used for the estimation of the thermodynamic efficiency of power cycles, for the optimization of the cycles, as well as for investigating the coupling of the thermodynamic cycles with renewable energy sources (e.g., solar thermal collectors).

According to the results, the real operation of the different cases was found to be a bit lower compared to the respective endoreversible cycle. Specifically, the present global model indicates that the thermodynamic efficiency is a function of the temperature ratio, as below:

$$\mathrm{T}\mathrm{h}\mathrm{e}\mathrm{r}\mathrm{m}\mathrm{o}\mathrm{d}\mathrm{y}\mathrm{n}\mathrm{a}\mathrm{m}\mathrm{i}\mathrm{c} \mathrm{e}\mathrm{f}\mathrm{f}\mathrm{i}\mathrm{c}\mathrm{i}\mathrm{e}\mathrm{n}\mathrm{c}\mathrm{y}=1-{\left(\frac{\mathrm{L}\mathrm{o}\mathrm{w} \mathrm{c}\mathrm{y}\mathrm{c}\mathrm{l}\mathrm{e} \mathrm{t}\mathrm{e}\mathrm{m}\mathrm{e}\mathrm{p}\mathrm{r}\mathrm{a}\mathrm{t}\mathrm{u}\mathrm{r}\mathrm{e}}{\mathrm{H}\mathrm{i}\mathrm{g}\mathrm{h} \mathrm{c}\mathrm{y}\mathrm{c}\mathrm{l}\mathrm{e} \mathrm{t}\mathrm{e}\mathrm{m}\mathrm{e}\mathrm{p}\mathrm{r}\mathrm{a}\mathrm{t}\mathrm{u}\mathrm{r}\mathrm{e}}\right)}^{0.4594}$$

The aforementioned formula can be used as a quick and accurate tool for estimating the thermodynamic efficiency of power plants by using the minimum possible input data. It presents an R² of 98.06%, which indicates high accuracy. Also, different equations have been separately developed for each power cycle with high-accuracy indexes. More specifically, the following points describe the examined cycles:

➢

In the LT-ORC case, the mean (a) is found at 0.3481 while the reported results indicate a variation from 0.2996 up to 0.3753.

➢

In the HT-ORC case, the mean (a) is found at 0.5813 while the reported results indicate a variation from 0.4770 up to 0.6399.

➢

In the WS-RC case, the mean (a) is found at 0.5295 while the reported results indicate a variation from 0.4750 up to 0.5793.

➢

In the Stirling cycle case, the mean (a) is found at 0.4267 while the reported results indicate a variation from 0.3432 up to 0.5653.

➢

In the SCO₂-GT case, the mean (a) is found at 0.5189 while the reported results indicate a variation from 0.5051 up to 0.5470.

➢

In the Air-GT case, the mean (a) is found at 0.4808 while the reported results indicate a variation from 0.4617 up to 0.4929.

➢

In the CC case, the mean (a) is found at 0.4220 while the reported results indicate a variation from 0.3667 up to 0.4440.

In the future, it will be interesting to separately investigate how parameter (a) varies among the different topologies of each thermodynamic cycle by investigating a greater amount of data from the literature. Specifically, the impact of internal recuperators, heat exchangers, etc., on the parameter (a) can be examined, as well as the impact of different working fluids on the parameter (a). Moreover, a more complex model can be examined with two “free parameters” for estimating the cycles’ performance with greater accuracy. Specifically, parameter (a) can be modeled as a polynomial of other parameters in order to take extra design aspects of each cycle into consideration.