Asymmetrical Thermal Boundary Condition Influence on the Flow Structure and Heat Transfer Performance of Paramagnetic Fluid-Forced Convection in the Strong Magnetic Field
Abstract
:1. Introduction
2. Materials and Methods
2.1. Mathematical Model
2.1.1. Important Dimensionless Parameters
2.1.2. Dimensionless Conservation Equations
- -
- Stationary flow,
- -
- Three-dimensional flow,
- -
- Laminar flow,
- -
- Incompressible flow,
- -
- No additional mass or energy sources,
- -
- The thermo-magnetic force was treated as the body force,
- -
- Constant values of thermo-physical properties,
- -
- Paramagnetic and electrically non-conductive fluid.
2.1.3. Biot-Savart Law
2.1.4. Energy Budget
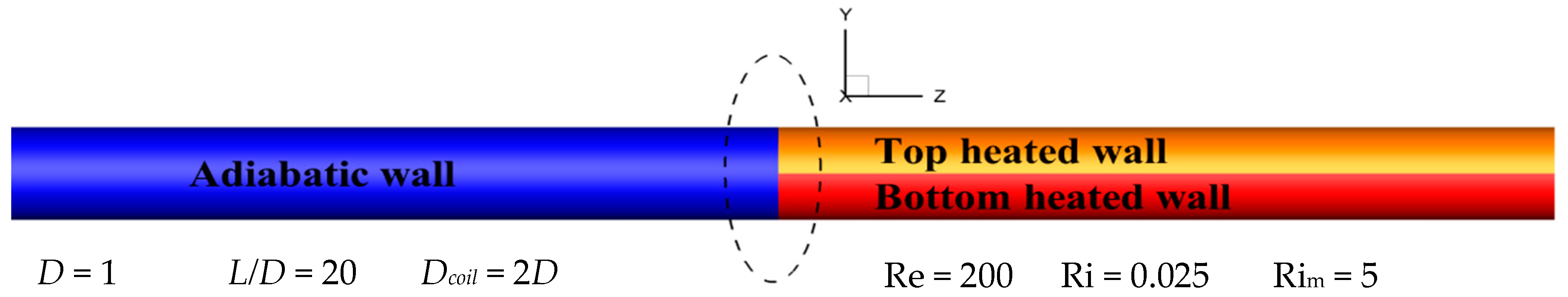
2.2. The Studied Case
- At the inlet: ; ,
- At the outlet: ,At the heated wall: ; ,
- At the adiabatic wall: ; ,
- In the center of the magnetic coil: .
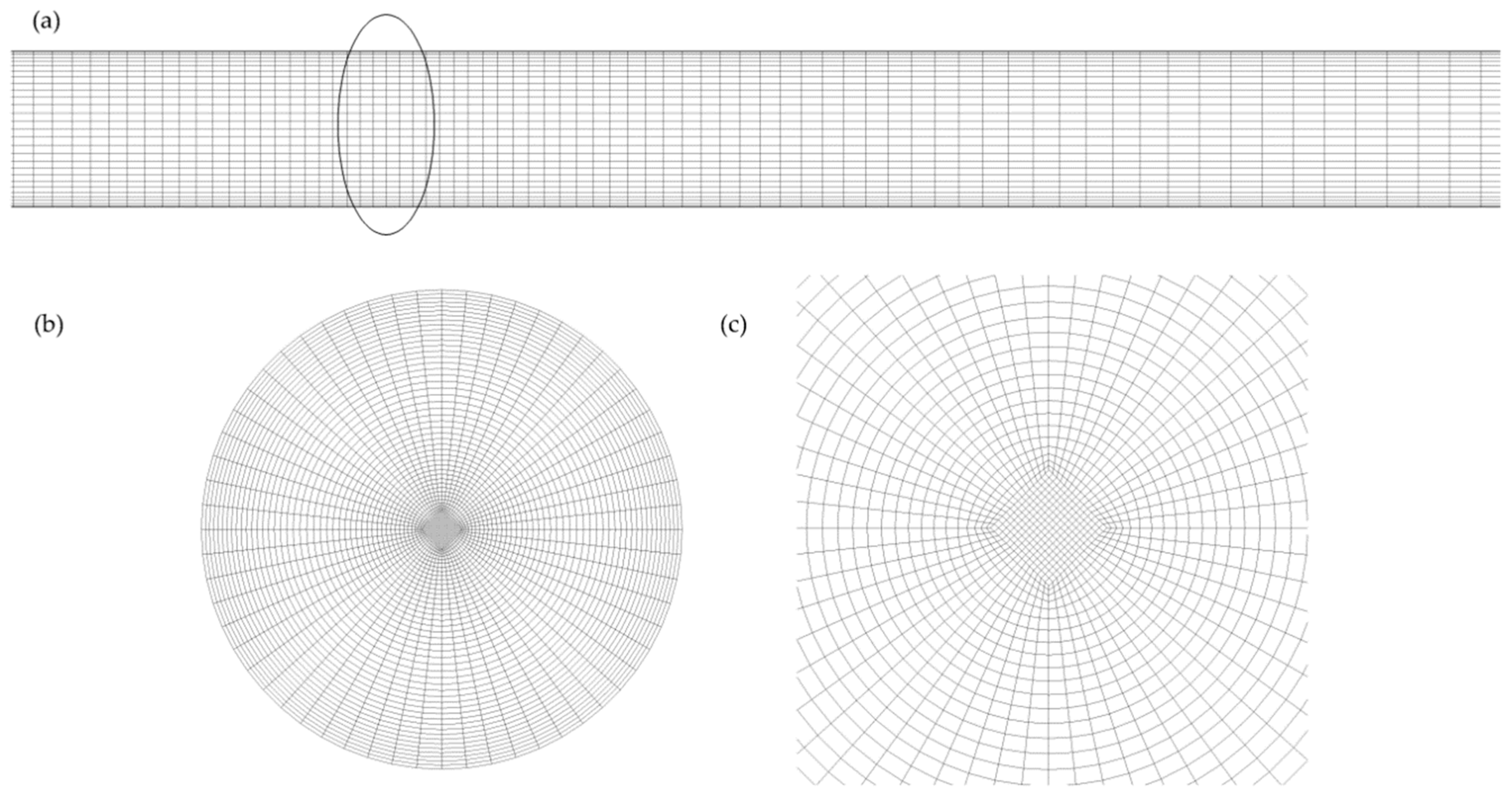
2.3. Numerical Approach
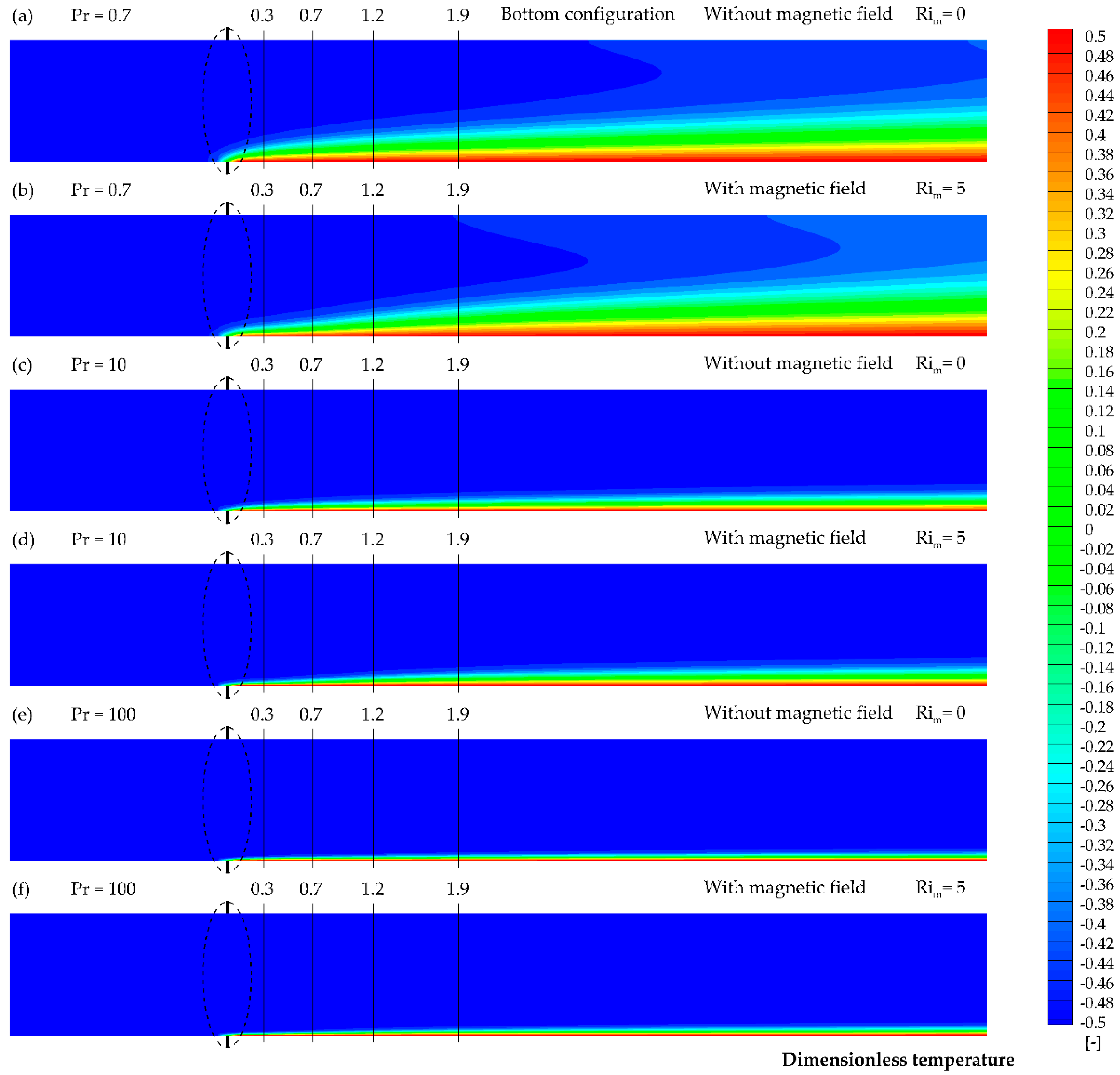
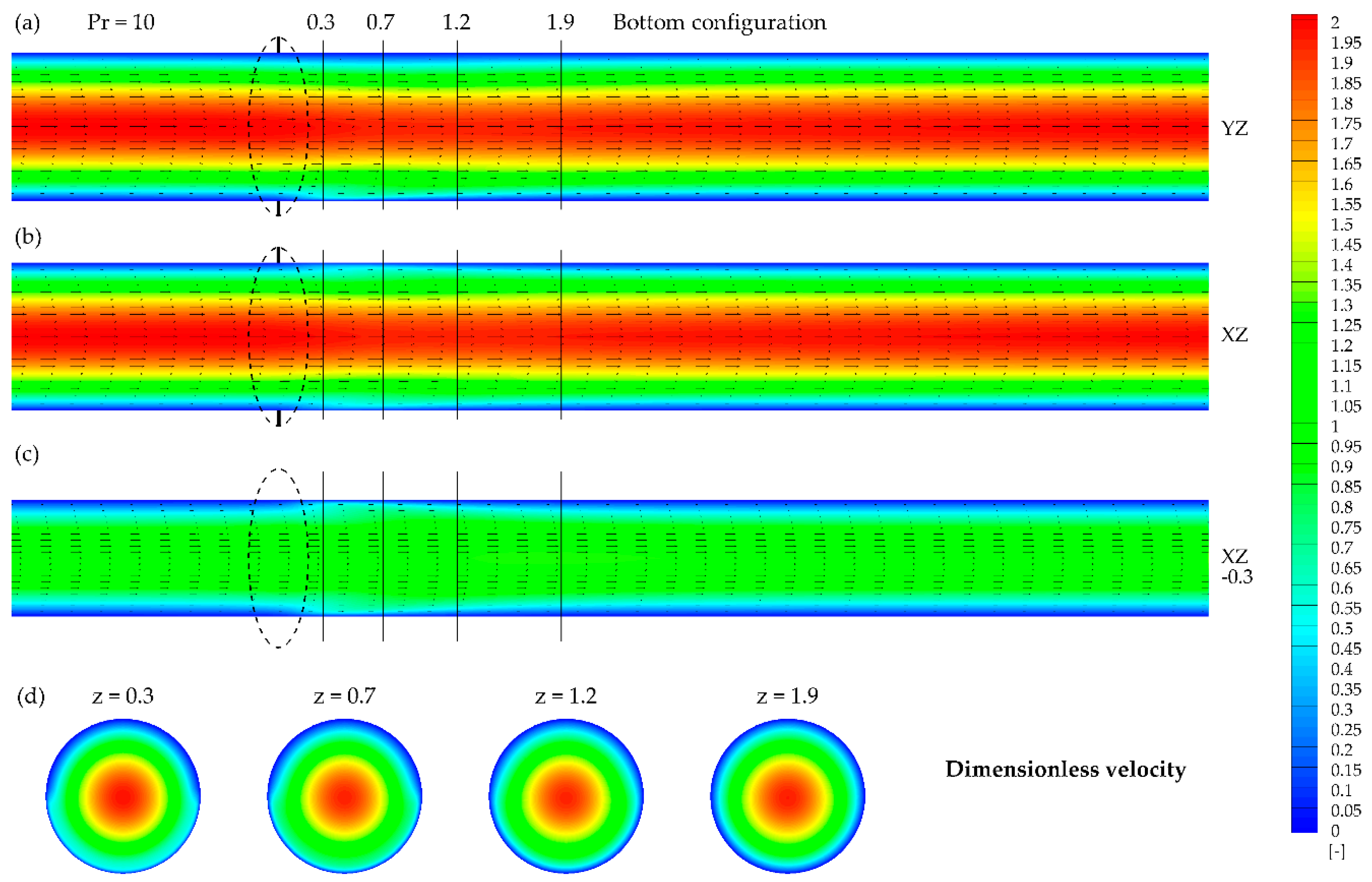
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Latin symbols | |
| dimensionless magnetic induction vector ( ) (-) | |
| magnetic induction vector (T) | |
| Conv | convective term of energy equation (-) |
| D | dimensionless diameter (-) |
| diameter (m) | |
| Diff | diffusive term of energy equation (-) |
| infinitely small element of the coil (m) | |
| G | dimensionless gravitational acceleration (-) |
| gravitational acceleration (m/s2) | |
| Grashof number (-) | |
| convective heat transfer coefficient (W/(m2K)) | |
| electrical current (A) | |
| thermal conductivity (W/(mK)) | |
| dimensionless pipe length ( (-) | |
| pipe length (m) | |
| Nu | Nusselt number (-) |
| dimensionless pressure () (-) | |
| pressure (Pa) | |
| Prandtl number (-) | |
| q | heat flux magnitude (W/m2) |
| r | position vector (m) |
| Reynolds number (-) | |
| Ri | Richardson number (-) |
| T | temperature (K) |
| dimensionless flow velocity vector (, ) (-) | |
| flow velocity vector (m/s) | |
| u | velocity magnitude (m/s) |
| y | dimensionless radial distance (y-coordinate) (-) |
| dimensionless axial distance (z-coordinate) (-) | |
| Greek symbols | |
| thermal diffusivity (m2/s) | |
| thermal expansion coefficient (1/K) | |
| dimensionless thermal expansion coefficient (-) | |
| dimensionless temperature ( ) (-) | |
| N | dimensionless kinematic viscosity (-) |
| magnetic permeability (H/m) | |
| fluid kinematic viscosity (m2/s) | |
| density (kg/m3) | |
| dimensionless density () (-) | |
| volumetric magnetic susceptibility (-) | |
| dimensionless vorticity magnitude () (-) | |
| vorticity magnitude (1/s) | |
| Subscripts | |
| 0 | reference value |
| b | bulk |
| C | cold (inlet) fluid |
| center | center |
| coil | coil |
| f | fluid |
| H | heated (hot) wall |
| m | magnetic |
| max | maximal |
| n | normal |
| p | pipe |
| v | vacuum |
References
- Braithwaite, D.; Beaugnon, E.; Tournier, R. Magnetically controlled convection in a paramagnetic fluid. Nature 1991, 354, 134–136. [Google Scholar] [CrossRef]
- Tagawa, T.; Ujihara, A.; Ozoe, H. Numerical computation for Rayleigh–Benard convection of water in a magnetic field. Int. J. Heat Mass Transf. 2003, 46, 4097–4104. [Google Scholar] [CrossRef]
- Tagawa, T.; Shigemitsu, R.; Ozoe, H. Magnetizing force modeled and numerically solved for natural convection of air in a cubic enclosure: Effect of the direction of the magnetic field. Int. J. Heat Mass Transf. 2002, 45, 267–277. [Google Scholar] [CrossRef]
- Fornalik-Wajs, E.; Filar, P.; Wajs, J.; Roszko, A.; Pleskacz, L.; Ozoe, H. Flow structure, heat transfer and scaling analysis in the case of thermo-magnetic convection in a differentially heated cylindrical enclosure. In Proceedings of the Journal of Physics: Conference Series, Krakow, Poland, 15–18 June 2014; Volume 530. [Google Scholar]
- Wrobel, W.A.; Fornalik-Wajs, E.; Szmyd, J.S. Analysis of the influence of a strong magnetic field gradient on convection process of paramagnetic fluid in the annulus between horizontal concentric cylinders. J. Phys. Conf. Ser. 2012, 395, 012124. [Google Scholar] [CrossRef]
- Kenjereš, S.; Fornalik-Wajs, E.; Wrobel, W.; Szmyd, J.S. Inversion of flow and heat transfer of the paramagnetic fluid in a differentially heated cube. Int. J. Heat Mass Transf. 2020, 151, 119407. [Google Scholar] [CrossRef]
- Fornalik-Wajs, E.; Roszko, A.; Donizak, J.; Kraszewska, A. Comparison of the experimental and numerical analyses of silver nanofluid under influence of strong magnetic field. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 3139–3162. [Google Scholar] [CrossRef]
- Fornalik-Wajs, E.; Roszko, A.; Donizak, J. Nanofluid flow driven by thermal and magnetic forces–Experimental and numerical studies. Energy 2020, 201, 117658. [Google Scholar] [CrossRef]
- Kraszewska, A.; Pyrda, L.; Donizak, J. Experimental analysis of enclosure aspect ratio influence on thermo-magnetic convection heat transfer. J. Phys. Conf. Ser. 2016, 745, 032153. [Google Scholar] [CrossRef]
- Zhang, D.; Peng, H.; Ling, X. Lattice Boltzmann method for thermomagnetic convection and entropy generation of paramagnetic fluid in porous enclosure under magnetic quadrupole field. Int. J. Heat Mass Transf. 2018, 127, 224–236. [Google Scholar] [CrossRef]
- Wada, K.; Kaneda, M.; Suga, K. Rayleigh-Bénard convection of paramagnetic liquid under a magnetic field from permanent magnets. Symmetry 2020, 12, 341. [Google Scholar] [CrossRef] [Green Version]
- Kaneda, M.; Fujiwara, H.; Wada, K.; Suga, K. Natural convection of paramagnetic fluid along a vertical heated wall under a magnetic field from a single permanent magnet. J. Magn. Magn. Mater. 2020, 502, 166574. [Google Scholar] [CrossRef]
- Song, K.; Wu, S.; Tagawa, T.; Shi, W.; Zhao, S. Thermomagnetic convection of paramagnetic gas in an enclosure under no gravity condition. Fluids 2019, 4, 49. [Google Scholar] [CrossRef] [Green Version]
- Furuichi, Y.; Tagawa, T. Numerical study of the magnetic damping effect on the sloshing of liquid oxygen in a propellant tank. Fluids 2020, 5, 88. [Google Scholar] [CrossRef]
- He, W.; Qin, G.; Wen, X.; Lin, J.; Jia, C. Resonant enhancement of thermomagnetic convection of paramagnetic fluid in an enclosure due to time-periodic magnetizing force. Numer. Heat Transf. Part A Appl. 2020, 78, 1–17. [Google Scholar] [CrossRef]
- Bahiraei, M.; Hangi, M. Automatic cooling by means of thermomagnetic phenomenon of magnetic nanofluid in a toroidal loop. Appl. Therm. Eng. 2016, 107, 700–708. [Google Scholar] [CrossRef]
- Ozoe, H. Magnetothermal wind tunnel. In Magnetic Convection, 1st ed.; Imperial College Press: London, UK, 2005; pp. 193–204. ISBN 10: 1-86094-578-3. [Google Scholar]
- Pleskacz, L.; Fornalik-Wajs, E. Low Reynolds number flow’s heat transfer influenced by strong magnetic field. J. Phys. Conf. Ser. 2016, 745, 032156. [Google Scholar] [CrossRef]
- Pleskacz, Ł.; Fornalik-Wajs, E. Identification of the structures for low Reynolds number flow in the strong magnetic field. Fluids 2019, 4, 36. [Google Scholar] [CrossRef] [Green Version]
- Graetz, L. Ueber die Wärmeleitungsfähigkeit von Flüssigkeiten. Ann. Phys. 1882, 254, 79–94. [Google Scholar] [CrossRef]
- Jackson, J.D. Classical Electrodynamics, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1975. [Google Scholar]



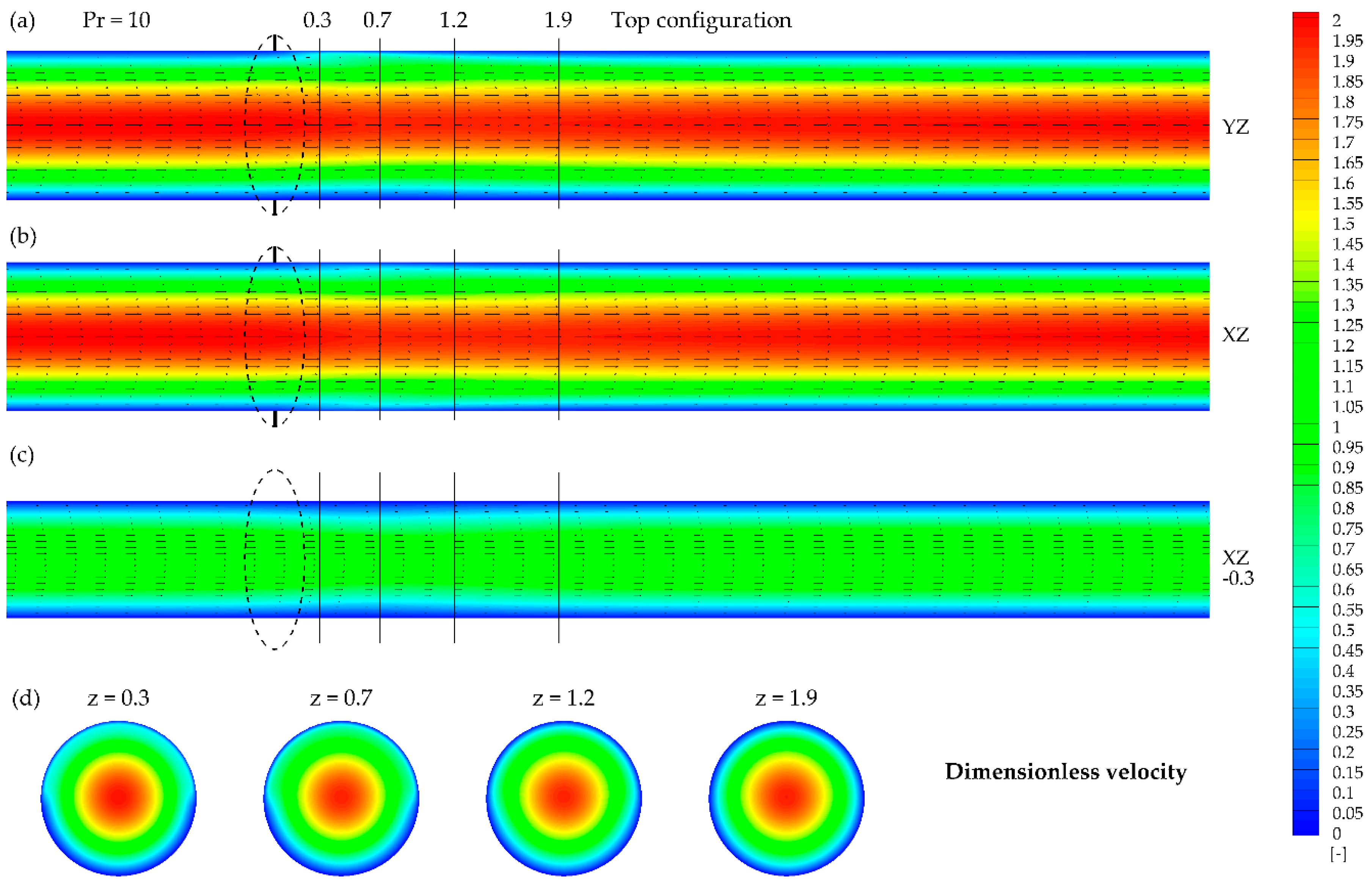


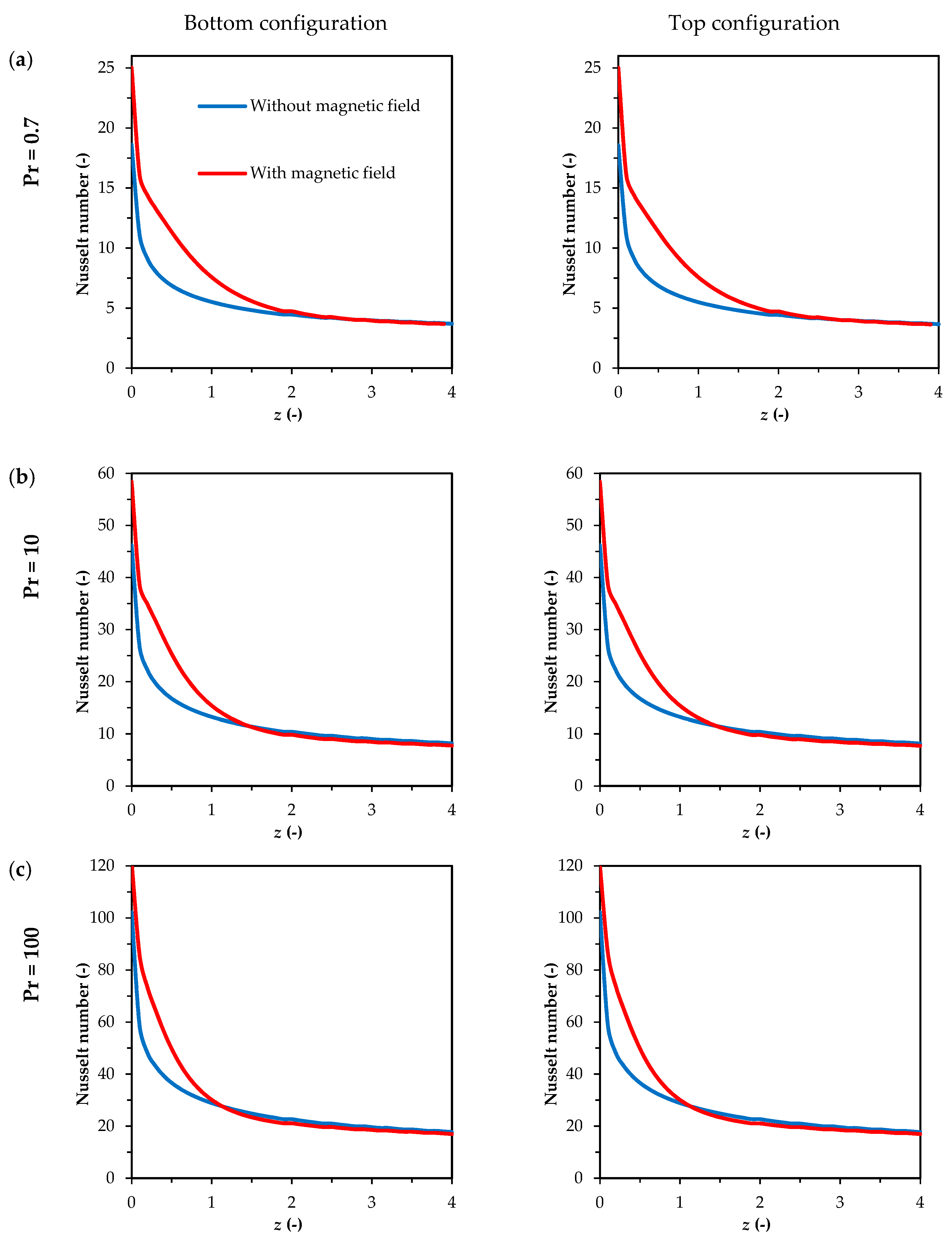
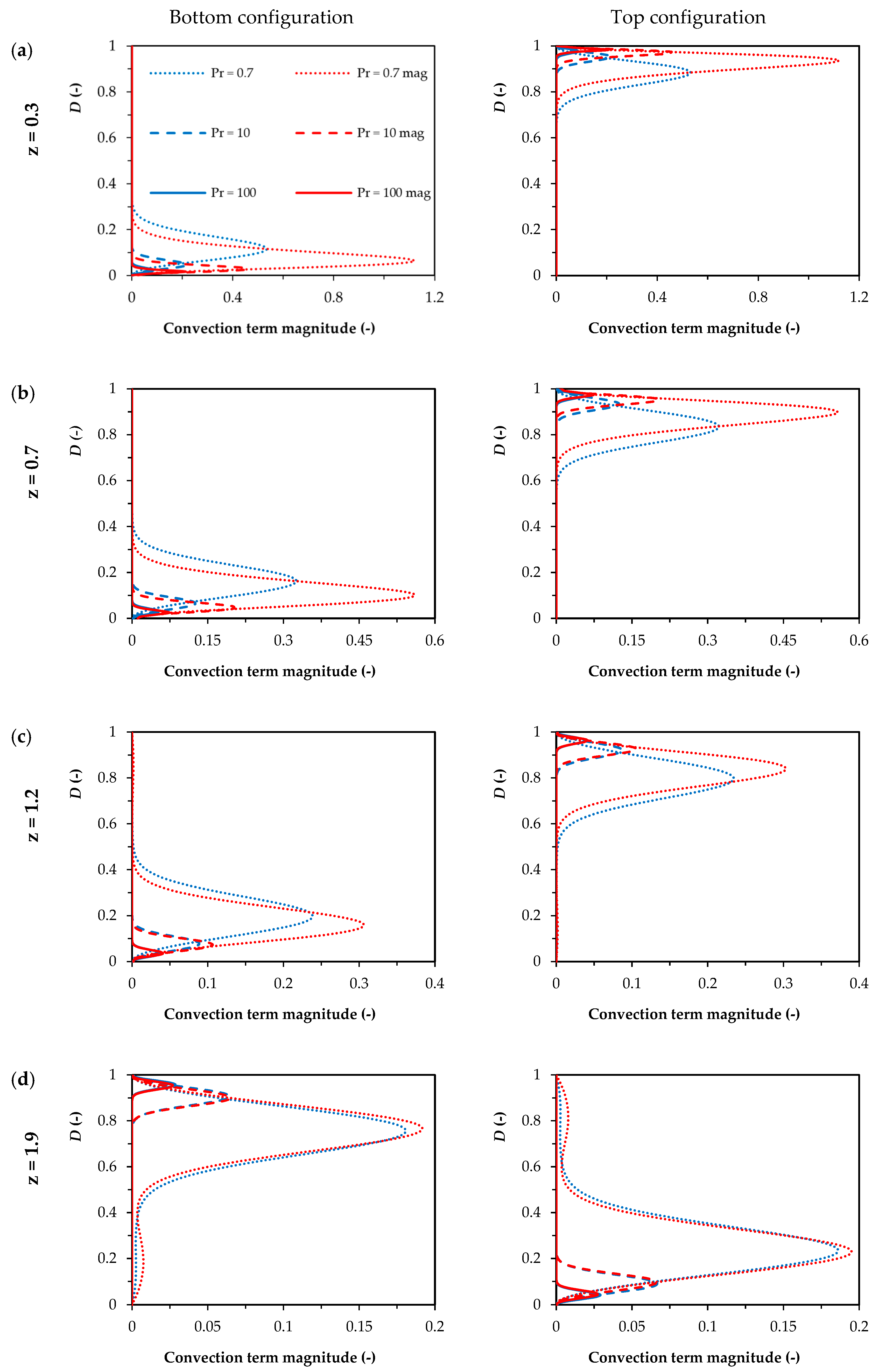
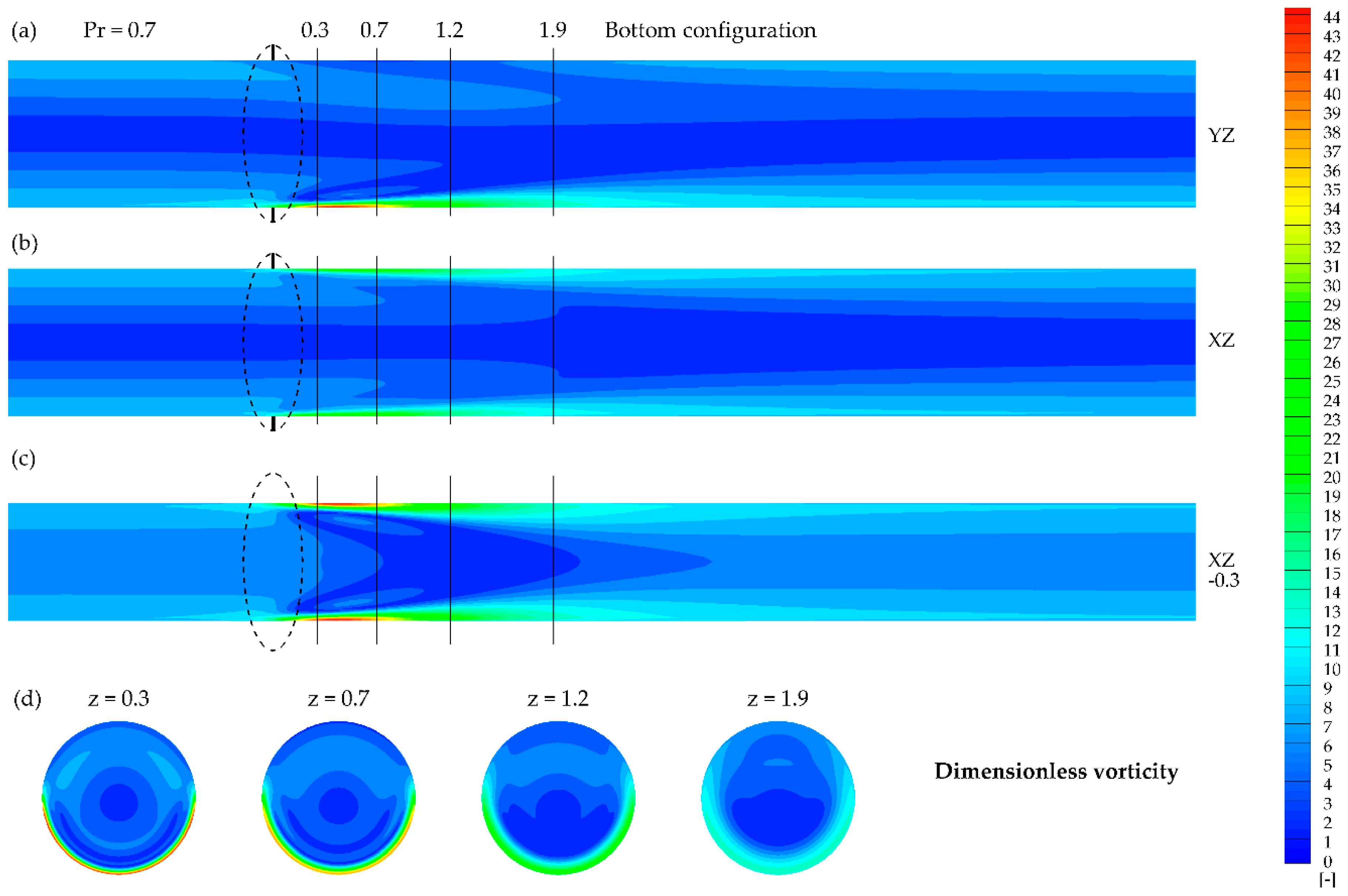
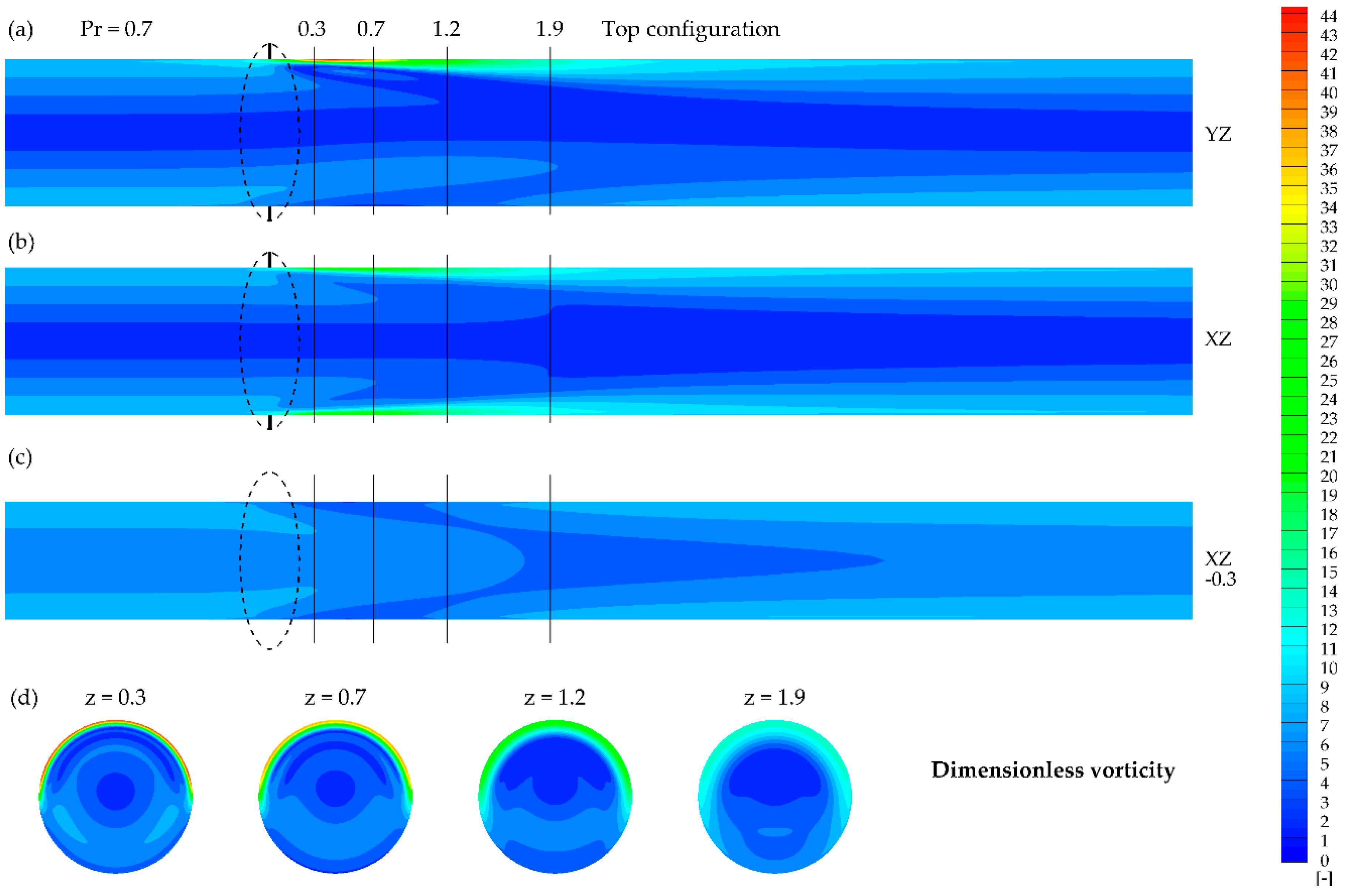
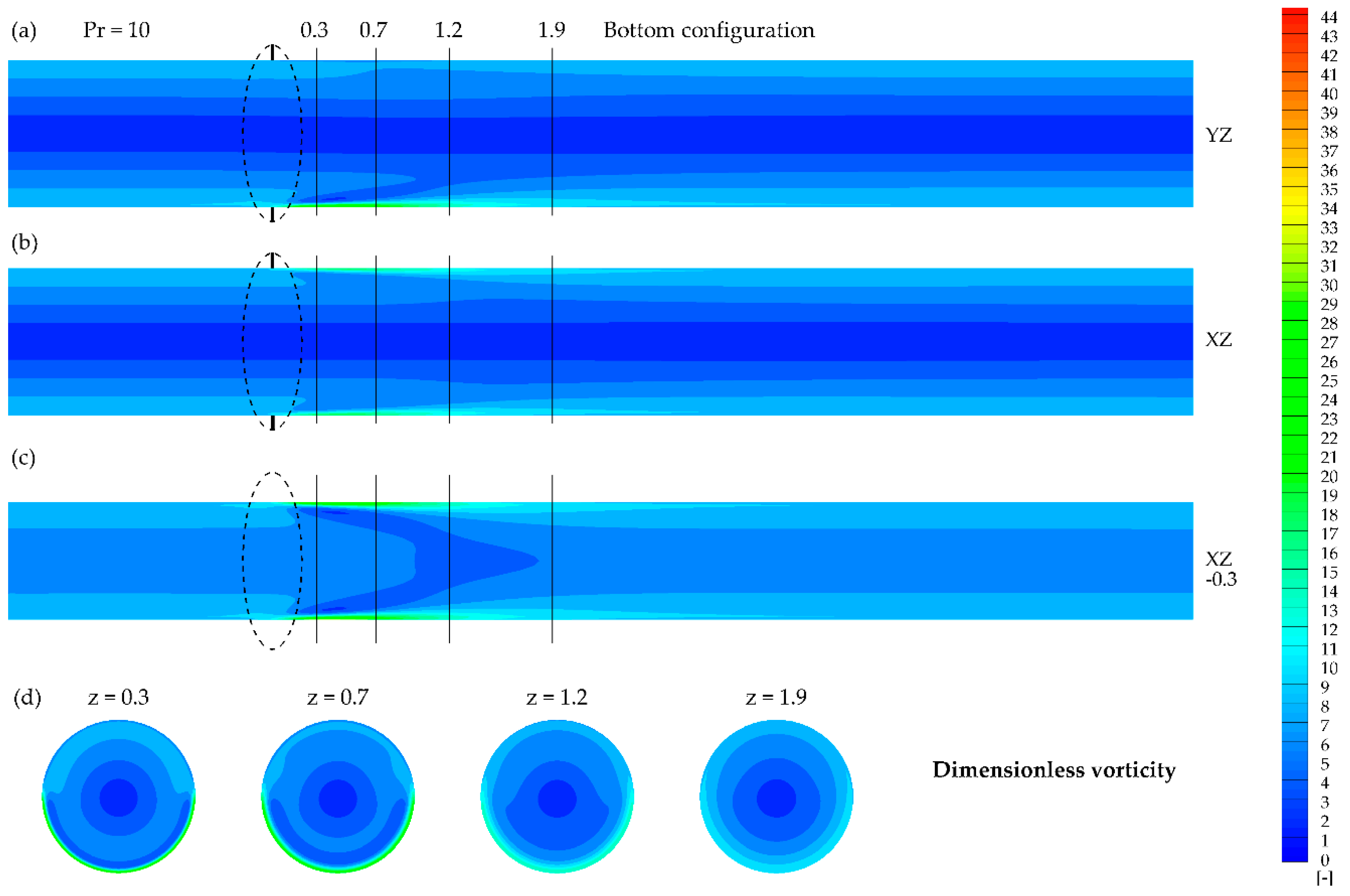
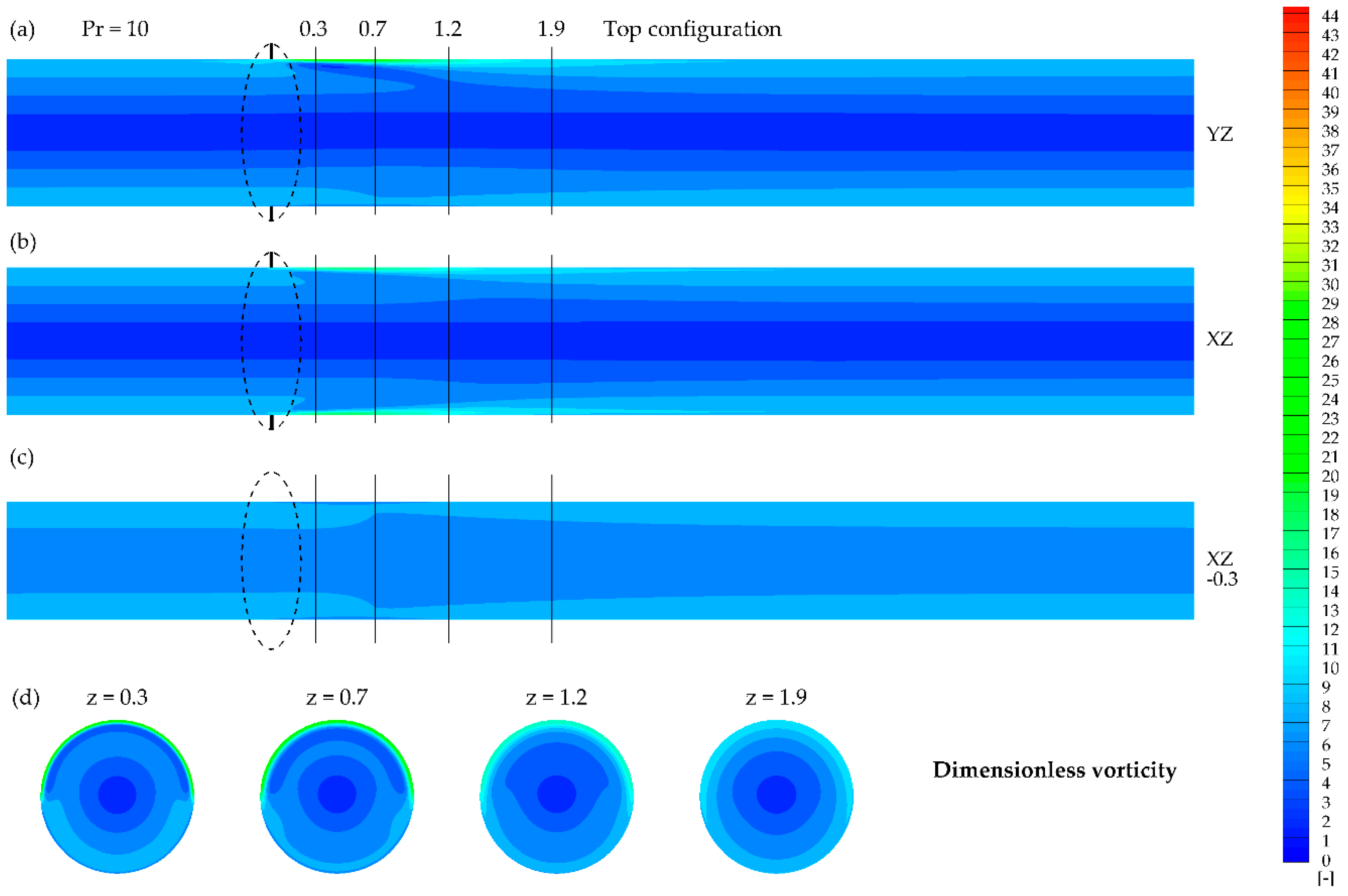

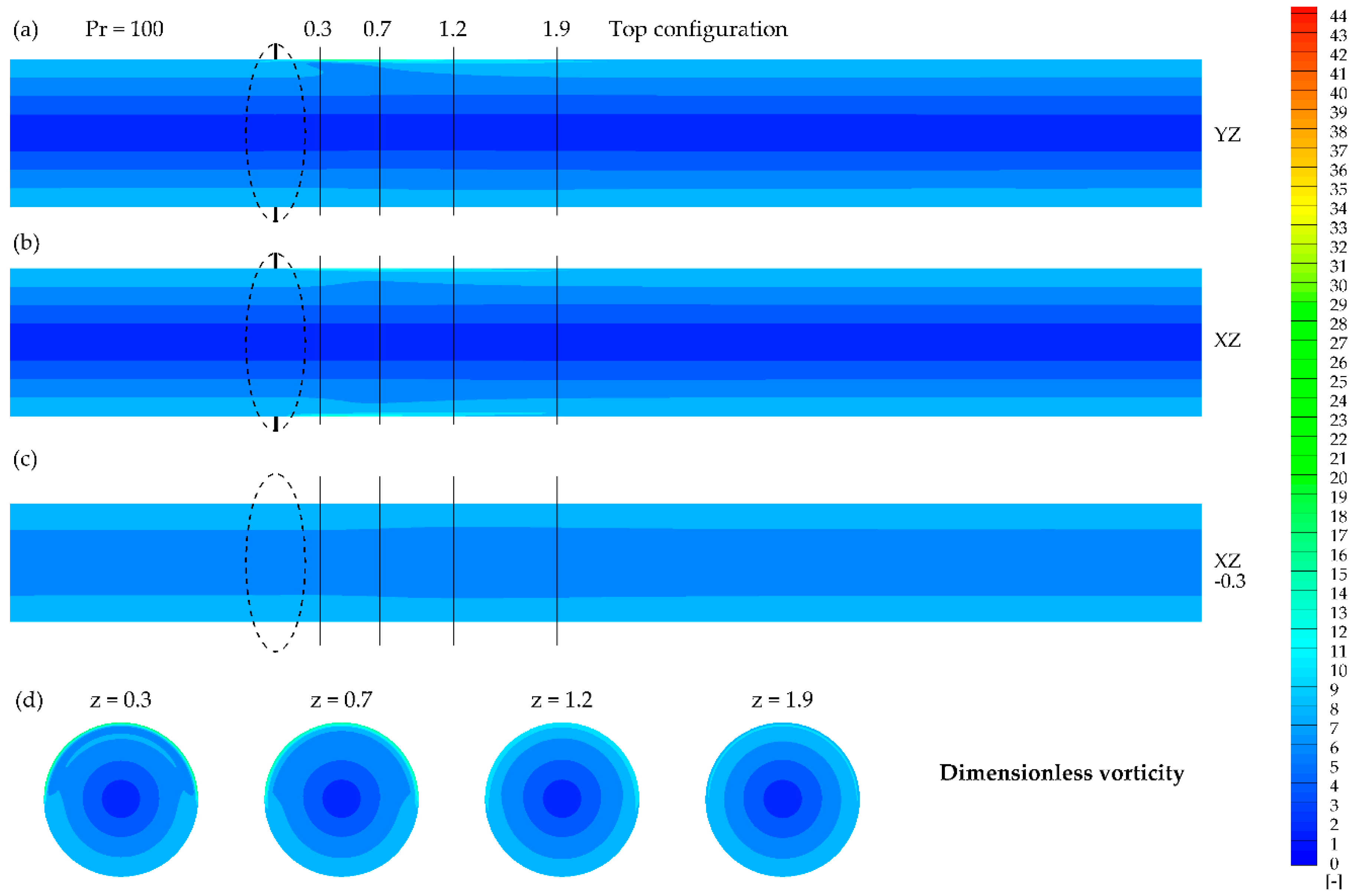
| Property | Symbol | Unit | Value |
|---|---|---|---|
| Pipe diameter | dp | m | 0.01 |
| Coil diameter | dcoil | m | 0.02 |
| Density | ρ0 | kg/m3 | 1.225 |
| Kinematic viscosity | ν0 | m2/s | 1.461 × 10−5 |
| Specific heat | cp | J/(kg·K) | 1006.43 |
| Thermal conductivity | k0 | W/(m·K) | 2.42 × 10−2 |
| Thermal expansion coefficient | β0 | K−1 | 3.33 × 10−3 |
| Volumetric magnetic susceptibility | χ0 | (-) | 3.77 × 10−7 |
| Magnetic permeability of the vacuum | μv | H/m | 4π × 10−7 |
| Electric current | i | A | 159,155 |
| Magnetic induction in the center of the system | bcenter | T | 10 |
| Pr | (Nu–Nu0)/Nu0 (%) | |
|---|---|---|
| Bottom | Top | |
| 0.7 | 24.48 | 24.73 |
| 10 | 14.94 | 14.91 |
| 100 | 9.82 | 9.82 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pleskacz, L.; Fornalik-Wajs, E.; Gurgul, S. Asymmetrical Thermal Boundary Condition Influence on the Flow Structure and Heat Transfer Performance of Paramagnetic Fluid-Forced Convection in the Strong Magnetic Field. Fluids 2020, 5, 246. https://doi.org/10.3390/fluids5040246
Pleskacz L, Fornalik-Wajs E, Gurgul S. Asymmetrical Thermal Boundary Condition Influence on the Flow Structure and Heat Transfer Performance of Paramagnetic Fluid-Forced Convection in the Strong Magnetic Field. Fluids. 2020; 5(4):246. https://doi.org/10.3390/fluids5040246
Chicago/Turabian StylePleskacz, Lukasz, Elzbieta Fornalik-Wajs, and Sebastian Gurgul. 2020. "Asymmetrical Thermal Boundary Condition Influence on the Flow Structure and Heat Transfer Performance of Paramagnetic Fluid-Forced Convection in the Strong Magnetic Field" Fluids 5, no. 4: 246. https://doi.org/10.3390/fluids5040246
APA StylePleskacz, L., Fornalik-Wajs, E., & Gurgul, S. (2020). Asymmetrical Thermal Boundary Condition Influence on the Flow Structure and Heat Transfer Performance of Paramagnetic Fluid-Forced Convection in the Strong Magnetic Field. Fluids, 5(4), 246. https://doi.org/10.3390/fluids5040246





