Collapse Dynamics of Vortex Beams in a Kerr Medium with Refractive Index Modulation and PT-Symmetric Lattices
Abstract
:1. Introduction
2. Theoretical Model
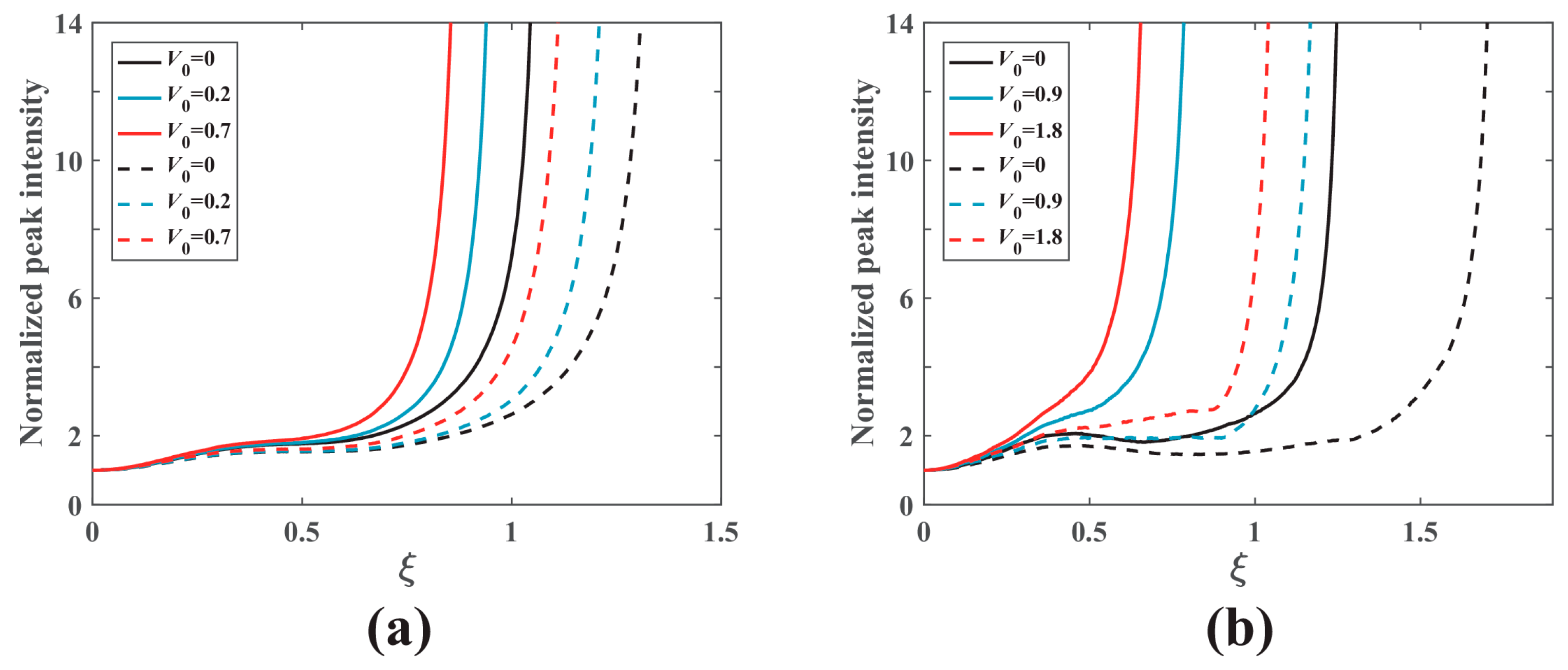
3. Numerical Simulation and Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bender, C.M.; Boettcher, S. Real spectra in non-Hermitian Hamiltonians having PT symmetry. Phys. Rev. Lett. 1998, 80, 5243. [Google Scholar] [CrossRef] [Green Version]
- Bender, C.M.; Dunne, G.V.; Meisinger, P.N. Complex periodic potentials with real band spectra. Phys. Lett. A 1999, 252, 272–276. [Google Scholar] [CrossRef] [Green Version]
- El-Ganainy, R.; Makris, K.; Christodoulides, D.; Musslimani, Z.H. Theory of coupled optical PT-symmetric structures. Opt. Lett. 2007, 32, 2632–2634. [Google Scholar] [CrossRef] [PubMed]
- Makris, K.G.; El-Ganainy, R.; Christodoulides, D.; Musslimani, Z.H. Beam dynamics in PT symmetric optical lattices. Phys. Rev. Lett. 2008, 100, 103904. [Google Scholar] [CrossRef] [PubMed]
- Klaiman, S.; Günther, U.; Moiseyev, N. Visualization of branch points in PT-symmetric waveguides. Phys. Rev. Lett. 2008, 101, 80402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schwarz, L.; Cartarius, H.; Musslimani, Z.H.; Main, J.; Wunner, G. Vortices in Bose-Einstein condensates with PT-symmetric gain and loss. Phys. Rev. A 2017, 95, 53613. [Google Scholar] [CrossRef] [Green Version]
- Zhu, X.; Yang, F.; Cao, S.; Xie, J.; He, Y. Multipole gap solitons in fractional Schrödinger equation with parity-time-symmetric optical lattices. Opt. Express 2020, 28, 1631–1639. [Google Scholar] [CrossRef]
- Hu, S.; Ma, X.; Lu, D.; Yang, Z.; Zheng, Y.; Hu, W. Solitons supported by complex PT-symmetric Gaussian potentials. Phys. Rev. A 2011, 84, 43818. [Google Scholar] [CrossRef] [Green Version]
- Fan, Z.; Malomed, B.A. Dynamical control of solitons in a parity-time-symmetric coupler by periodic management. Commun. Nonlinear Sci. Numer. Simul. 2019, 79, 104906. [Google Scholar] [CrossRef] [Green Version]
- Chen, Y.; Yan, Z.; Mihalache, D. Soliton formation and stability under the interplay between parity-time-symmetric generalized Scarf-II potentials and Kerr nonlinearity. Phys. Rev. E 2020, 102, 12216. [Google Scholar] [CrossRef] [PubMed]
- Yao, G.; Chew, K.H.; Wu, Y.; Li, Y.; Chen, R.P. Propagation dynamics of vector vortex beams in a strongly nonlocal nonlinear medium with parity-time-symmetric potentials. J. Opt. 2022, 24, 35606. [Google Scholar] [CrossRef]
- Chen, R.P.; Chew, K.H.; Zhou, G.; Dai, C.Q.; He, S. Vectorial effect of hybrid polarization states on the collapse dynamics of a structured optical field. Opt. Express 2016, 24, 28143–28153. [Google Scholar] [CrossRef] [PubMed]
- Lan, J.; Yu, C.; Liu, Y.; Feng, Z.; Fu, L.; Liu, J. Effects of delayed Kerr nonlinearity on the propagation of femtosecond annular Gaussian filaments in air. Phys. Scr. 2019, 94, 105225. [Google Scholar] [CrossRef]
- Thul, D.; Richardson, M.; Rostami Fairchild, S. Spatially resolved filament wavefront dynamics. Sci. Rep. 2020, 10, 8920. [Google Scholar] [CrossRef] [PubMed]
- Bergé, L. Wave collapse in physics: Principles and applications to light and plasma waves. Phys. Rep. 1998, 303, 259–370. [Google Scholar] [CrossRef]
- Bergé, L.; Gouédard, C.; Schjødt-Eriksen, J.; Ward, H. Filamentation patterns in Kerr media vs. beam shape robustness, nonlinear saturation and polarization states. Phys. D 2003, 176, 181–211. [Google Scholar] [CrossRef]
- Kolesik, M.; Wright, E.; Moloney, J. Femtosecond filamentation in air and higher-order nonlinearities. Opt. Lett. 2010, 35, 2550–2552. [Google Scholar] [CrossRef] [Green Version]
- Lü, J.Q.; Li, P.P.; Wang, D.; Tu, C.; Li, Y.; Wang, H.T. Control on helical filaments by twisted beams in a nonlinear CS2 medium. Opt. Express 2018, 26, 29527–29538. [Google Scholar] [CrossRef] [PubMed]
- Chen, R.P.; Yin, C.F.; Chu, X.X.; Wang, H. Effect of Kerr nonlinearity on an Airy beam. Phys. Rev. A 2010, 82, 43832. [Google Scholar] [CrossRef]
- Dubietis, A.; Tamosauskas, G.; Fibich, G.; Ilan, B. Multiple filamentation induced by input-beam ellipticity. Opt. Lett. 2004, 29, 1126–1128. [Google Scholar] [CrossRef] [Green Version]
- Jin, Z.; Zhang, J.; Xu, M.; Lu, X.; Li, Y.; Wang, Z.; Wei, Z.; Yuan, X.; Yu, W. Control of filamentation induced by femtosecond laser pulses propagating in air. Opt. Express 2005, 13, 10424–10430. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Zeng, J.; Lu, X.; Wang, Z.; Zhao, C.; Cai, Y. Review on fractional vortex beam. Nanophotonics 2022, 11, 241–273. [Google Scholar] [CrossRef]
- Berkhout, G.C.; Lavery, M.P.; Courtial, J.; Beijersbergen, M.W.; Padgett, M.J. Efficient sorting of orbital angular momentum states of light. Phys. Rev. Lett. 2010, 105, 153601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, Y.; Zhao, Q.; Liu, L.; Liu, Y.; Rosales-Guzmán, C.; Qiu, C.W. Manipulation of orbital-angular-momentum spectrum using pinhole plates. Phys. Rev. Appl. 2019, 12, 64007. [Google Scholar] [CrossRef]
- Bai, Y.; Lv, H.; Fu, X.; Yang, Y. Vortex beam: Generation and detection of orbital angular momentum. Chin. Opt. Lett. 2022, 20, 12601. [Google Scholar] [CrossRef]
- Dholakia, K.; Čižmár, T. Shaping the future of manipulation. Nat. Photonics 2011, 5, 335–342. [Google Scholar] [CrossRef]
- Yang, Y.; Ren, Y.; Chen, M.; Arita, Y.; Rosales-Guzmán, C. Optical trapping with structured light: A review. Adv. Photonics 2021, 3, 34001. [Google Scholar] [CrossRef]
- Padgett, M.; Bowman, R. Tweezers with a twist. Nat. Photonics 2011, 5, 343–348. [Google Scholar] [CrossRef]
- Paterson, L.; MacDonald, M.P.; Arlt, J.; Sibbett, W.; Bryant, P.; Dholakia, K. Controlled rotation of optically trapped microscopic particles. Science 2001, 292, 912–914. [Google Scholar] [CrossRef]
- Li, Z.X.; Ruan, Y.P.; Chen, P.; Tang, J.; Hu, W.; Xia, K.Y.; Lu, Y.Q. Liquid crystal devices for vector vortex beams manipulation and quantum information applications. Chin. Opt. Lett. 2021, 19, 112601. [Google Scholar] [CrossRef]
- Zeng, R.; Zhao, Q.; Shen, Y.; Liu, Y.; Yang, Y. Structural stability of open vortex beams. Appl. Phys. Lett. 2021, 119, 171105. [Google Scholar] [CrossRef]
- Song, L.; Yang, Z.; Zhang, S.; Li, X. Spiraling anomalous vortex beam arrays in strongly nonlocal nonlinear media. Phys. Rev. A 2019, 99, 63817. [Google Scholar] [CrossRef]
- Kruglov, V.; Logvin, Y.A.; Volkov, V. The theory of spiral laser beams in nonlinear media. J. Mod. Opt. 1992, 39, 2277–2291. [Google Scholar] [CrossRef]
- Fibich, G.; Gavish, N. Critical power of collapsing vortices. Phys. Rev. A 2008, 77, 45803. [Google Scholar] [CrossRef] [Green Version]
- Chen, R.P.; Zhong, L.X.; Chew, K.H.; Zhao, T.Y.; Zhang, X. Collapse dynamics of a vector vortex optical field with inhomogeneous states of polarization. Laser Phys. 2015, 25, 75401. [Google Scholar] [CrossRef]
- Perez-Garcia, V.M.; Torres, P.J.; Montesinos, G.D. The method of moments for nonlinear Schrödinger equations: Theory and applications. SIAM J. Appl. Math. 2007, 67, 990–1015. [Google Scholar] [CrossRef] [Green Version]
- Chen, R.P.; Chew, K.H.; He, S. Dynamic control of collapse in a vortex Airy beam. Sci. Rep. 2013, 3, 1406. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fibich, G.; Gaeta, A.L. Critical power for self-focusing in bulk media and in hollow waveguides. Opt. Lett. 2000, 25, 335–337. [Google Scholar] [CrossRef] [Green Version]
- Chiao, R.Y.; Garmire, E.; Townes, C.H. Self-trapping of optical beams. Phys. Rev. Lett. 1964, 13, 479. [Google Scholar] [CrossRef]
- Polynkin, P.; Ament, C.; Moloney, J.V. Self-focusing of ultraintense femtosecond optical vortices in air. Phys. Rev. Lett. 2013, 111, 23901. [Google Scholar] [CrossRef]
- Meyer, H.J.; Mamani, S.; Alfano, R.R. Steady-state stimulated Raman generation and filamentation using complex vector vortex beams. Appl. Opt. 2020, 59, 6245–6251. [Google Scholar] [CrossRef] [PubMed]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, G.; Li, Y.; Chen, R.-P. Collapse Dynamics of Vortex Beams in a Kerr Medium with Refractive Index Modulation and PT-Symmetric Lattices. Photonics 2022, 9, 249. https://doi.org/10.3390/photonics9040249
Yao G, Li Y, Chen R-P. Collapse Dynamics of Vortex Beams in a Kerr Medium with Refractive Index Modulation and PT-Symmetric Lattices. Photonics. 2022; 9(4):249. https://doi.org/10.3390/photonics9040249
Chicago/Turabian StyleYao, Gang, Yuhua Li, and Rui-Pin Chen. 2022. "Collapse Dynamics of Vortex Beams in a Kerr Medium with Refractive Index Modulation and PT-Symmetric Lattices" Photonics 9, no. 4: 249. https://doi.org/10.3390/photonics9040249




