A One Line Derivation of EGARCH
Abstract
:1. Introduction
2. EGARCH
 , ηt ~ iid (0, ω), |β|<1 and is the stability condition when log ht−1 is included in the model. Asymmetry exists if γ ≠ 0, while leverage exists if γ < 0 and γ < α < −γ. The specification in Equation (2) is EGARCH(1,1), but this can easily be extended to EGARCH(p,q).
, ηt ~ iid (0, ω), |β|<1 and is the stability condition when log ht−1 is included in the model. Asymmetry exists if γ ≠ 0, while leverage exists if γ < 0 and γ < α < −γ. The specification in Equation (2) is EGARCH(1,1), but this can easily be extended to EGARCH(p,q). , each of which is a function of the parameters through Equations (1) and (2), it is clear that quasi-maximum likelihood estimation of EGARCH is problematic as |ηt−1| is not differentiable with respect to the parameters. Moreover, invertibility of EGARCH is also problematic because of the presence of the logarithmic transformation as well as the absolute value function.
, each of which is a function of the parameters through Equations (1) and (2), it is clear that quasi-maximum likelihood estimation of EGARCH is problematic as |ηt−1| is not differentiable with respect to the parameters. Moreover, invertibility of EGARCH is also problematic because of the presence of the logarithmic transformation as well as the absolute value function.3. Random Coefficient Complex Nonlinear Moving Average Process
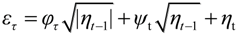
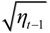 is a complex-valued function of ηt−1, φt ~ iid (0, α), and ψt ~ iid (0, γ).
is a complex-valued function of ηt−1, φt ~ iid (0, α), and ψt ~ iid (0, γ).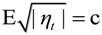 , which is a known constant. Moreover:
, which is a known constant. Moreover:
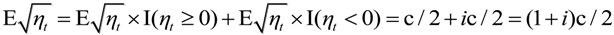
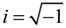 and E[I(ηt ≥ 0)] = E[I(ηt < 0)] = 0.5 are the expectations of two indicator functions. As the mean of the complex-valued function is a finite constant, it follows that both the unconditional and conditional means of εt in Equation (3) are zero.
and E[I(ηt ≥ 0)] = E[I(ηt < 0)] = 0.5 are the expectations of two indicator functions. As the mean of the complex-valued function is a finite constant, it follows that both the unconditional and conditional means of εt in Equation (3) are zero.4. One Line Derivation of EGARCH
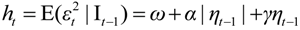
5. Conclusion
Acknowledgments
Author Contributions
Conflicts of Interest
References
- R.F. Engle. “Autoregressive conditional heteroscedasticity with estimates of the variance of United Kingdom inflation.” Econometrica 50 (1982): 987–1007. [Google Scholar] [CrossRef]
- T. Bollerslev. “Generalised autoregressive conditional heteroscedasticity.” J. Econom. 31 (1986): 307–327. [Google Scholar] [CrossRef]
- D.B. Nelson. “ARCH models as diffusion approximations.” J. Econom. 45 (1990): 7–38. [Google Scholar] [CrossRef]
- D.B. Nelson. “Conditional heteroskedasticity in asset returns: A new approach.” Econometrica 59 (1991): 347–370. [Google Scholar]
- L. Glosten, R. Jagannathan, and D. Runkle. “On the relation between the expected value and volatility of nominal excess return on stocks.” J. Financ. 46 (1992): 1779–1801. [Google Scholar]
- F. Black. “Studies of stock market volatility changes. In.” In 1976 Proceedings of the Meetings of the Business and Economic Statistics Section; Washington, DC, USA: American Statistical Association, 1976, pp. 177–181. [Google Scholar]
- Y. Baba, R.F. Engle, D. Kraft, K.F. Kroner, and Department of Economics, University of California, San Diego, CA, USA. “Multivariate Simultaneous Generalized ARCH.” 1985, Unpublished work. [Google Scholar]
- R.F. Engle, and K.F. Kroner. “Multivariate simultaneous generalized ARCH.” Econom. Theory 11 (1995): 122–150. [Google Scholar]
- S. Ling, and M. McAleer. “Asymptotic theory for a vector ARMA-GARCH model.” Econom. Theory 19 (2003): 278–308. [Google Scholar]
- M. McAleer, S. Hoti, and F. Chan. “Structure and asymptotic theory for multivariate asymmetric conditional volatility.” Econom. Rev. 28 (2009): 422–440. [Google Scholar] [CrossRef]
- H. Kawakatsu. “Matrix exponential GARCH.” J. Econom. 134 (2006): 95–128. [Google Scholar]
- D. Straumann, and T. Mikosch. “Quasi-maximum-likelihood estimation in conditionally heteroscedastic time series: A stochastic recurrence equation approach.” Ann. Stat. 34 (2006): 2449–2495. [Google Scholar]
- O. Wintenberger. “Continuous invertibility and stable QML estimation of the EGARCH(1,1) model.” Scand. J. Stat. 40 (2013): 846–867. [Google Scholar] [CrossRef]
- A. Demos, and D. Kyriakopoulou. “Asymptotic Normality of the QMLEs in the EGARCH(1,1) Model.” Available online: http://papers.ssrn.com/sol3/papers.cfm?abstract_id=2236055 (accessed on 14 June 2014).
- T. Marek. “On invertibility of a random coefficient moving average model.” Kybernetika 41 (2005): 743–756. [Google Scholar]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
McAleer, M.; Hafner, C.M. A One Line Derivation of EGARCH. Econometrics 2014, 2, 92-97. https://doi.org/10.3390/econometrics2020092
McAleer M, Hafner CM. A One Line Derivation of EGARCH. Econometrics. 2014; 2(2):92-97. https://doi.org/10.3390/econometrics2020092
Chicago/Turabian StyleMcAleer, Michael, and Christian M. Hafner. 2014. "A One Line Derivation of EGARCH" Econometrics 2, no. 2: 92-97. https://doi.org/10.3390/econometrics2020092
APA StyleMcAleer, M., & Hafner, C. M. (2014). A One Line Derivation of EGARCH. Econometrics, 2(2), 92-97. https://doi.org/10.3390/econometrics2020092





