The Numerical Analysis of Textile Reinforced Concrete Shells: Basic Principles
Abstract
:1. Introduction
2. Workflow Overview
3. Materials Models
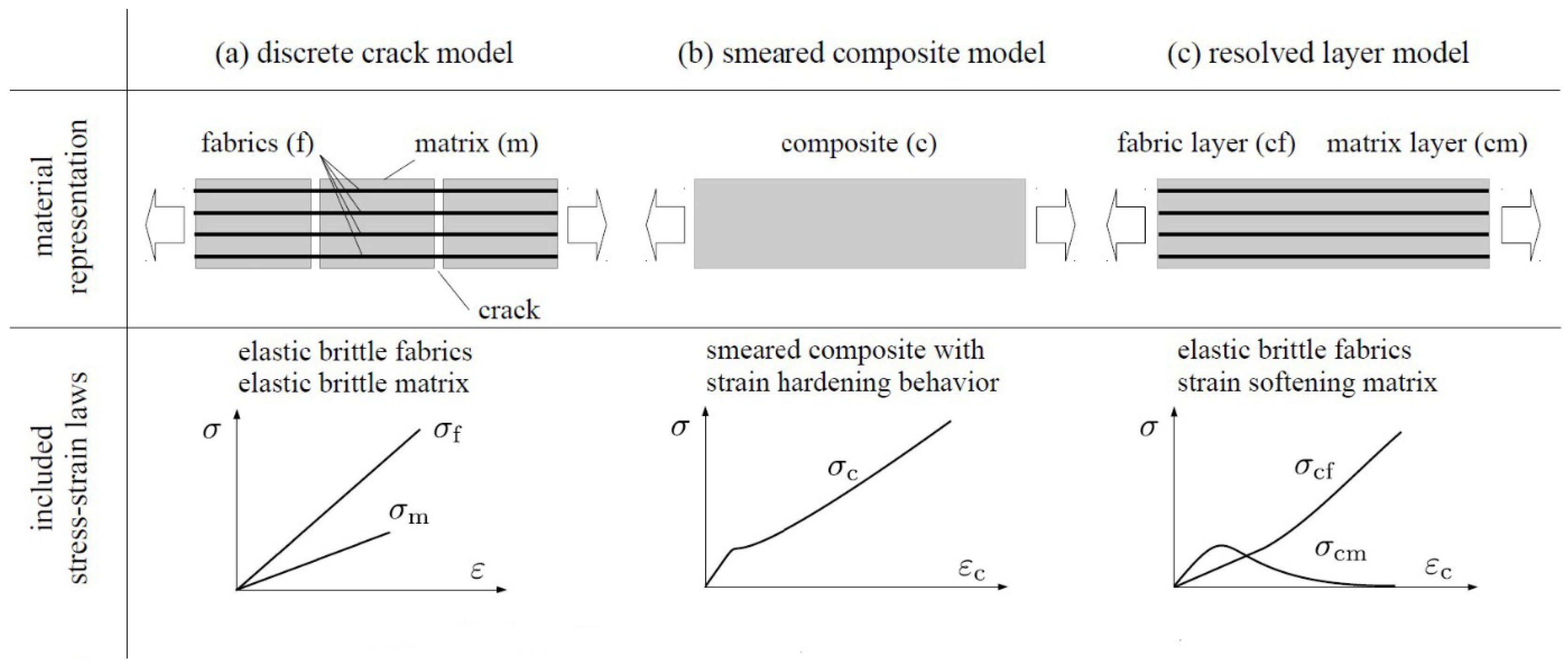
3.1. Short Overview of Material Models for TRC
- The ACK model is named after its creators, Aveston, Cooper, and Kelly, and was published in 1971. The key aspect of the model is that it is one of the most numerically simple and is based on simplified assumptions describing the processes that occur inside the TRC sample under the tensile action [20].
- The trilinear model is based on the approach of three linear, continuously ascending ranges which are adapted from the real stress–strain line of TRC. The slope and other parameters can be determined according to the rule of mixtures, appropriate efficiency factors, and recommendations from Model Code 90 [22,23,24].
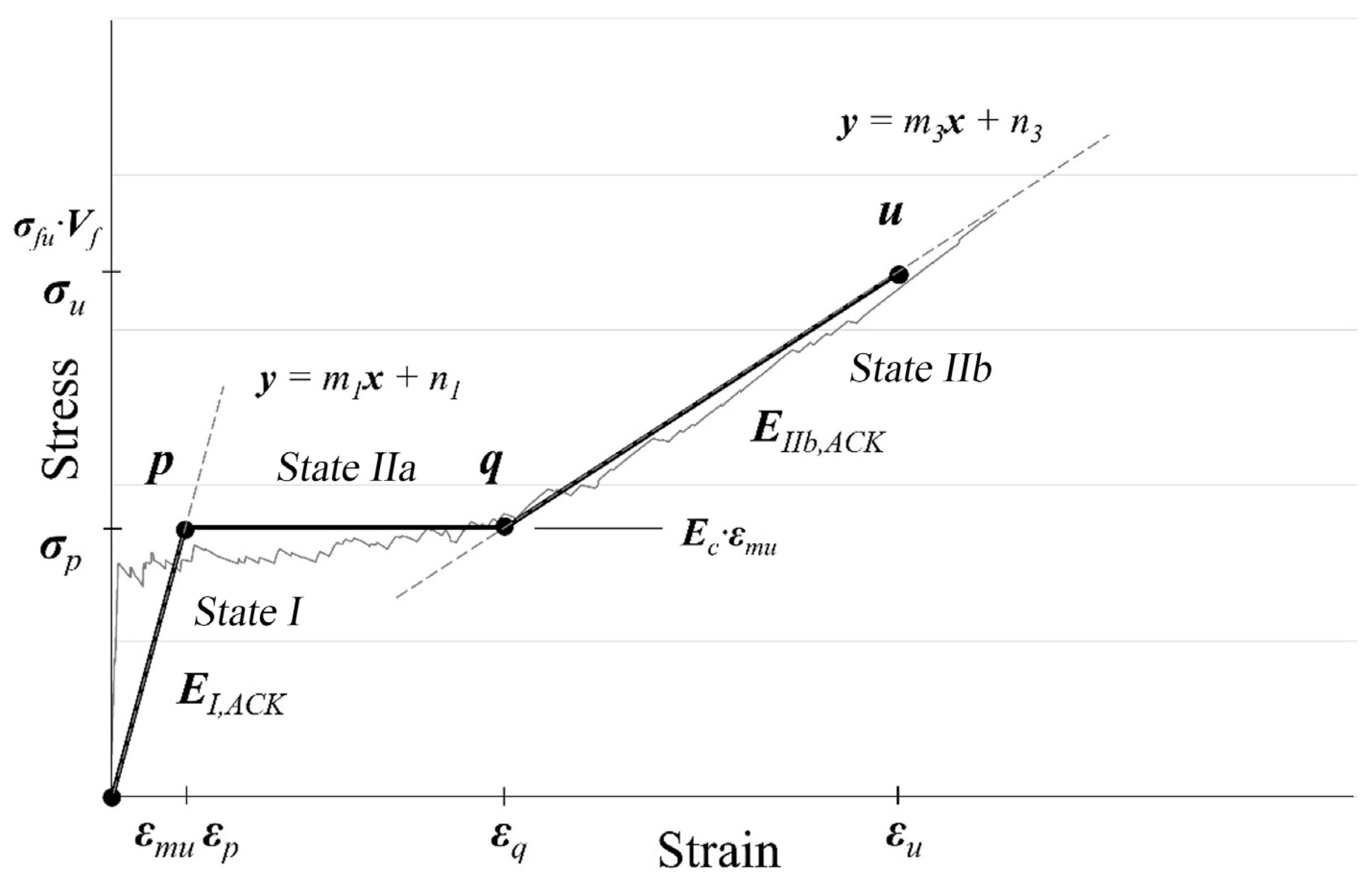
3.2. ACK Material Model
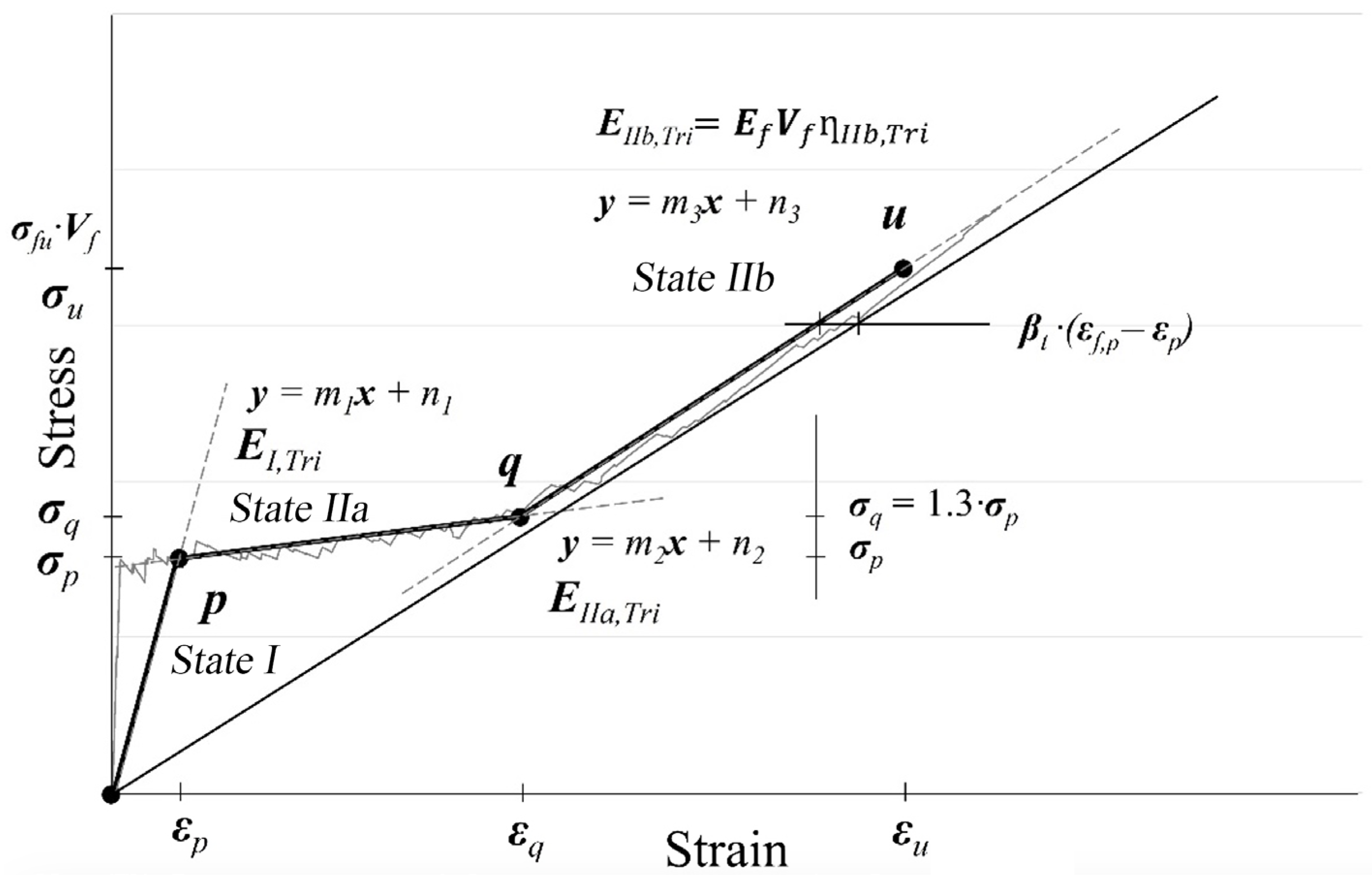
3.3. Trilinear Material Model
3.4. Calibrating the Selected Material Models
4. FEM Model Formulation
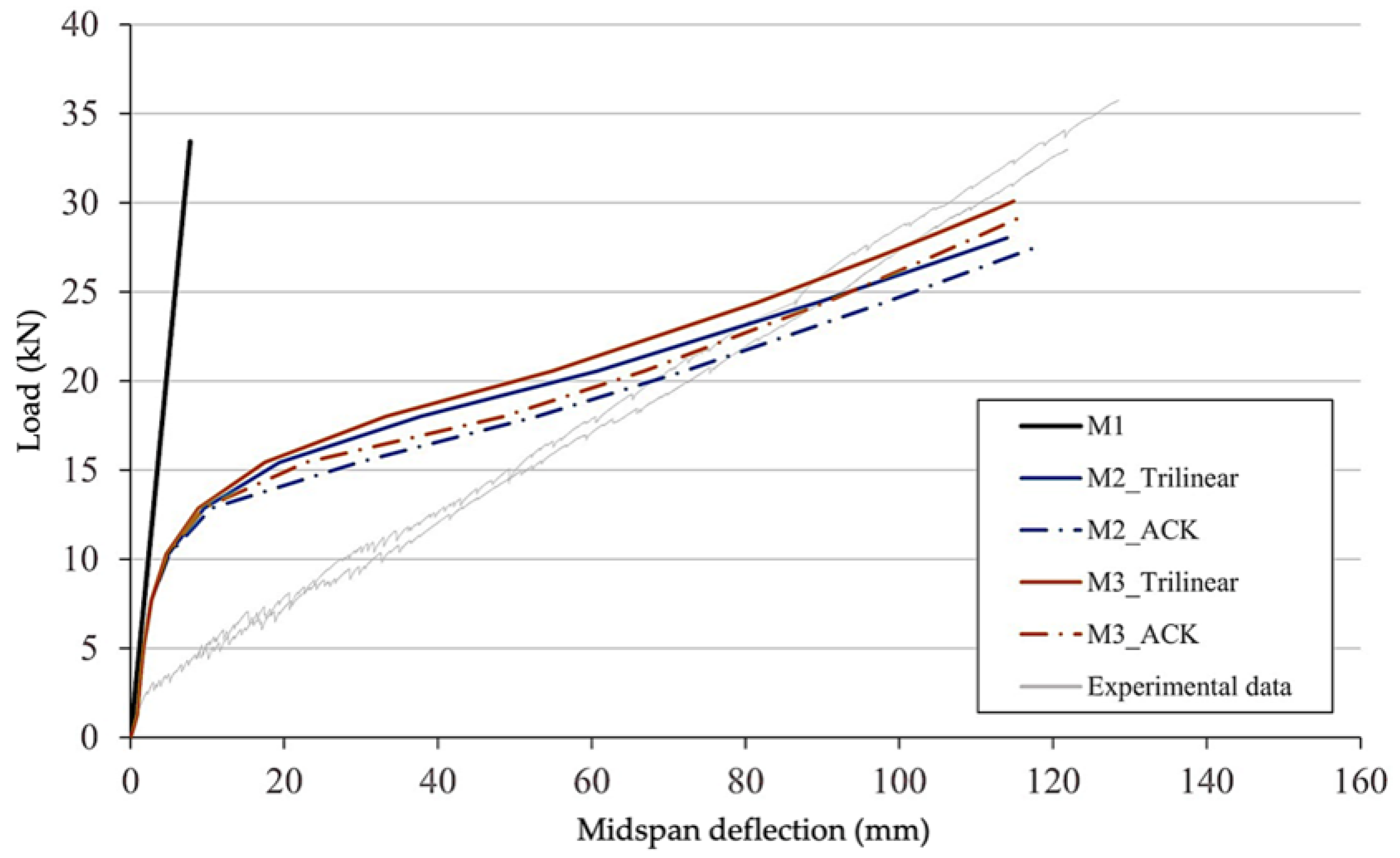
- For a broad representation of the available material models in the RFEM software package (Ver. 5.19), first, a linear elastic material model was considered, called M1 in further discussions. This approach is interesting because it reflects the material model that is considered and uses it as the preinstalled model for the calculation of concrete structures in RFEM. The CUBE project shows that such approaches can be properly used [37]. Here, the force flow over the whole structure was first simulated using the linear material model coupled with an appropriate cross-sectional stiffness. Then, for an appropriate deflection calculation, the cross-section stiffness was reduced.
- The next material model M2 is based on M1 but it is enriched for tension with a plastic zone and a consequent strain-hardening zone. Thus, the model is able to reflect either ACK or trilinear material behaviour as described above. Within the RFEM user interface, it is possible to find an Isotropic Nonlinear Elastic 2D/3D material model which is suitable for the calculation of non-linear materials in surfaces. One of the model’s features is the possibility to provide a stress–strain curve derived from uniaxial TRC tests. A Mohr–Coulomb yield criterion is used and suitable for describing brittle materials such as concrete. The linear envelope based on the yield criteria fits for concrete with a significantly higher compressive than tensile strength. As a result, an asymmetric stress–strain diagram can be used as an input.
- The further development step regarding the material models is M3. It also gives the possibility to model TRC with nonlinear behaviour and is named in the RFEM environment as the Isotropic Damage 2D/3D model. The difference is that the model is based on the assumptions of Mazars’ damage model [38,39]. This approach provides an isotropic description of the damaged state of concrete according to [39]. The used damage function depends on scalar value D that is split into two parts, namely for tension and for compression, that can be determined from uniaxial tests. Such special features make the model attractive to be used for the calculation of TRC structures after the conduction of uniaxial tests. However, it is important to note that Mazars’ model according to the RFEM description [38] was developed for the calculation of materials with strain-softening behaviour like plain or steel fibre concrete. Thus, Mazars’ model does not fit the strain-hardening response of TRC via a smeared approach. Nevertheless, in the present study, the M3 model was used for the comparative simulation of TRC.
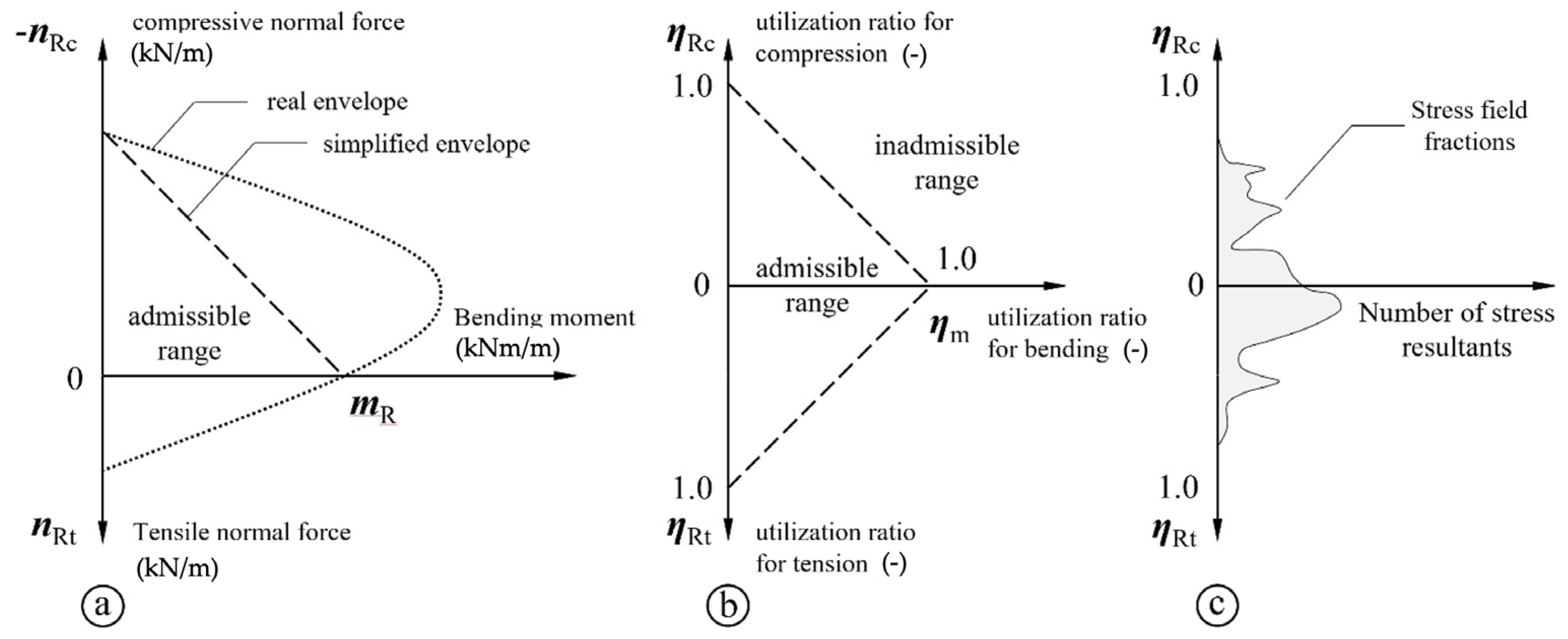
5. Textile Failure Criteria Post-Processing
- In the tension-bending range, linear interpolation gives a relatively good representation of real behaviour.
- In the compression-bending range, the ultimate strength is underestimated by the linear interpolation, staying on the safe side.
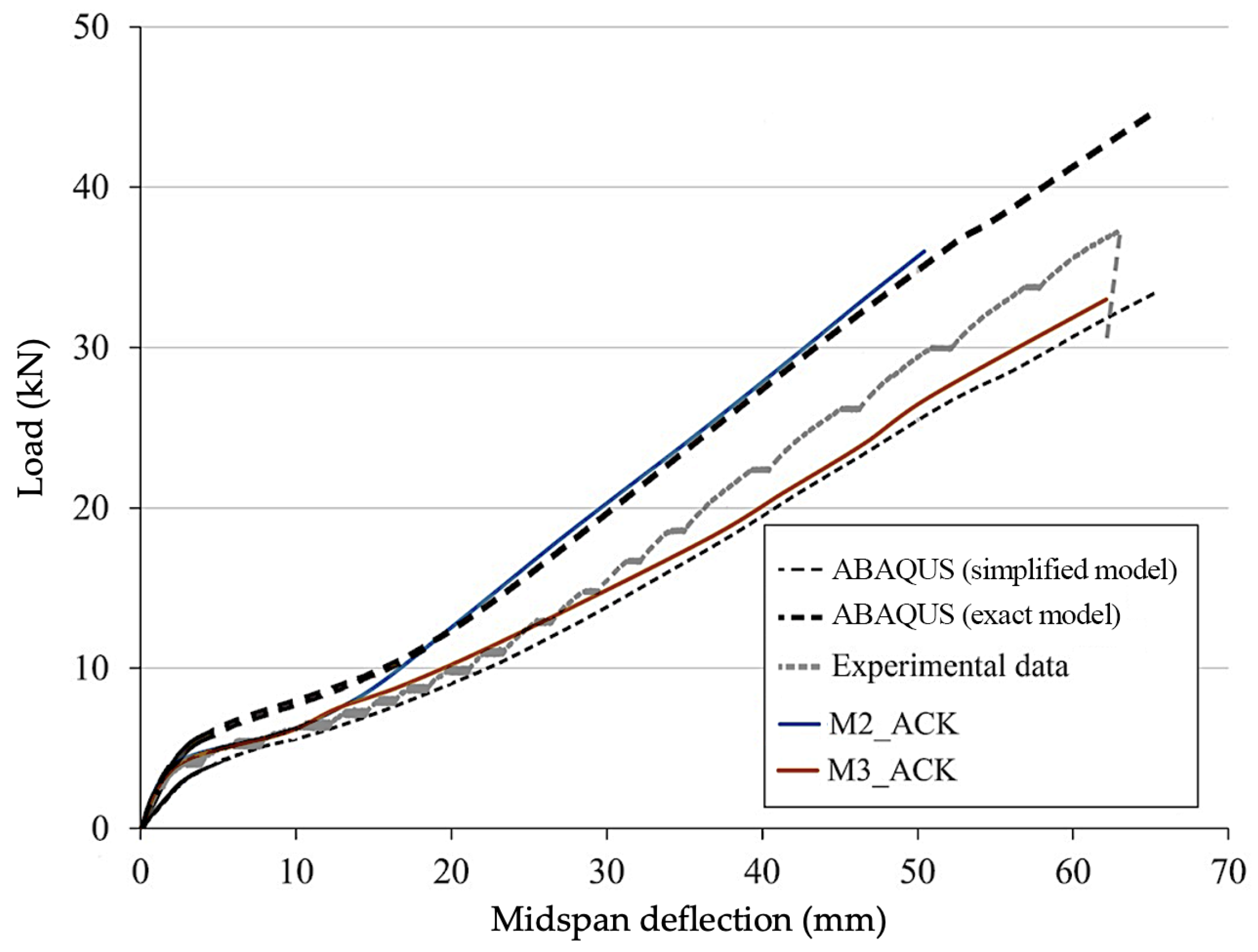
6. Trial-Based Calculation
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bologna, A.; Gargiani, R. The Rhetoric of Pier Luigi Nervi: Forms in Reinforced Concrete and Ferrocement; EPFL Presses: Lausanne, Switzerland, 2016. [Google Scholar]
- Mata-Falcon, J.; Bischof, P.; Huber, T.; Anton, A.; Burger, J.; Ranaudo, F.; Jipa, A.; Gebhard, L.; Reiter, L.; Lloret-Fritschi, E.; et al. Digitally Fabricated Ribbed Concrete Floor Slabs: A Sustainable Solution for Construction. RILEM Tech. Lett. 2022, 7, 68–78. [Google Scholar] [CrossRef]
- Frenzel, M.; Farwig, K.; Curbach, M. Leichte Deckentragwerke aus geschichteten Hochleistungsbetonen | Lightweight Ceiling Structures Made of Layered High-Performance Concrete. In SPP 1542: Leicht Bauen mit Beton. Grundlagen für das Bauen der Zukunft mit Bionischen und Mathematischen Entwurfsprinzipien (Abschlussbericht)|SPP 1542: Concrete Light. Future Concrete Structures Using Bionic, Mathematical and Engineering Formfinding Principles (Final Report); Scheerer, S., Curbach, M., Eds.; Institut für Massivbau der TU Dresden: Dresden, Germany, 2022; pp. 144–169. [Google Scholar] [CrossRef]
- Cuypers, H.; Wastiels, J. Analysis and Verification of the Performance of Sandwich Panels with Textile Reinforced Concrete Faces. J. Sandw. Struct. Mater. 2011, 13, 589–603. [Google Scholar] [CrossRef]
- Frenzel, M. Zum Tragverhalten von Leichten, Geschichteten Betondecken. Ph.D. Dissertation, Technischen Universität Dresden, Dresden, Germany, 2021. Available online: https://nbn-resolving.org/urn:nbn:de:bsz:14-qucosa2-787009 (accessed on 9 December 2023).
- Williams Portal, N.; Flansbjer, M.; Zandi, K.; Wlasak, L.; Malaga, K. Bending Behaviour of Novel Textile Reinforced Concrete-foamed Concrete (TRC-FC) Sandwich Elements. Compos. Struct. 2017, 177, 104–118. [Google Scholar] [CrossRef]
- Schmeer, D.; Sobek, W. Gradientenbeton. In Beton-Kalender 2019; Bergmeister, K., Fingerloos, F., Wörner, J.-D., Eds.; Ernst & Sohn: Berlin, Germany, 2019; pp. 455–476. [Google Scholar] [CrossRef]
- Schmeer, D.; Wörner, M.; Garrecht, K.; Sawodny, O.; Sobek, W. Effiziente automatisierte Herstellung multifunktional gradierter Bauteile mit mineralischen Hohlkörpern|Efficient Automated Production of Multifunctional Graded Components with Mineral Hollow Bodies. In SPP 1542: Leicht Bauen mit Beton. Grundlagen für das Bauen der Zukunft mit Bionischen und Mathematischen Entwurfsprinzipien (Abschlussbericht)|SPP 1542: Concrete Light. Future Concrete Structures Using Bionic, Mathematical and Engineering Formfinding Principles (Final Report); Scheerer, S., Curbach, M., Eds.; Institut für Massivbau der TU Dresden: Dresden, Germany, 2022; pp. 250–282. [Google Scholar] [CrossRef]
- Beckmann, B.; Bielak, J.; Bosbach, S.; Scheerer, S.; Schmidt, C.; Hegger, J.; Curbach, M. Collaborative Research on Carbon Reinforced Concrete Structures in the CRC/TRR 280 Project. Civ. Eng. Des. 2021, 3, 99–109. [Google Scholar] [CrossRef]
- Main Website of Collaborative Research Centre/Transregio 280, (CRC/TRR 280). Available online: https://www.sfbtrr280.de/en/ (accessed on 9 December 2023).
- Hegger, J.; Curbach, M.; Stark, A.; Wilhelm, S.; Farwig, K. Innovative Design Concepts: Application of Textile Reinforced Concrete to Shell Structures. Struct. Concr. 2018, 19, 637–646. [Google Scholar] [CrossRef]
- McNeel Europe SL, Rhinoceros 3D, Version 7.0. Available online: https://www.rhino3d.com/ (accessed on 9 December 2023).
- Robert McNeel & Associates, Grasshopper 3D, Version 7.0. Available online: https://www.grasshopper3d.com/ (accessed on 9 December 2023).
- RF-COM/RS-COM Add-on Module for RFEM/RSTAB. Available online: https://www.dlubal.com/en/products/rfem-and-rstab-add-on-modules/others/rf-com (accessed on 9 December 2023).
- Vakaliuk, I.; Frenzel, M.; Curbach, M. Application of Parametric Design Tools for the Roof of the C3 Technology Demonstration House—CUBE. In Form and Force—Proceedings of the IASS Annual Symp. 2019/Structural Membranes 2019; Lázaro, C., Bletzinger, K.-U., Oñate, E., Eds.; International Centre for Numerical Methods in Engineering (CIMNE): Barcelona, Spain, 2019; pp. 1077–1084. [Google Scholar]
- Koschemann, M.; Vakaliuk, I.; Curbach, M. An Ultra-Light Carbon Concrete Bridge: From Design to Realisation. In Proceedings of the Concrete Innovation for Sustainability—Proceedings for the 6th fib International Congress 2022, Oslo, Norway, 12–16 June 2022; Stokkeland, S., Braarud, H.C., Eds.; Novus Press: Oslo, Norway, 2022; pp. 2458–2467. [Google Scholar]
- Dlubal Software GmbH, RFEM, Version 5.19. Available online: www.dlubal.com (accessed on 9 December 2023).
- Vakaliuk, I.; Platen, J.; Klempt, V.; Scheerer, S.; Curbach, M.; Kaliske, M.; Löhnert, S. Development of Load-Bearing Shell-Type TRC Structures—Initial Numerical Analysis. In Proceedings of the Concrete Innovation for Sustainability—Proceedings for the 6th fib International Congress 2022, Oslo, Norway, 12–16 June 2022; Stokkeland, S., Braarud, H.C., Eds.; Novus Press: Oslo, Norway, 2022; pp. 1235–1244. [Google Scholar]
- Chudoba, R.; Sharei, E.; Senckiel-Peters, T.; Schladitz, F. Numerical Modelling of Non-Uniformly Reinforced Carbon Concrete Lightweight Ceiling Elements. Appl. Sci. 2019, 9, 2348. [Google Scholar] [CrossRef]
- Jesse, F. Tragverhalten von Filamentgarnen in Zementgebundener Matrix. Ph.D. Dissertation, Technische Universität Dresden, Dresden, Germany, 2005. Available online: https://nbn-resolving.org/urn:nbn:de:swb:14-1122970324369-39398 (accessed on 2 March 2024).
- Hartig, J. Numerical Investigations on the Uniaxial Tensile Behaviour of Textile Reinforced Concrete. Ph.D. Dissertation, Technische Universität Dresden, Dresden, Germany, 2011. [Google Scholar]
- Bentur, A.; Mindess, S. Fibre Reinforced Cementitious Composites; Modern Concrete Technology Series; E & FN Spon: London, UK, 1990; ISBN 0–415–25048–X (hbk). [Google Scholar]
- Nathan, G.K.; Paramasivam, P.; Lee, S.L. Tensile Behaviour of Fiber Reinforced Cement Paste. J. Ferrocem. 1977, 7, 59–79. [Google Scholar]
- Telford, T. CEB-FIP Model Code 1990: Design Code; Thomas Telford Services Ltd., Thomas Telford House: London, UK, 1993. [Google Scholar]
- Blom, J.; Wastiels, J. Modelling Textile Reinforced Cementitious Composites—Effect of Elevated Temperatures. In Proceedings of the 19th International Conference on Composite Materials, Montreal, QC, Canada, 28 July–2 August 2013; pp. 7098–7108. [Google Scholar]
- Blom, J.; Cuypers, H.; Van Itterbeeck, P.; Wastiels, J. Modelling the Behaviour of Textile Reinforced Cementitious Composites under Bending. In Proceedings of the Fibre Concrete 2007 Technology, Design, Application, Prague, Czech Republic, 12–13 September 2007; pp. 205–210. [Google Scholar]
- Jesse, F.; Ortlepp, R.; Curbach, M. Tensile Stress-Strain Behaviour of Textile Reinforced Concrete. In Proceedings of the towards a Better Built Environment—Inovation, Sustainability, Information Technology—Proceedings of the IABSE Symp, Melbourne, Australia, 11–13 September 2002; Volume 86, pp. 376–377. [Google Scholar]
- Zilch, K.; Zehetmaier, G. Bemessung im konstruktiven Betonbau: Nach DIN 1045-1 und EN 1992-1-1, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2010; ISBN 978-3-540-70637-3. [Google Scholar]
- Ng, H.K.; Nathan, G.K.; Paramasivam, P.; Lee, S.L. Tensile Properties of Steel Fiber Reinforced Mortar. J. Ferrocem. 1978, 8, 219–229. [Google Scholar]
- Carbon Concrete Composite [Homepage]. Available online: https://www.bauen-neu-denken.de/en/ (accessed on 9 December 2023).
- Wilhelm, K. Verbundverhalten von Mineralisch und Polymer Gebundenen Carbonbewehrungen und Beton bei Raumtemperatur und erhöhten Temperaturen bis 500 °C. Ph.D. Dissertation, Technische Universität Dresden, Dresden, Germany, 2021. Available online: https://nbn-resolving.org/urn:nbn:de:bsz:14-qucosa2-770999 (accessed on 9 December 2023).
- DIN EN 196-1:2016-11; Prüfverfahren für Zement—Teil 1: Bestimmung der Festigkeit. Beuth Verlag: Berlin, Germany, 2016.
- Technical Data Sheet Solidian GRID Q85-CCE-21-E5. 2023. Available online: https://solidian.com/wp-content/uploads/solidian-GRID-Qxx-CCE-xx-Technical-Product-Data-Sheets-v2402.pdf (accessed on 9 December 2023).
- Vakaliuk, I.; Scheerer, S.; Curbach, M. Initial Laboratory Test of Load-Bearing Shell-Shaped TRC Structures. In Concrete Innovation for Sustainability, Proceedings of the 6th fib International Congress, Oslo, Norway, 12–16 June 2022; Stokkeland, S., Braarud, H.C., Eds.; Novus Press: Oslo, Norway, 2022; pp. 675–684. [Google Scholar]
- Schütze, E.; Bielak, J.; Scheerer, S.; Hegger, J.; Curbach, M. Einaxialer Zugversuch für Carbonbeton mit textiler Bewehrung | Uniaxial Tensile Test for Carbon Reinforced Concrete with Textile Reinforcement. Beton Stahlbetonbau 2018, 113, 33–47. [Google Scholar] [CrossRef]
- Rempel, S. Zur Zuverlässigkeit der Bemessung von Biegebeanspruchten Betonbauteilen mit textiler Bewehrung. Ph.D. Dissertation, RWTH Aachen, Aachen, Germany, 2018. [Google Scholar] [CrossRef]
- Zavadski, V.; Frenzel, M. Aufbau, Bemessung und Planung der TWIST-Carbonbetonschalen. Beton Stahlbetonbau 2023, 118, 71–81. [Google Scholar] [CrossRef]
- Mazars, J.; Grange, S. Simplified Strategies Based on Damage Mechanics for Concrete under Dynamic Loading. Philos. Trans. R. Soc. A 2017, 375, 20160170. [Google Scholar] [CrossRef]
- Mazars, J. A Description of Micro- and Macroscale Damage of Concrete Structures. Eng. Fract. Mech. 1986, 25, 729–737. [Google Scholar] [CrossRef]
- Sharei, E. Simulation Concepts for Concrete Structures with Discrete and Smeared Representation of Reinforcement. Ph.D. Dissertation, RWTH Aachen, Aachen, Germany, 2018. [Google Scholar]
- Scholzen, A.; Chudoba, R.; Hegger, J. Thin-Walled Shell Structures Made of Textile-Reinforced Concrete. Struct. Concr. 2015, 16, 115–124. [Google Scholar] [CrossRef]
- Sharei, E.; Chudoba, R.; Scholzen, A. Cross-Sectional Failure Criterion Combined with Strain-Hardening Damage Model for Simulation of Thin-Walled Textile-Reinforced Concrete Shells. In Proceedings of the in ECCOMAS Congress 2016 VII European Congress on Computational Methods in Applied Sciences and Engineering; Papadrakakis, M., Papadopoulos, V., Stefanou, G., Plevris, V., Eds.; Institute of Structural Analysis and Antiseismic Research School of Civil Engineering National Technical University Athens: Crete Island, Greece, 2016; pp. 6823–6831. [Google Scholar]
- Scholzen, A.; Chudoba, R.; Hegger, J. Ultimate Limit State Assessment of TRC Shell Structures with Combined Normal and Bending Loading. In Proceedings of the 11th International Symposium on Ferrocement and Textile Reinforced Concrete, Aachen, Germany, 7–10 June 2015; RILEM Publ.: Bagneux, France, 2015; pp. 159–166. [Google Scholar]
- Senckpiel, T.; Häussler-Combe, U. Experimental and Computational Investigations on Shell Structures Made of Carbon Reinforced Concrete. In Proceedings of the Interfaces: Architecture, Engineering, Science—Proceedings of Annual Meeting of the International Association of Shell & Spatial Structures (IASS), Hamburg, Germany, 25–27 September 2017; pp. 1–8. [Google Scholar]
- EN 1991-1-1; Eurocode 1: Actions on Structures—Part 1-1: General Actions—Densities, Self-Weight, Imposed Loads for Buildings. 2002. Available online: https://www.phd.eng.br/wp-content/uploads/2015/12/en.1991.1.1.2002.pdf (accessed on 9 December 2023).

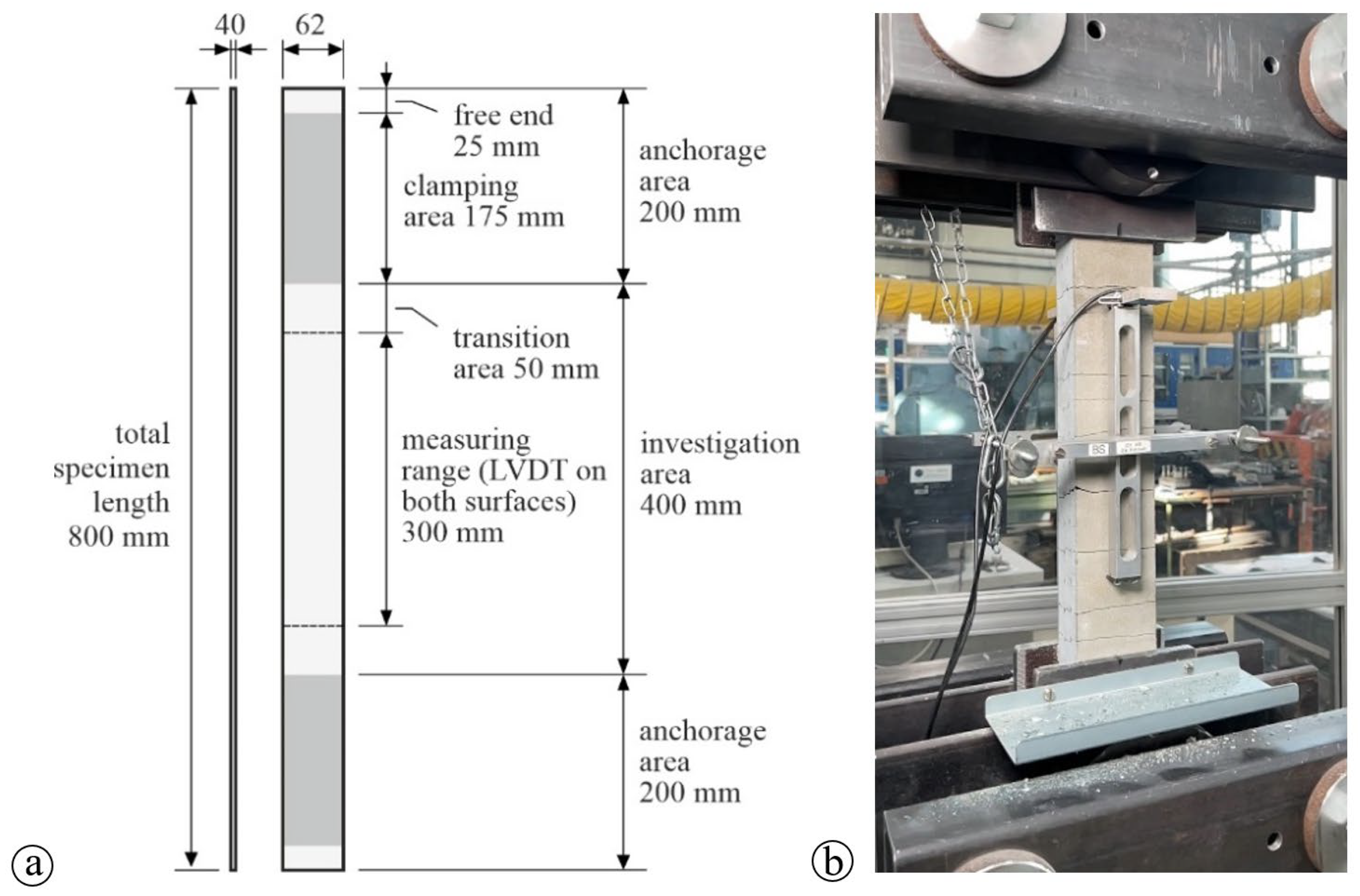
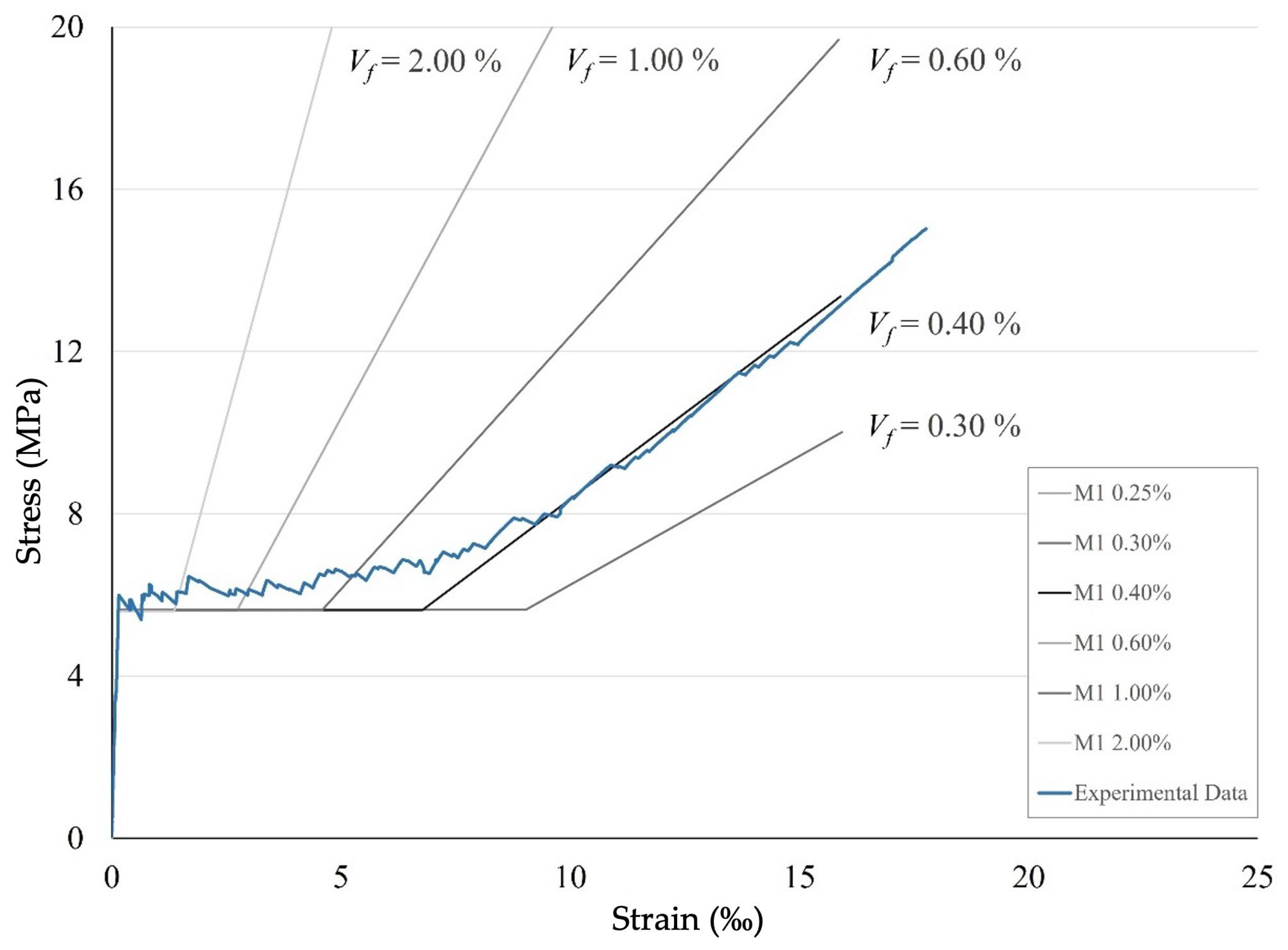
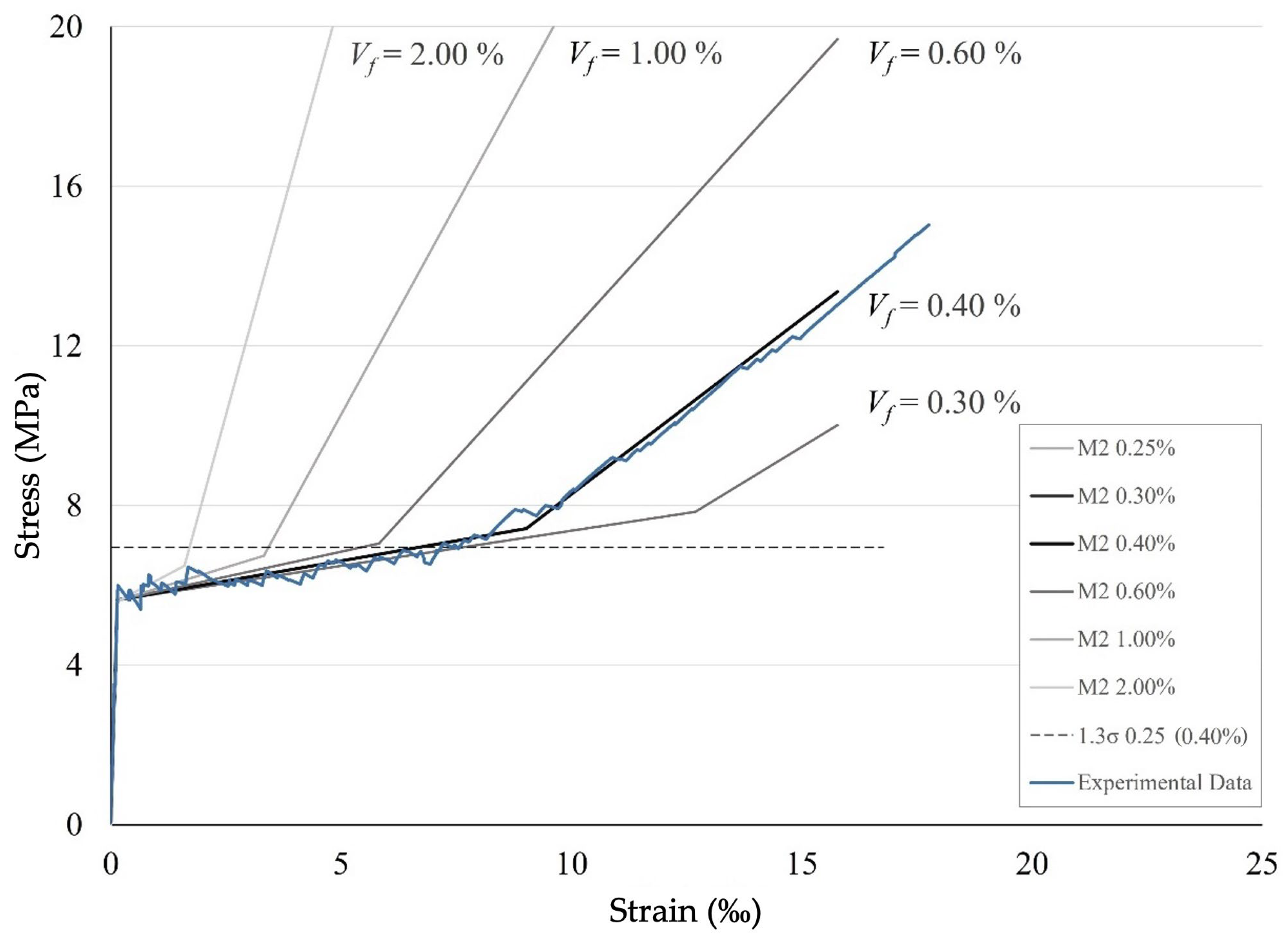


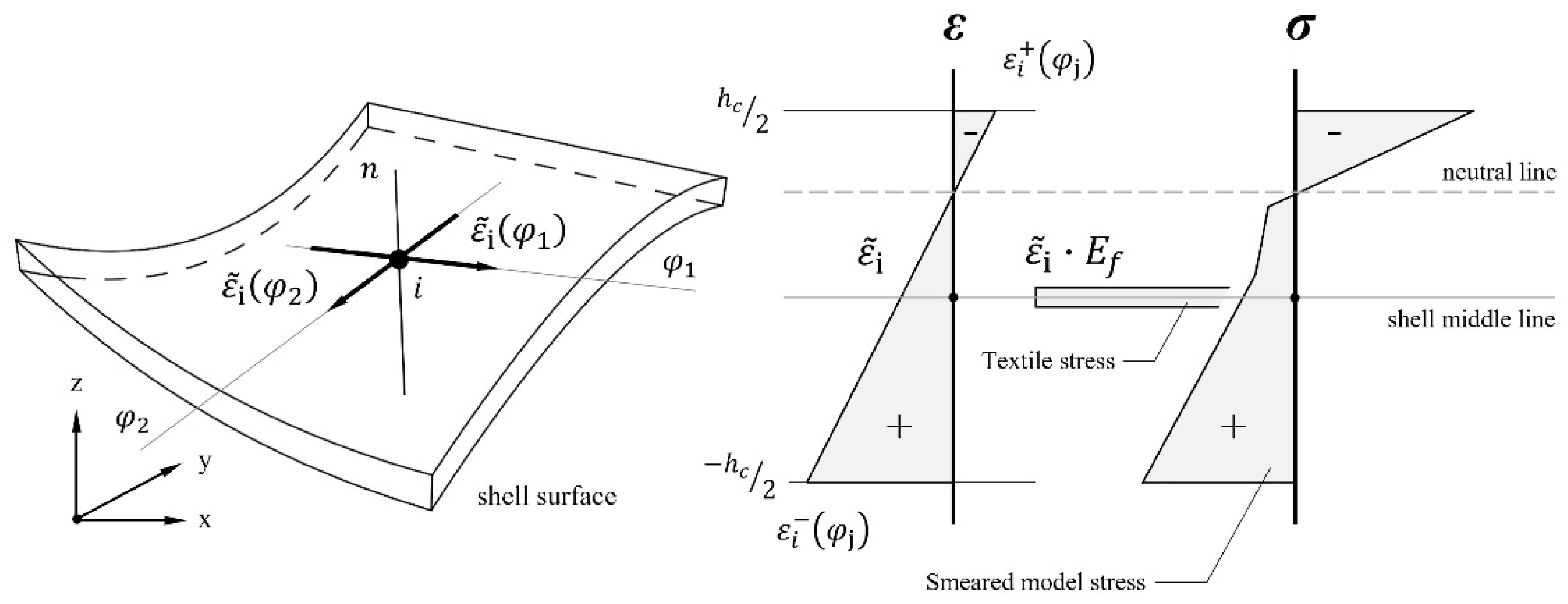


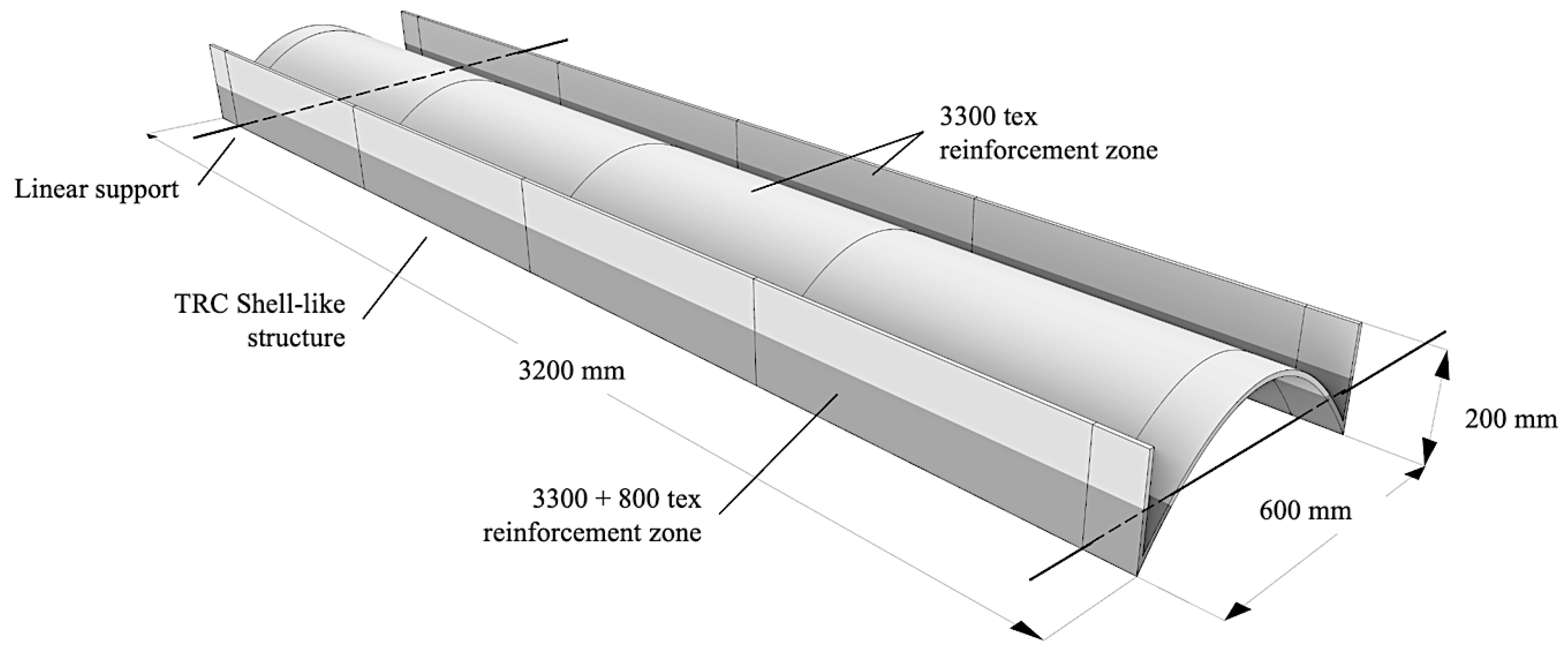
| Raw Materials | Quantity (kg/m³) |
|---|---|
| Binder compound BMK-DS-1 (Dyckerhoff, Germany) | 815 |
| Quartz sand 0.06/0.2 | 340 |
| Sand 0/2 | 965 |
| Superplasticizer (e.g., MC-VP-16-0205-02 from MC-Bauchemie, Germany) | 17 |
| Water | 190 |
| Property | Value | Unit |
|---|---|---|
| Compressive strength fcm | 114.8 | MPa |
| Bending tensile strength fctm, fl | 8.8 | MPa |
| Property | Longitudinal | Transversal |
|---|---|---|
| Roving axis distance etex (mm) | 21 | 21 |
| Cross-section of a roving Af (mm2) | 1.81 | 1.81 |
| Cross-section of the reinforcement grid Atex (mm2/m) | 85.4 | 85.6 |
| Tensile strength of the roving σu,f (MPa) | ≥3950 | ≥4250 |
| Tensile strength of the grid σu,tex (MPa) | ≥3950 (avg.)|≥3050 (char.) | ≥4250 (avg.)|≥3250 (char.) |
| Resisting force Ftex (kN/m) | ≥260.5 | ≥275.0 |
| Modulus of elasticity Etex (MPa) | ≥251,500 | ≥254,000 |
| Properties | ACK | Trilinear |
|---|---|---|
| (MPa) | 42,100.0 | 42,100.0 |
| (MPa) | 0.0 | 201.14 |
| (MPa) | 846.9 | 843.28 |
| 0.91 | 0.91 | |
| C | −0.02 | – |
| – | −0.04 | |
| k | – | 0.0025 |
| Diagram Points | Strain (‰) | Stress (MPa) |
|---|---|---|
| ACK|Trilinear | ACK|Trilinear | |
| Pt. 1+ | 0.14|0.14 | 5.70|5.70 |
| Pt. 2+ | 6.80|9.05 | 5.70|7.47 |
| Pt. 3+ | 15.90|15.77 | 13.36|13.36 |
| Pt. 1− | 0.0 | −60.0 |
| Pt. 2− | −3.0 | −106.6 |
| Diagram Points | Strain (‰) | Stress (MPa) |
|---|---|---|
| 3300 tex|3300 + 800 tex | 3300 tex|3300 + 800 tex | |
| Pt. 1+ | 0.086|0.086 | 2.40|2.40 |
| Pt. 2+ | 2.00|1.40 | 2.40|2.40 |
| Pt. 3+ | 7.20|7.30 | 19.00|30.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vakaliuk, I.; Scheerer, S.; Curbach, M. The Numerical Analysis of Textile Reinforced Concrete Shells: Basic Principles. Appl. Sci. 2024, 14, 2140. https://doi.org/10.3390/app14052140
Vakaliuk I, Scheerer S, Curbach M. The Numerical Analysis of Textile Reinforced Concrete Shells: Basic Principles. Applied Sciences. 2024; 14(5):2140. https://doi.org/10.3390/app14052140
Chicago/Turabian StyleVakaliuk, Iurii, Silke Scheerer, and Manfred Curbach. 2024. "The Numerical Analysis of Textile Reinforced Concrete Shells: Basic Principles" Applied Sciences 14, no. 5: 2140. https://doi.org/10.3390/app14052140
APA StyleVakaliuk, I., Scheerer, S., & Curbach, M. (2024). The Numerical Analysis of Textile Reinforced Concrete Shells: Basic Principles. Applied Sciences, 14(5), 2140. https://doi.org/10.3390/app14052140