Proposal of UWB-PPM with Additional Time Shift for Positioning Technique in Nondestructive Environments
Abstract
:1. Introduction
- Firstly, the UWB-PPM signal which is used in determining the distance under the homogeneous and heterogeneous propagation conditions is mathematically analyzed.
- Secondly, based on the analysis of received signal, the delay time and then the propagation distances are estimated.
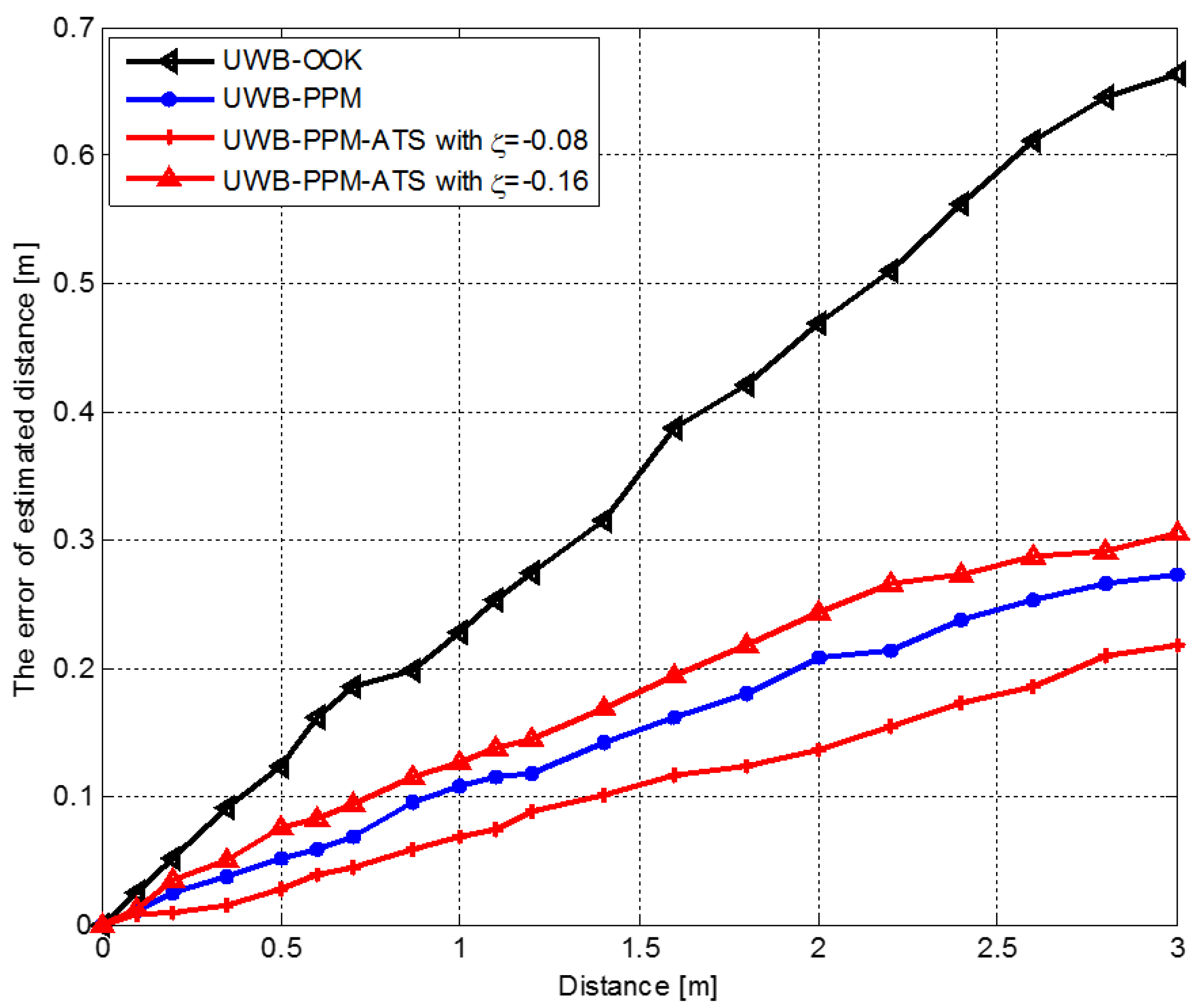
- Thirdly, to increase the accuracy of the estimated distance, an enhanced UWB-PPM modulation technique, called ultra-wide band pulse position modulation with additional time shift (UWB-PPM-ATS) is proposed and compared with other techniques such as conventional UWB-PPM, UWB- OOK with the properties of transmission environment is known.
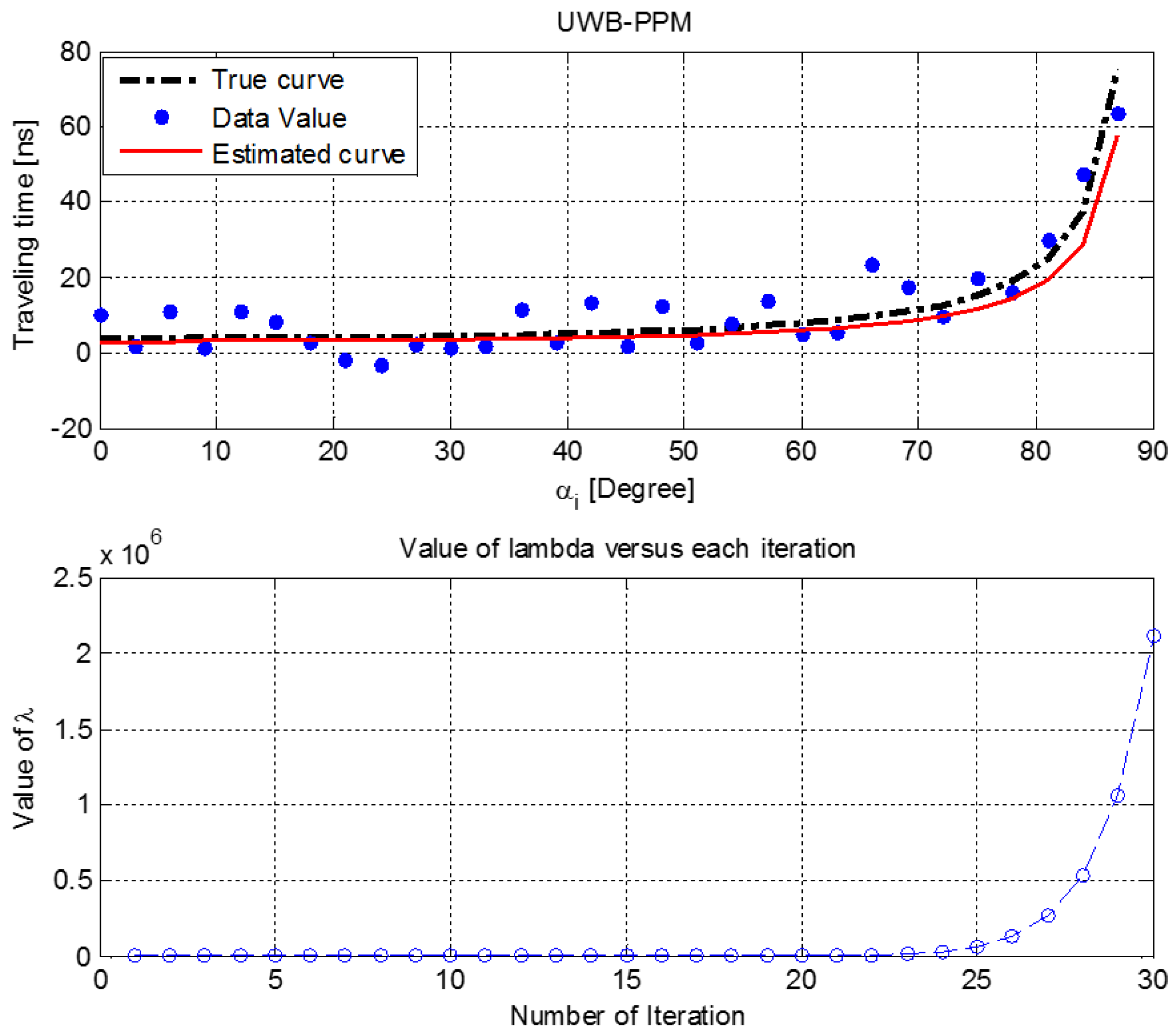
- Finally, the Levenberg–Marquardt nonlinear estimation algorithm is applied to estimate the system parameter and the target when the propagation environment is unknown.
2. System Model
3. Proposal of Positioning Approach in Nondestructive Environments
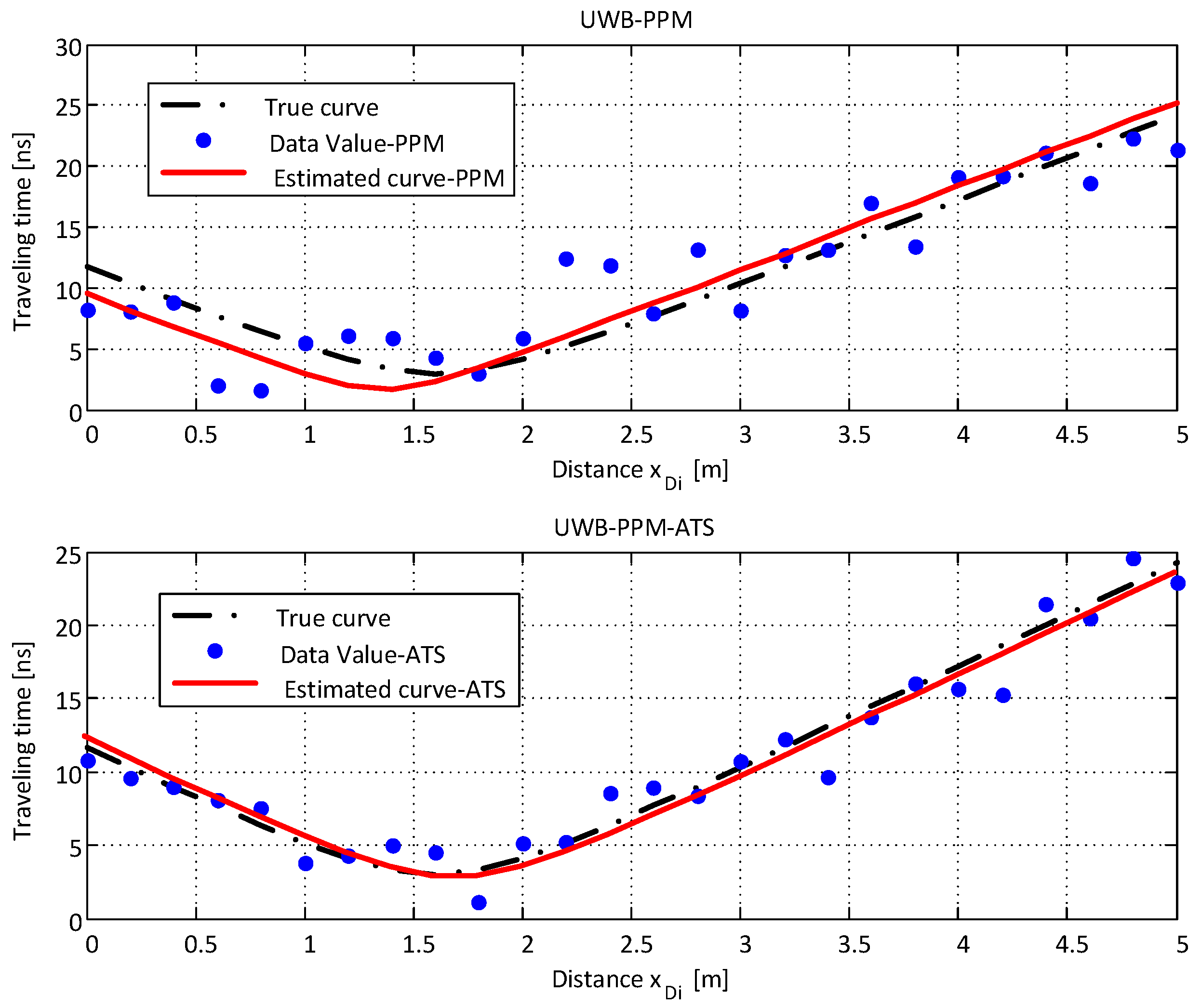
3.1. Proposal of UWB-PPM-ATS
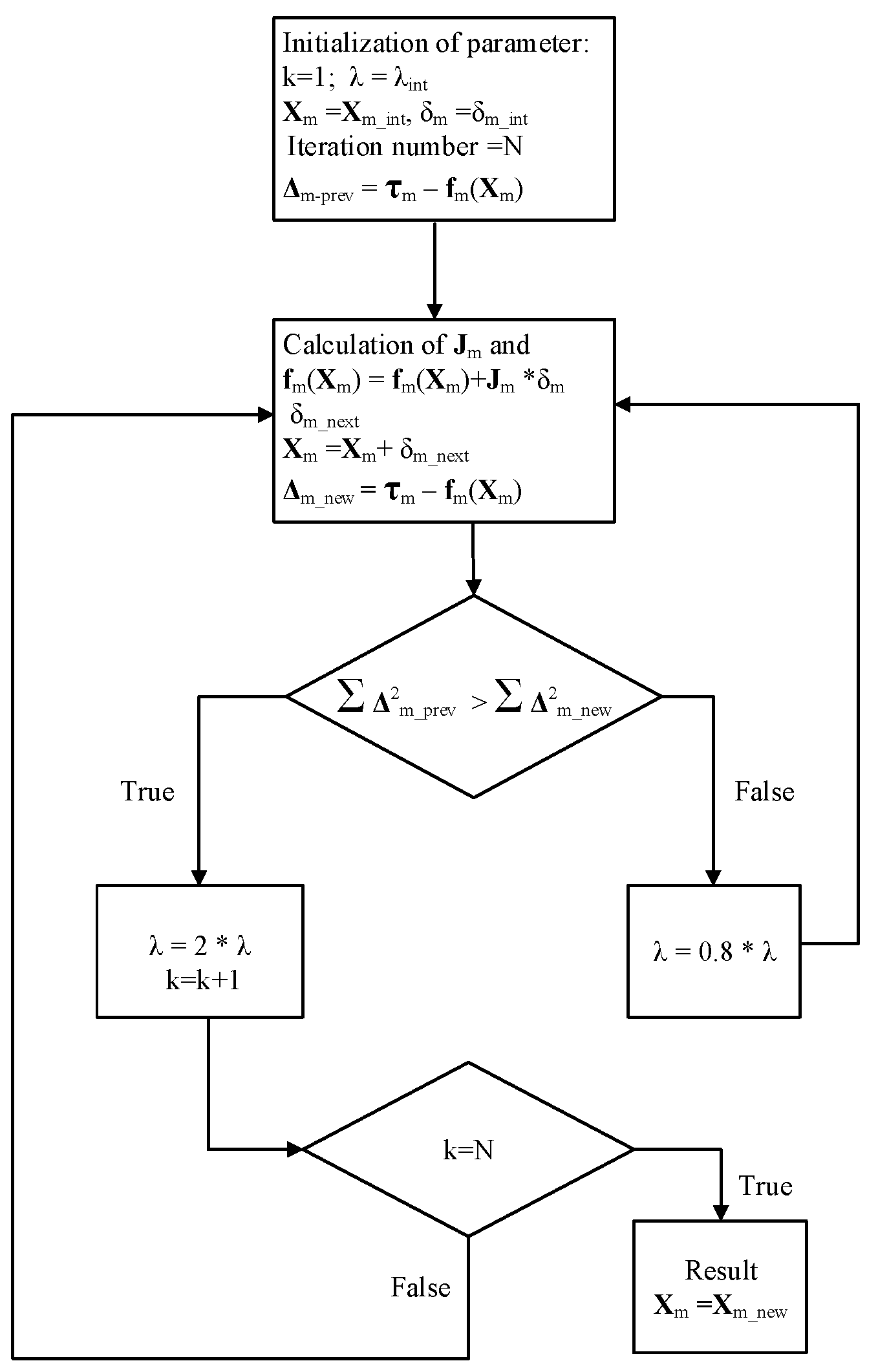
3.2. Estimation Algorithm
4. Simulation Results and Discussion
4.1. Simulation Parameters
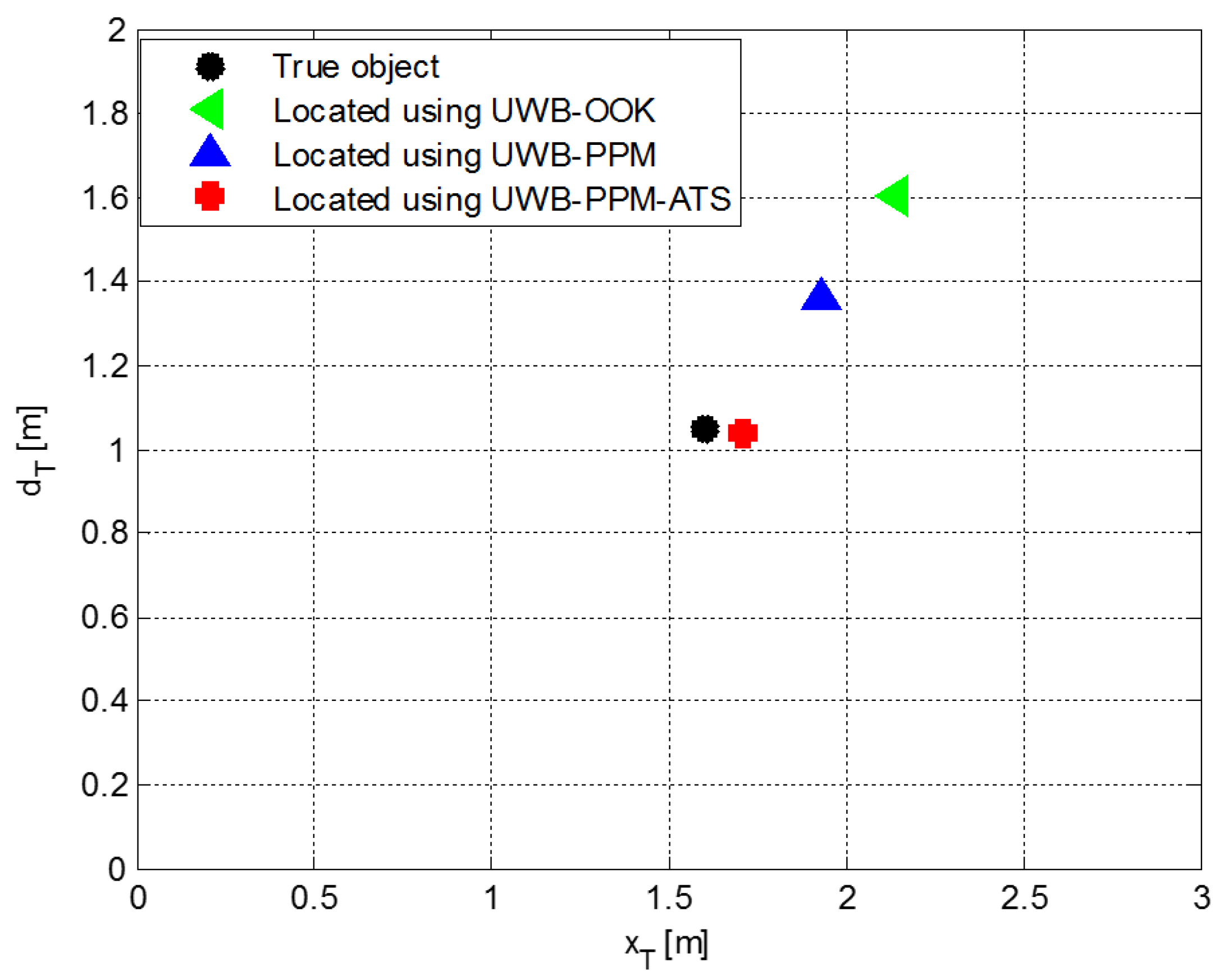
4.2. Determine the Location of Buried Object by LMFA
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| UWB | Ultra-wide band |
| UWB-PPM | Ultra-wide band pulse position modulation |
| UWB-OOOK | Ultra-wide band On-Off Keying |
| UWB-PPM-ATS | Ultra-wide band pulse position modulation with optimized additional time shift |
| LMFA | Levenberg–Marquardt Fletcher algorithm |
References
- Goyal, V. Pulse generation and analysis of ultra wide band system model. GESJ Comput. Sci. Telecommun. 2012, 2, 3–6. [Google Scholar]
- U. F. C. Commission. Revision of Part 15 of the Commission’s Rules Regarding Ultra-Wideband Transmission Systems; First report and order, technical report, Feb, Tech. Rep.; FCC: Washington, DC, USA, 2002.
- Goyal, V.; Dhaliwal, B. Optimal pulse generation for the improvement of ultra wideband system performance. In Proceedings of the 2014 Recent Advances in Engineering and Computational Sciences (RAECS), Chandigarh, India, 6–8 March 2014; IEEE: Piscataway, NJ, USA, 2014; pp. 1–6. [Google Scholar]
- Welborn, M.L. System considerations for ultra-wideband wireless networks. In Proceedings of the RAWCON 2001, 2001 IEEE Radio and Wireless Conference (Cat. No. 01EX514), Waltham, MA, USA, 19–22 August 2001; IEEE: Piscataway, NJ, USA, 2001; pp. 5–8. [Google Scholar]
- Hu, B.; Beaulieu, N.C. Accurate evaluation of multiple-access performance in th-ppm and th-bpsk uwb systems. IEEE Trans. Commun. 2004, 52, 1758–1766. [Google Scholar] [CrossRef]
- Khalesi, H.; Ghods, V. QPSK Modulation Scheme Based on Orthogonal Gaussian Pulses for IR-UWB Communication Systems. J. Circuits Syst. Comput. 2019, 28, 1950008. [Google Scholar] [CrossRef]
- Sharma, S.; Gupta, A.; Bhatia, V. A simple modified peak detection based UWB receiver for WSN and IoT application. In Proceedings of the 2017 IEEE 85th Vehicular Technology Conference (VTC Spring), Sydney, NSW, Australia, 4–7 June 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–6. [Google Scholar]
- Benotmane, N.B.; Elahmar, S.A.; Dayoub, I.; Hamouda, W. Improved eigenfilter design method for channel shortening equalizer in TH-UWB. IEEE Trans. Veh. Technol. 2018, 67, 7749–7753. [Google Scholar] [CrossRef]
- Zhao, L.; Haimovich, A.M. Capacity of m-ary ppm ultra-wideband communications over awgn channels. In Proceedings of the IEEE 54th Vehicular Technology Conference, VTC Fall 2001, Proceedings (Cat. No. 01CH37211), Atlantic City, NJ, USA, 7–11 October 2001; IEEE: Piscataway, NJ, USA, 2001; Volume 2, pp. 1191–1195. [Google Scholar]
- Venkatesan, V.; Liu, H.; Nilsen, C.; Kyker, R.; Magana, M.E. Performance of an optimally spaced ppm ultra-wideband system with direct sequence spreading for multiple access’. In Proceedings of the 2003 IEEE 58th Vehicular Technology Conference, VTC 2003-Fall (IEEE Cat. No. 03CH37484), Orlando, FL, USA, 6–9 October 2003; IEEE: Piscataway, NJ, USA, 2003; Volume 1, pp. 602–606. [Google Scholar]
- Wu, J.; Xiang, H.; Tian, Z. Weighted noncoherent receivers for uwb ppm signals. IEEE Commun. Lett. 2006, 10, 655–657. [Google Scholar]
- Almodovar-Faria, J.M.; McNair, J. Optimal integration time for energy-detection ppm uwb systems. In Proceedings of the 2012 IEEE Global Communications Conference (GLOBECOM), Anaheim, CA, USA, 3–7 December 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 4054–4059. [Google Scholar]
- Yin, H.B.; Yang, J.A.; Wang, W.D. A randomly permuted fourier measurement matrix for uwb-ppm signals. Appl. Mech. Mater. 2014, 556, 2646–2649. [Google Scholar] [CrossRef]
- Yajnanarayana, V.; Händel, P. Joint estimation of toa and ppm symbols using sub-nyquist sampled ir-uwb signal. IEEE Commun. Lett. 2017, 21, 949–952. [Google Scholar] [CrossRef]
- Jabbar, M. Improving PSD of PPM-IR for UWB Signal Using Turbo Encoder. J. Ind. Eng. Manag. S 2018, 3, 2169-0316. [Google Scholar] [CrossRef]
- Goyal, V.; Dhaliwal, B. Improving ultra wideband (uwb) system by modified random combination of pulses. Eng. Rev. 2018, 38, 189–203. [Google Scholar] [CrossRef]
- Maali, A.; Boukhelifa, A.; Mesloub, A.; Sadoudi, S.; Benssalah, M. An enhanced pulse position modulation (ppm) for both ir-uwb and dcc-uwb communication. In Proceedings of the 2019 13th European Conference on Antennas and Propagation (EuCAP), Krakow, Poland, 31 March–5 April 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 1–5. [Google Scholar]
- Daniels, D.J. Ground penetrating radar. In Encyclopedia of RF and Microwave Engineering; John Wiley & Sons, Inc.: New York, NY, USA, 2005. [Google Scholar]
- Cao, Y.; Labuz, J.; Guzina, B. Evaluation of pavement system based on ground-penetrating radar full-waveform simulation. Transp. Res. Rec. 2011, 2227, 71–78. [Google Scholar] [CrossRef]
- Karim, H.H.; Al-Qaissi, A.M. Assessment of the accuracy of road flexible and rigid pavement layers using gpr. Eng. Technol. J. Part (A) Eng. 2014, 32, 788–799. [Google Scholar]
- Nakayama, Y.; Kohno, R. Novel variable spreading sequence length system for improving the processing speed of ds-uwb radar. In Proceedings of the 2008 8th International Conference on ITS Telecommunications, Phuket, Thailand, 24 October 2008; IEEE: Piscataway, NJ, USA, 2008; pp. 357–361. [Google Scholar]
- Dabove, P.; Di Pietra, V.; Piras, M.; Jabbar, A.A.; Kazim, S.A. Indoor positioning using Ultra-wide band (UWB) technologies: Positioning accuracies and sensors performances. In Proceedings of the 2018 IEEE/ION Position, Location and Navigation Symposium (PLANS), Monterey, CA, USA, 23–26 April 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 175–184. [Google Scholar]
- Xie, W.; Li, X.; Long, X. Underground operator monitoring platform based on ultra-wide band WSN. Int. J. Online Biomed. Eng. (iJOE) 2018, 14, 219–229. [Google Scholar] [CrossRef] [Green Version]
- Huyen, N.T.; Hiep, P.T. Proposing adaptive PN sequence length scheme for testing non-destructive structure using DS-UWB’. In Proceedings of the 2019 3rd International Conference on Recent Advances in Signal Processing, Telecommunications & Computing (SigTelCom), Hanoi, Vietnam, 21–22 March 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 10–14. [Google Scholar]
- Chen, X.; Kiaei, S. Monocycle shapes for ultra wideband system. In Proceedings of the 2002 IEEE International Symposium on Circuits and Systems, Proceedings (Cat. No. 02CH37353), Phoenix-Scottsdale, AZ, USA, 26–29 May 2002; IEEE: Piscataway, NJ, USA, 2002; Volume 1, pp. I-597–I-600. [Google Scholar]
- De Abreu, G.T.F.; Mitchell, C.J.; Kohno, R. On the orthogonality of hermite pulses for ultra wideband communications systems. In Proceedings of the 6th International Symposium on Wireless Personal Multimedia Communications (WPMC), Yokosuka, Japan, 21–22 October 2003. [Google Scholar]
- Gao, X. Uwb Indoor Localization System. Ph.D. Thesis, The George Washington University, Washington, DC, USA, 2018. [Google Scholar]
- Kanzow, C.; Yamashita, N.; Fukushima, M. Withdrawn: Levenberg–marquardt methods with strong local convergence properties for solving nonlinear equations with convex constraints. J. Comput. Appl. Math. 2005, 173, 321–343. [Google Scholar] [CrossRef] [Green Version]
- Benedetto, D. Understanding ultra wide band radio fundamentals. In Understanding Ultra Wide Band Radio Fundamentals; Pearson Education India: Delhi, India, 2008. [Google Scholar]
- Hubbard, S.S.; Peterson, J.E., Jr.; Majer, E.L.; Zawislanski, P.T.; Williams, K.H.; Roberts, J.; Wobber, F. Estimation of permeable pathways and water content using tomographic radar data. Lead. Edge J. 1997, 16, 1623–1630. [Google Scholar] [CrossRef]



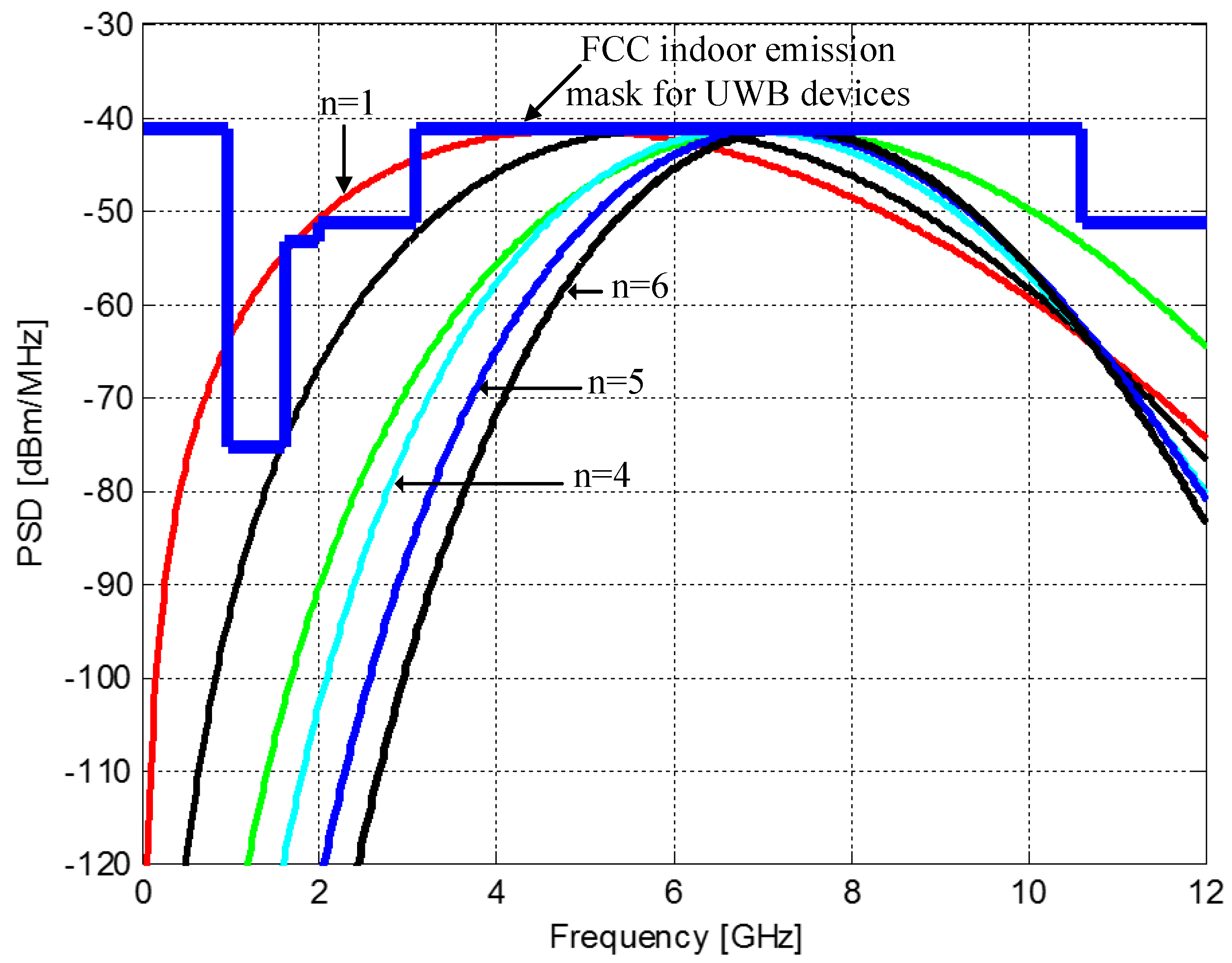


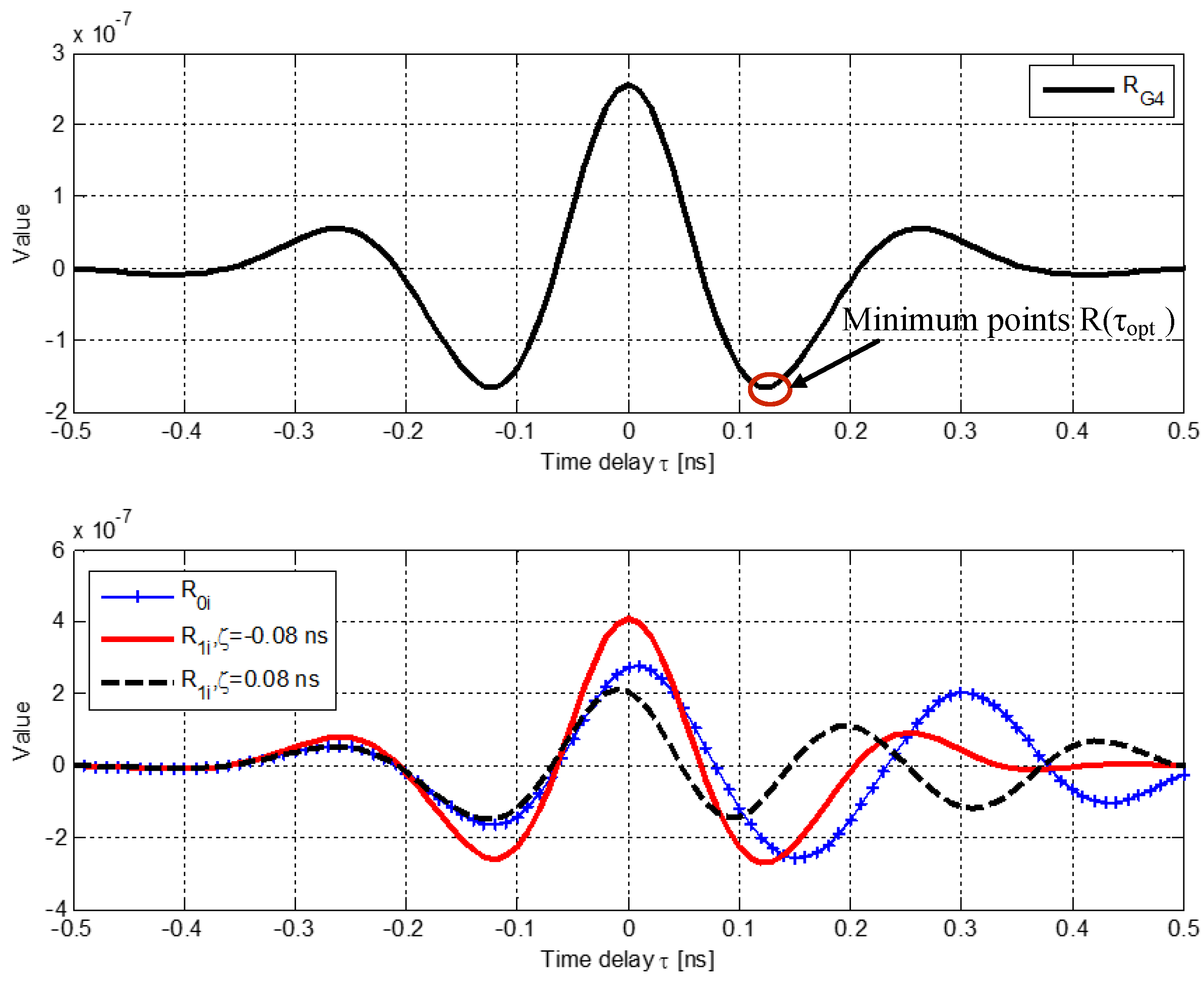
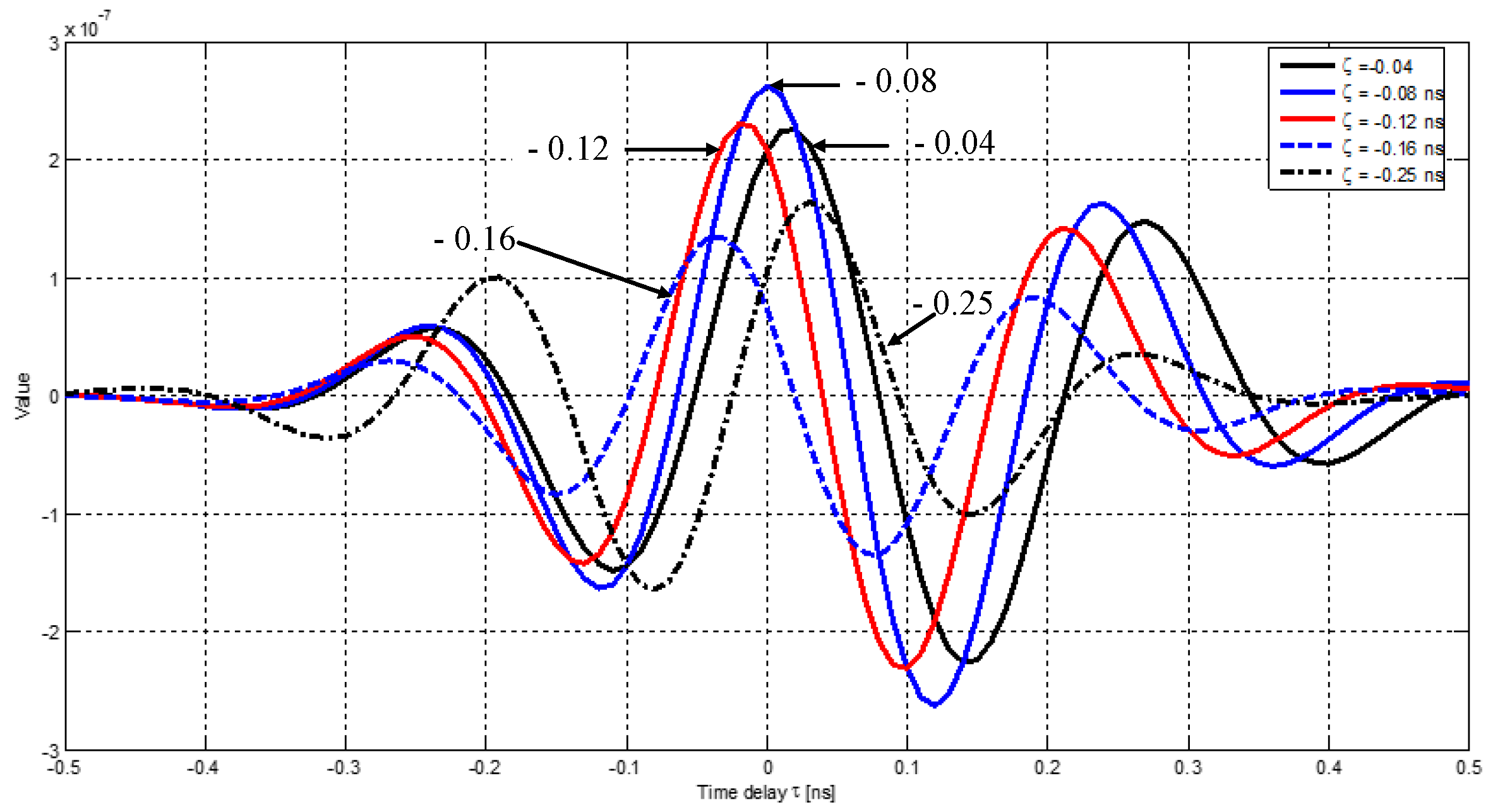
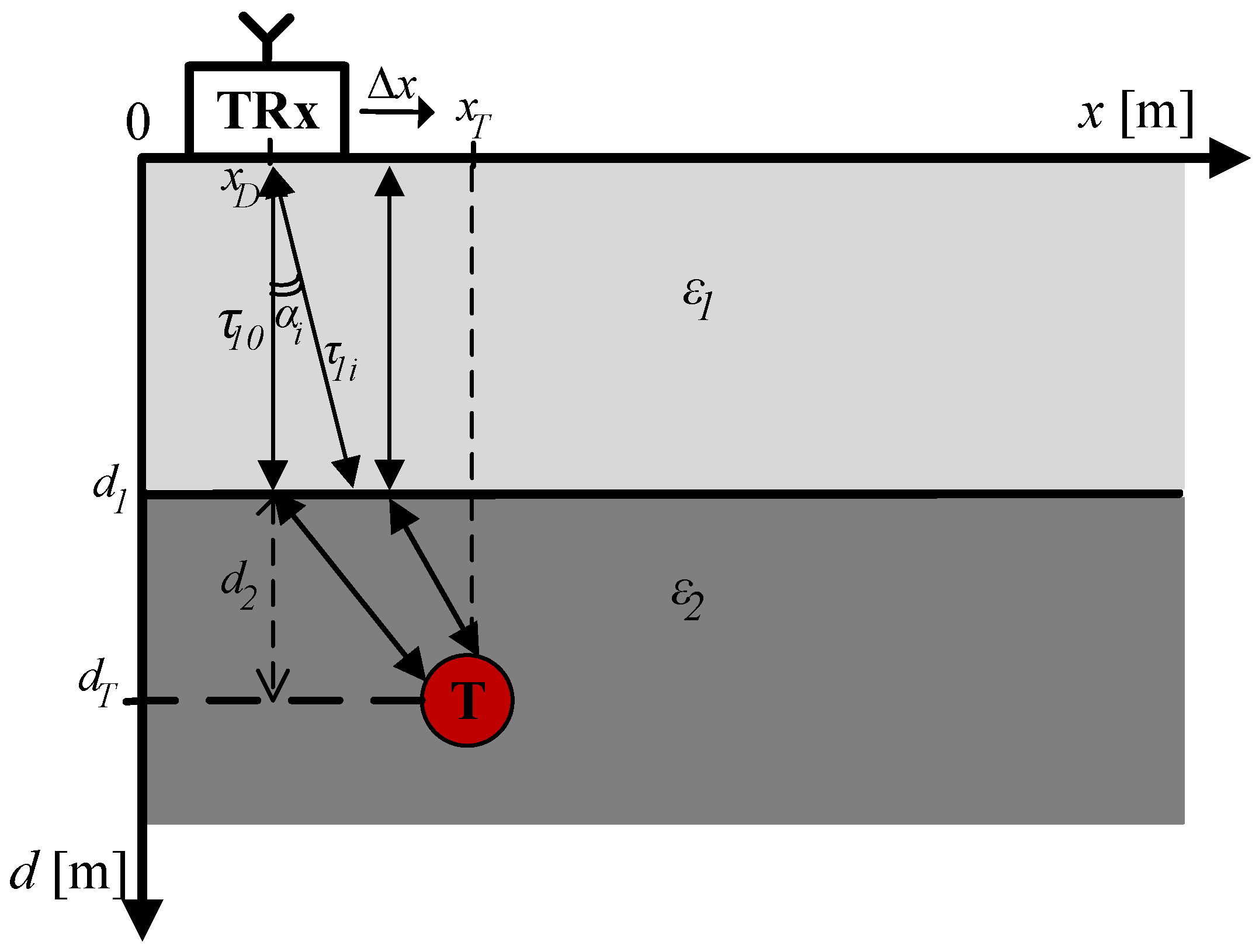

| Parameter | Notation | Value |
|---|---|---|
| Time normalization factor | 0.2877 ns | |
| Transmitted power | −35.4 dB | |
| The amplitude factors | 0.33, 0.13, 0.14 | |
| Number of pulses | N | 100 |
| Noise power | −102 dB | |
| Time shift of PPM | 0.2 ns | |
| Additional time shift | −0.08 ns, −0.16 ns | |
| Relative permittivity | ||
| with heterogeneous medium | , , | 4; 3; 5 |
| Parameter | Notation | Value |
|---|---|---|
| The thickness of the layer | 0.63 m | |
| Depth of ’T’ in the layer | 0.42 m | |
| X-coordinate of ’T’ | 1.6 m | |
| Relative permittivity | , | 3.5, 4.5 |
| Damping factor | 3 | |
| Movement step of the device | 20 cm |
| Parameter/Notation | |||||
|---|---|---|---|---|---|
| True value [m] | 0.63 | 0.42 | 1.6 | 3.5 | 4.5 |
| By UWB-OOK [m] | 0.9418 | 0.6623 | 2.1362 | 4.3216 | 5.7261 |
| By UWB-PPM [m] | 0.7923 | 0.5742 | 1.9316 | 2.7109 | 3.3590 |
| By UWB- | |||||
| PPM-ATS [m] | 0.6523 | 0.3899 | 1.7107 | 3.2829 | 4.8167 |
| Error of UWB-OOK | 0.3118 | 0.2423 | 0.5362 | 0.8216 | 1.2261 |
| Error of UWB-PPM | 0.1623 | 0.1542 | 0.3316 | 0.7891 | 1.141 |
| Error of | |||||
| UWB-PPM-ATS | 0.0223 | 0.0301 | 0.1107 | 0.2171 | 0.3167 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huyen, N.T.; Le Cuong, N.; Thanh Hiep, P. Proposal of UWB-PPM with Additional Time Shift for Positioning Technique in Nondestructive Environments. Appl. Sci. 2020, 10, 6011. https://doi.org/10.3390/app10176011
Huyen NT, Le Cuong N, Thanh Hiep P. Proposal of UWB-PPM with Additional Time Shift for Positioning Technique in Nondestructive Environments. Applied Sciences. 2020; 10(17):6011. https://doi.org/10.3390/app10176011
Chicago/Turabian StyleHuyen, Nguyen Thi, Nguyen Le Cuong, and Pham Thanh Hiep. 2020. "Proposal of UWB-PPM with Additional Time Shift for Positioning Technique in Nondestructive Environments" Applied Sciences 10, no. 17: 6011. https://doi.org/10.3390/app10176011
APA StyleHuyen, N. T., Le Cuong, N., & Thanh Hiep, P. (2020). Proposal of UWB-PPM with Additional Time Shift for Positioning Technique in Nondestructive Environments. Applied Sciences, 10(17), 6011. https://doi.org/10.3390/app10176011





