Pseudo Hermitian Interactions in the Dirac Equation

Abstract

We consider a (2 + 1)-dimensional massless Dirac equation in the presence of complex vector potentials. It is shown that such vector potentials (leading to complex magnetic fields) can produce bound states, and the Dirac Hamiltonians are η-pseudo Hermitian. Some examples have been explicitly worked out.

1. Introduction

In recent years, the massless Dirac equation in (2 + 1) dimensions has drawn a lot of attention, primarily because of its similarity to the equation governing the motion of charge carriers in graphene [1,2]. In view of the fact that electrostatic fields alone cannot provide confinement of the electrons, there have been quite a number of works on exact solutions of the relevant Dirac equation with different magnetic field configurations, for example, square well magnetic barriers [3–5], non-zero magnetic fields in dots [6], decaying magnetic fields [7], solvable magnetic field configurations [8], etc. On the other hand, at the same time, there have been some investigations into the possible role of non-Hermiticity and symmetry [9] in graphene [10–12], optical analogues of relativistic quantum mechanics [13] and relativistic non-Hermitian quantum mechanics [14], photonic honeycomb lattice [15], etc. Furthermore, the (2 + 1)-dimensional Dirac equation with non-Hermitian Rashba and scalar interaction was studied [16]. Here, our objective is to widen the scope of incorporating non-Hermitian interactions in the (2 + 1)-dimensional Dirac equation. We shall introduce η pseudo Hermitian interactions by using imaginary vector potentials. It may be noted that imaginary vector potentials have been studied previously in connection with the localization/delocalization problem [17,18], as well as phase transition in higher dimensions [19]. Furthermore, in the case of the Dirac equation, there are the possibilities of transforming real electric fields to complex magnetic fields and vice versa by the application of a complex Lorentz boost [20]. To be more specific, we shall consider η-pseudo Hermitian interactions [21] within the framework of the (2 + 1)-dimensional massless Dirac equation. In particular, we shall examine the exact bound state solutions in the presence of imaginary magnetic fields arising out of imaginary vector potentials. We shall also obtain the η operator, and it will be shown that the Dirac Hamiltonians are η-pseudo Hermitian.

2. The Model

The (2 + 1)-dimensional massless Dirac equation is given by:

$$\begin{array}{ccc}H\psi =E\psi ,& H=c\mathit{\sigma }\cdot \mathit{P}=c\left(\begin{array}{cc}0& P_{-}\\ P_{+}& 0\end{array}\right),& \psi =\left(\begin{array}{c}{\psi }_1\\ {\psi }_2\end{array}\right)\end{array}$$

(1)

where c is the velocity of light and:

$$P_{\pm }=(P_x\pm i{P}_y)=(p_x+A_x)\pm i(p_y+A_y)$$

(2)

In order to solve Equation (1), it is necessary to decouple the spinor components. Applying the operator, H, from the left in Equation (1), we find:

$$c^2\left(\begin{array}{cc}{P}_{-}{P}_{+}& 0\\ 0& P_{+}{P}_{-}\end{array}\right)\psi =E^2\psi$$

(3)

Let us now consider the vector potential to be:

$$\begin{array}{cc}{A}_x=0,& A_y=f(x)\end{array}$$

(4)

so that the magnetic field is given by:

$${\mathcal{B}}_z(x)=f^{\prime }(x)$$

(5)

For the above choice of vector potentials, the component wave functions can be taken of the form:

$${\psi }_{1,2}(x,y)=e^{i{k}_{y}y}{\varphi }_{1,2}(x)$$

(6)

Then, from (3), the equations for the components are found to be (in units of ℏ = 1):

$$\begin{array}{c}\left[-\frac{d^2}{d{x}^2}+W^2(x)+W^{\prime }(x)\right]{\varphi }_1(x)={\mathit{\epsilon }}^2{\varphi }_1(x)\\ \left[-\frac{d^2}{d{x}^2}+W^2(x)-W^{\prime }(x)\right]{\varphi }_2(x)={\mathit{\epsilon }}^2{\varphi }_2(x)\end{array}$$

(7)

where ϵ = (E/c), and the function, W(x), is given by:

$$W(x)=k_y+f(x)$$

(8)

2.1. Complex Decaying Magnetic Field

It is now necessary to choose the function, f(x). Our first choice for this function is:

$$\begin{array}{cc}f(x)=-(A+iB)e^{-x},& -\infty <x<\infty \end{array}$$

(9)

where A > 0 and B are constants. This leads to a complex exponentially decaying magnetic field:

$${\mathcal{B}}_z(x)=(A+iB)e^{-x}$$

(10)

For B = 0 or a purely imaginary number (such that (A + iB) > 0), the magnetic field is an exponentially decreasing one, and we recover the case considered in [7,8].

Now, from the second of Equation (7), we obtain:

$$\left[-\frac{d^2}{d{x}^2}+V_2(x)\right]{\varphi }_2=({\mathit{\epsilon }}^2-k_y^2){\varphi }_2$$

(11)

where:

$$V_2(x)=k_y^2+{\left(A+iB\right)}^2{e}^{-2x}-(2k_y+1)(A+iB)e^{-x}$$

(12)

It is not difficult to recognize V₂(x) in Equation (12) as the complex analogue of the Morse potential whose solutions are well known [22,23]. Using these results, we find:

$$\begin{array}{l}{E}_{2,n}=\pm c\sqrt{k_y^2-{(k_y-n)}^2}\hfill \\ \begin{array}{cc}{\varphi }_{2,n}=t^{k_y-n}{e}^{-t/2}{L}_n^{(2k_y-2n)}(t),& n=0,1,2,\dots .<[k_y]\end{array}\hfill \end{array}$$

(13)

where t = 2(A + iB)e^−x and $L_n^{(a)}(t)$ denote generalized Laguerre polynomials. The first point to note here is that for the energy levels to be real, it follows from Equation (13) that the corresponding eigenfunctions are normalizable when the condition k_y ≥ 0 holds. For k_y < 0, the wave functions are not normalizable, i.e., no bound states are possible.

Let us now examine the upper component, ϕ₁. Since ϕ₂ is known, one can always use the intertwining relation:

$$c{P}_{-}{\psi }_2=E{\psi }_1$$

(14)

to obtain ϕ₁. Nevertheless, for the sake of completeness, we present the explicit results for ϕ₁. In this case, the potential analogous to Equation (12) reads:

$$V_1(x)=k_y^2+{\left(A+iB\right)}^2{e}^{-2x}-(2k_y-1)(A+iB)e^{-x}$$

(15)

Clearly, V₁(x) can be obtained from V₂(x) by the replacement k_y → k_y − 1, and so, the solutions can be obtained from Equation (13) as:

$$\begin{array}{l}{E}_{1,n}=\pm c\sqrt{k_y^2-{(k_y-n-1)}^2}\hfill \\ \begin{array}{cc}{\varphi }_{1,n}=t^{k_y-n-1}{e}^{-t/2}{L}_n^{(2k_y-2n-2)}(t),& n=1,2,\dots .<[k_y-1]\end{array}\hfill \end{array}$$

(16)

Note that the n = 0 state is missing from the spectrum Equation (16), so that it is a singlet state. Furthermore, E_2,n+1 = E_1,n, so that the ground state is a singlet, while the excited ones are doubly degenerate. Similarly, the negative energy states are also paired. In this connection, we would like to note that {H, σ₃} = 0, and consequently, except for the ground state, there is particle hole symmetry. The wave functions for the holes are given by σ₃ψ. The precise structure of the wave functions of the original Dirac equation are as follows (we present only the positive energy solutions):

$$\begin{array}{l}{E}_0=0,{\psi }_0=\left(\begin{array}{c}0\\ {\varphi }_{2,0}\end{array}\right)\hfill \\ E_{n+1}=c\sqrt{k_y^2-{(k_y-n-1)}^2},{\psi }_{n+1}=\left(\begin{array}{c}{\varphi }_{1,n}\\ {\varphi }_{2,n+1}\end{array}\right),n=0,1,2,\dots \hfill \end{array}$$

(17)

It is interesting to note that the spectrum does not depend on the magnetic field. Furthermore, the dispersion relation is no longer linear, as it should be in the presence of interactions. It is also easily checked that when the magnetic field is reversed, i.e., A → −A and B → −B with the simultaneous change of k_y → −k_y, the two potentials V_1,2(x) = W(x) ± W′(x) go one into each other, V₁(x) ↔ V₂(x). Therefore, the solutions are correspondingly interchanged, ϕ_1,n ↔ ϕ_2,n and E_1,n ↔ E_2,n, but retain the same functional form as in Equations (13) and (16).

Therefore, we find that it is indeed possible to create bound states with an imaginary vector potential. We shall now demonstrate the above results for a second example.

2.2. Complex Hyperbolic Magnetic Field

Here, we choose f(x), which leads to an effective potential of the complex hyperbolic Rosen–Morse type:

$$\begin{array}{ccc}f(x)=Atanh(x-i\alpha ),& -\infty <x<\infty ,& A\text{and}\alpha \text{are real constants}\end{array}$$

(18)

In this case, the complex magnetic field is given by:

$${\mathcal{\text{B}}}_z(x)=A{\text{sech}}^2(x-i\alpha )$$

(19)

Note that for α = 0, we get back the results of [8,24]. Using Equation (18) in the second half of Equation (7), we find:

$$\left[-\frac{d^2}{d{x}^2}+U_2(x)\right]{\varphi }_2=({\mathit{\epsilon }}^2-k_y^2-A^2){\varphi }_2$$

(20)

where

$$U_2(x)=k_y^2-A(A+1){\text{sech}}^2(x-i\alpha )+2A{k}_{y}tanh(x-i\alpha )$$

(21)

This is the Hyperbolic Rosen–Morse potential with known energy values and eigenfunctions. In the present case, the eigenvalues and the corresponding eigenfunctions are given by [23,25]:

$$\begin{array}{l}\begin{array}{cc}{E}_{2,n}=\pm c\sqrt{A^2+k_y^2-{\left(A-n\right)}^2-\frac{A^2{k}_y^2}{{\left(A-n\right)}^2},}& n=0,1,2,\dots <[A-\sqrt{A{k}_y}]\end{array}\hfill \\ {\varphi }_{2,n}={\left(1-t\right)}^{s_1/2}{\left(1+t\right)}^{s_2/2}{P}_n^{(s_1,s_2)}(t)\hfill \end{array}$$

(22)

where $P_n^{(a,b)}(z)$ denotes Jacobi polynomials and:

$$\begin{array}{cc}t=tanhx,& s_{1,2}=A-n\pm \frac{A{k}_y}{A-n}\end{array}$$

(23)

The energy values corresponding to the upper component of the spinor can be found out by replacing A by (A − 1), and ϕ₁ can be found out using relation Equation (14).

3. η-Pseudo Hermiticity

Let us recall that a Hamiltonian is η-pseudo Hermitian if [21]:

$$\eta H{\eta }^{-1}=H^{†}$$

(24)

where η is a Hermitian operator. It is known that eigenvalues of a η-pseudo Hermitian Hamiltonian are either all real or are complex conjugate pairs [21]. In view of the fact that in the present examples, the eigenvalues are all real, one is tempted to conclude that the interactions are η pseudo Hermitian. To this end, we first consider case 1, and following [26], let us consider the Hermitian operator:

$$\begin{array}{cc}\eta =e^{-\theta p_x},& \theta =arctan\frac{B}{A}\end{array}$$

(25)

Then, it follows that:

$$\begin{array}{ccc}\eta c{\eta }^{-1}=c,& \eta p_x{\eta }^{-1}=p_x,& \eta V(x){\eta }^{-1}=V(x+i\theta )\end{array}$$

(26)

We recall that in both the cases considered here, the Hamiltonian is of the form:

$$H=c\mathit{\sigma }\cdot \mathit{P}=c\left(\begin{array}{ll}0\hfill & P_{-}\hfill \\ P_{+}\hfill & 0\hfill \end{array}\right)$$

(27)

where, for the first example:

$$P_{\pm }=p_x\pm i{p}_y+i\left(A+iB\right)e^{-x}$$

(28)

Then:

$$H^{†}=c\left(\begin{array}{ll}0\hfill & P_{+}^{†}\hfill \\ P_{-}^{†}\hfill & 0\hfill \end{array}\right)$$

(29)

Now, from Equation (28), it follows that:

$$\begin{array}{cc}{P}_{+}^{†}=p_x-i{p}_y-i(A-iB)e^{-x},& P_{-}^{†}\end{array}=p_x+i{p}_y+i(A-iB)e^{-x}$$

(30)

and using Equation (26), it can be shown that:

$$\begin{array}{cc}\eta P_{+}{\eta }^{-1}=p_x+i{p}_y+i(A-iB)e^{-x}=P_{-}^{†},& \eta P_{-}{\eta }^{-1}=p_x-i{p}_y-i(A-iB)e^{-x}=P_{+}^{†}\end{array}$$

(31)

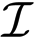Next, to demonstrate the pseudo Hermiticity of the Dirac Hamiltonian Equation (27), let us consider the operator η′ = η · ₂, where 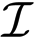₂ is the (2 × 2) unit matrix. Then, it can be shown that:

$${\eta }^{\prime }H{{\eta }^{\prime }}^{-1}=H^{†}$$

(32)

Thus, the Dirac Hamiltonian with a complex decaying magnetic field Equation (10) is η-pseudo Hermitian.

For the magnetic field given by Equation (19), the operator, η, can be found by using relations Equation (26). After a straightforward calculation, it can be shown that the η operator is given by:

$$\eta =e^{-2\alpha px}$$

(33)

so that, in this second example, also, the Dirac Hamiltonian is η-pseudo Hermitian.

4. Conclusions

Here, we have studied the (2 + 1)-dimensional massless Dirac equation (we note that if a massive particle of mass m is considered, the energy spectrum in the first example would become $E_n=c\sqrt{k_y^2+m^2{c}^2-{(k_y-n)}^2}$. Similar changes will occur in the second example, too). in the presence of complex magnetic fields, and it has been shown that such magnetic fields can create bound states. It has also been shown that Dirac Hamiltonians in the presence of such magnetic fields are η-pseudo Hermitian. We feel it would be of interest to study the generation of bound states using other types of magnetic fields, e.g., periodic magnetic fields.