Low-Order Moments of Velocity Gradient Tensors in Two-Dimensional Isotropic Turbulence
Abstract
:1. Introduction
2. Analytical Studies on the Components of Velocity Gradient Tensors in 2D Turbulence
2.1. Decomposition of the Velocity Gradient Tensor
2.2. Isotropic Expressions for and
2.3. Homogeneity Constraints to Second- and Third-Order Invariants
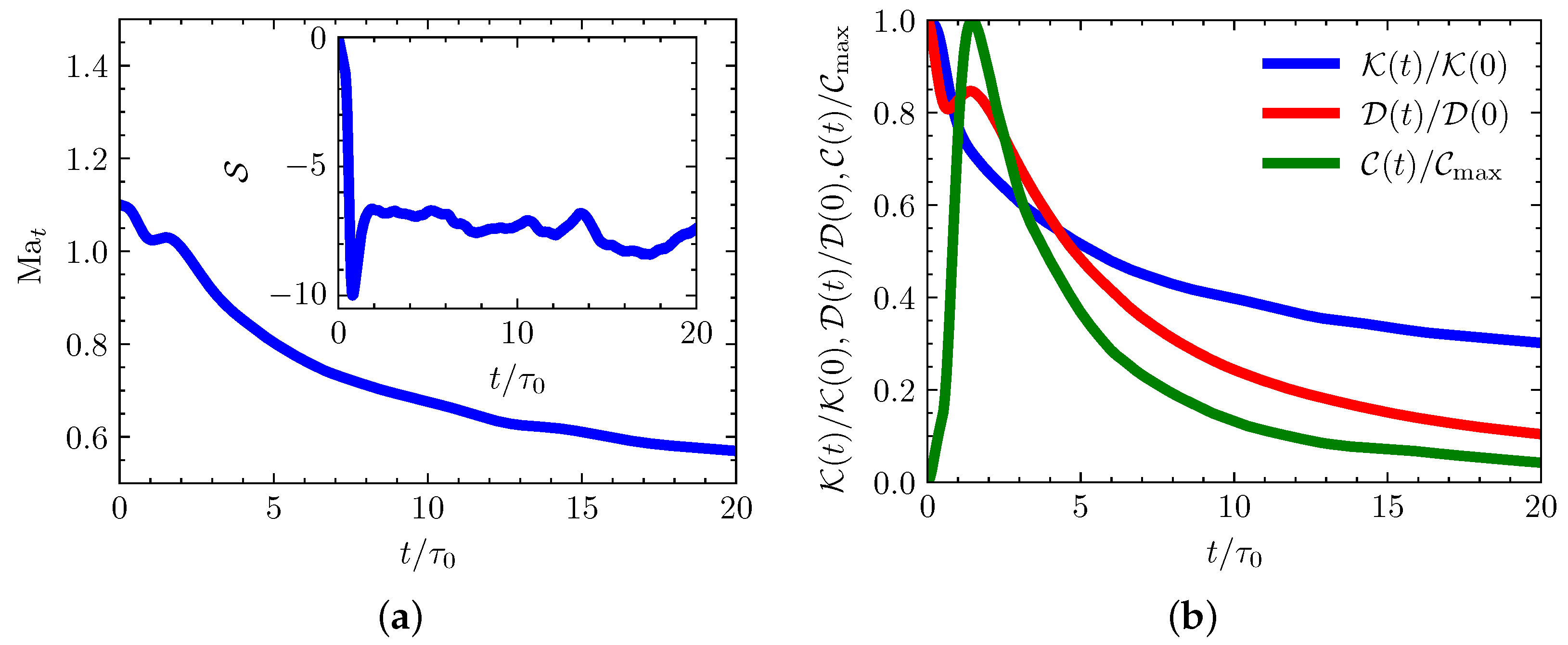
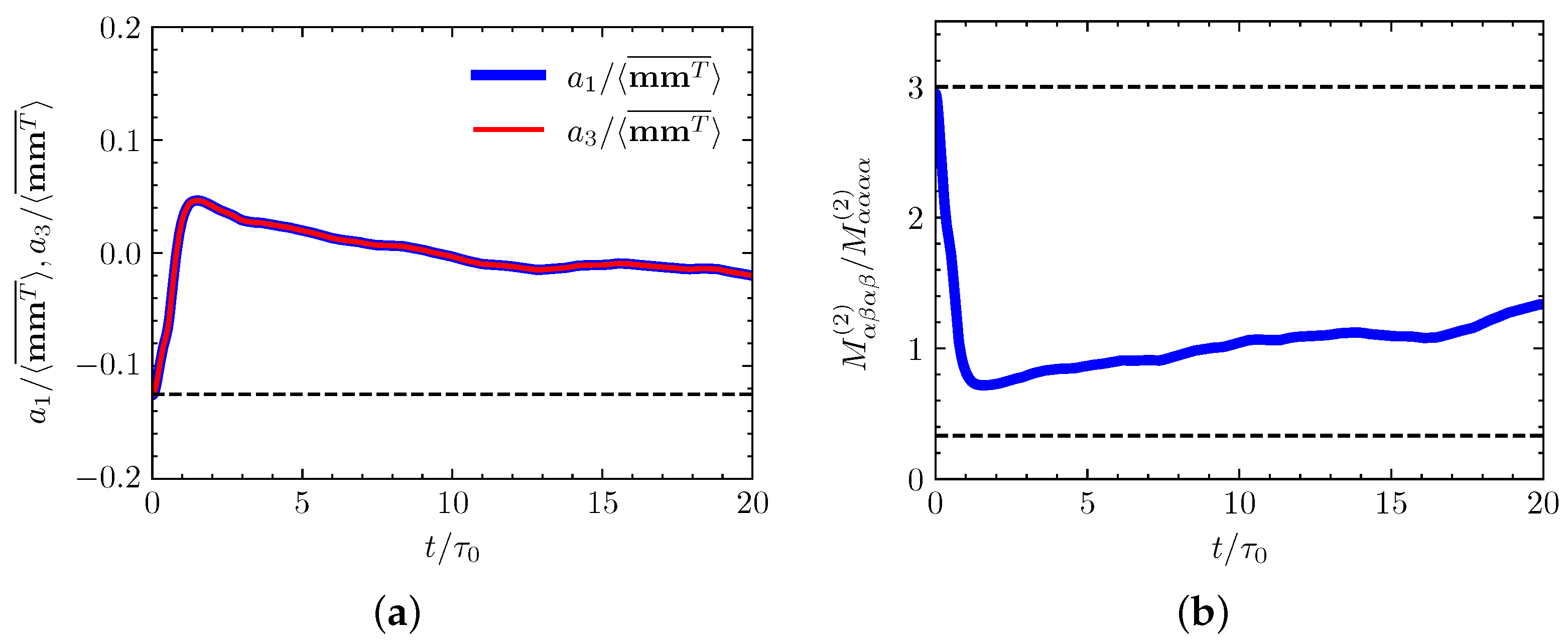
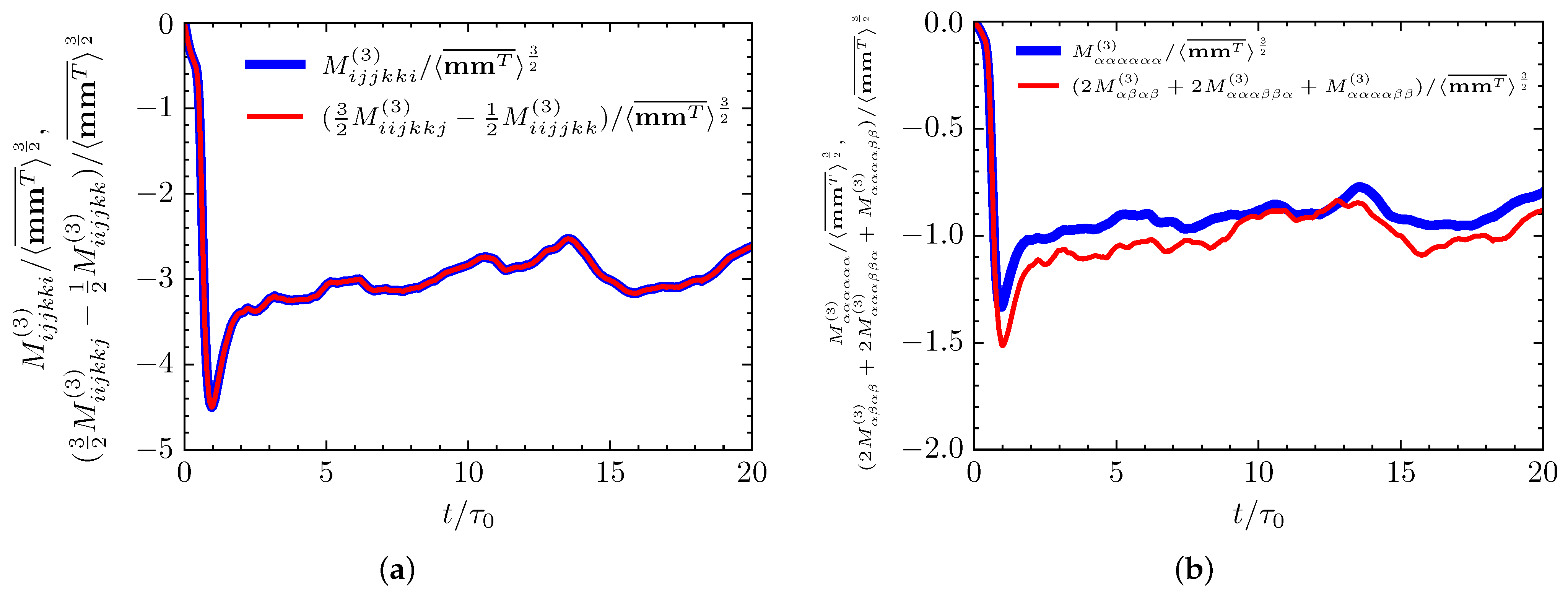
3. Numerical Validation of Independent Components
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. The Relation of θ, ω, ψ, ϕ in Fourier Space
References
- Kolmogorov, A. Dissipation of energy in locally isotropic turbulence. Dokl. Akad. Nauk SSSR 1941, 32, 16–18. [Google Scholar]
- Kolmogorov, A. The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Dokl. Akad. Nauk SSSR 1941, 30, 301–305. [Google Scholar]
- Tsinober, A. An Informal Conceptual Introduction to Turbulence; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Meneveau, C. Lagrangian Dynamics and Models of the Velocity Gradient Tensor in Turbulent Flows. Annu. Rev. Fluid Mech. 2011, 43, 219–245. [Google Scholar]
- Davidson, P.A. Turbulence: An Introduction for Scientists and Engineers; Oxford University Press: Oxford, UK, 2015. [Google Scholar] [CrossRef]
- Taylor, G.I. The spectrum of turbulence. Proc. R. Soc. Lond. Ser. A-Math. Phys. Sci. 1938, 164, 476–490. [Google Scholar] [CrossRef]
- Betchov, R. An inequality concerning the production of vorticity in isotropic turbulence. J. Fluid Mech. 1956, 1, 497–504. [Google Scholar] [CrossRef]
- Champagne, F.H. The fine-scale structure of the turbulent velocity field. J. Fluid Mech. 1978, 86, 67–108. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Yang, P.F.; Fang, J.; Fang, L.; Pumir, A.; Xu, H. Low-order moments of the velocity gradient in homogeneous compressible turbulence. J. Fluid Mech. 2022, 947, R1. [Google Scholar] [CrossRef]
- Carbone, M.; Wilczek, M. Only two Betchov homogeneity constraints exist for isotropic turbulence. J. Fluid Mech. 2022, 948, R2. [Google Scholar] [CrossRef]
- Siggia, E.D. Invariants for the one-point vorticity and strain rate correlation functions. Phys. Fluids 1981, 24, 1934–1936. [Google Scholar] [CrossRef]
- Hierro, J.; Dopazo, C. Fourth-order statistical moments of the velocity gradient tensor in homogeneous, isotropic turbulence. Phys. Fluids 2003, 15, 3434–3442. [Google Scholar] [CrossRef]
- Fang, L.; Zhang, Y.; Fang, J.; Zhu, Y. Relation of the fourth-order statistical invariants of velocity gradient tensor in isotropic turbulence. Phys. Rev. E 2016, 94, 023114. [Google Scholar] [CrossRef] [PubMed]
- Tabeling, P. Two-dimensional turbulence: A physicist approach. Phys. Rep. 2002, 362, 1–62. [Google Scholar] [CrossRef]
- Clercx, H.; van Heijst, G. Two-Dimensional Navier–Stokes Turbulence in Bounded Domains. Appl. Mech. Rev. 2009, 62, 020802. [Google Scholar] [CrossRef]
- Boffetta, G.; Ecke, R.E. Two-dimensional turbulence. Annu. Rev. Fluid Mech. 2012, 44, 427–451. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Inertial ranges in two-dimensional turbulence. Phys. Fluids 1967, 10, 1417–1423. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Inertial-range transfer in two- and three-dimensional turbulence. J. Fluid Mech. 1971, 47, 525–535. [Google Scholar] [CrossRef]
- Falkovich, G.; Kritsuk, A.G. How vortices and shocks provide for a flux loop in two-dimensional compressible turbulence. Phys. Rev. Fluids 2017, 2, 092603. [Google Scholar] [CrossRef]
- Dahlburg, J.; Dahlburg, R.; Gardner, J.; Picone, J. Inverse cascades in two-dimensional compressible turbulence. I. Incompressible forcing at low Mach number. Phys. Fluids A Fluid Dyn. 1990, 2, 1481–1486. [Google Scholar] [CrossRef]
- Alexakis, A.; Biferale, L. Cascades and transitions in turbulent flows. Phys. Rep. 2018, 767–769, 1–101. [Google Scholar] [CrossRef]
- Fouxon, I.; Kritsuk, A.G.; Mond, M. Compressible two-dimensional turbulence: Cascade reversal and sensitivity to imposed magnetic field. New J. Phys. 2023, 25, 113005. [Google Scholar] [CrossRef]
- Pan, S.; Johnsen, E. The role of bulk viscosity on the decay of compressible, homogeneous, isotropic turbulence. J. Fluid Mech. 2017, 833, 717–744. [Google Scholar] [CrossRef]
- Fang, J.; Li, Z.; Lu, L. An optimized low-dissipation monotonicity-preserving scheme for numerical simulations of high-speed turbulent flows. J. Sci. Comput. 2013, 56, 67–95. [Google Scholar] [CrossRef]
- Lele, S.K. Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 1992, 103, 16–42. [Google Scholar] [CrossRef]
- Fang, J.; Gao, F.; Moulinec, C.; Emerson, D. An improved parallel compact scheme for domain-decoupled simulation of turbulence. Int. J. Numer. Methods Fluids 2019, 90, 479–500. [Google Scholar] [CrossRef]
- Gottlieb, S.; Shu, C.W. Total variation diminishing Runge–Kutta schemes. Math. Comput. 1998, 67, 73–85. [Google Scholar] [CrossRef]
- Fang, J.; Yao, Y.; Li, Z.; Lu, L. Investigation of low-dissipation monotonicity-preserving scheme for direct numerical simulation of compressible turbulent flows. Comput. Fluids 2014, 104, 55–72. [Google Scholar] [CrossRef]
- Fang, J.; Yao, Y.; Zheltovodov, A.A.; Li, Z.; Lu, L. Direct numerical simulation of supersonic turbulent flows around a tandem expansion-compression corner. Phys. Fluids 2015, 27, 125104. [Google Scholar] [CrossRef]
- Fang, J.; Zheltovodov, A.A.; Yao, Y.; Moulinec, C.; Emerson, D.R. On the turbulence amplification in shock-wave/turbulent boundary layer interaction. J. Fluid Mech. 2020, 897, A32. [Google Scholar] [CrossRef]
| Type | Examples | Expression with Independent Scalar |
|---|---|---|
| Type | Examples | Expression with Independent Scalar |
|---|---|---|
| , etc. | ||
| and | , etc. | |
| , etc. |
| Tensor Notation | Subscript Notation | Expression of and | Independent Scalar Expression |
|---|---|---|---|
| Tensor Notation | Subscript Notation | Expression of and | Independent Scalar Expression |
|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, C.; Yang, P.-F.; Fang, L. Low-Order Moments of Velocity Gradient Tensors in Two-Dimensional Isotropic Turbulence. Symmetry 2024, 16, 175. https://doi.org/10.3390/sym16020175
Luo C, Yang P-F, Fang L. Low-Order Moments of Velocity Gradient Tensors in Two-Dimensional Isotropic Turbulence. Symmetry. 2024; 16(2):175. https://doi.org/10.3390/sym16020175
Chicago/Turabian StyleLuo, Chensheng, Ping-Fan Yang, and Le Fang. 2024. "Low-Order Moments of Velocity Gradient Tensors in Two-Dimensional Isotropic Turbulence" Symmetry 16, no. 2: 175. https://doi.org/10.3390/sym16020175





