Solitary Solutions for the Stochastic Fokas System Found in Monomode Optical Fibers
Abstract
:1. Introduction
2. Traveling Wave Equation for SFS
3. Exact Solutions of SFS
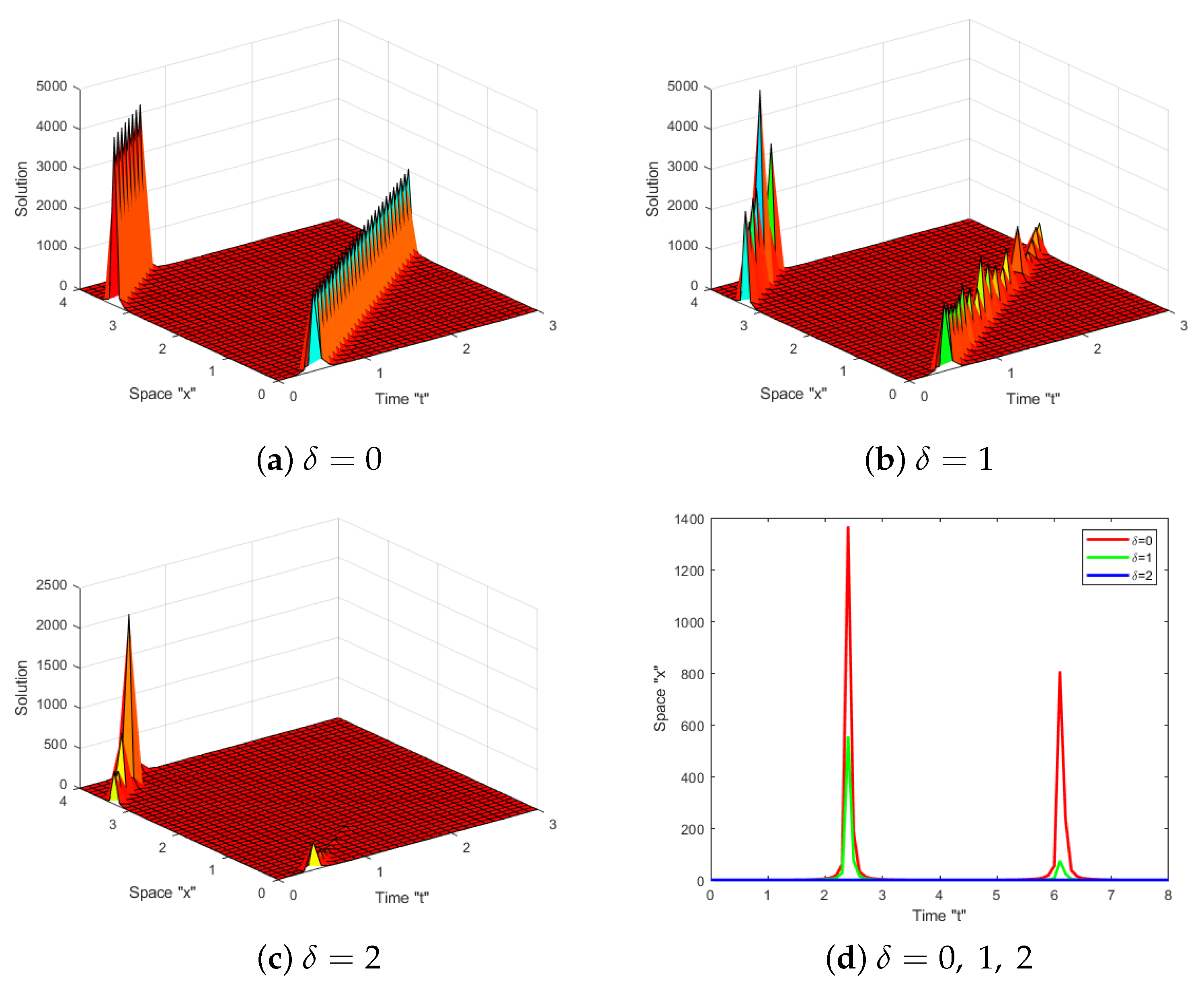
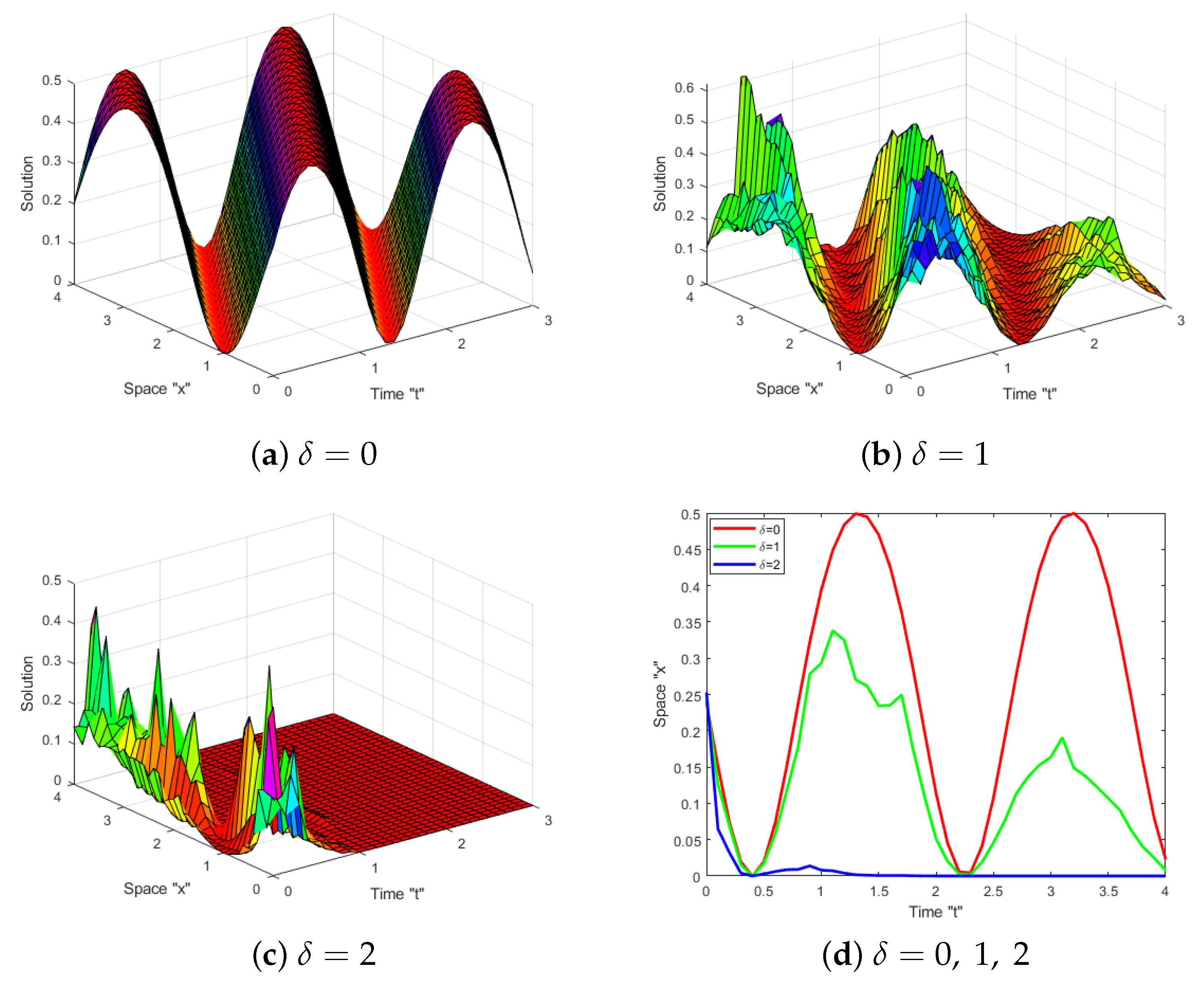
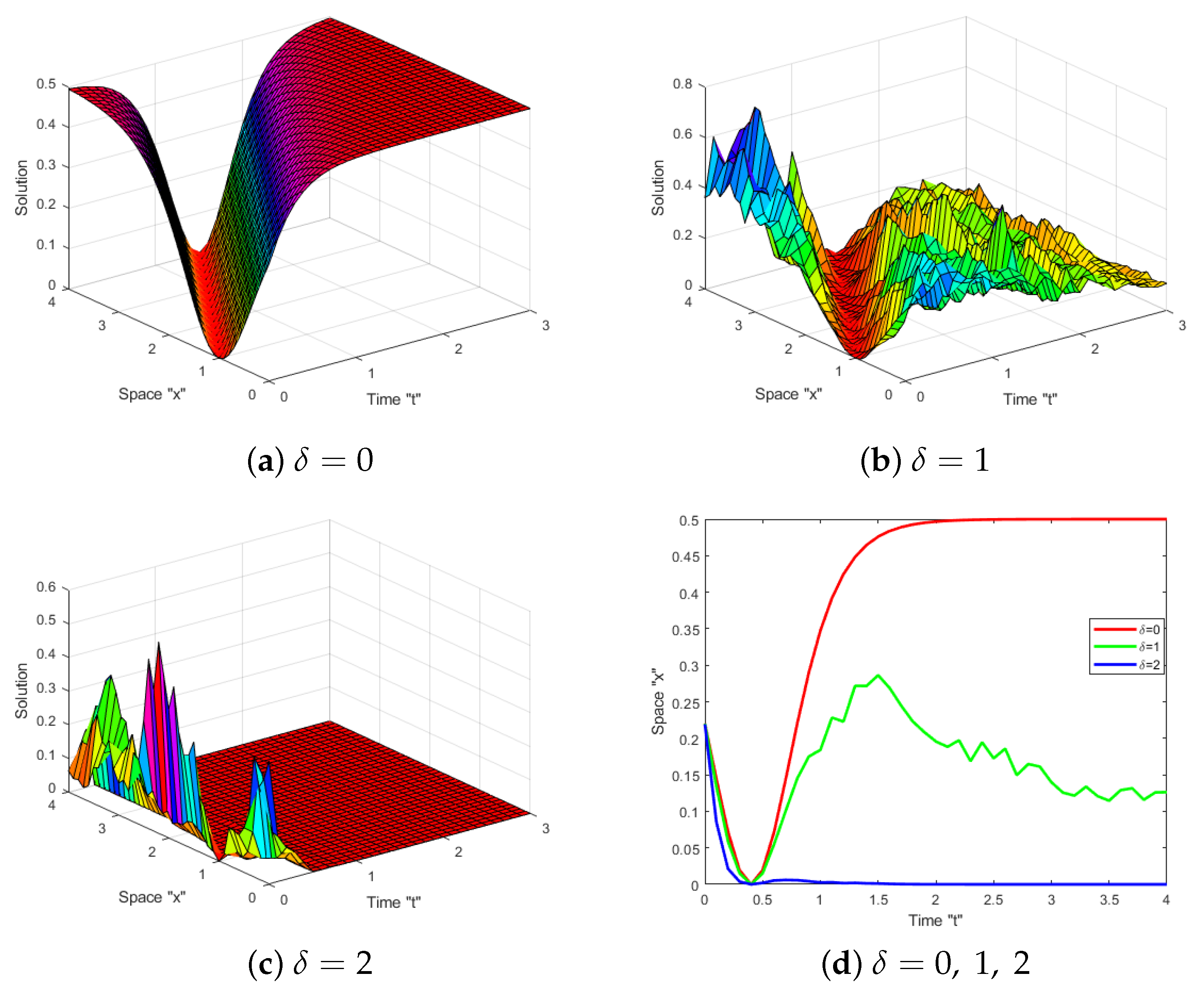
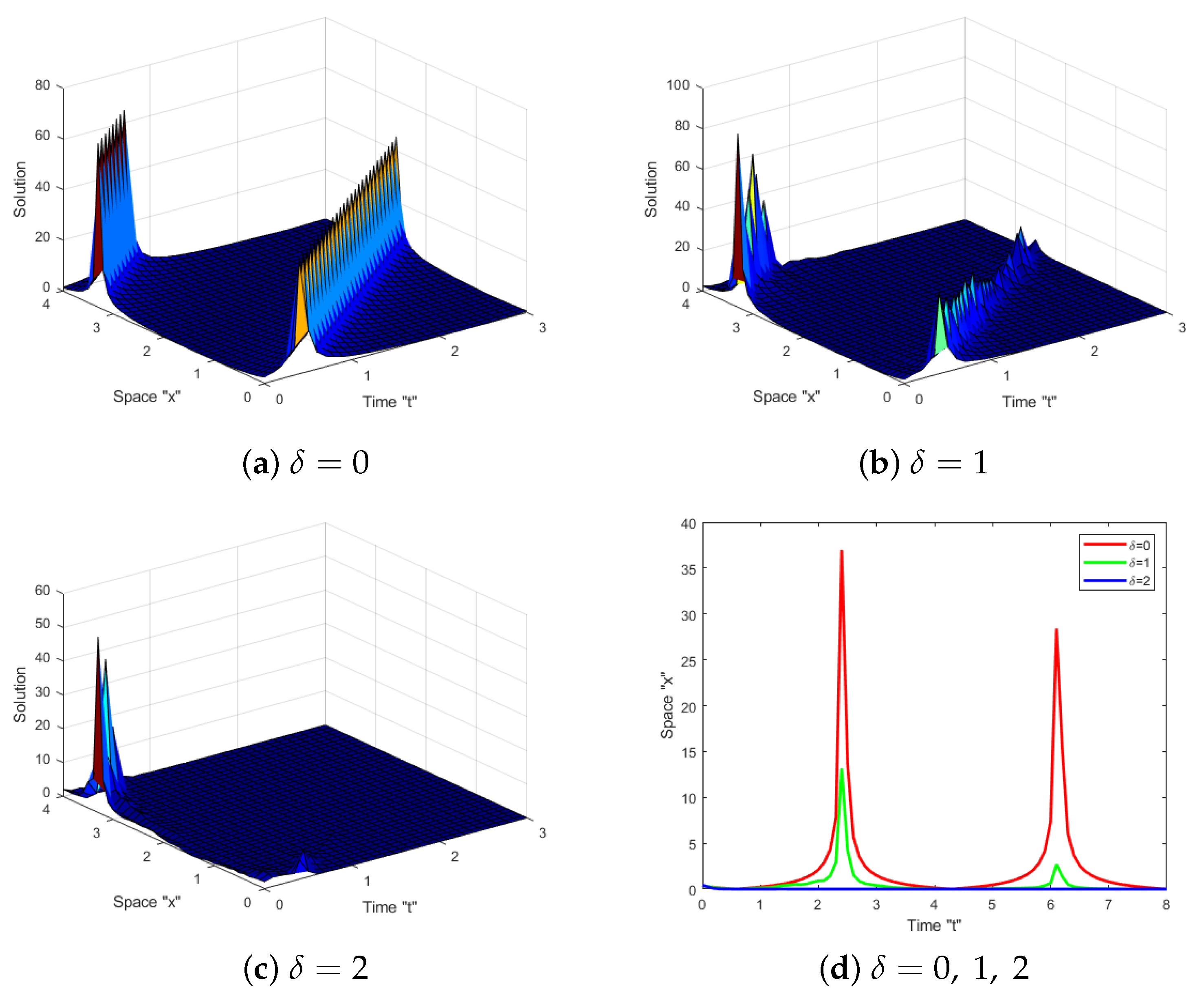
4. Impacts of Noise
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lu, B. The first integral method for some time fractional differential equations. J. Math Anal. Appl. 2012, 395, 684–693. [Google Scholar] [CrossRef] [Green Version]
- Wazwaz, A.M. The sine-cosine method for obtaining solutions with compact and noncompact structures. Appl. Math. Comput. 2004, 159, 559–576. [Google Scholar] [CrossRef]
- He, J.H.; Wu, X.H. Exp-function method for nonlinear wave equations. Chaos Solitons Fractals 2006, 30, 700–708. [Google Scholar] [CrossRef]
- Yan, Z.L. Abunbant families of Jacobi elliptic function solutions of the dimensional integrable Davey-Stewartson-type equation via a new method. Chaos Solitons Fractals 2003, 18, 299–309. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Al-Askar, F.M.; Cesarano, C. The Analytical Solutions of the Stochastic mKdV Equation via the Mapping Method. Mathematics 2022, 10, 4212. [Google Scholar] [CrossRef]
- Jiong, S. Auxiliary equation method for solving nonlinear partial differential equations. Phys. Lett. A 2003, 309, 387–396. [Google Scholar]
- Al-Askar, F.M.; Cesarano, C.; Mohammed, W.W. Abundant Solitary Wave Solutions for the Boiti-Leon-Manna-Pempinelli Equation with M-Truncated Derivative. Axioms 2023, 12, 466. [Google Scholar] [CrossRef]
- Arnous, A.H.; Mirzazadeh, M. Application of the generalized Kudryashov method to Eckhaus equation. Nonlinear Anal Model. Control. 2016, 21, 577–586. [Google Scholar] [CrossRef] [Green Version]
- Khan, K.; Akbar, M.A. The exp(-ϕ(ς))-expansion method for finding travelling wave solutions of Vakhnenko-Parkes equation. Int. J. Dyn. Syst. Differ. Equ. 2014, 5, 72–83. [Google Scholar]
- Al-Askar, F.M.; Cesarano, C.; Mohammed, W.W. The Influence of White Noise and the Beta Derivative on the Solutions of the BBM Equation. Axioms 2023, 12, 447. [Google Scholar] [CrossRef]
- Preethi, G.T.; Magesh, N.; Gatti, N.B. An Application of Conformable Fractional Differential Transform Method for Smoking Epidemic Model. Math. Comput. 2022, 415, 399–411. [Google Scholar]
- Veeresha, P.; Prakasha, D.G.; Magesh, N.; Christopher, A.J.; Sarwe, D.U. Solution for fractional potential KdV and Benjamin equations using the novel technique. J. Ocean Eng. Sci. 2021, 6, 265–275. [Google Scholar] [CrossRef]
- Elmandouh, A.; Fadhal, E. Bifurcation of Exact Solutions for the Space-Fractional Stochastic Modified Benjamin-Bona-Mahony Equation. Fractal Fract. 2022, 6, 718. [Google Scholar] [CrossRef]
- Alhamud, M.; Elbrolosy, M.; Elmandouh, A. New Analytical Solutions for Time-Fractional Stochastic (3 + 1)-Dimensional Equations for Fluids with Gas Bubbles and Hydrodynamics. Fractal Fract. 2023, 7, 16. [Google Scholar] [CrossRef]
- Wang, M.L.; Li, X.Z.; Zhang, J.L. The (G′/G)-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 2008, 372, 417–423. [Google Scholar] [CrossRef]
- Zhang, H. New application of the (G′/G)-expansion method. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 3220–3225. [Google Scholar] [CrossRef]
- Hydon, P.E. Symmetry Methode for differential Aquations; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Arnold, L. Random Dynamical Systems; Springer: New York, NY, USA, 1998. [Google Scholar]
- Imkeller, P.; Monahan, A.H. Conceptual stochastic climate models. Stoch. Dyn. 2002, 2, 311–326. [Google Scholar] [CrossRef] [Green Version]
- Al-Askar, F.M.; Cesarano, C.; Mohammed, W.W. The Solitary Solutions for the Stochastic JimboMiwa Equation Perturbed by White Noise. Symmetry 2023, 15, 1153. [Google Scholar] [CrossRef]
- Al-Askar, F.M.; Cesarano, C.; Mohammed, W.W. Multiplicative Brownian Motion Stabilizes the Exact Stochastic Solutions of the Davey–Stewartson Equations. Symmetry 2022, 14, 2176. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Cesarano, C. The soliton solutions for the (4 + 1)-dimensional stochastic Fokas equation. Math. Methods Appl. Sci. 2023, 46, 7589–7597. [Google Scholar] [CrossRef]
- Fokas, A.S. On the simplest integrable equation in 2 + 1. Inverse Probl. 1994, 10, L19. [Google Scholar] [CrossRef] [Green Version]
- Shulman, E.I. On the integrability of equations of Davey Stewartson type. Teor. Mat. Fiz. 1983, 56, 131–136. [Google Scholar] [CrossRef]
- Wang, K.J. Abundant exact soliton solutions to the Fokas system. Optik 2022, 249, 168265. [Google Scholar] [CrossRef]
- Tarla, S.; Ali, K.K.; Sun, T.C.; Yilmazer, R.; Osman, M.S. Nonlinear pulse propagation for novel optical solitons modeled by Fokas system in monomode optical fibers. Results Phys. 2022, 36, 1053. [Google Scholar] [CrossRef]
- Wang, K.J.; Liu, J.H.; Wu, J. Soliton solutions to the Fokas system arising in monomode optical fibers. Optik 2022, 251, 168319. [Google Scholar] [CrossRef]
- Zhang, P.L.; Wang, K.J. Abundant optical soliton structures to the Fokas system arising in monomode optical fibers. Open Phys. 2022, 20, 493–506. [Google Scholar] [CrossRef]
- Rao, J.; Mihalache, D.; Cheng, Y.; He, J. Lump-soliton solutions to the Fokas system. Phys. Lett. A 2019, 383, 1138–1142. [Google Scholar] [CrossRef]
- Kaplan, M.; Akbulut, A.; Alqahtani, R.T. New Solitary Wave Patterns of the Fokas System in Fiber Optics. Mathematics 2023, 11, 1810. [Google Scholar] [CrossRef]
- Bhrawy, A.H.; Abdelkawy, M.A.; Kumar, S.; Johnson, S.; Biswas, A. Solitons and other solutions to quantum Zakharov–Kuznetsov equation in quantum magneto-plasmas. Indian J. Phys. 2013, 87, 455–463. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammed, W.W.; Al-Askar, F.M.; Cesarano, C. Solitary Solutions for the Stochastic Fokas System Found in Monomode Optical Fibers. Symmetry 2023, 15, 1433. https://doi.org/10.3390/sym15071433
Mohammed WW, Al-Askar FM, Cesarano C. Solitary Solutions for the Stochastic Fokas System Found in Monomode Optical Fibers. Symmetry. 2023; 15(7):1433. https://doi.org/10.3390/sym15071433
Chicago/Turabian StyleMohammed, Wael W., Farah M. Al-Askar, and Clemente Cesarano. 2023. "Solitary Solutions for the Stochastic Fokas System Found in Monomode Optical Fibers" Symmetry 15, no. 7: 1433. https://doi.org/10.3390/sym15071433