Statistical Analyses of a Class of Random Cyclooctatetraene Chain Networks with Respect to Several Topological Properties

Abstract

In recent years, the research on complex networks has created a boom. The objective of the present paper is to study a random cyclooctatetraene chain whose graph-theoretic mathematical properties arose scientists’ interests. By applying the concept of symmetry and probability theory, we obtain the explicit analytical expressions for the variances of Schultz index, multiplicative degree-Kirchhoff index Gutman index, and additive degree-Kirchhoff index of a random cyclooctatetraene chain with n octagons, which plays a crucial role in the research and application of topological indices.

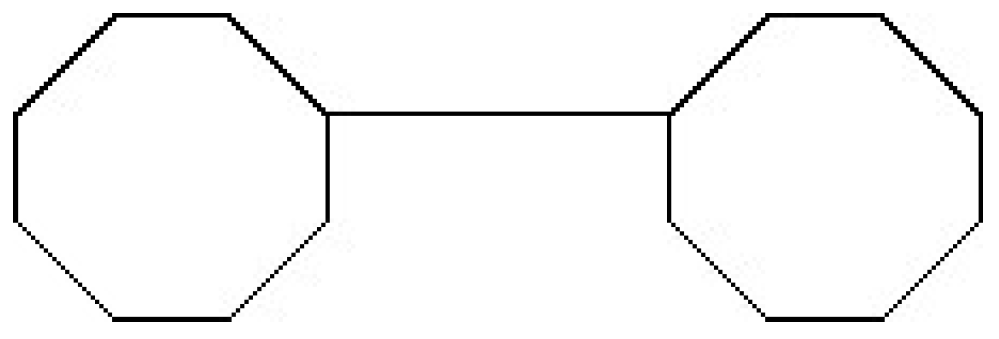
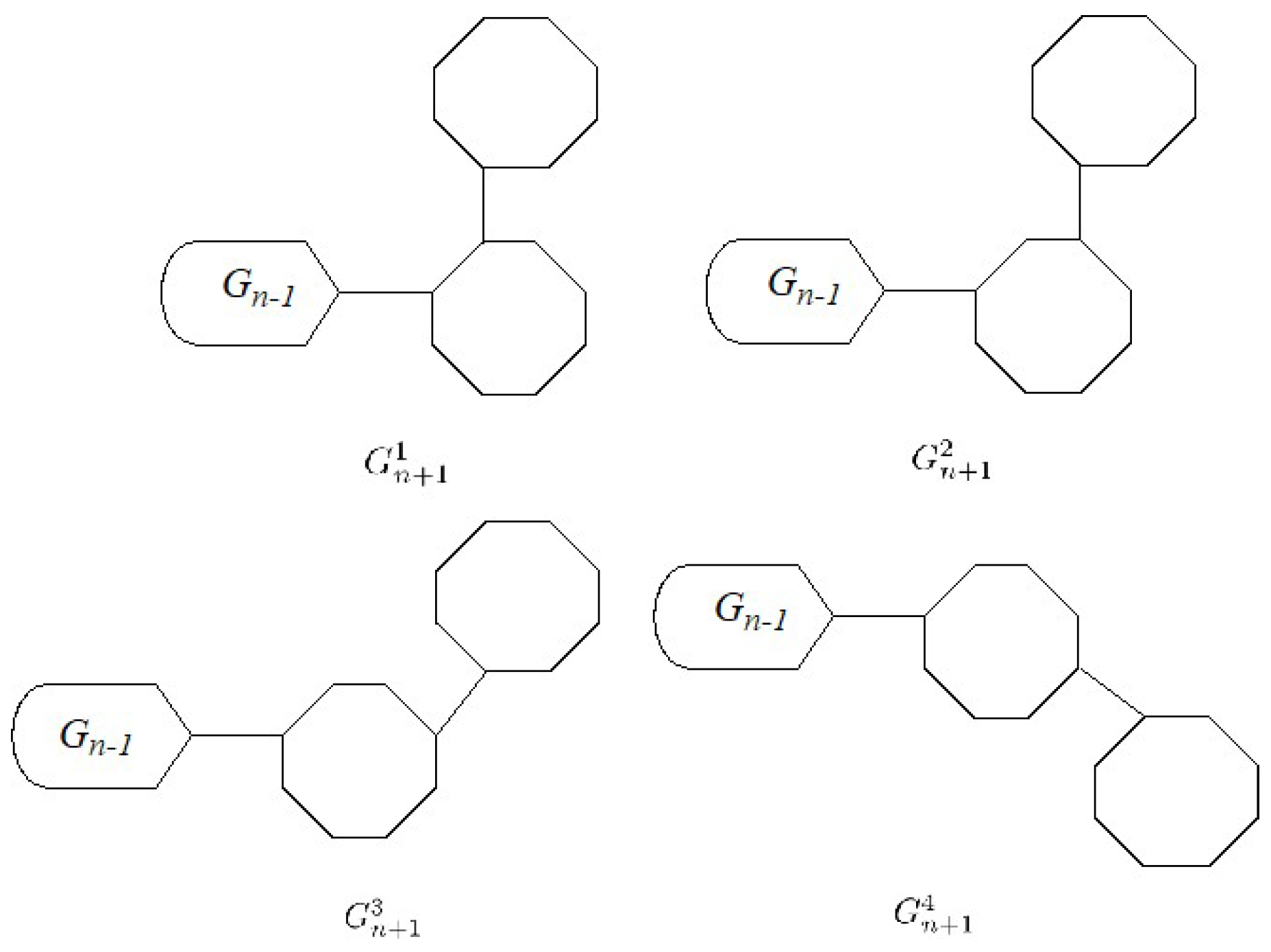
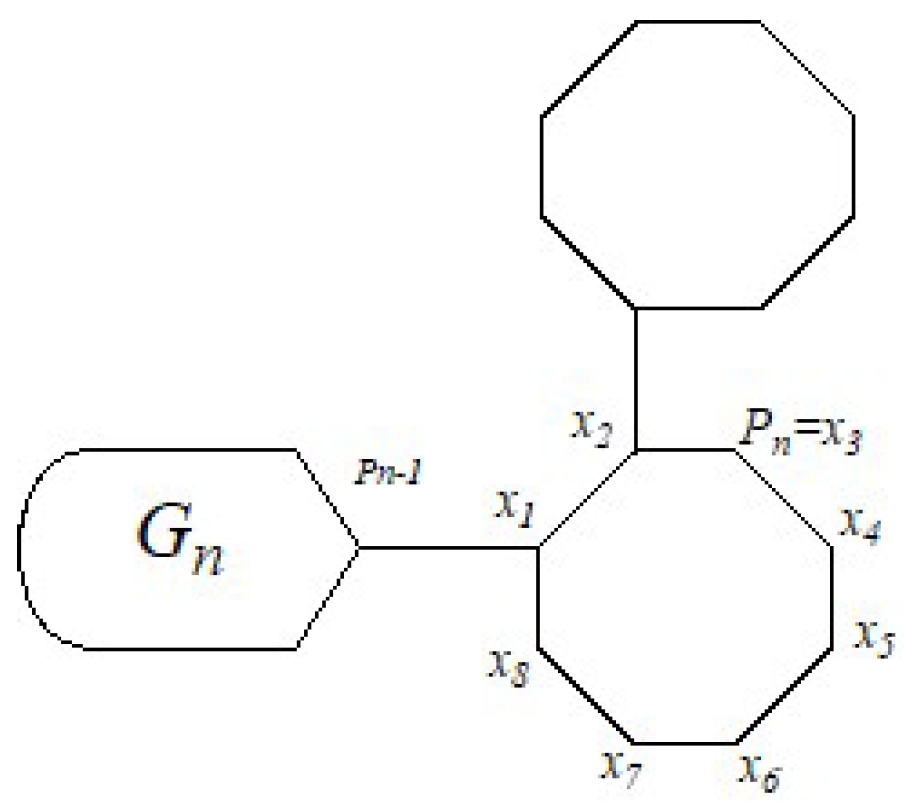
1. Introduction

In this paper, we just take finite and simple connected graphs into consideration, referring to [1] and the references cited therein. Chemistry is strongly linked to graph theory, and graph theory has been widely used in chemistry. In chemical graph theory, there are some alternatives between atoms that vertices represent the atoms and edges represent the covalent bonds. They are used to depict chemical compounds.

The molecular formula of a compound can represent different molecular structures and characteristics, but theoretical chemists are concerned with the physical and chemical properties of a compound and its relationship with the molecular formula of a compound. The physical and chemical properties of compounds are key areas of concern for theoretical chemists [2,3]. Polygonal chemicals have a wide diversity of molecular structures and their physical and chemical properties are becoming increasingly significant, which is referenced in [4,5,6,7].

There is no octagon in octagonal chains that has more than two cut-vertices, and such a octagonal chain is called a cyclooctatetraene chain. Cyclooctatetraene is the poster child for nonaromatic molecules. Cyclooctatetraene and its derivatives have fascinated chemists for a long time and have a wide range of applications in industry. Cyclooctatetraene differs from benzene in that it is not an aromatic hydrocarbon. It is chemically close to an unsaturated hydrocarbon. Not only can it be subjected to addition reactions, which is easily hydrogenated to form cyclooctane. It also oxidises and polymerises readily. A number of vital compounds of cyclooctatetraene can be served as substrates for the production of scientifically and commercially valuable materials. In this paper, we consider four kinds of indices of cyclooctatetraene chains with n octagons. We simply consider the situation starting from a vertex of a cyclooctatetraene chain, connecting an edge to another octagon. For more information about the cyclooctylene chain, we can refer to [8,9,10,11].

We give some basic notations. Set $G=(V_G,E_G)$ be a graph with vertices denoted $V_G$ and edges denoted $E_G$. The number of edges in a graph G is denoted by $\left|E\right(G\left)\right|$. We claim that two vertices p and m are adjacent (or neighbours) if they are attached by an edge, which we will write as $p\sim m$. In G, the shortest length $p,m$-path among the paths between two vertices p and m is denoted as $d_G(p,m)$ (or simply $d(p,m)$). The Wiener index of G refers to the aggregate of distances between all the vertex pairs of G. It was created by H. Wiener in 1947 [12], that is

$$W\left(G\right)=\sum _{\{p,m\}\subseteq V_G}d(p,m).$$

(1)

The Wiener index is among the best studied, most understood, and most widely used molecular shape descriptors, and is based on graph theory, see [13,14,15,16].

A graph $G=(V_G,E_G)$ together with the weight function $\omega$:$V_G\to {\mathbb{N}}^{+}$ is known as a weighted graph [17] $(G,\omega )$. Let ⊕ denote one of the four arithmetic operations $+,-,\times ,÷$ [18]. Consequently, the weighted Wiener index $W(G,\omega )$ is determined as

$$W(G,\omega )=\frac{1}{2}\sum _{p\in V_G}\sum _{m\in V_G}(\omega \left(p\right)\oplus \omega \left(m\right))d(p,m).$$

(2)

Obviously, if $\omega \equiv 1$ and ⊕ denotes the operation ×, then $W(G,\omega )=W\left(G\right)$.

In case ⊕ represents the operation × and $\omega (·)\equiv d_G(·)$, then (2) is equivalent to [19]

$$Gut\left(G\right)=\frac{1}{2}\sum _{p\in V_G}\sum _{m\in V_G}\left(d_G\left(p\right)d_G\left(m\right)\right)d(p,m)=\sum _{\{p.m\}\subseteq V_G}\left(d_G\left(p\right)d_G\left(m\right)\right)d(p,m).$$

(3)

This is just the Gutman index. Research on possible chemical applications of the Gutman index and similar quantities and their theoretical study, for which polycyclic molecules are more difficult cases, see [20].

In case ⊕ represents the operation + and $\omega (·)\equiv d_G(·)$, then (2) is equivalent to

$$S\left(G\right)=\frac{1}{2}\sum _{p\in V_G}\sum _{m\in V_G}(d_G\left(p\right)+d_G\left(m\right))d(p,m)=\sum _{\{p.m\}\subseteq V_G}(d_G\left(p\right)+d_G\left(m\right))d(p,m).$$

(4)

That’s what the Schultz Index is all about. For additional articles on developing aspects of the topology indexes for [21,22,23,24], including mathematical properties, discrimination and applications, refer to [25].

In case of $a,b\in V\left(G\right)$, the resistance distance between a and b in G, is defined as the effective resistance between nodes a and b in the electrical network, where the nodes correspond to vertices of G and each edge of G is replaced by a resistor of unit resistance. The resistance distance between vertices a and b in G [26] is denoted as $r(a,b)$. For more detailed information, see [27,28,29,30]. It is the Kirchhoff index when the Wiener index is for non-trees, and this distance function is proposed by Klein and Randić [31], defined as

$$Kf\left(G\right)=\sum _{\{a,b\}\subseteq V_G}r(a,b).$$

(5)

For a description of the sum of eccentricity distances and the sum of eccentricity resistance-distances, refer to [32,33,34,35,36].

$K{f}^{*}\left(G\right)$ was introduced by Chen and Zhang in 2007 [37] (see [38] for details), which is defined as

$$K{f}^{*}\left(G\right)=\sum _{\{a,b\}\subseteq V_G}d\left(a\right)d\left(b\right)r(a,b),$$

(6)

Thus, the invariance of this graph is represented as

$$K{f}^{*}\left(G\right)=2\left|E_G\right|\sum _{i=2}^n\frac{1}{{\lambda }_i},$$

(7)

from which $0={\lambda }_1<{\lambda }_2\le \cdots \le {\lambda }_n$ are the eigenvalues of $\mathcal{l}\left(G\right)$. Moreover, $\mathcal{l}\left(G\right)$ is the normalized Laplacian matrix of G, as proposed by Chung [39]. The normalized Laplacian index and multiplicative degree-Kirchhoff index play an essential application in mathematical chemistry and statistics. Research on these topics has attracted a wide range of attention from researchers.

$K{f}^{+}\left(G\right)$ was introduced by Gutman, Feng and Yu in 2012 [40], which is defined as

$$K{f}^{+}\left(G\right)=\sum _{\{a,b\}\subseteq V_G}(d\left(a\right)+d\left(b\right))r(a,b).$$

(8)

Probability properties are an important component of chemical graphs, which can more broadly describe the topological properties and structures of chemical graphs. In this paper, we focus on the cyclooctatetraene chain $G_n$ with n octagons, which is formed as follows.

Firstly, $G_1$ is an octagon and $G_2$ is the graph with two octagons, as shown in Figure 1.

Figure 1. Graph $G_2$.

Secondly, by appending a new terminal octagon $H_{n+1}$ to $G_n$, the random cyclooctatetraene chain $G_{n+1}$ with n octagons can be constructed. We illustrates this process in Figure 2. As demonstrated in Figure 3, we can append the terminal octagon $H_{n+1}$ to $G_n$ with four methods and indicate the generated figures with $G_{n+1}^1$, $G_{n+1}^2$, $G_{n+1}^3$, and $G_{n+1}^4$, respectively.

Figure 2. The construction of $G_{n+1}$ from $G_n$ and $H_{n+1}$.

Figure 3. Four ways to attach the new terminal octagon $H_{n+1}$ to $G_n$.

At each step, randomly pick one of the following possible outcomes:

• $p_1$ is the probability that $G_n\to G_{n+1}^1$;
• $p_2$ is the probability that $G_n\to G_{n+1}^2$
• $p_3$ is the probability that $G_n\to G_{n+1}^3$
• $p_4=1-p_1-p_2-p_3$ is the probability that $G_n\to G_{n+1}^4$.

We consider four random variables $Z_n^1$, $Z_n^2$, $Z_n^3$, and $Z_n^4$ for our choice. When $i=1,2,3,4$, in case our selection is $G_{n+1}^i$, we have $Z_n^i=1$; otherwise, $Z_n^i=0$, and we can easily derive that

$$P(Z_n^i=1)=p_i,P(Z_n^i=0)+P(Z_n^i=1)=1$$

(9)

and $Z_n^1+Z_n^2+Z_n^3+Z_n^4=1$.

Through the above process, we get a random cyclooctatetraene chain $G_n(p_1,p_2,p_3,p_4)$. We always abbreviate $G_n(p_1,p_2,p_3,p_4)$ to $G_n$. All of $S\left(G_n\right)$, $K{f}^{*}\left(G_n\right)$, $Gut\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$ are random variables in probability by noting that $G_n$ is a random graph. From a probabilistic point of view, a natural question arises: when n is big enough, will the distribution of $S\left(G_n\right)$, $K{f}^{*}\left(G_n\right)$, $Gut\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$ look like a probability distribution or not.

In this paper, we make researches on $S\left(G_n\right)$, $K{f}^{*}\left(G_n\right)$, $Gut\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$. There is a huge amount of relevant literature. For random polyphenylene chain, J.I. Zhang, X.H. Peng, H.I. Chen [18] established the limiting behaviours of $S\left(G_n\right)$, $K{f}^{*}\left(G_n\right)$, $Gut\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$. Here it is natural and interesting to consider limiting behaviours of $S\left(G_n\right)$, $K{f}^{*}\left(G_n\right)$, $Gut\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$ for random cyclooctatetraene chain. In this paper, by applying the concept of symmetry and probability theory, we derive definite analytical expressions for the variance of the Schultz index, multiplicative degree-Kirchhoff index, Gutman index and additive degree-Kirchhoff index of a random cyclooctatetraene chain with n octagons, which plays a crucial role in the research and application of topological indices.

In this paper, in order to better describe the probability properties of cyclooctylene chain, we propose the following hypothesis.

Hypothesis 1.

We choose to attach the new terminal octagon $H_{n+1}$ to $G_n$, where $n=2,3,...$, and it is random and independent. More precisely, a range of random variables ${Z_n^1,Z_n^2,Z_n^3,Z_n^4}_{n=2}^{\infty }$ is independent and has the same law (9). With regard to some $i\in 1,2,3$, $0<p_i<1$ is obvious. Based on Hypothesis 1, we present analytical expressions for the variances of $S\left(G_n\right)$, $K{f}^{*}\left(G_n\right)$, $Gut\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$.

2. The Variances for the Gutman Index and Schultz Index of a Random Cyclooctatetraene Chain

For all $G_n$, the Gutman index and Schultz index of a random cyclooctatetraene chain are random variables. We are going to take the variances of $Gut\left(G_n\right)$ and $S\left(G_n\right)$ into consideration in this section. Actually, $G_{n+1}$ is $G_n$ connected by an edge to a new terminal octagon $H_{n+1}$, where $H_{n+1}$ is extended by vertices $x_1$, $x_2$, $x_3$, $x_4$, $x_5$, $x_6$, $x_7$, and $x_8$, and $p_n{x}_1$ is the new edge; see Figure 2. On the one hand, for all $m\in V_{G_n}$,

$$d(x_1,m)=d(p_n,m)+1,d(x_2,m)=d(p_n,m)+2,d(x_3,m)=d(p_n,m)+3,d(x_4,m)=d(p_n,m)+4,$$

(10)

$$d(x_5,m)=d(p_n,m)+5,d(x_6,m)=d(p_n,m)+4,d(x_7,m)=d(p_n,m)+3,d(x_2,m)=d(p_n,m)+2,$$

(11)

$$\sum _{m\in V_{G_n}}{d}_{G_{n+1}}\left(m\right)=18n-1.$$

(12)

on the other hand, for all $t\in \{1,2,3,4,5,6,7,8\}$

$$\sum _{t=1}^{8}d\left(x_t\right)d(x_1,x_t)=32,\sum _{t=1}^{8}d\left(x_t\right)d(x_2,x_t)=33,\sum _{t=1}^{8}d\left(x_t\right)d(x_3,x_t)=34,\sum _{t=1}^{8}d\left(x_t\right)d(x_4,x_t)=35,$$

(13)

$$\sum _{t=1}^{8}d\left(x_t\right)d(x_5,x_t)=36,\sum _{t=1}^{8}d\left(x_t\right)d(x_6,x_t)=35,\sum _{t=1}^{8}d\left(x_t\right)d(x_7,x_t)=34,\sum _{t=1}^{8}d\left(x_t\right)d(x_8,x_t)=33.$$

(14)

In [41] Theorem 1, the author proves that

$$\begin{array}{cc}\hfill E\left(Gut\left(G_n\right)\right)=& (270-162p_1-108p_2-54p_3)n^3+(486p_1+324p_2+162p_3-90)n^2\hfill \\ \hfill & +(77-324p_1-216p_2-108p_3)n-1.\hfill \end{array}$$

where $E(Gut\left(G_n\right)$ is the mathematical expectations of $Gut\left(G_n\right)$.

We now present the first main result of this section.

Theorem 1.

The results are as follows, if Hypothesis 1 holds. As to the random cyclooctatetraene chain $G_n$, the variance of Gutman index $Gut\left(G_n\right)$, computed as

$$\begin{array}{cc}\hfill Var\left(Gut\left(G_n\right)\right)& =\frac{1}{30}({\sigma }^2{n}^5-5r{n}^4+10{\tilde{\sigma }}^2{n}^3+(65r-30{\sigma }^2-45{\tilde{\sigma }}^2)n^2\hfill \\ \hfill & +(-120r+59{\sigma }^2+65{\tilde{\sigma }}^2)n+(60r-30{\sigma }^2-30{\tilde{\sigma }}^2)).\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill {\sigma }^2=& {648}^2{p}_1+{972}^2{p}_2+{1296}^2{p}_3+{1620}^2{p}_4-{(648p_1+972p_2+1296p_3+1620p_4)}^2\hfill \\ \hfill {\tilde{\sigma }}^2=& {90}^2{p}_1+{414}^2{p}_2+{738}^2{p}_3+{1062}^2{p}_4-{(90p_1+414p_2+738p_3+1062p_4)}^2\hfill \\ \hfill r=& 648·90·p_1+972·414·p_2+1296·738·p_3+1620·1062·p_4\hfill \\ \hfill & -(648p_1+972p_2+1296p_3+1620p_4)·(90p_1+414p_2+738p_3+1062p_4)\hfill \end{array}$$

Proof. 

Let

$$\begin{array}{c}\hfill A_n:=18\sum _{m\in V_{G_n}}d\left(m\right)d(p_n,m).\end{array}$$

then, by (5.1) of [41], we obtain

$$Gut\left(G_{n+1}\right)=Gut\left(G_n\right)+A_n+882n+239.$$

(15)

□

Recalling from Section 1 that $Z_n^1$, $Z_n^2$, $Z_n^3$, and $Z_n^4$ are random variables, this indicates our option in constructing $G_{n+1}$ from $G_n$. We have four equalities as follows:

Equality 1.

$$\begin{array}{c}\hfill A_n{Z}_n^1=(A_{n-1}+648n-90)Z_n^1.\end{array}$$

If $Z_n^1=0$, the equality mentioned above is evident. Thus, we only have to regard the case $Z_n^1=1$, which means that $G_n⟶G_{n+1}^1$. In this view, $p_n$ (of $G_n$) is coincident with the vertex labeled $x_2$ or $x_8$ (of $H_n$), see Figure 4. In this scenario, $A_n$ turns into

$$\begin{array}{cc}\hfill 18\sum _{m\in V_{G_n}}d\left(m\right)d(x_2,m)& =18\sum _{m\in V_{G_{n-1}}}d\left(m\right)d(x_2,m)+18\sum _{m\in V_{H_n}}d\left(m\right)d(x_2,m)\hfill \\ \hfill & =18\sum _{m\in V_{G_{n-1}}}d\left(m\right)(d(m,p_{n-1})+d(x_2,p_{n-1}))+18\times 33\hfill \\ \hfill & =18\sum _{m\in V_{G_{n-1}}}d\left(m\right)(d(m,p_{n-1})+2)+18\times 33\hfill \\ \hfill & =A_{n-1}+36\sum _{m\in V_{G_{n-1}}}d\left(m\right)+594\hfill \\ \hfill & =A_{n-1}+36(18n-19)+594\hfill \\ \hfill & =A_{n-1}+648n-90,\hfill \end{array}$$

Figure 4. $G_n\to G_{n+1}^1$.

In the foregoing, we utilised (10)–(12). As a result, we arrive at the required equivalence conclusion.

Equality 2.

$$\begin{array}{c}\hfill A_n{Z}_n^2=(A_{n-1}+972n-414)Z_n^2.\end{array}$$

As in the proof of Equality 1, we only have to regard the case $Z_n^2=1$, which is $G_n⟶G_{n+1}^2$. The proof process is analogous, and we leave out the details.

Equality 3.

$$\begin{array}{c}\hfill A_n{Z}_n^3=(A_{n-1}+1296n-738)Z_n^3\end{array}$$

We have to regard the case $Z_n^3=1$, which is $G_n⟶G_{n+1}^3$. The proof is the same as Equality 1 and we omit the details.

Equality 4.

$$\begin{array}{c}\hfill A_n{Z}_n^4=(A_{n-1}+1620n-1062)Z_n^4\end{array}$$

We have to regard the case $Z_n^4=1$, which is $G_n⟶G_{n+1}^4$. The proof process is also analogous, and we leave out the details.

Recalling that $Z_n^1+Z_n^2+Z_n^3+Z_n^4=1$, according to the above discussion, it holds that

$$\begin{array}{cc}\hfill A_n& =A_n(Z_n^1+Z_n^2+Z_n^3+Z_n^4)\hfill \\ \hfill & =(A_{n-1}+648n-90)Z_n^1+(A_{n-1}+972n-414)Z_n^2+(A_{n-1}+1296n-738)Z_n^3+(A_{n-1}+1620n-1062)Z_n^4\hfill \\ \hfill & =A_{n-1}+(648Z_n^1+972Z_n^2+1296Z_n^3+1620Z_n^4)n-(90Z_n^1+414Z_n^2+738Z_n^3+1062Z_n^4)\hfill \\ \hfill & =A_{n-1}+n·U_n-V_n.\hfill \end{array}$$

For each n, it indicates that $U_n=648Z_n^1+972Z_n^2+1296Z_n^3+1620Z_n^4$, $V_n=90Z_n^1+414Z_n^2+738Z_n^3+1062Z_n^4$.

Therefore, by (15) we obtain

$$\begin{array}{cc}\hfill Gut\left(G_n\right)& =Gut\left(G_1\right)+\sum _{t=1}^{n-1}{A}_t+\sum _{t=1}^{n-1}(882t+239)\hfill \\ \hfill & =Gut\left(G_1\right)+\sum _{t=1}^{n-1}(\sum _{q=1}^{t-1}(A_{q+1}-A_q)+A_1)+\sum _{t=1}^{n-1}(882t+239)\hfill \\ \hfill & =Gut\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}(A_{q+1}-A_q)+(n-1)A_1+\sum _{t=1}^{n-1}(882t+239)\hfill \\ \hfill & =Gut\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+(n-1)A_1+\sum _{t=1}^{n-1}(882t+239)\hfill \\ \hfill & =Gut\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+O\left(n^2\right)\hfill \end{array}$$

where $O\left(n^2\right)$ representing high-order infinitesimal of $n^2$.

By direct calculation, one sees that $Var\left(U_q\right)={\sigma }^2$, $Var\left(V_q\right)={\tilde{\sigma }}^2$, and $Cov(U_q,V_q)=r$, where for any two random variables X and Y, $Cov(X,Y)=E\left(XY\right)-E\left(X\right)·E\left(Y\right)$. Based on the nature of the variance and the order in which the sums are exchanged, it can be concluded that

$$\begin{array}{cc}\hfill Var\left(Gut\left(G_n\right)\right)& =Var(\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1}))\hfill \\ \hfill & =Var(\sum _{q=1}^{n-2}\sum _{t=q+1}^{n-1}((q+1)U_{q+1}-V_{q+1}))\hfill \\ \hfill & =Var(\sum _{q=1}^{n-2}((q+1)U_{q+1}-V_{q+1})(n-q-1))\hfill \\ \hfill & =\sum _{q=1}^{n-2}{(n-q-1)}^{2}Var((q+1)U_{q+1}-V_{q+1})\hfill \\ \hfill & =\sum _{q=1}^{n-2}{(n-q-1)}^{2}Cov((q+1)U_{q+1}-V_{q+1},(q+1)U_{q+1}-V_{q+1})\hfill \\ \hfill & =\sum _{q=1}^{n-2}{(n-q-1)}^2({(q+1)}^{2}Cov(U_{q+1},U_{q+1})-2(q+1)Cov(U_{q+1},V_{q+1})+Cov(V_{q+1},V_{q+1}))\hfill \\ \hfill & =\sum _{q=1}^{n-2}{(n-q-1)}^2({(q+1)}^2{\sigma }^2-2(q+1)r+{\tilde{\sigma }}^2).\hfill \end{array}$$

By means of computational, ad hoc tools, the above equality gives the required results, $Var\left(Gut\left(G_n\right)\right)$.

Now, we discuss the variance of the Schultz index.

By Theorem 2.3 of [41], we have

$$\begin{array}{cc}\hfill E\left(S\left(G_n\right)\right)=& (240-144p_1-96p_2-48p_3)n^3+(432p_1+288p_2+144p_3-40)n^2\hfill \\ \hfill & +(56-288p_1-192p_2-96p_3)n.\hfill \end{array}$$

After that we illustrate our results.

Theorem 2.

Supposing that Hypothesis 1, then the following results are true. As to the random cyclooctatetraene chain $G_n$, the variance of Schultz index $S\left(G_n\right)$, computed as

$$\begin{array}{cc}\hfill Var\left(S\left(G_n\right)\right)=& \frac{1}{30}({\sigma }^2{n}^5-5r{n}^4+10{\tilde{\sigma }}^2{n}^3+(65r-30{\sigma }^2-45{\tilde{\sigma }}^2)n^2\hfill \\ \hfill & +(-120r+59{\sigma }^2+65{\tilde{\sigma }}^2)n+(60r-30{\sigma }^2-30{\tilde{\sigma }}^2)).\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill {\sigma }^2=& {576}^2{p}_1+{864}^2{p}_2+{1152}^2{p}_3+{1440}^2{p}_4-{(576p_1+864p_2+1152p_3+1440p_4)}^2\hfill \\ \hfill {\tilde{\sigma }}^2=& {40}^2{p}_1+{328}^2{p}_2+{616}^2{p}_3+{904}^2{p}_4-{(40p_1+328p_2+616p_3+904p_4)}^2\hfill \\ \hfill r=& 576·40·p_1+864·328·p_2+1152·616·p_3+1440·904·p_4\hfill \\ \hfill & -(576p_1+864p_2+1152p_3+1440p_4)·(40p_1+328p_2+616p_3+904p_4)\hfill \end{array}$$

Proof. 

By [41] (5.2), we obtain

$$S\left(G_{n+1}\right)=S\left(G_n\right)+18\sum _{m\in V_{G_n}}d(p_n,m)+8\sum _{m\in V_{G_n}}d\left(m\right)d(p_n,m)+824n+248.$$

(16)

and

$$\begin{array}{c}\hfill B_n:=\sum _{m\in V_{G_n}}(18+8d\left(m\right))d(p_n,m).\end{array}$$

$$\begin{array}{c}\hfill S\left(G_{n+1}\right)=S\left(G_n\right)+B_n+824n+248.\end{array}$$

□

After similar discussions, we have four equalities as follows:

Equality 1.

$$\begin{array}{c}\hfill B_n{Z}_n^1=(B_{n-1}+576n-40)Z_n^1\end{array}$$

If $Z_n^1=0$, the equality mentioned above is evident, so we only have to regard the case $Z_n^1=1$, which means that $G_n⟶G_{n+1}^1$. In this view, $p_n$ (of $G_n$) is coincident with the vertex labeled $x_2$ or $x_8$ (of $H_n$), see Figure 4. In this scenario, $B_n$ turns into

$$\begin{array}{cc}\hfill B_n& =\sum _{m\in V_{G_n}}(18+8d\left(m\right))d(x_2,m)\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))d(x_2,m)+\sum _{m\in V_{H_n}}(18+8d\left(m\right))d(x_2,m)\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))(d(p_{n-1},m)+2)+18\times 16+8\times 33\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))d(p_{n-1},m)+2\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))+18\times 16+8\times 33\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))d(p_{n-1},m)+2(18\times 8(n-1)+8\times (18(n-1)-1))+18\times 16+8\times 33\hfill \\ \hfill & =B_{n-1}+2(288n-296)+552\hfill \\ \hfill & =B_{n-1}+576n-40\hfill \end{array}$$

In the foregoing, we utilised (10)–(12). As a result, we arrive at the required equivalence conclusion.

Equality 2.

$$\begin{array}{c}\hfill B_n{Z}_n^2=(B_{n-1}+864n-328)Z_n^2\end{array}$$

As in the proof of Equality 1, we only have to regard the case $Z_n^2=1$, which is $G_n⟶G_{n+1}^2$. The proof process is analogous, and we leave out the details.

Equality 3.

$$\begin{array}{c}\hfill B_n{Z}_n^3=(B_{n-1}+1152n-616)Z_n^3\end{array}$$

We have to regard the case $Z_n^3=1$, which is $G_n⟶G_{n+1}^3$. The proof is the same as Equality 1 and we omit the details.

Equality 4.

$$\begin{array}{c}\hfill B_n{Z}_n^4=(B_{n-1}+1440n-904)Z_n^4\end{array}$$

We have to regard the case $Z_n^4=1$, which is $G_n⟶G_{n+1}^4$. The proof process is also analogous, and we leave out the details.

Recalling that $Z_n^1+Z_n^2+Z_n^3+Z_n^4=1$, according to the above discussion, it holds that

$$\begin{array}{cc}\hfill B_n& =B_n(Z_n^1+Z_n^2+Z_n^3+Z_n^4)\hfill \\ \hfill & =B_{n-1}+n·U_n-V_n\hfill \end{array}$$

where for each n, $U_n=576Z_n^1+864Z_n^2+1152Z_n^3+1440Z_n^4$, $V_n=40Z_n^1+328Z_n^2+616Z_n^3+904Z_n^4$.

Therefore, by (15)

$$\begin{array}{cc}\hfill S\left(G_n\right)& =S\left(G_1\right)+\sum _{t=1}^{n-1}{B}_t+\sum _{t=1}^{n-1}(824t+248)\hfill \\ \hfill & =S\left(G_1\right)+\sum _{t=1}^{n-1}(\sum _{q=1}^{t-1}(B_{q+1}-B_q)+B_1)+\sum _{t=1}^{n-1}(824t+2489)\hfill \\ \hfill & =S\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}(B_{q+1}-B_q)+(n-1)B_1+\sum _{t=1}^{n-1}(824t+248)\hfill \\ \hfill & =S\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+(n-1)B_1+\sum _{t=1}^{n-1}(824t+248)\hfill \\ \hfill & =S\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+O\left(n^2\right).\hfill \end{array}$$

If we replace $Gut\left(G_n\right)$ from the proof of Theorem 1 by $S\left(G_n\right)$, the rest of the proof of this theorem is the same as the proof of Theorem 1, and therefore the details are omitted.

3. The Variances of Multiplicative and Additive Degree-Kirchhoff Indices of a Random Cyclooctatetraene Chain

In the section, we talk about the variances for the multiplicative degree-Kirchhoff index $K{f}^{*}\left(G_n\right)$ and the additive degree-Kirchhoff index $K{f}^{+}\left(G_n\right)$. For a random cyclooctatetraene chain $G_n$, $K{f}^{*}\left(G_n\right)$ and $K{f}^{+}\left(G_n\right)$ are random variables.

Recall that $G_{n+1}$ is $G_n$ connected by an edge to a new terminal octagon $H_{n+1}$, where $H_{n+1}$ is extended by vertices $x_1$, $x_2$, $x_3$, $x_4$, $x_5$, $x_6$, $x_7$, and $x_8$, and $p_n{x}_1$ is the new edge; see Figure 2. On the one hand, for all $m\in V_{G_n}$,

$$r(x_1,m)=r(p_n,m)+1,r(x_2,m)=r(p_n,m)+1+\frac{7}{8},r(x_3,m)=r(p_n,m)+1+\frac{12}{8},r(x_4,m)=r(p_n,m)+1+\frac{15}{8},$$

(17)

$$r(x_5,m)=r(p_n,m)+1+\frac{16}{8},r(x_6,m)=r(p_n,m)+1+\frac{15}{8},r(x_7,m)=r(p_n,m)+1+\frac{12}{8},r(x_8,m)=r(p_n,m)+1+\frac{7}{8}.$$

(18)

$$\sum _{m\in V_{G_n}}{d}_{G_{n+1}}\left(m\right)=18n-1.$$

(19)

On the other hand, for all $t\in \{1,2,3,4,5,6,7,8\}$

$$\sum _{t=1}^{8}d\left(x_t\right)r(x_1,x_t)=21,\sum _{t=1}^{8}d\left(x_t\right)r(x_2,x_t)=\frac{175}{8},\sum _{t=1}^{8}d\left(x_t\right)r(x_3,x_t)=\frac{45}{2},\sum _{t=1}^{8}d\left(x_t\right)r(x_4,x_t)=\frac{183}{8},$$

(20)

$$\sum _{t=1}^{8}d\left(x_t\right)r(x_5,x_t)=23,\sum _{t=1}^{8}d\left(x_t\right)r(x_6,x_t)=\frac{183}{8},\sum _{t=1}^{8}d\left(x_t\right)r(x_7,x_t)=\frac{45}{2},\sum _{t=1}^{8}d\left(x_t\right)r(x_8,x_t)=\frac{175}{8}.$$

(21)

By Theorem 3.1 of [41], we have

$$\begin{array}{cc}\hfill E\left(K{f}^{*}\left(G_n\right)\right)=& (162-\frac{243}{4}{p}_1-27p_2-\frac{27}{4}{p}_3)n^3+(36+\frac{729}{4}{p}_1+81p_2+\frac{81}{4}{p}_3)n^2\hfill \\ \hfill & -(29+\frac{243}{2}{p}_1+54p_2+\frac{27}{2}{p}_3)n-1.\hfill \end{array}$$

we now present the first main result of this section.

Theorem 3.

The results are as follows, if Hypothesis 1 holds. As to the random cyclooctatetraene chain $G_n$, the variance of the multiplicative degree-Kirchhoff index $K{f}^{*}\left(G_n\right)$, computed as

$$\begin{array}{cc}\hfill Var\left(K{f}^{*}\left(G_n\right)\right)=& \frac{1}{30}({\sigma }^2{n}^5-5r{n}^4+10{\tilde{\sigma }}^2{n}^3+(65r-30{\sigma }^2-45{\tilde{\sigma }}^2)n^2\hfill \\ \hfill & +(-120r+59{\sigma }^2+65{\tilde{\sigma }}^2)n+(60r-30{\sigma }^2-30{\tilde{\sigma }}^2)).\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill {\sigma }^2=& {\left(\frac{1215}{2}\right)}^2{p}_1+{810}^2{p}_2+{\left(\frac{1863}{2}\right)}^2{p}_3+{972}^2{p}_4-{(\frac{1215}{2}{p}_1+810p_2+\frac{1863}{2}{p}_3+972p_4)}^2\hfill \\ \hfill {\tilde{\sigma }}^2=& {\left(\frac{495}{2}\right)}^2{p}_1+{450}^2{p}_2+{\left(\frac{1143}{2}\right)}^2{p}_3+{612}^2{p}_4-{(\frac{495}{2}{p}_1+450p_2+\frac{1143}{2}{p}_3+612p_4)}^2\hfill \\ \hfill r=& \frac{1215}{2}·\frac{495}{2}·p_1+810·450·p_2+\frac{1863}{2}·\frac{1143}{2}·p_3+972·612·p_4\hfill \\ \hfill & -(\frac{1215}{2}{p}_1+810p_2+\frac{1863}{2}{p}_3+972p_4)·(\frac{495}{2}{p}_1+450p_2+\frac{1143}{2}{p}_3+612p_4)\hfill \end{array}$$

Proof. 

By [41] (5.3), we have

$$K{f}^{*}\left(G_{n+1}\right)=K{f}^{*}\left(G_n\right)+18\sum _{m\in V_{G_n}}d\left(m\right)r(p_n,m)+684n+151.$$

(22)

and

$$C_n:=18\sum _{m\in V_{G_n}}d\left(m\right)r(p_n,m).$$

(23)

$$K{f}^{*}\left(G_{n+1}\right)=K{f}^{*}\left(G_n\right)+C_n+684n+151.$$

(24)

□

Recalling from Section 1 that $Z_n^1$, $Z_n^2$, $Z_n^3$, and $Z_n^4$ are random variables, this indicates our option in constructing $G_{n+1}$ from $G_n$. We have four equalities as follows:

Equality 1.

$$\begin{array}{c}\hfill C_n{Z}_n^1=(C_{n-1}+\frac{1215}{2}n-\frac{495}{2})Z_n^1\end{array}$$

If $Z_n^1=0$, the above equality is obvious, so we only need to consider the case $Z_n^1=1$, which implies $G_n⟶G_{n+1}^1$. In this view, $p_n$ (of $G_n$) is coincident with the vertex labeled $x_2$ or $x_8$ (of $H_n$), see Figure 4. In this scenario, $C_n$ turns into

$$\begin{array}{cc}\hfill C_n& =18\sum _{m\in V_{G_n}}d\left(m\right)r(x_2,m)\hfill \\ \hfill & =18\sum _{m\in V_{G_{n-1}}}d\left(m\right)r(x_2,m)+18\sum _{m\in V_{H_n}}d\left(m\right)r(x_2,m)\hfill \\ \hfill & =18\sum _{m\in V_{G_{n-1}}}d\left(m\right)(1+\frac{7}{8}+r(p_{n-1},m))+\frac{175}{8}\times 18\hfill \\ \hfill & =18\sum _{m\in V_{G_{n-1}}}d\left(m\right)r(p_{n-1},m)+\frac{270}{8}\sum _{m\in V_{G_{n-1}}}d\left(m\right)+\frac{175}{8}\times 18\hfill \\ \hfill & =C_{n-1}+\frac{270}{8}(18n-19)+\frac{3150}{8}\hfill \\ \hfill & =C_{n-1}+\frac{1215}{2}n-\frac{495}{2}\hfill \end{array}$$

In the foregoing, we utilised (17)–(19). As a result, we arrive at the required equivalence conclusion.

Equality 2.

$$\begin{array}{c}\hfill C_n{Z}_n^2=(C_{n-1}+810n-450)Z_n^2\end{array}$$

As in the proof of Equality 1, we only have to regard the case $Z_n^2=1$, which is $G_n⟶G_{n+1}^2$. The proof process is analogous, and we leave out the details.

Equality 3.

$$\begin{array}{c}\hfill C_n{Z}_n^3=(C_{n-1}+\frac{1863}{2}n-\frac{1143}{2})Z_n^3\end{array}$$

We have to regard the case $Z_n^3=1$, which is $G_n⟶G_{n+1}^3$. The proof is the same as Equality 1 and we omit the details.

Equality 4.

$$\begin{array}{c}\hfill C_n{Z}_n^4=(C_{n-1}+972n-612)Z_n^4\end{array}$$

We have to regard the case $Z_n^4=1$, which is $G_n⟶G_{n+1}^4$. The proof process is also analogous, and we leave out the details.

Recalling that $Z_n^1+Z_n^2+Z_n^3+Z_n^4=1$, according to the above discussion, it holds that

$$\begin{array}{cc}\hfill C_n& =C_n(Z_n^1+Z_n^2+Z_n^3+Z_n^4)\hfill \\ \hfill & =C_{n-1}+n·U_n-V_n\hfill \end{array}$$

where for each n, $U_n=\frac{1215}{2}{Z}_n^1+810Z_n^2+\frac{1863}{2}{Z}_n^3+972Z_n^4$, $V_n=\frac{495}{2}{Z}_n^1+450Z_n^2+\frac{1143}{2}{Z}_n^3+612Z_n^4$.

Therefore, by (22)

$$\begin{array}{cc}\hfill K{f}^{*}\left(G_n\right)& =K{f}^{*}\left(G_1\right)+\sum _{t=1}^{n-1}{C}_t+\sum _{t=1}^{n-1}(684t+151)\hfill \\ \hfill & =K{f}^{*}\left(G_1\right)+\sum _{t=1}^{n-1}(\sum _{q=1}^{t-1}(C_{q+1}-C_q)+C_1)+\sum _{t=1}^{n-1}(684t+151)\hfill \\ \hfill & =K{f}^{*}\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}(C_{q+1}-C_q)+(n-1)C_1+\sum _{t=1}^{n-1}(684t+151)\hfill \\ \hfill & =K{f}^{*}\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+(n-1)C_1+\sum _{t=1}^{n-1}(684t+151)\hfill \\ \hfill & =K{f}^{*}\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+O\left(n^2\right)\hfill \end{array}$$

Now, we consider $K{f}^{+}\left(G_n\right)$. By Theorem 3.3 of [41] we have

$$\begin{array}{cc}\hfill E\left(K{f}^{+}\left(G_n\right)\right)=& (144-54p_1-24p_2-6p_3)n^3+(61+162p_1+72p_2+18p_3)n^2\hfill \\ \hfill & -(37+108p_1+48p_2+12p_3)n.\hfill \end{array}$$

$Var\left(K{f}^{+}\left(G_n\right)\right)$ is given by

Theorem 4.

$$\begin{array}{cc}\hfill Var\left(K{f}^{+}\left(G_n\right)\right)=& \frac{1}{30}({\sigma }^2{n}^5-5r{n}^4+10{\tilde{\sigma }}^2{n}^3+(65r-30{\sigma }^2-45{\tilde{\sigma }}^2)n^2\hfill \\ \hfill & +(-120r+59{\sigma }^2+65{\tilde{\sigma }}^2)n+(60r-30{\sigma }^2-30{\tilde{\sigma }}^2)).\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill {\sigma }^2=& {540}^2{p}_1+{720}^2{p}_2+{828}^2{p}_3+{864}^2{p}_4-{(540p_1+720p_2+828p_3+864p_4)}^2\hfill \\ \hfill {\tilde{\sigma }}^2=& {191}^2{p}_1+{371}^2{p}_2+{479}^2{p}_3+{515}^2{p}_4-{(191p_1+371p_2+479p_3+515p_4)}^2\hfill \\ \hfill r=& 540·191·p_1+720·371·p_2+828·479·p_3+864·515·p_4\hfill \\ \hfill & -(540p_1+720p_2+828p_3+864p_4)·(191p_1+371p_2+479p_3+515p_4)\hfill \end{array}$$

Proof. 

By [41] (5.4), we see that

$$K{f}^{+}\left(G_{n+1}\right)=K{f}^{+}\left(G_n\right)+18\sum _{m\in V_{G_n}}r(p_n,m)+8\sum _{m\in V_{G_n}}d\left(m\right)r(p_n,m)+637n+160.$$

(25)

where

$$D_n:=\sum _{m\in V_{G_n}}(18+8d\left(m\right))r(p_n,m).$$

(26)

$$K{f}^{+}\left(G_{n+1}\right)=K{f}^{+}\left(G_n\right)+D_n+637n+160.$$

(27)

□

Recalling from Section 1 that $Z_n^1$, $Z_n^2$, $Z_n^3$, and $Z_n^4$ are random variables, this indicates our option in constructing $G_{n+1}$ from $G_n$. We have four equalities as follows:

Equality 1.

$$\begin{array}{c}\hfill D_n{Z}_n^1=(D_{n-1}+540n-191)Z_n^1\end{array}$$

If $Z_n^1=0$, the equality mentioned above is evident, so we only have to regard the case $Z_n^1=1$, which means that $G_n⟶G_{n+1}^1$. In this view, $p_n$ (of $G_n$) is coincident with the vertex labeled $x_2$ or $x_8$ (of $H_n$), see Figure 4. In this scenario, $D_n$ turns into

$$\begin{array}{cc}\hfill \sum _{m\in V_{G_n}}(18+8d\left(m\right))r(x_2,m)& =18\sum _{m\in V_{G_n}}d\left(m\right)r(x_2,m)\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))r(x_2,m)+\sum _{m\in V_{H_n}}(18+8d\left(m\right))r(x_2,m)\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))(r(p_{n-1},m)+1+\frac{7}{8})+18\sum _{m\in V_{H_n}}r(x_2,x_i)+8\sum _{m\in V_{H_n}}d\left(x_i\right)r(x_2,x_i)\hfill \\ \hfill & =\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))r(p_{n-1},m)+\frac{15}{8}\sum _{m\in V_{G_{n-1}}}(18+8d\left(m\right))+364\hfill \\ \hfill & =D_{n-1}+\frac{15}{8}(288n-296)+364\hfill \\ \hfill & =D_{n-1}+540n-191\hfill \end{array}$$

In the foregoing, we utilised (17)–(19). As a result, we arrive at the required equivalence conclusion.

Equality 2.

$$\begin{array}{c}\hfill D_n{Z}_n^2=(D_{n-1}+720n-371)Z_n^2\end{array}$$

As in the proof of Equality 1, we only have to regard the case $Z_n^2=1$, which is $G_n⟶G_{n+1}^2$. The proof process is analogous, and we leave out the details.

Equality 3.

$$\begin{array}{c}\hfill D_n{Z}_n^3=(D_{n-1}+828n-479)Z_n^3\end{array}$$

We have to regard the case $Z_n^3=1$, which is $G_n⟶G_{n+1}^3$. The proof is the same as Equality 1 and we omit the details.

Equality 4.

$$\begin{array}{c}\hfill D_n{Z}_n^4=(D_{n-1}+864n-515)Z_n^4\end{array}$$

We have to regard the case $Z_n^4=1$, which is $G_n⟶G_{n+1}^4$. The proof process is also analogous, and we leave out the details.

Recalling that $Z_n^1+Z_n^2+Z_n^3+Z_n^4=1$, according to the above discussion, it holds that

$$\begin{array}{cc}\hfill D_n& =D_n(Z_n^1+Z_n^2+Z_n^3+Z_n^4)\hfill \\ \hfill & =D_{n-1}+n·U_n-V_n\hfill \end{array}$$

where for each n, $U_n=540Z_n^1+720Z_n^2+828Z_n^3+864Z_n^4$, $V_n=191Z_n^1+371Z_n^2+479Z_n^3+515Z_n^4$.

Therefore, by (25)

$$\begin{array}{cc}\hfill K{f}^{+}\left(G_n\right)& =K{f}^{+}\left(G_1\right)+\sum _{t=1}^{n-1}{D}_t+\sum _{t=1}^{n-1}(637t+160)\hfill \\ \hfill & =K{f}^{+}\left(G_1\right)+\sum _{t=1}^{n-1}(\sum _{q=1}^{t-1}(D_{q+1}-D_q)+D_1)+\sum _{t=1}^{n-1}(637t+160)\hfill \\ \hfill & =K{f}^{+}\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}(D_{q+1}-D_q)+(n-1)D_1+\sum _{t=1}^{n-1}(637t+160)\hfill \\ \hfill & =K{f}^{+}\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+(n-1)D_1+\sum _{t=1}^{n-1}(637t+160)\hfill \\ \hfill & =K{f}^{+}\left(G_1\right)+\sum _{t=1}^{n-1}\sum _{q=1}^{t-1}((q+1)U_{q+1}-V_{q+1})+O\left(n^2\right)\hfill \end{array}$$

If we replace $Gut\left(G_n\right)$ with $K{f}^{+}\left(G_n\right)$ in the proof of Theorem 1, the rest of the proof of this theorem is the same as that in the proof of Theorem 1; we thus omit the details.

4. Concluding Remarks

In this paper, we obtain explicit analytical expressions for the variances of Schultz index, multiplicative degree-Kirchhoff index, Gutman index and additive degree-Kirchhoff index of a random cyclooctatetraene chain with n octagons. All of these results will contribute to the study of Schultz index, multiplicative degree-Kirchhoff index, Gutman index and additive degree-Kirchhoff index of graphs. With the continuous development and progress of science, more and more molecules are being discovered and created.

The polygonal chain problem in chemical graph theory has been extensively studied and discussed by researchers. For the variance of some certain indices of a random polygon chain that has n regular polygons, it is feasible to establish exact formulas.

Not only that, through these studies, we can hopefully obtain the variance of the n sided chain graph and some of their physicochemical properties in the near future.