1. Introduction
The assumption that individuals behave as if maximizing their material payoffs, despite its central role in economic analysis, is at odds with a large body of evidence from psychology and from experimental economics. Economic agents often pursue objectives other than actual payoff maximization. Many observed departures from material payoff maximizing behavior arise through actions that favor fairness or reciprocity.
Fairness and reciprocity have been shown to explain behavior in bargaining games and in trust games. For example, in ultimatum games offers are usually much more generous than predicted by subgame perfect equilibrium and low offers are often rejected. These offers are consistent with an equilibrium in which proposers make offers knowing that responders may reject allocations that appear unfair.
The same effect is observed in markets. [
1] show that a Stackelberg leader finds it hard to exploit his advantage in experimental markets. The reason is that the Stackelberg follower acts more aggressively than predicted by the subgame perfect equilibrium. In fact, followers punish the leader by supplying a higher quantity than their most profitable response to the leader’s quantity. This behavior is in line with the observed negative reciprocity of responders in the ultimatum game when the proposer tries to exploit his first-mover advantage.
The impact of fairness and reciprocity on market outcomes is an active area of research. [
2] and [
3] show that fairness concerns on the part of consumers can improve consumer welfare. For example, [
2] finds that a monopolist ought to set a price lower than “the monopoly price” if consumers have concerns about fairness.
In this paper we ask whether reciprocity may help to sustain collusive behavior. For instance, if a collusive agreement is seen by the parties as a kind outcome, then if one party reneges on the agreement and undercuts the price (or boosts its output), its rivals may be offended and hence punish the deviator aggressively (even at extra cost to themselves). Thus, because negative reciprocity induces more aggressive punishments for deviating, it could help sustain collusion relative to situations in which firms care only about their own profits.
[
4] present evidence–real world and experimental–that firms (or experimental subjects playing the role of firms) sometimes depart from the profit-maximizing paradigm. For example, CEOs may be overly optimistic about the profitability of mergers or other actions they undertake; managers might face incentives which induce them to care about relative rather than absolute profits; and firms might punish rivals who behave “unfairly” towards them.
[
4] argue that sometimes managers are motivated in part by personal animosity–or respect–towards a rival. Thus, firms might punish rivals who behave “unfairly” towards them. For example, firms might sometimes care when their rivals obtain an “unfair” share of industry profits, by, for instance, cheating on a collusive agreement. Also, many experiments find that collusion can still be observed even when there is a known fixed number of periods; a finding that goes against the prediction of rational play.
To model reciprocity we follow [
5] and assume that players in a strategic environment have preferences not only over the outcomes but also the strategies. A player’s utility is additively separable in monetary and fairness payoffs. Monetary payoffs are revenues minus costs and fairness payoffs are a weighted average of the rivals’ monetary payoffs where the weights depend on the expected play of the rivals. If a player expects a rival to play a kind (mean) strategy, then he places a positive (negative) weight on that rival’s monetary payoff.
In a standard setting, collusion can be sustained as an equilibrium by self-interested players if they interact infinitely often and are sufficiently patient. A player is said to be patient if his discount factor is sufficiently close to one. In order to determine whether collusion is or is not facilitated by reciprocity we compare the minimal discount factor that allows the same collusive outcome to be sustained when players are reciprocal and when they are self-interested.
We find that reciprocity facilitates collusion under dynamic price competition if players consider collusive prices to be kind and punishment prices to be unkind. This happens because the future losses of deviating from collusion are higher for reciprocal players than for self-interested ones and the short-run deviation gain of a reciprocal player is less than the short-run deviation gain of a self-interested one. The intuition behind this result is as follows.
If players expect the collusive prices to be played and consider those prices to be kind, then collusion is a positive reciprocity state, i.e., a state where players place a positive weight on their rivals’ monetary payoffs. Additionally, if players expect punishments to happen and consider that punishment prices are unkind, then the punishment phase is a negative reciprocity state, i.e., a state where players place a negative weight on their rivals’ monetary payoffs. These two effects imply that the future losses of deviating from collusion are higher for reciprocal players than for self-interested ones.
If a player considers the collusive prices of the rivals as kind, then when he deviates from collusion he attains a lower monetary payoff than a self-interested player because he cares about the monetary payoffs of his rivals and so he is less willing to undercut the collusive price. Additionally, when a reciprocal player deviates from collusion he suffers a loss in fairness payoffs because he lowers the monetary payoffs of his rivals. These two effects imply that the short-run deviation gain of a reciprocal player is less than the short-run deviation gain of a self-interested one.
Our paper is an additional contribution to the literature on the factors that help or hinder collusion. It is now well known that concentration, barriers to entry, cross-ownership, symmetry and multi-market contracts facilitate collusion–see [
6]. We provide sufficient conditions under which fairness and reciprocity facilitate collusion. We show that if a collusive agreement is seen as a positive reciprocity state, then the parties internalize part of the harm they impose on rivals when deviating from it. This effect is similar to the impact of partial cross ownership on incentives to collude analyzed in [
7]. We also show that if mutual punishments are seen as a negative reciprocity state, then the parties to a collusive scheme are willing to impose harsher punishments on rivals following a deviation.
The main policy implication of our paper is that if firms have reciprocal preferences this can have adverse welfare consequences for consumers. In contrast, [
2] and [
3] find that fairness concerns on the part of consumers can increase consumer welfare. Thus, social preferences in imperfectly competitive markets might lead to different outcomes depending on who has such preferences (producers or consumers) and what the comparison group is.
The rest of the paper proceeds as follows.
Section 2 sets-up the model.
Section 3 and
Section 4 analyze the impact of fairness and reciprocity on static and dynamic price competition, respectively.
Section 5 consists of an example that illustrates our findings.
Section 6 concludes the paper. The
appendix contains the proofs of the results.
2. Set-up
The existing theories of social preferences can be classified into three broad categories. The first one is the distributional preference approach where social preferences only depend on the distribution of material payoffs. This includes [
8] and [
9]. These models are highly tractable and capture a wide range of phenomena but fail to explain the fact that preferences depend on more than outcomes, namely, intentions also matter.
The second category consists of intention-based models and includes [
2], [
10], and [
11], among others. These models assume that reciprocity depends on overall strategies and beliefs (and beliefs about beliefs) building on [
12] theory of psychological games. In [
2] utility is additively separable in monetary and fairness payoffs and the weight a player places on rivals’ monetary payoffs depends on his perception of the rivals’ intentions, which are evaluated using (i) beliefs about the rivals’ strategy choices, and (ii) beliefs about the rivals’ beliefs about his strategy. [
10] develop a theory of reciprocity for extensive form games where players update beliefs about intentions as the game unfolds and make a choice accordingly. [
11] model reciprocity in incomplete information games. Intention-based models have two major weaknesses: they use specific functional forms and use complex belief structures (see [
13]).
The third category explores the axiomatic foundations that generate utility functions that display social preferences. [
14] proposes a preference axiom which leads to a foundation of [
8] inequity aversion model. [
5] provide an axiomatic foundation for interdependent preferences that can reflect reciprocity, inequity aversion, altruism and spitefulness. The key innovation of their approach is that in addition to conventional preferences over outcomes, players in a strategic environment also have preferences over strategy profiles. This allows one to study situations where a player’s preference is affected by the behavior of other players.
We apply [
5] approach to a dynamic price competition game where
N players play the same stage game over an infinite horizon. More precisely, given common knowledge of preferences and a price profile
p describing how the game is expected to be played, in each stage player
i chooses price
, given its rivals are expected to play
, and his payoff in that stage is
where
is the vector of weights
i places on the monetary payoffs of his rivals when the rivals are expected to play
.
The first term on the right-hand side of (1) is the monetary payoff of player
i, given by
where
is the constant marginal cost of production of player
i and
is the demand faced by player
i. We assume that
is twice differentiable, is strictly decreasing in
. and strictly increasing in every
with
. The second term on the right-hand side of (1) is the fairness payoff, where
specifies the weight player
i attaches to rival
j’s monetary payoff when player
i expects rival
j to set price
.
1 We assume that if player
i expects rival
j’s price to be kind, then the weight is positive,
, capturing positive reciprocity. If player
i expects rival
j’s price to be unkind, then the weight is negative,
, capturing negative reciprocity. If player
i expects rival
j’s price to be neither kind nor unkind, then the weight is zero,
. Finally, we assume that the weight player
i attaches to rival
j’s monetary payoff is an increasing and differentiable function of
,
i.e.,
3. Static Price Competition
In this section we analyze how fairness and reciprocity change the outcome of static price competition. Let
and
denote the static game with self-interested and reciprocal players, respectively. The first-order conditions of player
i respectively in
and in
are:
and
The conditions (2) and (3) characterize the static equilibrium prices in
and in
, respectively. In the
appendix we report the second-order conditions which guarantee the existence of a Nash equilibrium in
and
, respectively. We also report the contraction conditions which guarantee that
and
, respectively, have a unique Nash equilibrium.
We denote the Nash equilibrium price of a self-interested player i by and the Nash equilibrium price profile of by . Similarly, we denote the Nash equilibrium price of a reciprocal player i by and the Nash equilibrium price profile of by . We denote by the weight player i places on j’s material payoff when i expects j to choose . We denote by the vector of weights player i places on his rivals’ material payoffs when player i expects the rivals to play according to , i.e., when the rivals choose Nash reciprocal prices.
Our first results compares the Nash equilibrium prices in and in
Proposition 1:
- (i)
If for all i and , then for all i;
- (ii)
f for all i and , then for all i.
This result characterizes the impact of fairness and reciprocity on equilibrium prices under static price competition. The intuition behind Proposition 1 is as follows: when players expect their rivals to set kind prices they place a positive weight on the monetary payoffs of the rivals, i.e., for all i and . In this case the Nash equilibrium is a positive reciprocity state: players expect their rivals to set kind prices and therefore wish to reward them. The reward consists in setting higher price than the price a self-interested player would set. In contrast, when players expect their rivals to set unkind prices they place a negative weight on the monetary payoffs of the rivals, i.e., for all i and . In this case the Nash equilibrium is a negative reciprocity state: players expect rivals to set unkind prices and therefore wish to punish them. The punishment consists in setting a lower price than the price a self-interested player would set.
To analyze the impact of fairness and reciprocity on the Nash equilibrium payoffs we focus on the symmetric case of and . This requires three symmetry assumptions. First, the demand system is symmetric, i.e., interchanging the prices of rival goods does not affect the demand for any good, as a function of its own price, and any two goods which sell at the same price have the same demand. Second, marginal costs across firms are symmetric, i.e., . Third, the weights reciprocal players place on the monetary payoffs of their rivals are symmetric, i.e., for all i and all . Since and are symmetric each game will have a unique symmetric Nash equilibrium price profile.
Proposition 2: Let and be symmetric N player games.
- (i)
If , then for all i;
- (ii)
If , then for all i.
Proposition 2 part (i) says that if reciprocal players expect their rivals to set kind prices, then they attain a higher equilibrium payoff than that obtained by self-interested players. This happens because reciprocal players will choose higher equilibrium prices than self-interested players. This increases reciprocal players’ monetary payoffs and, in addition, leads to fairness payoff gains due to the rivals’ kind behavior. In contrast, Proposition 2 part (ii) says that if reciprocal players expect their rivals to set unkind prices, then they attain a lower equilibrium payoff than that obtained by self-interested players. This happens because reciprocal players will choose lower equilibrium prices than self-interested players. This lowers reciprocal players’ monetary payoffs and, in addition, leads to fairness payoff losses due to the rivals’ unkind behavior.
4. Dynamic Price Competition
In this section we study the impact of fairness and reciprocity on collusion using a dynamic price competition set-up. More precisely, the symmetric static price games described in the previous section will be played over an infinite horizon. Let denote the infinitely repeated game with reciprocal players and the infinitely repeated game of self-interested players. Players discount the future at rate .
Players are able to sustain a collusive outcome when the payoff from collusion is no less than the payoff from deviation. To understand how fairness and reciprocity influence collusion we compare the incentives to cooperate of self-interested players in to those of reciprocal players in . To do that we assume that the two games are identical in all respects (monetary payoffs and the number of players) except that in players have reciprocal preferences whereas in they are self-interested.
The standard approach to study collusion in infinitely repeated games assumes that players use grim trigger strategies to punish any deviation from collusion,
i.e., following a deviation players switch to a Nash equilibrium of the stage game forever after. Thus, when self-interested players use grim trigger punishments in
, each player
i will prefer to play his collusive strategy if the payoff from collusion is no less than the payoff from defection, which consists of the one period gain from deviating plus the discounted payoff of inducing Nash reversion forever,
i.e.,
or
where
is the vector of collusive prices that result from joint profit maximization and
stands for the best response of a self-interested player
i to
. Solving (4) for
δ we obtain the critical discount factor above which
can be sustained by self-interested players:
The same reasoning applies when players have reciprocal preferences. A reciprocal player
i plays the collusive price
in
using a grim trigger strategy as long as
or
where
stands for the best response of reciprocal player
i to
and
where
is the weight player
i places on
j’s monetary payoff when
i expects
j to choose
. Solving (5) for
δ we obtain the critical discount factor above which
can be sustained by reciprocal players:
Reciprocal players expect the rivals to play collusive prices if no deviation occurs and expect them to play Nash reciprocal prices after a deviation occurs.
We say that fairness and reciprocity facilitate collusion when the collusive price profile can be sustained at a lower critical discount factor when players are reciprocal than when they are self-interested. If the opposite happens we say that fairness and reciprocity make collusion harder.
We are now ready to state the main result of the paper.
Proposition 3: Let and be symmetric N player games. If and , then the critical (minimum) discount factor needed to sustain collusion at in is less than that in , i.e., .
This result provides sufficient conditions for collusion to be easier to sustain in the infinitely repeated price game with reciprocal players than in the infinitely repeated price game with self-interested players.
If collusion is a positive reciprocity state and the punishment phase is a negative reciprocity state, i.e., , then the future losses of deviating from collusion for self-interested players–the left-hand side of ( 4)–are lower than those for reciprocal players–the left-hand side of (5). This happens for two reasons. First, if collusion is a positive reciprocity state, then reciprocal players’ monetary payoffs from collusion are the same as those of self-interested players but, in addition, there are fairness payoff gains since players consider rivals to be kind. Second, if reciprocal players expect the rivals to set unkind Nash prices in the punishment phase, then they expect to be in a negative reciprocity state following a deviation from collusion. In this case, reciprocal players wish to punish their rivals for their expected unkindness. They do it by setting a price lower than the price a self-interested player would set. This reduces players’ monetary payoffs and in addition leads to fairness payoff losses due to the unkind behavior of the rivals. Hence, the punishment imposed after cheating occurs is more severe.
If collusion is a positive reciprocity state, i.e., , then the short-run deviation gain of a self-interested player–the right-hand side of (4)–is greater than the short-run deviation gain of a reciprocal player–the right-hand side of (5). This happens due to two effects. First, a reciprocal player who deviates from collusion attains a lower monetary gain than a self-interested one since he places a positive weight on his rivals’ monetary payoffs and so he is less willing to undercut the collusive price. Second, when a player deviates from collusion he lowers the monetary payoffs of his rivals. A self-interested player does not care about this effect but a reciprocal player suffers fairness payoff losses since his rivals are being kind by sticking to their collusive prices.
Proposition 3 follows from two critical assumptions. First, the assumption that players place a positive weight on the monetary payoffs of the rivals when they expect them to play the collusive prices, i.e., . Second, the assumption that players place a non-positive weight on the monetary payoffs of the rivals when they expect them to play the Nash prices, i.e., If one of these assumptions is violated, then the impact of fairness and reciprocity on the critical discount factor needed to sustain collusion at is ambiguous. The symmetry assumptions are not critical to this result but greatly simplify the analysis.
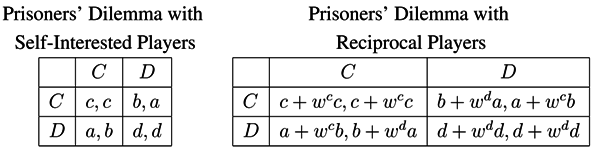
To better illustrate the intuition behind Proposition 3 consider the two prisoners’ dilemma (PD) games.
Each player has the choice between cooperate, C, or defect, D. The game with self-interested players represents a prisoners’ dilemma if cooperation is a strictly dominated strategy and mutual cooperation is better than mutual defection. Hence, we assume from now on that .
A reciprocal player who expects the rival to cooperate places weight
on the rival’s monetary payoff. Therefore, according to (1), a reciprocal player who expects the rival to cooperate obtains a payoff of
from
C and a payoff of
from
D. We assume from now on that a reciprocal player considers
C a kind action and so places a strictly positive weight on the rival’s monetary payoff when he expects the rival to play
C,
i.e.,
.
2A reciprocal player who expects the rival to defect places weight
on the rival’s monetary payoff. Therefore, according to (1), a reciprocal player who expects the rival to defect obtains a payoff of
from
D and a payoff of
from
C. We leave open the possibility that a reciprocal player can think of
D as either a kind or an unkind action by the rival,
i.e., we do not restrict the sign of
.
3Cooperation is a strictly dominated strategy in the PD game with reciprocal players if and Mutual cooperation is better than mutual defection in the PD game with reciprocal players if . We assume these three conditions hold from now on.
Now suppose the two PD games are played an infinite number of times and that players discount the future at rate
. Cooperation can be sustained in the infinitely repeated PD with self-interested players as long as
Solving (6) for
δ we obtain the critical discount factor above which cooperation can be sustained with self-interested players:
Similarly, cooperation can be sustained in the infinitely repeated PD with reciprocal players as long as
Solving (7) for
δ we obtain the critical discount factor above which cooperation can be sustained with reciprocal players:
The critical discount factor above which cooperation can be sustained with reciprocal players is lower than the one with self-interested players if and only if
Inequality (8) is a necessary and sufficient condition for cooperation to be easier to sustain in the infinitely PD game with reciprocal players than in the infinitely repeated PD game with self-interested players. The inequality tells us that reciprocity facilitates cooperation in the infinitely repeated PD game if and only if the weight a reciprocal player places on his rival’s monetary payoff when he expects the rival to play
D is not too high compared to the weight he places on the rival’s monetary payoff when he expects the rival to play
C.
A sufficient condition for (8) to hold is
,
i.e., a reciprocal player considers
C and
D as kind actions and places a weakly higher weight on the rivals’ monetary payoff when he expects the rival to play
C than when he expects the rival to play
D. Another sufficient condition for (8) to hold is
i.e., a reciprocal player considers
C a kind action and
D an unkind action.
4 This example shows that the result of Proposition 3 might also extend to the case where players consider the Nash prices as kind in the punishment phase and
.
5. Example
In this section we present an example that illustrates our results. Two identical firms operate in the market, selling possibly differentiated final products. Each firm faces the following inverse demand function (see [
15]):
in which
measures the degree of substitutability. By inverting (9), the direct demand function obtains:
Marginal production cost is constant and normalized to zero. Hence, the static payoff of a self-interested player is given by
We see from (10) that prices are strategic complements in the game with self-interested players since
.
The static payoff of a reciprocal player is given by
where
represents the weight the player places on the monetary payoff of rival
j. We assume that
is increasing and differentiable in
. We see from (11) that prices are strategic complements in the game with reciprocal players as long as
Since
a sufficient condition for (12) to be satisfied is
which we assume from now on. In addition we assume that
,
i.e., a reciprocal player places less weight on the monetary payoff of the rival than on his own.
The best-response function of a reciprocal player is
The Nash price of a reciprocal player is
Assuming symmetry,
i.e.,
the Nash price of a reciprocal player is
We see from (14) that the Nash price of a reciprocal player is less than the symmetric collusive price of
given that
. The static payoff of a reciprocal player under Nash prices is
We see from (14) and (15) that if
, then
and
If players are reciprocal and consider the Nash price to be kind,
i.e.,
, then
and
. Finally, if players are reciprocal and consider the Nash price to be unkind,
i.e.,
, then
and
. These results illustrate Propositions 1 and 2.
Let us now consider how reciprocity affects collusion. The collusive prices are the solution to
Solving this problem we obtain
,
. The collusive payoff of a reciprocal player is
where
A reciprocal player’s optimal price when he deviates from the collusive price and the rival plays the collusive price is
The unilateral deviation payoff of a reciprocal player is
and the unilateral deviation payoff of a self-interested player is
. Cooperation can be sustained in the infinitely repeated game with reciprocal players as long as
Cooperation can be sustained in the infinitely repeated game with self-interested players as long as
We know that
since
implies
and
implies
. From (16) and (17) we have that
Since
and
, condition (18) can be satisfied with a lower
δ than condition (19). Hence, we have shown that if
and
, then
is less than
i.e., it is easier to sustain collusion with reciprocal players than with self-interested ones. This result illustrates Proposition 3.
5 6. Conclusion
This paper contributes to the literature on the effect of fairness and reciprocity on market outcomes. Most of this literature has focused on the impact of fairness concerns by consumers on welfare. Here we focus on the role fairness and reciprocity play for firms’ incentives to collude.
We depart from the standard model of firm behavior by assuming that firms are motivated in part by personal animosity–or respect–towards a rival. Hence, firms might punish rivals who behave “unfairly” towards them and reward rivals who behave “fairly.”
We find that collusion is easier to sustain when firms have a concern for reciprocity towards competing firms provided that they consider collusive prices to be kind and punishment prices to be unkind.
Our results hold provided certain conditions are met. For example, we rule out fairness concerns on the part of consumers. This assumption was made on methodological grounds, to better isolate the effect of fairness and reciprocity among firms on collusive outcomes.
We also rule out that firms have fairness considerations with respect to consumers. Contrary to this assumption, [
16] reports that when subjects know that they are playing against human buyers (instead of simulated demand), collusion rates decrease substantially. This might undermine the effects predicted by the model.
We study the impact of fairness and reciprocity on collusion when players use Nash reversion to punish deviations. [
17] theory of optimal punishments can be an alternative framework of analysis. However, neither the players’ incentives, nor the impact of fairness and reciprocity on these incentives would change by employing optimal punishments. Therefore, the qualitative nature of our results would remain unchanged.