Rotor Eddy Current Loss Calculation of a 2DoF Direct-Drive Induction Motor
Abstract
:1. Introduction
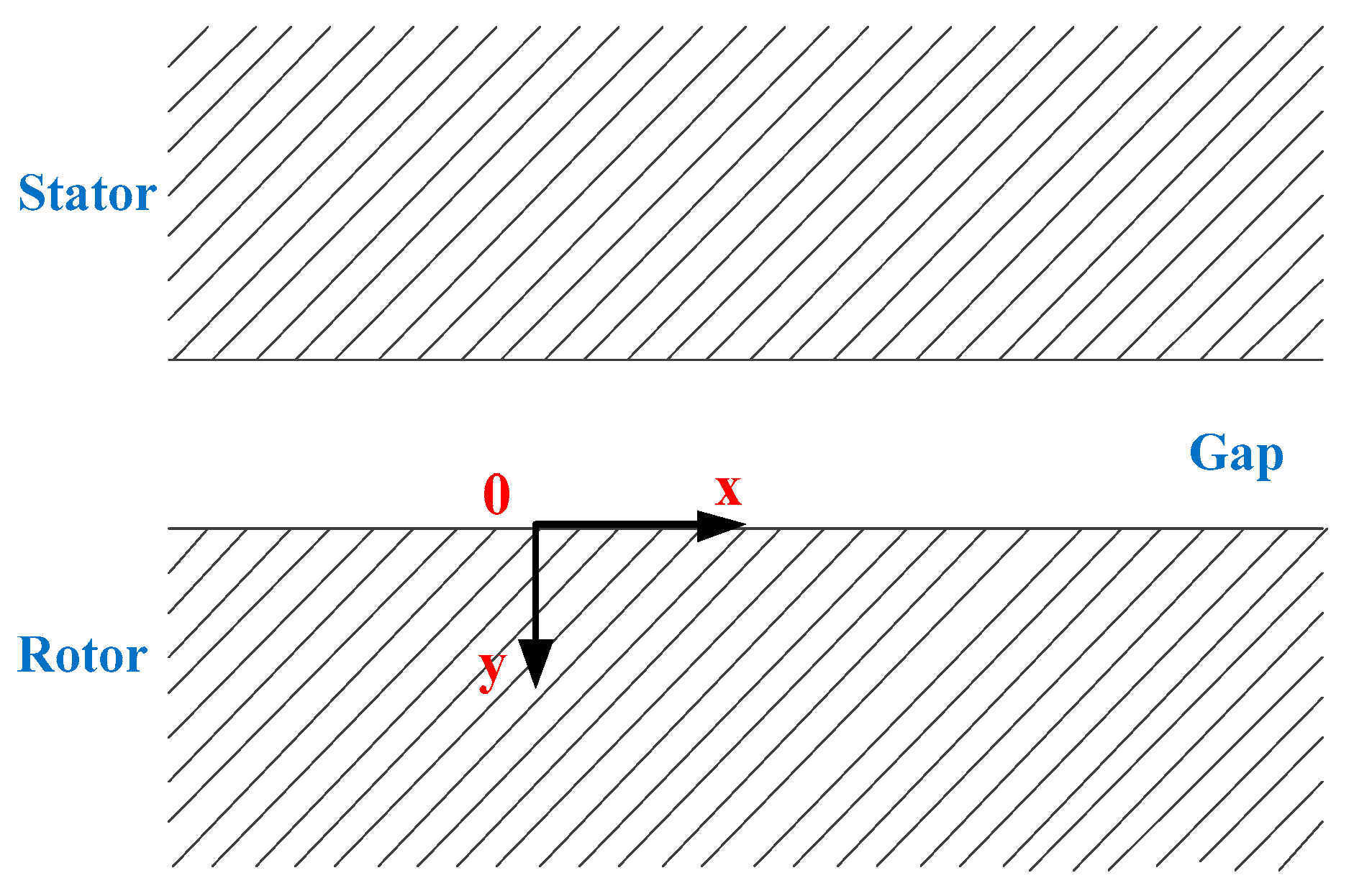
2. Structure and Parameters
3. Rotor Calculation
3.1. Linear Analytical Method of ECLCL and ECLRC
3.1.1. Magnetic Field Analysis
- (1)
- The curvature effect is disregarded. The rotor and stator are expanded into a semi-infinite flat model [13].
- (2)
- The phenomenon wherein the no-load speed exceeds the synchronous speed caused by the longitudinal edge effect is disregarded [14]. The synchronous speed is
- (3)
- The primary core has infinite permeability, and the conductivity is zero.
- (4)
- The end, hysteresis, and saturation effects are ignored. The rotor material is isotropic, and permeability and conductivity are constant.
- (5)
- The displacement current and influence of asymmetric three-phase current are ignored. The inducted current in the rotor only includes the z-component.
- (6)
- Each field includes a fundamental component, and the time variations of each field are sinusoidal.
3.1.2. Mathematical Model of ECLCL
3.1.3. Mathematical Model of ECLRC
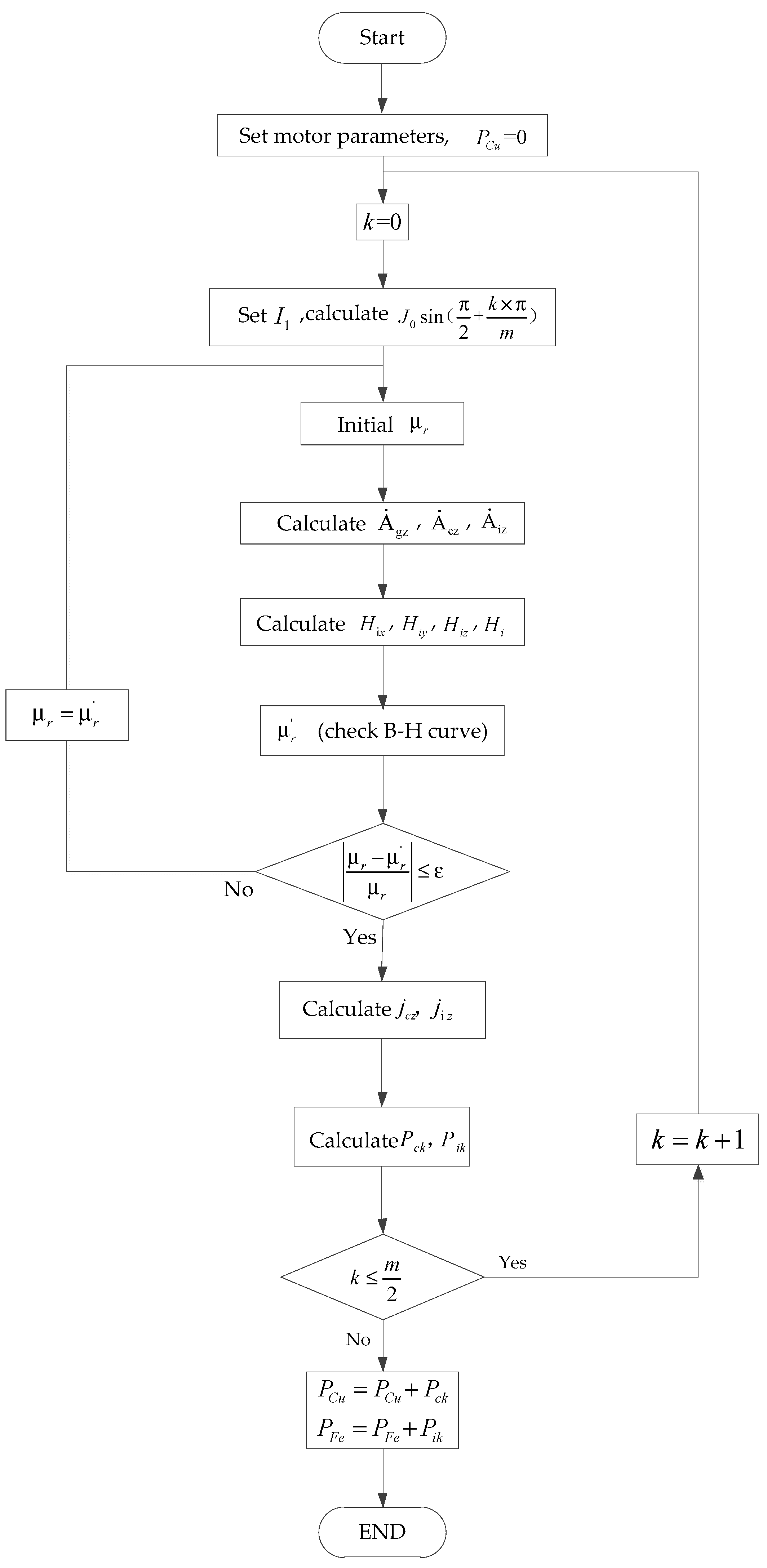
3.2. Nonlinear Analytical Method of ECLCL and ECLRC
- (1)
- Select the calculating point and divide the time period into 2m segments. Owing to the symmetry and parity of , calculating ECLCL and ECLRC in a quarter of the period is sufficient.
- (2)
- Use as an amplitude to establish the expression of , , (when m is even), (when m is odd); k is an integer.
- (3)
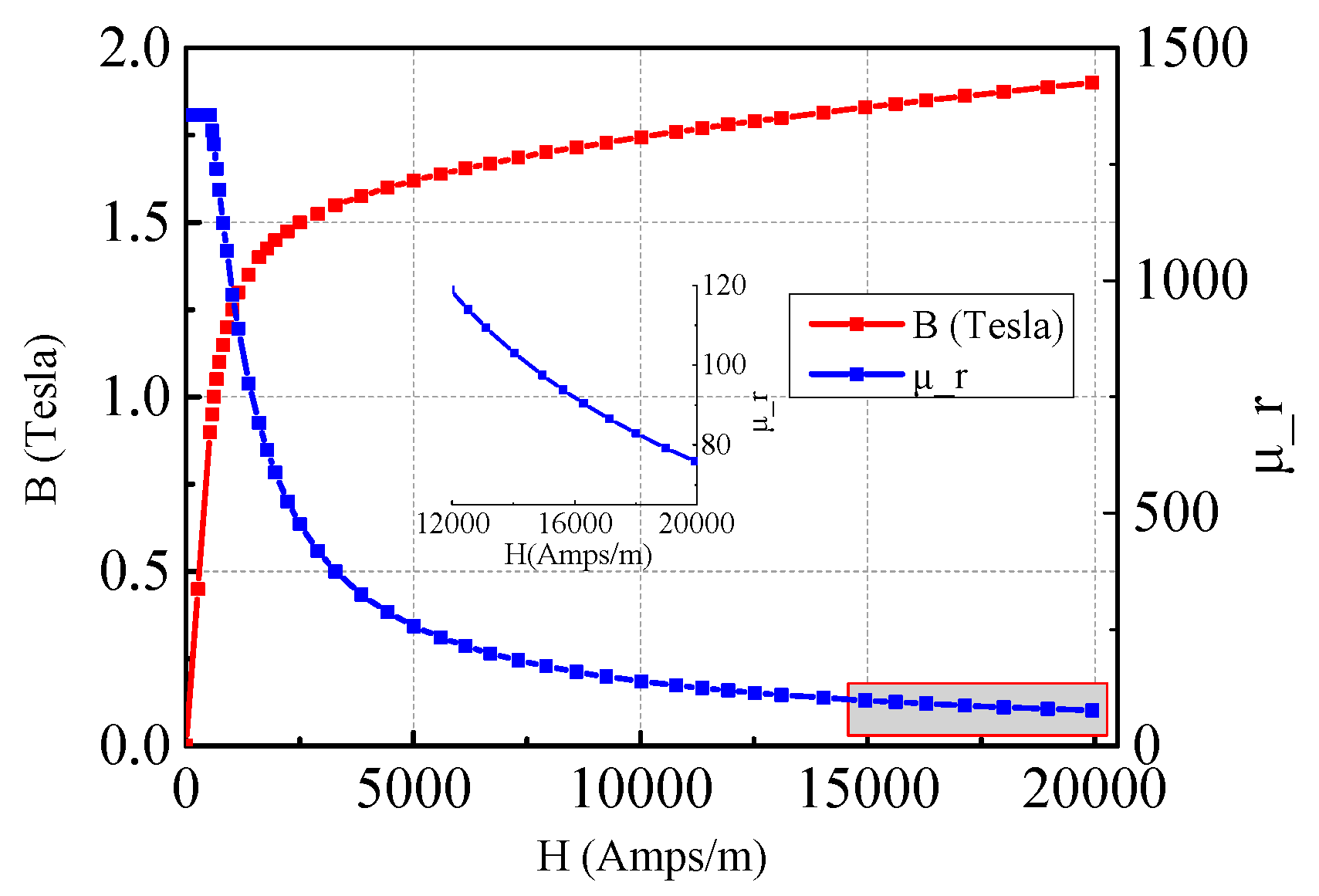
- Assume the initial relative permeability of the calculating point .
- (4)
- Count the vector magnetic potentials by using .
- (5)
- On the basis of the relationship between vector magnetic potential and magnetic field strength (), obtain the magnetic field strength of the rotor core (Hx, Hy, Hz).
- (6)
- Compute the magnetic field strength and obtain the relative permeability corresponding to H by checking the B-H curve.
- (7)
- Calculate the error. If , , return to step 4; otherwise, proceed to the following steps.
- (8)
- Calculate the inducted current density and the copper layer and rotor core loss powers in one segment by using Equations (15) to (17).
- (9)
- Multiply the obtained loss powers by the corresponding volumes to obtain ECLCL and ECLRC in one segment.
3.3. Analytical Model of SECLCL and SECLRC
- (1)
- The materials of the rotor and stator are isotropic. The primary cores have infinite permeability and resistance.
- (2)
- The curvature effect is neglected. The currents only include the z-component.
- (3)
- The time variation of each harmonic field is sinusoidal.
4. Results Comparison and Analysis
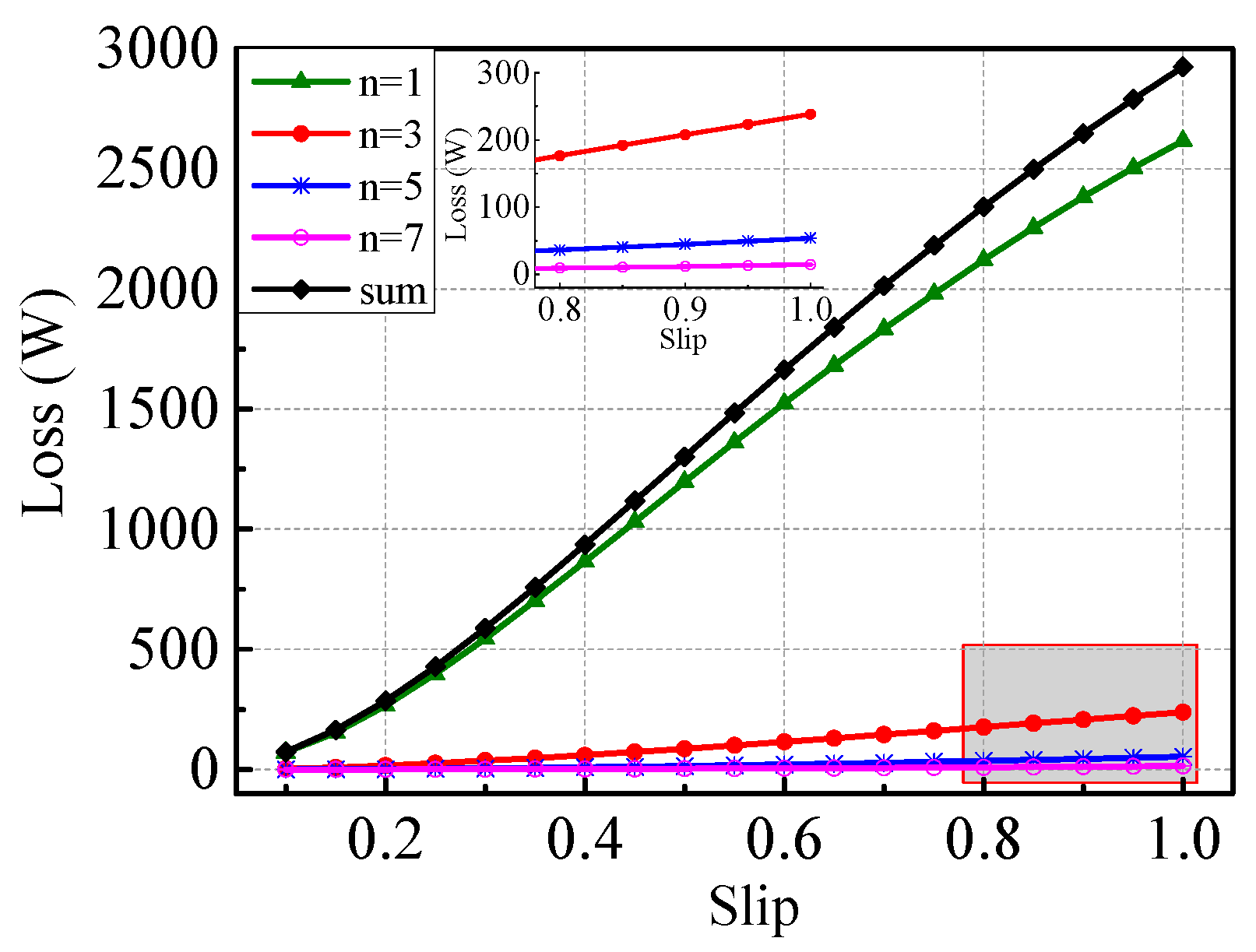
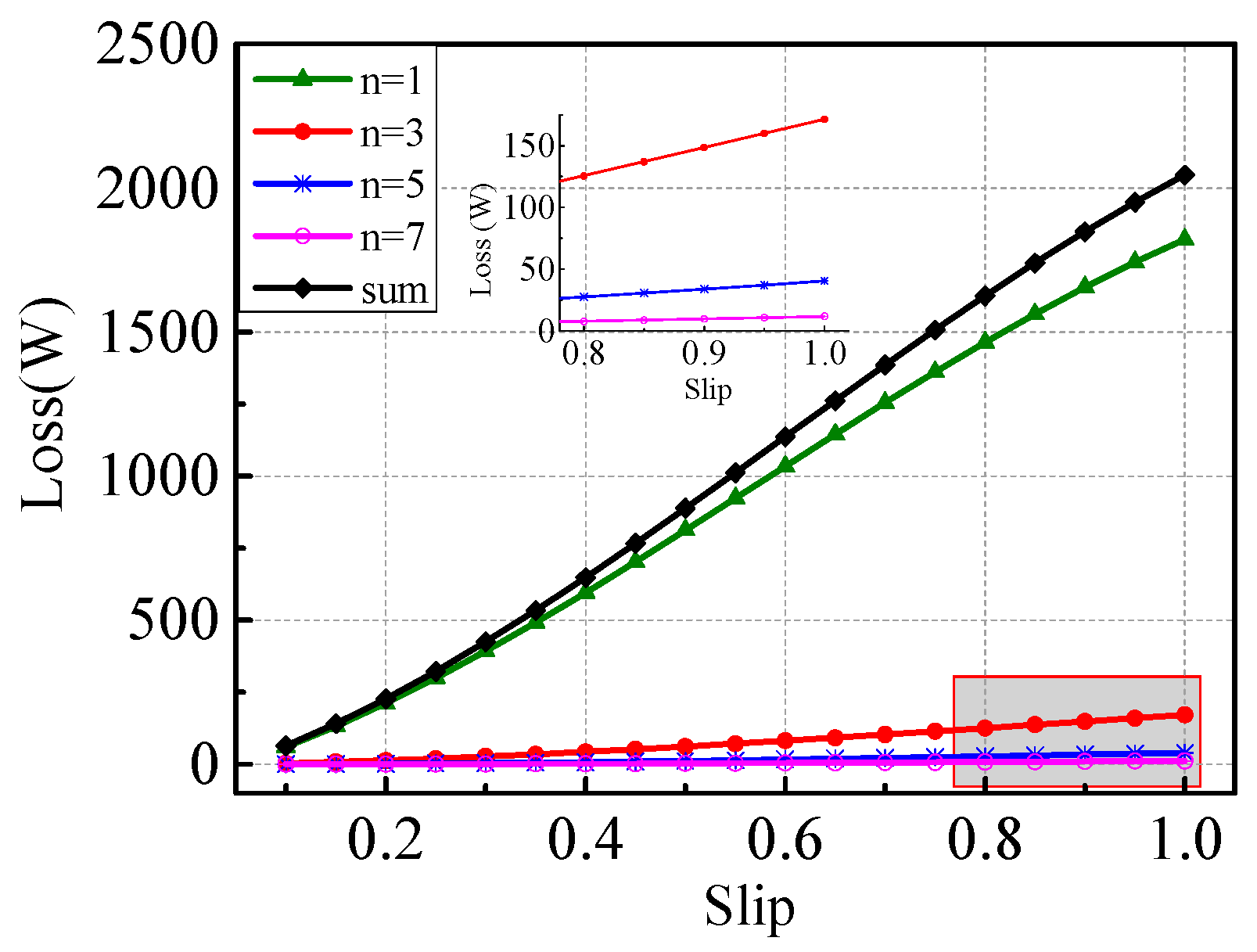
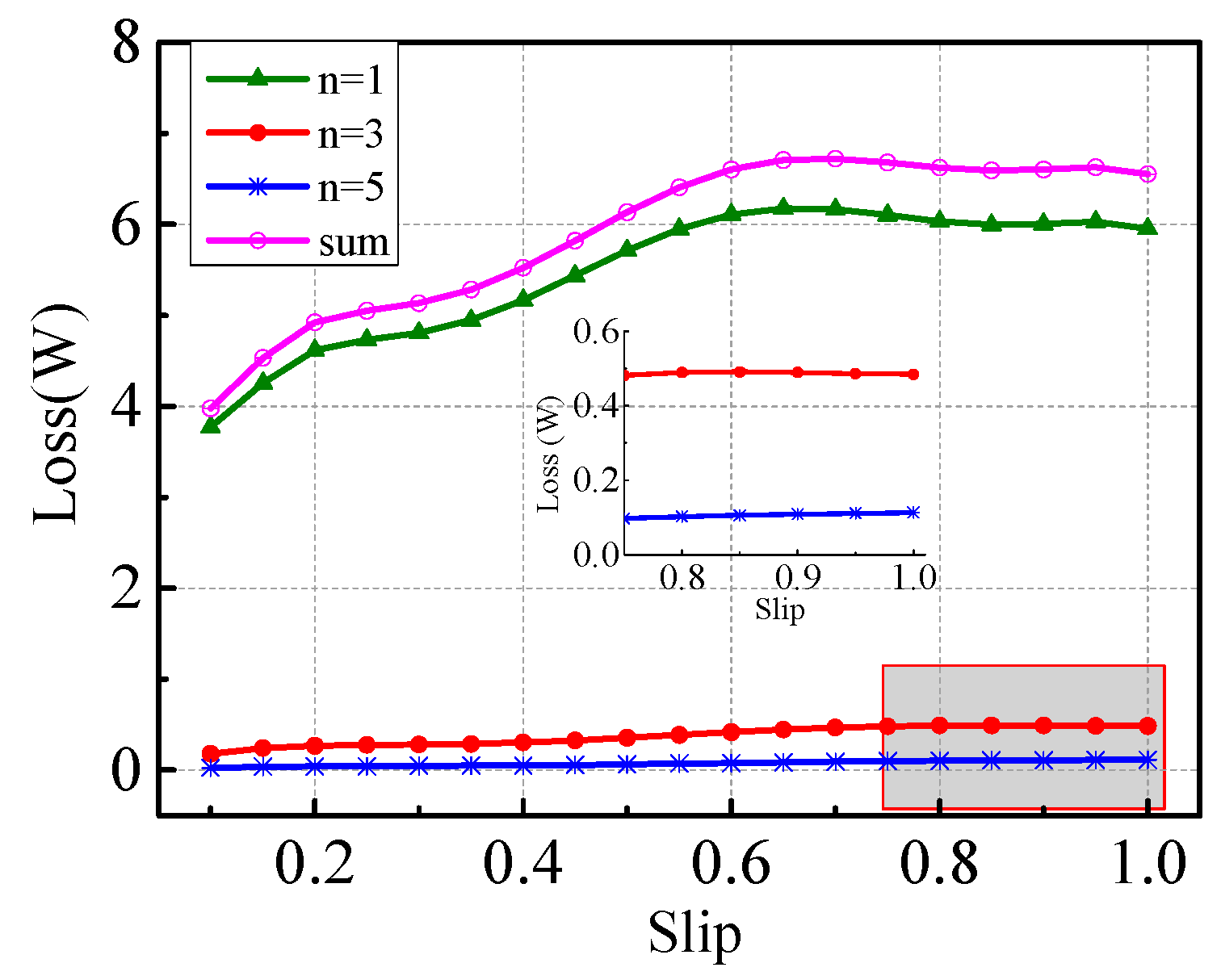
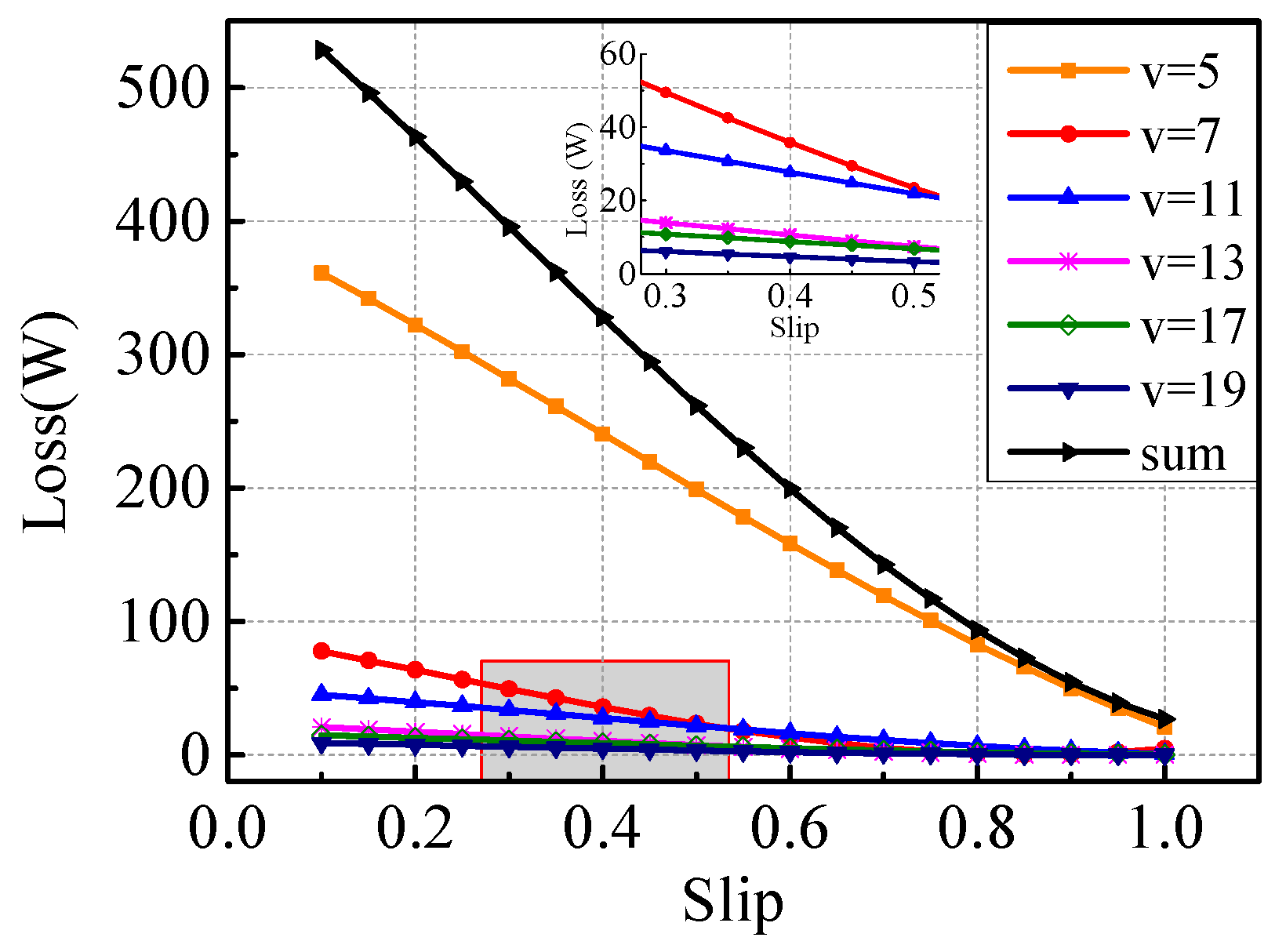
4.1. Copper Layer TECL
- (1)
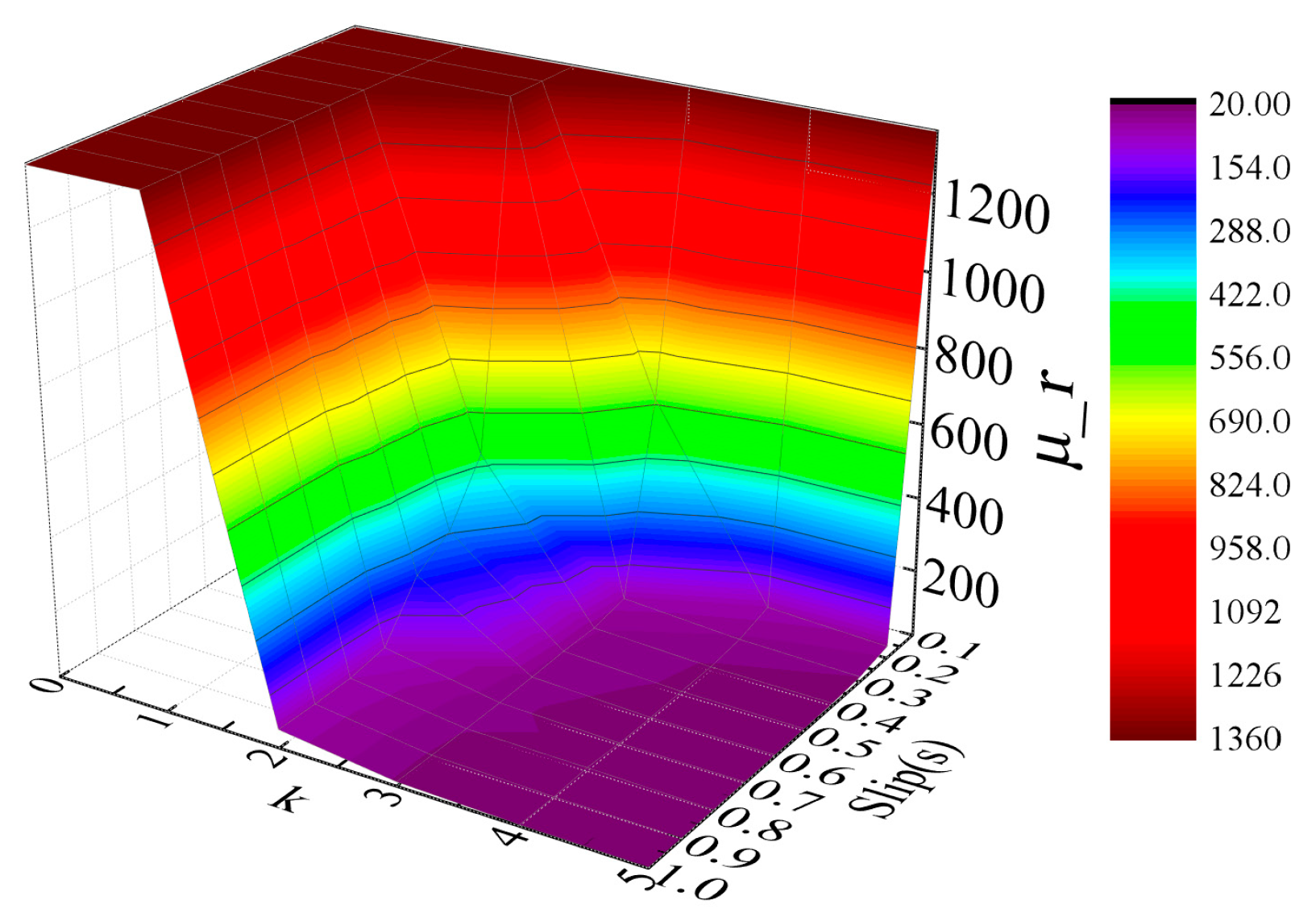
- With the increase in slip, the saturation of the rotor core increases. When the saturation is ignored, the relative permeability of the rotor core is much higher than the actual value, and the magnetic density and analysis value of the loss are large. The new method, which considers the nonlinearity of the rotor core, can improve the accuracy of the rotor core’s relative permeability. Thus, the rotor core’s relative permeability decreases, and the loss analysis value decreases.
- (2)
- The radial inducted current is disregarded. The FEM can consider the loss caused by the radial inducted current.
- (3)
- Owing to the stator circumferential breaking structure, the longitudinal edge effect is formed at the stator end. The end magnetic field and the end induced current caused by the longitudinal edge effect increase the loss in the rotor. According to Assumptions 2 in Section 3.1, the edge effect is ignored in the analysis calculation, so the error increases.
- (4)
- In this work, the period of surface current density is divided into 22 segments (m = 11); m is not large enough and influences the accuracy.
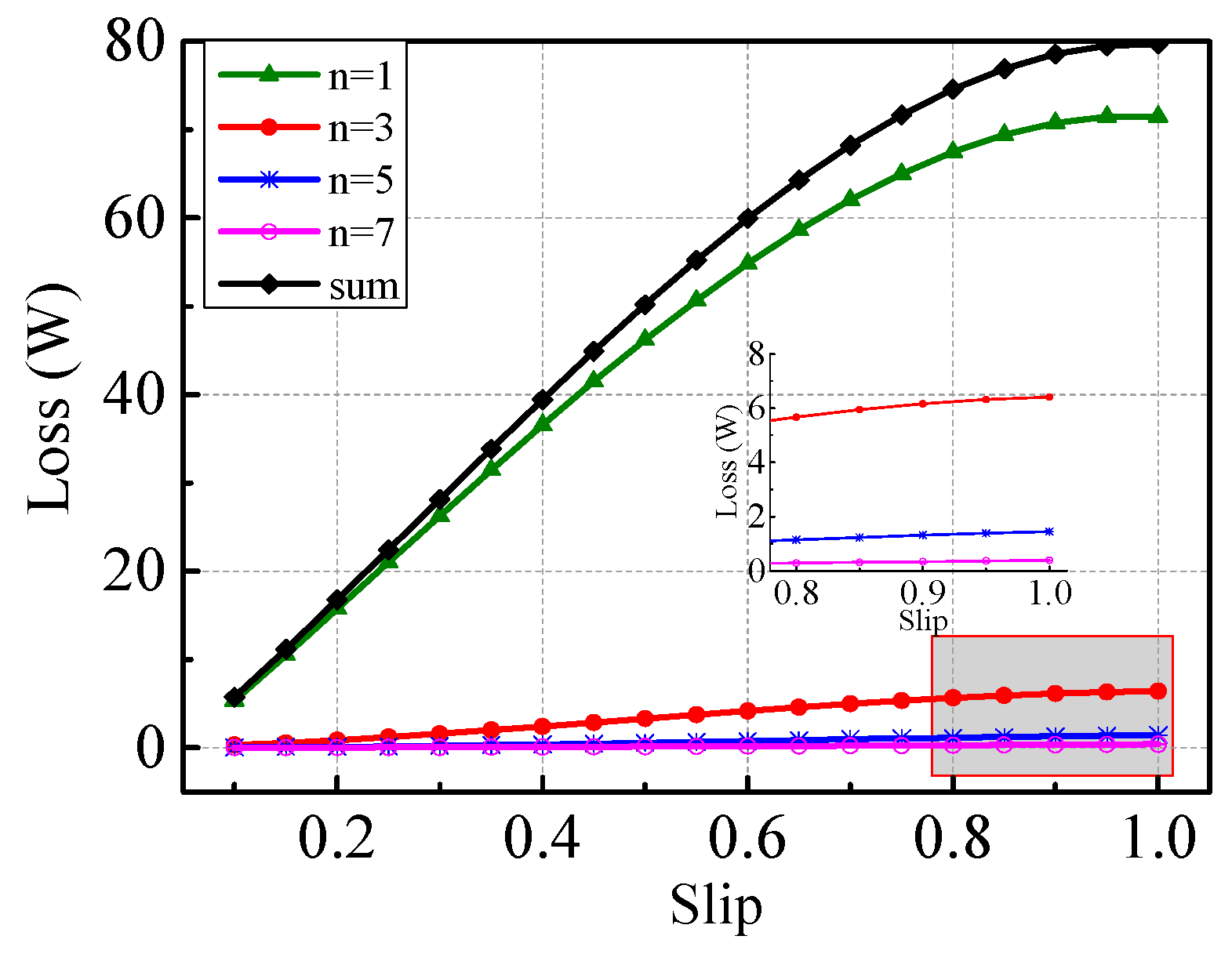
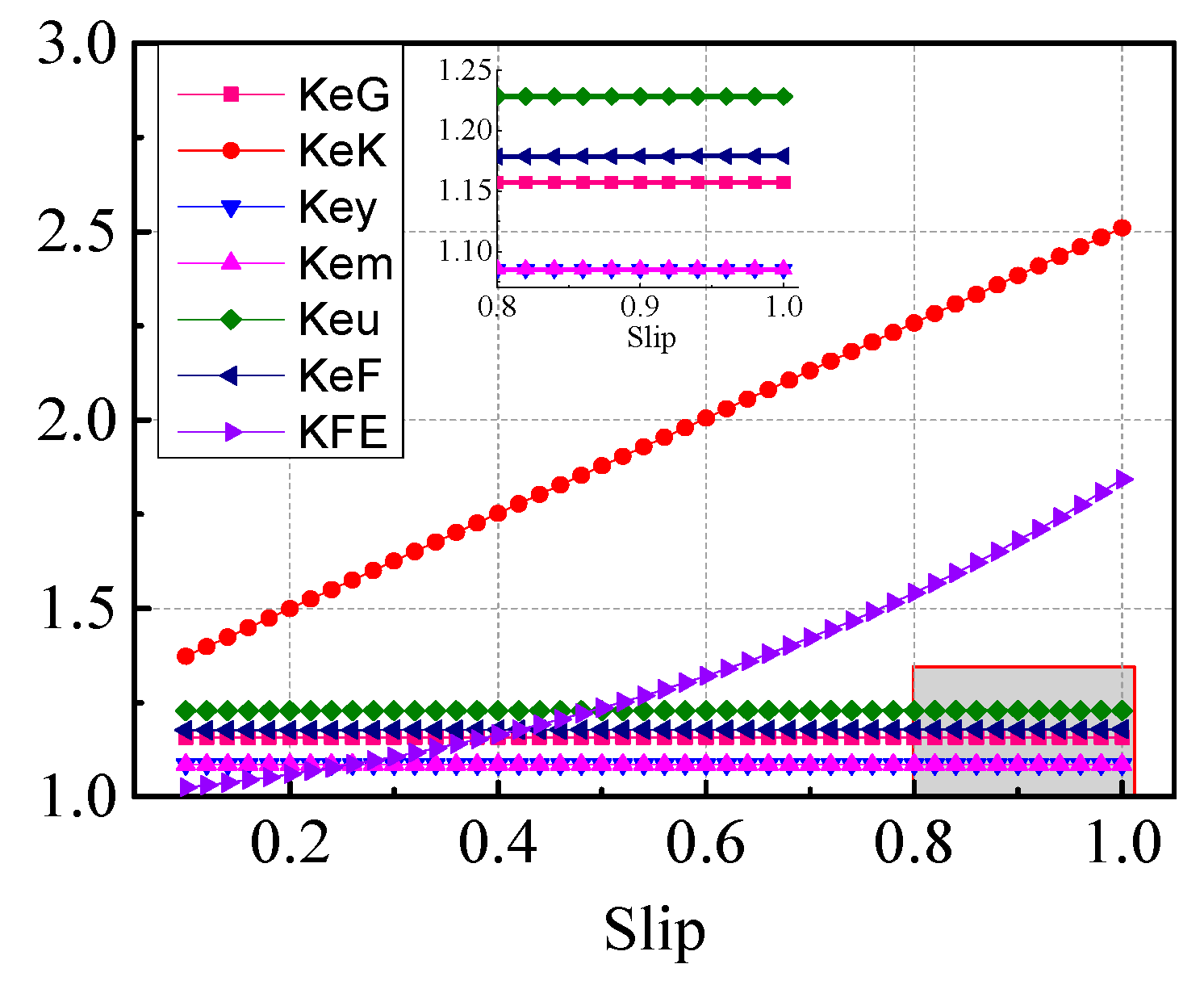
4.2. Rotor Core TECL
5. Conclusions
- (1)
- For TECL in the copper layer, most errors are less than 6%, except for = 0.1–0.2. For the rotor core, the errors of the corrected nonlinear analysis results are less than . Hence, the new analytical method is valid.
- (2)
- In this paper, the time period of is divided into 2m = 22 segments. The value of m influences calculation accuracy. Calculation accuracy and computation time increase with the increase of m.
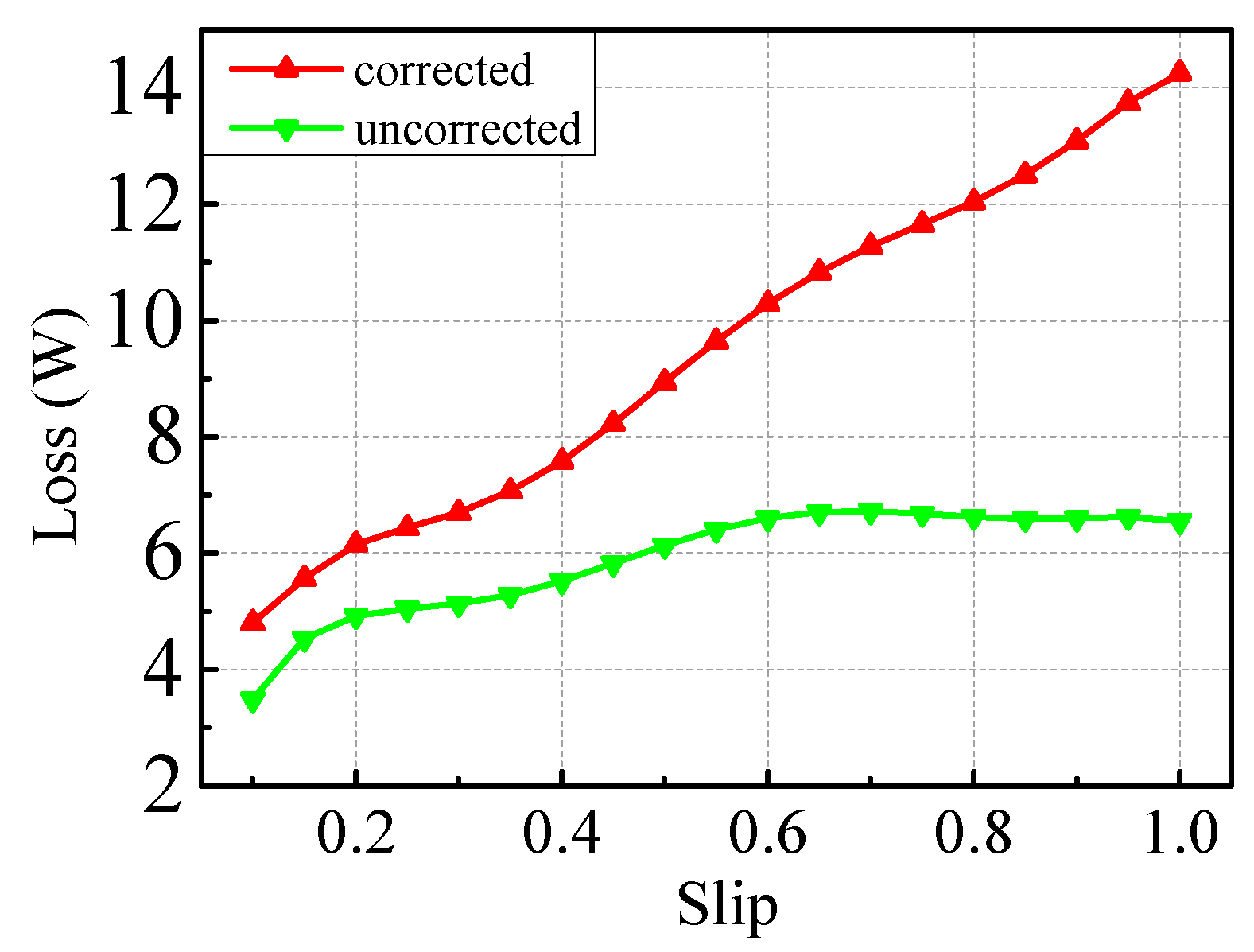
- (3)
- Induced current is observed in the radial direction, and the edge effect occurs at the stator end. Hence, the calculation values are lower than those determined in the simulation, as shown in Figure 17 and Figure 18. The new nonlinear analytical method requires modifications to consider the edge effect and radial induced current.
Author Contributions
Funding
Conflicts of Interest
References
- Si, J.K.; Ai, L.W.; Xie, L.J. Analysis on Electro-magnetic Field and Performance Calculation of 2-DoF Direct Drive Induction Motor. Trans. China Electrotech. Soc. 2015, 30, 153–160. [Google Scholar]
- Si, J.K.; Xie, L.J.; Cao, W.P. Performance analysis of the 2DoF direct drive induction motor applying composite multilayer method. IET Electr. Power Appl. 2017, 11, 524–531. [Google Scholar] [CrossRef]
- Si, J.K.; Si, M.; Xu, X.Z. Effects of rotor conduction material and air gap length on performance of linear are-shaped induction motor. Electr. Mach. Control 2012, 16, 31–37. [Google Scholar]
- Si, J.K.; Feng, H.C.; Ai, L.W. Design and Analysis of a 2-DOF Split-Stator Induction Motor. IEEE Trans. Energy Convers. 2015, 30, 1200–1208. [Google Scholar] [CrossRef]
- Lv, G.; Zeng, D.H.; Zhou, T. Analysis of Secondary Losses and Efficiency in Linear Induction Motors with Composite Secondary Based on Space Harmonic Method. IEEE Trans. Energy Convers. 2017, 32, 1583–1591. [Google Scholar] [CrossRef]
- Lv, G.; Zeng, D.; Zhou, T. Investigation of Forces and Secondary Losses in Linear Induction Motor with the Solid and Laminated Back Iron Secondary for Metro. IEEE Trans. Energy Convers. 2017, 64, 4382–4390. [Google Scholar] [CrossRef]
- Kou, B.Q.; Jin, Y.X.; He, Z. Nonlinear Analytical Modeling of Hybrid-Excitation Double-Sided Linear Eddy-Current Brake. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Shah, M.R.; Lee, S.B. Optimization of Shield Thickness of Finite-Length Solid Rotors for Eddy-Current Loss Minimization. IEEE Trans. Ind. Appl. 2009, 45, 1947–1953. [Google Scholar] [CrossRef]
- Shah, M.R.; Lee, S.B. Rapid Analytical Optimization of Eddy Current Shield Thickness for Associated Loss Minimization in Electrical Machines. IEEE Trans. Ind. Appl. 2006, 42, 642–649. [Google Scholar] [CrossRef]
- Chang, Z.F.; Huang, W.X.; Hu, Y.W. Research on Stray Loss of Smooth Surface Solid Rotor Induction Motor Based on 2D Analytical Approach. Proc. CSEE 2007, 27, 83–88. [Google Scholar]
- Si, J.K.; Xie, L.J.; Feng, H.C. Mathematical Model of Two-Degree-of-Freedom Direct Drive Induction Motor Considering Coupling Effect. J. Electr. Eng. Technol. 2017, 12, 1227–1234. [Google Scholar] [CrossRef]
- Si, J.K.; Xie, L.J.; Xu, X.Z. Static coupling effect of a two-degree-of-freedom direct drive induction motor. IET Electr. Power Appl. 2017, 11, 532–539. [Google Scholar] [CrossRef]
- Zhang, M.Y.; Xia, D.W.; Wang, D.Q. Distribution on the magnetic field of a composite-rotor induction motor. J. Qingdao Univ. 1995, 10, 1–9. [Google Scholar]
- Si, J.K.; Ai, L.W.; Han, J.B. Characteristic analysis of no-load speed of linear induction motor. Electr. Mach. Control 2014, 18, 37–43. [Google Scholar]
- Zhang, M.Y.; Pan, M.Y.; Xia, D.W. Electromagnetic field analysis and end factor determination for a composite rotor motor. Proc. CSEE 1995, 15, 239–246. [Google Scholar]
- Xiao, R.H.; Zhang, M.Y. The analyses and calculation on induction motor with copper-surfaced solid rotors by multi-layer theory. J. Shandong Univ. 1991, 21, 42–49. [Google Scholar]
- Lv, G.; Liu, Z.M.; Sun, S.G. Electromagnetism calculation of single-sided linear induction motor with transverse asymmetry using finite-element method. IET Electr. Power Appl. 2016, 10, 63–73. [Google Scholar] [CrossRef]
- Cheaytani, J.; Benabou, A.; Tounzi, A. Stray Load Losses Analysis of Cage Induction Motor Using 3-D Finite-Element Method with External Circuit Coupling. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Yamada, T.; Fujisaki, K. Basic Characteristic of Electromagnetic Force in Induction Heating Application of Linear Induction Motor. IEEE Trans. Magn. 2008, 44, 4070–4073. [Google Scholar] [CrossRef]
- Aho, T.; Nerg, J.; Pyrhonen, J. Analyzing the effect of the rotor coating on the rotor losses of medium-speed solid-rotor induction motor. In Proceedings of the International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM 2006), Taormina, Italy, 23–26 May 2006. [Google Scholar]
- Bi, D.Q.; Wang, X.H.; Li, D.C. Magnetic analysis and design method for solid rotor induction motors based on directly coupled field-circuit. In Proceedings of the Third International Power Electronics and Motion Control Conference (IPEMC 2000), Beijing, China, 15–18 August 2000. [Google Scholar]
- Gibbs, W. Induction and synchronous motors with unlaminated rotors. J. Inst. Electr. Eng. 1948, 95, 411–420. [Google Scholar]
- Kesavamurthy, N.; Rajagopolan, P.K. The Polyphase Induction Machine with Solid Iron Rotor. Trans. Am. Inst. Electr. Eng. 1959, 78, 1092–1098. [Google Scholar] [CrossRef]
- Yee, H. Effects of finite length in solid-rotor induction machines. Proc. Inst. Electr. Eng. 1971, 118, 1025–1033. [Google Scholar] [CrossRef]
- Tang, X.G.; Ning, Y.Q.; Fu, F.L. Solid Rotor Asynchronous Motor and Its Application, 1st ed.; China Machine Press: Beijing, China, 1991. (In Chinese) [Google Scholar]
- Fu, F.L.; Tang, X.G. Handbook of Induction Motor Design, 2nd ed.; China Machine Press: Beijing, China, 2006. (In Chinese) [Google Scholar]
- Fu, F.L.; Lin, J.M. End coefficient calculation of solid rotor asynchronous motor. S&M Electr. Mach. 1986, 3, 5–9. [Google Scholar]
- Hu, Y.; Chen, J.Z.; Ding, X.Y. Analysis and Computation on Magnetic Field of Solid Rotor Induction Motor. IEEE Trans. Appl. Supercon. 2010, 20, 1844–1847. [Google Scholar]
- Chang, Z.F. Composite Rotor Induction Motor Electromagnetic Calculation. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2007. [Google Scholar]
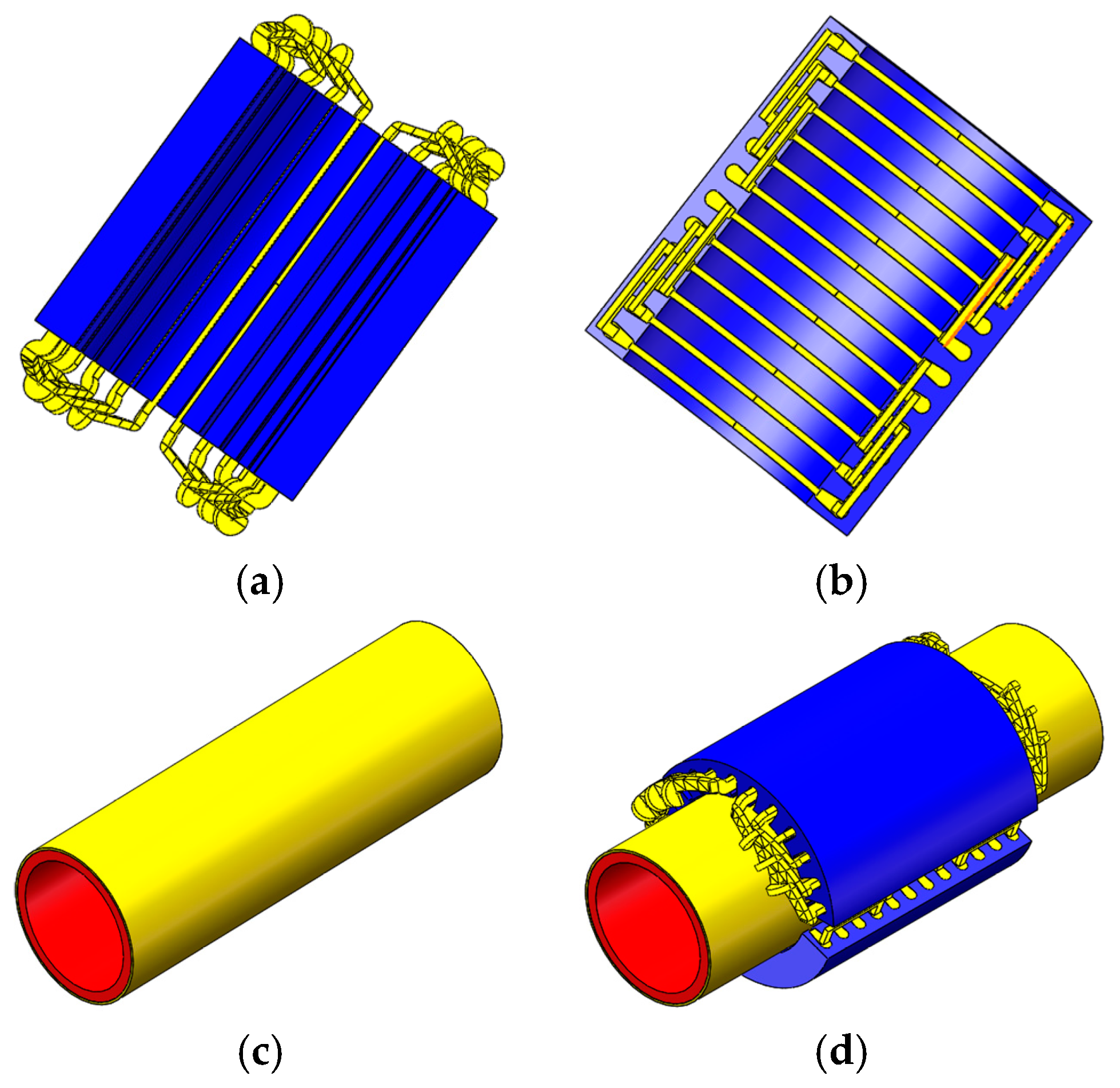
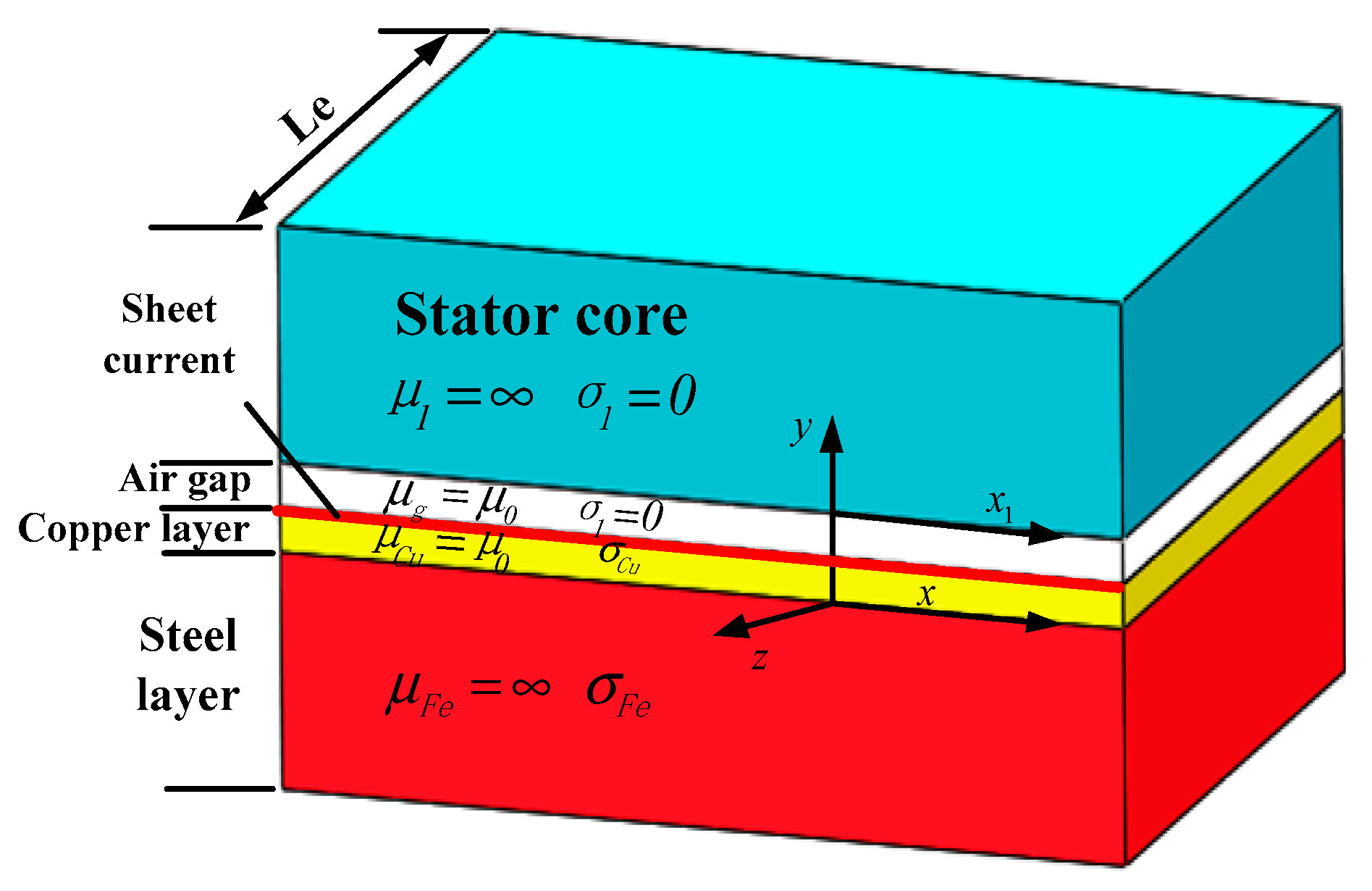





| Item | Parameter |
|---|---|
| rated voltage | 220 V (Y) |
| stator outer diameter | 155 mm |
| stator inter diameter | 98 mm |
| stator axial length | 156 mm |
| slot number | 12 |
| phase | 3 |
| pole pair | 2 |
| thickness of air gap | 1 mm |
| thickness of copper layer | 1.5 mm |
| frequency | 50 Hz |
| Slip | FEM (W) | Linear (W) | Error (%) | Nonlinear (W) | Error (%) |
|---|---|---|---|---|---|
| 0.1 | 717.33 | 602.037 | 16.07 | 592.333 | 17.43 |
| 0.2 | 769.09 | 748.601 | 2.664 | 690.507 | 10.22 |
| 0.3 | 867.74 | 983.466 | 13.34 | 820.599 | 5.433 |
| 0.4 | 1004.3 | 1264.13 | 25.87 | 976.030 | 2.818 |
| 0.5 | 1169.9 | 1563.02 | 33.60 | 1150.22 | 1.684 |
| 0.6 | 1355.6 | 1863.41 | 37.46 | 1336.60 | 1.399 |
| 0.7 | 1552.3 | 2155.96 | 38.89 | 1528.57 | 1.529 |
| 0.8 | 1751.2 | 2435.95 | 39.10 | 1719.57 | 1.806 |
| 0.9 | 1943.3 | 2701.24 | 39.04 | 1903.02 | 2.074 |
| 1 | 2119.7 | 2950.84 | 39.21 | 2072.34 | 2.235 |
| Slip | FEM (W) | Linear (W) | Error (%) | Nonlinear (W) | Error (%) |
|---|---|---|---|---|---|
| 0.1 | 4.837 | 5.719 | 18.24 | 4.808 | 0.593 |
| 0.2 | 6.028 | 16.75 | 177.8 | 6.158 | 2.167 |
| 0.3 | 7.100 | 28.14 | 296.3 | 6.702 | 5.596 |
| 0.4 | 8.078 | 39.44 | 388.3 | 7.587 | 6.068 |
| 0.5 | 8.985 | 50.19 | 458.7 | 8.942 | 0.474 |
| 0.6 | 9.844 | 59.94 | 508.9 | 10.29 | 4.546 |
| 0.7 | 10.68 | 68.22 | 538.7 | 11.28 | 5.591 |
| 0.8 | 11.52 | 74.59 | 547.6 | 12.04 | 4.551 |
| 0.9 | 12.38 | 78.57 | 534.7 | 13.09 | 5.738 |
| 1 | 13.29 | 79.72 | 499.8 | 14.25 | 7.233 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, W.; Si, J.; Feng, H.; Cheng, Z.; Hu, Y.; Gan, C. Rotor Eddy Current Loss Calculation of a 2DoF Direct-Drive Induction Motor. Energies 2019, 12, 1134. https://doi.org/10.3390/en12061134
Wu W, Si J, Feng H, Cheng Z, Hu Y, Gan C. Rotor Eddy Current Loss Calculation of a 2DoF Direct-Drive Induction Motor. Energies. 2019; 12(6):1134. https://doi.org/10.3390/en12061134
Chicago/Turabian StyleWu, Wei, Jikai Si, Haichao Feng, Zhiping Cheng, Yihua Hu, and Chun Gan. 2019. "Rotor Eddy Current Loss Calculation of a 2DoF Direct-Drive Induction Motor" Energies 12, no. 6: 1134. https://doi.org/10.3390/en12061134





