Probing Red Blood Cell Membrane Microviscosity Using Fluorescence Anisotropy Decay Curves of the Lipophilic Dye PKH26
Abstract
:1. Introduction
2. Results
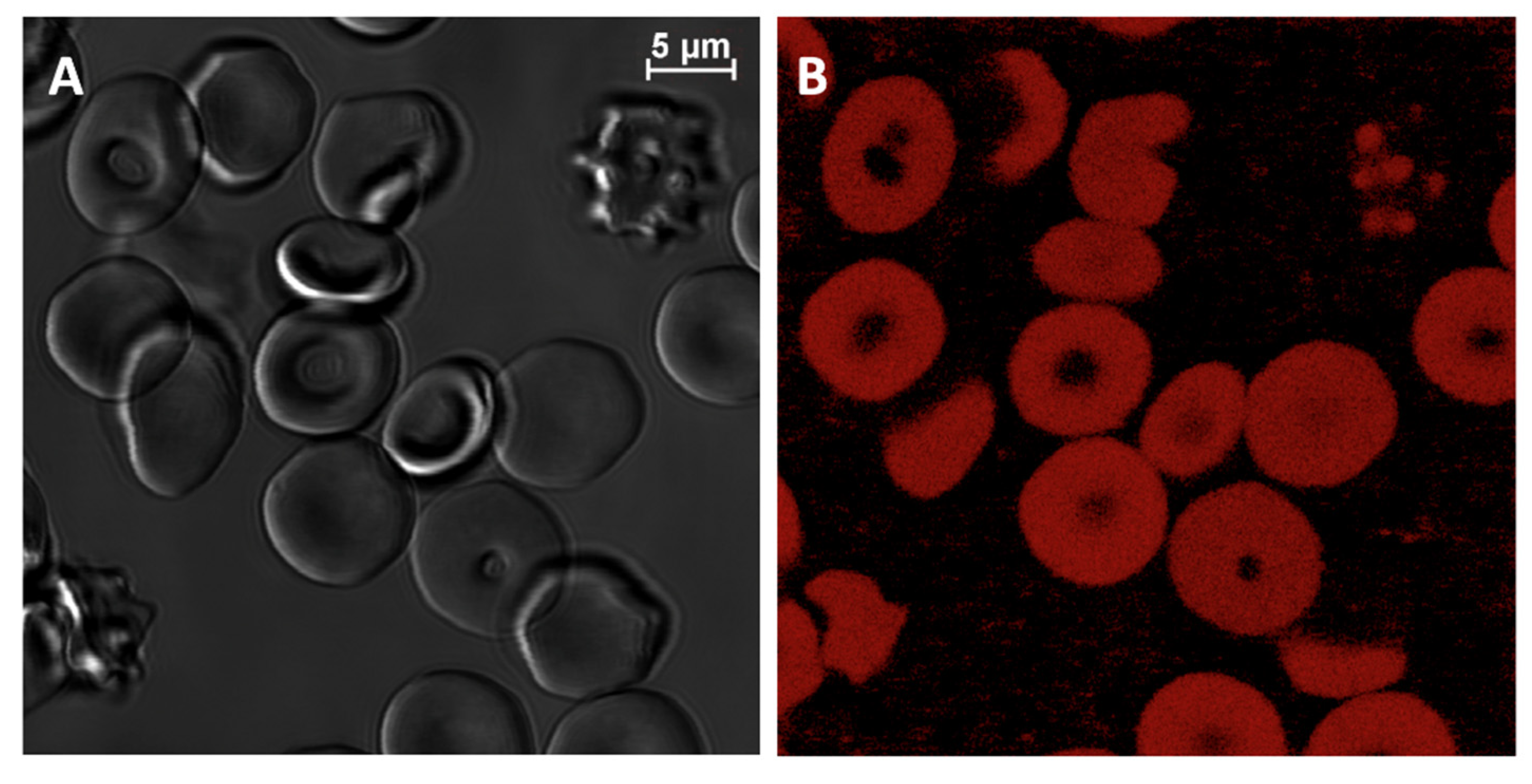
2.1. Laser Confocal Microscopy Measurements of RBC, Stained with PKH26 Fluorescent Probe
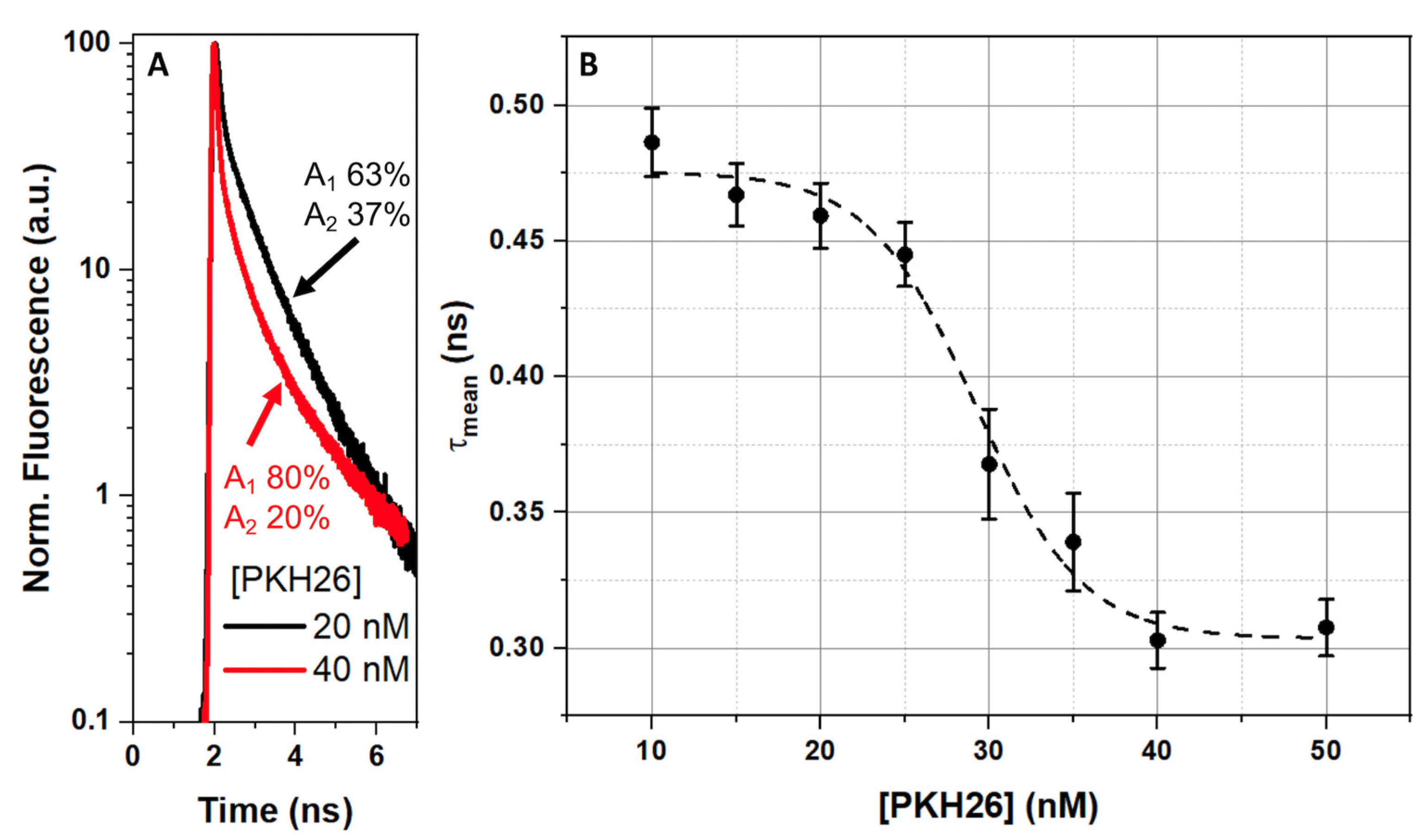
2.2. Measurements of Time-Resolved Fluorescence of PKH26 Probe Bounded by RBC
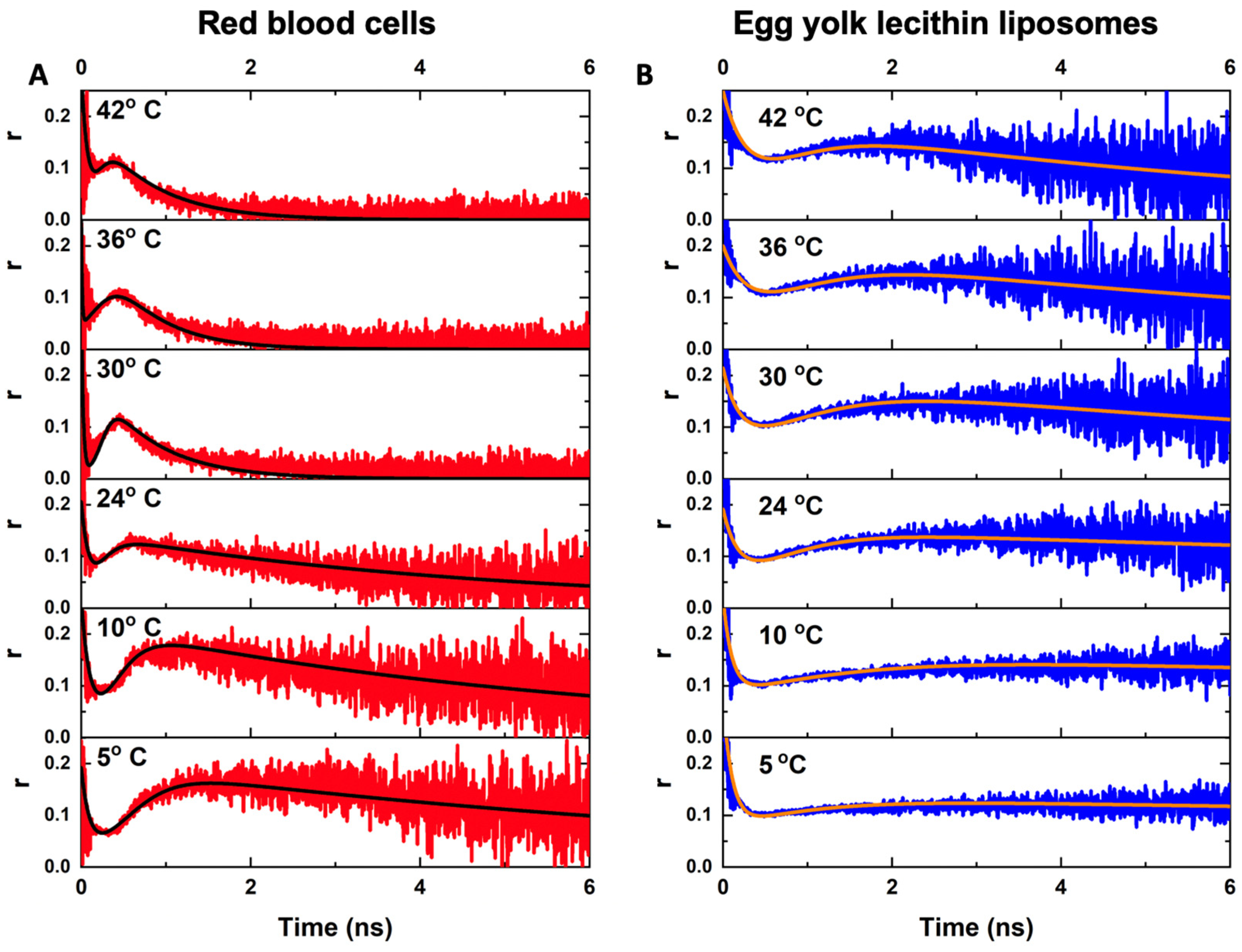
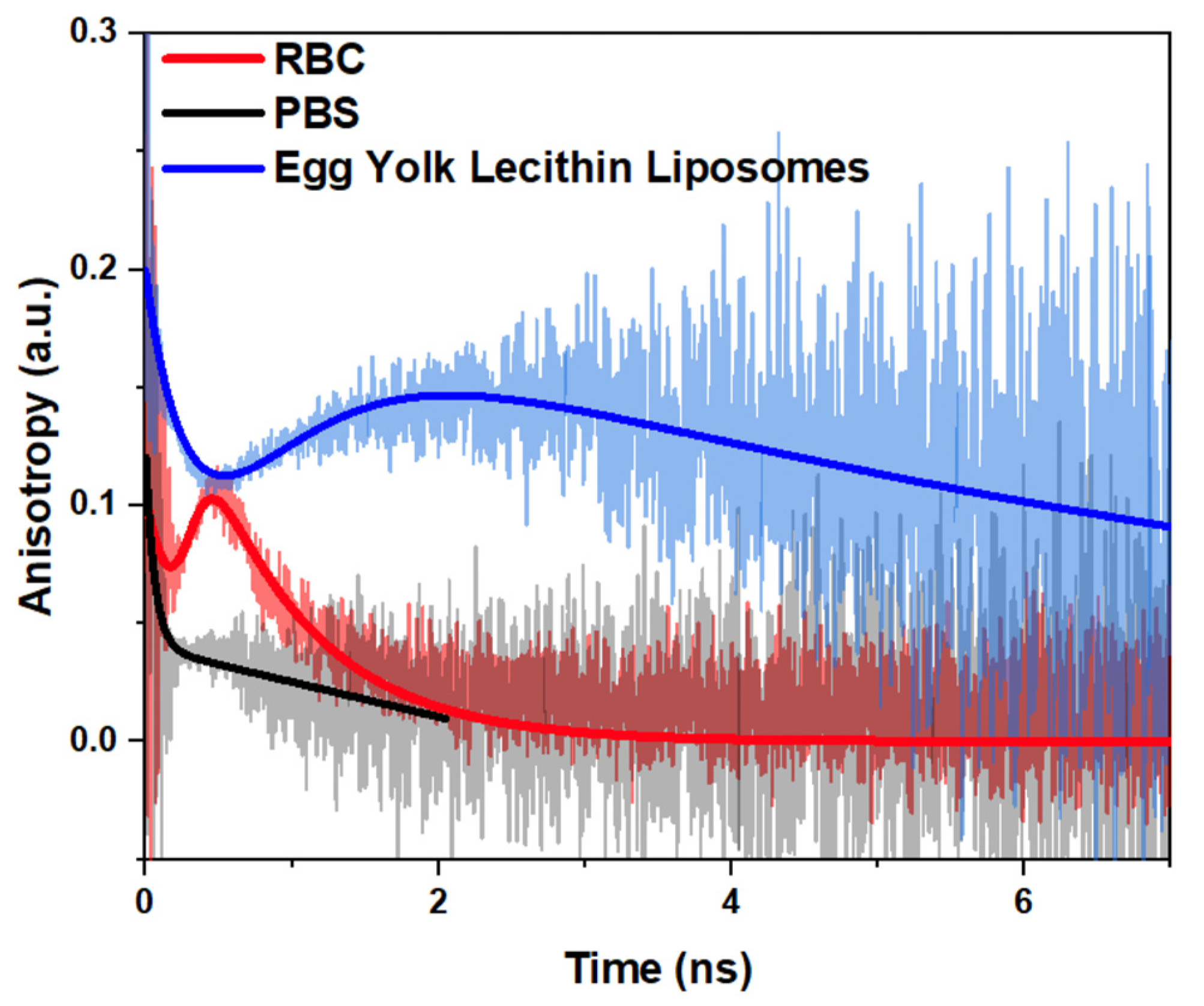
2.3. Anisotropy Decay of PKH26 Fluorescent Probe within the Membranes of RBCs and Egg Yolk Lecithin Liposomes at Different Temperatures
3. Discussion
4. Materials and Methods
4.1. Blood Sampling and RBC Extraction
4.2. Liposomes Synthesis
4.3. Confocal Microscopy
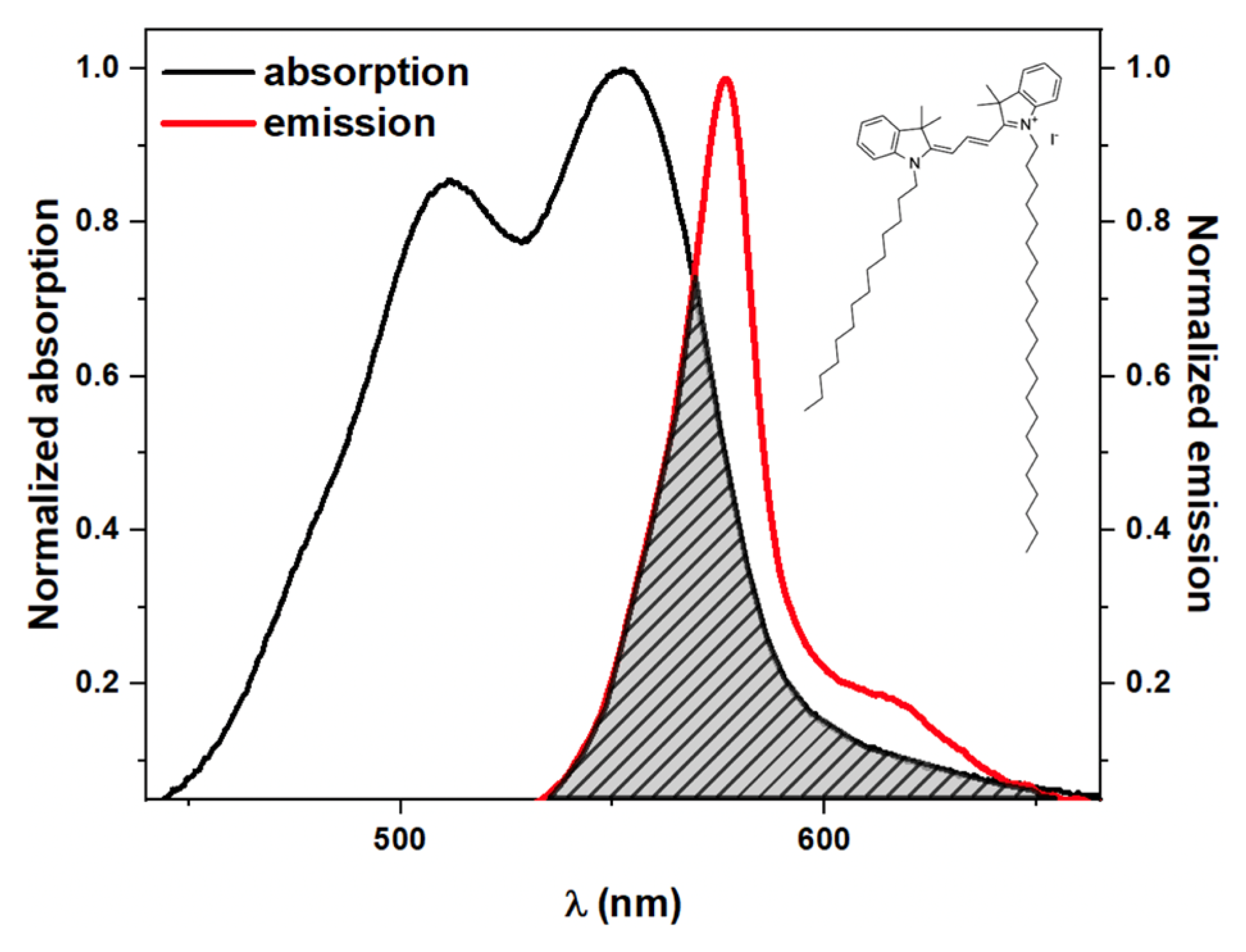
4.4. Time-Resolved Fluorescence Anisotropy, Absorption, and Emission Spectroscopy Measurements
4.5. Assessment of Microviscosity Using Time-Resolved Fluorescence Anisotropy
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Huisjes, R.; Bogdanova, A.; van Solinge, W.W.; Schiffelers, R.M.; Kaestner, L.; van Wijk, R. Squeezing for Life—Properties of Red Blood Cell Deformability. Front. Physiol. 2018, 9, 1–22. [Google Scholar] [CrossRef] [Green Version]
- Baskurt, O.K.; Hardeman, M.R.; Rampling, M.W.; Meiselman, H.J. Handbook of Hemorheology and Hemodynamics; IOS Press: Amsterdam, The Netherlands, 2007; ISBN 9781586037710. [Google Scholar]
- Antonova, N.; Velcheva, I.; Paskova, V. Hemorheological and Microvascular Disturbances in Patients with Type 2 Diabetes Mellitus. Clin. Hemorheol. Microcirc. 2022, 81, 325–341. [Google Scholar] [CrossRef]
- Li, Q.; Li, L.; Li, Y. Enhanced RBC Aggregation in Type 2 Diabetes Patients. J. Clin. Lab. Anal. 2015, 29, 387–389. [Google Scholar] [CrossRef]
- Singh, M.; Shin, S. Changes in Erythrocyte Aggregation and Deformability in Diabetes Mellitus: A Brief Review. Indian J. Exp. Biol. 2009, 47, 7–15. [Google Scholar]
- Deng, Y.; Papageorgiou, D.P.; Li, X.; Perakakis, N.; Mantzoros, C.S.; Dao, M.; Karniadakis, G.E. Quantifying Fibrinogen-Dependent Aggregation of Red Blood Cells in Type 2 Diabetes Mellitus. Biophys. J. 2020, 119, 900–912. [Google Scholar] [CrossRef] [PubMed]
- Lugovtsov, A.E.; Gurfinkel, Y.I.; Ermolinskiy, P.B.; Maslyanitsina, A.I.; Dyachuk, L.I.; Priezzhev, A.V. Optical Assessment of Alterations of Microrheologic and Microcirculation Parameters in Cardiovascular Diseases. Biomed. Opt. Express 2019, 10, 3974–3986. [Google Scholar] [CrossRef] [PubMed]
- Ermolinskiy, P.; Lugovtsov, A.; Maslyanitsina, A.; Semenov, A.; Dyachuk, L.; Priezzhev, A. Interaction of Erythrocytes in the Process of Pair Aggregation in Blood Samples from Patients with Arterial Hypertension and Healthy Donors: Measurements with Laser Tweezers. J. Biomed. Photonics Eng. 2018, 4, 030303. [Google Scholar] [CrossRef] [Green Version]
- Maslianitsyna, A.; Ermolinskiy, P.; Lugovtsov, A.; Pigurenko, A.; Sasonko, M.; Gurfinkel, Y.; Priezzhev, A. Multimodal Diagnostics of Microrheologic Alterations in Blood of Coronary Heart Disease and Diabetic Patients. Diagnostics 2021, 11, 76. [Google Scholar] [CrossRef]
- Kim, J.; Chung, H.; Cho, M.; Lee, B.-K.; Karimi, A.; Shin, S. The Role of Critical Shear Stress on Acute Coronary Syndrome. Clin. Hemorheol. Microcirc. 2013, 55, 101–109. [Google Scholar] [CrossRef] [Green Version]
- Lee, H.; Na, W.; Lee, S.B.; Ahn, C.W.; Moon, J.S.; Won, K.C.; Shin, S. Potential Diagnostic Hemorheological Indexes for Chronic Kidney Disease in Patients With Type 2 Diabetes. Front. Physiol. 2019, 10, 01062. [Google Scholar] [CrossRef]
- Lee, S.B.; Kim, Y.-S.; Kim, J.H.; Park, K.; Nam, J.S.; Kang, S.; Park, J.S.; Shin, S.; Ahn, C.W. Use of RBC Deformability Index as an Early Marker of Diabetic Nephropathy. Clin. Hemorheol. Microcirc. 2019, 72, 75–84. [Google Scholar] [CrossRef] [PubMed]
- Renoux, C.; Fort, R.; Nader, E.; Boisson, C.; Joly, P.; Stauffer, E.; Robert, M.; Girard, S.; Cibiel, A.; Gauthier, A.; et al. Impact of COVID-19 on Red Blood Cell Rheology. Br. J. Haematol. 2021, 192, e108–e111. [Google Scholar] [CrossRef] [PubMed]
- Nader, E.; Nougier, C.; Boisson, C.; Poutrel, S.; Catella, J.; Martin, F.; Charvet, J.; Girard, S.; Havard-Guibert, S.; Martin, M.; et al. Increased Blood Viscosity and Red Blood Cell Aggregation in Patients with COVID-19. Am. J. Hematol. 2022, 97, 283–292. [Google Scholar] [CrossRef]
- Jung, E.M.; Stroszczynski, C.; Jung, F. Contrast Enhanced Ultrasonography (CEUS) to Detect Abdominal Microcirculatory Disorders in Severe Cases of COVID-19 Infection: First Experience. Clin. Hemorheol. Microcirc. 2020, 74, 353–361. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Koutsiaris, A.G.; Riri, K.; Boutlas, S.; Panagiotou, T.N.; Kotoula, M.; Daniil, Z.; Tsironi, E.E. COVID-19 Hemodynamic and Thrombotic Effect on the Eye Microcirculation after Hospitalization: A Quantitative Case-Control Study. Clin. Hemorheol. Microcirc. 2022, 1–12. [Google Scholar] [CrossRef]
- Baskurt, O.K.; Neu, B.; Meiselman, H. Red Blood Cell Aggregation; CRC Press: Boca Raton, FL, USA, 2012; ISBN 9781439841815. [Google Scholar]
- Lee, K.; Wagner, C.; Priezzhev, A.V. Assessment of the “Cross-Bridge”—Induced Interaction of Red Blood Cells by Optical Trapping Combined with Microfluidics. J. Biomed. Opt. 2017, 22, 091516-1–091516-6. [Google Scholar] [CrossRef]
- Wagner, C.; Steffen, P.; Svetina, S. Aggregation of Red Blood Cells: From Rouleaux to Clot Formation. Comptes Rendus Phys. 2013, 14, 459–469. [Google Scholar] [CrossRef] [Green Version]
- de Oliveira, S.; de Almeida, V.V.; Calado, A.; Rosário, H.S.; Saldanha, C. Integrin-Associated Protein (CD47) Is a Putative Mediator for Soluble Fibrinogen Interaction with Human Red Blood Cells Membrane. Biochim. Biophys. Acta Biomembr. 2012, 1818, 481–490. [Google Scholar] [CrossRef] [Green Version]
- Lee, K.; Shirshin, E.; Rovnyagina, N.; Yaya, F.; Boujja, Z.; Priezzhev, A.; Wagner, C. Dextran Adsorption onto Red Blood Cells Revisited: Single Cell Quantification by Laser Tweezers Combined with Microfluidics. Biomed Opt. Express 2018, 9, 2755–2764. [Google Scholar] [CrossRef]
- Semenov, A.N.; Lugovtsov, A.E.; Shirshin, E.A.; Yakimov, B.P.; Ermolinskiy, P.B.; Bikmulina, P.Y.; Kudryavtsev, D.S.; Timashev, P.S.; Muravyov, A.V.; Wagner, C.; et al. Assessment of Fibrinogen Macromolecules Interaction with Red Blood Cells Membrane by Means of Laser Aggregometry, Flow Cytometry, and Optical Tweezers Combined with Microfluidics. Biomolecules 2020, 10, 1448. [Google Scholar] [CrossRef]
- Mohandas, N.; Gallagher, P.G. Red Cell Membrane: Past, Present, and Future. Blood 2008, 112, 3939–3948. [Google Scholar] [CrossRef]
- Muravyov, A.V.; Tikhomirova, I.A.; Maimistova, A.A.; Bulaeva, S.V. Extra- and Intracellular Signaling Pathways under Red Blood Cell Aggregation and Deformability Changes. Clin. Hemorheol. Microcirc. 2009, 43, 223–232. [Google Scholar] [CrossRef] [PubMed]
- Ugurel, E.; Goksel, E.; Cilek, N.; Kaga, E.; Yalcin, O. Proteomic Analysis of the Role of the Adenylyl Cyclase–CAMP Pathway in Red Blood Cell Mechanical Responses. Cells 2022, 11, 1250. [Google Scholar] [CrossRef] [PubMed]
- Lazari, D.; Freitas Leal, J.K.; Brock, R.; Bosman, G. The Relationship Between Aggregation and Deformability of Red Blood Cells in Health and Disease. Front. Physiol. 2020, 11, 288. [Google Scholar] [CrossRef] [PubMed]
- Maslyanitsyna, A.I.; Ermolinsky, P.B.; Lugovtsov, A.E.; Priezzhev, A.V. Study by Optical Techniques of the Dependence of Aggregation Parameters of Human Red Blood Cells on Their Deformability. J. Biomed. Photonics. Eng. 2020, 6, 020305. [Google Scholar] [CrossRef]
- de Franceschi, L.; Bosman, G.J.C.G.M.; Mohandas, N. Abnormal Red Cell Features Associated with Hereditary Neurodegenerative Disorders: The Neuroacanthocytosis Syndromes. Curr. Opin. Hematol. 2014, 21, 201–209. [Google Scholar] [CrossRef] [Green Version]
- Cluitmans, J.C.A.; Tomelleri, C.; Yapici, Z.; Dinkla, S.; Bovee-Geurts, P.; Chokkalingam, V.; de Franceschi, L.; Brock, R.; Bosman, G.J.G.C.M. Abnormal Red Cell Structure and Function in Neuroacanthocytosis. PLoS ONE 2015, 10, e0125580. [Google Scholar] [CrossRef]
- Gounley, J.; Peng, Y. Computational Modeling of Membrane Viscosity of Red Blood Cells. Commun. Comput. Phys. 2015, 17, 1073–1087. [Google Scholar] [CrossRef]
- Hoore, M.; Yaya, F.; Podgorski, T.; Wagner, C.; Gompper, G.; Fedosov, D.A. Effect of Spectrin Network Elasticity on the Shapes of Erythrocyte Doublets. Soft. Matter. 2018, 14, 6278–6289. [Google Scholar] [CrossRef]
- Trejo-Soto, C.; Lázaro, G.R.; Pagonabarraga, I.; Hernández-Machado, A. Microfluidics Approach to the Mechanical Properties of Red Blood Cell Membrane and Their Effect on Blood Rheology. Membranes 2022, 12, 217. [Google Scholar] [CrossRef]
- Kang, Y.J.; Serhrouchni, S.; Makhro, A.; Bogdanova, A.; Lee, S.S. Simple Assessment of Red Blood Cell Deformability Using Blood Pressure in Capillary Channels for Effective Detection of Subpopulations in Red Blood Cells. ACS Omega 2022, 7, 38576–38588. [Google Scholar] [CrossRef] [PubMed]
- Louka, M.; Kaliviotis, E. Development of an Optical Method for the Evaluation of Whole Blood Coagulation. Biosensors 2021, 11, 113. [Google Scholar] [CrossRef] [PubMed]
- Shimolina, L.E.; Izquierdo, M.A.; López-Duarte, I.; Bull, J.A.; Shirmanova, M.V.; Klapshina, L.G.; Zagaynova, E.V.; Kuimova, M.K. Imaging Tumor Microscopic Viscosity in Vivo Using Molecular Rotors. Sci. Rep. 2017, 7, srep41097. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shimolina, L.; Gulin, A.; Ignatova, N.; Druzhkova, I.; Gubina, M.; Lukina, M.; Snopova, L.; Zagaynova, E.; Kuimova, M.K.; Shirmanova, M.; et al. The Role of Plasma Membrane Viscosity in the Response and Resistance of Cancer Cells to Oxaliplatin. Cancers 2021, 13, 1752. [Google Scholar] [CrossRef] [PubMed]
- Kubánková, M.; Summers, P.A.; López-Duarte, I.; Kiryushko, D.; Kuimova, M.K. Microscopic Viscosity of Neuronal Plasma Membranes Measured Using Fluorescent Molecular Rotors: Effects of Oxidative Stress and Neuroprotection. ACS Appl. Mater. Interfaces 2019, 11, 36307–36315. [Google Scholar] [CrossRef] [PubMed]
- Uematsu, M.; Shimizu, T. Raman Microscopy-Based Quantification of the Physical Properties of Intracellular Lipids. Commun. Biol. 2021, 4, 1176. [Google Scholar] [CrossRef] [PubMed]
- Buech, G.; Herrmann, W.; Borchert, H.H. Measurement of Microviscosity in Vesicular Membranes by Electron Spin Resonance. Appl. Magn. Reson. 2008, 33, 269–283. [Google Scholar] [CrossRef]
- Lorén, N.; Hagman, J.; Jonasson, J.K.; Deschout, H.; Bernin, D.; Cella-Zanacchi, F.; Diaspro, A.; McNally, J.G.; Ameloot, M.; Smisdom, N.; et al. Fluorescence Recovery after Photobleaching in Material and Life Sciences: Putting Theory into Practice. Q Rev. Biophys. 2015, 48, 323–387. [Google Scholar] [CrossRef]
- Pužar Dominkuš, P.; Stenovec, M.; Sitar, S.; Lasič, E.; Zorec, R.; Plemenitaš, A.; Žagar, E.; Kreft, M.; Lenassi, M. PKH26 Labeling of Extracellular Vesicles: Characterization and Cellular Internalization of Contaminating PKH26 Nanoparticles. Biochim. Biophys. Acta Biomembr. 2018, 1860, 1350–1361. [Google Scholar] [CrossRef]
- Wallace, P.K.; Tario, J.D.; Fisher, J.L.; Wallace, S.S.; Ernstoff, M.S.; Muirhead, K.A. Tracking Antigen-Driven Responses by Flow Cytometry: Monitoring Proliferation by Dye Dilution. Cytom. Part A 2008, 73, 1019–1034. [Google Scholar] [CrossRef]
- Wang, J.; Wagner-Britz, L.; Bogdanova, A.; Ruppenthal, S.; Wiesen, K.; Kaiser, E.; Tian, Q.; Krause, E.; Bernhardt, I.; Lipp, P.; et al. Morphologically Homogeneous Red Blood Cells Present a Heterogeneous Response to Hormonal Stimulation. PLoS ONE 2013, 8, e67697. [Google Scholar] [CrossRef] [PubMed]
- Shirshin, E.A.; Yakimov, B.P.; Rodionov, S.A.; Omelyanenko, N.P.; Priezzhev, A.V.; Fadeev, V.V.; Lademann, J.; Darvin, M.E. Formation of Hemoglobin Photoproduct Is Responsible for Two-Photon and Single Photon-Excited Fluorescence of Red Blood Cells. Laser Phys. Lett. 2018, 15, 075604. [Google Scholar] [CrossRef]
- Prokhorov, V.V.; Perelygina, O.M.; Mal’tsev, E.I.; Vannikov, A.V. Crystalline Structure of Two-Dimensional Cyanine Dye J-Aggregates. Crystallogr. Rep. 2014, 59, 896–899. [Google Scholar] [CrossRef]
- Shinitzky, M.; Barenholz, Y. Dynamics of the Hydrocarbon Layer in Liposomes of Lecithin and Sphingomyelin Containing Dicetylphosphate. J. Biol. Chem. 1974, 249, 2652–2657. [Google Scholar] [CrossRef]
- Semenov, A.N.; Gvozdev, D.A.; Zlenko, D.V.; Protasova, E.A.; Khashimova, A.R.; Parshina, E.Y.; Baizhumanov, A.A.; Lotosh, N.Y.; Kim, E.E.; Kononevich, Y.N.; et al. Modulation of Membrane Microviscosity by Protein-Mediated Carotenoid Delivery as Revealed by Time-Resolved Fluorescence Anisotropy. Membranes 2022, 12, 905. [Google Scholar] [CrossRef]
- Palacios, L.E.; Wang, T. Egg-Yolk Lipid Fractionation and Lecithin Characterization. J. Am. Oil. Chem. Soc. 2005, 82, 571–578. [Google Scholar] [CrossRef]
- Hughes, B.D.; Pailthorpe, B.A.; White, L.R.; Sawyer, W.H. Extraction of Membrane Microviscosity from Translational and Rotational Diffusion Coefficients. Biophys. J. 1982, 37, 673–676. [Google Scholar] [CrossRef]
- Cooper, R.A. Influence of Increased Membrane Cholesterol on Membrane Fluidity and Cell Function in Human Red Blood Cells. J. Supramol. Struct. 1978, 8, 413–430. [Google Scholar] [CrossRef]
- Tsuda, K.; Kinoshita, Y.; Nishio, I.; Masuyama, Y. Adrenomedullin and Membrane Fluidity of Erythrocytes in Mild Essential Hypertension. J. Hypertens. 1999, 17, 201–210. [Google Scholar] [CrossRef]
- Ivanova, S.M.; Brazhe, N.A.; Luneva, O.G.; Yarlikova, Y.V.; Labetskaya, O.I.; Parshina, E.Y.; Baizhumanov, A.A.; Maksimov, G.V.; Morukov, B.V. Physicalchemical Properties of Plasma Membrane and Function of Erythrocytes of Cosmonauts after Long-Term Space Flight. Acta Astronaut. 2011, 68, 1517–1522. [Google Scholar] [CrossRef]
- Shimolina, L.E.; Gulin, A.A.; Paez-Perez, M.; López-Duarte, I.; Druzhkova, I.N.; Lukina, M.M.; Gubina, M.V.; Brooks, N.J.; Zagaynova, E.V.; Kuimova, M.K.; et al. Mapping Cisplatin-Induced Viscosity Alterations in Cancer Cells Using Molecular Rotor and Fluorescence Lifetime Imaging Microscopy. J. Biomed. Opt. 2020, 25, 126004_1–126004_16. [Google Scholar] [CrossRef] [PubMed]
- Baskurt, O.K.; Boynard, M.; Cokelet, G.C.; Connes, P.; Cooke, B.M.; Forconi, S.; Liao, F.; Hardeman, M.R.; Jung, F.; Meiselman, H.J.; et al. New Guidelines for Hemorheological Laboratory Techniques. Clin. Hemorheol. Microcirc. 2009, 42, 75–97. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kondratowicz, A.; Neunert, G.; Niezgoda, N.; Bryś, J.; Siger, A.; Rudzińska, M.; Lewandowicz, G. Egg Yolk Extracts as Potential Liposomes Shell Material: Composition Compared with Vesicles Characteristics. J. Food Sci. 2018, 83, 2527–2535. [Google Scholar] [CrossRef] [PubMed]
- Keith, A.D.; Snipes, W. Viscosity of Cellular Protoplasm. Science 1974, 183, 666–668. [Google Scholar] [CrossRef] [PubMed]
- Shinitzky, M.; Barenholz, Y. Fluidity Parameters of Lipid Regions Determined by Fluorescence Polarization. Biochim Biophys. Acta 1978, 515, 367–394. [Google Scholar] [CrossRef]
- Puchkov, E.O. Intracellular Viscosity: Methods of Measurements and Role in Metabolism. Biol. Membr. 2014, 31, 3–13. [Google Scholar] [CrossRef]
- Lakowicz, J.R. Principles of Fluorescence Spectroscopy, 2nd ed.; Springer Science+Business Media: New York, NY, USA, 1999. [Google Scholar]
- Maksimov, E.G.; Moldenhauer, M.; Shirshin, E.A.; Parshina, E.A.; Sluchanko, N.N.; Klementiev, K.E.; Tsoraev, G.V.; Tavraz, N.N.; Willoweit, M.; Schmitt, F.J.; et al. A Comparative Study of Three Signaling Forms of the Orange Carotenoid Protein. Photosynth. Res. 2016, 130, 389–401. [Google Scholar] [CrossRef]
- Szmacinski, H.; Jayaweera, R.; Cherek, H.; Lakowicz, J.R. Demonstration of an Associated Anisotropy Decay by Frequency-Domain Fluorometry. Biophys. Chem. 1987, 27, 233–241. [Google Scholar] [CrossRef]
- Barshtein, G.; Ben-Ami, R.; Yedgar, S. Role of Red Blood Cell Flow Behavior in Hemodynamics and Hemostasis. Expert Rev. Cardiovasc. Ther. 2007, 5, 743–752. [Google Scholar] [CrossRef]
- Forsyth, A.M.; Wan, J.; Ristenpart, W.D.; Stone, H.A. The Dynamic Behavior of Chemically “Stiffened” Red Blood Cells in Microchannel Flows. Microvasc. Res. 2010, 80, 37–43. [Google Scholar] [CrossRef]
| Temperature | 5 | 10 | 24 | 30 | 36 | 42 | |
|---|---|---|---|---|---|---|---|
| RBC | (ns) | 0.13 ± 0.03 | 0.14 ± 0.04 | 0.14 ± 0.03 | 0.11 ± 0.03 | 0.11 ± 0.04 | 0.16 ± 0.04 |
| (ns) | 8.5 ± 1.3 | 6.0 ± 0.8 | 4.3 ± 0.5 | 1.7 ± 0.4 | 1.5 ± 0.4 | 1.7 ± 0.3 | |
| (cP) | 490 ± 140 | 350 ± 110 | 310 ± 90 | 110 ± 30 | 97 ± 29 | 94 ± 28 | |
| Egg lecithin liposome | (ns) | 0.15 ± 0.02 | 0.16 ± 0.02 | 0.16 ± 0.03 | 0.17 ± 0.02 | 0.16 ± 0.02 | 0.17 ± 0.05 |
| (ns) | 46 ± 7 | 35 ± 5 | 25 ± 3 | 11 ± 3 | 8 ± 2 | 6 ± 2 | |
| (cP) | 2740 ± 420 | 2110 ± 290 | 1610 ± 180 | 690 ± 190 | 560 ± 150 | 450 ± 150 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Semenov, A.N.; Gvozdev, D.A.; Moysenovich, A.M.; Zlenko, D.V.; Parshina, E.Y.; Baizhumanov, A.A.; Budylin, G.S.; Maksimov, E.G. Probing Red Blood Cell Membrane Microviscosity Using Fluorescence Anisotropy Decay Curves of the Lipophilic Dye PKH26. Int. J. Mol. Sci. 2022, 23, 15767. https://doi.org/10.3390/ijms232415767
Semenov AN, Gvozdev DA, Moysenovich AM, Zlenko DV, Parshina EY, Baizhumanov AA, Budylin GS, Maksimov EG. Probing Red Blood Cell Membrane Microviscosity Using Fluorescence Anisotropy Decay Curves of the Lipophilic Dye PKH26. International Journal of Molecular Sciences. 2022; 23(24):15767. https://doi.org/10.3390/ijms232415767
Chicago/Turabian StyleSemenov, Alexey N., Daniil A. Gvozdev, Anastasia M. Moysenovich, Dmitry V. Zlenko, Evgenia Yu. Parshina, Adil A. Baizhumanov, Gleb S. Budylin, and Eugene G. Maksimov. 2022. "Probing Red Blood Cell Membrane Microviscosity Using Fluorescence Anisotropy Decay Curves of the Lipophilic Dye PKH26" International Journal of Molecular Sciences 23, no. 24: 15767. https://doi.org/10.3390/ijms232415767
APA StyleSemenov, A. N., Gvozdev, D. A., Moysenovich, A. M., Zlenko, D. V., Parshina, E. Y., Baizhumanov, A. A., Budylin, G. S., & Maksimov, E. G. (2022). Probing Red Blood Cell Membrane Microviscosity Using Fluorescence Anisotropy Decay Curves of the Lipophilic Dye PKH26. International Journal of Molecular Sciences, 23(24), 15767. https://doi.org/10.3390/ijms232415767







