Correlated Electronic Properties of a Graphene Nanoflake: Coronene
Abstract
:1. Introduction
2. Methodology
2.1. Model Hamiltonian
2.2. The DMRG Technique
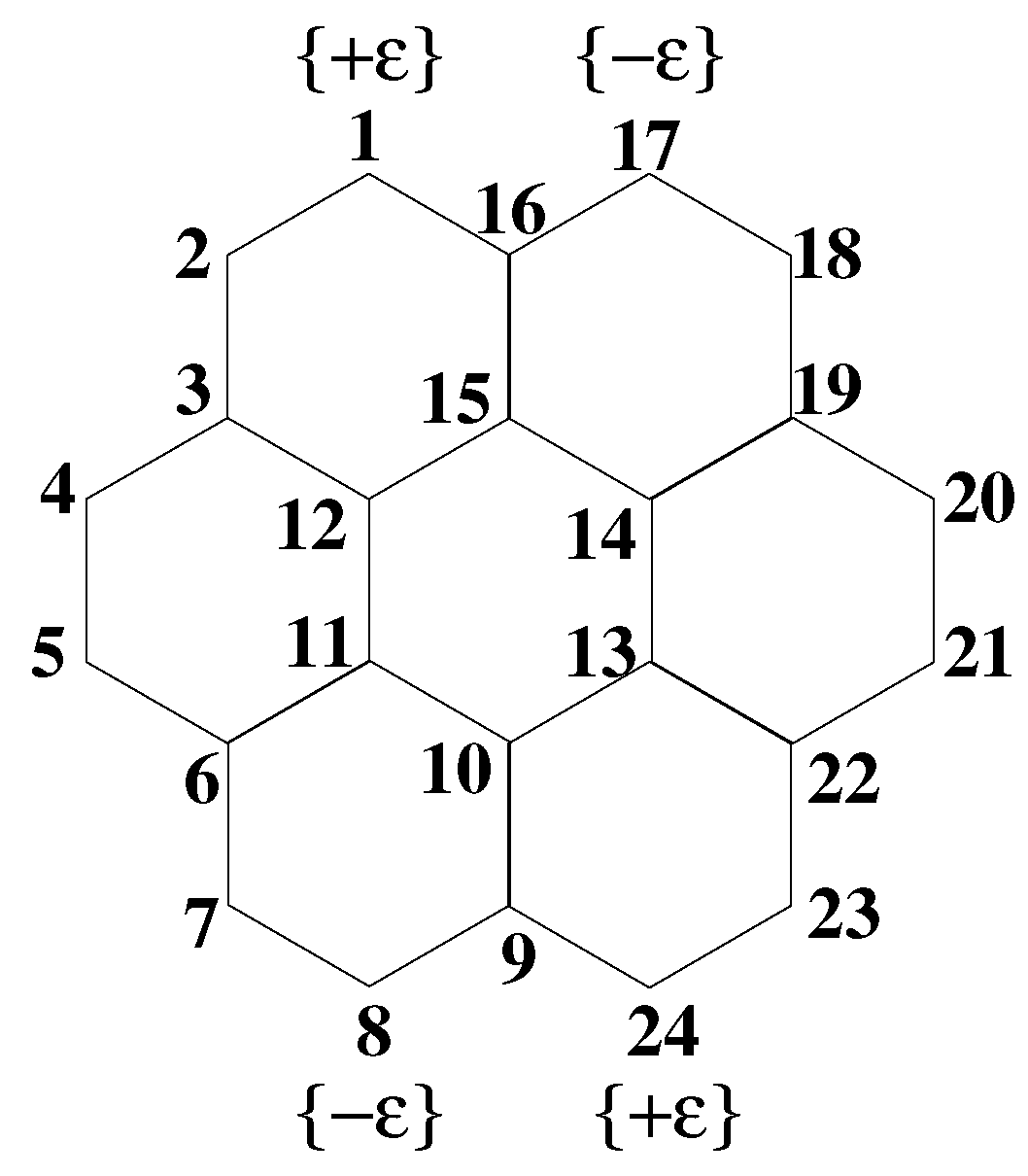
2.3. Symmetries of the Hamiltonian
2.4. Two-Photon Absorption Cross-Section
3. Computational Results
3.1. Correlation Strength and Ordering of Excited States
3.2. TPA Cross-Section
4. Discussion and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zhao, H.; Mazumdar, S. Electron-electron interaction effects on the optical excitations of semiconducting single-walled carbon nanotubes. Phys. Rev. Lett. 2004, 93, 157402. [Google Scholar] [CrossRef] [PubMed]
- Hudson, B.S.; Kohler, B.E.; Schulten, K. Excited States; Academic Press: New York, NY, USA, 1982; Volume 6, p. 1. [Google Scholar]
- Soos, Z.G.; Ramasesha, S. Valence-bond theory of linear hubbard and Pariser–Parr–Pople models. Phys. Rev. B 1984, 29, 5410–5422. [Google Scholar] [CrossRef]
- Tavan, P.; Schulten, K. The 21Ag−11Bu energy gap in the polyenes: An extended configuration interaction study. J. Chem. Phys. 1979, 70, 5407–5414. [Google Scholar] [CrossRef]
- Tavan, P.; Schulten, K. Correlation effects in the spectra of polyacenes. J. Chem. Phys. 1979, 70, 5414–5421. [Google Scholar] [CrossRef]
- Tavan, P.; Schulten, K. Electronic excitations in finite and infinite polyenes. Phys. Rev. B 1987, 36, 4337–4358. [Google Scholar] [CrossRef]
- Tavan, P.; Schulten, K. The low-lying electronic excitations in long polyenes: A PPP-MRD-CI study. J. Chem. Phys. 1986, 85, 6602–6609. [Google Scholar] [CrossRef]
- Soos, Z.G.; Ramasesha, S.; Galvao, D.S. Band to correlated crossover in alternating hubbard and Pariser-Parr-Pople chains: Nature of the lowest singlet excitation of conjugated polymers. Phys. Rev. Lett. 1993, 71, 1609–1612. [Google Scholar] [CrossRef] [PubMed]
- Baeriswyl, D.; Campbell, D.K.; Mazumdar, S. Conjugated Conducting Polymers; Springer Series in Solid-State Sciences; Springer: Berlin, Germany, 1992; Volume 102. [Google Scholar]
- Shuai, Z.; Beljonne, D.; Silbey, R.J.; Brédas, J.L. Singlet and triplet exciton formation rates in conjugated polymer light-emitting diodes. Phys. Rev. Lett. 2000, 84, 131–134. [Google Scholar] [CrossRef]
- Yamamoto, T.; Noguchi, T.; Watanabe, K. Edge-state signature in optical absorption of nanographenes: Tight-binding method and time-dependent density functional theory calculations. Phys. Rev. B 2006, 74, 121409. [Google Scholar] [CrossRef]
- Wong, B.M. Optoelectronic properties of carbon nanorings: excitonic effects from time-dependent density functional theory. J. Phys. Chem. C 2009, 113, 21921–21927. [Google Scholar] [CrossRef]
- Malloci, G.; Cappellini, G.; Mulas, G.; Mattoni, A. Electronic and optical properties of families of polycyclic aromatic hydrocarbons: A systematic (time-dependent) density functional theory study. Chem. Phys. 2011, 384, 19–27. [Google Scholar] [CrossRef] [Green Version]
- Spataru, C.D.; Ismail-Beigi, S.; Benedict, L.X.; Louie, S.G. Excitonic effects and optical spectra of single-walled carbon nanotubes. Phys. Rev. Lett. 2004, 92, 077402. [Google Scholar] [CrossRef] [PubMed]
- Chang, E.; Bussi, G.; Ruini, A.; Molinari, E. Excitons in carbon nanotubes: An ab initio symmetry-based approach. Phys. Rev. Lett. 2004, 92, 196401. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Deslippe, J.; Park, C.H.; Cohen, M.L.; Louie, S.G. Excitonic effects on the optical response of graphene and bilayer graphene. Phys. Rev. Lett. 2009, 103, 186802. [Google Scholar] [CrossRef] [PubMed]
- Son, Y.W.; Cohen, M.L.; Louie, S.G. Half-metallic graphene nanoribbons. Nature 2006, 444, 347–349. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schreiber, M.; Silva-Junior, M.R.; Sauer, S.P.A.; Thiel, W. Benchmarks for electronically excited states: CASPT2, CC2, CCSD, and CC3. J. Chem. Phys. 2008, 128, 134110–134134. [Google Scholar] [CrossRef]
- Silva-Junior, M.R.; Schreiber, M.; Sauer, S.P.A.; Thiel, W. Benchmarks for electronically excited states: Basis set effects on CASPT2 results. J. Chem. Phys. 2010, 133, 174318–174330. [Google Scholar] [CrossRef]
- Starcke, J.H.; Wormit, M.; Schirmer, J.; Dreuw, A. How much double excitation character do the lowest excited states of linear polyenes have? Chem. Phys. 2006, 329, 39–49. [Google Scholar] [CrossRef]
- Knippenberg, S.; Rehn, D.R.; Wormit, M.; Starcke, J.H.; Rusakova, I.L.; Trofimov, A.B.; Dreuw, A. Calculations of nonlinear response properties using the intermediate state representation and the algebraic-diagrammatic construction polarization propagator approach: Two-photon absorption spectra. J. Chem. Phys. 2012, 136, 064107–064121. [Google Scholar] [CrossRef]
- Krauter, C.M.; Pernpointner, M.; Dreuw, A. Application of the scaled-opposite-spin approximation to the algebraic diagrammatic construction schemes of second order. J. Chem. Phys. 2013, 138, 044107–044118. [Google Scholar] [CrossRef]
- Aryanpour, K.; Roberts, A.; Sandhu, A.; Rathore, R.; Shukla, A.; Mazumdar, S. Subgap two-photon states in polycyclic aromatic hydrocarbons: Evidence for strong electron correlations. J. Phys. Chem. C 2014, 118, 3331–3339. [Google Scholar] [CrossRef]
- Aryanpour, K.; Shukla, A.; Mazumdar, S. Electron correlations and two-photon states in polycyclic aromatic hydrocarbon molecules: A peculiar role of geometry. J. Chem. Phys. 2014, 140, 104301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- White, S.R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 1992, 69, 2863–2866. [Google Scholar] [CrossRef] [PubMed]
- White, S.R. Density-matrix algorithms for quantum renormalization groups. Phys. Rev. B 1993, 48, 10345–10356. [Google Scholar] [CrossRef]
- Schollwöck, U. The density-matrix renormalization group. Rev. Mod. Phys. 2005, 77, 259–315. [Google Scholar] [CrossRef] [Green Version]
- Hallberg, K.A. New trends in density matrix renormalization. Adv. Phys. 2006, 55, 477–526. [Google Scholar] [CrossRef] [Green Version]
- Ramasesha, S.; Pati, S.K.; Shuai, Z.; Brédas, J.L. Advances in Quantum Chemistry; Academic Press: San Diego, CA, USA, 2000; Volume 38, pp. 121–215. [Google Scholar]
- Wallace, P.R. The band theory of graphite. Phys. Rev. 1947, 71, 622–634. [Google Scholar] [CrossRef]
- Min, H.; Sahu, B.; Banerjee, S.K.; MacDonald, A.H. Ab initio theory of gate induced gaps in graphene bilayers. Phys. Rev. B 2007, 75, 155115. [Google Scholar] [CrossRef]
- Yu, E.K.; Stewart, D.A.; Tiwary, S. Ab initio study of polarizability and induced charge densities in multilayer graphene films. Phys. Rev. B 2008, 77, 195406. [Google Scholar] [CrossRef]
- Gava, P.; Lazzeri, M.; Saitta, A.M.; Mauri, F. Ab initio study of gap opening and screening effects in gated bilayer graphene. Phys. Rev. B 2009, 79, 165431. [Google Scholar] [CrossRef]
- Giovannetti, G.; Khomyakov, P.A.; Brocks, G.; Kelly, P.J.; van den Brink, J. Substrate-induced band gap in graphene on hexagonal boron nitride: Ab initio density functional calculations. Phys. Rev. B 2007, 76, 073103. [Google Scholar] [CrossRef]
- Ni, Z.H.; Yu, T.; Lu, Y.H.; Wang, Y.Y.; Feng, Y.P.; Shen, Z.X. Uniaxial strain on graphene: Raman spectroscopy study and band-gap opening. ACS Nano 2008, 2, 2301–2305. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro, R.M.; Peres, N.M.R.; Coutinho, J.; Briddon, P.R. Inducing energy gaps in monolayer and bilayer graphene: Local density approximation calculations. Phys. Rev. B 2008, 78, 075442. [Google Scholar] [CrossRef]
- Latil, S.; Henrard, L. Charge carriers in few-layer graphene films. Phys. Rev. Lett. 2006, 97, 036803. [Google Scholar] [CrossRef] [PubMed]
- Feng, C.; Lin, C.S.; Fan, W.; Zhang, R.Q.; Van Hove, M.A. Stacking of polycyclic aromatic hydrocarbons as prototype for graphene multilayers, studied using density functional theory augmented with a dispersion term. J. Chem. Phys. 2009, 131, 194702. [Google Scholar] [CrossRef] [PubMed]
- Martins, T.B.; Miwa, R.H.; da Silva, A.J.R.; Fazzio, A. Electronic and transport properties of boron-doped graphene nanoribbons. Phys. Rev. Lett. 2007, 98, 196803. [Google Scholar] [CrossRef] [PubMed]
- Xu, B.; Lu, Y.H.; Feng, Y.P.; Lin, J.Y. Density functional theory study of bn-doped graphene superlattice: Role of geometrical shape and size. J. Appl. Phys. 2010, 108, 073711. [Google Scholar] [CrossRef]
- Sanyal, S.; Mannaa, A.K.; Pati, S.K. BN-decorated graphene nanoflakes with tunable opto-electronic and charge transport properties. J. Mater. Chem. C 2014, 2, 2918–2928. [Google Scholar] [CrossRef]
- Fürst, J.A.; Pedersen, T.G.; Brandbyge, M.; Jauho, A.P. Density functional study of graphene antidot lattices: Roles of geometrical relaxation and spin. Phys. Rev. B 2009, 80, 115117. [Google Scholar] [CrossRef]
- Tachikawa, H.; Nagoya, Y.; Kawabata, H. A density functional theory study of ground and low-lying excited electronic states in defective graphenes. J. Chem. Theory Comput. 2009, 5, 2101–2107. [Google Scholar] [CrossRef]
- Barone, V.; Hod, O.; Peralta, J.E.; Scuseria, G.E. Accurate prediction of the electronic properties of low-dimensional graphene derivatives using a screened hybrid density functional. Acc. Chem. Res. 2011, 44, 269–279. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.L.; Meng, S.; Kaxiras, E. Graphene nanoflakes with large spin. Nano Lett. 2008, 8, 241–245. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.L.; Yazyev, O.V.; Meng, S.; Kaxiras, E. Topological frustration in graphene nanoflakes: Magnetic order and spin logic devices. Phys. Rev. Lett. 2009, 102, 157201. [Google Scholar] [CrossRef] [PubMed]
- Ezawa, M. Metallic graphene nanodisks: electronic and magnetic properties. Phys. Rev. B 2007, 76, 245415. [Google Scholar] [CrossRef]
- Fernańdez-Rossier, J.; Palacios, J.J. Magnetism in graphene nanoislands. Phys. Rev. Lett. 2007, 99, 177204. [Google Scholar] [CrossRef] [PubMed]
- Sheng, W.; Sun, M.; Zhou, A. Violation of hund’s rule and quenching of long-range electron-electron interactions in graphene nanoflakes. Phys. Rev. B 2013, 88, 085432. [Google Scholar] [CrossRef]
- Sheng, W.; Sun, M.; Zhou, A.; Xu, S.J. Substrate effects on quasiparticles and excitons in graphene nanoflakes. Appl. Phys. Lett. 2013, 103, 143109. [Google Scholar] [CrossRef] [Green Version]
- Zhou, A.; Sheng, W.; Xu, S.J. Electric field driven magnetic phase transition in graphene nanoflakes. Appl. Phys. Lett. 2013, 103, 133103. [Google Scholar] [CrossRef] [Green Version]
- Dutta, S.; Pati, S.K. Half-metallicity in undoped and boron doped graphene nanoribbons in the presence of semilocal exchange-correlation interactions. J. Phys. Chem. B 2008, 112, 1333–1335. [Google Scholar] [CrossRef]
- Goli, V.M.L.D.P.; Prodhan, S.; Mazumdar, S.; Ramasesha, S. Correlated electronic properties of some graphene nanoribbons: A DMRG study. Phys. Rev. B 2016, 94, 035139. [Google Scholar] [CrossRef]
- Cocchi, C.; Prezzi, D.; Ruini, A.; Caldas, M.J.; Molinari, E. Anisotropy and size effects on the optical spectra of polycyclic aromatic hydrocarbons. J. Phys. Chem. A 2014, 118, 6507–6513. [Google Scholar] [CrossRef] [PubMed]
- Olivares-Amaya, R.; Hu, W.; Nakatani, N.; Sharma, S.; Yang, J.; Chan, G.K.L. The ab-initio density matrix renormalization group in practice. J. Chem. Phys. 2015, 142, 034102. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Raghu, C.; Pati, Y.A.; Ramasesha, S. Density-matrix renormalization-group study of low-lying excitations of polyacene within a Pariser–Parr–Pople model. Phys. Rev. B 2002, 66, 035116. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Ramasesha, S. Study of linear and nonlinear optical properties of dendrimers using density matrix renormalization group method. J. Chem. Phys. 2009, 131, 074111. [Google Scholar] [CrossRef] [PubMed]
- Thomas, S.; Pati, Y.A.; Ramasesha, S. Linear and nonlinear optical properties of expanded porphyrins: A DMRG study. J. Phys. Chem. A 2013, 117, 7804–7809. [Google Scholar] [CrossRef]
- Das, M. Low-lying excited states in armchair polyacene within Pariser–Parr–Pople model: A density matrix renormalization group study. J. Chem. Phys. 2014, 140, 124317. [Google Scholar] [CrossRef] [PubMed]
- Pariser, R.; Parr, R.G. A semi-empirical theory of the electronic spectra and electronic structure of complex unsaturated molecules. I. J. Chem. Phys. 1953, 21, 466–471. [Google Scholar] [CrossRef]
- Pople, J.A. Electron interaction in unsaturated hydrocarbons. Trans. Faraday Soc. 1953, 49, 1375–1385. [Google Scholar] [CrossRef]
- Ohno, K. Some remarks on the Pariser–Parr–Pople method. Theor. Chim. Acta 1964, 2, 219–227. [Google Scholar] [CrossRef]
- Klopman, G. A semiempirical treatment of molecular structures. II. Molecular terms and application to diatomic molecules. J. Am. Chem. Soc. 1964, 86, 4550–4557. [Google Scholar] [CrossRef]
- Salem, L. The Molecular Orbital Theory of Conjugated Systems; W. A. Benjamin, Inc.: New York, NY, USA, 1966; p. 420. [Google Scholar]
- Suzuki, H. Electronic Absorption Spectra and Geometry of Organic Molecules; Academic Press: New York, NY, USA, 1967. [Google Scholar]
- White, S.R.; Martin, R.L. Ab initio quantum chemistry using the density matrix renormalization group. J. Chem. Phys. 1999, 110, 4127–4130. [Google Scholar] [CrossRef] [Green Version]
- Chan, G.K.L.; Head-Gordon, M. Highly correlated calculations with a polynomial cost algorithm: A study of the density matrix renormalization group. J. Chem. Phys. 2002, 116, 4462–4476. [Google Scholar] [CrossRef] [Green Version]
- Chan, G.K.L.; Head-Gordon, M. Exact solution (within a triple-zeta, double polarization basis set) of the electronic Schrödinger equation for water. J. Chem. Phys. 2003, 118, 8551–8554. [Google Scholar] [CrossRef]
- Sahoo, S.; Goli, V.M.L.D.P.; Ramasesha, S.; Sen, D. Exact entanglement studies of strongly correlated systems: role of long-range interactions and symmetries of the system. J. Phys. Condens. Matter 2012, 24, 115601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Prodhan, S.; Ramasesha, S. Symmetrized density matrix renormalization group algorithm for low-lying excited states of conjugated carbon systems: Application to 1,12-benzoperylene and polychrysene. Phys. Rev. B 2018, 97, 195125. [Google Scholar] [CrossRef] [Green Version]
- Ramasesha, S. A new algorithm for solving large inhomogeneous linear system of algebraic equations. J. Comput. Chem. 1990, 11, 545–547. [Google Scholar] [CrossRef]
- Luo, Y.; Norman, P.; Macak, P.; Ågren, H. Solvent-induced two-photon absorption of a push-pull molecule. J. Phys. Chem. A 2000, 104, 4718–4722. [Google Scholar] [CrossRef]
- Hudson, B.S.; Kohler, B.E. A low-lying weak transition in the polyene α,ω-diphenyloctatetraene. Chem. Phys. Lett. 1972, 14, 299–304. [Google Scholar] [CrossRef]
- Schulten, K.; Karplus, M. On the origin of a low-lying forbidden transition in polyenes and related molecules. Chem. Phys. Lett. 1972, 14, 305–309. [Google Scholar] [CrossRef]
- Ramasesha, S.; Soos, Z.G. Correlated states in linear polyenes, radicals, and ions: Exact PPP transition moments and spin densities. J. Chem. Phys. 1984, 80, 3278–3287. [Google Scholar] [CrossRef]
- Clar, E. Polycyclic Hydrocarbons; Springer: Berlin/Heidelberg, Germany, 1964; Volume 2. [Google Scholar]
- Clar, E.; Schmidt, W. Correlations between photoelectron and ultraviolet absorption spectra of polycyclic hydrocarbons. The perylene, coronene and bisanthene series. Tetrahedron 1977, 33, 2093–2097. [Google Scholar] [CrossRef]
- Patterson, J.W. Theultraviolet absorption spectra of coronene. J. Am. Chem. Soc. 1942, 64, 1485–1486. [Google Scholar] [CrossRef]
- Tanaka, J. The electronic spectra of pyrene, chrysene, azulene, coronene and tetracene crystals. Bull. Chem. Soc. Jpn. 1965, 38, 86–103. [Google Scholar] [CrossRef]
- Stephens, P.J.; Schatz, P.N.; Ritchie, A.B.; McCaffery, A.J. Magnetic circular dichroism of benzene, triphenylene, and coronene. J. Chem. Phys. 1968, 48, 132–138. [Google Scholar] [CrossRef]
- Abouaf, R.; Díaz-Tendero, S. Electron Energy Loss Spectroscopy and Anion Formation in Gas Phase Coronene. Phys. Chem. Chem. Phys. 2009, 11, 5686–5694. [Google Scholar] [CrossRef] [PubMed]
- Aihara, J.; Ohno, K.; Inokuchi, H. Absorption spectra of gaseous benzo[g,h,i]perylene and coronene. Bull. Chem. Soc. Jpn. 1970, 43, 2435–2439. [Google Scholar] [CrossRef]
- Ohno, K.; Kajiwara, T.; Inokuchi, H. Vibrational analysis of electronic transition bands of coronene. Bull. Chem. Soc. Jpn. 1972, 45, 996–1004. [Google Scholar] [CrossRef]
- Ehrenfreund, P.; d’Hendecourt, L.; Verstraete, L.; Leger, A.; Schmidt, W.; Defourneau, D. Search for the 4430 Å DIB in the spectra of coronene cation and neutral ovalene. Astron. Astrophys. 1992, 259, 257–264. [Google Scholar]
- Nijegorodov, N.; Mabbs, R.; Downey, W. Evolution of absorption, fluorescence, laser and chemical properties in the series of compounds perylene, benzo(ghi)perylene and coronene. Spectrochim. Acta Part A 2001, 57, 2673–2685. [Google Scholar] [CrossRef]
- Kropp, J.L.; Dawson, W.R. Radiationless deactivation of triplet coronene in plastics. J. Phys. Chem. 1967, 71, 4499–4506. [Google Scholar] [CrossRef]
- Dutta, A.K. Spectroscopic studies of nonamphiphilic coronene assembled in langmuir-blodgett films: Aggregation-induced reabsorption effects. Langmuir 1998, 14, 3036–3040. [Google Scholar] [CrossRef]


| Nature of the State | Hückel | Symmetrized DMRG |
|---|---|---|
| Ground state | ||
| Optical state |
| Coronene | ||||
|---|---|---|---|---|
| Nature of the State | State | Energy Gap (eV) | ||
| Two-photon | ||||
| Optical | ||||
| Triplet | ||||
| Dark states | ||||
| Substituted Coronene | ||||
|---|---|---|---|---|
| Nature of the State | State | Energy Gap (eV) | ||
| Two-photon | ||||
| Optical | ||||
| Triplet | ||||
| Two-Photon State | |||||
|---|---|---|---|---|---|
| Coronene | |||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Prodhan, S.; Mazumdar, S.; Ramasesha, S. Correlated Electronic Properties of a Graphene Nanoflake: Coronene. Molecules 2019, 24, 730. https://doi.org/10.3390/molecules24040730
Prodhan S, Mazumdar S, Ramasesha S. Correlated Electronic Properties of a Graphene Nanoflake: Coronene. Molecules. 2019; 24(4):730. https://doi.org/10.3390/molecules24040730
Chicago/Turabian StyleProdhan, Suryoday, Sumit Mazumdar, and S. Ramasesha. 2019. "Correlated Electronic Properties of a Graphene Nanoflake: Coronene" Molecules 24, no. 4: 730. https://doi.org/10.3390/molecules24040730





