Mesoscopic Kinetic Approach of Nonequilibrium Effects for Shock Waves
Abstract
:1. Introduction
2. Mesoscopic LBM Nonequilibrium Moments
2.1. Compressible LBM Model
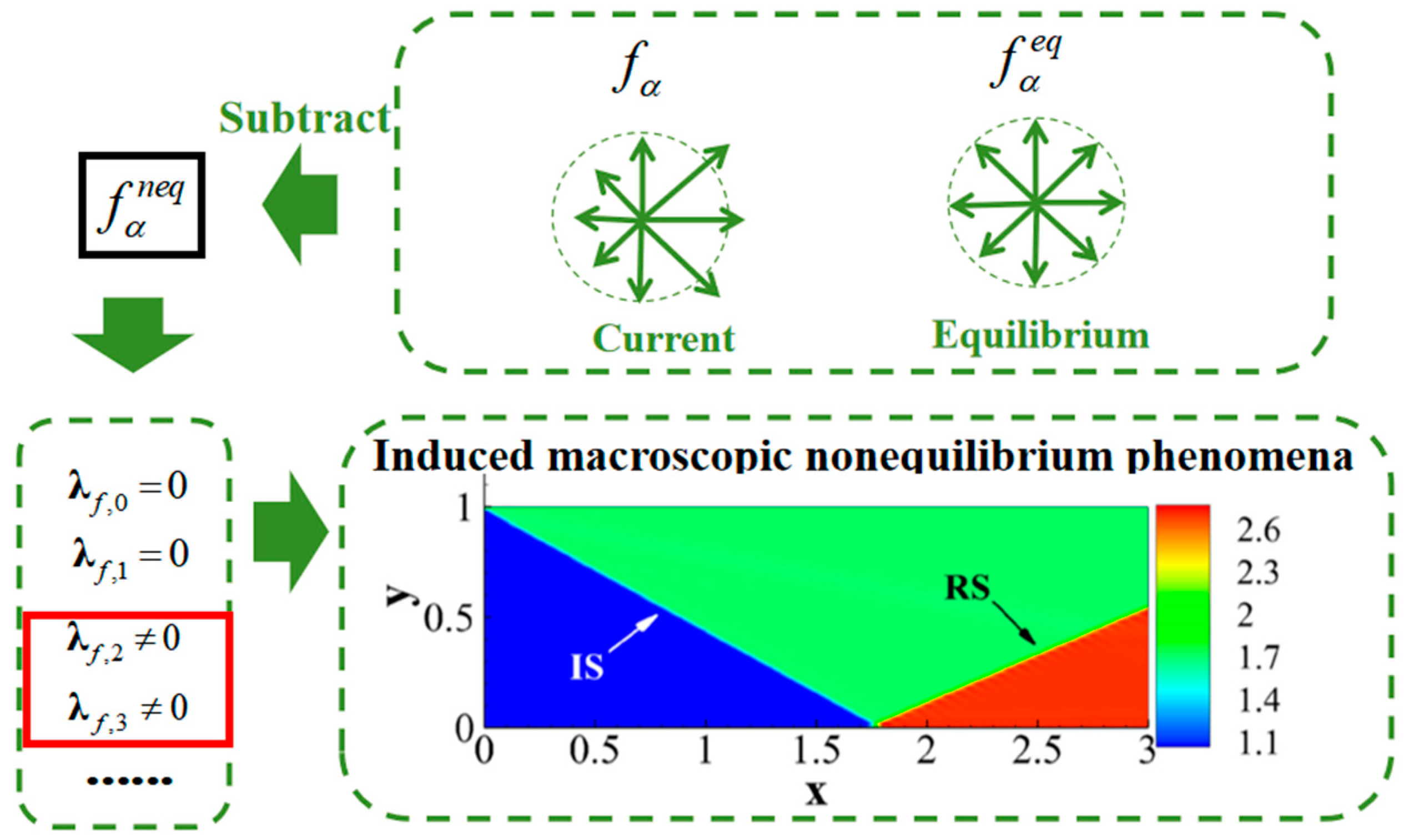
2.2. Nonequilibrium Effects
3. Research Methods for Nonequilibrium Effects
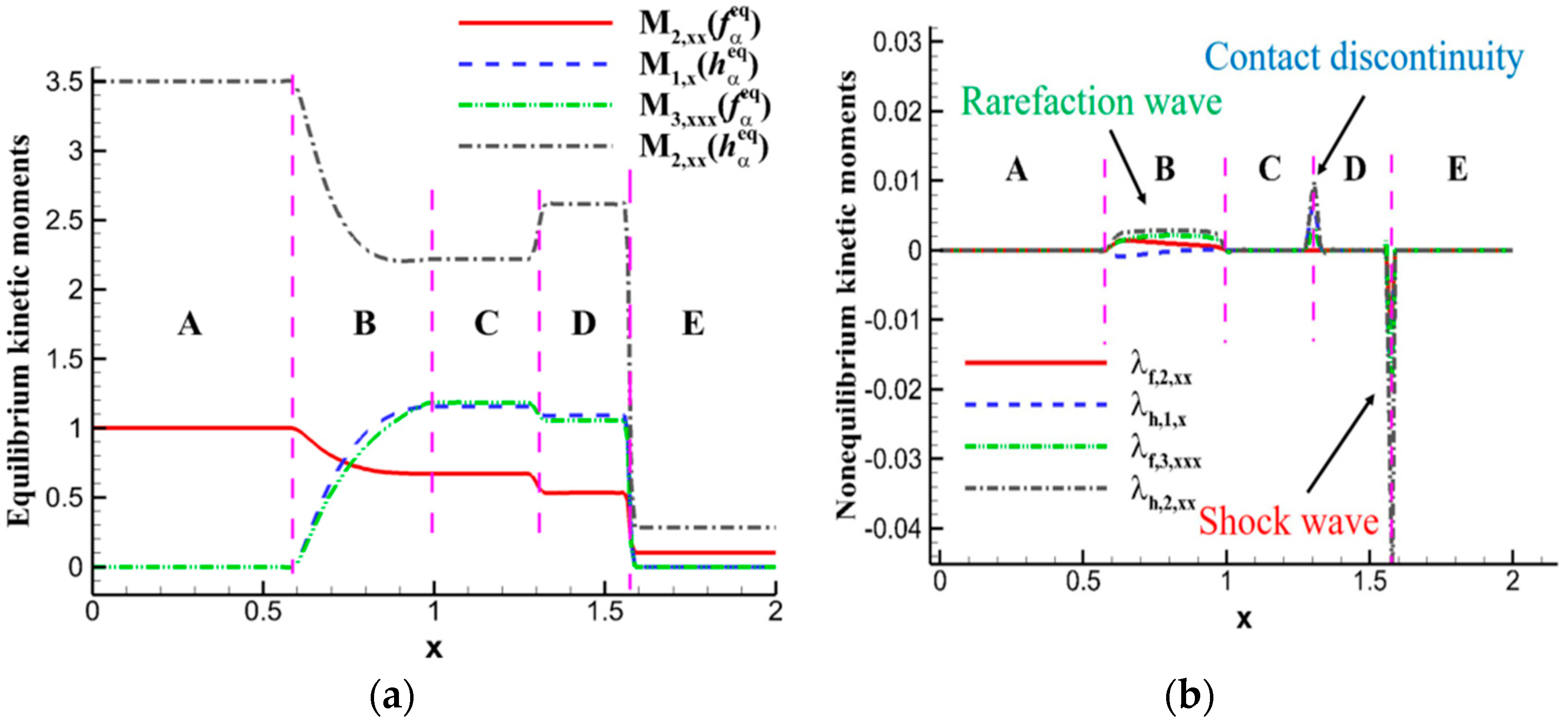
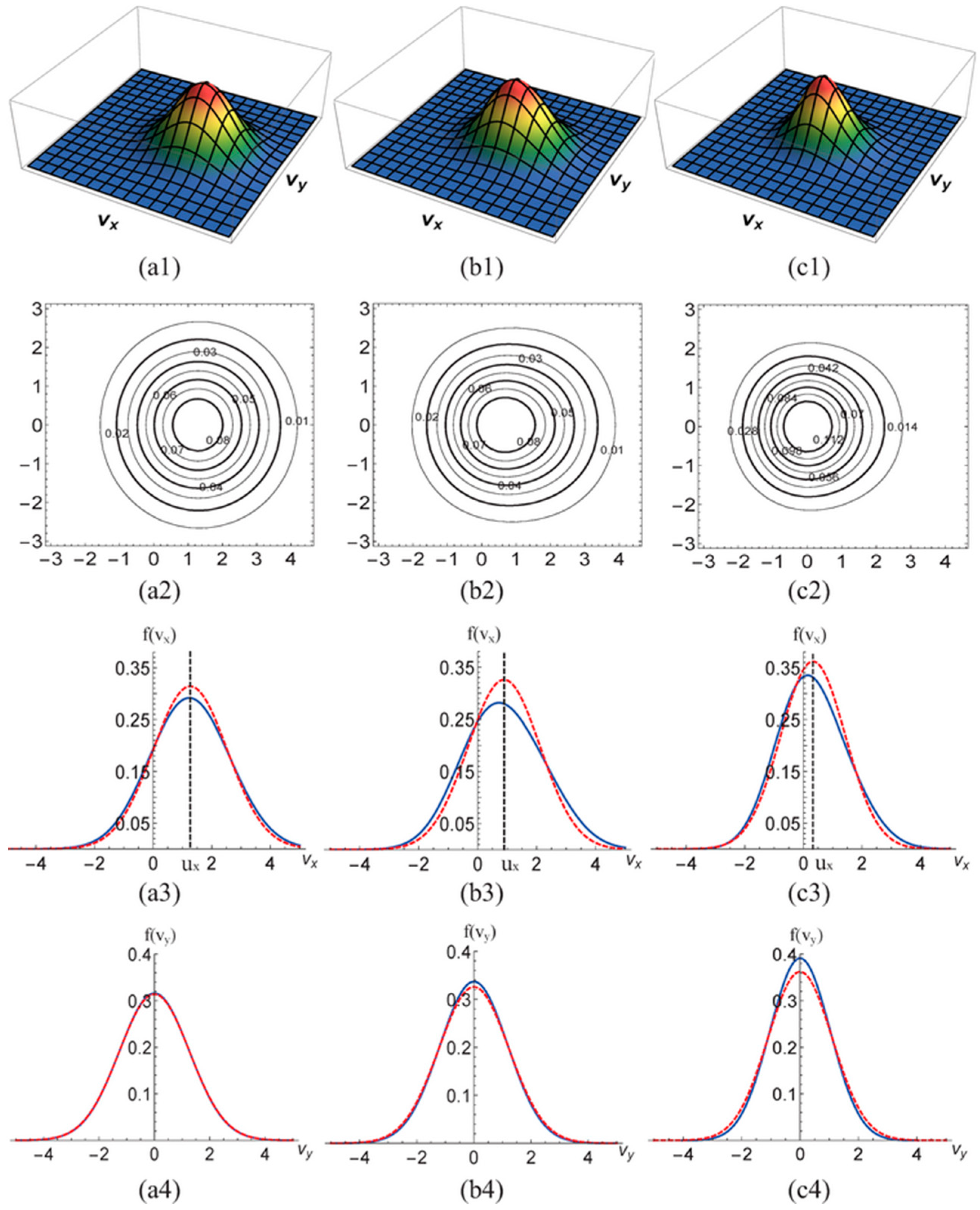
3.1. Equilibrium Kinetic Moment
- The discussion here focuses on physical meanings rather than precise definitions.
- The units of each equilibrium moment are provided in the tables to aid in understanding the physical meanings of higher-order moments.
- A state variable with motion multipliers is referred to as an -order state. Higher-order states contain stronger information about material “motion action”.
- represents the -directional component of the momentum density for the component in the equilibrium state, and is the -directional component of the translational kinetic energy density for the component in the equilibrium state.
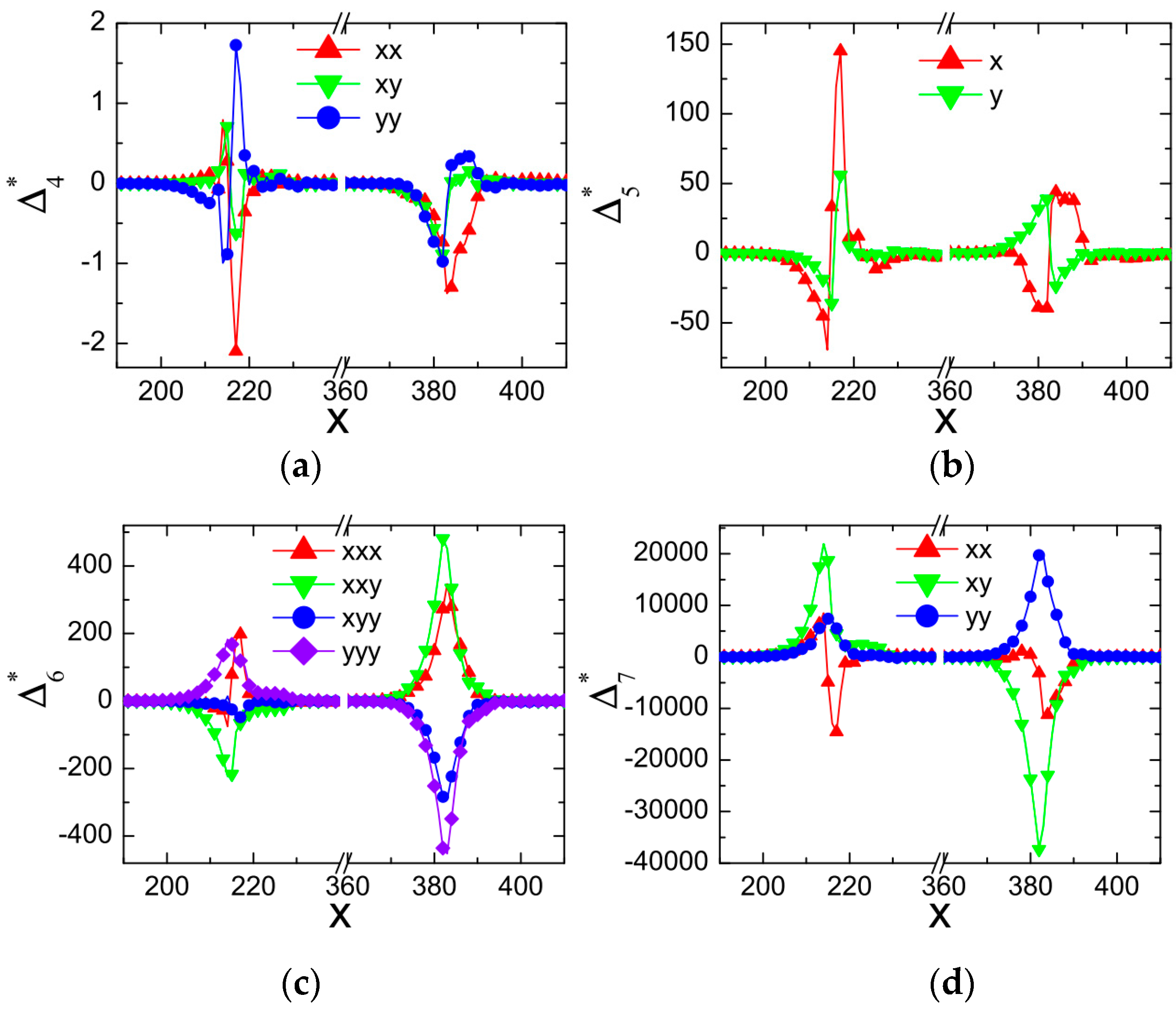
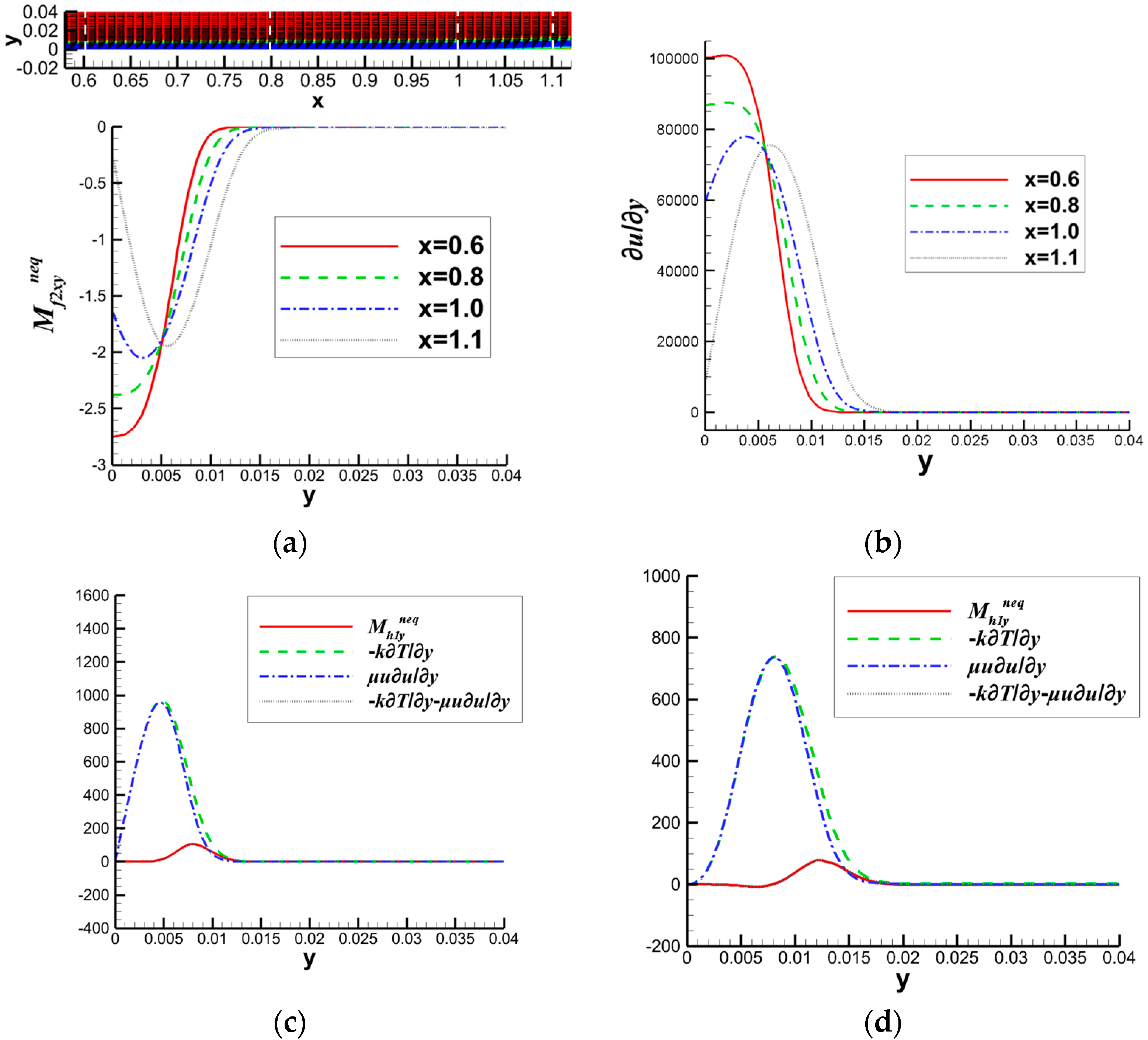
3.2. Nonequilibrium Kinetic Moment
- The current-state kinetic moments remain positive, indicating a weaker motion compared to the kinetic moments of the local equilibrium state.
- The current-state kinetic moments are negative, with an absolute value smaller than those of the local equilibrium kinetic moments. In this case, not only is the motion weaker in the former compared to the latter, but their directions of motion also differ.
- The current-state kinetic moments are negative, with an absolute value greater than those of the local equilibrium kinetic moments. In this scenario, the motion in the former is stronger than in the latter, and their directions of motion are distinct.
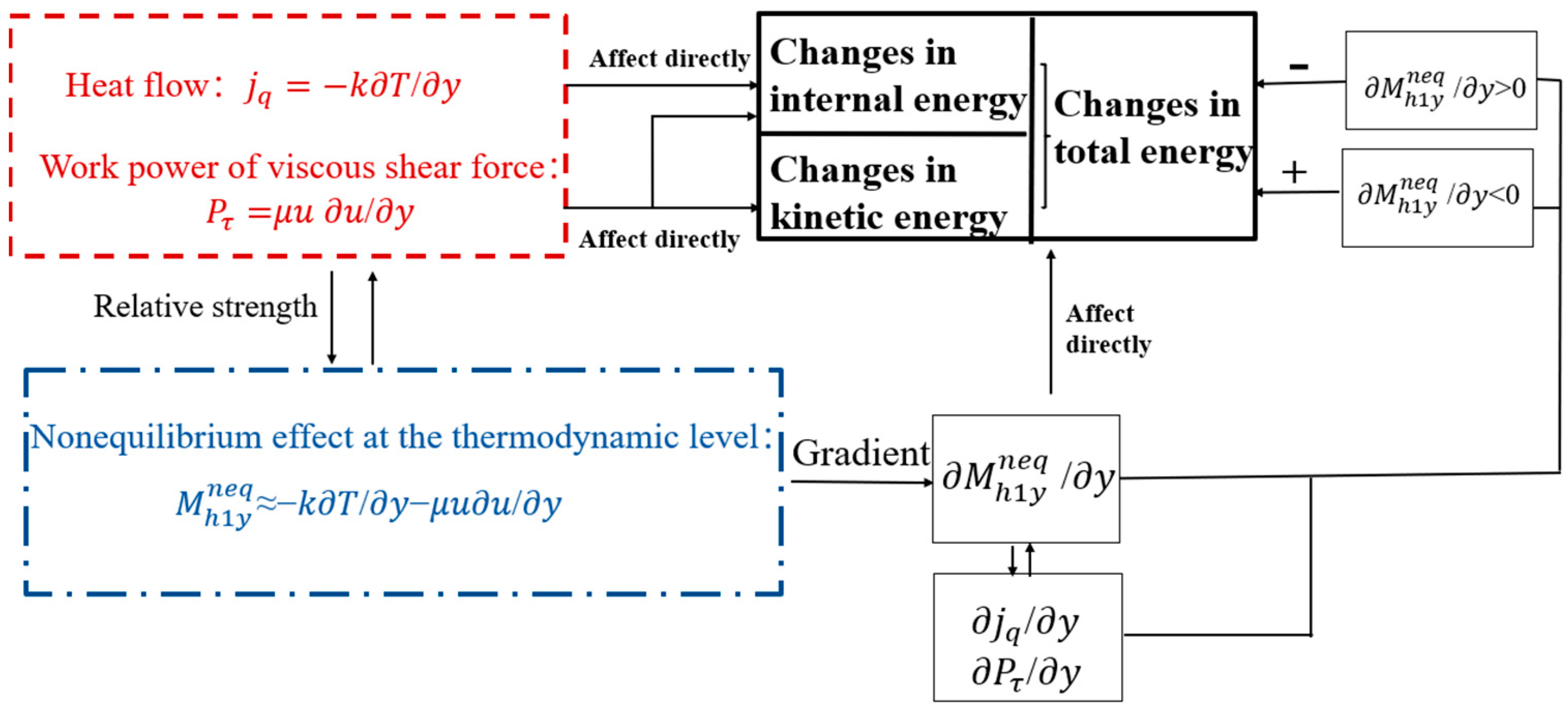
- Nonequilibrium effects reflect the extent to which molecular motion deviates from local equilibrium states, mapped onto the corresponding physical levels of mass and total energy. This deviation can be understood as a kind of “instability” of the local system relative to equilibrium states.
- The sign of nonequilibrium effects indicates the direction in which molecular motion deviates from its local equilibrium state.
- The magnitude of nonequilibrium effects reflects the local “instability” level of molecular motion at the corresponding hierarchy.
- With this perspective, nonequilibrium behavior can be interpreted in a more intuitive manner. The nonequilibrium effects research method discussed in this paper is not limited to specific LBM models. At the Navier-Stokes level, any model that satisfies the moment relations in Equations (2) and (3) can be applied.
4. Nonequilibrium Effects Study on Shock-Related Problems
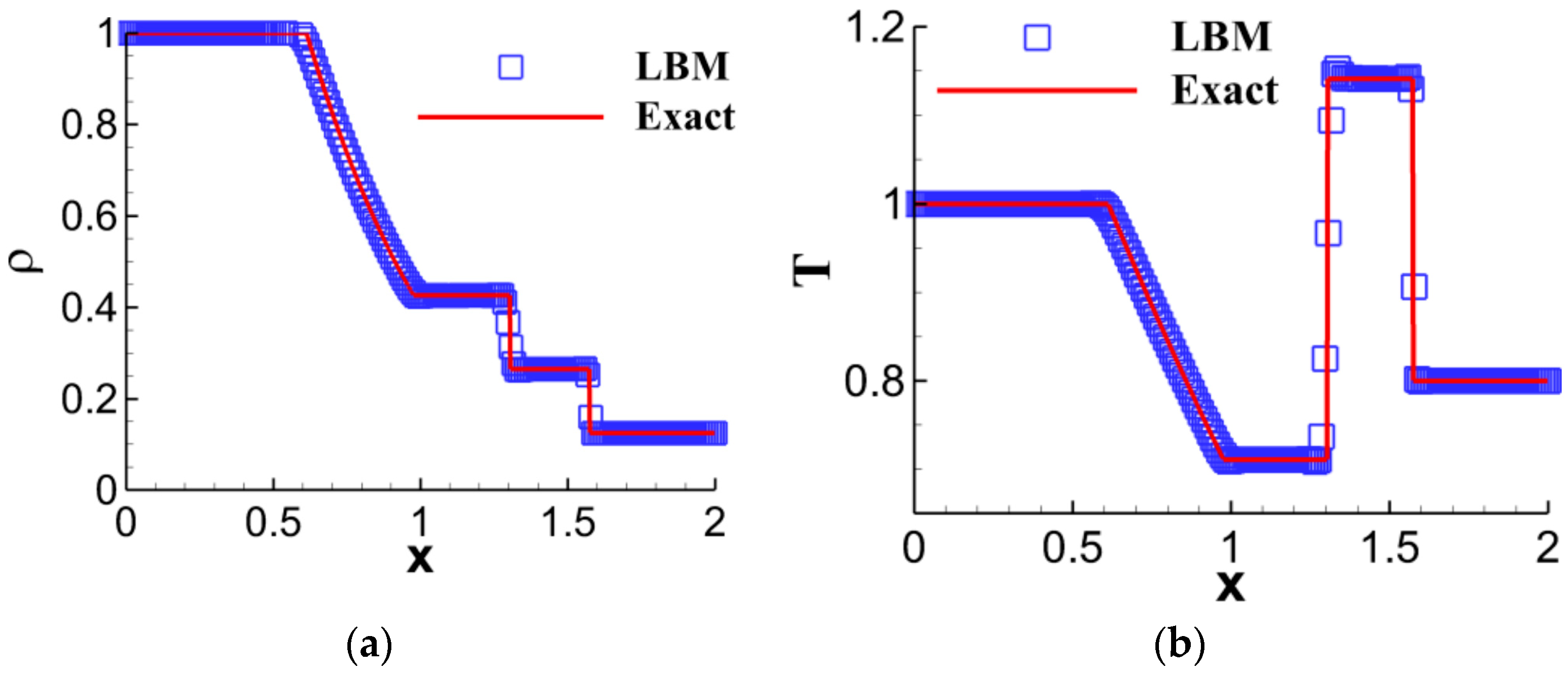
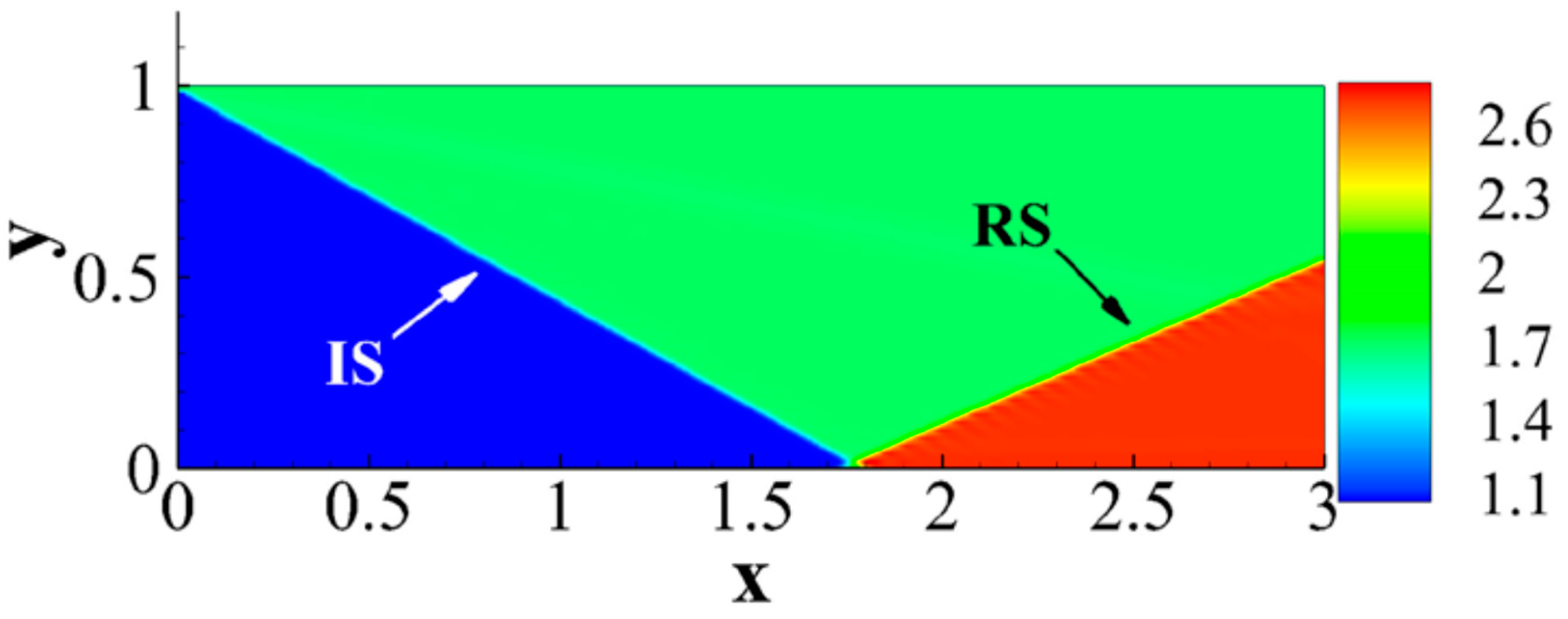
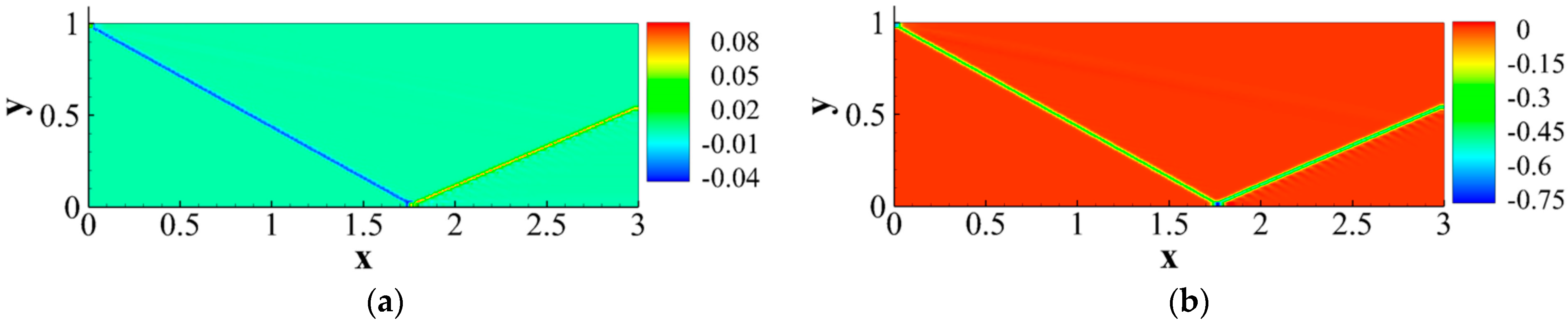
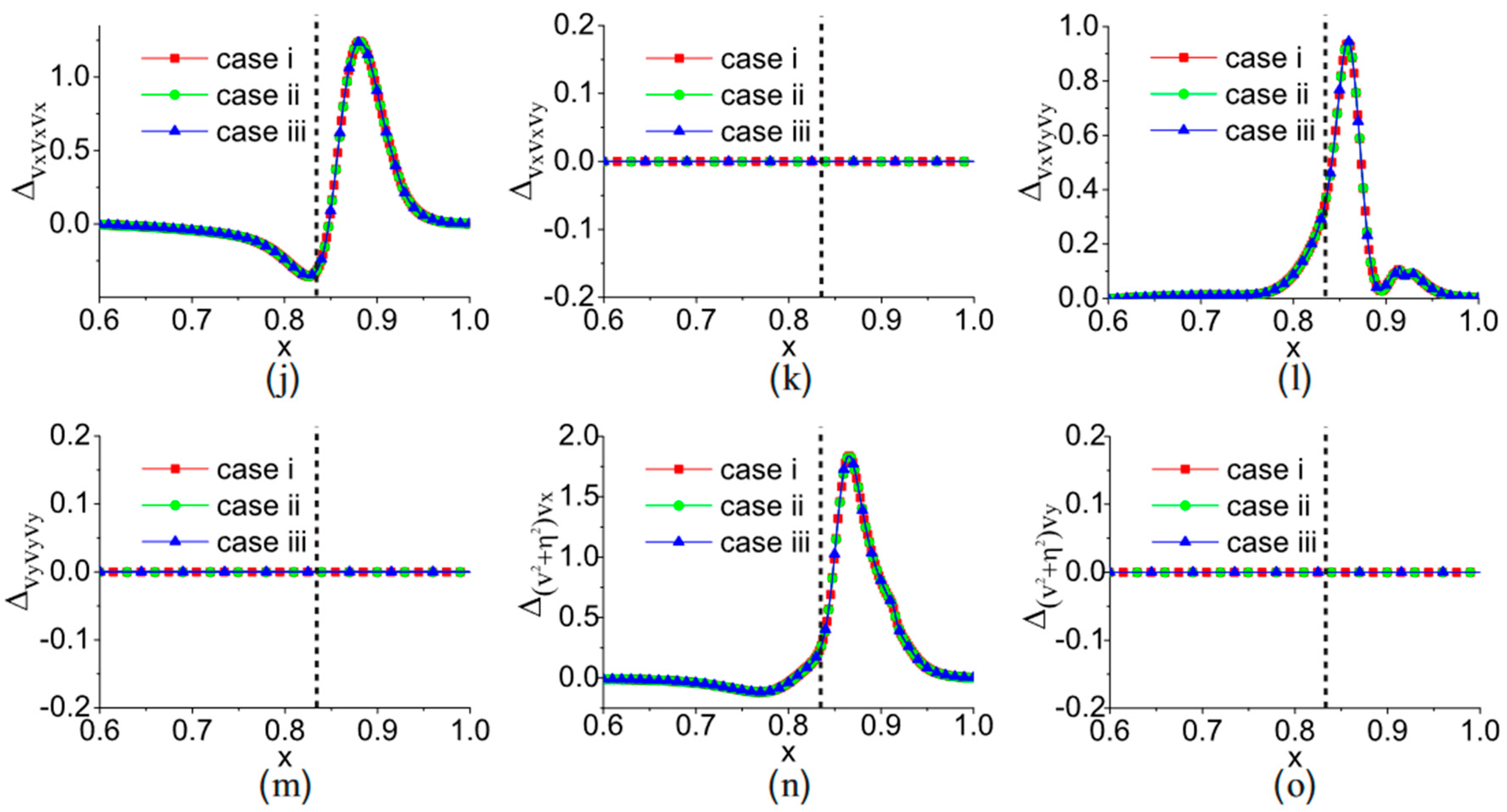
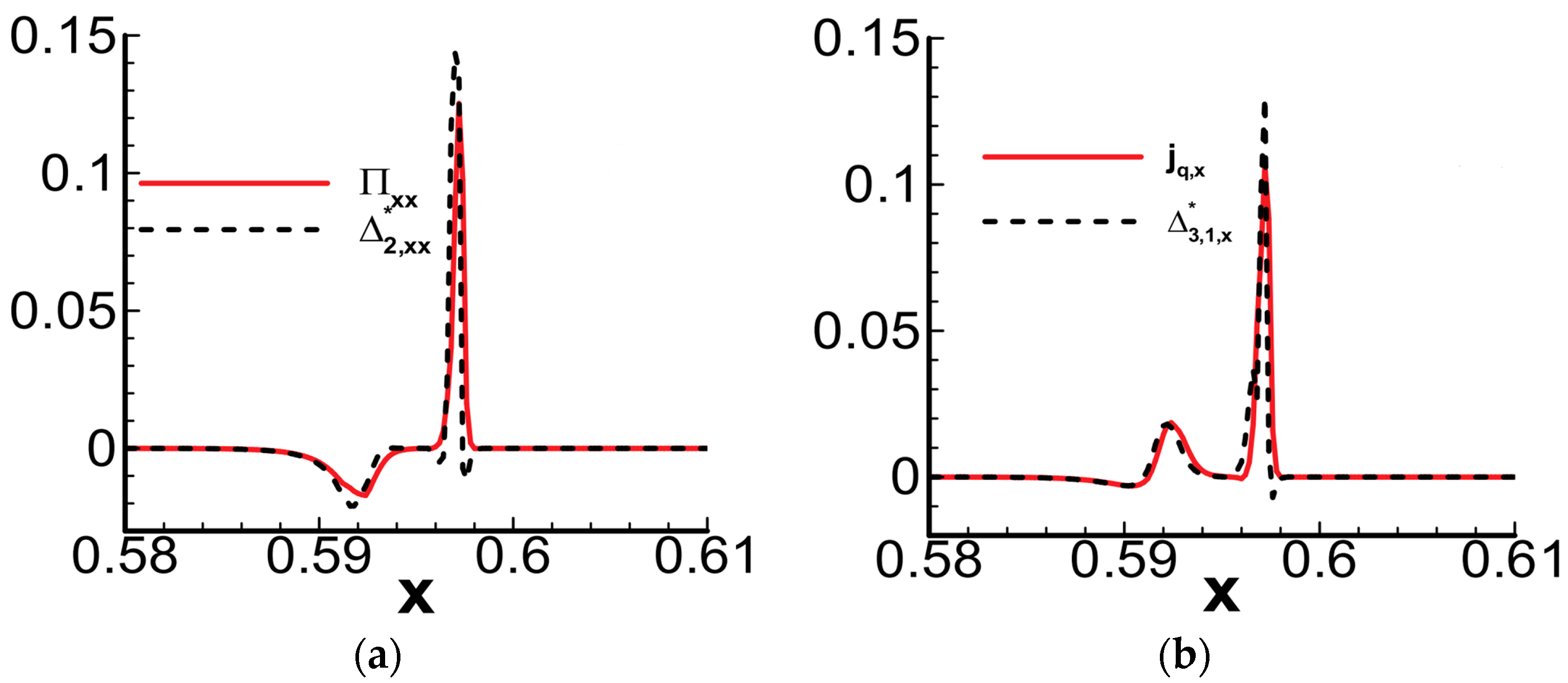
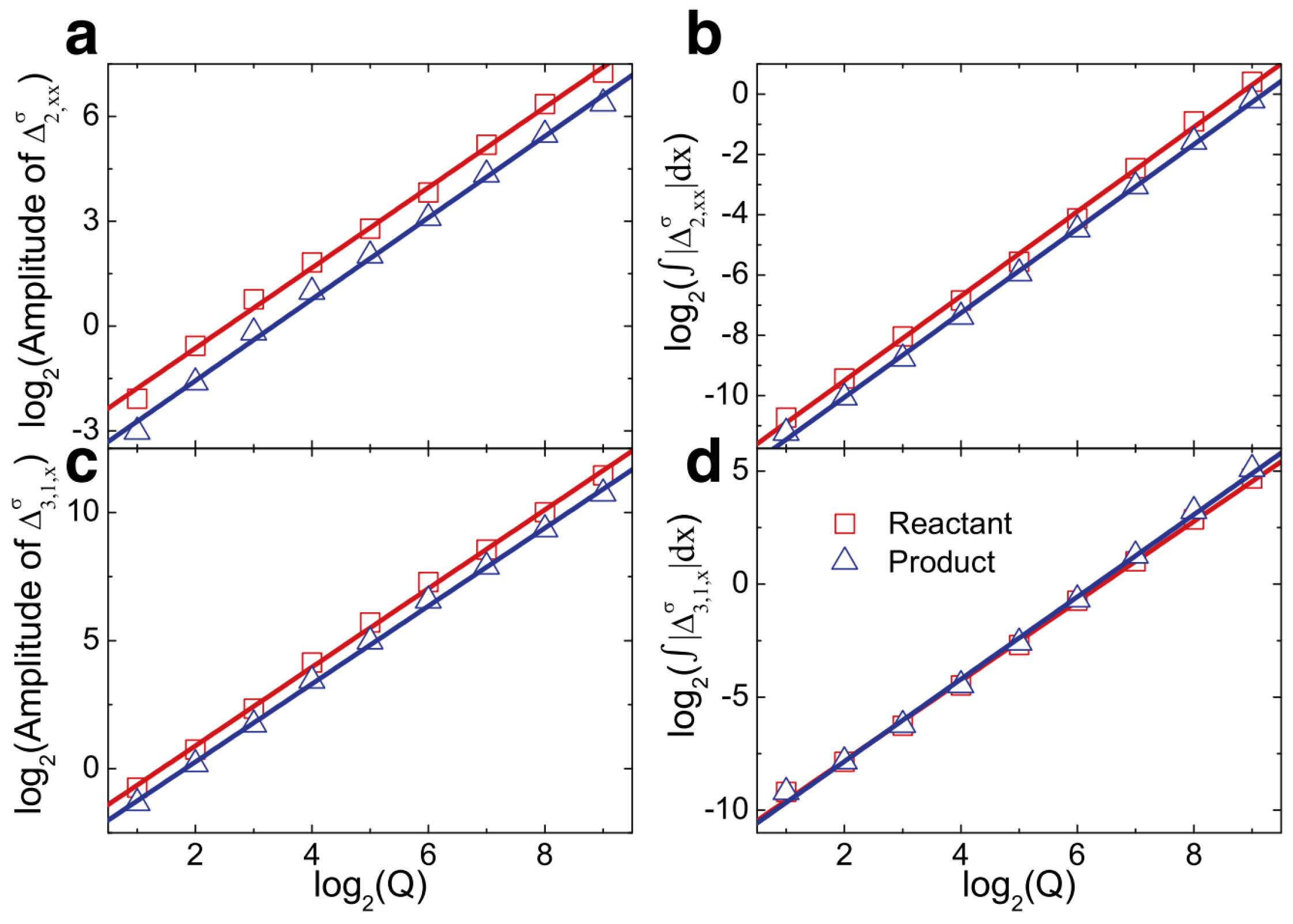
4.1. Shock Wave
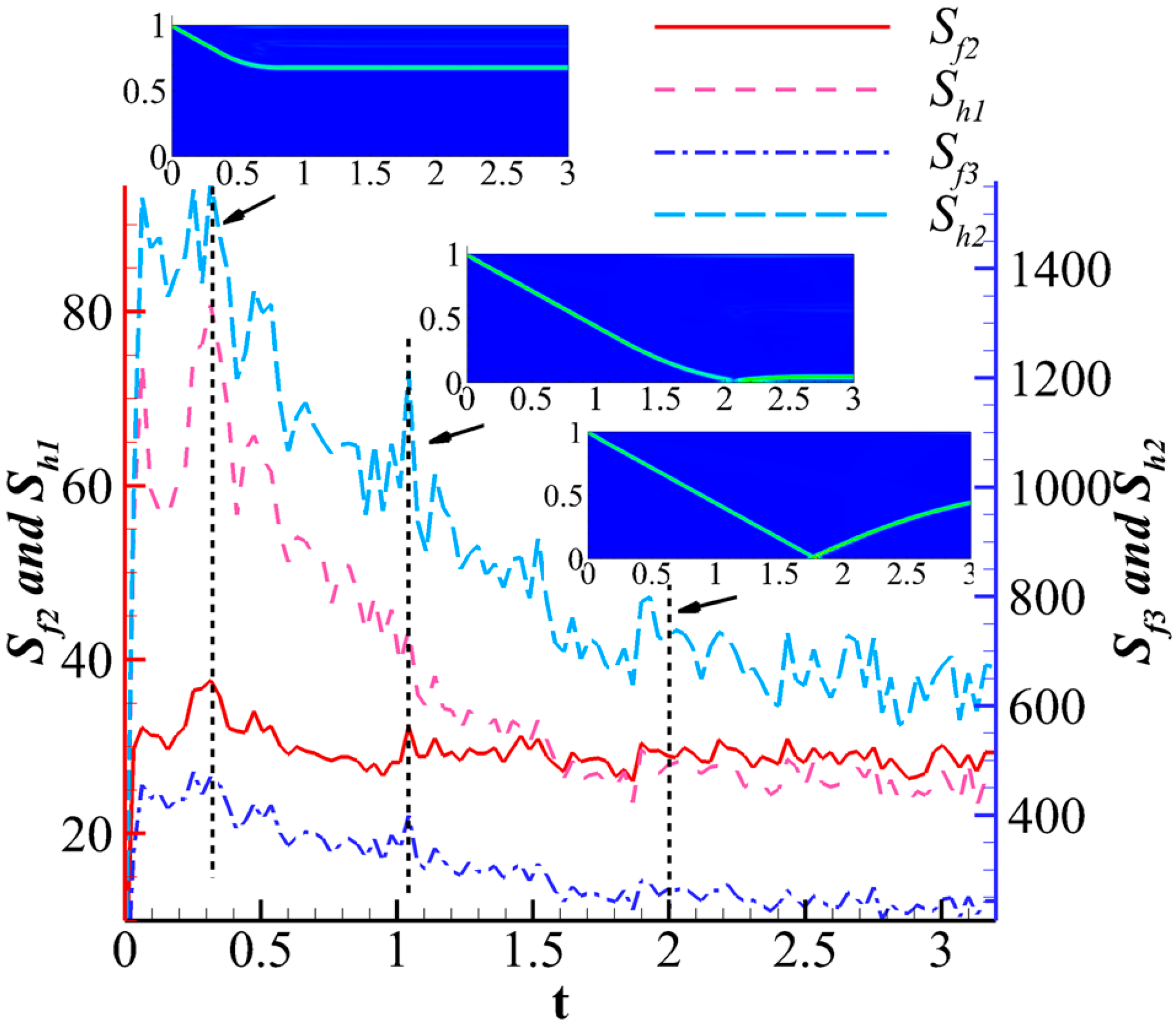
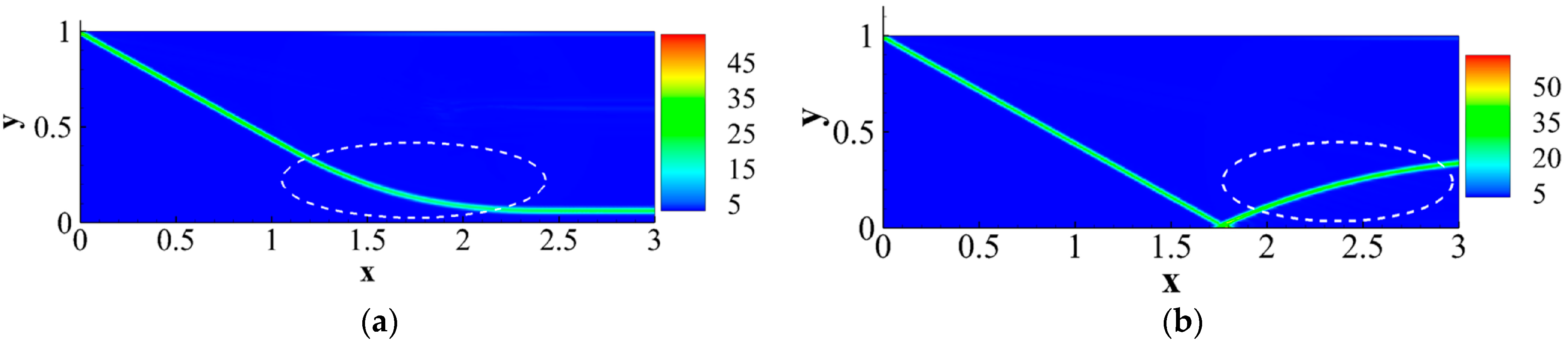
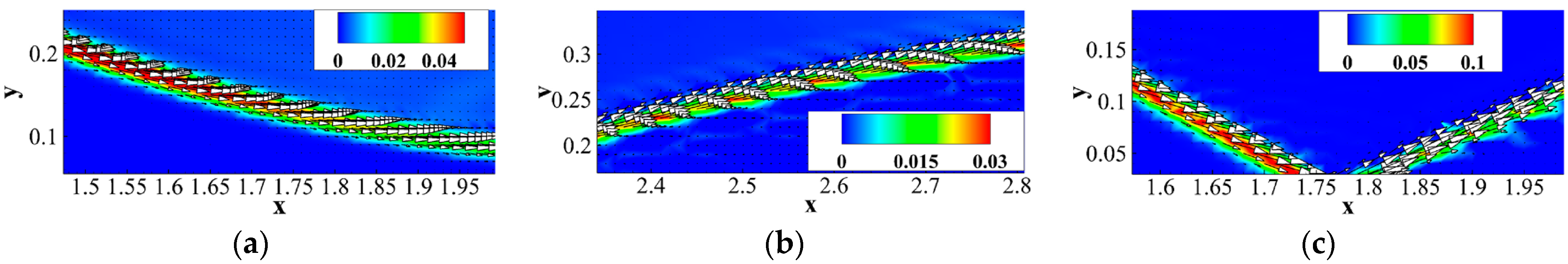
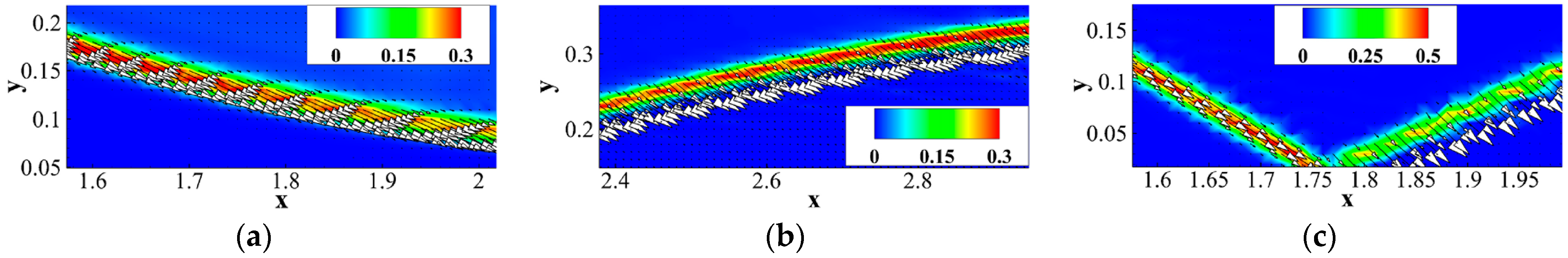
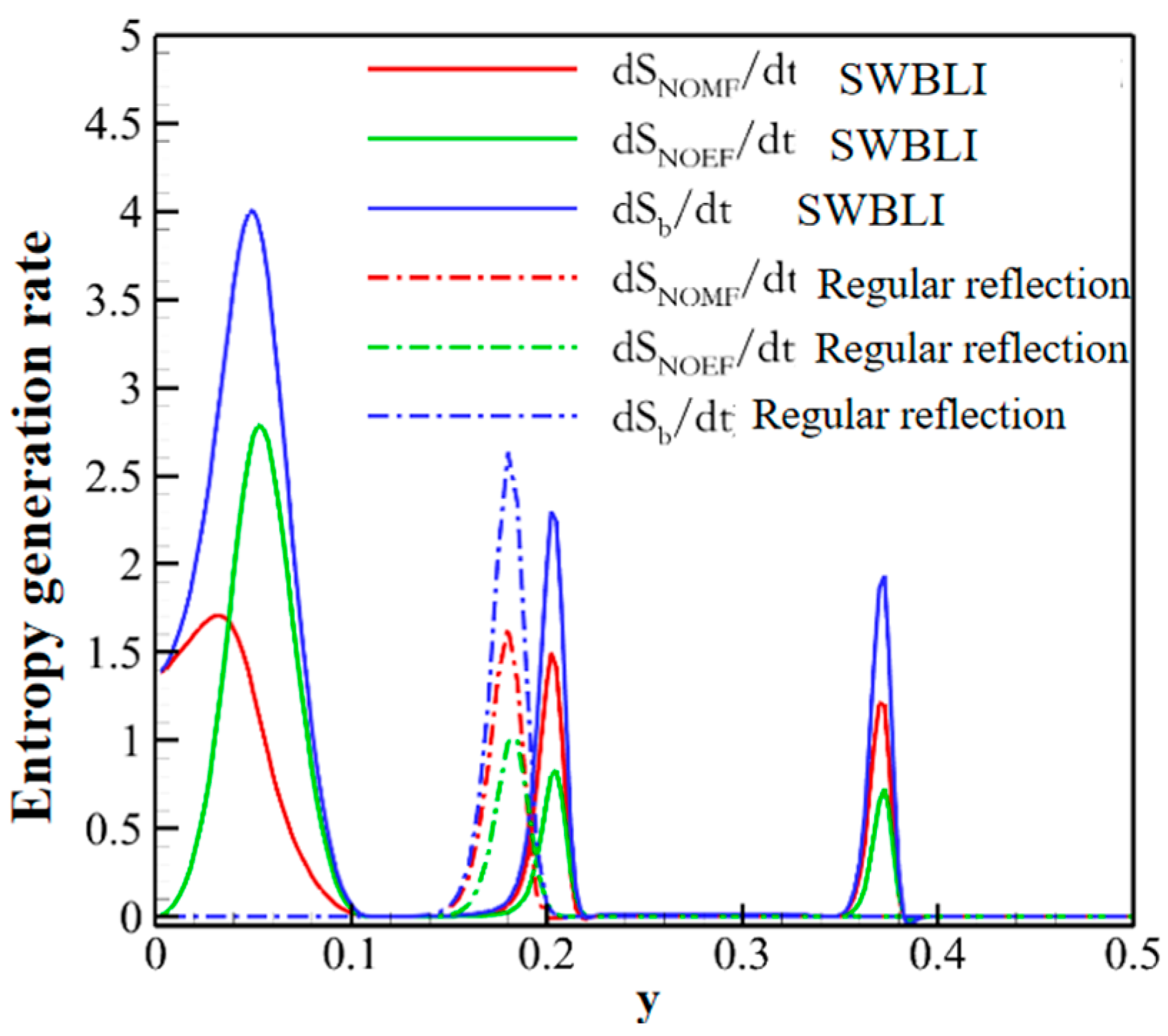
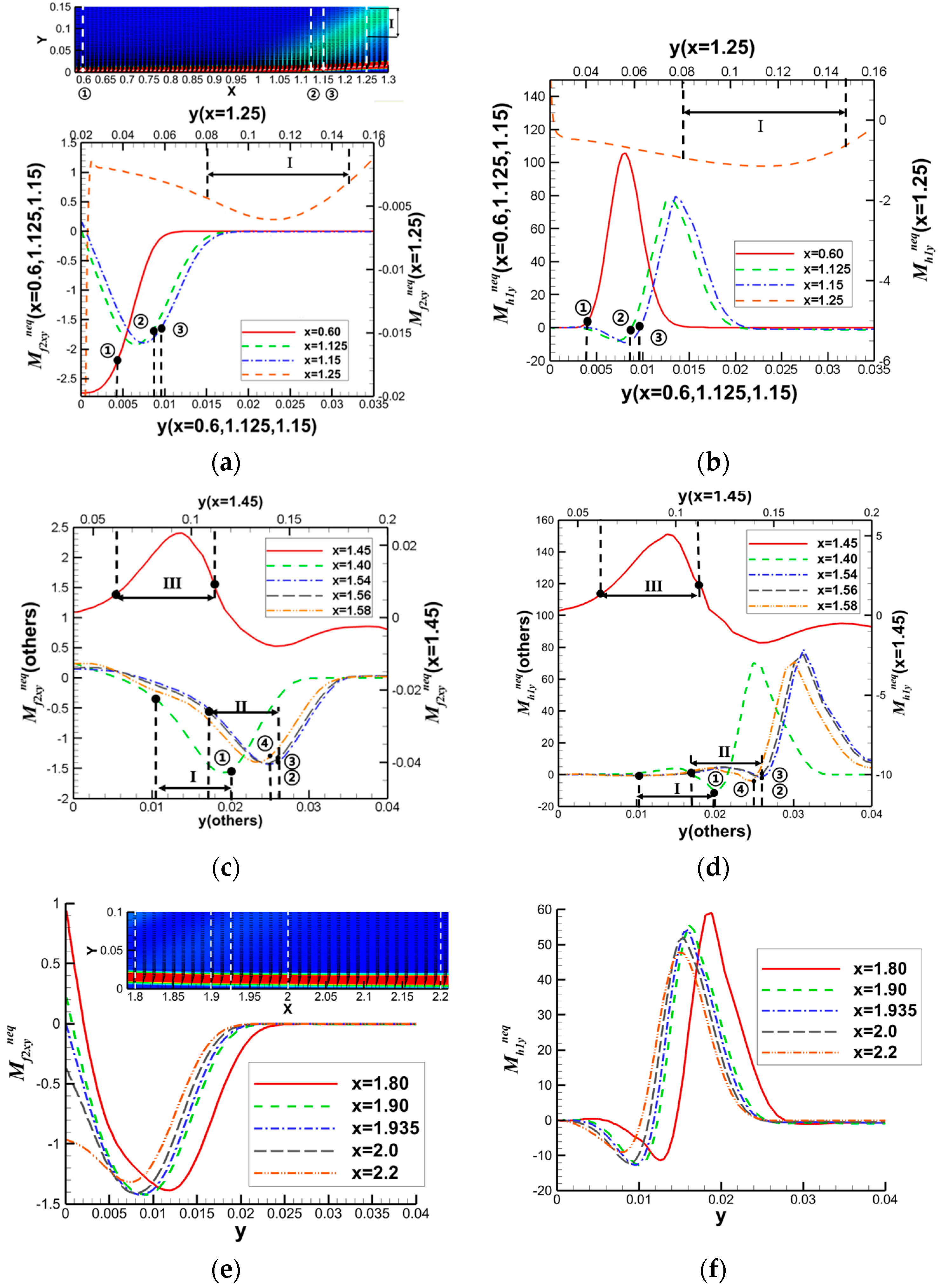
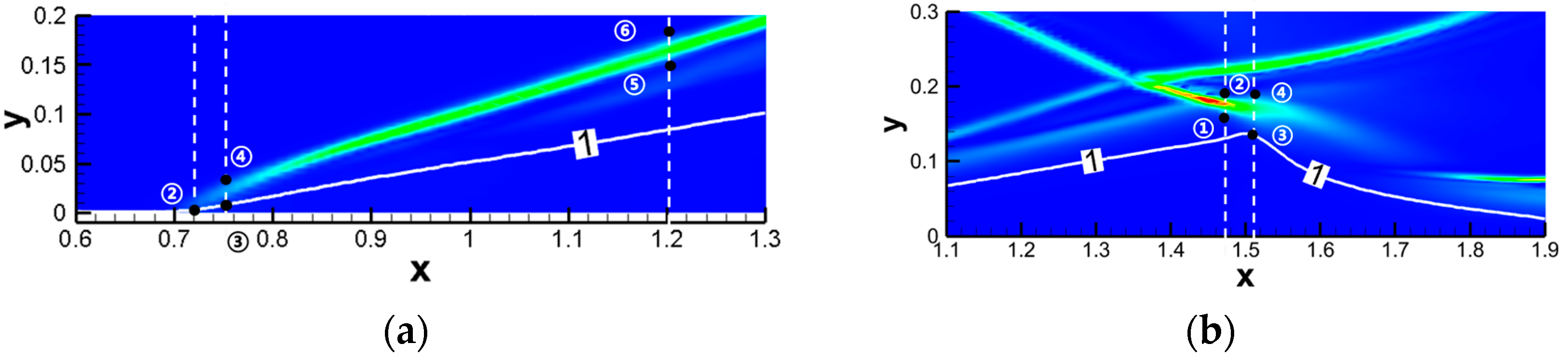
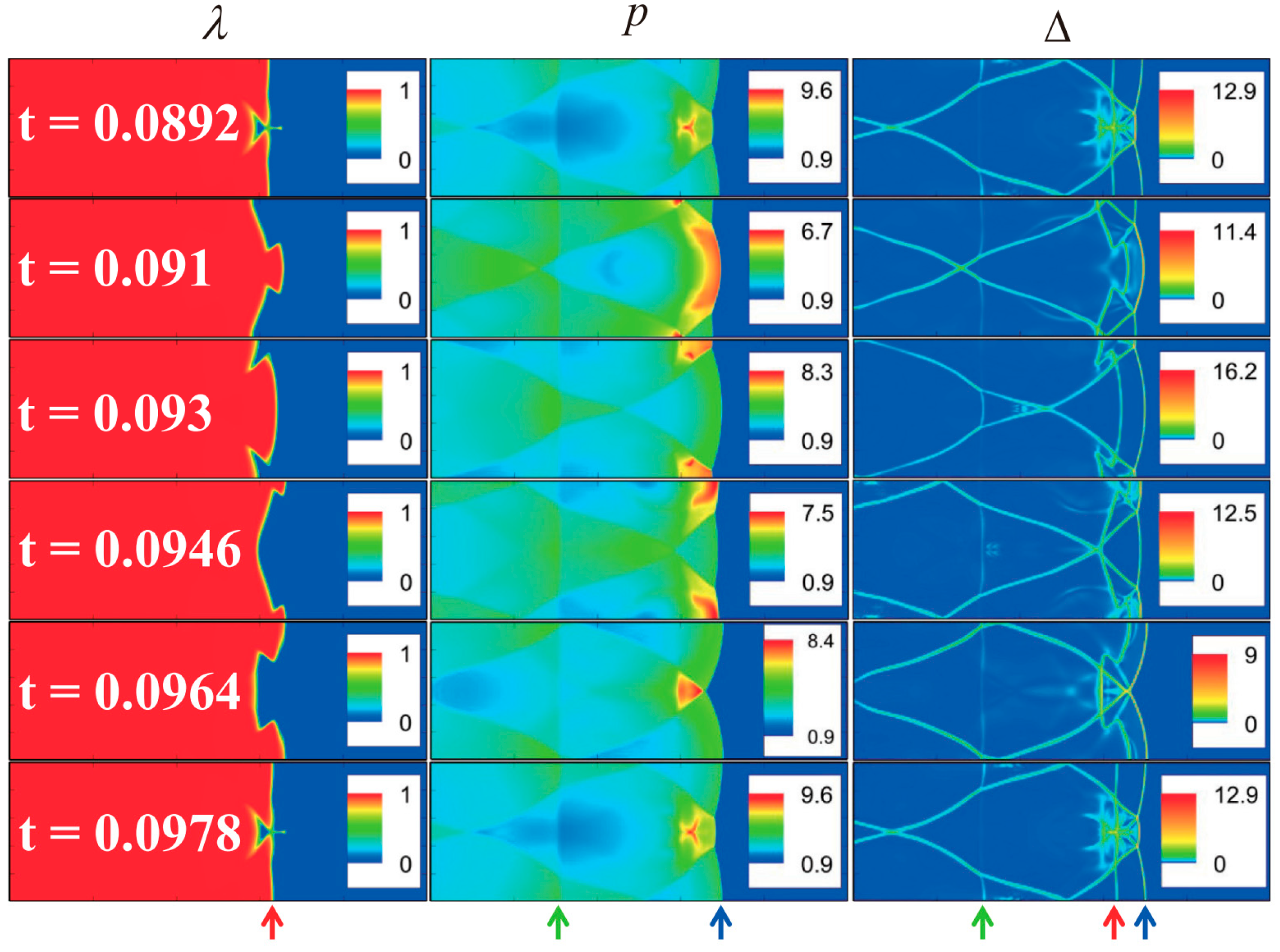
4.2. Shock Wave/Boundary Layer Interaction
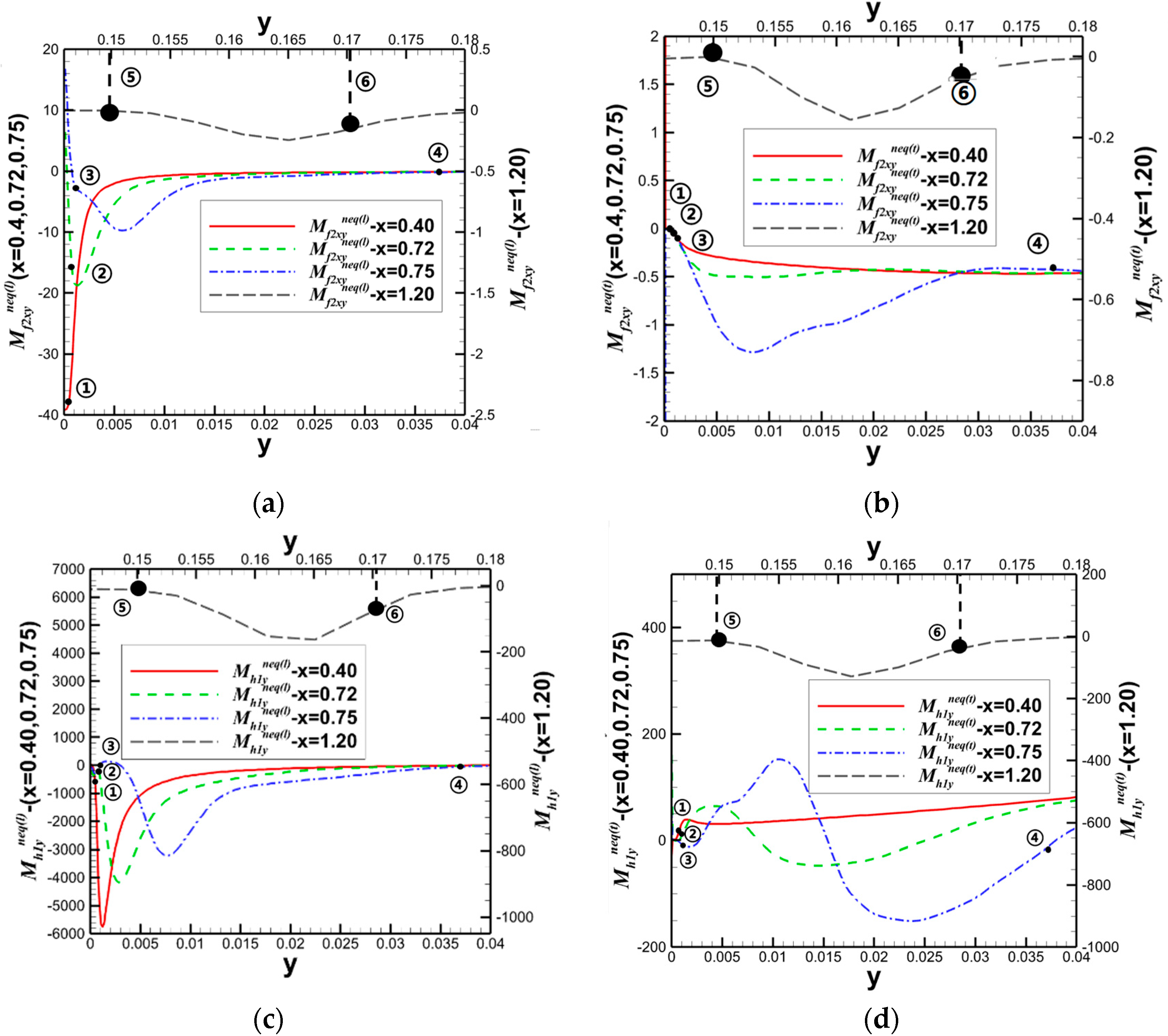
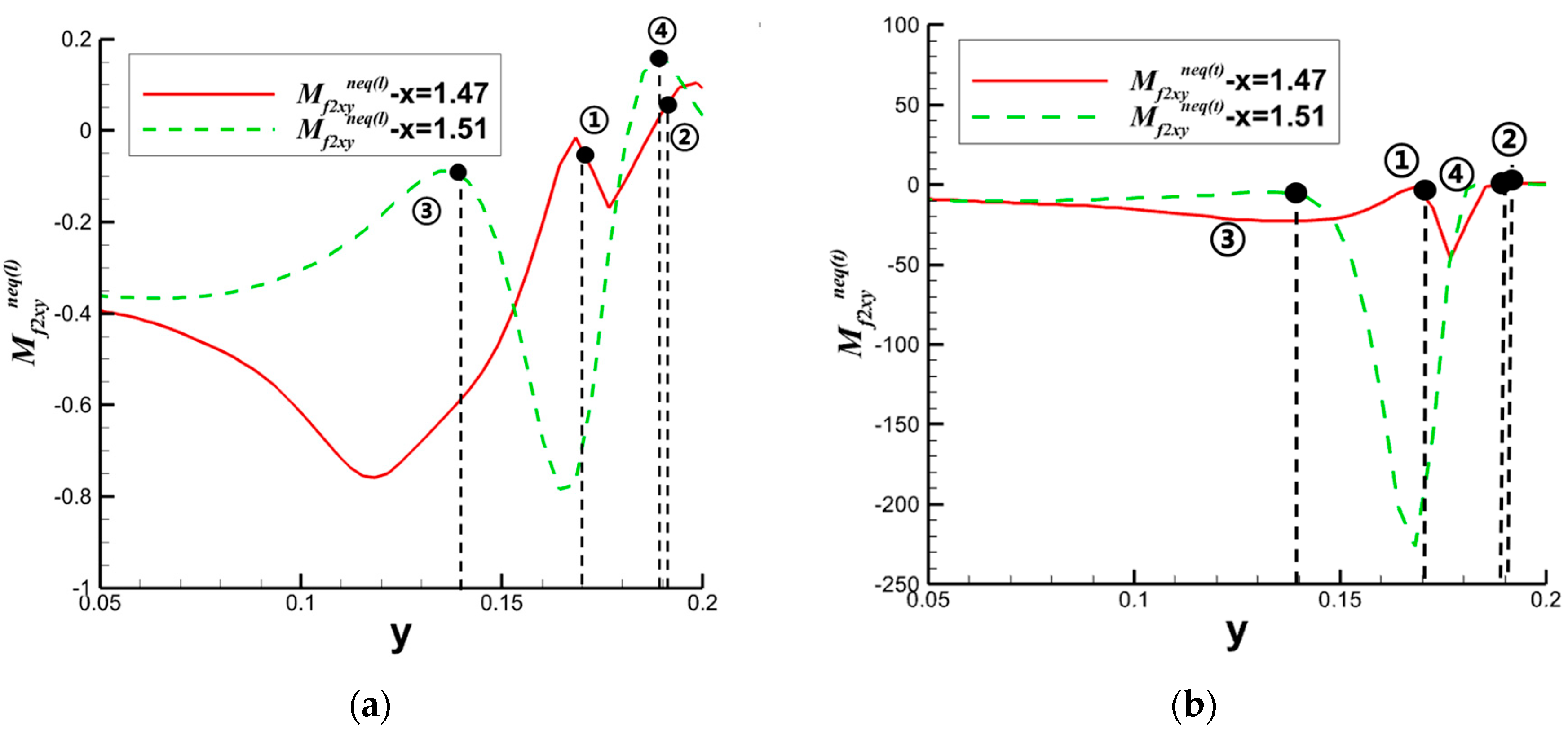
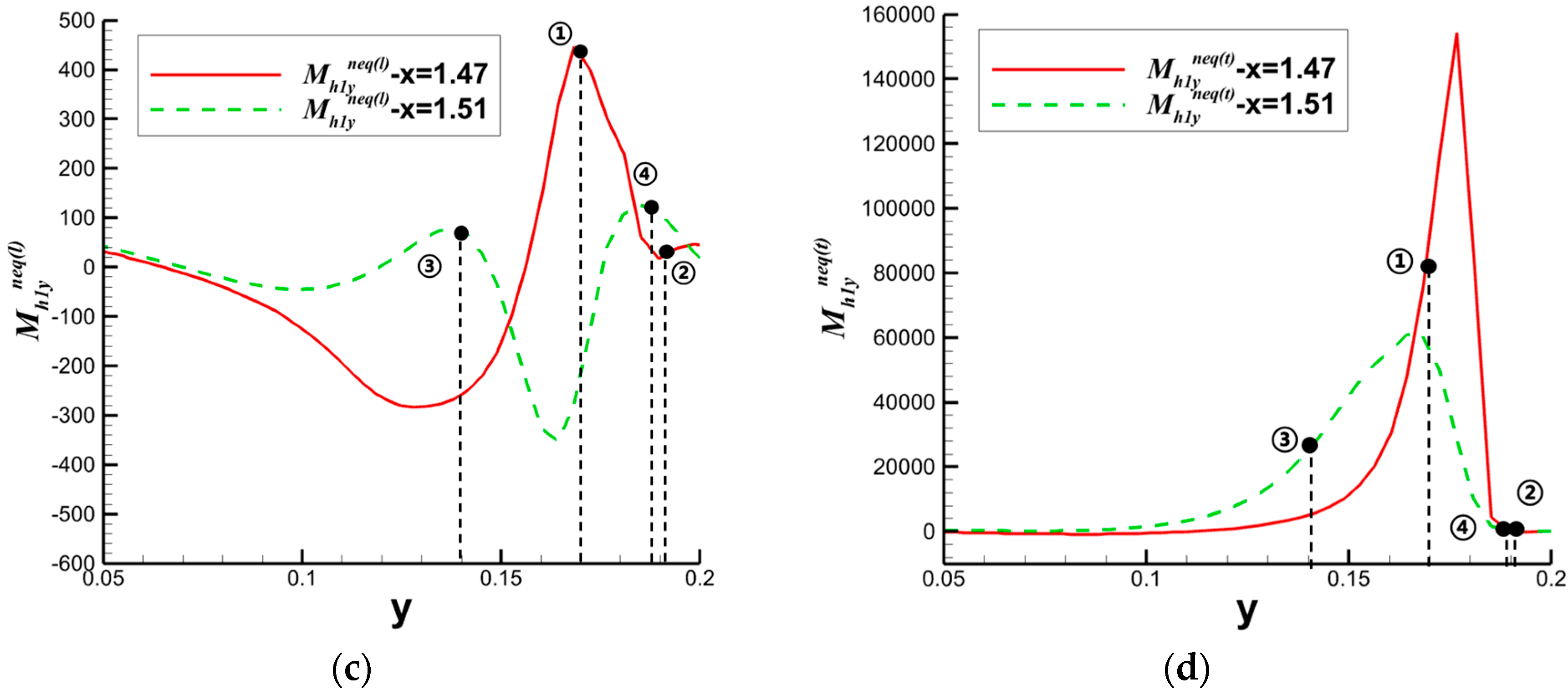
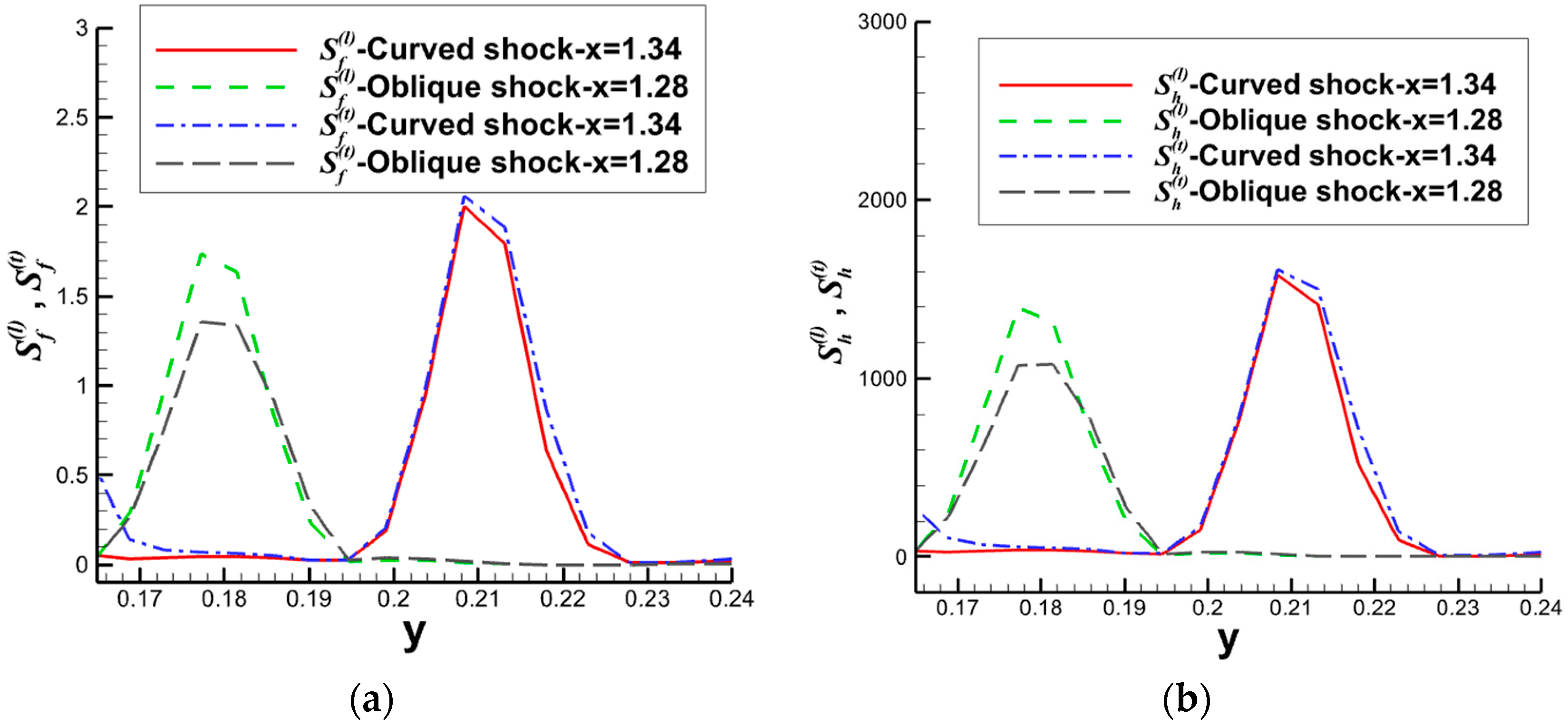
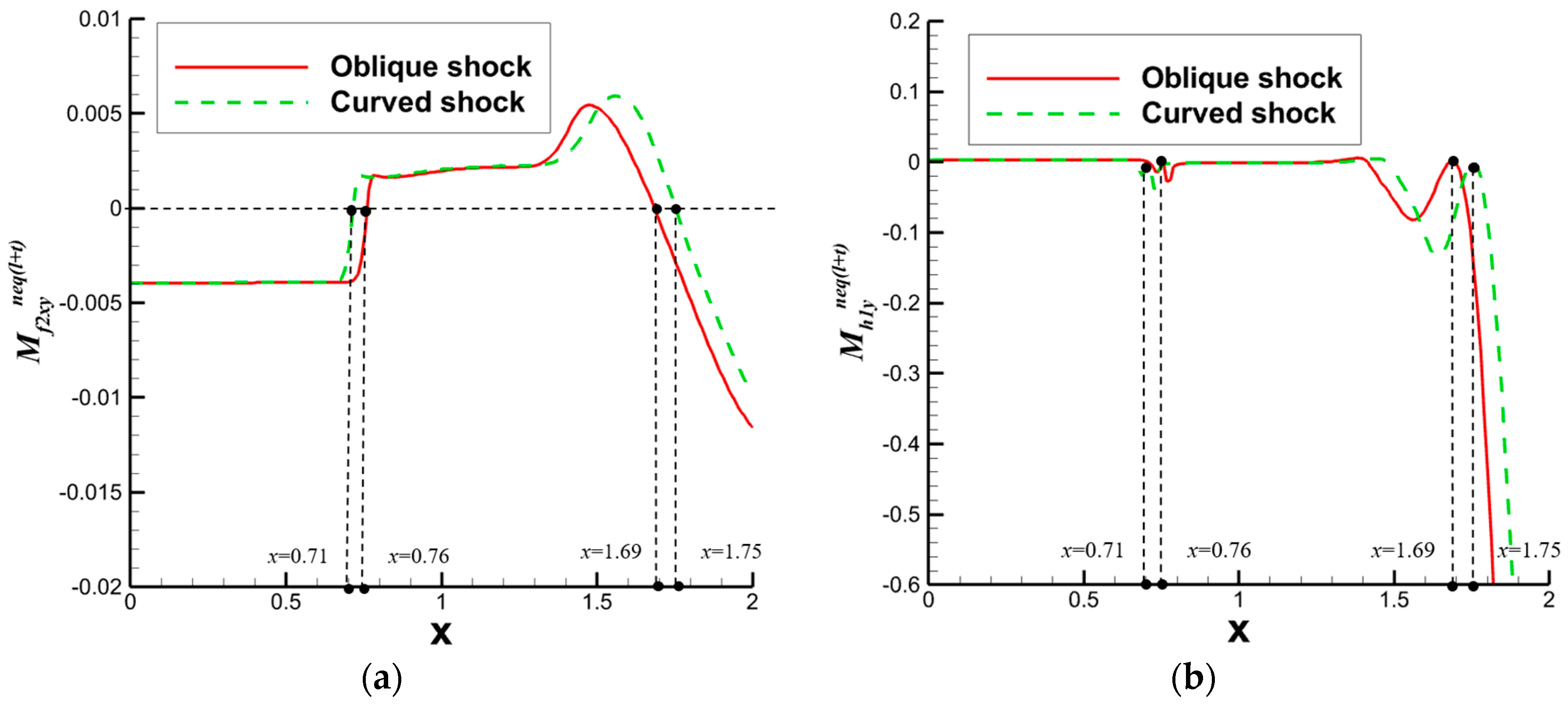
4.2.1. Oblique Shock Wave/Boundary Layer Interaction
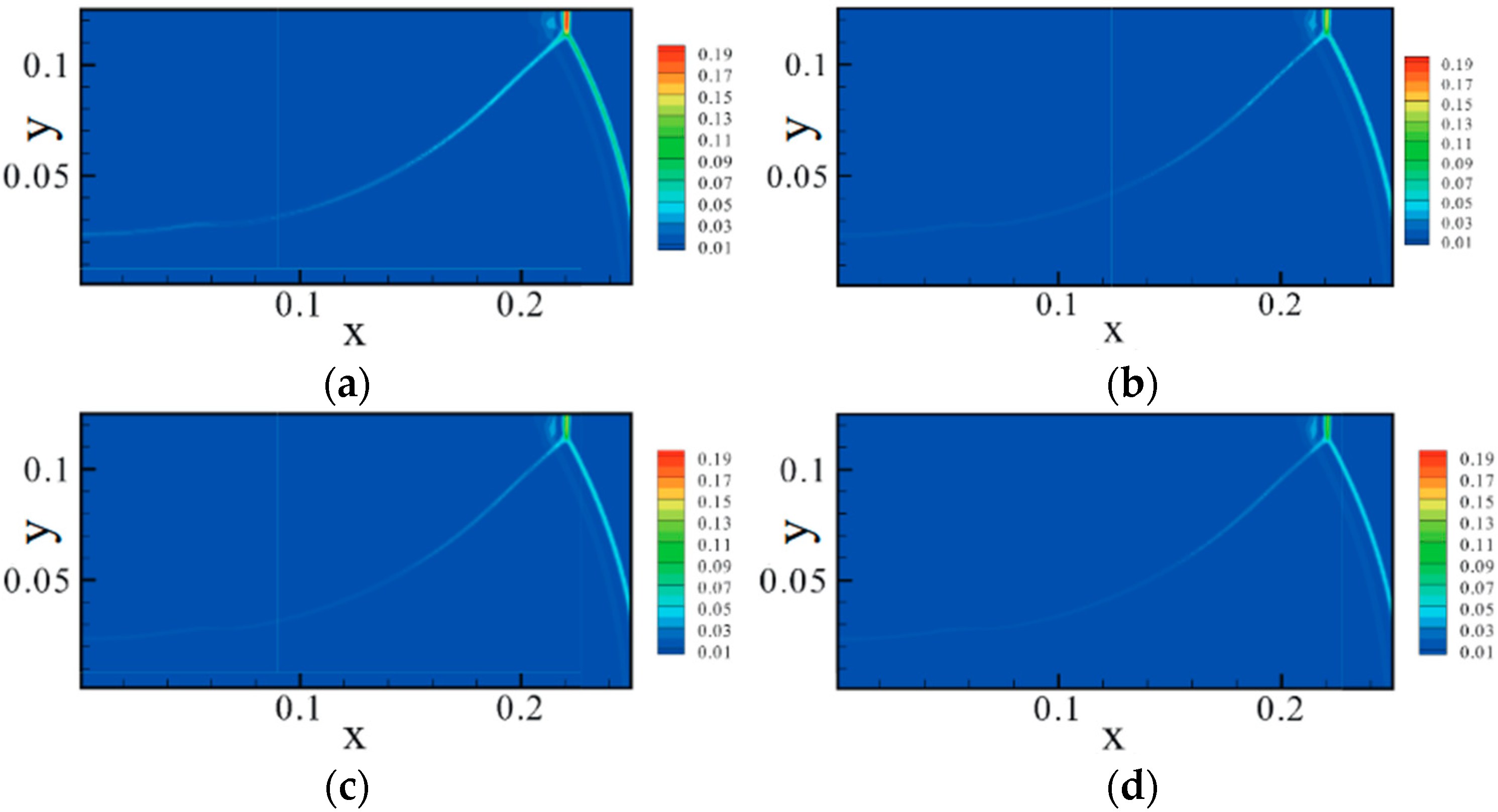
4.2.2. Curved Shock Wave/Boundary Layer Interaction
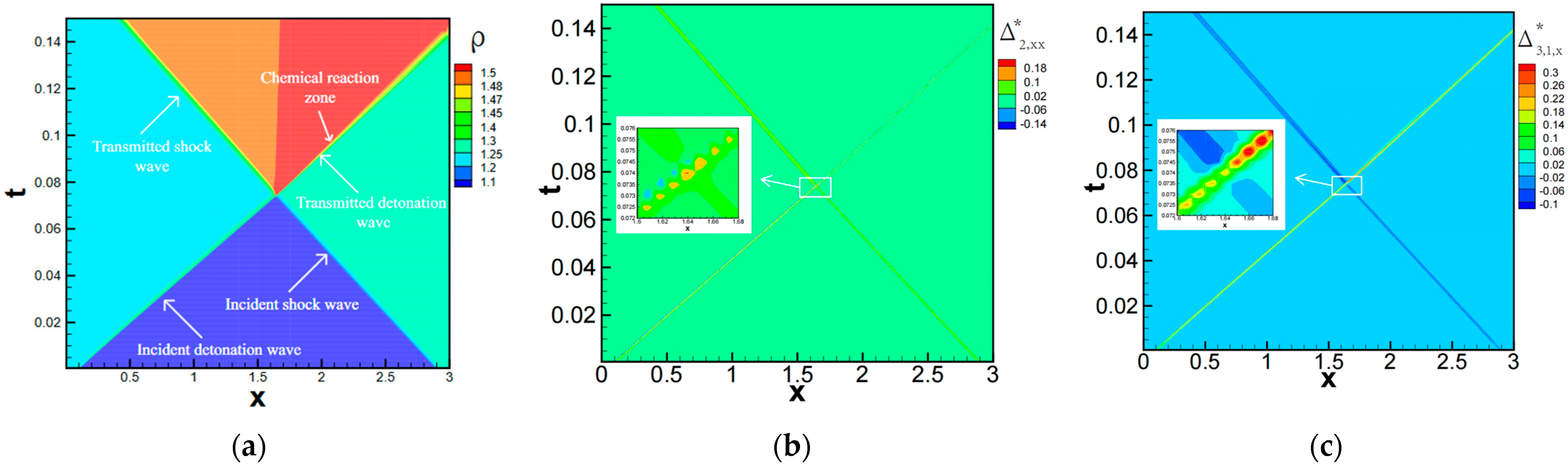
4.3. Detonation Wave
5. Summary and Discussion
- The method presented in the article offers additional flow field information beyond macroscopic variables from the perspective of molecular nonequilibrium motion for shock wave systems, enhancing the understanding of shock wave phenomena.
- The discussion in this article uses the Navier-Stokes level as an example, but the method is not limited to the Navier-Stokes level. It is applicable to LBM models with higher physical accuracy.
- Nonequilibrium effects are the root cause of the deviation of fluid systems from equilibrium. In the absence of chemical reactions, they are macroscopically related to the viscous stress and heat flux in fluid flow. In other words, viscous stress and heat flux act as the “instigators” of nonequilibrium behavior in fluid systems.
- Nonequilibrium effects represent a kind of “instability” of molecular motion relative to local equilibrium, and their numerical magnitude reflects the strength of the system’s “instability”.
- Strong nonequilibrium effects exist within shock waves. Interactions such as shock waves with solid walls, contact discontinuities, expansion waves, and boundary layers lead to variations in nonequilibrium behavior.
- Delve deeper into exploring the nonequilibrium effects of fluid systems. Utilize information on nonequilibrium behavior to reveal the formation mechanisms of flow phenomena at the mesoscopic level, further enabling the prediction of complex fluid flow systems.
- Trace the origin of fluid systems deviating from equilibrium using nonequilibrium moments. Employ nonequilibrium effects to control fluid flow systems and integrate them into the aerodynamic design of high-speed aircraft and engines.
- Extend the application of this research method to complex flow phenomena such as turbulence, aerodynamic heating, rarefied flow, etc.
- For problems with source terms, such as chemical reaction, further develop a nonequilibrium effect extraction approach to obtain the source-term related to nonequilibrium effects.
- Develop an approach for the nonequilibrium effect based on the unified mesoscopic kinetic method applicable to simulating fluids from continuous to rarefied.
Funding
Data Availability Statement
Conflicts of Interest
References
- Pham-Van-Diep, G.; Erwin, D.; Muntz, E. Nonequilibrium molecularmotion in a hypersonic shock-wave. Science 1989, 245, 624–626. [Google Scholar] [CrossRef] [PubMed]
- Bhatnagar, P.; Gross, E.; Krook, M. A Model for Collision Processes in Gases. I. Small Amplitude Processes in Charged and Neutral One-Component Systems. Phys. Rev. 1954, 94, 511–525. [Google Scholar] [CrossRef]
- Xu, A.; Lin, C.; Zhang, G.; Li, Y. Multiple-relaxation-time lattice Boltzmann kinetic model for combustion. Phys. Rev. E 2015, 91, 043306. [Google Scholar] [CrossRef]
- Qian, Y.; D’Humires, D.; Lallemand, P. Lattice BGK model for Navier-Stokes equation. Europhys. Lett. 1992, 17, 479–484. [Google Scholar] [CrossRef]
- Chen, S.; Doolen, G. Lattice Boltzmann method for fluid flows. Annu. Rev. Fluid Mech. 1998, 30, 329–364. [Google Scholar] [CrossRef]
- Chen, H.; Kandasamy, S.; Orszag, S.; Shock, R.; Succi, S.; Yakhot, V. Extended Boltzmann Kinetic Equation for Turbulent Flows. Science 2003, 301, 633–636. [Google Scholar] [CrossRef]
- Eshghinejadfard, A.; Zhao, L.; Thévenin, D. Lattice Boltzmann simulation of resolved oblate spheroids in wall turbulence. J. Fluid Mech. 2018, 849, 510–540. [Google Scholar] [CrossRef]
- Xu, A.; Chen, J.; Song, J.; Chen, D.; Chen, Z. Progress of discrete Boltzmann study on multiphase complex flows. Acta Aerodyn. Sin. 2021, 39, 138–169. (In Chinese) [Google Scholar] [CrossRef]
- Liu, H.; Ba, Y.; Wu, L.; Li, Z.; Xi, G.; Zhang, Y. A hybrid lattice Boltzmann and finite difference method for droplet dynamics with insoluble surfactants. J. Fluid Mech. 2018, 837, 381–412. [Google Scholar] [CrossRef]
- Lamarti, H.; Mahdaoui, M.; Bennacer, R.; Chahboun, A. Numerical simulation of mixed convection heat transfer of fluid in a cavity driven by an oscillating lid using lattice Boltzmann method. Int. J. Heat Mass Transf. 2019, 137, 615–629. [Google Scholar] [CrossRef]
- Mu, Y.; Gu, Z.; He, P.; Tao, W. Lattice Boltzmann method for conjugated heat and mass transfer with general interfacial conditions. Phys. Rev. E 2018, 98, 043309. [Google Scholar] [CrossRef]
- Xu, L.; Tian, F.; Young, J.; Lai, J. A novel geometry-adaptive Cartesian grid based immersed boundary–lattice Boltzmann method for fluid–structure interactions at moderate and high Reynolds numbers. J. Comput. Phys. 2018, 375, 22–56. [Google Scholar] [CrossRef]
- Liu, F.; Liu, G.; Shu, C. Fluid–structure interaction simulation based on immersed boundary-lattice Boltzmann flux solver and absolute nodal coordinate formula. Phys. Fluids 2020, 32, 047109. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Influence of magnetic field on nanofluid free convection in an open porous cavity by means of Lattice Boltzmann method. J. Mol. Liq. 2017, 234, 364–374. [Google Scholar] [CrossRef]
- Silva, G.; Talon, L.; Ginzburg, I. Low-and high-order accurate boundary conditions: From Stokes to Darcy porous flow modeled with standard and improved Brinkman lattice Boltzmann schemes. J. Comput. Phys. 2017, 335, 50–83. [Google Scholar] [CrossRef]
- Kataoka, T.; Tsutahara, M. Lattice Boltzmann model for the compressible Navier-Stokes equations with flexible specific-heat ratio. Phys. Rev. E 2004, 69, 035701. [Google Scholar] [CrossRef] [PubMed]
- Gan, Y.; Xu, A.; Zhang, G.; Yang, Y. Lattice BGK kinetic model for high-speed compressible flows: Hydrodynamic and nonequilibrium behaviors. Europhys. Lett. 2013, 103, 24003. [Google Scholar] [CrossRef]
- Shan, X.; Yuan, X.F.; Chen, H. Kinetic theory representation of hydrodynamics: A way beyond the Navier–Stokes equation. J. Fluid Mech. 2006, 550, 413–441. [Google Scholar] [CrossRef]
- Qu, K.; Shu, C.; Chew, Y.T. Alternative method to construct equilibrium distribution functions in lattice-Boltzmann method simulation of inviscid compressible flows at high Mach number. Phys. Rev. E 2007, 75, 036706. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; He, Y.L.; Wang, Y.; Tao, W. Coupled double-distribution-function lattice Boltzmann method for the compressible Navier-Stokes equations. Phys. Rev. E 2007, 76, 056705. [Google Scholar] [CrossRef] [PubMed]
- Frapolli, N.; Chikatamarla, S.; Karlin, I. Entropic lattice Boltzmann model for compressible flows. Phys. Rev. E 2015, 92, 061301. [Google Scholar] [CrossRef] [PubMed]
- Latt, J.; Coreixas, C.; Beny, J.; Parmigiani, A. Efficient supersonic flow simulations using lattice Boltzmann methods based on numerical equilibria. Philos. Trans. R. Soc. A 2020, 378, 20190559. [Google Scholar] [CrossRef] [PubMed]
- Zhang, D.; Xu, A.; Song, J.; Gan, Y.; Zhang, Y.; Li, Y. Specific-heat ratio effects on the interaction between shock wave and heavy-cylindrical bubble: Based on discrete Boltzmann method. arXiv 2023, arXiv:2302.05687. [Google Scholar] [CrossRef]
- Gan, Y.; Xu, A.; Zhang, G.; Succi, S. Discrete Boltzmann modeling of multiphase flows: Hydrodynamic and thermodynamic nonequilibrium effects. Soft Matter 2015, 11, 5336–5345. [Google Scholar] [CrossRef] [PubMed]
- Lai, H.; Xu, A.; Zhang, G.; Gan, Y.; Ying, Y.; Succi, S. Nonequilibrium thermohydrodynamic effects on the Rayleigh-Taylor instability in compressible flows. Phys. Rev. E 2016, 94, 023106. [Google Scholar] [CrossRef]
- Gan, Y.; Xu, A.; Lai, H.; Li, W.; Sun, G.; Succi, S. Discrete Boltzmann multi-scale modelling of non-equilibrium multiphase flows. J. Fluid Mech. 2022, 951, A8. [Google Scholar] [CrossRef]
- Chen, F.; Xu, A.; Zhang, Y.; Zhang, Y.; Zeng, Q. Morphological and nonequilibrium analysis of coupled Rayleigh–Taylor–Kelvin–Helmholtz instability. Phys. Fluids 2020, 32, 104111. [Google Scholar] [CrossRef]
- Lin, C.; Su, X.; Zhang, Y. Hydrodynamic and Thermodynamic Nonequilibrium Effects around Shock Waves: Based on a Discrete Boltzmann Method. Entropy 2020, 22, 1397. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, A.; Zhang, G.; Zhu, C.; Lin, C. Kinetic modeling of detonation and effects of negative temperature coefficient. Combust. Flame 2016, 173, 483–492. [Google Scholar] [CrossRef]
- Lin, C.; Luo, K. Mesoscopic simulation of nonequilibrium detonation with discrete Boltzmann method. Combust. Flame 2018, 198, 356–362. [Google Scholar] [CrossRef]
- Lin, C.; Luo, K. Discrete Boltzmann modeling of unsteady reactive flows with nonequilibrium effects. Phys. Rev. E 2019, 99, 012142. [Google Scholar] [CrossRef]
- Chapman, S.; Cowling, T. The Mathematical Theory of Non-Uniform Gases: An Account of the Kinetic Theory of Viscosity, Thermal Conduction and Diffusion in Gases; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Struchtrup, H. Macroscopic Transport Equations for Rarefied Gas Flows; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Bird, G.A. Recent advances and current challenges for DSMC. Comput. Math. Appl. 1998, 35, 1–14. [Google Scholar] [CrossRef]
- Broadwell, J. Study of rarefied shear flow by the discrete velocity method. J. Fluid Mech. 1964, 19, 401–414. [Google Scholar] [CrossRef]
- Hadjiconstantinou, N.; Garcia, A.; Bazant, M.; He, G. Statistical error in particle simulations of hydrodynamic phenomena. J. Comput. Phys. 2003, 187, 274–297. [Google Scholar] [CrossRef]
- Shi, Y.; Wu, L.; Shan, X. Accuracy of high-order lattice Boltzmann method for non-equilibrium gas flow. J. Fluid Mech. 2021, 907, A25. [Google Scholar] [CrossRef]
- Yang, L.; Shu, C.; Wu, J.; Wang, Y. Comparative study of discrete velocity method and high-order lattice Boltzmann method for simulation of rarefied flows. Comput. Fluids 2017, 146, 125–142. [Google Scholar] [CrossRef]
- Guo, S.; Feng, Y.; Jacob, J.; Renard, F.; Sagaut, P. An efficient lattice Boltzmann method for compressible aerodynamics on D3Q19 lattice. J. Comput. Phys. 2020, 418, 109570. [Google Scholar] [CrossRef]
- Zhang, D.; Xu, A.; Zhang, Y.; Gan, Y.; Li, Y. Discrete Boltzmann modeling of high-speed compressible flows with various depths of non-equilibrium. Phys. Fluids 2022, 34, 086104. [Google Scholar] [CrossRef]
- Gan, Y.; Xu, A.; Zhang, G.; Zhang, Y.; Succi, S. Discrete Boltzmann trans-scale modeling of high-speed compressible flows. Phys. Rev. E 2018, 97, 053312. [Google Scholar] [CrossRef]
- Xu, A.; Zhang, G.; Gan, Y.; Chen, F.; Yu, X. Lattice Boltzmann modeling and simulation of compressible flows. Front. Phys. 2012, 7, 582–600. [Google Scholar] [CrossRef]
- Meskos, S.; Stefanov, S. A direct simulation Monte Carlo approach on the Riemann problem for gas mixtures. AIP Conf. Proc. 2019, 2164, 110010. [Google Scholar]
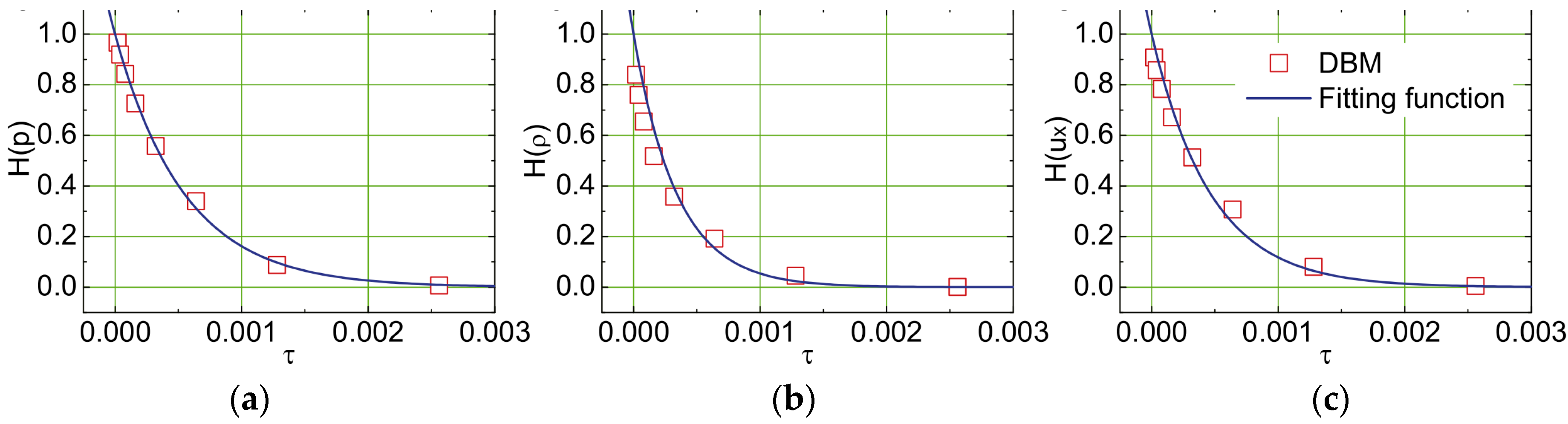
- Qiu, R.; Bao, Y.; Zhou, T.; Che, H.; Chen, R.; You, Y. Study of regular reflection shock waves using a mesoscopic kinetic approach: Curvature pattern and effects of viscosity. Phys. Fluids 2020, 32, 106106. [Google Scholar] [CrossRef]
- Bao, Y.; Qiu, R.; Zhou, K.; Zhou, T.; Weng, Y.; Lin, K.; You, Y. Study of shock wave/boundary layer interaction from the perspective of nonequilibrium effects. Phys. Fluids 2022, 34, 046109. [Google Scholar] [CrossRef]
- Song, J.; Xu, A.; Miao, L.; Liao, Y.; Liang, F.; Tian, F.; Nie, M.; Wang, N. Entropy increase characteristics of shock wave/plate laminar boundary layer interaction. Acta Aeronaut. Astronaut. Sin. 2023, 44, 528520. [Google Scholar] [CrossRef]
- Shan, Y.; Xu, A.; Zhang, Y.; Wang, L.; Chen, F. Discrete Boltzmann modeling of detonation: Based on the Shakhov model. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2023, 237, 2517–2531. [Google Scholar] [CrossRef]
- Qiu, R.; Zhou, T.; Bao, Y.; Kang, Z.; Che, H.; You, Y. Mesoscopic kinetic approach for studying nonequilibrium hydrodynamic and thermodynamic effects of shock wave, contact discontinuity, and rarefaction wave in the unsteady shock tube. Phys. Rev. E 2021, 103, 053113. [Google Scholar] [CrossRef] [PubMed]
- Daru, V.; Tenaud, C. Evaluation of TVD high resolution schemes for unsteady viscous shocked flows. Comput. Fluids 2000, 30, 89–113. [Google Scholar] [CrossRef]
- Mölder, S. Curved shock theory. Shock. Waves 2016, 26, 337–353. [Google Scholar] [CrossRef]
- Shi, C.; Han, W.; Deiterding, R.; Zhu, C.; You, Y. Second-order curved shock theory. J. Fluid Mech. 2020, 891, A21. [Google Scholar] [CrossRef]
- Shi, C.; Zhu, C.; You, Y.; Zhu, G. Method of curved-shock characteristics with application to inverse design of supersonic flowfields. J. Fluid Mech. 2021, 920, A36. [Google Scholar] [CrossRef]
- Liu, C.; Xu, K.; Sun, Q.; Cai, Q. A unified gas-kinetic scheme for continuum and rarefied flows IV: Full Boltzmann and model equations. J. Comput. Phys. 2016, 314, 305–340. [Google Scholar] [CrossRef]
- Kailasanath, K. Recent developments in the research on pulse detonation engines. AIAA J. 2003, 41, 145–159. [Google Scholar] [CrossRef]
- Bussing, T.; Pappas, G. An introduction to pulse detonation engines. In Proceedings of the 32nd Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 1994; Volume 263. [Google Scholar]
- Rankin, B.; Fotia, M.; Naples, A.; Stevens, C.; Hoke, J.; Kaemming, T. Overview of performance, application, and analysis of rotating detonation engine technologies. J. Propuls. Power 2017, 33, 131–143. [Google Scholar] [CrossRef]
- Zhou, R.; Wu, D.; Wang, J. Progress of continuously rotating detonation engines. Chin. J. Aeronaut. 2016, 29, 15–29. [Google Scholar] [CrossRef]
- Ashford, S.A.; Emanuel, G. Oblique detonation wave engine performance prediction. J. Propuls. Power 1996, 12, 322–327. [Google Scholar] [CrossRef]
- Zhang, Z.; Ma, K.; Zhang, W.; Han, X.; Liu, Y.; Jiang, Z. Numerical investigation of a Mach 9 oblique detonation engine with fuel pre-injection. Aerosp. Sci. Technol. 2020, 105, 106054. [Google Scholar] [CrossRef]
- Xiong, H.; Qiu, R.; Han, X.; Hao, Y.; You, Y. Investigating the flow characteristics and thermodynamic performance of curved detonation waves. Phys. Fluids 2023, 35, 087119. [Google Scholar] [CrossRef]
- Nakayama, H.; Kasahara, J.; Matsuo, A.; Funaki, I. Front shock behavior of stable curved detonation waves in rectangular-cross-section curved channels. Proc. Combust. Inst. 2013, 34, 1939–1947. [Google Scholar] [CrossRef]













| Equilibrium Moments | Physical Meaning | Dimension (Unit) |
|---|---|---|
| M0 (feq) | Molecular mass density | kg m−3 |
| M1,i (feq) | Molecular momentum density in the i-direction | N s m−3 |
| M2,ij (feq), i = j | Molecular translational kinetic energy density in the i-direction at equilibrium | N m−2 or J m−3 |
| M2,ij (feq), i ≠ j | Moments of the first-order motion state of in the j-direction | N m−2 or J m−3 |
| M3,ijk (feq), i = j = k | Moments of the first-order motion state of fαeqeαieαi | N m−1 s−1 or J m−2 s−1 |
| M3,ijk (feq), i = j ≠ k | Moments of the first-order motion state of fαeqeαieαi in the k-direction | N m−1 s−1 or J m−2 s−1 |
| M3,ijk (feq), i ≠ j ≠ k | The moment of the first-order motion state in the j-direction and the first-order motion state in the k-direction for fαeqeαi | N m−1 s−1 or J m−2 s−1 |
| Equilibrium Moments | Physical Meaning | Dimension (Unit) |
|---|---|---|
| M0 (heq) | Total energy density of molecules | J m−3 or N m−2 |
| M1,i (heq) | The moment of the first-order motion state in the i-direction for hαeq | J m−2 s−1 or N m−1 s−1 |
| M2,ij (heq), i = j | The moment of the second-order motion state in the i-direction for hαeq | J m−1 s−2 or N s−2 |
| M2,ij (heq), i ≠ j | The moment of the first-order motion state in the i-direction and the first-order motion state in the j-direction for hαeq | J m−1 s−2 or N s−2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, R.; Yang, X.; Bao, Y.; You, Y.; Jin, H. Mesoscopic Kinetic Approach of Nonequilibrium Effects for Shock Waves. Entropy 2024, 26, 200. https://doi.org/10.3390/e26030200
Qiu R, Yang X, Bao Y, You Y, Jin H. Mesoscopic Kinetic Approach of Nonequilibrium Effects for Shock Waves. Entropy. 2024; 26(3):200. https://doi.org/10.3390/e26030200
Chicago/Turabian StyleQiu, Ruofan, Xinyuan Yang, Yue Bao, Yancheng You, and Hua Jin. 2024. "Mesoscopic Kinetic Approach of Nonequilibrium Effects for Shock Waves" Entropy 26, no. 3: 200. https://doi.org/10.3390/e26030200






