Computing Bi-Invariant Pseudo-Metrics on Lie Groups for Consistent Statistics †
Abstract
:1. Introduction
1.1. Modeling with Lie Groups
1.2. Statistics on Lie Groups
1.3. Using Riemannian and Pseudo-Riemannian Structures for Statistics on Lie Groups
1.4. Lie Groups and Lie Algebras with Bi-Invariant Pseudo-Metrics
1.5. Contributions and Outline
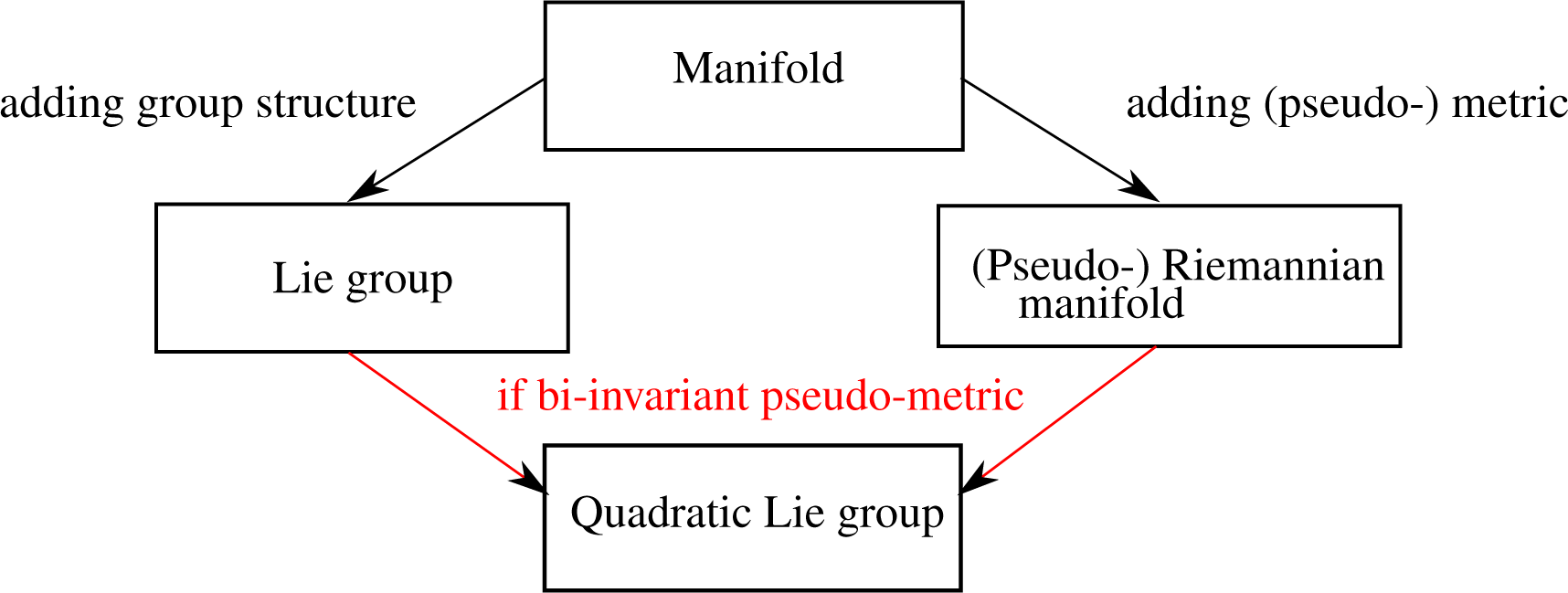
2. Introduction to Lie Groups with Bi-Invariant Pseudo-Metrics
2.1. Quadratic Lie Groups and Lie Algebras
2.1.1. Lie Groups
2.1.2. Lie Algebras
2.1.3. Pseudo-Metrics
2.1.4. Quadratic Lie Groups and Algebras
2.1.5. Characterization of Quadratic Lie Algebras
2.1.6. How to Compute Bi-Invariant Pseudo-Metrics?
2.2. Lie Algebra Representations
2.2.1. Lie Algebras Representations
2.2.2. Adjoint and Co-adjoint Representation
2.2.3. Some Vocabulary of Algebra
2.2.4. Some Vocabulary of Geometry
2.3. Constructions with Lie Algebra Representations
2.3.1. Definition of Direct Sum
- B = B1 ⊕ B2 in terms of vector spaces,
- [B, B1]B ⊂ B1 and [B, B2]B ⊂ B2, making B1 and B2 subrepresentations of the adjoint representation of B, in other words: ideals of B.
2.3.2. Direct Sum Decomposition and Bi-Invariant Pseudo-Metrics
2.3.3. Computing the Direct Sum
2.3.4. Definition of Double Extension
- B = W ⊕ S ⊕ S* in terms of vector spaces,
- (W, [, ]W) is a Lie algebra and [S, W ]B ⊂ W makes W a S-representation,
- (S, [, ]S) is a simple Lie subalgebra of B: [s, s′]B = [s, s′]S,
- S* is the dual space of S and [S, S*]B ⊂ S* makes S* the co-adjoint representation,
- ∀w, w′ ∈ W : [w, w′]B = [w, w′]W + β(w, w′) where β : Λ2W ↦S* is a (skew-symmetric)
2.3.5. Double Extension Decomposition and Bi-Invariant Pseudo-Metrics
2.3.6. Computing Double Extensions
- I is abelian,
- I⊥ is a maximal ideal,
- I ⊂ I⊥ (total isotropy),
- [I, I⊥] = 0 (commutativity),
- codim(I⊥) = dim(I).
3. Structure of Quadratic Lie Groups
3.1. A Classification Theorem
- Type (1): B is simple (or one-dimensional),
- Type (2): B = W ⊕ S ⊕ S* is a double extension of a quadratic W by S simple (or one-dimensional).
3.1.1. Elementary Bi-Invariant Pseudo-Metrics
3.2. Riemannian and Pseudo-Riemannian Quadratic Lie Groups
3.2.1. Studying the Signature
3.2.2. Comparison
3.3. From a Bi-Invariant Pseudo-Metric to a Bi-Invariant Dual Metric?
3.3.1. Dual Numbers and Vectors
3.3.2. From the Double Extension to Its Dual
3.3.3. Towards Statistics on Dual Riemannian Manifolds
3.3.4. Generalization?
4. An Algorithm to Compute Bi-Invariant Pseudo-Metrics on a Given Lie Group
4.1. The Algorithm: Computation of One Bi-Invariant Pseudo-Metric
4.1.1. Core of the Algorithm
- B is of Type (2),
- B is quadratic,
- ∀I minimal, I abelian, there is a double extension decomposition of B,
- ∃I minimal, abelian, such that there is a double extension decomposition of B.
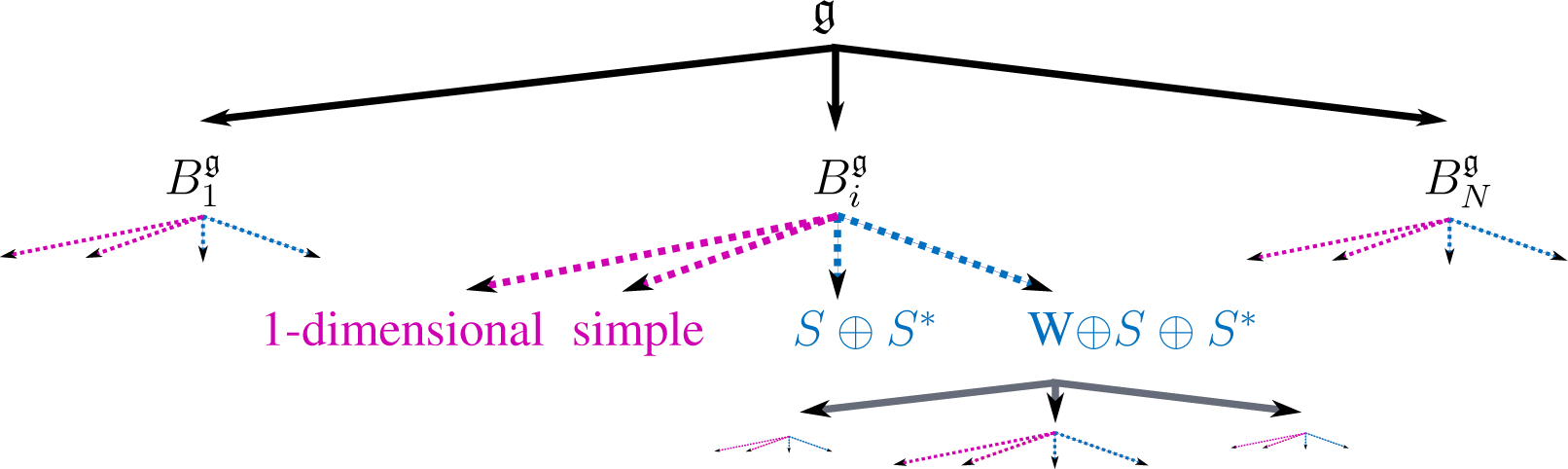
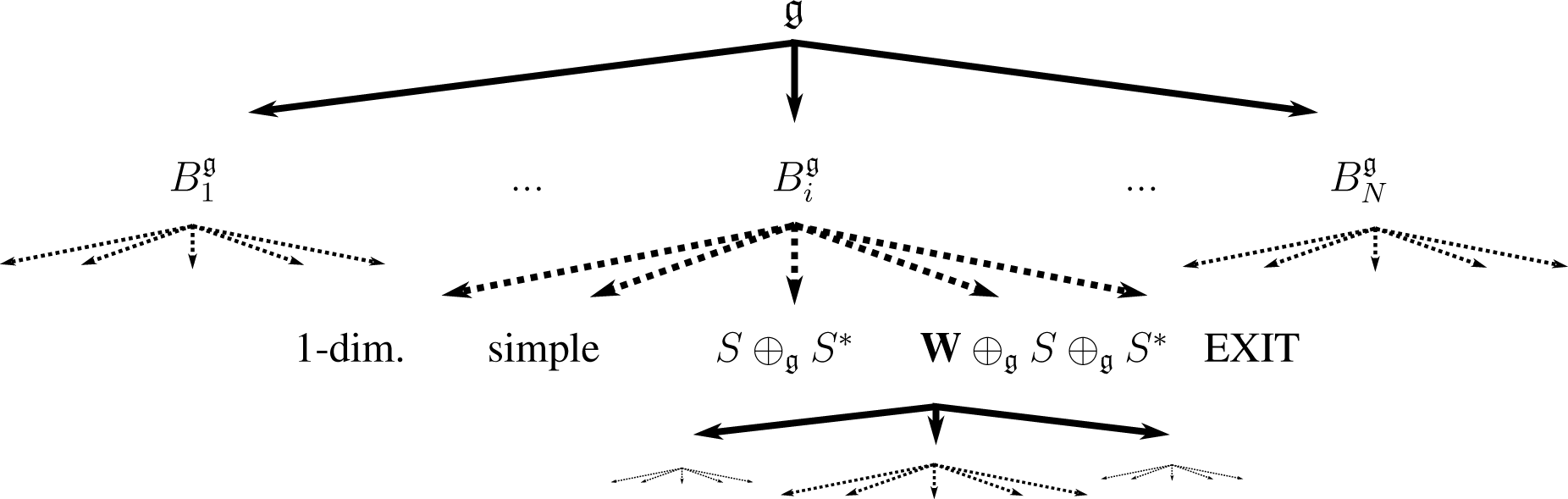
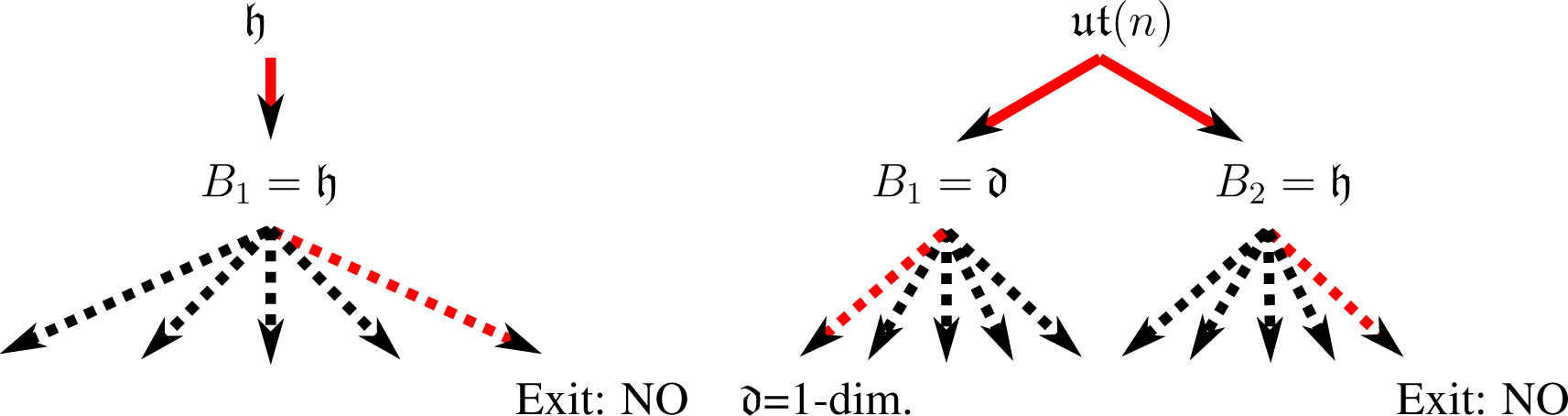
4.1.2. Tree Structure of the Algorithm
 |
4.2. Generalization of the Algorithm: Computation of All Bi-Invariant Pseudo-Metrics
4.2.1. Computing the Quadratic Space of Indecomposable Lie Algebras
4.2.2. Computing the Quadratic Space of a Direct Sum
4.3. Results of the Algorithm on Selected Lie Groups
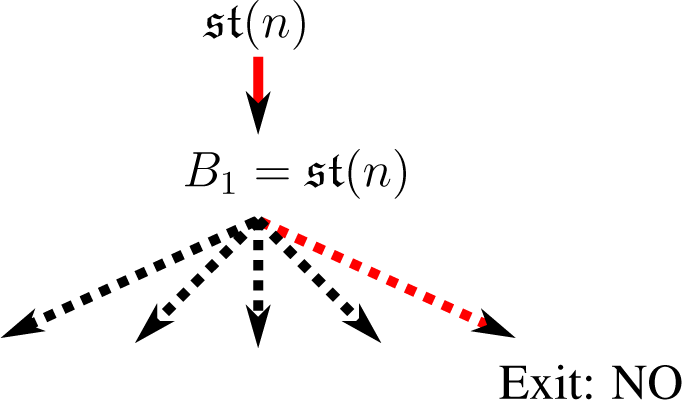
4.3.1. Scalings and Translations ST (n)
4.3.2. Heisenberg Group H
4.3.3. The Group of Scaled Upper Unitriangular Matrices UT (n)
4.3.4. Rigid Body Transformations SE(n)
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Thompson, D.W.; Bonner, J.T. On Growth and Form; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Pennec, X.; Arsigny, V. Exponential Barycenters of the Canonical Cartan Connection and Invariant Means on Lie Groups. In Matrix Information Geometry; Springer: New York, NY, USA, 2012; pp. 123–168. [Google Scholar]
- Fréchet, M. L’intégrale abstraite d’une fonction abstraite d’une variable abstraite et son application a la moyenne d’un élément aléatoire de nature quelconque; La Revue Scientifique: Paris, France, 1944. [Google Scholar]
- Fréchet, M. Les éléments aléatoires de nature quelconque dans un espace distancié. Annales de l’institut Henri Poincaré 1948, 10, 215–310. [Google Scholar]
- Karcher, H. Riemannian center of mass and mollifier smoothing. Commun. Pure Appl. Math. 1977, 30, 509–541. [Google Scholar]
- Kendall, W.S. Probability, Convexity, and Harmonic Maps with Small Image I: Uniqueness and Fine Existence. Proc. Lond. Math. Soc. 1990, s3-61, 371–406. [Google Scholar]
- Émery, M.; Mokobodzki, G. Sur le barycenter d’une probabilité dans une variété. Séminaire de probabilités de Strasbourg 1991, 25, 220–233. [Google Scholar]
- Corcuera, J.M.; Kendall, W.S. Riemannian Barycentres and Geodesic Convexity. Math. Proc. Camb. Philos. Soc. 1998, 127, 253–269. [Google Scholar]
- Huiling, L. Estimation of Riemannian Barycentres. LMS J. Comput. Math. 2004, 7, 193–200. [Google Scholar]
- Yang, L. Riemannian median and its estimation. LMS J. Comput. Math. 2010, 13, 461–479. [Google Scholar]
- Afsari, B. Riemannian Lp center of mass: existence, uniqueness, and convexity. Proc. Am. Math. Soc. 2011, 139, 655–673. [Google Scholar]
- Sternberg, S. Lectures on Differential Geometry; Prentice-Hall Mathematics Series; Prentice-Hall: Englewood Cliffs, NJ, USA, 1964. [Google Scholar]
- Cartan, E. Sur la Structure des Groupes de Transformations Finis et Continus, 2nd ed.; Vuibert.: Paris, France, 1933; p. 157 S. [Google Scholar]
- Tsou, S.T.; Walker, A.G. XIX. Metrisable Lie Groups and Algebras. Proc. R. Soc. Edinb. Sect. A Math. Phys. Sci. 1957, 64, 290–304. [Google Scholar]
- Tsou, S.T. XI. On the Construction of Metrisable Lie Algebras. Proc. R. Soc. Edinb. Sect. A Math. Phys. Sci. 1962, 66, 116–127. [Google Scholar]
- Astrakhantsev, V.V. Decomposability of metrizable Lie algebras. Funct. Anal. Appl. 1978, 12, 210–212. [Google Scholar]
- Keith, V. On Invariant Bilinear Forms on Finite-dimensional Lie Algebras. Ph.D. Thesis, Tulane University, New Orleans, LA, USA, 1984. [Google Scholar]
- Medina, A.; Revoy, P. Les groupes oscillateurs et leurs reseaux. Manuscr. Math. 1985, 52, 81–95. [Google Scholar]
- Guts, A.K.; Levichev, A.V. On the Foundations of Relativity Theory. Doklady Akademii Nauk SSSR 1984, 277, 1299–1303. [Google Scholar]
- Medina, A. Groupes de Lie munis de pseudo-métriques de Riemann bi-invariantes. Sémin. géométrie différentielle 1981-1982, Montpellier 1982, Exp. 637 p. (1982) 1982. [Google Scholar]
- Medina, A.; Revoy, P. Algèbres de Lie et produit scalaire invariant. Annales scientifiques de l’École Normale Supérieure 1985, 18, 553–561. [Google Scholar]
- Hofmann, K.H.; Keith, V.S. Invariant quadratic forms on finite dimensional lie algebras. Bull. Aust. Math. Soc. 1986, 33, 21–36. [Google Scholar]
- Bordemann, M. Nondegenerate invariant bilinear forms on nonassociative algebras. Acta Mathematica Universitatis Comenianae 1997, 66, 151–201. [Google Scholar]
- Favre, G.; Santharoubane, L. Symmetric, invariant, non-degenerate bilinear form on a Lie algebra. J. Algebra 1987, 105, 451–464. [Google Scholar]
- Campoamor-Stursberg, R. Quasi-Classical Lie Algebras and their Contractions. Int. J. Theor. Phys. 2008, 47, 583–598. [Google Scholar]
- Benayadi, S.; Elduque, A. Classification of quadratic Lie algebras of low dimension. J. Math. Phys. 2014, 55, 081703. [Google Scholar]
- Hilgert, J.; Hofmann, K. Lorentzian cones in real Lie algebras. Monatsh. Math. 1985, 100, 183–210. [Google Scholar]
- Kath, I.; Olbrich, M. Metric Lie algebras with maximal isotropic centre. Math. Z. 2004, 246, 23–53. [Google Scholar]
- Kath, I.; Olbrich, M. Metric Lie algebras and quadratic extensions. Transform. Groups 2006, 11, 87–131. [Google Scholar]
- Duong, M.T. A New Invariant of Quadratic Lie Algebras and Quadratic Lie Superalgebras. Ph.D. Theses, Université de Bourgogne, Dijon, France, 2011. [Google Scholar]
- Drinfeld, V.G. Quantum Groups; American Mathematics Society: Providence, RI, USA, 1987; pp. 798–820. [Google Scholar]
- Belavin, A.; Drinfeld, V. Triangle Equations and Simple Lie Algebras. In Math. Phys. Rev.; Harwood Academic: Newark, NJ, USA, 1998; Volume 4. [Google Scholar]
- Delorme, P. Classification des triples de Manin pour les algebres de Lie reductives complexes: Avec un appendice de Guillaume Macey. J. Algebra 2001, 246, 97–174. [Google Scholar]
- Rand, D. {PASCAL} programs for identification of Lie algebras: Part 1. Radical—A program to calculate the radical and nil radical of parameter-free and parameter-dependent lie algebras. Comput. Phys. Commun. 1986, 41, 105–125. [Google Scholar]
- Rand, D.; Winternitz, P.; Zassenhaus, H. On the identification of a Lie algebra given by its structure constants. I. Direct decompositions, levi decompositions, and nilradicals. Linear Algebra Appl. 1988, 109, 197–246. [Google Scholar]
- Cohen, A.M.; Graaf, W.A.D.; Rónyai, L. Computations in finite-dimensional Lie algebras. Discret. Math. Theor. Comput. Sci. 1997, 1, 129–138. [Google Scholar]
- Ronyai, L.; Ivanyos, G.; Küronya, A.; de Graaf, W.A. Computing Levi Decompositions in Lie algebras. Appl. Algebra Eng. Commun. Comput. 1997, 8, 291–303. [Google Scholar]
- De Graaf, W. Lie Algebras: Theory and Algorithms; North-Holland Mathematical Library; Elsevier: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Postnikov, M. Geometry VI: Riemannian Geometry; Encyclopaedia of Mathematical Sciences; Springer: New York, NY, USA, 2001. [Google Scholar]
- Bourbaki, N. Lie Groups and Lie Algebras; Springer: Paris, France, 1989; Chapters 1–3. [Google Scholar]
- Milnor, J. Curvatures of left invariant metrics on lie groups. Adv. Math. 1976, 21, 293–329. [Google Scholar]
- Bartels, R.H.; Stewart, G.W. Solution of the Matrix Equation AX + XB = C [F4]. Commun. ACM 1972, 15, 820–826. [Google Scholar]
- Kitagawa, G. An algorithm for solving the matrix equation X = FXF T + S. Int. J. Control 1977, 25, 745–753. [Google Scholar]
- Grünwald, J. Über duale Zahlen und ihre Anwendung in der Geometrie. Monatsh. Math. Phys. 1906, 17, 81–136. [Google Scholar]
- Levi, E. Sulla struttura dei gruppi finiti e continui; Atti della Reale Accademia delle Scienze di Torino: Turin, Italy, 1905; Volume 40, pp. 551–565. [Google Scholar]
- Ceballos, M.; Núñez, J.; Tenorio, A.F. Algorithmic Method to Obtain Abelian Subalgebras and Ideals in Lie Algebras. Int. J. Comput. Math. 2012, 89, 1388–1411. [Google Scholar]
- Motsak, O. Computation of the Central Elements and Centralizers of Sets of Elements in Non-Commutative Polynomial Algebras. Ph.D. Thesis, Technische Universität Kaiserslautern, Kaiserslautern, Germany, 2006. [Google Scholar]
- Schur, I. Neue Begründung der Theorie der Gruppencharaktere; Sitzungsberichte der Königlich Preußischen Akademie der Wissenschaften zu Berlin: Berlin, Germany, 1905; pp. 406–432. [Google Scholar]
- Frobenius, G. Ueber lineare Substitutionen und bilineare Formen. J. Reine Angew. Math. 1877, 87, 1–63. [Google Scholar]
- Brooksbank, P.A.; Luks, E.M. Testing isomorphism of modules. J. Algebra 2008, 320, 4020–4029. [Google Scholar]
- Karger, A.; Josef, N. Space Kinematics and Lie Groups; Gordon and Breach Science Publishers: New York, NY, USA, 1985; Translation of: Prostorová kinematika a Liehovy grupy. [Google Scholar]
- Kenwright, B. A Beginners Guide to Dual-Quaternions: What They Are, How They Work, and How to Use Them for 3D Character Hierarchies, Proceedings of the The 20th International Conference on Computer Graphics, Visualization and Computer Vision, Plzen, Czech, 25–28 June 2012; pp. 1–13.



© 2015 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miolane, N.; Pennec, X. Computing Bi-Invariant Pseudo-Metrics on Lie Groups for Consistent Statistics. Entropy 2015, 17, 1850-1881. https://doi.org/10.3390/e17041850
Miolane N, Pennec X. Computing Bi-Invariant Pseudo-Metrics on Lie Groups for Consistent Statistics. Entropy. 2015; 17(4):1850-1881. https://doi.org/10.3390/e17041850
Chicago/Turabian StyleMiolane, Nina, and Xavier Pennec. 2015. "Computing Bi-Invariant Pseudo-Metrics on Lie Groups for Consistent Statistics" Entropy 17, no. 4: 1850-1881. https://doi.org/10.3390/e17041850





