Bivariate Rainfall and Runoff Analysis Using Entropy and Copula Theories
Abstract
:1. Introduction
2. Determination of Maximum Entropy-Based Univariate Distributions
2.1. Entropy and Specification of Constraints
2.2. Entropy and Specification of Constraints
3. Bivariate Rainfall and Runoff Distribution Using Copula Theory
3.1. Dependence Measure for Bivariate Random Variables and Choice of Copulas
3.2. Estimation of Copula Parameters
3.3. Tail Dependence of Copula
3.4. Return Period of Bivariate Variables Using the Copula Theory
3.4.1. Joint Return Period “AND” Case Using Copula Theory
3.4.2. Conditional Return Period of Runoff Events Given Rainfall Events
4. Goodness-of-Fit Statistics
4.1. Goodness-of-Fit Statistics for Univariate Distribution
4.2. Goodness-of-Fit statistics for Copula
- (1)
- Estimate parameter vector for the copula function using MLE with pseudo-observations.
- (2)
- Calculate from Equation (21).
- (3)
- Determine and . The Archimedean copula family has the analytical formulation of , and thus the statistics defined in Equation (22) may be calculated directly. Otherwise the Monte Carlo simulation can be applied to approximate with the following steps:
- Generate a random sample from the fitted copula function with the sample size at least as the same length of the observed data.
- Calculate the approximated using an approach similar to Equation (21) as:
- Calculate the approximated as
- (4)
- Use parametric bootstrap procedure with a large number N to determine the associated P-value as follows:
- Generate N bivariate random samples from the fitted copula function of the observed data.
- Estimate the parameters for the fitted copula functions using the generated bivariate random samples.
- Calculate for each bivariate samples using Equation (21).
- Repeat step (3) to determine for each sample.
- Approximate the associated P-Value for the Cramér-von Mises statistic:
5. Results and Discussion
5.1. Data
- (1)
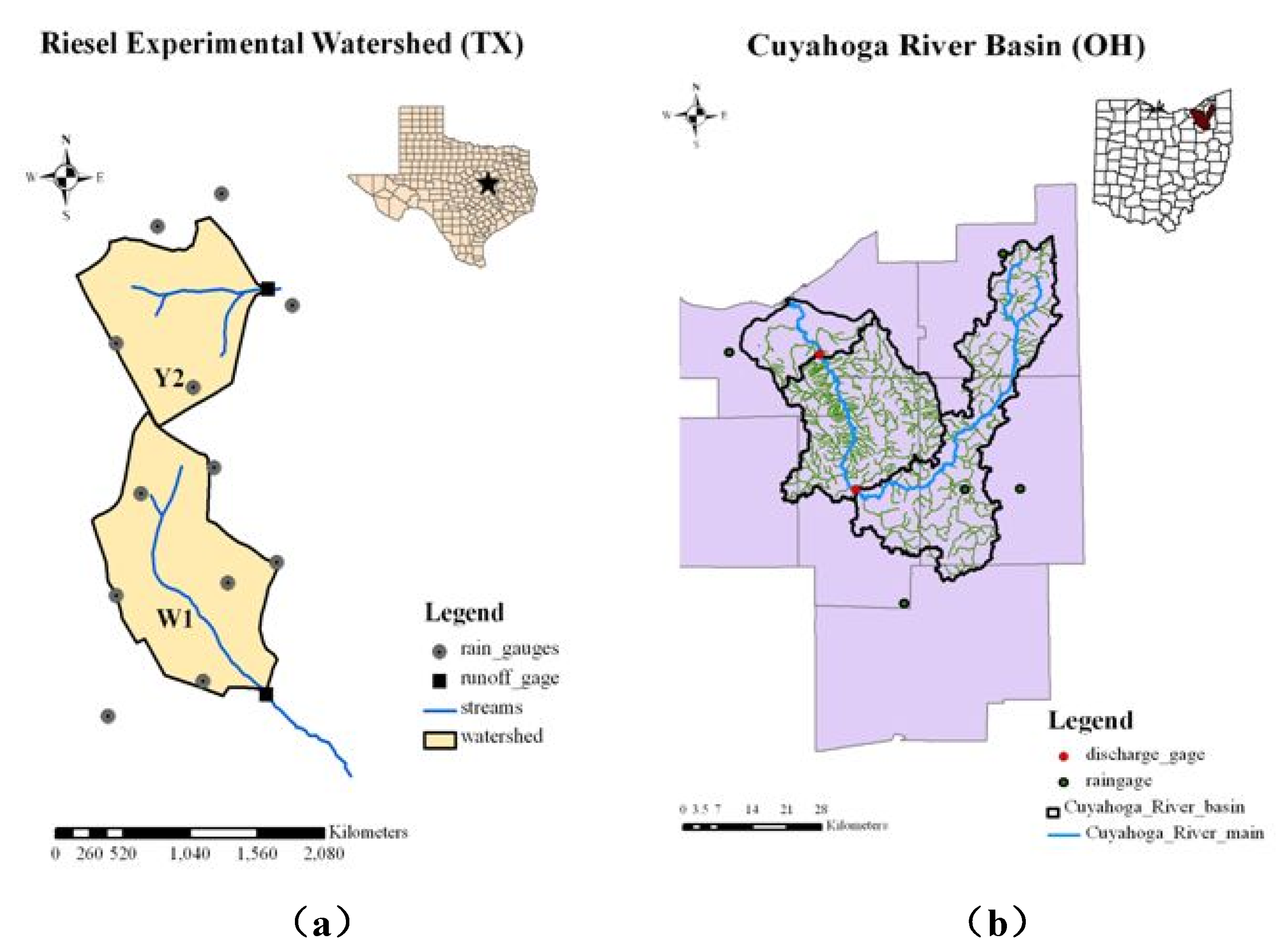
- Agricultural experimental watershed near Riesel (Waco), Texas:The experimental watersheds near Riesel (Waco) are, W1 and Y2 watersheds [Figure 1(a)] and these were selected based on the watershed area and the length of records maintained. There are multiple raingages in both watersheds, so the Thiessen polygon method was applied to determine daily areal rainfall depth. The Thiessen polygon weights and daily rainfall and corresponding runoff were obtained from the USDA-ARS data warehouse. Furthermore, annual maximum daily rainfall amounts and the resulting daily discharges were applied for rainfall and runoff analysis.
- (2)
- Cuyahoga River Watershed, Ohio:The discharge gages at Old Portage (USGS 04206000) and Independence (USGS 04208000) were selected for analysis. The digital terrain model (DTM) flow lines were obtained from USGS. The watersheds contributing to Old Portage and Independence are delineated in the Geographical Information System (GIS), as shown in Figure 1(b). The raingages within the watersheds were identified from the raingage information maintained by National Oceanic and Atmospheric Administration (NOAA). Again, the Thiessen polygon method was applied to determine the daily areal rainfall. The annual maximum daily rainfall amount and the resulting daily discharge were applied for rainfall and runoff analysis.
| Watersheds | Area (km2) | Rain gauge | Duration | |
|---|---|---|---|---|
| Riesel TX | W1 | 0.72 | Rgs: 75a, 89, w1b, w2, w2a, w3, w4, w5a | 1940–2011 |
| Y2 | 0.53 | Rgs: 69, 69b, 70, 75a, 84a | 1940–2011 | |
| Cuyahoga OH | Old Portage (04206000) | 1,046 | Rgs: 330058, 336949, 333780, 331458 | 1953–2011 |
| Independence (04208000) | 1,831 | Rgs: 331657, 330058, 336949, 333780, 331458 | 1953–2011 | |
| Raingages | Thiessen Polygon Weight | |
|---|---|---|
| Old Portage | Independence | |
| 330058 | 12.18% | 9.82% |
| 336949 | 48.99% | 52.00% |
| 333780 | 4.61% | 2.58% |
| 331458 | 34.22% | 19.16% |
| 331657 | N/A | 16.44% |
5.2. Entropy-Based Univariate Rainfall and Runoff Distributions
| Variables | Watershed | E[ln(X)] | E[X] | E[X2] | E[X3] | E[X4] | γ1 | γ2 |
|---|---|---|---|---|---|---|---|---|
| Rainfall (mm) | W1 | 4.40 | 86.02 | 8217.74 | 8.73E+05 | 1.03E+08 | 1.09 | 4.51 |
| Y2 | 4.41 | 86.96 | 8557.03 | 9.58E+05 | 1.21E+08 | 1.30 | 4.83 | |
| Old Portage | 3.77 | 45.71 | 2294.86 | 1.26E+05 | 7.46E+06 | 0.72 | 3.30 | |
| Independence | 3.73 | 43.71 | 2107.30 | 1.12E+05 | 6.55E+06 | 0.98 | 4.24 | |
| Runoff (m3/s) | W1 | −1.51 | 0.34 | 0.18 | 0.13 | 0.11 | 1.15 | 4.58 |
| Y2 | −2.14 | 0.23 | 0.10 | 0.06 | 0.04 | 1.43 | 5.64 | |
| Old Portage | 3.52 | 44.08 | 3146.42 | 3.17E+05 | 3.93E+07 | 1.74 | 5.99 | |
| Independence | 4.58 | 134.27 | 2.88E+04 | 8.02E+06 | 2.61E+09 | 1.16 | 3.76 |
| Variables | Watershed | λ0 | λ1 | λ2 | λ3 | λ4 | λ5 |
|---|---|---|---|---|---|---|---|
| Rainfall (mm) | W1 | 18.36 | 0.46 | −0.58 | 0.007 | −3.77E-05 | 7.09E-08 |
| Y2 | 18.08 | 0.89 | −0.64 | 0.008 | −4.15E-05 | 7.71E-08 | |
| Old portage | 8.60 | 0 | −0.22 | 0.002 | 1.38E-07 | N/A | |
| Independence | 19.28 | −0.57 | −1.01 | 0.026 | −2.60E-04 | 9.69E-07 | |
| Runoff (m3/s) | W1 | −0.74 | 0 | 0.61 | 2.37 | −0.19 | 0.004 |
| Y2 | 0.60 | 0.46 | −3.29 | 7.2 | −0.63 | 0.014 | |
| Old portage | 10.00 | −3.24 | 0.19 | −0.001 | 7.37E-07 | 6.49E-09 | |
| Independence | 5.31 | 0 | 0.001 | 1.58E-05 | 1.67E-10 | N/A |
| Variables | Watersheds | E[ln(X)] | E[X] | E[X2] | E[X3] | E[X4] |
|---|---|---|---|---|---|---|
| Rainfall (mm) | W1 | −2.39E-05 | −8.79E-07 | −8.61E-09 | −5.84E-08 | 3.09E-07 |
| Y2 | −6.33E-05 | −4.51E-06 | −7.51E-08 | −6.13E-08 | −1.97E-08 | |
| Old portage | −6.75E-03 | −9.34E-03 | −1.74E-02 | −4.17E-02 | N/A | |
| Independence | −4.39E-05 | 2.01E-06 | −8.16E-08 | −5.30E-09 | −4.05E-08 | |
| Runoff (m3/s) | W1 | −8.07E-03 | 2.88E-04 | 5.36E-07 | 2.98E-04 | 7.06E-03 |
| Y2 | 5.62E-02 | −3.02E-03 | −1.64E-03 | −3.07E-03 | −2.00E-02 | |
| Old Portage | −9.47E-10 | 1.50E-11 | 5.98E-09 | 1.34E-08 | 2.07E-08 | |
| Independence | −2.34E-02 | −3.57E-03 | −8.31E-03 | −2.90E-02 | N/A |
| Variables | Watersheds | K-S Statistics | AD statistics | RMSE | ||||
|---|---|---|---|---|---|---|---|---|
| H* | Statistics | P-value | H* | Statistics | P-Value | |||
| Rainfall (mm) | W1 | 0 | 0.07 | 0.92 | 0 | 0.19 | 0.99 | 3.49 |
| Y2 | 0 | 0.07 | 0.89 | 0 | 0.23 | 0.98 | 3.62 | |
| Old portage | 0 | 0.10 | 0.64 | 0 | 0.66 | 0.60 | 2.99 | |
| Independence | 0 | 0.08 | 0.81 | 0 | 0.37 | 0.88 | 2.03 | |
| Runoff (m3/s) | W1 | 0 | 0.08 | 0.77 | 0 | 0.53 | 0.71 | 6.22 |
| Y2 | 0 | 0.06 | 0.97 | 0 | 0.61 | 0.64 | 6.84 | |
| Old portage | 0 | 0.08 | 0.78 | 0 | 0.39 | 0.86 | 4.70 | |
| Independence | 0 | 0.08 | 0.79 | 0 | 0.57 | 0.67 | 15.83 | |
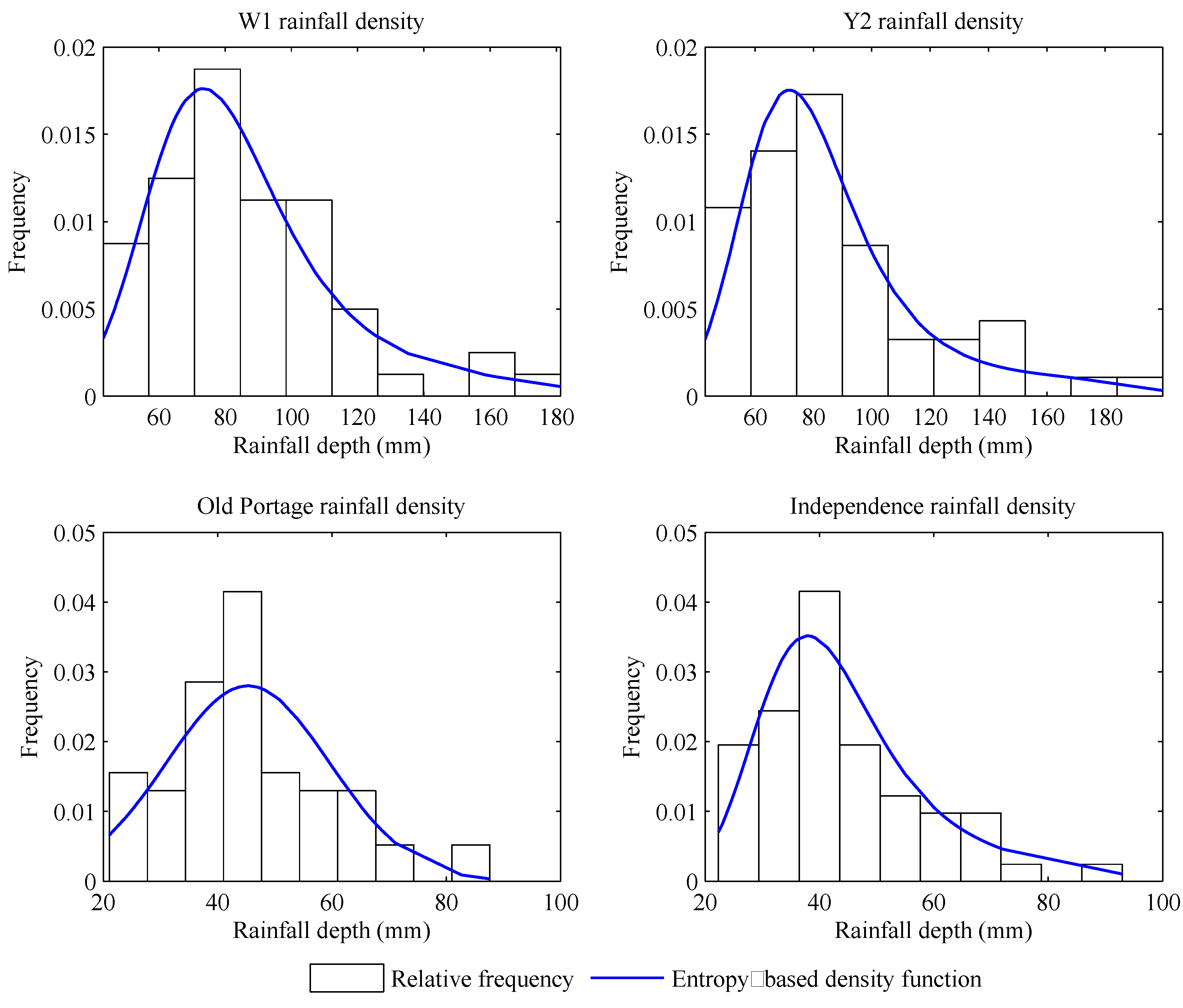
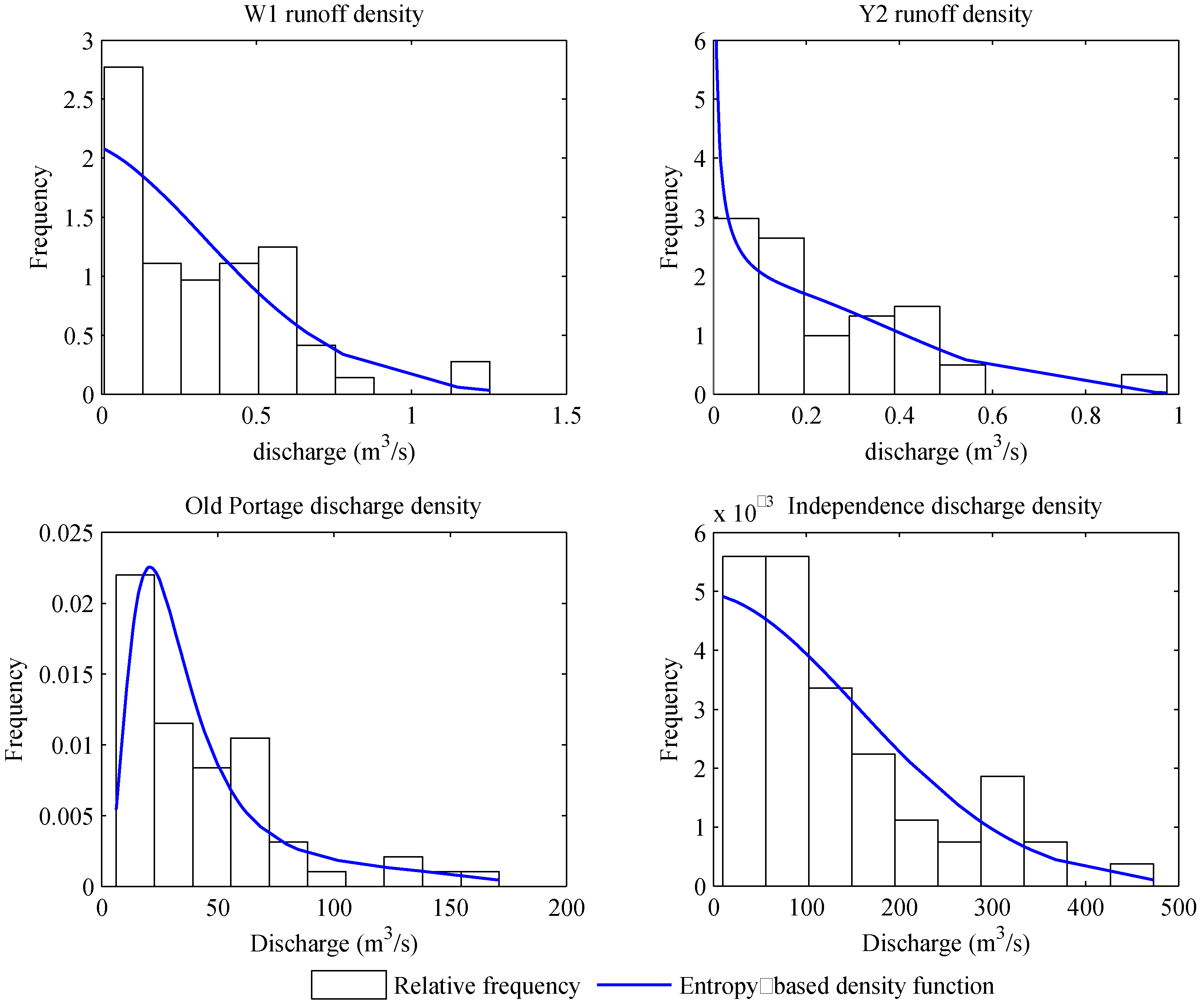
5.3. Bivariate Rainfall and Runoff Distribution
| Watersheds | Kendall’s tau | Spearman’s rho |
|---|---|---|
| W1 | 0.454 | 0.632 |
| Y2 | 0.475 | 0.646 |
| Old Portage | 0.276 | 0.394 |
| Independence | 0.397 | 0.564 |
| Copula | Estimated parameters | Likelihood | Estimated parameters | Likelihood |
|---|---|---|---|---|
| W1 | Y2 | |||
| Clayton | 0.85 | 7.10 | 0.88 | 6.93 |
| Gumbel-Hougaard | 1.73 | 13.98 | 1.86 | 17.16 |
| Frank | 4.40 | 12.26 | 4.55 | 12.92 |
| Joe | 2.10 | 13.56 | 2.42 | 17.02 |
| A12 | 1.17 | 8.44 | 1.24 | 9.38 |
| BB1[a] | (8.65E-6, 1.73) | 13.98 | (2.03E-4, 1.86) | 17.15 |
| BB5[b] | (1.21, 0.73) | 14.52 | (1.47, 0.54) | 17.21 |
| BB7[c] | (1, 0.85) | 7.10 | (1, 0.88) | 6.93 |
| Galambos | 1.04 | 14.54 | 1.16 | 17.30 |
| Plackett | 6.03 | 11.38 | 7.08 | 12.72 |
| Old Portage | Independence | |||
| Clayton | 0.62 | 4.30 | 0.96 | 8.55 |
| Gumbel-Hougaard | 1.39 | 5.64 | 1.52 | 7.79 |
| Frank | 2.64 | 4.92 | 4.15 | 10.71 |
| Joe | 1.53 | 4.83 | 1.63 | 5.29 |
| A12 | 1 | 2.97 | 1.05 | 8.65 |
| BB1 | (0.20, 1.29) | 5.89 | (0.57, 1.24) | 9.20 |
| BB5 | (1.06, 0.60) | 6 | (1.19, 0.53) | 8.22 |
| BB7 | (1, 0.62) | 4.30 | (1, 0.96) | 8.55 |
| Glambos | 0.67 | 6.02 | 0.80 | 8.22 |
| Plackett | 3.28 | 4.81 | 5.83 | 10.23 |
| Copula | Goodness-of-fit statistics | |||
|---|---|---|---|---|
| Sn | P-value | Sn | P-value | |
| W1 | Y2 | |||
| Clayton | 0.19 | 0.05 | 0.21 | 0.04 |
| Gumbel-Hougaard | 8.57 | 0.59 | 8.72 | 0.52 |
| Frank | 0.05 | 0.63 | 0.08 | 0.33 |
| Joe | 0.07 | 0.50 | 0.03 | 0.93 |
| A12 | 0.15 | 0.07 | 0.20 | 0.02 |
| BB1 | 0.05 | 0.78 | 0.06 | 0.52 |
| BB5 | 7.74 | 0.44 | 7.71 | 0.54 |
| BB7 | 8.48 | 0 | 8.40 | 0 |
| Galambos | 7.70 | 0.52 | 7.64 | 0.72 |
| Plackett | 8.10 | 0.05 | 8.03 | 0.10 |
| Old Portage | Independence | |||
| Clayton | 0.06 | 0.75 | 0.14 | 0.15 |
| Gumbel-Hougaard | 5.49 | 0.45 | 5.49 | 0.45 |
| Frank | 0.06 | 0.64 | 0.07 | 0.42 |
| Joe | 0.12 | 0.26 | 0.30 | 0.01 |
| A12 | 0.13 | 0.22 | 0.11 | 0.23 |
| BB1 | 0.05 | 0.80 | 0.10 | 0.19 |
| BB5 | 8.15 | 0.06 | 7.89 | 0.24 |
| BB7 | 8.96 | 0 | 7.92 | 0 |
| Galambos | 7.52 | 0.81 | 7.95 | 0.19 |
| Plackett | 7.83 | 0.30 | 7.51 | 0.82 |
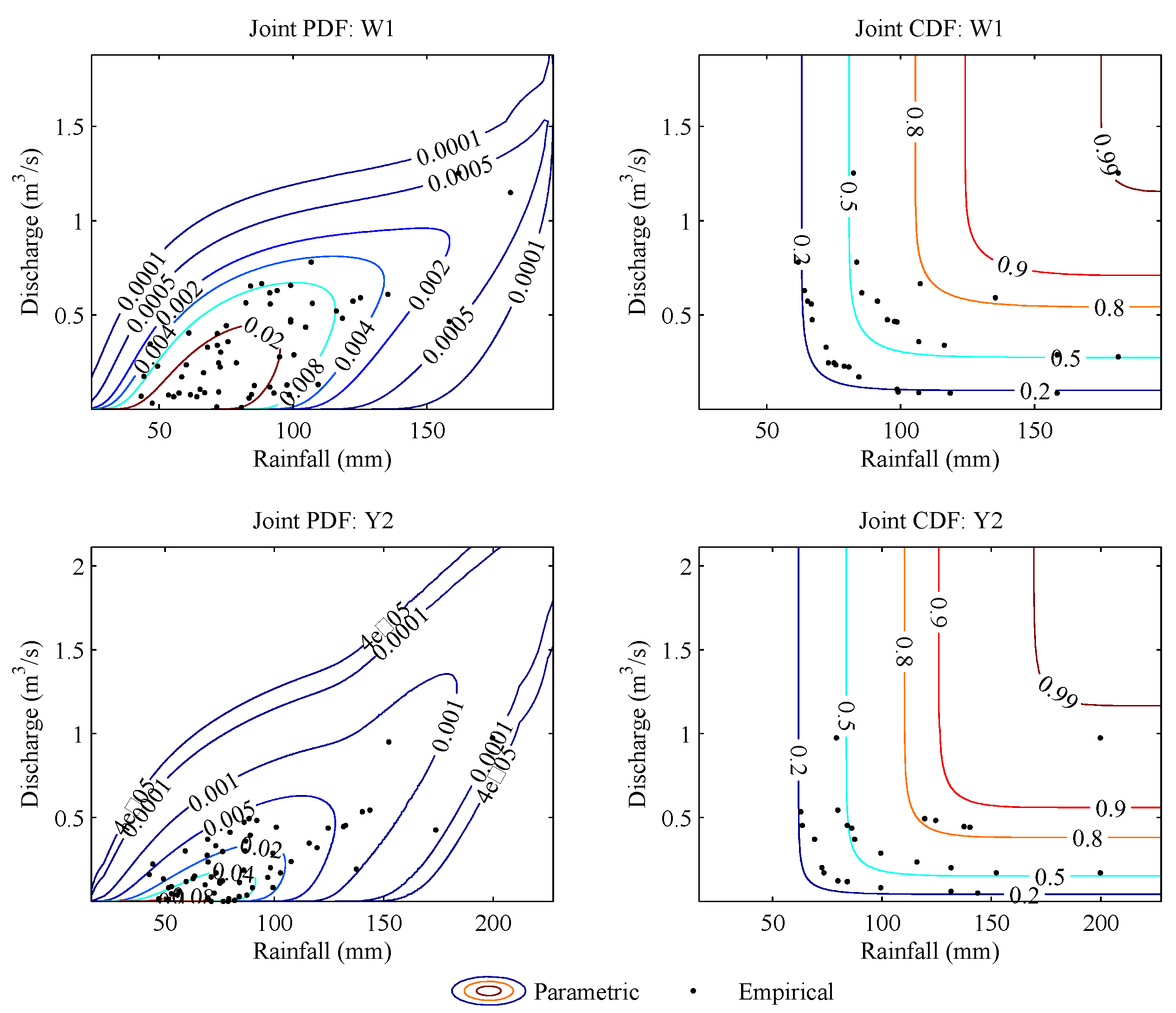
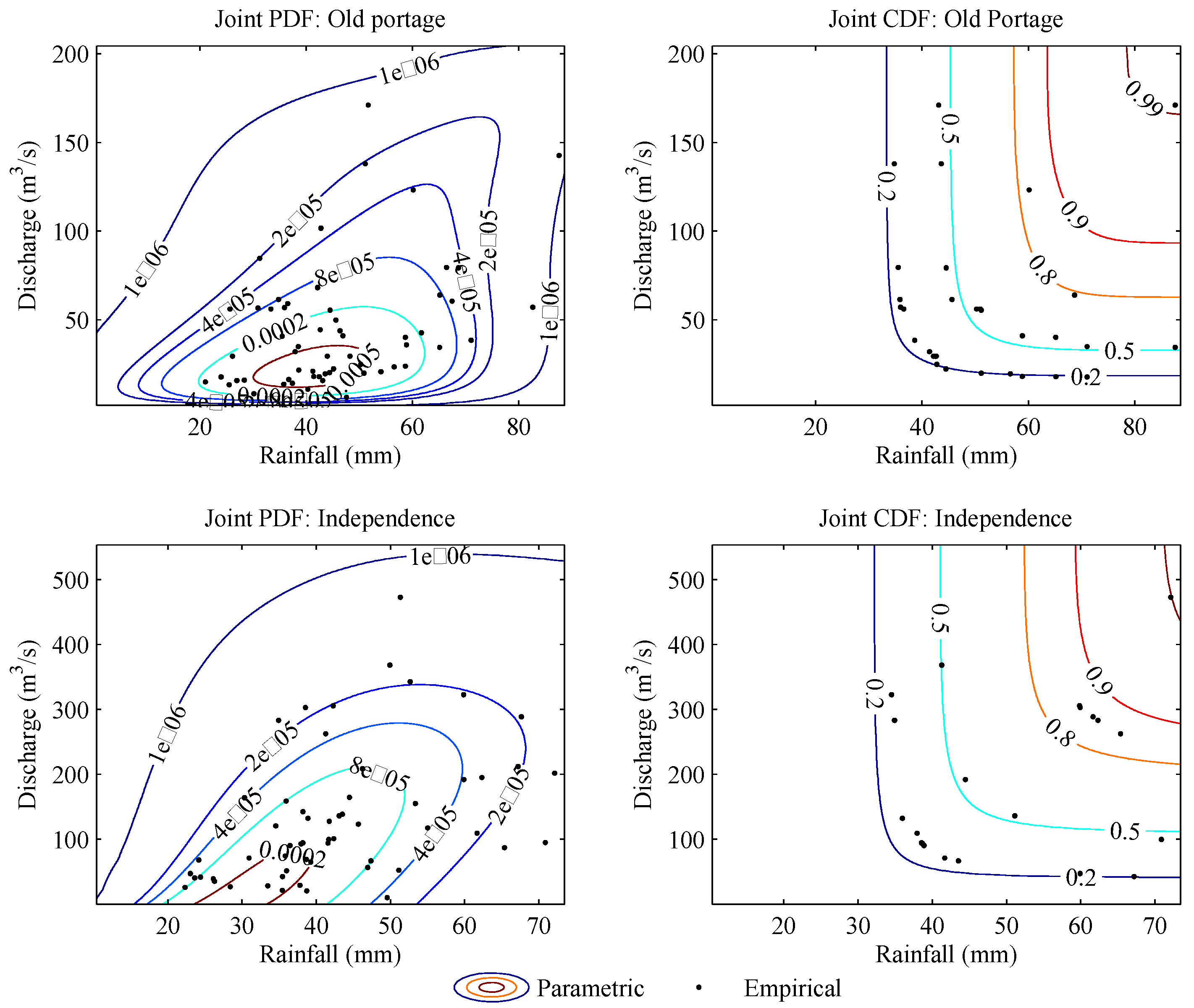
| Tail dependence | LOG [a] | SEC[a] | CFG[b] | LOG | SEC | CFG |
|---|---|---|---|---|---|---|
| W1 | Y2 | |||||
| Empirical | 0.53 | 0.56 | 0.50 | 0.58 | 0.58 | 0.56 |
| Clayton | 0 | 0 | ||||
| Gumbel-Hougaard | 0.51 | 0.55 | ||||
| Frank | 0 | 0 | ||||
| Joe | 0.61 | 0.67 | ||||
| A12 | 0.19 | 0.25 | ||||
| BB1 | 0.51 | 0.55 | ||||
| BB5 | 0.51 | 0.55 | ||||
| BB7 | 0 | 0 | ||||
| Galambos | 0.51 | 0.55 | ||||
| Placektt | 0 | 0 | ||||
| Old Portage | Independence | |||||
| Empirical | 0.36 | 0.32 | 0.35 | −0.01 | 0.02 | 0.43 |
| Clayton | 0 | 0 | ||||
| Gumbel-Hougaard | 0.35 | 0.42 | ||||
| Frank | 0 | 0 | ||||
| Joe | 0.43 | 0.47 | ||||
| A12 | 0 | 0.07 | ||||
| BB1 | 0.29 | 0.25 | ||||
| BB5 | 0.36 | 0.42 | ||||
| BB7 | 0 | 0 | ||||
| Galambos | 0.36 | 0.42 | ||||
| Plackett | 0 | 0 | ||||
5.4. Return Period of Rainfall and Runoff Events
5.4.1. Joint Return Period of Rainfall and Runoff Events
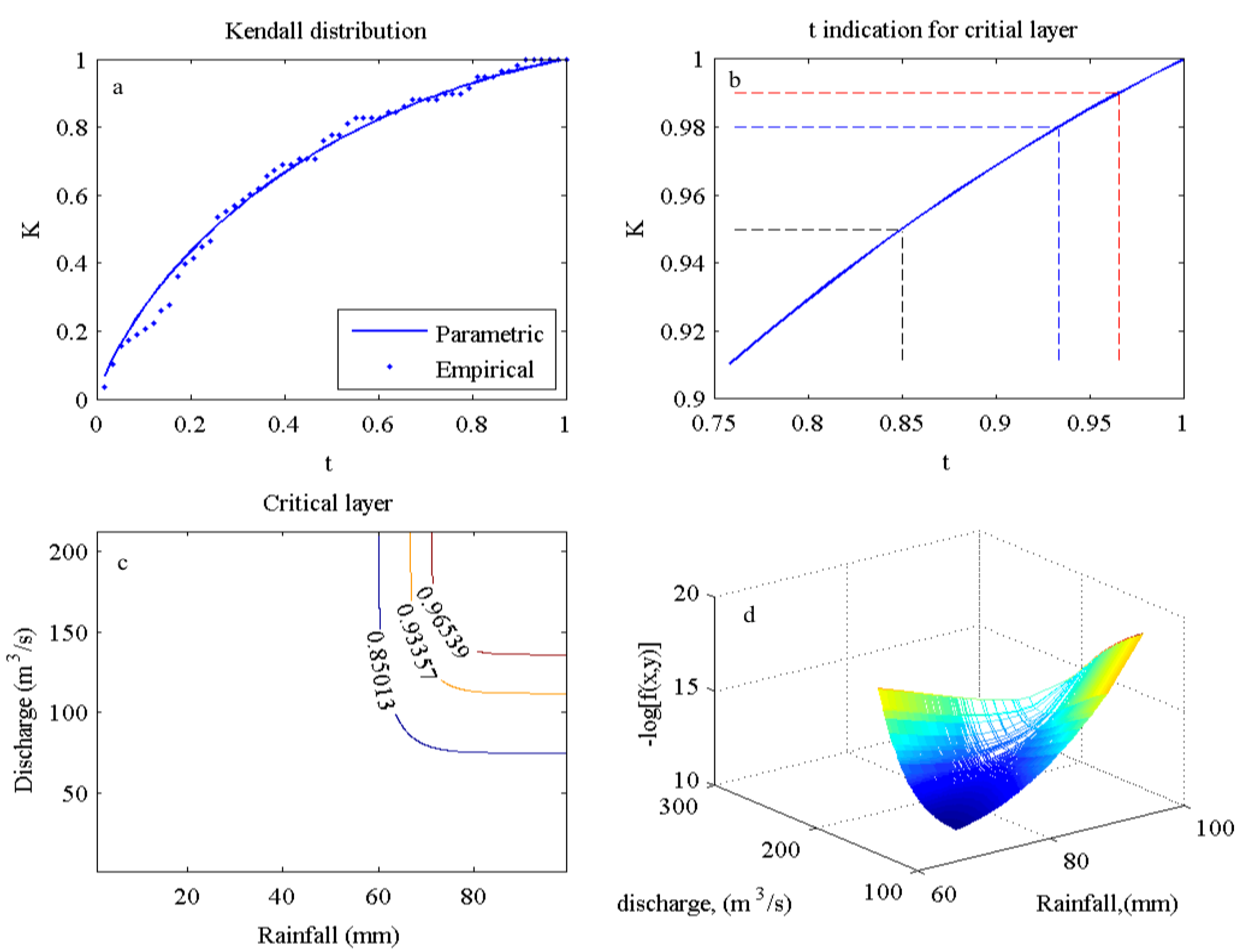
| Joint return period | 25-year | 50-year | 100-year | |||
|---|---|---|---|---|---|---|
| Rainfall | Runoff | Rainfall | Runoff | Rainfall | Runoff | |
| W1 | 122.13 | 0.69 | 145.79 | 0.88 | 161.27 | 1.01 |
| Y2 | 126.06 | 0.51 | 157.31 | 0.66 | 176.19 | 0.84 |
| Old Portage | 60.09 | 74.75 | 66.75 | 111.8 | 71.25 | 135.94 |
| Independence | 54.59 | 237.13 | 60.84 | 296.35 | 64.38 | 329.38 |
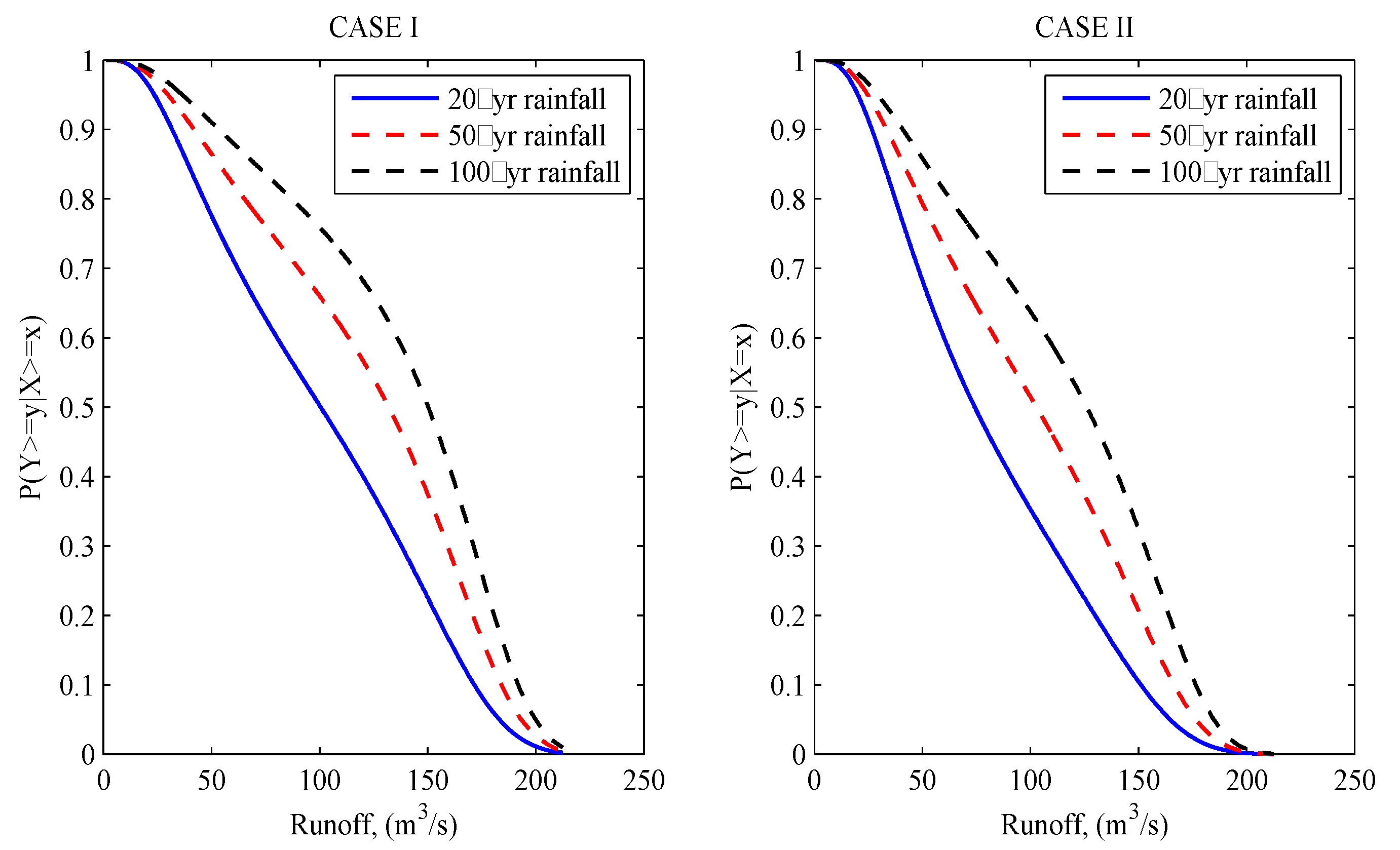
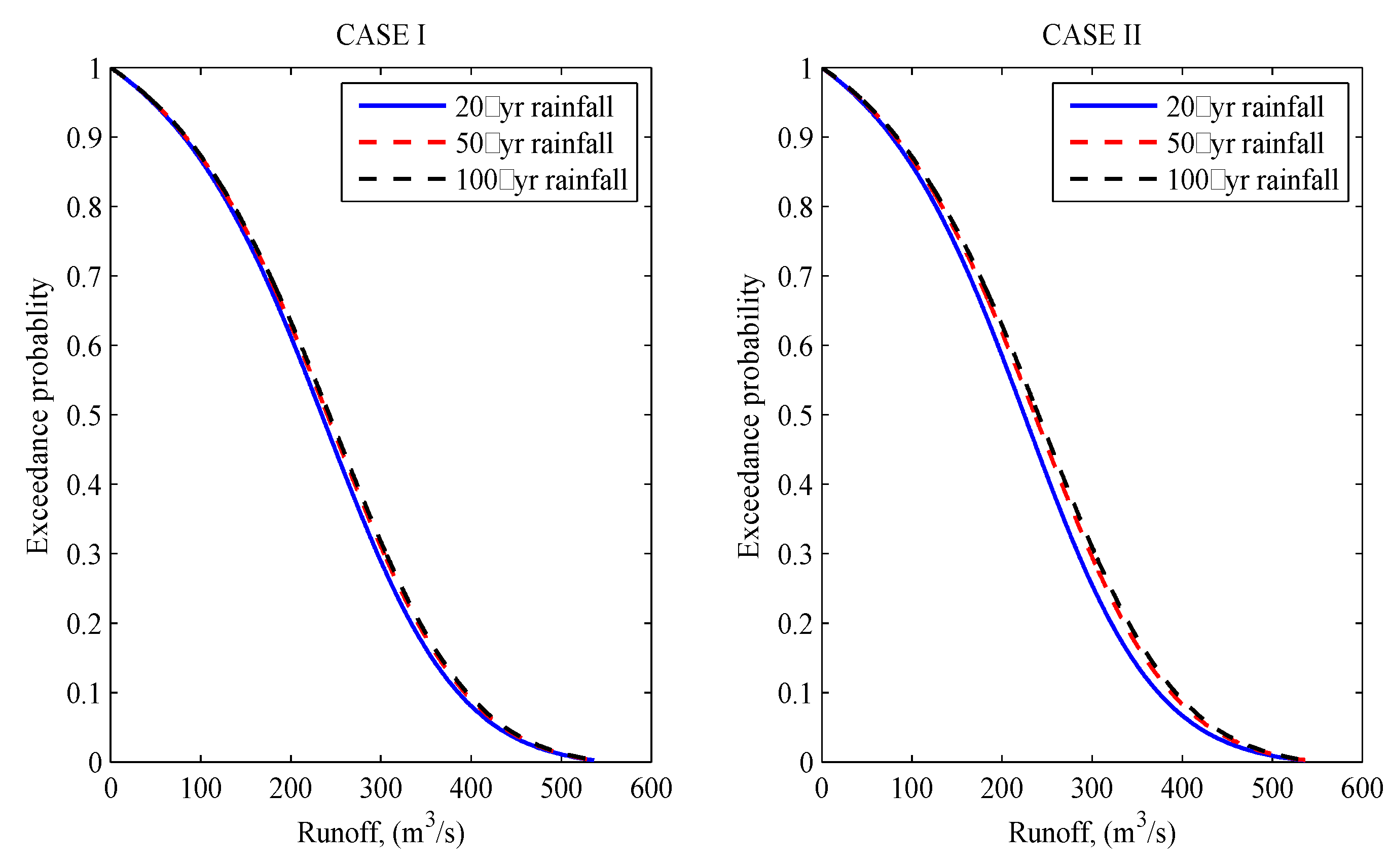
5.4.2. Conditional Return Period of Runoff Events of Given Rainfall Events
| Watersheds | Return period | ||
|---|---|---|---|
| 25-year | 50-year | 100-year | |
| W1 | 142.87 | 163.59 | 174.83 |
| Y2 | 155.04 | 178.58 | 189.97 |
| Old Portage | 68.75 | 74.57 | 78.44 |
| Independence | 64.72 | 69.37 | 71.31 |
| Watersheds | Return period | ||
|---|---|---|---|
| 25-year | 50-year | 100-year | |
| Case I | |||
| W1 | 1.37 | 1.64 | 1.8 |
| Y2 | 1.1 | 1.32 | 1.46 |
| Old Portage | 183.09 | 202.49 | 212.28 |
| Independence | 429.12 | 481.02 | 508.52 |
| Case II | |||
| W1 | 1.14 | 1.36 | 1.51 |
| Y2 | 0.89 | 1.08 | 1.21 |
| Old Portage | 164.54 | 186.32 | 198.06 |
| Independence | 417.64 | 477.16 | 507.03 |
6. Conclusions
- (1)
- The rainfall and runoff variables are fat tailed except for rainfall variable at Old Portage and runoff variable at Independence. Thus, except for these two cases, the fourth non-central moment is necessary to be considered as one of the constraints for the derivation of maximum entropy-based distribution. The maximum entropy-based univariate distribution can successfully model the rainfall and runoff variables, and it also provides the universal solution for the univariate rainfall and runoff frequency analysis.
- (2)
- The copula functions capturing the positive dependence structure may appropriately model the bivariate rainfall and runoff distribution. The Galambos copula (belonging to extreme value copula family) appropriately models the dependence between rainfall and runoff variables for watersheds W1, Y2 and Old Portage based on the MLE and formal goodness-of-fit statistics. Similarly, the Plackett copula appropriately models the dependence for watershed Independence.
- (3)
- Upper tail dependence is found for watersheds W1, Y2, and Old Portage, and the nonparametric/parametric estimation of upper tail dependence coefficient indicates that the Galambos copula may again model the extreme events which in turn can be applied to study the joint and conditional return periods for these 3 watersheds.
- (4)
- No upper tail dependence is found for watershed Independence. It may be explained by the natural flow of the stream affected by diversion, storage reservoirs and power plants located in the watersheds. The fitted Plackett copula can be applied to study the joint and conditional return periods for watershed Independence.
- (5)
- The positive dependence structure and joint return period (“AND” case) study of the rainfall and runoff variables show that rainfall and runoff are positive quadrant dependent.
- (6)
- For watersheds W1, Y2, and Old Portage, Case I conditional return period indicates the right tail increasing (RTI) property, and Case II conditional return period indicates the stochastic increasing (SI) property. These findings are in agreement with the upper tail dependence identified for the above three watersheds.
- (7)
- For watershed Independence, Case I and II conditional return periods indicate that there does not exist RTI or SI (i.e., with given rainfall events of different return periods, the conditional exceedance probability exhibits minimal difference). This finding is in agreement with no upper tail dependence found for the watershed.
Appendix I
| Copulas | Parameters | ||
|---|---|---|---|
| One-parameter Archimedean Copula[b] | Clayton | ||
| Gumbel-Hougaard[a] | |||
| Frank | |||
| Joe[c] | |||
| A12 | |||
| Two-parameter Archimedean Copula[c] | BB1 | ||
| BB5 | |||
| BB7 | |||
| Extreme value Copula | Galambos | ||
| Others | Plackett | ||
Appendix II
| Copulas | UTD | LTD | |
|---|---|---|---|
| One-parameter Archimedean copula | Clayton | 0 | |
| Frank | 0 | 0 | |
| Joe | 0 | ||
| Gumbe-Hougaard | 0 | ||
| A12 | |||
| Two-parameter Archimedean copula | BB1 | ||
| BB5 | 0 | ||
| BB7 | |||
| Extreme value copula | Galambos | 0 | |
| Others | Plackett | 0 | 0 |
References
- Haan, C.T.; Wilson, B.N. Another look at the joint probability of rainfall and runoff. In Hydrologic Frequency Modeling, Proceedings of the International Symposium on Flood Frequency and Risk Analyses, Baton Rouge, LA, USA, May 1986; D. Reidel Publishing Company: Boston, MA, USA, 1987; pp. 555–569. [Google Scholar]
- Singh, K.; Singh, V.P. Derivation of bivariate probability density functions with exponential marginals. Stoch. Hydrol. Hydraul. 1991, 5, 55–68. [Google Scholar] [CrossRef]
- Yue, S.; Ouarda, T.B.M.J.; Bobée, B.; Legendre, P.; Bruneau, P. The Gumbel mixed model for flood frequency analysis. J. Hydrol. 1999, 226, 88–100. [Google Scholar] [CrossRef]
- Yue, S. A bivariate extreme value distribution applied to flood frequency analysis. Nord. Hydrol. 2001, 32, 49–64. [Google Scholar]
- Yue, S. The bivariate lognormal distribution to model a multivariate flood episode. Hydrol. Processes 2001, 14, 2575–2588. [Google Scholar] [CrossRef]
- Bárdossy, A.; Pegram, G.G.S. Copula based multisite model for daily precipitation simulations. Hydrol. Earth Syst. Sci. 2009, 13, 2299–2314. [Google Scholar] [CrossRef]
- Evin, G.; Favre, A.-C. A new rainfall model based on the Neyman-Scott process using cubic copulas. Water Resour. Res. 2008, 44, W03433. [Google Scholar] [CrossRef]
- De Michele, C.; Salvadori, G.; Canossi, M.; Petaccia, A.; Rosso, R. Bivariate statsitical approach to check adequacy of dam spillway. J. Hydro. Eng. 2005, 10, 1084–0699. [Google Scholar]
- Favre, A.-C.; Adlouni, E.; Perreault, L.; Monge, N.T.; Bobée, B. Multivariate hydrological frequency analysis using copulas. Water Resour. Res. 2004, 40, W01101. [Google Scholar] [CrossRef]
- Genest, C.; Favre, A.-C.; Béliveau, J.; Jacques, C. Metaelliptical copulas and their use in frequency analysis of multivariate hydrological data. Water Resour. Res. 2007, 43, W09401. [Google Scholar] [CrossRef]
- Genest, C.; Favre, A.-C. Everything you always wanted to know about copula modeling but were afraid to ask. J. Hydro. Eng. 2007, 12, 347–368. [Google Scholar] [CrossRef]
- Grimaldi, S.; Serinaldi, F. Asymmetric copula in multivariate flood frequency analysis. Adv. Water resour. 2006, 29, 1155–1167. [Google Scholar] [CrossRef]
- Kao, S.-C.; Govindaraju, R.S. A Bivariate frequency analysis of extreme rainfall with implications for design. J. Geophys. Res. 2007, 112, D13119. [Google Scholar] [CrossRef]
- Kao, S.-C.; Govindaraju, R.S. Probabilistic structure of storm surface runoff considering the dependence between average intensity and storm duration of rainfall events. Water Resour. Res. 2007, 43, W06410. [Google Scholar] [CrossRef]
- Serinaldi, F.; Bonaccroso, B.; Cancelliere, A.; Grimaldi, S. Probabilistic characterization of drought properties through copulas. J. Phys. Chem. Earth 2009, 34, 596–605. [Google Scholar] [CrossRef]
- Song, S.; Singh, V.P. Meta-elliptical copulas for drought frequency analysis of periodic hydrologic data. Stoch. Environ. Res. Risk Assess 2010, 24, 425–444. [Google Scholar] [CrossRef]
- Song, S.; Singh, V.P. Frequency analysis of droughts using the Plackett copula and parameter estimation by genetic algorithm. Stoch. Environ. Res. Risk Assess 2010, 24, 783–805. [Google Scholar] [CrossRef]
- Vandenberghe, S.; Verhoest, N.E.C.; de Baets, B. Fitting bivariate copulas to the dependence structure between storm characteristics: A detailed analysis based on 105 year 10 min rainfall. Water Resour. Res. 2010, 46, W01512. [Google Scholar] [CrossRef]
- Vandenberghe, S.; Verhoest, N.E.C.; Onof, C.; de Baets, B. A comparative copula-based bivariate frequency analysis of observed and simulated storm events: A case study on Bartlett-Lewis modeled rainfall. Water Resour. Res. 2011, 47, W07529. [Google Scholar] [CrossRef]
- Wang, C.N.; Chang, N.-B.; Yeh, G.-T. Copula-based flood frequency (COEF) analysis at the confluences of river systems. Hydrol. Process. 2009, 23, 1471–1486. [Google Scholar] [CrossRef]
- Zhang, L.; Singh, V.P. Bivariate flood frequency analysis using the copula method. J. Hydrol. Eng. 2006, 11, 150–164. [Google Scholar] [CrossRef]
- Zhang, L.; Singh, V.P. Bivariate rainfall frequency distributions using Archimedean copulas. J. Hydrol. 2007, 332, 93–109. [Google Scholar] [CrossRef]
- Zhang, L.; Singh, V.P. Gumbel-Hougaard copula for trivariate rainfall frequency analysis. J. Hydrol. Eng. 2007, 12, 409–419. [Google Scholar] [CrossRef]
- Zhang, L.; Singh, V.P. Trivariate flood frequency analysis using the Gumbel-Hougaard copula. J. Hydrol. Eng. 2007, 12, 431–439. [Google Scholar] [CrossRef]
- Agrawal, D.; Singh, J.K.; Kumar, A. Maximum Entropy-based Conditional Probability Distribution Runoff Model. Biosystem Eng. 2005, 90, 103–113. [Google Scholar] [CrossRef]
- Hao, Z.; Singh, V.P. Single-site monthly streamflow simulation using entropy theory. Water Resour. Res. 2011, 47, W09528. [Google Scholar] [CrossRef]
- Krstanovic, P.F.; Singh, V.P. A Real-Time Flood Forecasting Model Based on Maximum-Entropy Spectral Analysis: I. Development. Water Resour. Mgmt. 1993, 7, 109–129. [Google Scholar] [CrossRef]
- Krstanovic, P.F.; Singh, V.P. A Real-Time Flood Forecasting Model Based on Maximum-Entropy Spectral Analysis: II. Application. Water Resour. Mgmt. 1993, 7, 131–151. [Google Scholar] [CrossRef]
- Singh, V.P.; Krstanovic, P.F. A stochastic model for sediment yield using the principle of maximum entropy. Water Resour. Res. 1987, 23, 78l–793. [Google Scholar] [CrossRef]
- Chang, T.P. Wind speed and power density analysis based on mixture weibull and maximum entropy distributions. Int. J. Appl. Sci. Eng. 2010, 8, 39–46. [Google Scholar]
- Papalexiou, S.M.; Koutsoyiannis, D. Entropy based derivation of probability distributions: A case study to daily rainfall. Adv. Water Resour. 2012, 45, 51–57. [Google Scholar] [CrossRef]
- Singh, V.P. Entropy-Based Parameter Estimation in Hdyrology; Kluwer Academic Publishers: Boston, MA, USA, 1998. [Google Scholar]
- Singh, V.P. Entropy theory for derivation of infiltration equations. Water Resour. Res. 2010, 46, W03527. [Google Scholar] [CrossRef]
- Singh, V.P. Entropy theory for movement of moisture in soils. Water Resour. Res. 2010, 46, W03516. [Google Scholar] [CrossRef]
- Singh, V.P. Derivation of rating curves using entropy theory. Trans. ASABE 2010, 53, 1811–1821. [Google Scholar]
- Singh, V.P. Hydrologic synthesis using entropy theory: Review. J. Hydrol. Eng. 2011, 16, 421–433. [Google Scholar] [CrossRef]
- Shannon, C.E. The mathematical theory of communications. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Jaynes, E. Information theory and statistical mechanics, I. Phys. Rev. 1957, 106, 620–630. [Google Scholar] [CrossRef]
- Jaynes, E. Information theory and statistical mechanics, II. Phys. Rev. 1957, 108, 171–190. [Google Scholar] [CrossRef]
- Kapur, J.N. Maximum Entropy Models in Science and Engineering, 1st ed.; John Wiley & Sons INC.: New York, NY, USA, 1989. [Google Scholar]
- Kapur, J.N.; Kesavan, H.K. Entropy Optimization Principles with Applications, 1st ed.; Academic Press: Boston, MA, USA, 1992. [Google Scholar]
- Zellner, A.; Highfield, R.A. Calculation of maximum entropy distributions and approximation of marginal posterior distributions. J. Econometrics 1988, 37, 95–209. [Google Scholar] [CrossRef]
- Sklar, A. Fonctions de repartition à n dimensions et leurs marges. Publ. Inst. Statist. Univ. Paris 1959, 8, 229–231. [Google Scholar]
- Nelsen, R.B. An Introduction to Copulas, 2nd ed.; Springer Science+Business Media, Inc: New York, NY, USA, 2006. [Google Scholar]
- Poulin, A.; Huard, D.; Favre, A.-C.; Pugin, S. Important of tail dependence in bivariate frequency analysis. J. Hydrol. Eng. 2007, 12, 394–403. [Google Scholar] [CrossRef]
- Abberger, K. A simple graphical method to explore tail dependence in stock-return pairs. Appl. Financial Economics 2005, 15, 43–51. [Google Scholar] [CrossRef]
- Frahm, G.; Junker, M.; Schmidt, R. Estimating the tail dependence coefficient: properties and pitfalls. Insur. Math. Econ. 2005, 37, 80–100. [Google Scholar] [CrossRef]
- Cole, S.; Heffernan, J.; Tawn, J. Dependence measures for extreme value analysis. Extremes 1999, 2, 339–365. [Google Scholar] [CrossRef]
- Joe, H. Multivariate Models and Dependence Concepts, 1st ed.; Chapman & Hall/CRC: New York, USA, 1997. [Google Scholar]
- Capéraà, P.; Fougéres, A.-L.; Genest, C. Bivariate distributions with given extreme value attractor. J. Multivariate Anal. 1997, 72, 567–577. [Google Scholar] [CrossRef]
- Salvadori, G.; de Michele, C.; Durante, F. On the return period and design in a multivariate framework. Hydrol. Earth Syst. Sci. 2011, 15, 3293–3305. [Google Scholar] [CrossRef]
- Miller, L.H. Table of percentage points of Kolmogorov statistics. J. Am. Stat. Assoc. 1956, 51, 111–121. [Google Scholar] [CrossRef]
- NIST. Engineering statistics handbook. Available online: http://www.itl.nist.gov/div898/handbook/ (accessed on 12 May 2012).
- Genest, C.; Quessy, J.-F.; R Rémillard, B. Goodness-of-fit procedures for copula models based on the integral probability transformation. Scand. J. Stat. 2006, 33, 337–366. [Google Scholar] [CrossRef]
- Genest, C.; Rémillard, B.; Beaudoin, D. Goodness-of-fit tests for copulas: A review and a power study. Insur. Math. Econ. 2009, 44, 199–213. [Google Scholar] [CrossRef]
© 2012 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Zhang, L.; Singh, V.P. Bivariate Rainfall and Runoff Analysis Using Entropy and Copula Theories. Entropy 2012, 14, 1784-1812. https://doi.org/10.3390/e14091784
Zhang L, Singh VP. Bivariate Rainfall and Runoff Analysis Using Entropy and Copula Theories. Entropy. 2012; 14(9):1784-1812. https://doi.org/10.3390/e14091784
Chicago/Turabian StyleZhang, Lan, and Vijay P. Singh. 2012. "Bivariate Rainfall and Runoff Analysis Using Entropy and Copula Theories" Entropy 14, no. 9: 1784-1812. https://doi.org/10.3390/e14091784
APA StyleZhang, L., & Singh, V. P. (2012). Bivariate Rainfall and Runoff Analysis Using Entropy and Copula Theories. Entropy, 14(9), 1784-1812. https://doi.org/10.3390/e14091784





