3D-Modulational Stability of Envelope Soliton in a Quantum Electron–Ion Plasma—A Generalised Nonlinear Schrödinger Equation
Abstract
:1. Introduction
2. Methods and Materials
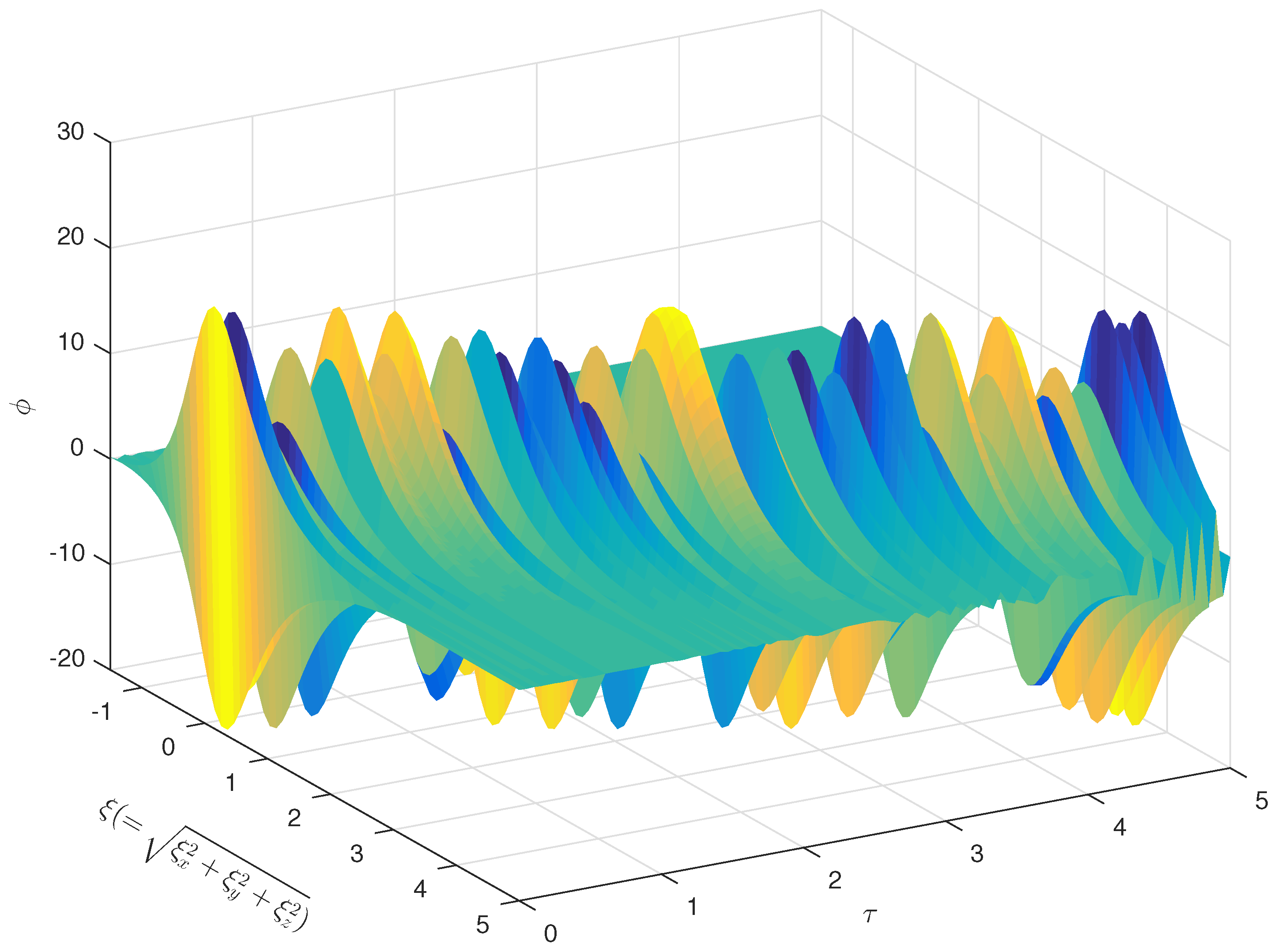
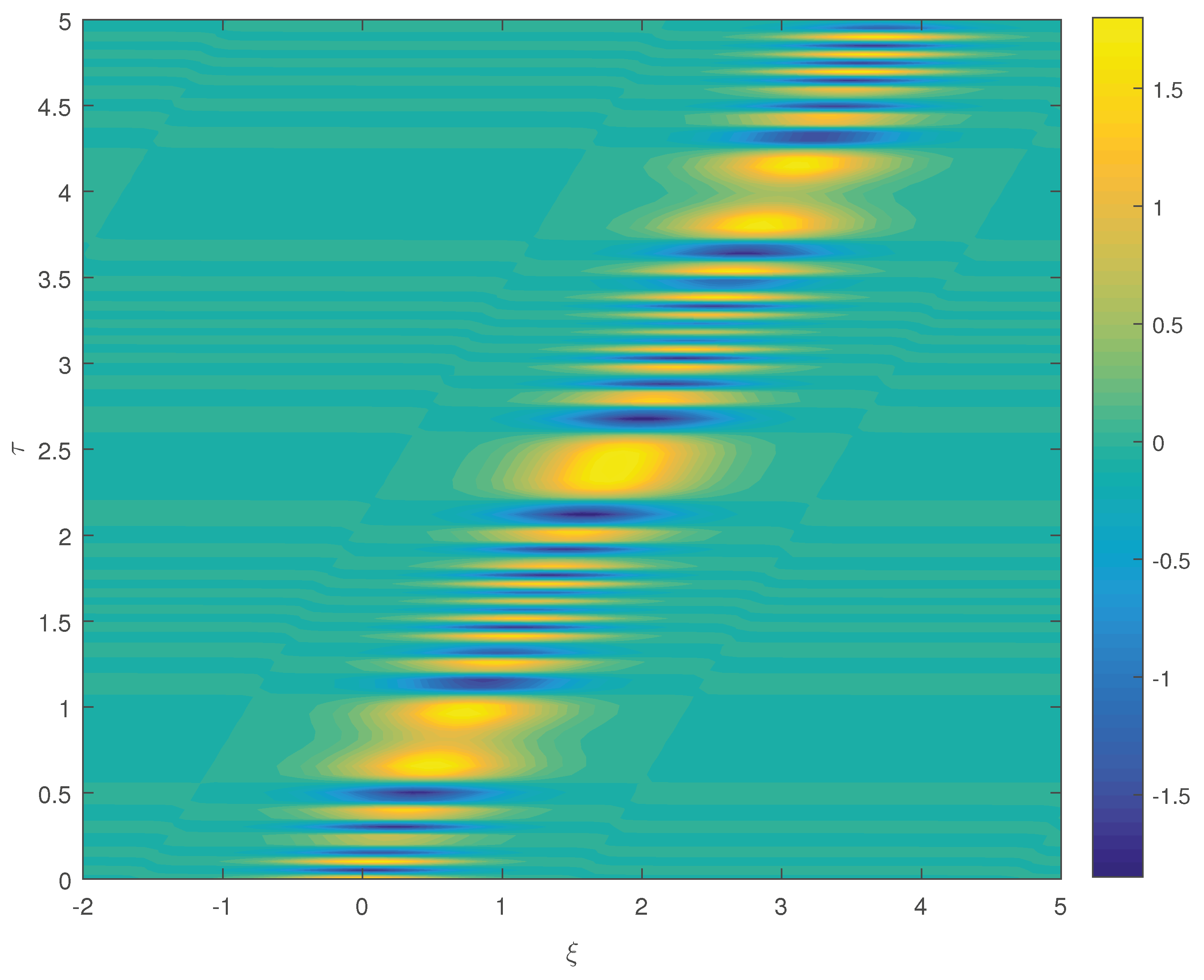
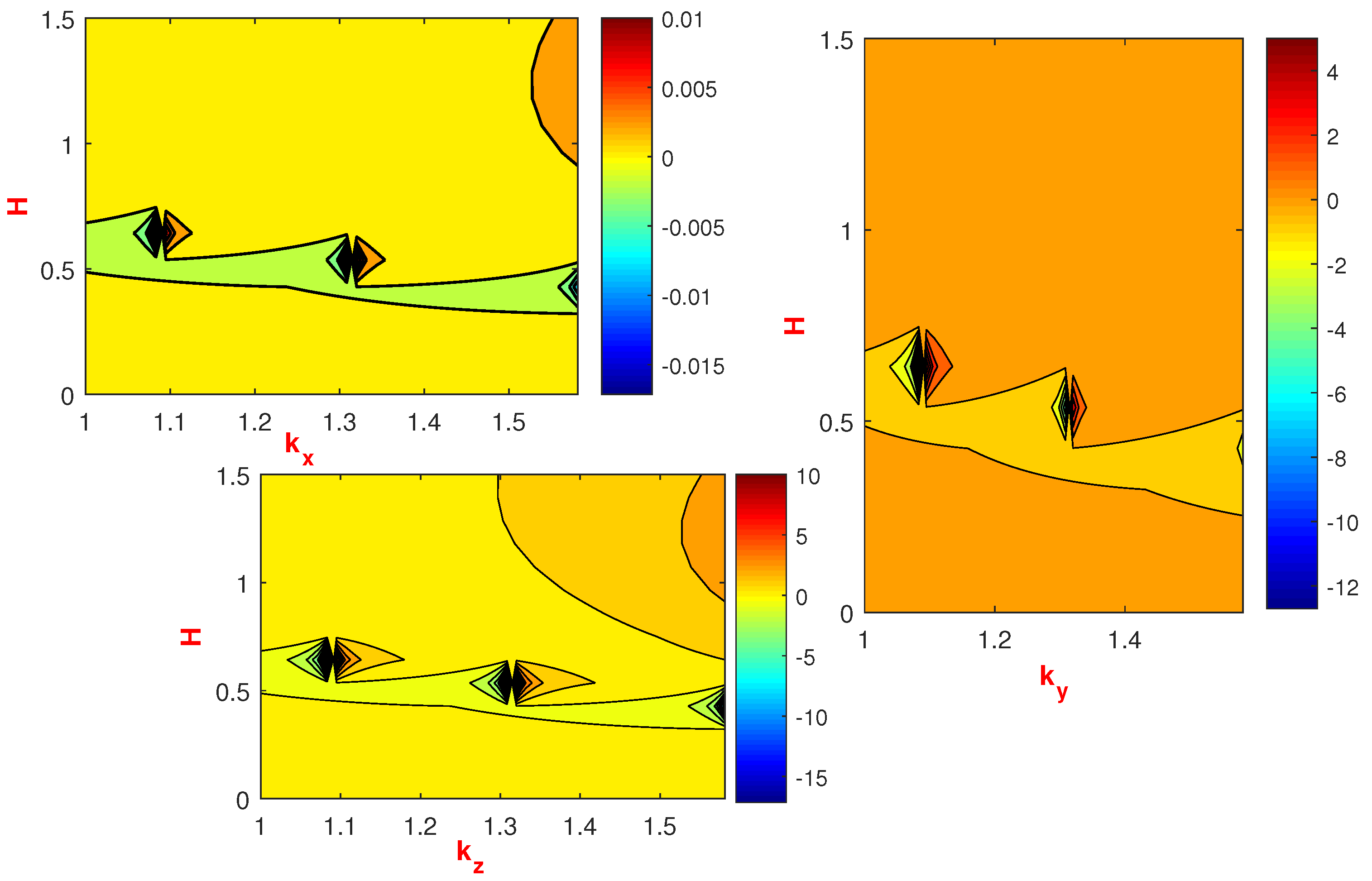
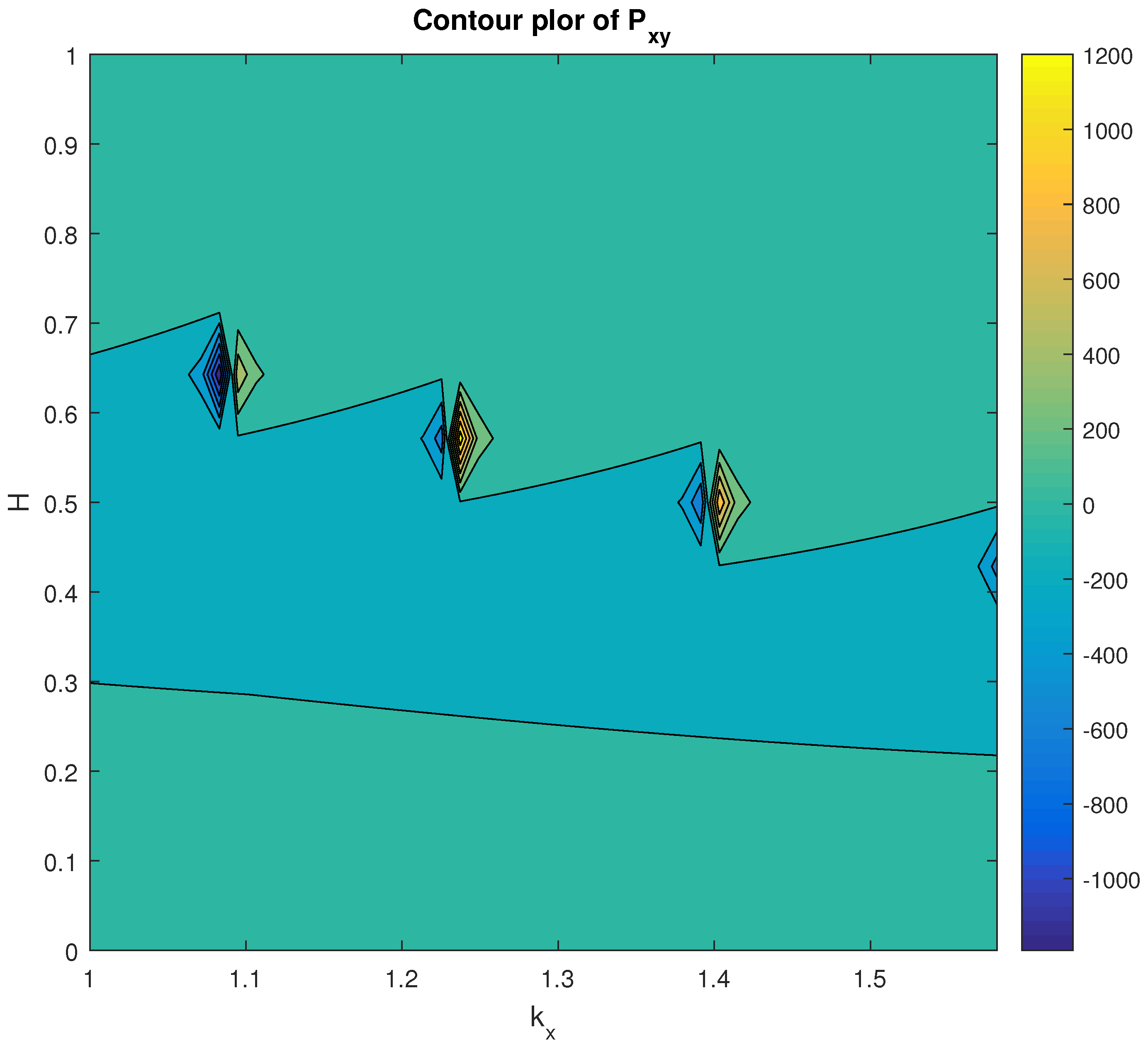
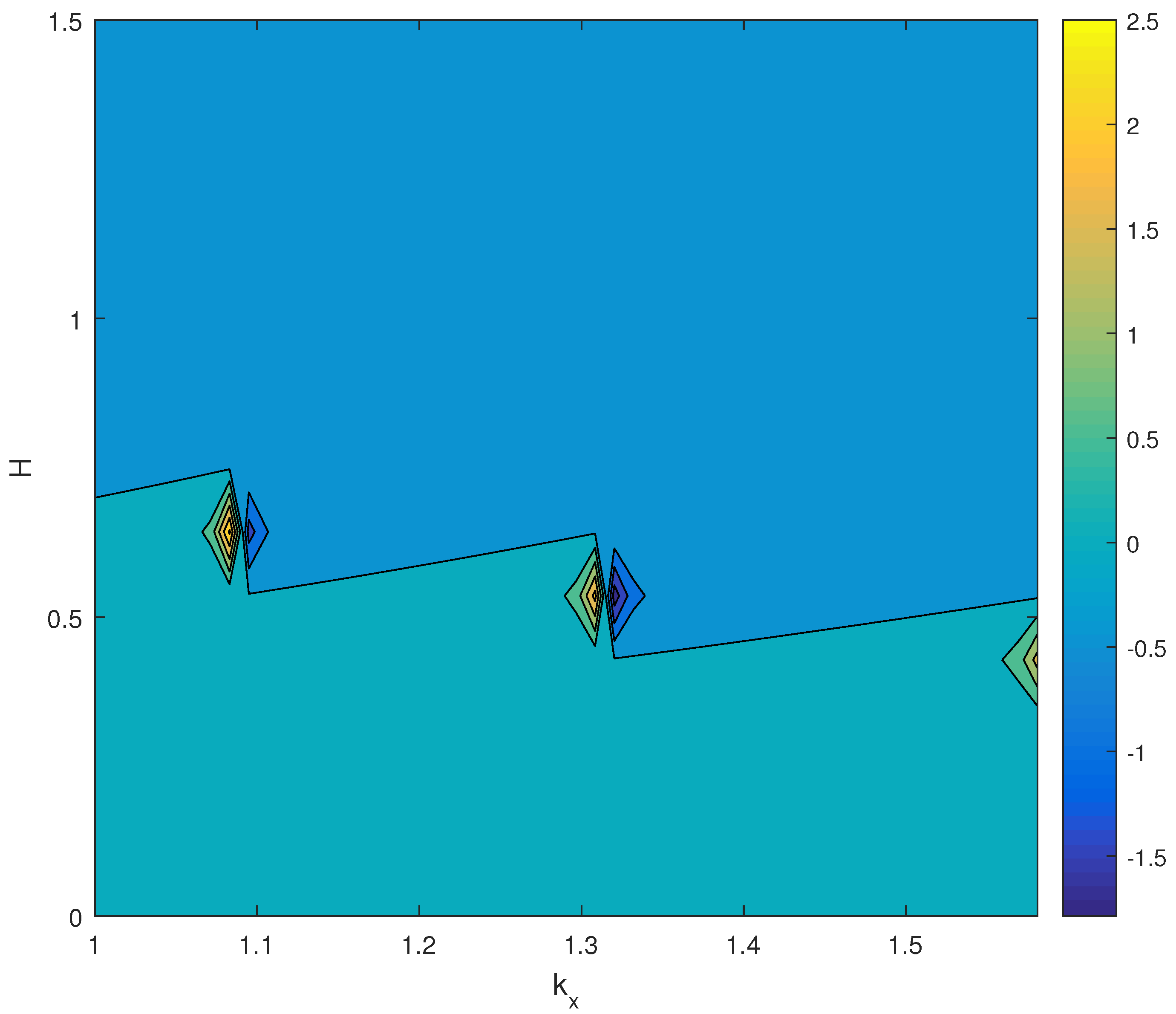
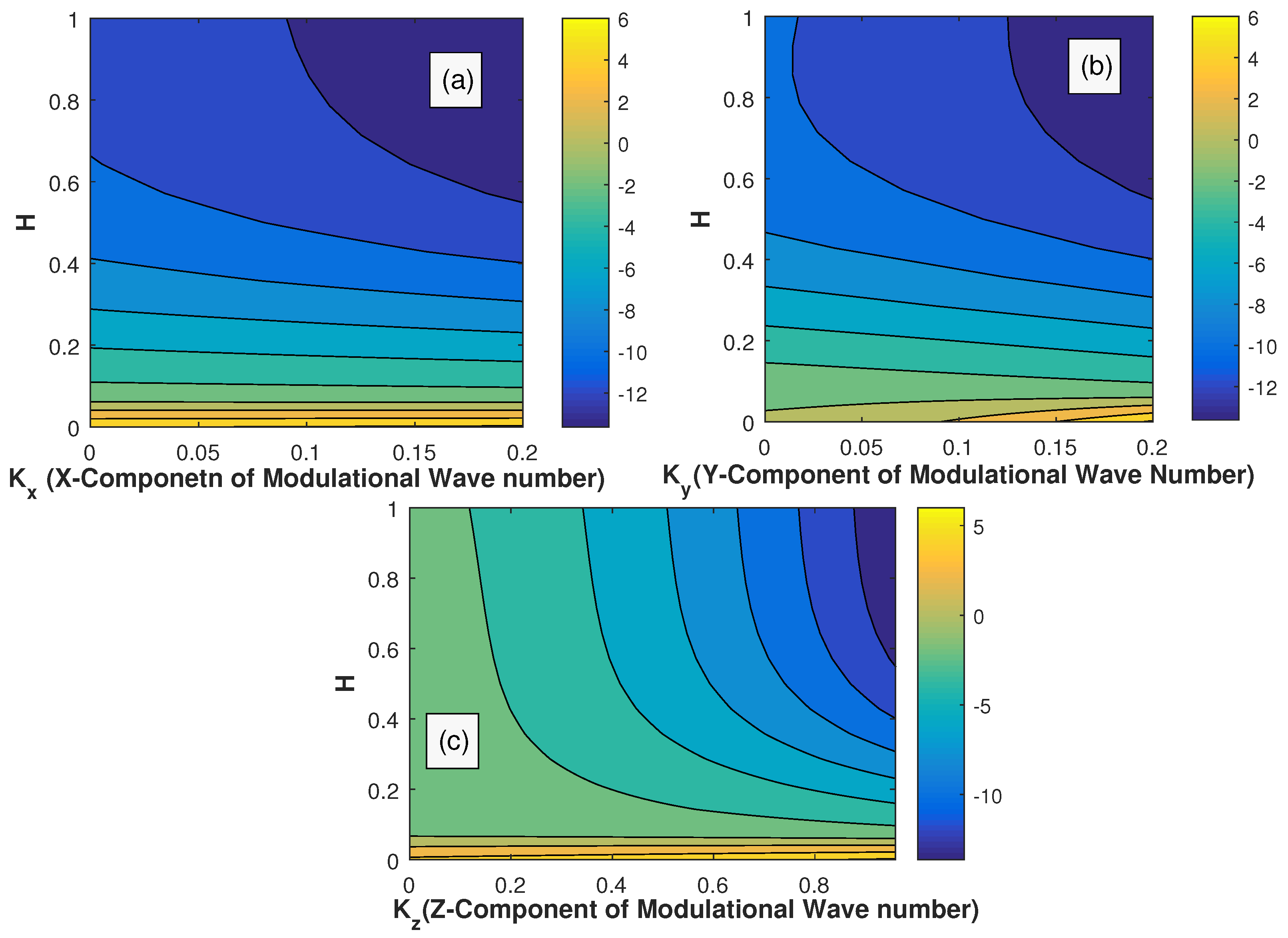
Dispersion and Stability
3. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| K B M | Krylov–Bogoliubov–Mitropolsky |
| NLSE | nonlinear Schrödinger equation |
| TLA | three-letter acronym |
| LD | linear dichroism |
Appendix A
References
- Haas, F. Quantum Plasmas: An Hydrodynamic Approach, 1st ed.; Springer Series on Atomic, Optical, and Plasma Physics 65; Springer: New York, NY, USA, 2011. [Google Scholar]
- Manfredi, G. How to model quantum plasma. Fields Inst. Commun. 2005, 46, 263–287. [Google Scholar]
- Shukla, P.K.; Eliasson, B. Nonlinear aspects of quantum plasma physics. Physics-Uspekhi 2010, 53, 51–76. [Google Scholar] [CrossRef]
- Shukla, P.K.; Eliasson, B. Recent developments in quantum plasma physics. Plasma Phys. Control. Fusion 2010, 52, 124040. [Google Scholar] [CrossRef]
- Brodin, G.; Marklund, M.; Manfredi, G. Quantum plasma effects in the classical regime. Phys. Rev. Lett. 2008, 100, 175001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wigner, E. On the quantum correction for thermodynamic equilibrium. Phys. Rev. 1932, 40, 749–759. [Google Scholar] [CrossRef]
- Khater, M.M.; Mohamed, M.S.; Attia, R.A. On semi analytical and numerical simulations for a mathematical biological model; the time-fractional nonlinear Kolmogorov–Petrovskii–Piskunov (KPP) equation. Chaos Solitons Fractals 2021, 144, 110676. [Google Scholar] [CrossRef]
- Khater, M.M.; Mohamed, M.S.; Elagan, S.K. Diverse accurate computational solutions of the nonlinear Klein–Fock–Gordon equation. Results Phys. 2021, 23, 104003. [Google Scholar] [CrossRef]
- Khater, M.; Ahmed, A.; El-Shorbagy, M. Abundant stable computational solutions of Atangana–Baleanu fractional nonlinear HIV-1 infection of CD4+ T–cells of immunodeficiency syndrome. Results Phys. 2021, 22, 103890. [Google Scholar] [CrossRef]
- Khater, M.M.; Elagan, S.K.; Mousa, A.A.; El-Shorbagy, M.A.; Alfalqi, S.H.; Alzaidi, J.F.; Lu, D. Sub-10-fs-pulse propagation between analytical and numerical investigation. Results Phys. 2021, 25, 104133. [Google Scholar] [CrossRef]
- Khater, M.M.; Attia, R.A.; Bekir, A.; Lu, D. Optical soliton structure of the sub-10-fs-pulse propagation model. J. Opt. 2021, 50, 109–119. [Google Scholar] [CrossRef]
- Khater, M.M.; Anwar, S.; Tariq, K.U.; Mohamed, M.S. Some optical soliton solutions to the perturbed nonlinear Schrödinger equation by modified Khater method. AIP Adv. 2021, 11, 025130. [Google Scholar] [CrossRef]
- Khater, M.M.; Ahmed, A.E.S. Strong Langmuir turbulence dynamics through the trigonometric quintic and exponential B-spline schemes. AIMS Math. 2021, 6, 5896–5908. [Google Scholar] [CrossRef]
- Khater, M.M.; Mohamed, M.S.; Alotaibi, H.; El-Shorbagy, M.A.; Alfalqi, S.H.; Alzaidi, J.F.; Lu, D. Novel explicit breath wave and numerical solutions of an Atangana conformable fractional Lotka–Volterra model. Alex. Eng. J. 2021, 60, 4735–4743. [Google Scholar] [CrossRef]
- Khater, M.M.; Nofal, T.A.; Abu-Zinadah, H.; Lotayif, M.S.; Lu, D. Novel computational and accurate numerical solutions of the modified Benjamin–Bona–Mahony (BBM) equation arising in the optical illusions field. Alex. Eng. J. 2021, 60, 1797–1806. [Google Scholar] [CrossRef]
- Alshahrani, B.; Yakout, H.A.; Khater, M.M.; Abdel-Aty, A.H.; Mahmoud, E.E.; Baleanu, D.; Eleuch, H. Accurate novel explicit complex wave solutions of the (2+1)-dimensional Chiral nonlinear Schrödinger equation. Results Phys. 2021, 23, 104019. [Google Scholar] [CrossRef]
- Siddiki, F.B.; Mamun, A.A.; Amin, M.R. Modulational instability of quantum elctron-acoustic waves and associated envelope solitons in a degenerate quantum plasma. arXiv 2016, arXiv:1611.09678. [Google Scholar]
- Eliasson, B.; Shukla, P.K. Dispersion properties of electrostatic oscillations in quantum plasmas. J. Plasma Phys. 2010, 76, 7–17. [Google Scholar] [CrossRef] [Green Version]
- Shukla, P.; Eliasson, B. Colloquium: Nonlinear collective interactions in quantum plasmas with degenerate electron fluids. Rev. Mod. Phys. 2011, 83, 885. [Google Scholar] [CrossRef] [Green Version]
- Abourabia, A.M.; Shahein, R.A. Modulational instability and exact solutions of nonlinear cubic complex ginzburg-landau equation of thermodynamically open and dissipative warm ion acoustic waves system. Eur. Phys. J. Plus 2011, 126, 23. [Google Scholar] [CrossRef]
- Chaudhuri, S.; Chowdhury, A.R. Nonlinear landau damping in a relativistic electron-ion plasma–non-local nonlinear schrödinger-equation and krylov bogoliubov mitropolsky method. Phys. Scr. 2018, 93, 075601. [Google Scholar] [CrossRef]
- Chaudhuri, S.; Chowdhury, A.R. Wigner transform and stability of kinetic envelope soliton in an electron depleted dusty plasma with two temperature non-thermal ions. Phys. Scr. 2019, 94, 035601. [Google Scholar] [CrossRef]
- Keane, A.J.; Mushtaq, A.; Wheatland, M.S. Alfvén solitons in a fermionic quantum plasma. Phys. Rev. E 2011, 83, 066407. [Google Scholar] [CrossRef] [Green Version]
- Chowdhury, A.R.; Pakira, G. On a kinetic approach to the modulational stability of envelope solitons in a relativistic plasma in an external electric field. Il Nuovo Cimento D 1992, 14, 527–539. [Google Scholar] [CrossRef]
- Jehan, N.; Salahuddin, M.; Mirza, A.M. Perpendicular propagating electromagnetic envelope solitons in electron-positron-ion plasma. Phys. Plasmas 2010, 17, 052308. [Google Scholar] [CrossRef]
- Akhtar, N.; El-Taibany, W.F.; Mahmood, S.; Behery, E.E.; Khan, S.A.; Ali, S.; Hussain, S. Transverse instability of ion acoustic solitons in a magnetized plasma including q -nonextensive electrons and positrons. J. Plasma Phys. 2015, 81, 905810518. [Google Scholar] [CrossRef]
- Bogoliubov, N.; Mitropolskii, Y.A. Asymptotic Methods in the Theory of Nonlinear Oscillations; Hindustan Publishing Corporation: Delhi, India, 1961; p. 51. [Google Scholar]
- Kakutani, T.; Sugimoto, N. Krylov-Bogoliubov-Mitropolsky method for nonlinear wave modulation. Phys. Fluids 1974, 17, 1617–1625. [Google Scholar] [CrossRef]
- Benjamin, T.B.; Feir, J.E. The disintegration of wave trains on deep water part 1. theory. J. Fluid Mech. 1967, 27, 417–430. [Google Scholar] [CrossRef]
- Bespalov, V.I.; Talanov, V.I. Filamentary Structure of Light Beams in Nonlinear Liquids. Sov. J. Exp. Theor. Phys. Lett. 1966, 3, 307. [Google Scholar]
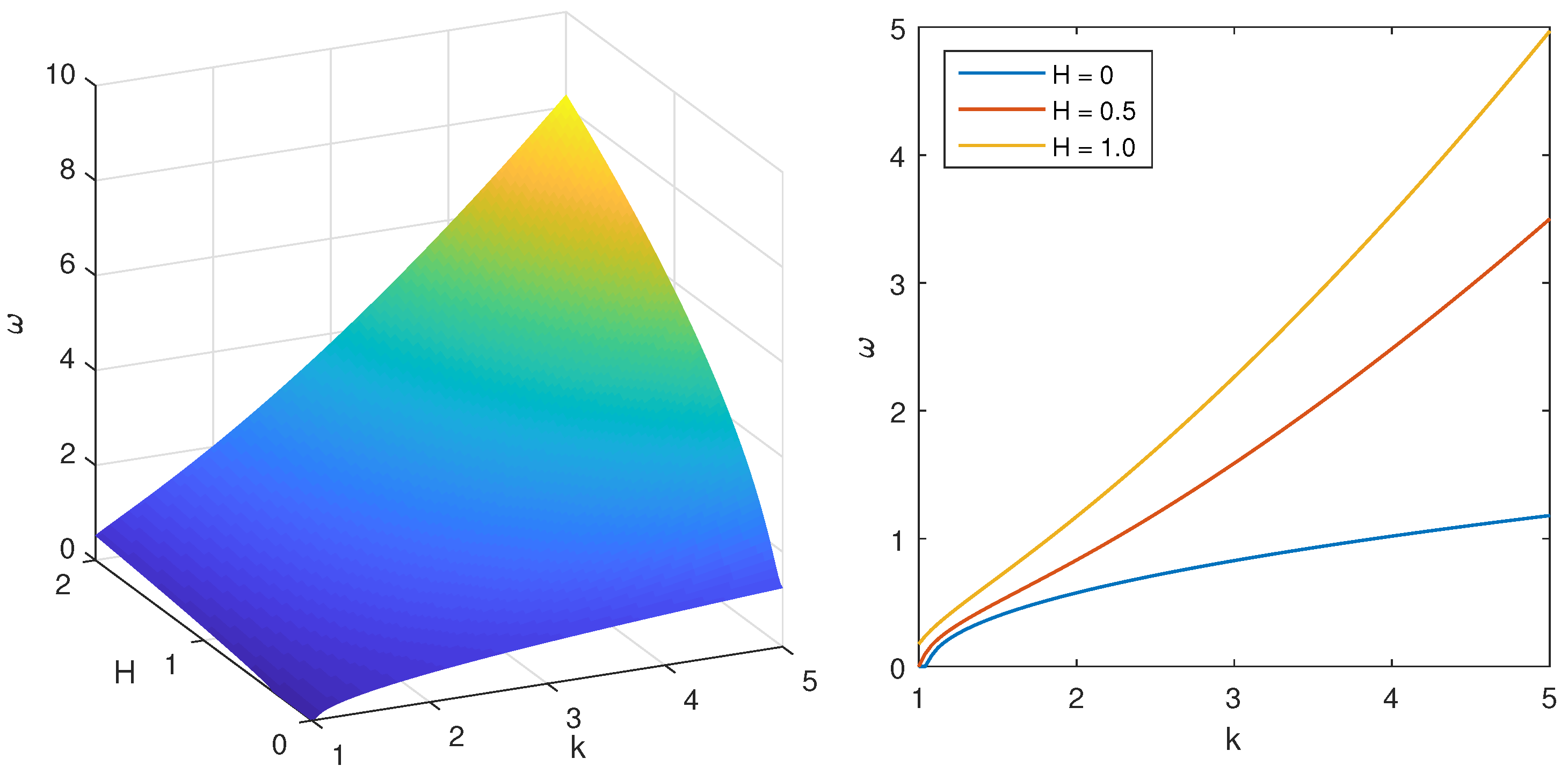
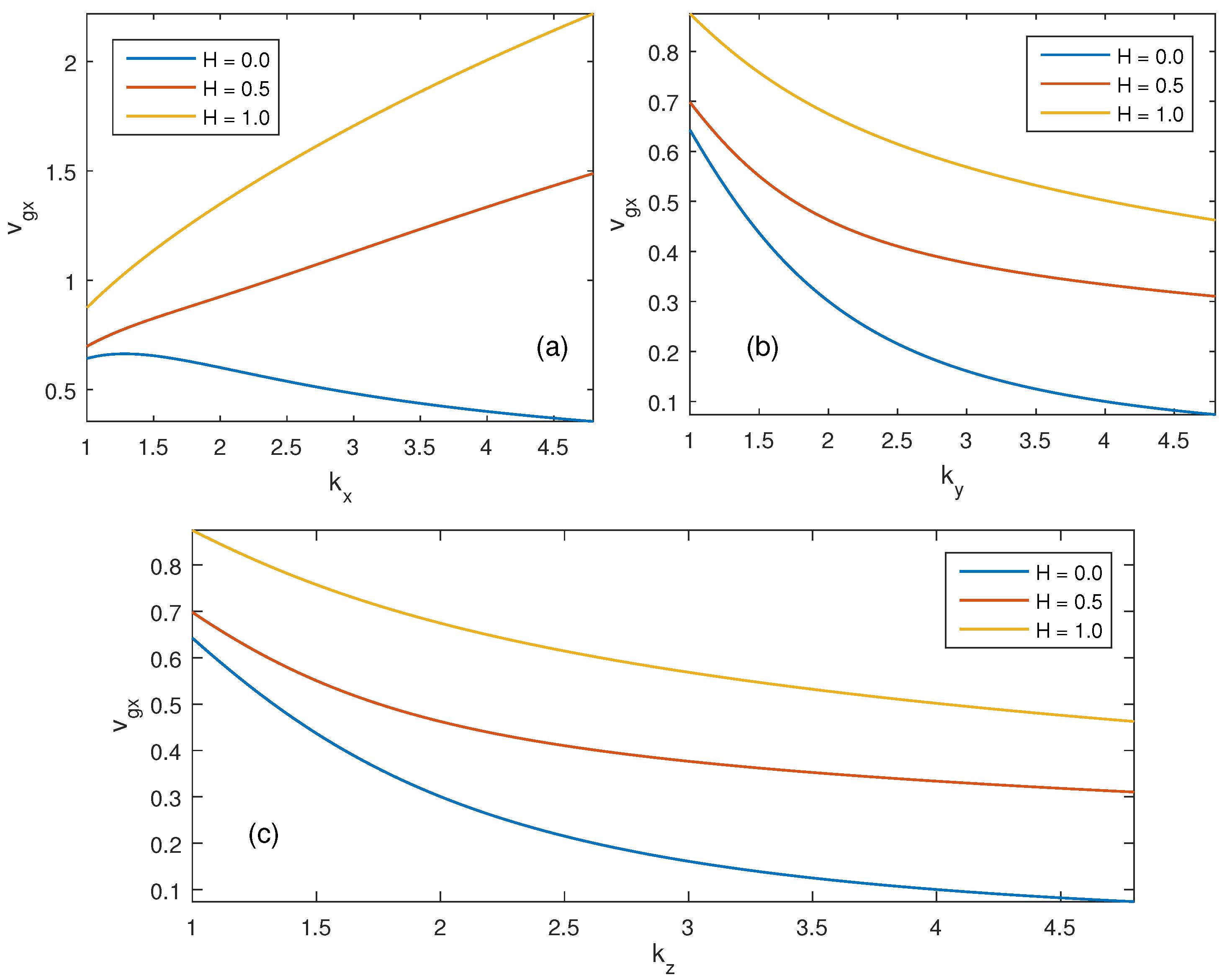


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chaudhuri, S.; Chowdhury, A.R.; Ghosh, B. 3D-Modulational Stability of Envelope Soliton in a Quantum Electron–Ion Plasma—A Generalised Nonlinear Schrödinger Equation. Plasma 2022, 5, 60-73. https://doi.org/10.3390/plasma5010005
Chaudhuri S, Chowdhury AR, Ghosh B. 3D-Modulational Stability of Envelope Soliton in a Quantum Electron–Ion Plasma—A Generalised Nonlinear Schrödinger Equation. Plasma. 2022; 5(1):60-73. https://doi.org/10.3390/plasma5010005
Chicago/Turabian StyleChaudhuri, Shatadru, Asesh Roy Chowdhury, and Basudev Ghosh. 2022. "3D-Modulational Stability of Envelope Soliton in a Quantum Electron–Ion Plasma—A Generalised Nonlinear Schrödinger Equation" Plasma 5, no. 1: 60-73. https://doi.org/10.3390/plasma5010005





