Next Article in Journal
Nonlocal Cauchy Problem via a Fractional Operator Involving Power Kernel in Banach Spaces
Next Article in Special Issue
Impulsive Fractional-Like Differential Equations: Practical Stability and Boundedness with Respect to h-Manifolds
Previous Article in Journal
Analogues to Lie Method and Noether’s Theorem in Fractal Calculus
Previous Article in Special Issue
Residual Power Series Method for Fractional Swift–Hohenberg Equation
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Fractal Fract
Volume 3
Issue 2
10.3390/fractalfract3020026
fractalfract-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Approximate Solutions of the Damped Wave Equation and Dissipative Wave Equation in Fractal Strings

by
Dumitru Baleanu
1,2,* and
Hassan Kamil Jassim
3
1
Department of Mathematics, Faculty of Arts and Sciences, Cankaya University, 06530 Ankara, Turkey
2
Institute of Space Sciences, Magurele, P.O.Box MG-23, Ro 077125 Bucharest, Romania
3
Department of Mathematicas, Faculty of Education for Pure Sciences, University of Thi Qar, Nasiriyah 64001, Iraq
*
Author to whom correspondence should be addressed.
Fractal Fract. 2019, 3(2), 26; https://doi.org/10.3390/fractalfract3020026
Submission received: 13 April 2019 / Revised: 4 May 2019 / Accepted: 7 May 2019 / Published: 11 May 2019
(This article belongs to the Special Issue 2019 Selected Papers from Fractal Fract’s Editorial Board Members)
Browse Figures
Review Reports Versions Notes

Abstract

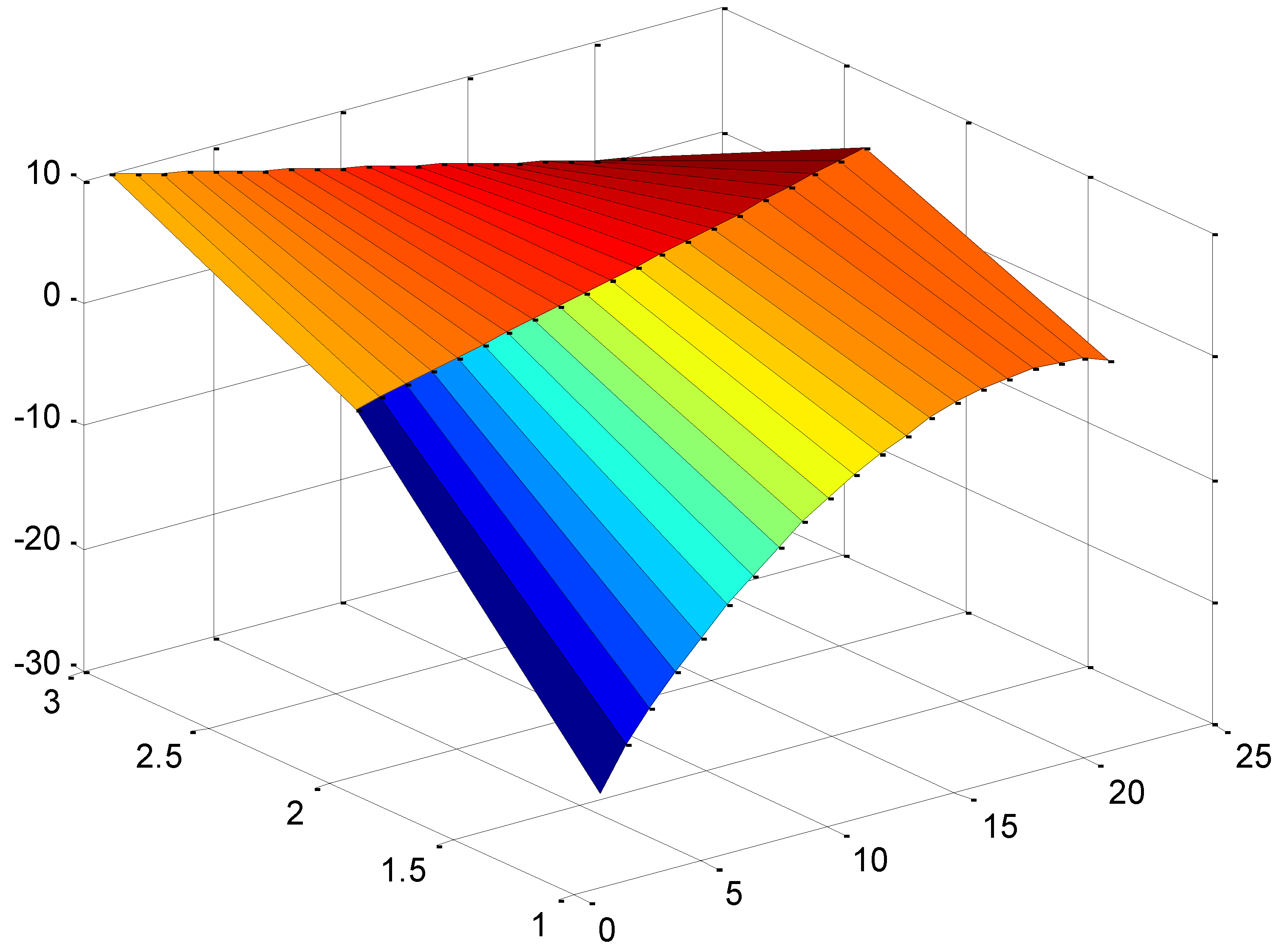
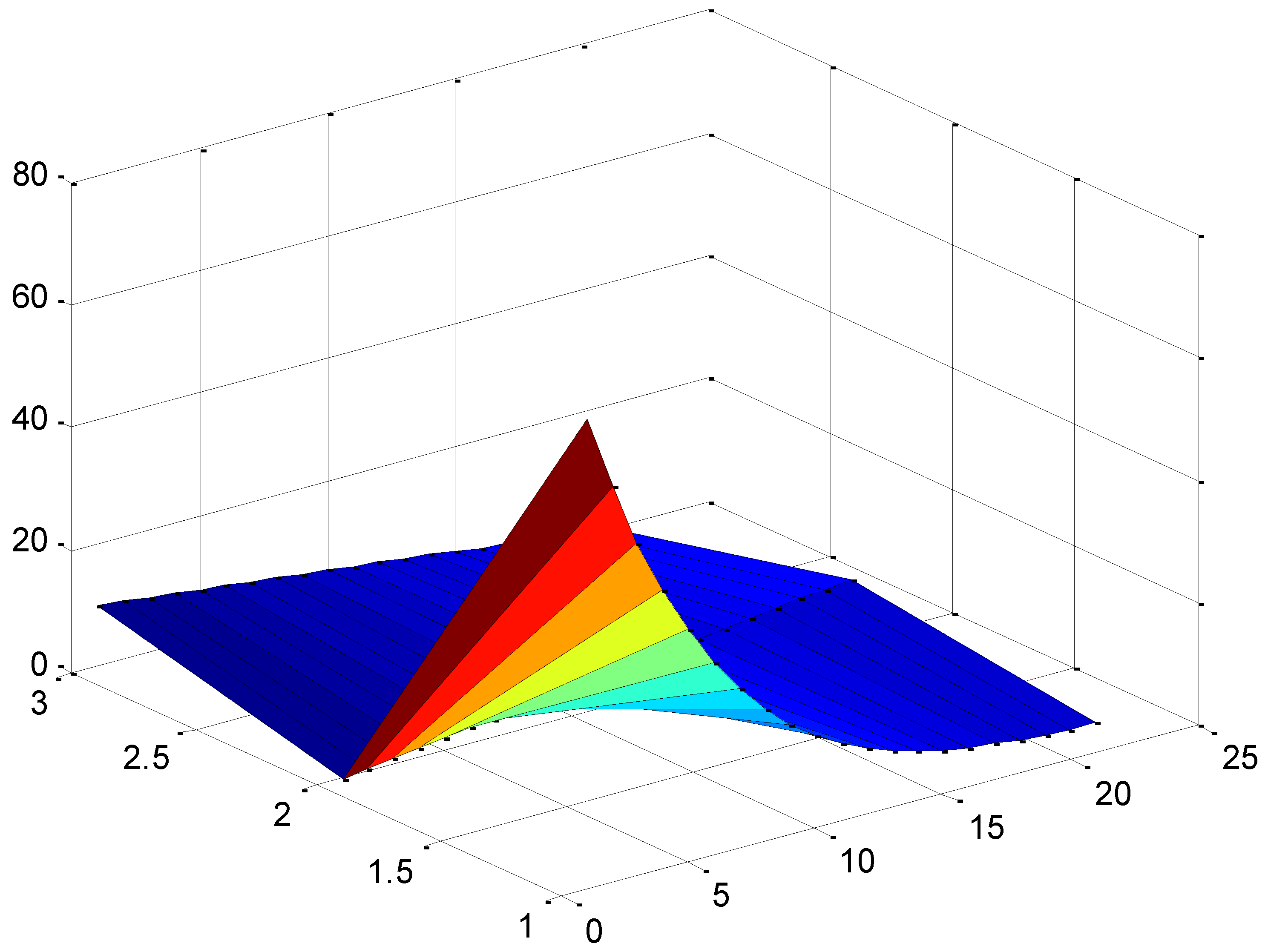
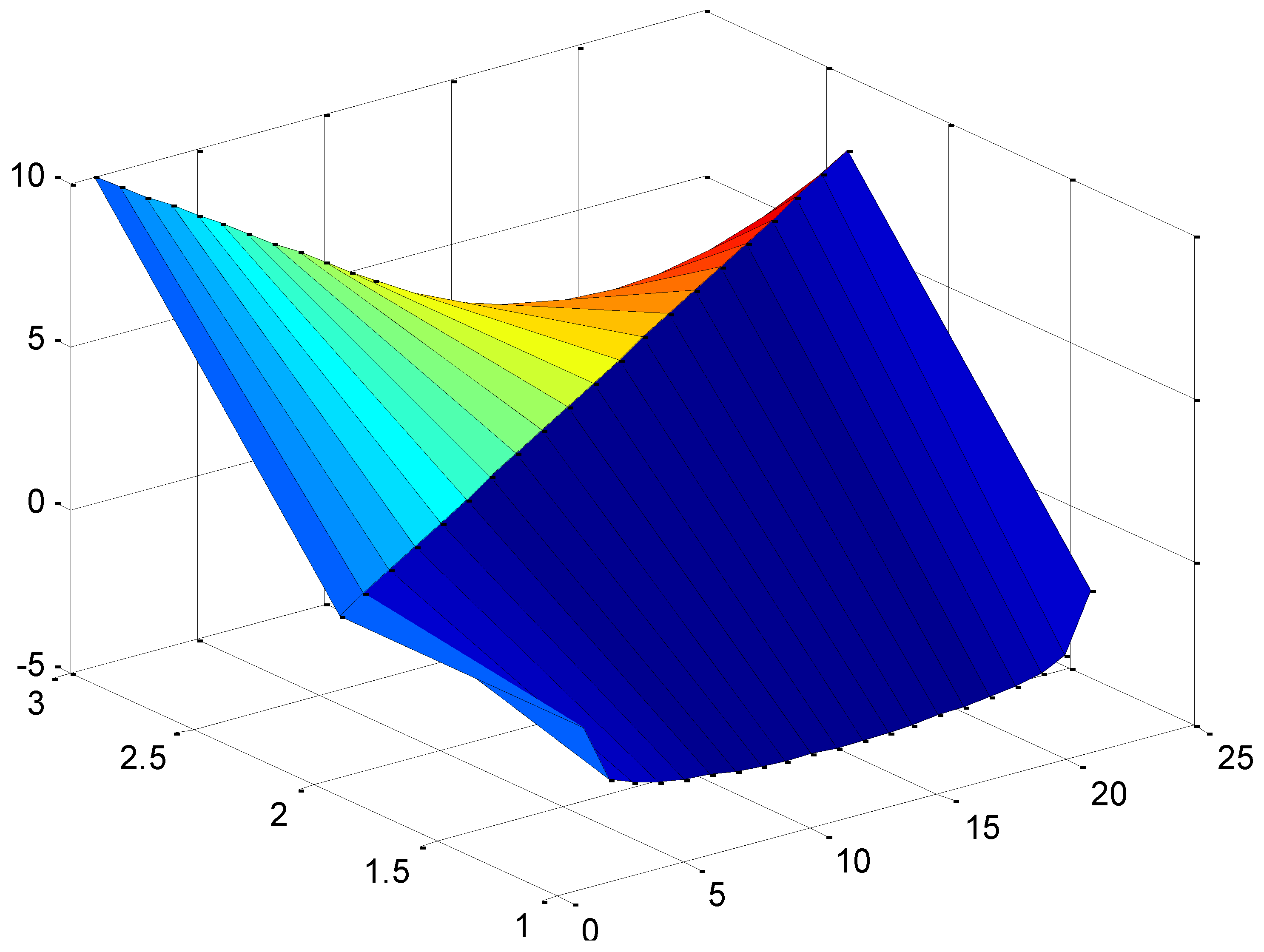
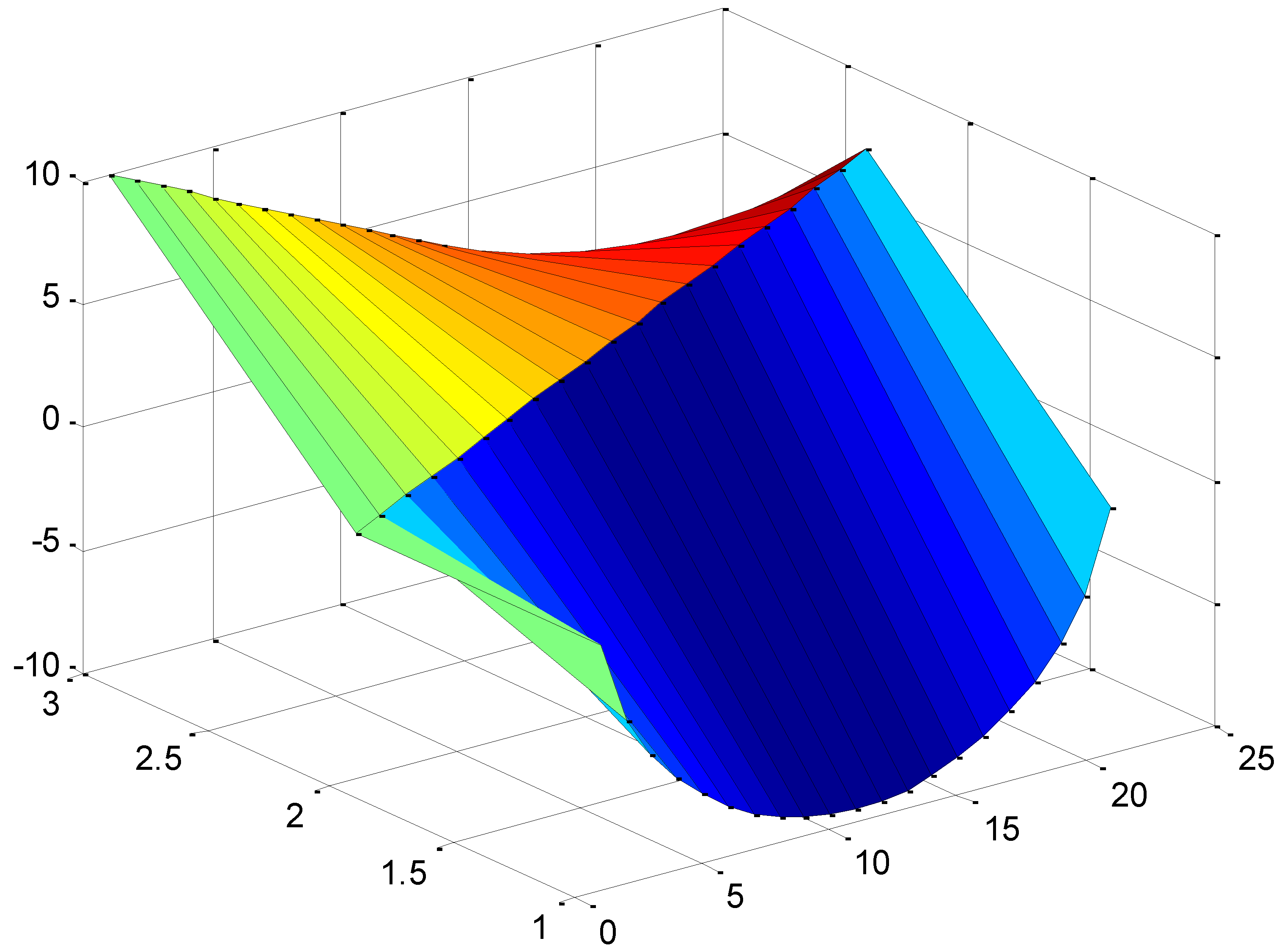
In this paper, we apply the local fractional Laplace variational iteration method (LFLVIM) and the local fractional Laplace decomposition method (LFLDM) to obtain approximate solutions for solving the damped wave equation and dissipative wave equation within local fractional derivative operators (LFDOs). The efficiency of the considered methods are illustrated by some examples. The results obtained by LFLVIM and LFLDM are compared with the results obtained by LFVIM. The results reveal that the suggested algorithms are very effective and simple, and can be applied for linear and nonlinear problems in sciences and engineering.
Keywords: LFDOs; LFLVIM; LFLDM; damped wave equation; dissipative wave equation LFDOs; LFLVIM; LFLDM; damped wave equation; dissipative wave equation

Share and Cite

MDPI and ACS Style

Baleanu, D.; Jassim, H.K. Approximate Solutions of the Damped Wave Equation and Dissipative Wave Equation in Fractal Strings. Fractal Fract. 2019, 3, 26. https://doi.org/10.3390/fractalfract3020026

AMA Style

Baleanu D, Jassim HK. Approximate Solutions of the Damped Wave Equation and Dissipative Wave Equation in Fractal Strings. Fractal and Fractional. 2019; 3(2):26. https://doi.org/10.3390/fractalfract3020026

Chicago/Turabian Style

Baleanu, Dumitru, and Hassan Kamil Jassim. 2019. "Approximate Solutions of the Damped Wave Equation and Dissipative Wave Equation in Fractal Strings" Fractal and Fractional 3, no. 2: 26. https://doi.org/10.3390/fractalfract3020026

APA Style

Baleanu, D., & Jassim, H. K. (2019). Approximate Solutions of the Damped Wave Equation and Dissipative Wave Equation in Fractal Strings. Fractal and Fractional, 3(2), 26. https://doi.org/10.3390/fractalfract3020026

Article Metrics

Back to TopTop