Surface Density of Disk Galaxies in MOND
Abstract
:1. Introduction
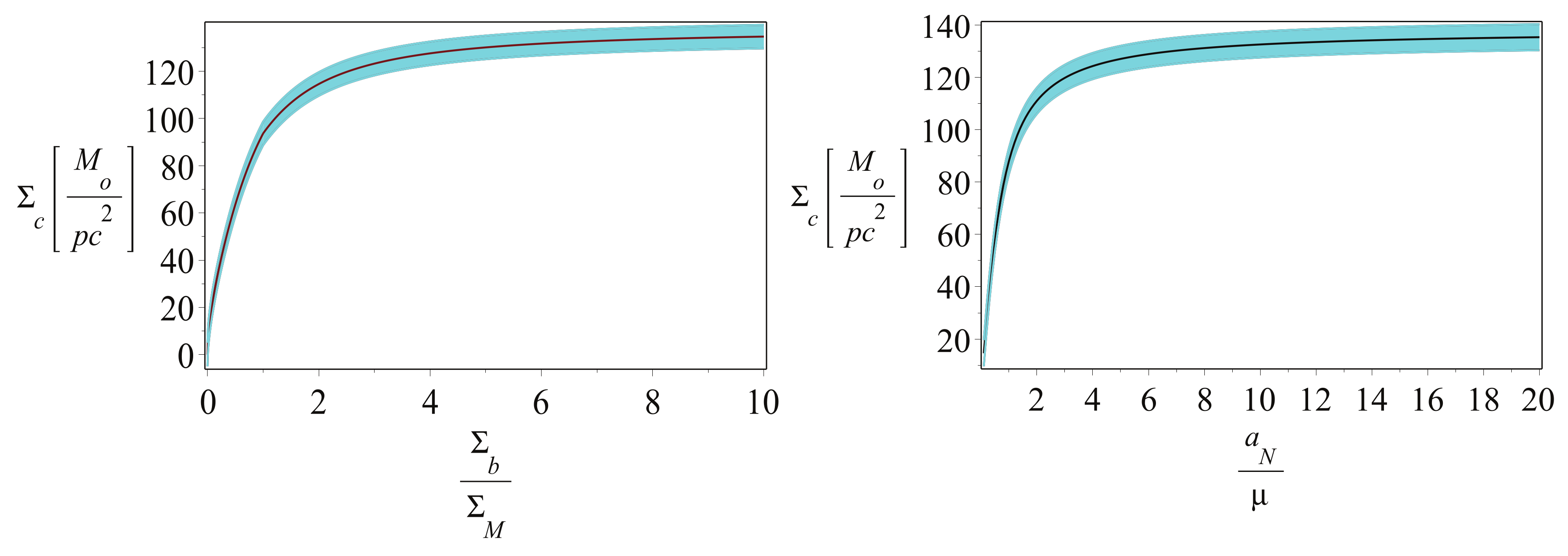
2. Surface Density
3. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | |
| 2 | Please note that since the profile is spherically symmetric, integrating along an axis direction z is equivalent to integrating twice along the positive radial direction |
References
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1989, 61, 1–23. [Google Scholar] [CrossRef]
- Astashenok, A.V.; Popolo, A. Cosmological measure with volume averaging and the vacuum energy problem. Class. Quantum Gravity 2012, 29, 085014. [Google Scholar] [CrossRef] [Green Version]
- Spergel, D.N.; Verde, L.; Peiris, H.V.; Komatsu, E.; Nolta, M.R.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Jarosik, N.; Kogut, A.; et al. First-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Determination of Cosmological Parameters. Astrophys. J. Suppl. Ser. 2003, 148, 175–194. [Google Scholar] [CrossRef] [Green Version]
- Kowalski, M.; Rubin, D.; Aldering, G.; Agostinho, R.J.; Amadon, A.; Amanullah, R.; Balland, C.; Barbary, K.; Blanc, G.; Challis, P.J.; et al. Improved Cosmological Constraints from New, Old, and Combined Supernova Data Sets. Astrophys. J. 2008, 686, 749–778. [Google Scholar] [CrossRef] [Green Version]
- Percival, W.J.; Reid, B.A.; Eisenstein, D.J.; Bahcall, N.A.; Budavari, T.; Frieman, J.A.; Fukugita, M.; Gunn, J.E.; Ivezić, E.A. Baryon acoustic oscillations in the Sloan Digital Sky Survey Data Release 7 galaxy sample. Mon. Not. R. Astron. Soc. 2010, 401, 2148–2168. [Google Scholar] [CrossRef] [Green Version]
- Komatsu, E.; Smith, K.M.; Dunkley, J.; Bennett, C.L.; Gold, B.; Hinshaw, G.; Jarosik, N.; Larson, D.; Nolta, M.R.; Page, L.E.A. Seven-year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Cosmological Interpretation. Astrophys. J. Suppl. Ser. 2011, 192, 18. [Google Scholar] [CrossRef] [Green Version]
- Popolo, A. Non-baryonic dark matter in cosmology. Am. Inst. Phys. 2013, 1548, 2–63. [Google Scholar] [CrossRef]
- Popolo, A. Nonbaryonic Dark Matter in Cosmology. Int. J. Mod. Phys. D 2014, 23, 30005. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. The Structure of Cold Dark Matter Halos. Astrophys. J. 1996, 462, 563. [Google Scholar] [CrossRef] [Green Version]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. A Universal Density Profile from Hierarchical Clustering. Astrophys. J. 1997, 490, 493. [Google Scholar] [CrossRef]
- Navarro, J.F.; Ludlow, A.; Springel, V.; Wang, J.; Vogelsberger, M.; White, S.D.M.; Jenkins, A.; Frenk, C.S.; Helmi, A. The diversity and similarity of simulated cold dark matter haloes. Mon. Not. R. Astron. Soc. 2010, 402, 21–34. [Google Scholar] [CrossRef] [Green Version]
- Moore, B. Evidence against dissipation-less dark matter from observations of galaxy haloes. Nature 1994, 370, 629–631. [Google Scholar] [CrossRef]
- Flores, R.A.; Primack, J.R. Observational and theoretical constraints on singular dark matter halos. arXiv 1994, 427, L1–L4. [Google Scholar] [CrossRef]
- Burkert, A. The Structure of Dark Matter Halos in Dwarf Galaxies. Astrophys. J. 1995, 447, L25. [Google Scholar] [CrossRef] [Green Version]
- Blok, W.J.G.; Bosma, A.; McGaugh, S. Simulating observations of dark matter dominated galaxies: Towards the optimal halo profile. Mon. Not. R. Astron. Soc. 2003, 340, 657–678. [Google Scholar] [CrossRef] [Green Version]
- Swaters, R.A.; Madore, B.F.; den Bosch, F.C.; Balcells, M. The Central Mass Distribution in Dwarf and Low Surface Brightness Galaxies. Astrophys. J. 2003, 583, 732–751. [Google Scholar] [CrossRef] [Green Version]
- Popolo, A. The Cusp/Core Problem and the Secondary Infall Model. Astrophys. J. 2009, 698, 2093–2113. [Google Scholar] [CrossRef] [Green Version]
- Popolo, A.; Kroupa, P. Density profiles of dark matter haloes on galactic and cluster scales. Astron. Astrophys. 2009, 502, 733–747. [Google Scholar] [CrossRef] [Green Version]
- Popolo, A. Density profile slopes of dwarf galaxies and their environment. Mon. Not. R. Astron. Soc. 2012, 419, 971–984. [Google Scholar] [CrossRef] [Green Version]
- Popolo, A.; Hiotelis, N. Cusps and cores in the presence of galactic bulges. J. Cosmol. Astropart. Phys. 2014, 1, 47. [Google Scholar] [CrossRef]
- Klypin, A.; Kravtsov, A.V.; Valenzuela, O.; Prada, F. Where Are the Missing Galactic Satellites? Astrophys. J. 1999, 522, 82–92. [Google Scholar] [CrossRef] [Green Version]
- Moore, B.; Quinn, T.; Governato, F.; Stadel, J.; Lake, G. Cold collapse and the core catastrophe. Mon. Not. R. Astron. Soc. 1999, 310, 1147–1152. [Google Scholar] [CrossRef] [Green Version]
- Garrison-Kimmel, S.; Rocha, M.; Boylan-Kolchin, M.; Bullock, J.S.; Lally, J. Can feedback solve the too-big-to-fail problem? Mon. Not. R. Astron. Soc. 2013, 433, 3539–3546. [Google Scholar] [CrossRef] [Green Version]
- Garrison-Kimmel, S.; Boylan-Kolchin, M.; Bullock, J.S.; Kirby, E.N. Too big to fail in the Local Group. Mon. Not. R. Astron. Soc. 2014, 444, 222–236. [Google Scholar] [CrossRef] [Green Version]
- Feng, J.Q. Rotating disk galaxies without dark matter based on scientific reasoning. Galaxies 2020, 8, 9. [Google Scholar] [CrossRef] [Green Version]
- Marr, J.H. Entropy and mass distribution in disc galaxies. Galaxies 2020, 8, 12. [Google Scholar] [CrossRef] [Green Version]
- Criss, R.E.; Hofmeister, A.M. Density profiles of 51 galaxies from parameter-free inverse models of their measured rotation curves. Galaxies 2020, 8, 19. [Google Scholar] [CrossRef] [Green Version]
- McGaugh, S. Predictions and outcomes for the dynamics of rotating galaxies. Galaxies 2020, 8, 35. [Google Scholar] [CrossRef]
- Sipols, A.; Pavlovich, A. Dark matter dogma: A study of 214 galaxies. Galaxies 2020, 8, 36. [Google Scholar] [CrossRef]
- Sofue, Y. Rotation curve of the milky way and the dark matter density. Galaxies 2020, 8, 37. [Google Scholar] [CrossRef]
- Sofue, Y. Gravitational focusing of low-velocity dark matter on the earth’s surface. Galaxies 2020, 8, 42. [Google Scholar] [CrossRef]
- Hofmeister, A.M.; Criss, R.E. Debated models for galactic rotation curves: A review and mathematical assessment. Galaxies 2020, 8, 47. [Google Scholar] [CrossRef]
- Sipols, A.; Pavlovich, A. Surface brightness plateau in s4g galaxies. Galaxies 2020, 8, 48. [Google Scholar] [CrossRef]
- Hofmeister, A.M.; Criss, R.E. Debate on the physics of galactic rotation and the existence of dark matter. Galaxies 2020, 8, 54. [Google Scholar] [CrossRef]
- Colín, P.; Avila-Reese, V.; Valenzuela, O. Substructure and Halo Density Profiles in a Warm Dark Matter Cosmology. Astrophys. J. 2000, 542, 622–630. [Google Scholar] [CrossRef] [Green Version]
- Sommer-Larsen, J.; Dolgov, A. Formation of Disk Galaxies: Warm Dark Matter and the Angular Momentum Problem. Astrophys. J. 2001, 551, 608–623. [Google Scholar] [CrossRef] [Green Version]
- Goodman, J. Repulsive dark matter. New Astron. 2000, 5, 103–107. [Google Scholar] [CrossRef] [Green Version]
- Peebles, P.J.E. Fluid Dark Matter. Astrophys. J. 2000, 534, L127–L129. [Google Scholar] [CrossRef] [Green Version]
- Zentner, A.R.; Bullock, J.S. Halo Substructure and the Power Spectrum. Astrophys. J. 2003, 598, 49–72. [Google Scholar] [CrossRef] [Green Version]
- Buchdahl, H.A. Non-linear Lagrangians and cosmological theory. Mon. Not. R. Astron. Soc. 1970, 150, 1. [Google Scholar] [CrossRef]
- Starobinsky, A.A. A new type of isotropic cosmological models without singularity. Phys. Lett. B 1980, 91, 99–102. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365–370. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics—Implications for galaxies. Astrophys. J. 1983, 270, 371–389. [Google Scholar] [CrossRef]
- Ferraro, R. f(R) and f(T) theories of modified gravity. AIP Conf. Proc. 2012, 1471, 103–110. [Google Scholar] [CrossRef] [Green Version]
- Kormendy, J.; Freeman, K.C. Scaling Laws for Dark Matter Halos in Late-Type and Dwarf Spheroidal Galaxies. arXiv 2004, 220, 377. [Google Scholar] [CrossRef] [Green Version]
- Donato, F.; Gentile, G.; Salucci, P.; Martins, C.; Wilkinson, M.I.; Gilmore, G.; Grebel, E.K.; Koch, A.; Wyse, R. A constant dark matter halo surface density in galaxies. Mon. Not. R. Astron. Soc. 2009, 397, 1169–1176. [Google Scholar] [CrossRef] [Green Version]
- Milgrom, M. The central surface density of ‘dark haloes’ predicted by MOND. Mon. Not. R. Astron. Soc. 2009, 398, 1023–1026. [Google Scholar] [CrossRef] [Green Version]
- Gentile, G.; Famaey, B.; Zhao, H.; Salucci, P. Universality of galactic surface densities within one dark halo scale-length. Nature 2009, 461, 627–628. [Google Scholar] [CrossRef] [Green Version]
- Gentile, G.; Salucci, P.; Klein, U.; Vergani, D.; Kalberla, P. The cored distribution of dark matter in spiral galaxies. Mon. Not. R. Astron. Soc. 2004, 351, 903–922. [Google Scholar] [CrossRef] [Green Version]
- Gentile, G.; Salucci, P.; Klein, U.; Granato, G.L. NGC 3741: The dark halo profile from the most extended rotation curve. Mon. Not. R. Astron. Soc. 2007, 375, 199–212. [Google Scholar] [CrossRef]
- Simon, J.D.; Bolatto, A.D.; Leroy, A.; Blitz, L.; Gates, E.L. High-Resolution Measurements of the Halos of Four Dark Matter-Dominated Galaxies: Deviations from a Universal Density Profile. Astrophys. J. 2005, 621, 757–776. [Google Scholar] [CrossRef]
- Blok, W.J.G.; Walter, F.; Brinks, E.; Trachternach, C.; Oh, S.-H.; Kennicutt, R.C., Jr. High-Resolution Rotation Curves and Galaxy Mass Models from THINGS. Astron. J. 2008, 136, 2648–2719. [Google Scholar] [CrossRef]
- Popolo, A. On the density-profile slope of clusters of galaxies. Mon. Not. R. Astron. Soc. 2012, 424, 38–51. [Google Scholar] [CrossRef]
- Napolitano, N.R.; Romanowsky, A.J.; Tortora, C. The central dark matter content of early-type galaxies: Scaling relations and connections with star formation histories. Mon. Not. R. Astron. Soc. 2010, 405, 2351–2371. [Google Scholar] [CrossRef] [Green Version]
- Boyarsky, A.; Ruchayskiy, O.; Iakubovskyi, D.; Maccio’, A.V.; Malyshev, D. New evidence for dark matter. arXiv 2009, arXiv:0911.1774. [Google Scholar]
- Cardone, V.F.; Tortora, C. Dark matter scaling relations in intermediate z haloes. Mon. Not. R. Astron. Soc. 2010, 409, 1570–1576. [Google Scholar] [CrossRef] [Green Version]
- Popolo, A.; Cardone, V.F.; Belvedere, G. Surface density of dark matter haloes on galactic and cluster scales. Mon. Not. R. Astron. Soc. 2013, 429, 1080–1087. [Google Scholar] [CrossRef] [Green Version]
- Cardone, V.F.; Popolo, A. Newtonian acceleration scales in spiral galaxies. Mon. Not. R. Astron. Soc. 2012, 427, 3176–3187. [Google Scholar] [CrossRef] [Green Version]
- Saburova, A.; Popolo, A. On the surface density of dark matter haloes. Mon. Not. R. Astron. Soc. 2014, 445, 3512–3524. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Y.; Popolo, A.; Chang, Z. On the absence of a universal surface density, and a maximum Newtonian acceleration in dark matter haloes: Consequences for MOND. Phys. Dark Universe 2020, 28, 100468. [Google Scholar] [CrossRef]
- Milgrom, M. Can the Hidden Mass Be Negative? Astrophys. J. 1986, 306, 9. [Google Scholar] [CrossRef]
- Binney, J.; Tremaine, S. Galactic Dynamics, 2nd ed.; Princeton University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Begum, A.; Chengalur, J.N.; Karachentsev, I.D. A dwarf galaxy with a giant HI disk. Astron. Astrophys. 2005, 433, L1–L4. [Google Scholar] [CrossRef] [Green Version]
- Begum, A.; Chengalur, J.N. Kinematics of two dwarf galaxies in the NGC 6946 group. Astron. Astrophys. 2004, 424, 509–517. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Del Popolo, A.; Le Delliou, M. Surface Density of Disk Galaxies in MOND. Universe 2023, 9, 32. https://doi.org/10.3390/universe9010032
Del Popolo A, Le Delliou M. Surface Density of Disk Galaxies in MOND. Universe. 2023; 9(1):32. https://doi.org/10.3390/universe9010032
Chicago/Turabian StyleDel Popolo, Antonino, and Morgan Le Delliou. 2023. "Surface Density of Disk Galaxies in MOND" Universe 9, no. 1: 32. https://doi.org/10.3390/universe9010032



