Curvature Invariants for Lorentzian Traversable Wormholes

Abstract

The curvature invariants of three Lorentzian wormholes are calculated and plotted in this paper. The plots may be inspected for discontinuities to analyze the traversability of a wormhole. This approach was formulated by Henry, Overduin, and Wilcomb for black holes (Henry et al., 2016). Curvature invariants are independent of coordinate basis, so the process is free of coordinate mapping distortions and the same regardless of your chosen coordinates (Christoffel, E.B., 1869; Stephani, et al., 2003). The four independent Carminati and McLenaghan (CM) invariants are calculated and the nonzero curvature invariant functions are plotted (Carminati et al., 1991; Santosuosso et al., 1998). Three traversable wormhole line elements analyzed include the (i) spherically symmetric Morris and Thorne, (ii) thin-shell Schwarzschild wormholes, and (iii) the exponential metric (Visser, M., 1995; Boonserm et al., 2018).

1. Introduction

Lorentzian traversable wormholes were first described by Kip Thorne and collaborators who used Einstein’s general relativistic field equations to explore the possibility of faster-than-light (FTL) interstellar spaceflight without violating special relativity [1,2]. Earlier studies demonstrated the possibility of traversable wormholes in general relativity [3,4]. A Lorentzian traversable wormhole is a topological opening in spacetime which manifests traversable intra-universe and/or inter-universe connections, as well as possible different chronological connections between distant spacetime points. The condition for a Lorentzian wormhole to be traversable is that it is free of both event horizons and singularities [5]. Such a wormhole is fully traversable in both directions, geodesically complete, and possesses no crushing gravitational tidal forces found anywhere inside. Consequently, Lorentzian traversable wormholes are unlike the nontraversable Schwarzschild wormhole, or Einstein–Rosen bridge, associated with eternal black holes in the maximally extended version of the Schwarzschild metric. Exotic matter, which violates the point-wise and averaged energy conditions, is required to open and stabilize a Lorentzian traversable wormhole. A comprehensive technical overview of this subject is found in [5].

Previous studies of Lorentzian traversable wormholes rely on either calculating the elements of the Riemann curvature tensor, $R_{jkl}^i$, to “observe” the effects of the wormhole’s spacetime curvature on photons and matter moving through it or by embedding diagrams. However, the $R_{jkl}^i$ cannot be calculated in an invariant manner because it is a function of the chosen coordinates [6]. Analysis of $R_{jkl}^i$ can be misleading because a different choice of coordinate basis will result in different tensor components. These coordinate mapping distortions arise purely as an artifact of the coordinate choice. Embedding diagrams offer a narrow view of the spacetime manifold. In [1], the embedding diagrams depicts the wormhole geometry along just an equatorial ($\theta =\frac{\pi }{2}$) slice through space at a specific moment in time. The embedding diagram only offers a limited view of the physics involved in the wormhole. Curvature invariants allow a manifestly coordinate invariant characterization of certain geometrical properties of spacetime [7]. The best way to illustrate wormhole spacetimes without such issues is to plot their independent curvature invariants to provide proper visualization of any hidden surprises.

Christoffel proved that scalars constructed from the metric and its derivatives must be functions of the metric itself, the Riemann tensor and its covariant derivatives [8]. Curvature invariants are scalar products of Riemann, Ricci, or Weyl tensors, or their covariant derivatives. Curvature invariants are of particular interest in general relativity for identifying intrinsic singularities in spacetimes, classifying the Petrov type of the Weyl tensor and the Segre type of the trace-free Ricci tensor, and studying the equivalence problem [7,9,10,11,12,13,14,15]. The study of curvature invariants is a subject of recent black hole research with a priority on locating their horizons [16,17,18,19,20,21]. Specific curvature invariants of certain wormholes have been calculated to locate any singularities and horizons, but a complete set has not been presented and calculated [22,23,24]. The set of Carminati and McLenaghan (CM) invariants are of lowest degree and contains a minimal independent set for any Petrov or Segre types [25]. For class B warped product spacetimes1, four of the CM invariants, R, $r_1$, $r_2$, and $w_2$, are needed to satisfy the syzygies2 [27]. In this paper, the CM curvature invariants of popular wormholes will be plotted and the geometrical properties analyzed.

In [16], Henry et al. computed and plotted a number of independent curvature invariants for the hidden interiors of Kerr–Newman black holes. They produced visually stunning 3D plots which revealed the surprisingly complex nature of spacetime curvature in Kerr–Newman black hole interiors. Their work motivated the present authors to undertake a similar study for the case of Lorentzian traversable wormholes. Reported here are the computations and 3D plots for three selected Lorentzian traversable wormholes that are described in [5,22]: (i) the Morris and Thorne (MT) wormhole, (ii) the thin-shell Schwarzschild wormhole, and (iii) the exponential metric. The thin-shell flat-face (TS) wormhole was also analyzed, but all of its invariants were trivial as they were identically zero.

2. Method to Compute the Invariants

The CM curvature invariants can be calculated from any given line element. From the line element, identify the metric $g_{ij}$ with the indices $\{i,j,k,l\}$ ranging from $\{0,n-1\}$, where n is the number of spacetime dimensions. In this paper, a basis or a tetrad will be chosen for each metric. It is emphasized that the scalar invariants are independent of the choice of coordinates in a tetrad. A different choice of coordinates for each spacetimes’ tetrad will result in the same invariants plotted in this paper. From the metric, the Ricci scalar R, the trace-free Ricci tensor $S_{ij}$ and the Weyl tensor $C_{ijkl}$ can be calculated [10].

The literature defines fourteen curvature invariants, but the total rises to seventeen when certain nondegenerate cases are taken into account [7,25]. The four CM invariants as required by the syzygies are [27]:

$$R=g_{ij}{R}^{ij},$$

(1)

$$r_1=\frac{1}{4}{S}_i^j{S}_j^i,$$

(2)

$$r_2=-\frac{1}{8}{S}_i^j{S}_k^i{S}_j^k,$$

(3)

$$w_2=-\frac{1}{8}{\overline{C}}_{ijkl}{\overline{C}}^{ijmn}{\overline{C}}_{mn}^{kl}.$$

(4)

The full solutions to the wormhole metrics studied herein were found using Wolfram Mathematica $10.4$^®.

3. Morris and Thorne Wormhole

The MT wormhole is defined by a spacetime, which is spherically symmetric and Lorentzian. The spacetime describes the required traversable wormhole geometry. In the standard Schwarzschild coordinates [1], the line element is:

$$d{s}^2=-e^{2{\varphi }^{\pm }\left(r\right)}d{t}^2+\frac{d{r}^2}{\left(1-\frac{b^{\pm }\left(r\right)}{r}\right)}+r^2(d{\theta }^2+si{n}^2\theta d{\phi }^2).$$

(5)

The tetrad for the MT line element uses the spherical coordinates $(r$: with circumference $=2\pi r;0\le \theta \le \pi ;0\le \phi \le 2\pi )$, and $(-\infty <t<\infty )$ is the proper time of a static observer. ${\varphi }^{\pm }\left(r\right)$ is the freely specifiable redshift function that defines the proper time lapse through the wormhole throat. $b^{\pm }\left(r\right)$ is the freely specifiable shape function that defines the wormhole throat’s spatial (hypersurface) geometry. The ± indicates the side of the wormhole. The throat described by Equation (5) is spherical. A fixed constant, $r_0$, is chosen to define the radius of the wormhole throat such that $b^{\pm }\left(r_0\right)=r_0$, which is an isolated minimum. Two coordinate patches of the manifold are then joined at $r_0$. Each patch represents either a different part of the same universe or another universe, and the patches range from $r_0\le r<\infty$. The condition that the wormhole is horizon free requires that $g_{tt}=-e^{2{\varphi }^{\pm }\left(r\right)}\ne 0$. This implies that $|{\varphi }^{\pm }\left(r\right)|$ must be finite everywhere [5,28]. The use of Schwarzschild coordinates in Equation (5) leads to more efficient computations of the Riemann and Ricci curvature tensors, the Ricci scalar, and all four invariants.

The four CM invariants for the Morris-Thorne wormhole are

$$R=\frac{1}{r^2}\left(b^{\prime }\left(r{\mathsf{\Phi }}^{\prime }+2\right)+2r\left(b-r\right){\mathsf{\Phi }}^{″}-2r\left(r-b\right){\mathsf{\Phi }}^{{}^{\prime }2}+\left(3b-4r\right){\mathsf{\Phi }}^{\prime }\right),$$

(6)

$$\begin{array}{cc}\hfill r_1=\frac{1}{16r^6}& (r^2\left(b^{{}^{\prime }2}\left(r^2{\mathsf{\Phi }}^{{}^{\prime }2}+2\right)-4r{b}^{\prime }{\mathsf{\Phi }}^{\prime }\left(r^2{\mathsf{\Phi }}^{″}+r^2{\mathsf{\Phi }}^{{}^{\prime }2}-2\right)+4r^2\left(r^2{\mathsf{\Phi }}^{{}^{″}2}+r^2{\mathsf{\Phi }}^{{}^{\prime }4}+2{\mathsf{\Phi }}^{{}^{\prime }2}\left(r^2{\mathsf{\Phi }}^{″}+1\right)\right)\right)\hfill \\ & -2rb(b^{\prime }\left(-2r^3{\mathsf{\Phi }}^{{}^{\prime }3}+{\mathsf{\Phi }}^{\prime }\left(6r-2r^3{\mathsf{\Phi }}^{″}\right)+r^2{\mathsf{\Phi }}^{{}^{\prime }2}+2\right)+2r(2r^3{\mathsf{\Phi }}^{{}^{\prime }4}+2{\mathsf{\Phi }}^{{}^{\prime }2}\left(2r^3{\mathsf{\Phi }}^{″}+r\right)\hfill \\ & +2r{\mathsf{\Phi }}^{″}\left(r^2{\mathsf{\Phi }}^{″}-1\right)-r^2{\mathsf{\Phi }}^{{}^{\prime }3}+{\mathsf{\Phi }}^{\prime }\left(2-r^2{\mathsf{\Phi }}^{″}\right)\left)\right)\hfill \\ & +b^2\left(4r^4{\mathsf{\Phi }}^{{}^{″}2}+4r^4{\mathsf{\Phi }}^{{}^{\prime }4}-4r^3{\mathsf{\Phi }}^{{}^{\prime }3}-8r^2{\mathsf{\Phi }}^{″}-4r{\mathsf{\Phi }}^{\prime }\left(r^2{\mathsf{\Phi }}^{″}-3\right)+{\mathsf{\Phi }}^{{}^{\prime }2}\left(8r^4{\mathsf{\Phi }}^{″}+r^2\right)+6\right)),\hfill \end{array}$$

(7)

$$r_2=-\frac{3}{64r^9}\left(b\left(2r{\mathsf{\Phi }}^{\prime }+1\right)-r\left(b+2r{\mathsf{\Phi }}^{\prime }\right){)}^2(r^2\left(b^{\prime }{\mathsf{\Phi }}^{\prime }-2r\left({\mathsf{\Phi }}^{″}+{\mathsf{\Phi }}^{{}^{\prime }2}\right)\right)+b\left(2r^2{\mathsf{\Phi }}^{″}+2r^2{\mathsf{\Phi }}^{{}^{\prime }2}-r{\mathsf{\Phi }}^{\prime }-2\right)\right),$$

(8)

$$w_2=\frac{1}{144r^9}{\left(r\left(b^{\prime }\left(1-r{\mathsf{\Phi }}^{\prime }\right)+2r\left(r{\mathsf{\Phi }}^{″}+r{\mathsf{\Phi }}^{{}^{\prime }2}-{\mathsf{\Phi }}^{\prime }\right)\right)-b\left(2r^2{\mathsf{\Phi }}^{″}+2r^2{\mathsf{\Phi }}^{{}^{\prime }2}-3r{\mathsf{\Phi }}^{\prime }+3\right)\right)}^3.$$

(9)

All the invariants are nonzero and depend only on the radial coordinate, r, implying they are spherically symmetric. The invariants are plotted in Figure 1 after selecting a redshift function of $\varphi \left(r\right)=0$ and the shape function of

$$b\left(r\right)=2GM\left(1-e^{r_0-r}\right)+r_0{e}^{r_0-r}.$$

(10)

These functions satisfy the constraints on the asymptotic behavior and continuity at the wormhole’s throat [5]. At a distant greater than $0.5r_0$, all the figures are asymptotically flat. For $r\to 0$, the figures diverge to infinity. The divergence at $r=0$ is not pathological as the radial coordinate r has a minimum $r_0>0$ at the wormhole’s throat. Thus, a traveler passing through the wormhole would not experience any divergence. Any tidal forces on the traveler would be minimal. Consequently, the MT wormhole would be traversable as indicated by the included invariant plots.

4. Thin-Shell Schwarzschild Wormhole

A second example wormhole is the thin-shell Schwarzschild wormhole. The Schwarzschild geometry in natural units is given by the line element:

$$d{s}^2=-\left(1-\frac{2M}{r}\right)d{t}^2+\frac{d{r}^2}{\left(1-\frac{2M}{r}\right)}+r^2\left(d{\theta }^2+si{n}^2\theta d{\phi }^2\right),$$

(11)

where M is the mass of the wormhole. The tetrad of the line element is the spherical coordinates. The thin shell formalism developed in [5] is used to construct the two sides of the wormhole. Each side is described by Equation (11). The thin-shell formalism is applied with a unit normal $n_i=\left(0,\sqrt{1-\frac{2M}{r}},0,0\right)$. Regions described by ${\mathsf{\Omega }}_{1,2}\equiv \left\{r_{1,2}\le a|a>\frac{3M}{2}\right\}$ are removed from the two spacetimes leaving two separate and incomplete regions with boundaries given by the time-like hypersurfaces $\partial {\mathsf{\Omega }}_{1,2}\equiv \left\{r_{1,2}=a|a>\frac{3M}{2}\right\}$. The boundaries $\partial {\mathsf{\Omega }}_1=\partial {\mathsf{\Omega }}_2$ at the wormhole throat of $r=a$ are identified and connected. The boundary at $a=\frac{3M}{2}$ is chosen to satisfy the Einstein equations and equation of state in [5]; however, an event horizon is expected. The resulting spacetime manifold is geodesically complete and contains two asymptotically flat regions connected by the wormhole.

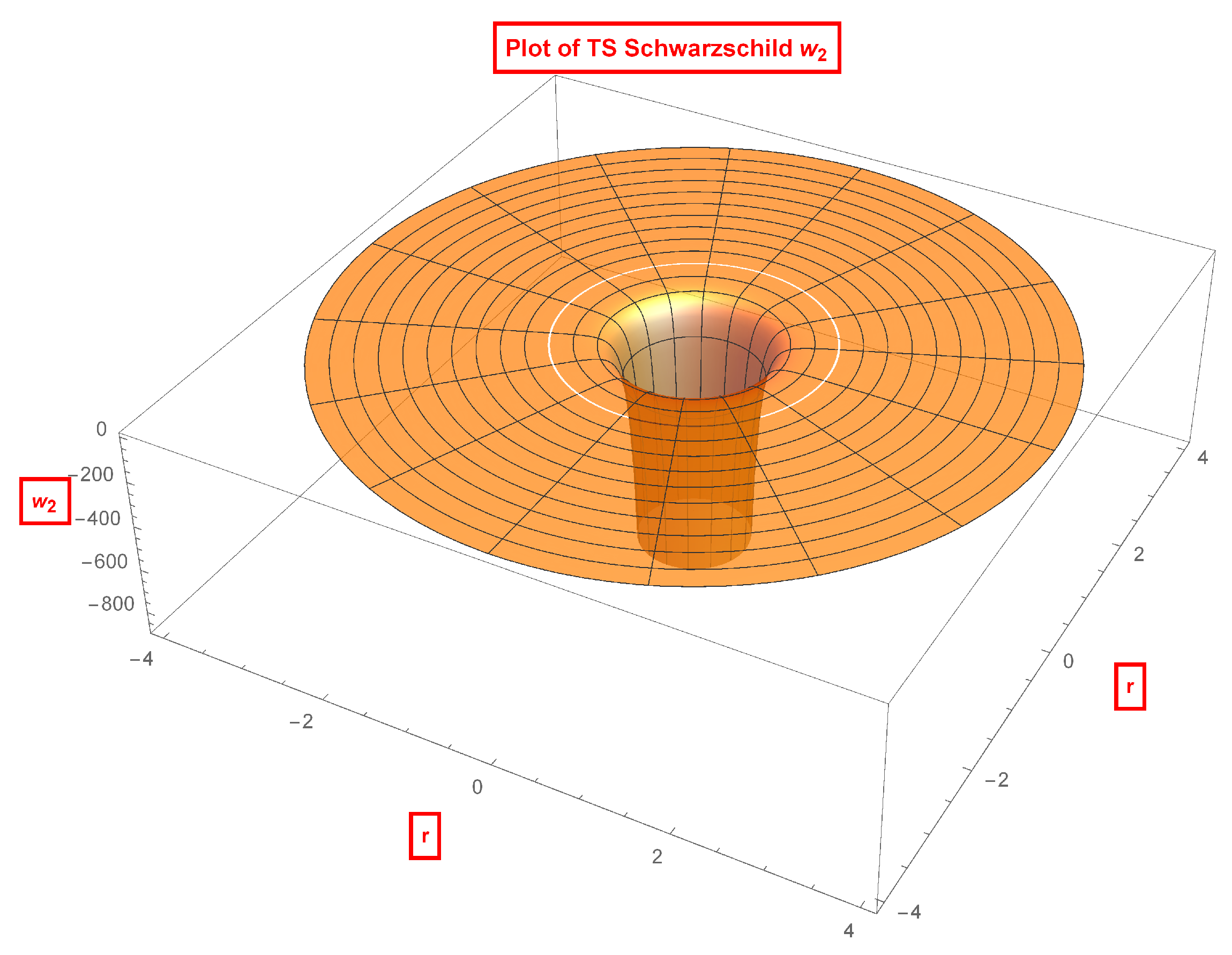
Of the four CM invariants computed for the Schwarzschild wormhole, three invariants R, $r_1$, and $r_2$ equal zero. The remaining invariant is

$$\begin{array}{cc}\hfill w_2=& -\frac{12M^3}{r^9}+\frac{6M^2}{a^2{r}^9}\sqrt{1-\frac{2M}{a}}\left(a\left(r-2M\right)+2M\left(2M+2r^3-r\right)\right)\delta \left(r-a\right)\hfill \\ & +\frac{12M}{a^5{r}^9}\left(a-2M\right)\left(4M^2{\left(a-2M\right)}^2+r^2{\left(a-2M\right)}^2-4Mr{\left(a-2M\right)}^2-2M^2{r}^6\right)\delta {\left(r-a\right)}^2\hfill \\ & +\frac{8}{a^6{r}^9}{\left(1-\frac{2M}{a}\right)}^{3/2}\left({(a-2M)}^3{\left(r-2M\right)}^3+M^3{r}^9\right)\delta {\left(r-a\right)}^3.\hfill \end{array}$$

(12)

The $w_2$ invariant is broken into two main portions. The leading term of $\frac{-12M^3}{r^9}$ is the Schwarzschild black hole’s $w_2$ invariant. The remaining portions of the function are all proportional to different powers of $\delta \left(r-a\right)$. Consequently, they contribute to the throat of the wormhole. Evaluating $w_2$ at the throat gives

$$\begin{array}{cc}\hfill w_2{|}_{r=a}=\frac{2}{a^{14}}& (-6a^5{M}^3+4a^8\left(\frac{{\left(a-2M\right)}^6}{a^9}+M^3\right){\left(1-\frac{2M}{a}\right)}^{\frac{3}{2}}\hfill \\ & +3a^3{M}^2\left(4a^{3}M+a^2-4aM+4M^2\right)\sqrt{1-\frac{2M}{a}}\hfill \\ & -6M\left(a-2M\right)\left(2a^6{M}^2-a^4+8a^{3}M-24a^2{M}^2+32a{M}^3-16M^4\right)).\hfill \end{array}$$

(13)

Since $w_2\propto \frac{1}{a^{14}}$, the throat will experience greater curvature the smaller it is and vice versa.

The only nonzero invariant, $w_2$, is plotted in Figure 2. The mass and radius of the throat are normalized to $M=1$ and $a=\frac{3}{2}$ in the plot. Its plot has one divergence and one discontinuity. The divergence occurs at $r=0$, which is outside the manifold of ${\mathsf{\Omega }}_{1,2}$. By the same argument for the apparent MT divergence, the first Schwarzschild divergence would not impede the traversability of the wormhole. The discontinuity occurs at $r=a=\frac{3M}{2}$ and is located at the throat where the horizons are connected by the Schwarzschild wormholes. In these invariants, it is represented by a discontinuous jump to the value in Equation (13). Since the invariants at the horizon are inversely proportional to $a^{-14}$, the tidal forces on a traveler is benign at the horizon, and the thin-shell Schwarzschild wormhole would be traversable.

5. The Exponential Metric

The exponential metric was demonstrated recently in [22] to have a traversable wormhole throat. In natural units, its line element is

$$d{s}^2=-e^{\frac{-2M}{r}}d{t}^2+e^{\frac{+2M}{r}}\{d{r}^2+r^2\left(d{\theta }^2+si{n}^2\theta d{\phi }^2\right)\},$$

(14)

where M is the mass of the wormhole. The tetrad utilizes the spherical coordinates. It has a traversable wormhole throat at $r=M$. The area of the wormhole is a concave function with a minima at the throat where it satisfies the “flare out” condition. It does not have a horizon since $g_{tt}\ne 0$ for all $r\ge 0$. The region $r<M$ on the other side of the wormhole is an infinite volume “other universe” that exhibits an “underhill effect” where time runs slower since $e^{\frac{-2M}{r}}>0$ in this region.

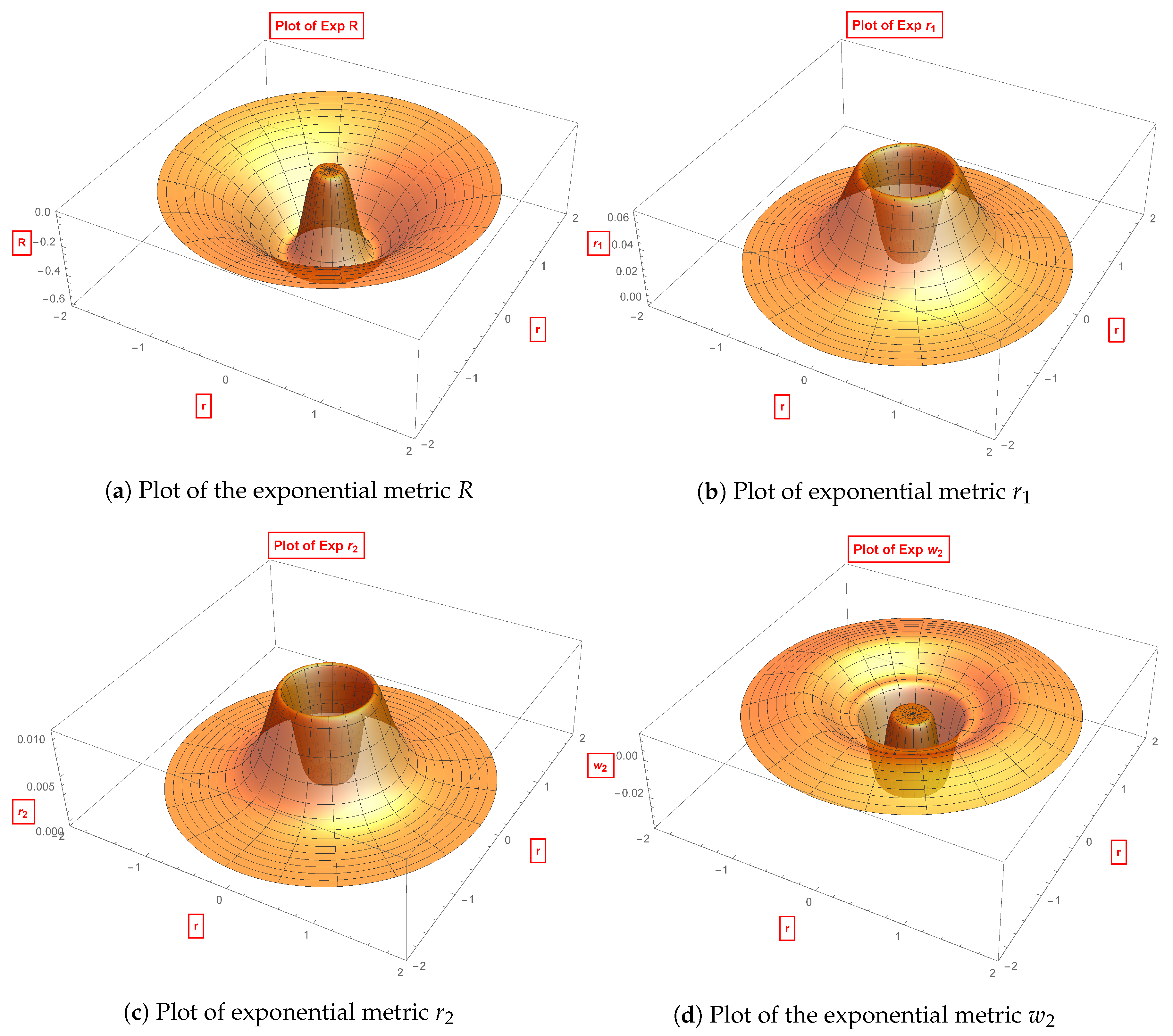
Computing the four curvature invariants for the exponential metric with Wolfram Mathematica $10.4$^® gives

$$R=-\frac{2M^2}{r^4}{e}^{-\frac{2M}{r}},$$

(15)

$$r_1=\frac{3M^4}{4r^8}{e}^{-\frac{4M}{r}},$$

(16)

$$r_2=\frac{3M^6}{8r^{12}}{e}^{-\frac{6M}{r}},$$

(17)

$$w_2=-\frac{32M^3{(2M-3r)}^3}{9r^{12}}{e}^{-\frac{6M}{r}}.$$

(18)

They are all nonzero and depend only the radial coordinate r implying spherical symmetry. In addition, they are finite at the throat $r=M$ and go to zero as $r\stackrel{}{\to }\infty$ in accordance with [22]. $w_2$ and R have a minima near the throat, while $r_1$ and $r_2$ have a maxima. The plots are finite everywhere and completely connected confirming the lack of a horizon. The encountered tidal forces would be minimal. It can be concluded that the exponential metric represents a traversable wormhole.

6. Conclusions

This paper demonstrates how to compute and plot the curvature invariants of various wormhole line elements. The curvature invariants reveal the entire wormhole spacetime manifold and whether the wormhole is traversable or not. As examples, plotting the CM curvature invariants of the (i) spherically symmetric MT, (ii) thin-shell Schwarzschild, and (iii) exponential metric wormholes showed they are traversable in agreement with [1,2,5]. The scalar polynomial invariants of the MT wormhole were found to be nonzero and are plotted in Figure 1a–d. A divergence is found in all four, but it does not affect the wormhole’s traversability since the divergence is outside the physical range of the radial coordinate, $r\in \left(r_0,\infty \right)$. For the thin-shell Schwarzschild wormhole, $w_2$ is found to be the single nonzero invariant. As plotted in Figure 2, it has a divergence at the center and a ring discontinuity. The divergence is outside the physical radial coordinate and can be safely ignored. The ring discontinuity represents a jump due to the $\delta$-function from the thin-shell formalism. It is shown to be inversely proportional to $a^{-14}$, not affecting traversability through the wormhole. The scalar polynomial invariants of the exponential metric were found to be nonzero and were plotted in Figure 3a–d. The plots are continuous across the entire manifold and traversable.

Potentially, the ring discontinuity in the thin-shell Schwarzschild wormhole could lead to a redshift of light rays passing through the wormhole. The redshift could be used to distinguish wormholes from black holes. While significant research remains to answer traversable wormhole issues, especially with regards to the exotic mass requirements and understanding averaged null energy condition violations, the present paper hopes to establish several methods for understanding the traversal through wormholes.

Computing and plotting the invariant functions has significant advantages for the inspection of wormholes. As mentioned previously, the advantage of plotting the invariants is that they are free from coordinate mapping distortions and other artifacts of the chosen coordinates. The resulting invariants properly illustrate the entire underlying spacetime independent of the coordinate system chosen. Plotting the invariants exposes the presence of any artifacts, divergences, or discontinuities anywhere on the manifold. Once the artifacts are revealed by the invariants, they can be related mathematically to the textbook tensors. Any artifact’s effect can then be analyzed based on where the curvature invariant locates it, what type of artifact the curvature invariant reveals it to be, and how the artifact may affect the object’s motion based on the plots of the curvature invariant at that location. A second advantage is the relative ease with which the invariants can be plotted. Software packages exist or can be developed to calculate the textbook tensors. Then, the CM invariants can be derived from the textbook using minimal edits to the software packages. The CM invariants were chosen to be computed and plotted in this paper because they had general independence, were of lowest possible degree, and a minimal independent set for any Petrov Type and for any specific choice of the Ricci tensor [25]. It is of research interest that other choices for the set of invariants exist, such as the Cartan invariants and the Witten and Petrov invariants [7,29]. These can be also computed and plotted without difficulty, but they will be related to the CM invariants by polynomial functions [7]. Since the invariants are either scalars or pseudoscalars, they can be straightforwardly plotted and visually interpreted.

The thin-shell Schwarzschild wormhole is the most common example of a large class of wormholes. The class includes wormholes with different radii of curvature and/or masses on either side of the throats, wormholes with same or different charge, Q, on either side of the wormhole, and time-dependent wormholes. For charged wormholes, a second ring artifact at $r=Q$ is likely to exist since the metric has a singularity at that point.

A prospective future application of this work is an investigation of wormholes with throats that change dynamically over time. This implies that the ring discontinuity in the invariant functions will change as a function of time. Hence, dynamic wormholes are technically more intricate to study as compared to static wormholes. Consequently, it can be expected that the computation of a dynamic wormhole’s invariants and their plots increase in difficulty and computational runtime. In a broader perspective, the calculation and plotting of curvature invariants can be made to encompass other types of FTL spaceflight such as the Alcubierre warp drive [30].