Engineering Four-Qubit Fuel States for Protecting Quantum Thermalization Machine from Decoherence
Abstract
:1. Introduction
2. Methods
2.1. Working Basis
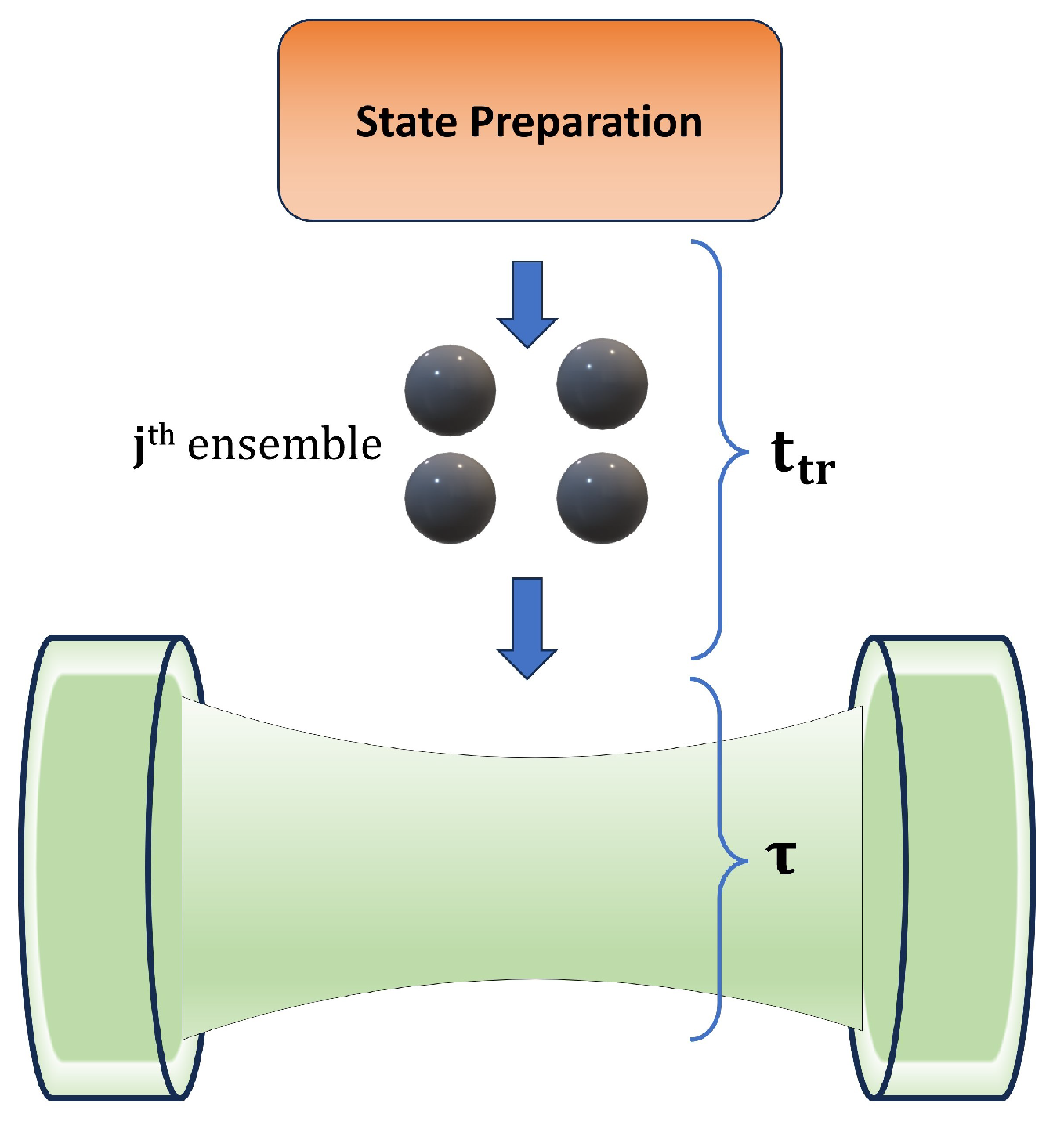
2.2. Quantum Thermalization Machine
2.3. Decoherence Channel
2.4. Realistic Parameter Space
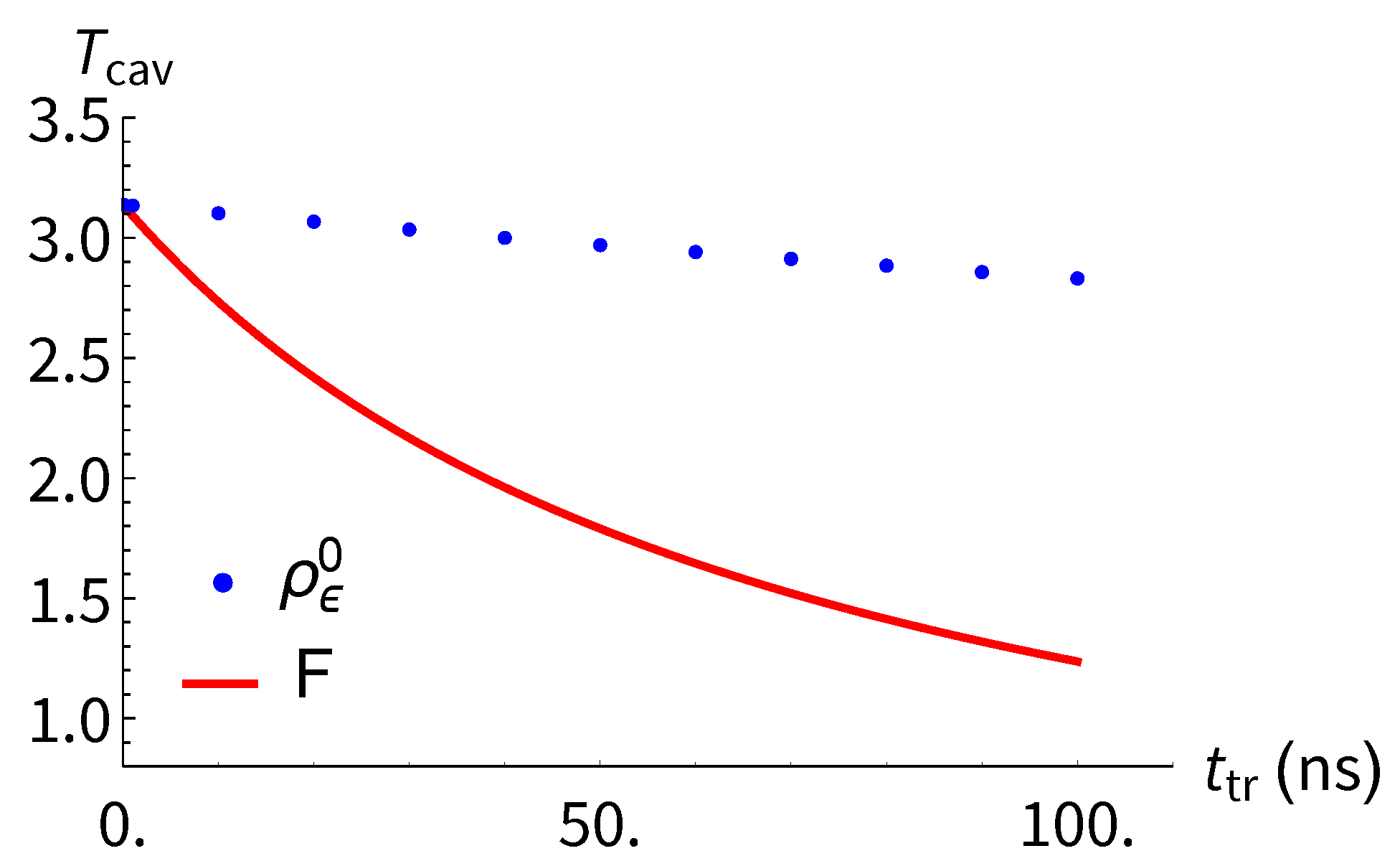
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Horodecki, R.; Horodecki, P.; Horodecki, M.; Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 2009, 81, 865. [Google Scholar] [CrossRef]
- Modi, K.; Brodutch, A.; Cable, H.; Paterek, T.; Vedral, V. The classical-quantum boundary for correlations: Discord and related measures. Rev. Mod. Phys. 2012, 84, 1655. [Google Scholar] [CrossRef]
- Streltsov, A.; Adesso, G.; Plenio, M.B. Colloquium: Quantum coherence as a resource. Rev. Mod. Phys. 2017, 89, 041003. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information: 10th Anniversary Edition; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Madhok, V.; Datta, A. Quantum discord as a resource in quantum communication. Int. J. Mod. Phys. 2013, 27, 1345041. [Google Scholar] [CrossRef]
- Dakić, B.; Lipp, Y.O.; Ma, X.; Ringbauer, M.; Kropatschek, S.; Barz, S.; Paterek, T.; Vedral, V.; Zeilinger, A.; Brukner, Č.; et al. Quantum discord as resource for remote state preparation. Nat. Phys. 2012, 8, 666–670. [Google Scholar] [CrossRef]
- Deffner, S.; Campbell, S. Quantum Thermodynamics: An introduction to the Thermodynamics of Quantum Information; Morgan & Claypool Publishers: San Rafael, CA, USA, 2019. [Google Scholar] [CrossRef]
- Kosloff, R. Quantum thermodynamics: A dynamical viewpoint. Entropy 2013, 15, 2100–2128. [Google Scholar] [CrossRef]
- Horodecki, M. Reversible path to thermodynamics. Nat. Phys. 2008, 4, 833–834. [Google Scholar] [CrossRef]
- Bender, C.M.; Brody, D.C.; Meister, B.K. Quantum mechanical Carnot engine. J. Phys. Math. Gen. 2000, 33, 4427. [Google Scholar] [CrossRef]
- Abe, S. Maximum-power quantum-mechanical Carnot engine. Phys. Rev. 2011, 83, 041117. [Google Scholar] [CrossRef]
- Scully, M.O.; Zubairy, M.S.; Agarwal, G.S.; Walther, H. Extracting Work from a Single Heat Bath via Vanishing Quantum Coherence. Science 2003, 299, 862–864. [Google Scholar] [CrossRef]
- Quan, H.T.; Liu, Y.X.; Sun, C.P.; Nori, F. Quantum thermodynamic cycles and quantum heat engines. Phys. Rev. E 2007, 76, 031105. [Google Scholar] [CrossRef] [PubMed]
- Thomas, G.; Das, D.; Ghosh, S. Quantum heat engine based on level degeneracy. Phys. Rev. E 2019, 100, 012123. [Google Scholar] [CrossRef] [PubMed]
- Çakmak, S. Benchmarking quantum Stirling and Otto cycles for an interacting spin system. JOSA B 2022, 39, 1209–1215. [Google Scholar] [CrossRef]
- Mukherjee, V.; Kofman, A.G.; Kurizki, G. Anti-Zeno quantum advantage in fast-driven heat machines. Commun. Phys. 2020, 3, 8. [Google Scholar] [CrossRef]
- Bouton, Q.; Nettersheim, J.; Burgardt, S.; Adam, D.; Lutz, E.; Widera, A. A quantum heat engine driven by atomic collisions. Nat. Commun. 2021, 12, 2063. [Google Scholar] [CrossRef] [PubMed]
- Horodecki, M.; Horodecki, P.; Horodecki, R. Mixed-state entanglement and distillation: Is there a “bound” entanglement in nature? Phys. Rev. Lett. 1998, 80, 5239. [Google Scholar] [CrossRef]
- Werner, R.F.; Wolf, M.M. Bound entangled Gaussian states. Phys. Rev. Lett. 2001, 86, 3658. [Google Scholar] [CrossRef]
- Yang, D.; Horodecki, M.; Horodecki, R.; Synak-Radtke, B. Irreversibility for all bound entangled states. Phys. Rev. Lett. 2005, 95, 190501. [Google Scholar] [CrossRef]
- Horodecki, M.; Oppenheim, J.; Horodecki, R. Are the laws of entanglement theory thermodynamical? Phys. Rev. Lett. 2002, 89, 240403. [Google Scholar] [CrossRef]
- Lavagno, A.; Scarfone, A.; Swamy, P.N. q-Deformed structures and generalized thermodynamics. Rep. Math. Phys. 2005, 55, 423–433. [Google Scholar] [CrossRef]
- Lavagno, A.; Swamy, P.N. Generalized thermodynamics of q-deformed bosons and fermions. Phys. Rev. E 2002, 65, 036101. [Google Scholar] [CrossRef] [PubMed]
- Ozaydin, F.; Müstecaplıoğlu, Ö.E.; Hakioğlu, T. Powering quantum Otto engines only with q-deformation of the working substance. Phys. Rev. E 2023, 108, 054103. [Google Scholar] [CrossRef] [PubMed]
- Dillenschneider, R.; Lutz, E. Energetics of quantum correlations. Europhys. Lett. 2009, 88, 50003. [Google Scholar] [CrossRef]
- Quan, H. Maximum efficiency of ideal heat engines based on a small system: Correction to the Carnot efficiency at the nanoscale. Phys. Rev. E 2014, 89, 062134. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.; Song, Z. Non-Hermitian heat engine with all-quantum-adiabatic-process cycle. J. Phys. A Math. Theor. 2016, 49, 475301. [Google Scholar] [CrossRef]
- Gardas, B.; Deffner, S. Thermodynamic universality of quantum Carnot engines. Phys. Rev. E 2015, 92, 042126. [Google Scholar] [CrossRef] [PubMed]
- Dağ, C.B.; Niedenzu, W.; Müstecaplıoğlu, Ö.E.; Kurizki, G. Multiatom quantum coherences in micromasers as fuel for thermal and nonthermal machines. Entropy 2016, 18, 244. [Google Scholar] [CrossRef]
- Dag, C.B.; Niedenzu, W.; Ozaydin, F.; Müstecaplıoğlu, O.E.; Kurizki, G. Temperature control in dissipative cavities by entangled dimers. J. Phys. Chem. C 2019, 123, 4035–4043. [Google Scholar] [CrossRef]
- Dür, W.; Vidal, G.; Cirac, J.I. Three qubits can be entangled in two inequivalent ways. Phys. Rev. A 2000, 62, 062314. [Google Scholar] [CrossRef]
- Dicke, R.H. Coherence in spontaneous radiation processes. Phys. Rev. 1954, 93, 99. [Google Scholar] [CrossRef]
- Raussendorf, R.; Briegel, H.J. A one-way quantum computer. Phys. Rev. Lett. 2001, 86, 5188. [Google Scholar] [CrossRef] [PubMed]
- Brassard, G.; Broadbent, A.; Tapp, A. Quantum pseudo-telepathy. Found. Phys. 2005, 35, 1877–1907. [Google Scholar] [CrossRef]
- Briegel, H.J.; Browne, D.E.; Dür, W.; Raussendorf, R.; Van den Nest, M. Measurement-based quantum computation. Nat. Phys. 2009, 5, 19–26. [Google Scholar] [CrossRef]
- Zwerger, M.; Briegel, H.; Dür, W. Measurement-based quantum communication. Appl. Phys. B 2016, 122, 1–15. [Google Scholar] [CrossRef]
- Tame, M.; Özdemir, Ş.; Koashi, M.; Imoto, N.; Kim, M. Compact Toffoli gate using weighted graph states. Phys. Rev. A 2009, 79, 020302. [Google Scholar] [CrossRef]
- D’Hondt, E.; Panangaden, P. The computational power of the W and GHZ States. Quantum Inf. Comput. 2006, 6, 173–183. [Google Scholar] [CrossRef]
- Ma, J.; Huang, Y.X.; Wang, X.; Sun, C. Quantum Fisher information of the Greenberger-Horne-Zeilinger state in decoherence channels. Phys. Rev. A 2011, 84, 022302. [Google Scholar] [CrossRef]
- Ozaydin, F.; Yesilyurt, C.; Bugu, S.; Koashi, M. Deterministic preparation of W states via spin-photon interactions. Phys. Rev. A 2021, 103, 052421. [Google Scholar] [CrossRef]
- Loss, D.; DiVincenzo, D.P. Quantum computation with quantum dots. Phys. Rev. A 1998, 57, 120. [Google Scholar] [CrossRef]
- Häffner, H.; Roos, C.F.; Blatt, R. Quantum computing with trapped ions. Phys. Rep. 2008, 469, 155–203. [Google Scholar] [CrossRef]
- Rotter, I.; Bird, J. A review of progress in the physics of open quantum systems: Theory and experiment. Rep. Prog. Phys. 2015, 78, 114001. [Google Scholar] [CrossRef]
- Weimer, H.; Kshetrimayum, A.; Orús, R. Simulation methods for open quantum many-body systems. Rev. Mod. Phys. 2021, 93, 015008. [Google Scholar] [CrossRef]
- Zhang, Q.; Xia, Y.J.; Man, Z.X. Effects of one-way correlations on thermodynamics of a multipartite open quantum system. Phys. Rev. A 2023, 108, 062211. [Google Scholar] [CrossRef]
- Çakmak, S.; Türkpençe, D.; Altintas, F. Special coupled quantum Otto and Carnot cycles. Eur. Phys. J. Plus 2017, 132, 1–10. [Google Scholar] [CrossRef]
- Türkpençe, D.; Müstecaplıoğlu, O.E. Quantum fuel with multilevel atomic coherence for ultrahigh specific work in a photonic Carnot engine. Phys. Rev. E 2016, 93, 012145. [Google Scholar] [CrossRef]
- Gassab, L.; Pusuluk, O.; Müstecaplıoğlu, Ö.E. Geometrical optimization of spin clusters for the preservation of quantum coherence. arXiv 2023, arXiv:2306.15232. [Google Scholar] [CrossRef]
- Hardal, A.Ü.; Müstecaplıoğlu, Ö.E. Superradiant quantum heat engine. Sci. Rep. 2015, 5, 12953. [Google Scholar] [CrossRef]
- Kim, J.; Oh, S.h.; Yang, D.; Kim, J.; Lee, M.; An, K. A photonic quantum engine driven by superradiance. Nat. Photonics 2022, 16, 707–711. [Google Scholar] [CrossRef]
- Gallock-Yoshimura, K. Relativistic quantum Otto engine: Instant work extraction from a quantum field. arXiv 2023, arXiv:2312.04485. [Google Scholar] [CrossRef]
- Calderbank, A.R.; Shor, P.W. Good quantum error-correcting codes exist. Phys. Rev. A 1996, 54, 1098. [Google Scholar] [CrossRef]
- Knill, E.; Laflamme, R. Theory of quantum error-correcting codes. Phys. Rev. A 1997, 55, 900. [Google Scholar] [CrossRef]
- Lidar, D.A.; Chuang, I.L.; Whaley, K.B. Decoherence-free subspaces for quantum computation. Phys. Rev. Lett. 1998, 81, 2594. [Google Scholar] [CrossRef]
- Yamamoto, T.; Hayashi, K.; Özdemir, Ş.K.; Koashi, M.; Imoto, N. Robust photonic entanglement distribution by state-independent encoding onto decoherence-free subspace. Nat. Photonics 2008, 2, 488–491. [Google Scholar] [CrossRef]
- Bernu, J.; Deléglise, S.; Sayrin, C.; Kuhr, S.; Dotsenko, I.; Brune, M.; Raimond, J.M.; Haroche, S. Freezing coherent field growth in a cavity by the quantum Zeno effect. Phys. Rev. Lett. 2008, 101, 180402. [Google Scholar] [CrossRef] [PubMed]
- Kondo, Y.; Matsuzaki, Y.; Matsushima, K.; Filgueiras, J.G. Using the quantum Zeno effect for suppression of decoherence. New J. Phys. 2016, 18, 013033. [Google Scholar] [CrossRef]
- Yamamoto, T.; Shimamura, J.; Özdemir, Ş.; Koashi, M.; Imoto, N. Faithful qubit distribution assisted by one additional qubit against collective noise. Phys. Rev. Lett. 2005, 95, 040503. [Google Scholar] [CrossRef]
- Mavadia, S.; Frey, V.; Sastrawan, J.; Dona, S.; Biercuk, M.J. Prediction and real-time compensation of qubit decoherence via machine learning. Nat. Commun. 2017, 8, 14106. [Google Scholar] [CrossRef]
- Yu, W.; Chen, Y.; Zhang, C.; Chen, Y.; Wei, H.; Chen, Z.; Zhang, Y. A Software Architecting for Quantum Machine Learning Platform in Noisy Intermediate-Scale Quantum Era. Res. Sq. 2023; preprint. [Google Scholar] [CrossRef]
- Tuncer, A.; Izadyari, M.; Dağ, C.B.; Ozaydin, F.; Müstecaplıoğlu, O.E. Work and heat value of bound entanglement. Quantum Inf. Process. 2019, 18, 373. [Google Scholar] [CrossRef]
- Meschede, D.; Walther, H.; Müller, G. One-atom maser. Phys. Rev. Lett. 1985, 54, 551. [Google Scholar] [CrossRef]
- Tavis, M.; Cummings, F.W. Exact solution for an N-molecule—Radiation-field Hamiltonian. Phys. Rev. 1968, 170, 379. [Google Scholar] [CrossRef]
- Filipowicz, P.; Javanainen, J.; Meystre, P. Theory of a microscopic maser. Phys. Rev. A 1986, 34, 3077. [Google Scholar] [CrossRef] [PubMed]
- Liao, J.Q.; Dong, H.; Sun, C. Single-particle machine for quantum thermalization. Phys. Rev. A 2010, 81, 052121. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Commun. Math. Phys. 1976, 48, 119–130. [Google Scholar] [CrossRef]
- Khatri, S.; Sharma, K.; Wilde, M.M. Information-theoretic aspects of the generalized amplitude-damping channel. Phys. Rev. A 2020, 102, 012401. [Google Scholar] [CrossRef]
- Louisell, W.H. Quantum Statistical Properties of Radiation; John Wiley: Hoboken, NJ, USA, 1990. [Google Scholar]
- Zhong, W.; Sun, Z.; Ma, J.; Wang, X.; Nori, F. Fisher information under decoherence in Bloch representation. Phys. Rev. A 2013, 87, 022337. [Google Scholar] [CrossRef]
- Wallraff, A.; Schuster, D.I.; Blais, A.; Frunzio, L.; Majer, J.; Devoret, M.H.; Girvin, S.M.; Schoelkopf, R.J. Approaching unit visibility for control of a superconducting qubit with dispersive readout. Phys. Rev. Lett. 2005, 95, 060501. [Google Scholar] [CrossRef]
- Yesilyurt, C.; Bugu, S.; Ozaydin, F.; Altintas, A.A.; Tame, M.; Yang, L.; Özdemir, S.K. Deterministic Local Doubling of W states. J. Opt. Soc. Am. B 2016, 33, 2313. [Google Scholar] [CrossRef]
- Zang, X.P.; Yang, M.; Ozaydin, F.; Song, W.; Cao, Z.L. Generating Multi-Atom Entangled W States via Light-Matter Interface Based Fusion Mechanism. Sci. Rep. 2015, 5, 16245. [Google Scholar] [CrossRef]
- Zang, X.P.; Yang, M.; Ozaydin, F.; Song, W.; Cao, Z.L. Deterministic Generation of Large Scale Atomic W States. Opt. Exp. 2015, 24, 12293. [Google Scholar] [CrossRef]
- Kobayashi, T.; Ikuta, R.; Özdemir, Ş.K.; Tame, M.; Yamamoto, T.; Koashi, M.; Imoto, N. Universal gates for transforming multipartite entangled Dicke states. New J. Phys. 2014, 16, 023005. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ozaydin, F.; Sarkar, R.; Bayrakci, V.; Bayındır, C.; Altintas, A.A.; Müstecaplıoğlu, Ö.E. Engineering Four-Qubit Fuel States for Protecting Quantum Thermalization Machine from Decoherence. Information 2024, 15, 35. https://doi.org/10.3390/info15010035
Ozaydin F, Sarkar R, Bayrakci V, Bayındır C, Altintas AA, Müstecaplıoğlu ÖE. Engineering Four-Qubit Fuel States for Protecting Quantum Thermalization Machine from Decoherence. Information. 2024; 15(1):35. https://doi.org/10.3390/info15010035
Chicago/Turabian StyleOzaydin, Fatih, Ramita Sarkar, Veysel Bayrakci, Cihan Bayındır, Azmi Ali Altintas, and Özgür E. Müstecaplıoğlu. 2024. "Engineering Four-Qubit Fuel States for Protecting Quantum Thermalization Machine from Decoherence" Information 15, no. 1: 35. https://doi.org/10.3390/info15010035





