Kinematic Analysis of a New 3-DOF Parallel Wrist-Gripper Assembly with a Large Singularity-Free Workspace
Abstract
:1. Introduction
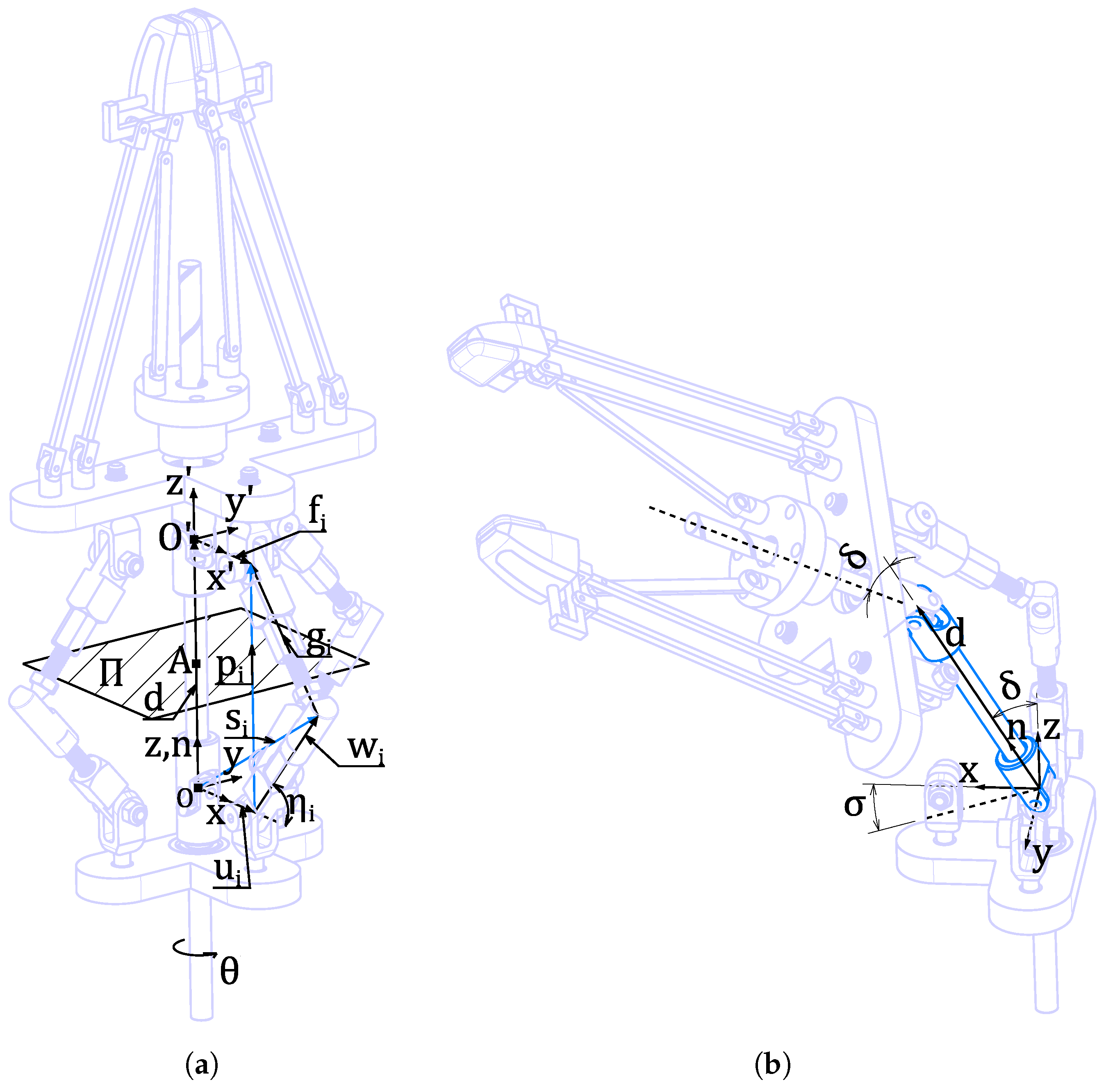
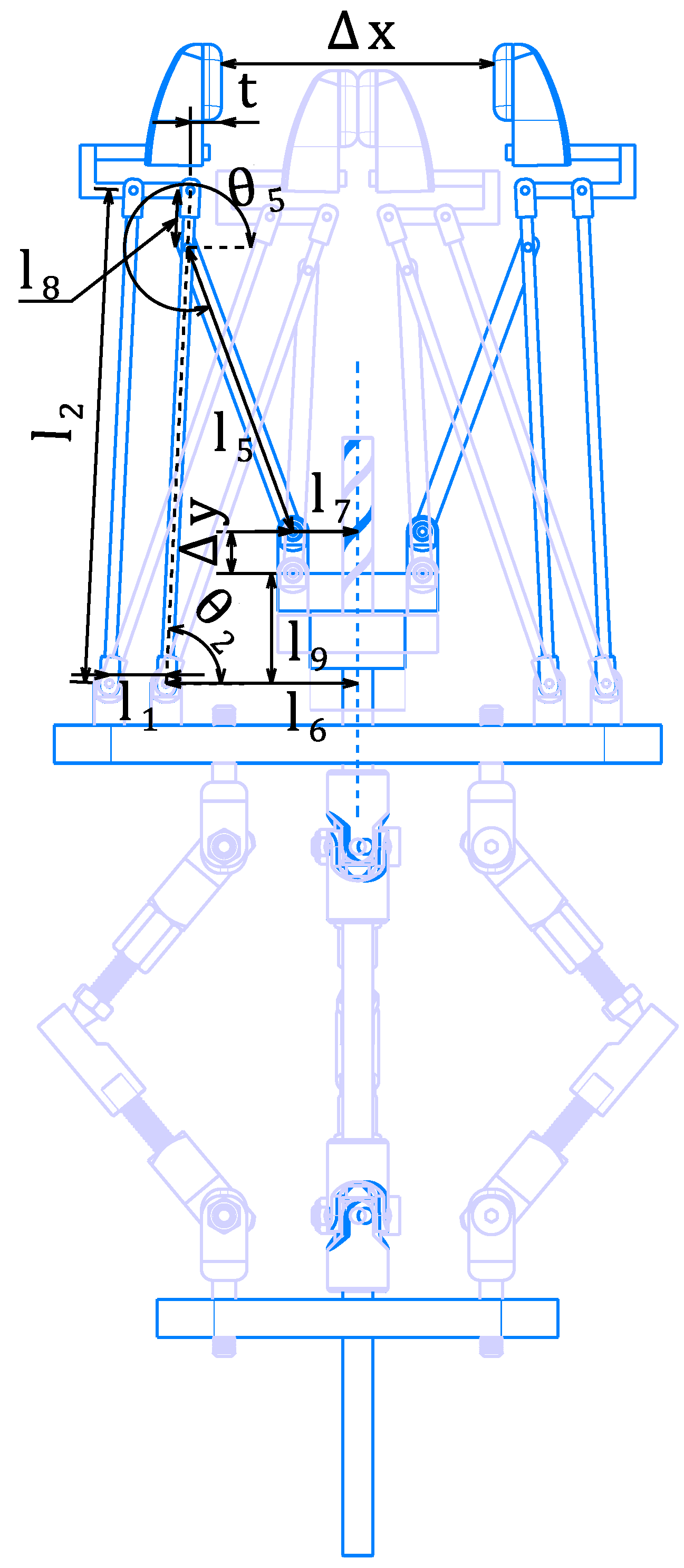
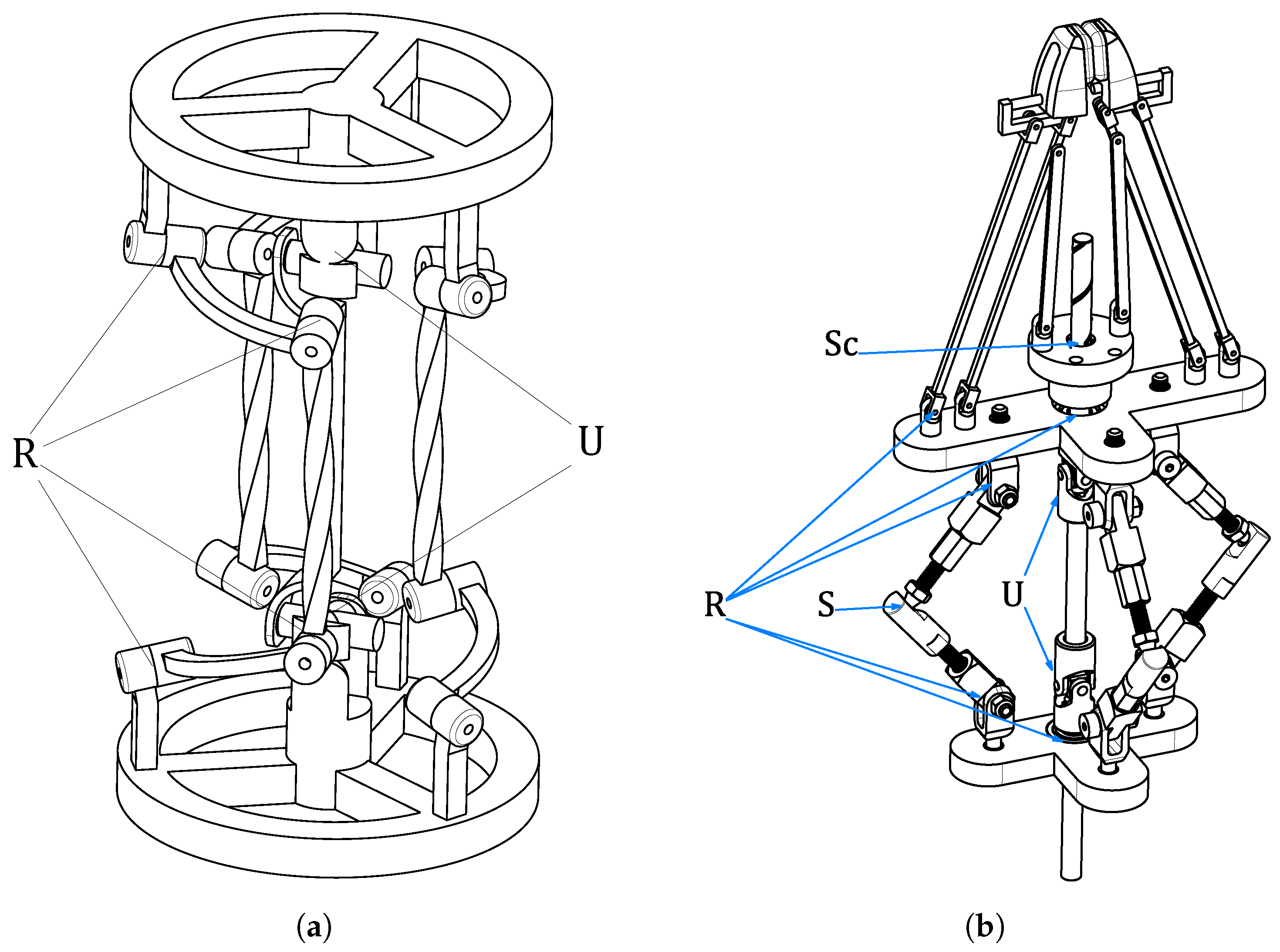
2. Robot Architecture
3. Kinematic Modelling
3.1. Inverse Kinematics Modelling (Wrist)
3.2. Forward Kinematic Modelling (Wrist)
3.3. Inverse Kinematic Modelling (Gripper)
3.4. Forward Kinematic Modelling (Gripper)
3.5. Wrist Jacobian Matrices (Method I)
3.6. Wrist Jacobian Matrices (Method II)
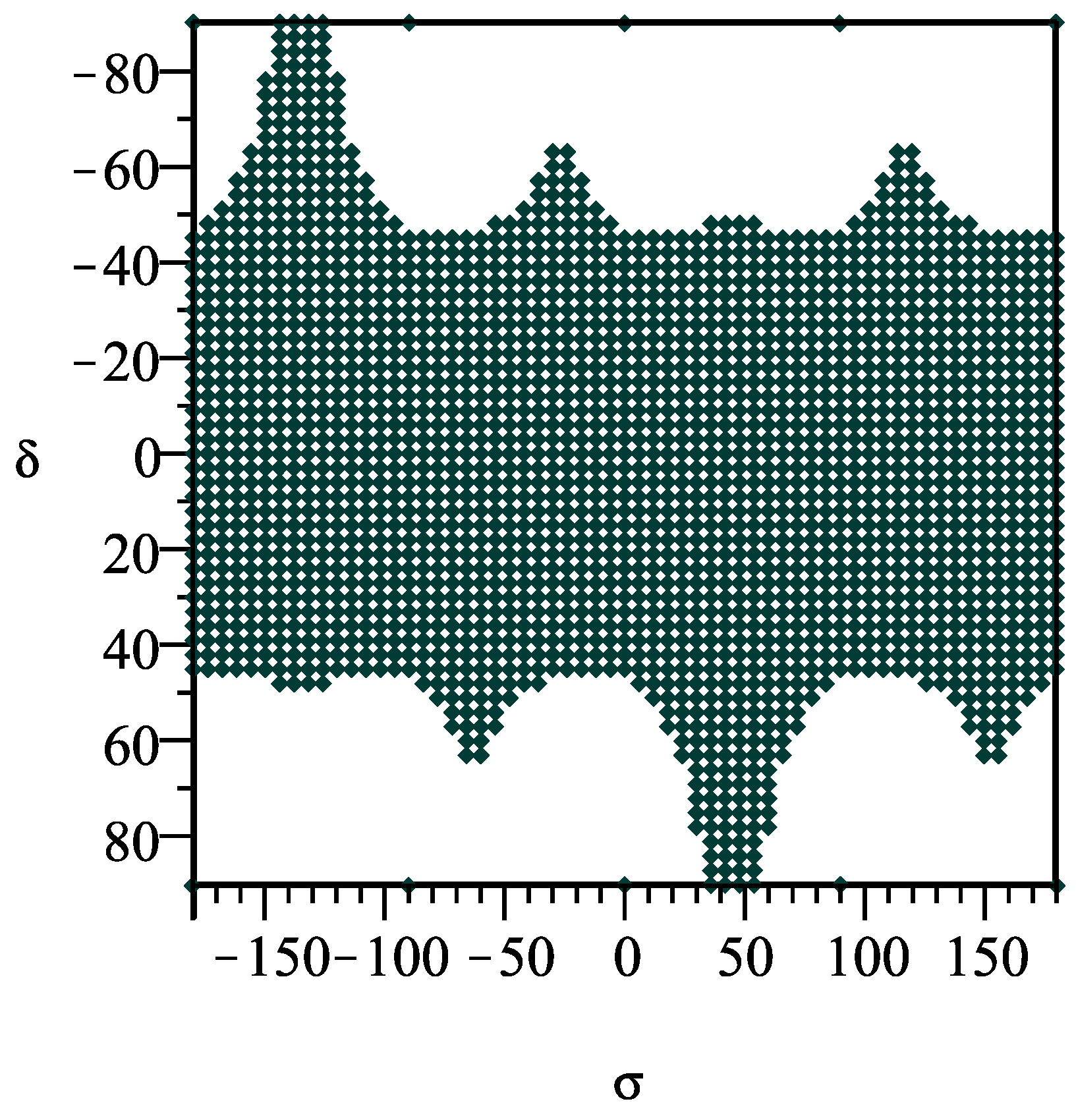
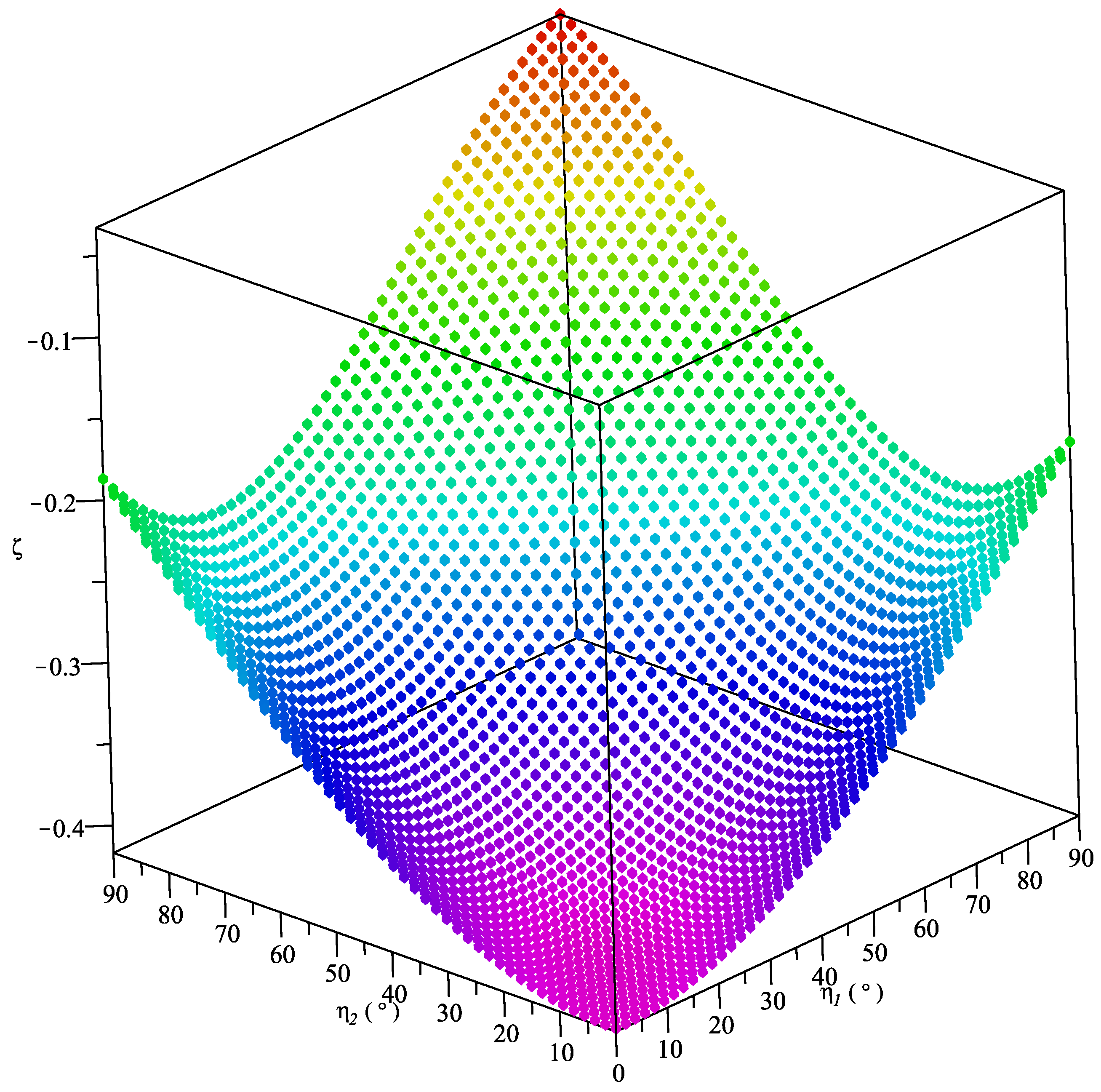
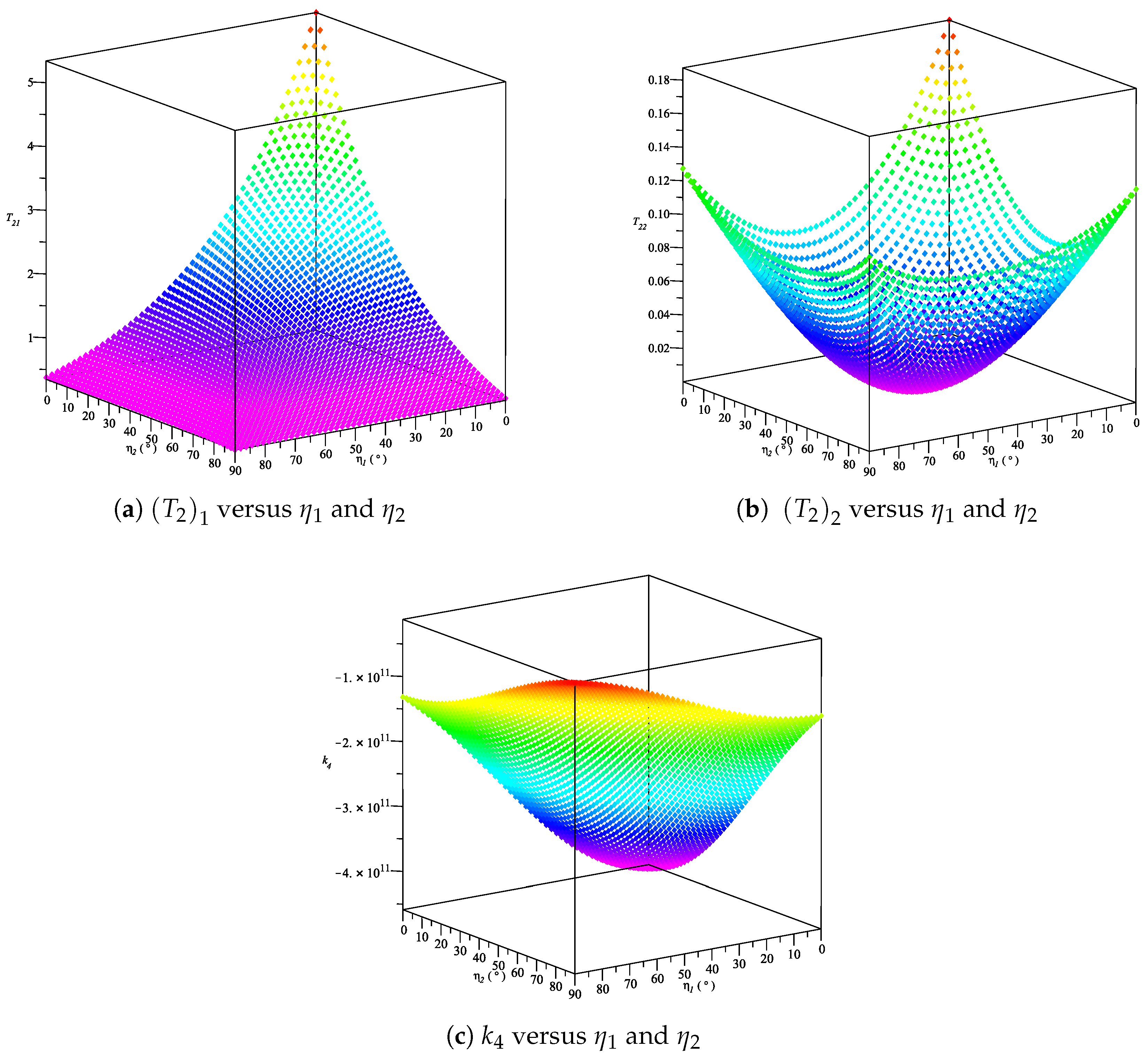
4. Singularity Analysis (Wrist)
5. Workspace Analysis (Wrist)
6. Simulation
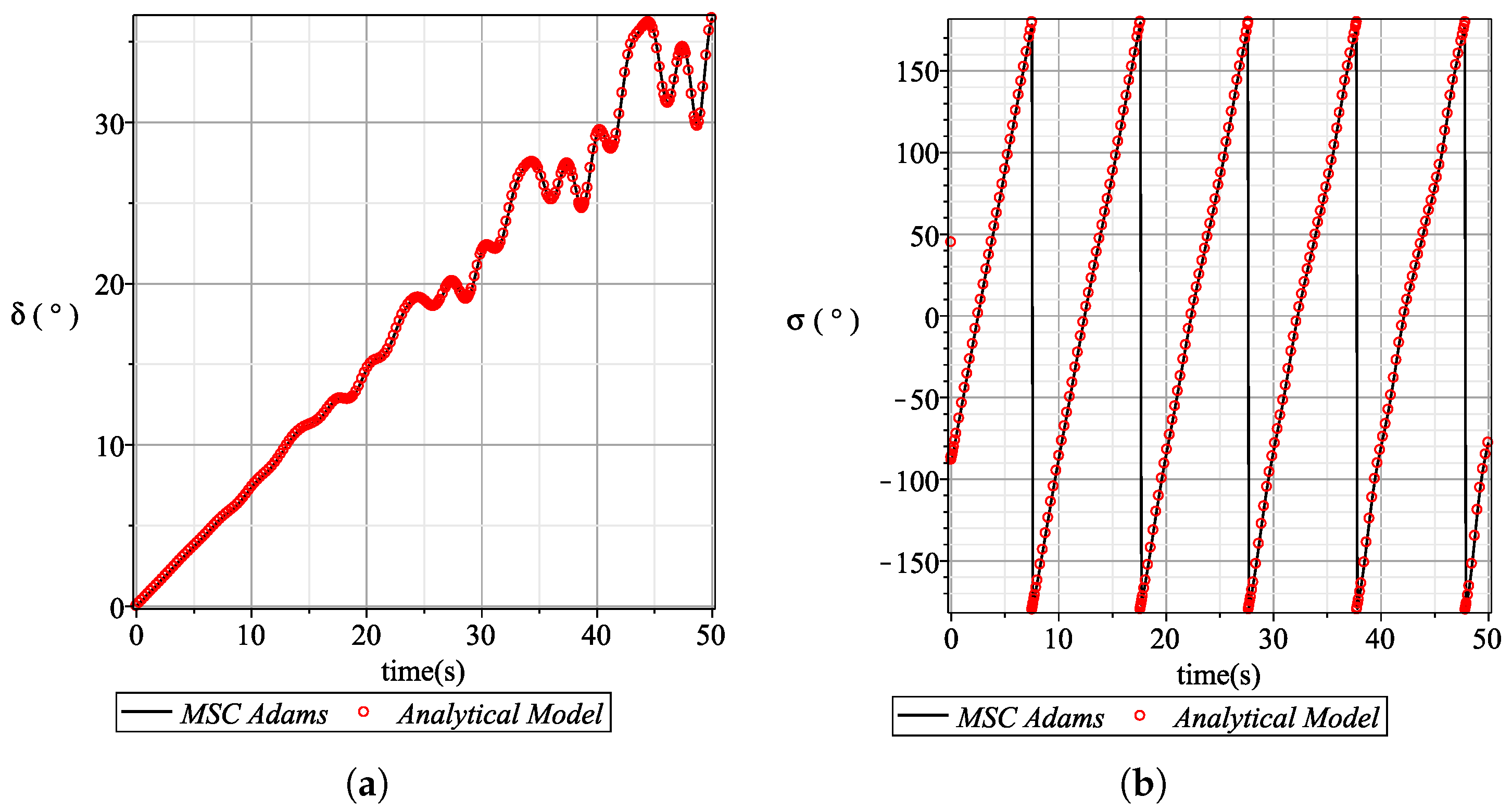
6.1. Inverse Kinematics Verification
6.2. Forward Kinematics Verification
7. Comparison with the State of the Art
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| PMs | Parallel manipulators |
| SPMs | Spherical parallel manipulators |
| FCOR | Fixed centre of rotation |
| ISA | Instantaneous screw axis |
| RPM | Rotational parallel manipulators |
| ESPRs | Equal spherical pure rotations |
| FCCPs | Freedom and complementary constraint patterns |
| MIS | Minimally invasive surgery |
| RMSE | Root mean square error |
References
- Merlet, J.P.; Gosselin, C.; Huang, T. Parallel mechanisms. In Springer Handbook of Robotics; Springer International Publishing: Berlin/Heidelberg, Germany, 2016; pp. 443–462. [Google Scholar]
- Ghaedrahmati, R.; Raoofian, A.; Kamali, A.; Taghvaeipour, A. An enhanced inverse dynamic and joint force analysis of multibody systems using constraint matrices. Multibody Syst. Dyn. 2019, 46, 329–353. [Google Scholar] [CrossRef]
- Gosselin, C.; Schreiber, L.T. Kinematically redundant spatial parallel mechanisms for singularity avoidance and large orientational workspace. IEEE Trans. Robot. 2016, 32, 286–300. [Google Scholar] [CrossRef]
- Gosselin, C.; Hamel, J.F. The agile eye: A high-performance three-degree-of-freedom camera-orienting device. In Proceedings of the 1994 IEEE International Conference on Robotics and Automation, San Diego, CA, USA, 8–13 May 1994; Volume 1, pp. 781–786. [Google Scholar]
- Bidault, F.; Teng, C.P.; Angeles, J. Structural optimization of a spherical parallel manipulator using a two-level approach. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Pittsburgh, PA, USA, 9–12 September 2001; Volume 80227, pp. 215–224. [Google Scholar]
- Bai, S.; Li, X.; Angeles, J. A review of spherical motion generation using either spherical parallel manipulators or spherical motors. Mech. Mach. Theory 2019, 140, 377–388. [Google Scholar] [CrossRef]
- Gosselin, C.M.; Caron, F. Two Degree-of-Freedom Spherical Orienting Device. U.S. Patent 5966991, 19 October 1999. [Google Scholar]
- Ueda, K.; Yamada, H.; Ishida, H.; Hirose, S. Design of Large Motion Range and Heavy Duty 2-DOF Spherical Parallel Wrist Mechanism. J. Robot. Mechatronics 2013, 25, 294–305. [Google Scholar] [CrossRef]
- Duan, X.; Yang, Y.; Cheng, B. Modeling and Analysis of a 2-DOF Spherical Parallel Manipulator. Sensors 2016, 16, 1485. [Google Scholar] [CrossRef] [PubMed]
- Cammarata, A. Optimized design of a large-workspace 2-DOF parallel robot for solar tracking systems. Mech. Mach. Theory 2015, 83, 175–186. [Google Scholar] [CrossRef]
- Carricato, M.; Parenti-Castelli, V. A novel fully decoupled two-degrees-of-freedom parallel wrist. Int. J. Robot. Res. 2004, 23, 661–667. [Google Scholar] [CrossRef]
- Bajaj, N.M.; Spiers, A.J.; Dollar, A.M. State of the Art in Artificial Wrists: A Review of Prosthetic and Robotic Wrist Design. IEEE Trans. Robot. 2019, 35, 261–277. [Google Scholar] [CrossRef]
- Sofka, J.; Skormin, V.A.; Nikulin, V.V.; Nicholson, D.J.; Rosheim, M. New generation of gimbal systems for laser positioning applications. In Proceedings of the Free-Space Laser Communication and Active Laser Illumination III, International Society for Optics and Photonics, San Diego, CA, USA, 27 January 2004; Volume 5160, pp. 182–191. [Google Scholar]
- Dong, X.; Yu, J.; Chen, B.; Zong, G. Geometric approach for kinematic analysis of a class of 2-DOF rotational parallel manipulators. Chin. J. Mech. Eng. 2012, 25, 241–247. [Google Scholar] [CrossRef]
- Wu, Y.; Carricato, M. Synthesis and singularity analysis of N-UU parallel wrists: A symmetric space approach. J. Mech. Robot. 2017, 9, 051013. [Google Scholar] [CrossRef]
- Shah, D.; Metta, G.; Parmiggiani, A. Workspace analysis and the effect of geometric parameters for parallel mechanisms of the N-UU class. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Quebec City, QC, Canada, 26–29 August 2018; Volume 51807, p. 05. [Google Scholar]
- Chang-Siu, E.; Snell, A.; McInroe, B.W.; Balladarez, X.; Full, R.J. How to use the Omni-Wrist III for dexterous motion: An exposition of the forward and inverse kinematic relationships. Mech. Mach. Theory 2022, 168, 104601. [Google Scholar] [CrossRef]
- Dunlop, G.; Jones, T. Position analysis of a two DOF parallel mechanism—The Canterbury tracker. Mech. Mach. Theory 1999, 34, 599–614. [Google Scholar] [CrossRef]
- Rosheim, M.E.; Sauter, G.F. New high-angulation omnidirectional sensor mount. In Proceedings of the Free-Space Laser Communication and Laser Imaging II; Ricklin, J.C., Voelz, D.G., Eds.; International Society for Optics and Photonics; SPIE: Seattle, WA, USA, 2002; Volume 4821, pp. 163–174. [Google Scholar]
- Wu, K.; Yu, J.; Zong, G.; Kong, X. A Family of Rotational Parallel Manipulators with Equal-Diameter Spherical Pure Rotation. J. Mech. Robot. 2013, 6, 011008. [Google Scholar] [CrossRef]
- Yu, J.; Dong, X.; Pei, X.; Zong, G.; Kong, X.; Qiu, Q. Mobility and Singularity Analysis of a Class of 2-DOF Rotational Parallel Mechanisms Using a Visual Graphic Approach. In Proceedings of the ASME 2011 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Washington, DC, USA, 28–31 August 2011; Volume 4. [Google Scholar]
- Yu, J.; Duan, Z.; Xie, Y. Kinematics analysis of n-4R reconfigurable parallel mechanisms. In Advances in Reconfigurable Mechanisms and Robots II; Springer: Berlin/Heidelberg, Germany, 2016; pp. 275–285. [Google Scholar]
- Gosselin, C.; Isaksson, M.; Marlow, K.; Laliberté, T. Workspace and Sensitivity Analysis of a Novel Nonredundant Parallel SCARA Robot Featuring Infinite Tool Rotation. IEEE Robot. Autom. Lett. 2016, 1, 776–783. [Google Scholar] [CrossRef]
- Van Meer, F.; Giraud, A.; Esteve, D.; Dollat, X. A disposable plastic compact wrist for smart minimally invasive surgical tools. In Proceedings of the 2005 IEEE/RSJ International Conference on Intelligent Robots and Systems, Edmonton, AB, Canada, 2–6 August 2005; pp. 919–924. [Google Scholar]
- Li, K.; Li, J.; Li, L.; Ji, S.; Meng, X.; Fu, Y. Mechanical Design of a 4-DOF Minimally Invasive Surgical Instrument. In Proceedings of the 2019 IEEE International Conference on Robotics and Biomimetics (ROBIO), Dali, China, 6–8 December 2019; pp. 930–935. [Google Scholar]
- Navarro, J.S.; Garcia, N.; Perez, C.; Fernandez, E.; Saltaren, R.; Almonacid, M. Kinematics of a robotic 3UPS1S spherical wrist designed for laparoscopic applications. Int. J. Med. Robot. Comput. Assist. Surg. 2010, 6, 291–300. [Google Scholar] [CrossRef] [PubMed]
- Haouas, W.; Dahmouche, R.; Le Fort-Piat, N.; Laurent, G.J. 4-DoF spherical parallel wrist with embedded grasping capability for minimally invasive surgery. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Republic of Korea, 9–14 October 2016; pp. 2363–2368. [Google Scholar]
- Yamashita, H.; Kim, D.; Hata, N.; Dohi, T. Multi-slider linkage mechanism for endoscopic forceps manipulator. In Proceedings of the 2003 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS 2003) (Cat. No. 03CH37453), Las Vegas, NV, USA, 27–31 October 2003; Volume 3, pp. 2577–2582. [Google Scholar]
- Hong, M.B.; Jo, Y.H. Design of a Novel 4-DOF Wrist-Type Surgical Instrument With Enhanced Rigidity and Dexterity. IEEE/ASME Trans. Mechatron. 2014, 19, 500–511. [Google Scholar] [CrossRef]
- Bazman, M.; Yilmaz, N.; Tumerdem, U. An Articulated Robotic Forceps Design With a Parallel Wrist-Gripper Mechanism and Parasitic Motion Compensation. J. Mech. Des. 2022, 144, 063303. [Google Scholar] [CrossRef]
- Sanchez, D. Surgical Instrument with a Universal Wrist. U.S. Patent 7121781, 17 October 2006. [Google Scholar]
- Ghaedrahmati, R.; Gosselin, C. Kinematic analysis of a new 2-DOF parallel wrist with a large singularity-free rotational workspace. Mech. Mach. Theory 2022, 175, 104942. [Google Scholar] [CrossRef]
- Bonev, I.A. Direct kinematics of zero-torsion parallel mechanisms. In Proceedings of the 2008 IEEE International Conference on Robotics and Automation, Pasadena, CA, USA, 19–23 May 2008; pp. 3851–3856. [Google Scholar]
- Laidacker, M. Another theorem relating Sylvester’s matrix and the greatest common divisor. Math. Mag. 1969, 42, 126–128. [Google Scholar] [CrossRef]
- Sone, K.; Isobe, H.; Yamada, K. High angle active link. In Special Issue Special Supplement to Industrial Machines, NTN Technical Review No.71; NTN: Osaka, Japan, 2004. [Google Scholar]
- Ogata, M.; Hirose, S. Study on ankle mechanism for walking robots: Development of 2 dof coupled drive ankle mechanism with wide motion range. In Proceedings of the 2004 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS) (IEEE Cat. No. 04CH37566), Sendai, Japan, 28 September–2 October 2004; Volume 4, pp. 3201–3206. [Google Scholar]
- Kim, Y.J.; Kim, J.I.; Jang, W. Quaternion Joint: Dexterous 3-DOF Joint Representing Quaternion Motion for High-Speed Safe Interaction. In Proceedings of the 2018 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Madrid, Spain, 1–5 October 2018; pp. 935–942. [Google Scholar]
- Takeda, Y.; Funabashi, H.; Sasaki, Y. Development of a spherical in-parallel actuated mechanism with three degrees of freedom with large working space and high motion transmissibility: Evaluation of motion transmissibility and analysis of working space. JSME Int. J. Ser. C Dyn. Control Robot. Des. Manuf. 1996, 39, 541–548. [Google Scholar] [CrossRef]
- Bazman, M.; Yilmaz, N.; Tumerdem, U. Dexterous and back-drivable parallel robotic forceps wrist for robotic surgery. In Proceedings of the 2018 IEEE 15th International Workshop on Advanced Motion Control (AMC), Tokyo, Japan, 9–11 March 2018; pp. 153–159. [Google Scholar]


| Reference | DOFs | Range of Motion | Parallel Gripper |
|---|---|---|---|
| Sone et al. [35] | 2 | Tilt = 90, Azimuth = | |
| Ogata et al. [36] | 2 | Pitch = , Yaw = | |
| Ueda et al. [8] | 2 | Pitch = , Yaw = | |
| Cammarata [10] | 2 | Tilt = 90, Azimuth = | |
| Ghaedrahmati and Gosselin [32] | 2 | Tilt = 90, Azimuth = | |
| OMNI Wrist III [19] | 2 | Tilt = 90, Azimuth = | |
| Kim et al. [37] | 3 | Tilt = 90, Azimuth = , Pitch = | |
| Takeda et al. [38] | 3 | Tilt = , Azimuth = , Roll = −40–0 | |
| Bazman et al. [39] | 4 | Pitch = , Yaw = , Roll = |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghaedrahmati, R.; Gosselin, C. Kinematic Analysis of a New 3-DOF Parallel Wrist-Gripper Assembly with a Large Singularity-Free Workspace. Actuators 2023, 12, 421. https://doi.org/10.3390/act12110421
Ghaedrahmati R, Gosselin C. Kinematic Analysis of a New 3-DOF Parallel Wrist-Gripper Assembly with a Large Singularity-Free Workspace. Actuators. 2023; 12(11):421. https://doi.org/10.3390/act12110421
Chicago/Turabian StyleGhaedrahmati, Ramin, and Clément Gosselin. 2023. "Kinematic Analysis of a New 3-DOF Parallel Wrist-Gripper Assembly with a Large Singularity-Free Workspace" Actuators 12, no. 11: 421. https://doi.org/10.3390/act12110421





