The Collins Asymmetry in Λ Hyperon Produced SIDIS Process at Electron–Ion Colliders
Abstract
:1. Introduction
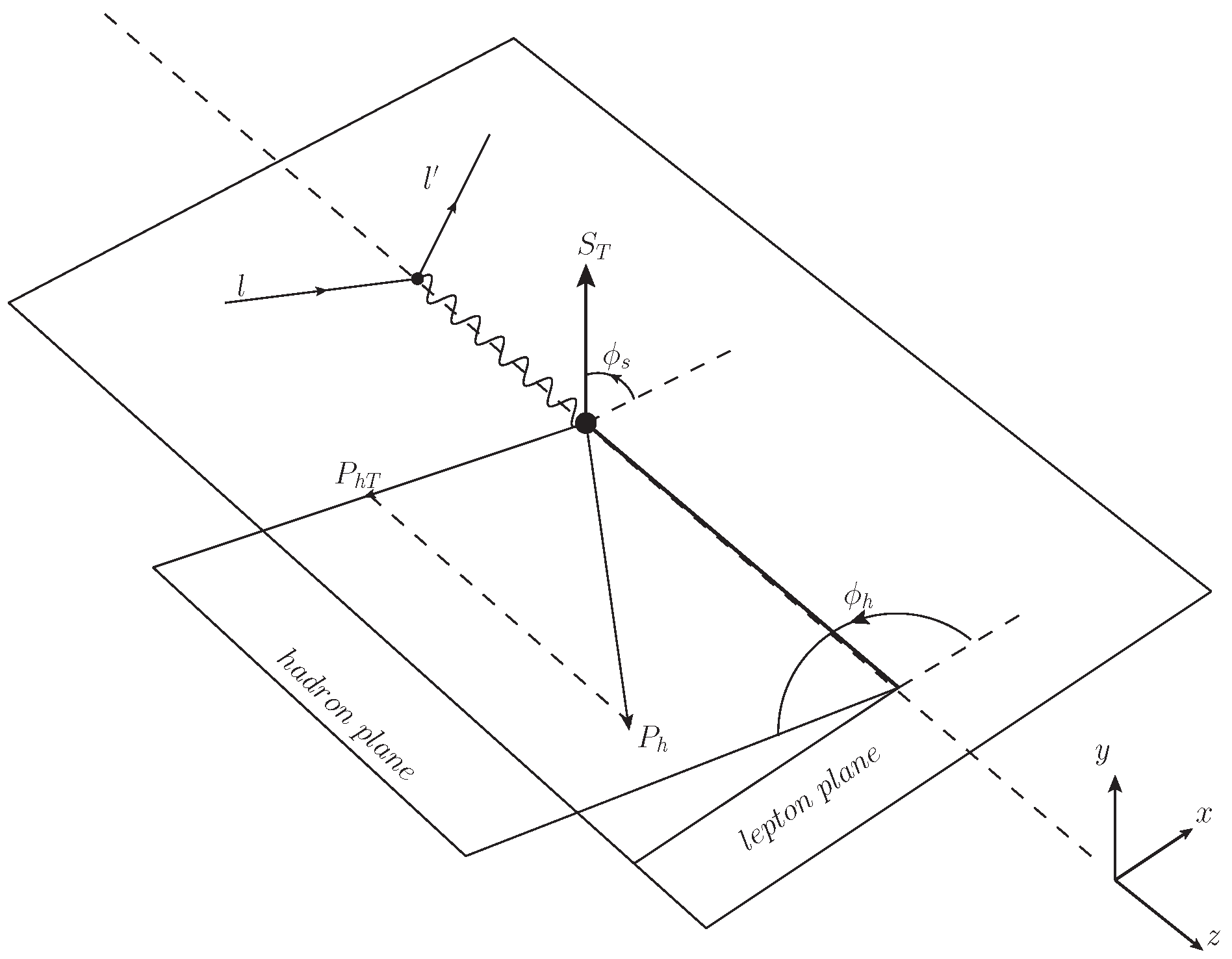
2. Formalism of the Collins Asymmetry in SIDIS Process
2.1. TMD Evolution Effects
2.2. The Solution of the Unpolarized Structure Function
2.3. The Solution of the Transverse Spin-Dependent Structure Function
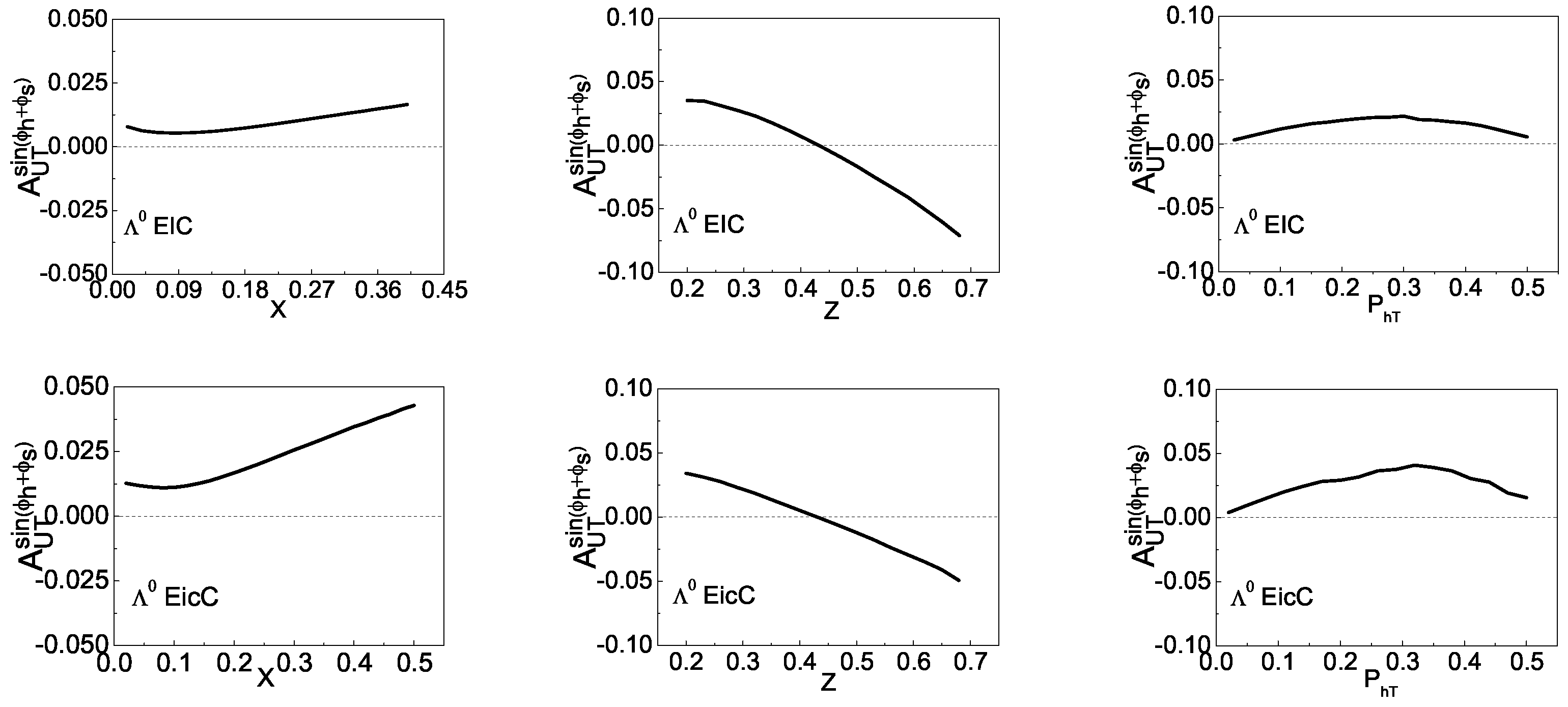
3. Numerical Estimate
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| CS | Collins-Soper |
| CSS | Collins-Soper-Sterman |
| DGLAP | Dokshitzer-Gribov-Lipatov-Altarelli-Parisi |
| DIS | Deeply inelastic scattering |
| EIC | Electron–ion collider |
| EicC | Electron–ion collider in China |
| FF | Fragmentation functions |
| NICA | Nuclotron-based Ion Collider fAcility |
| Parton Distribution Function | |
| QCD | Quantum Chromodynamics |
| SIDIS | Semi-inclusive deep inelastic scattering |
| SPD | Spin Physics Detector |
| TMD | Transverse Momentum Dependent |
References
- Ashman; Badelek, J.; Baum, B.; Beaufays, G.; Bee, J.; Benchouk, C.P.; Bird, C.; Brown, I.G.; Caputo, S.C.; Cheung, M.C.; et al. A Measurement of the Spin Asymmetry and Determination of the Structure Function g(1) in Deep Inelastic Muon-Proton Scattering. Phys. Lett. B 1988, 206, 364. [Google Scholar] [CrossRef] [Green Version]
- Ashman, J.; Badelek, B.; Baum, G.; Beaufays, J.; Bee, C.P.; Benchouk, C.; Bird, I.G.; Brown, S.C.; Caputo, M.C.; Cheung, H.W.K.; et al. An Investigation of the Spin Structure of the Proton in Deep Inelastic Scattering of Polarized Muons on Polarized Protons. Nucl. Phys. B 1989, 328, 1. [Google Scholar] [CrossRef] [Green Version]
- Jaffe, R.L.; Ji, X. Chiral odd parton distributions and polarized Drell–Yan. Phys. Rev. Lett. 1991, 67, 552. [Google Scholar] [CrossRef] [PubMed]
- Bacchetta, A.; Courtoy, A.; Radici, M. First glances at the transversity parton distribution through dihadron fragmentation functions. Phys. Rev. Lett. 2011, 107, 012001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, X.; Lu, Z. Predicting the sinϕS transverse single-spin asymmetry of pion production at an electron–ion collider. Phys. Rev. D 2016, 93, 074009. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Wang, X.; Lu, Z. Predicting the sinϕS Single-Spin-Asymmetry of Λ Production off transversely polarized nucleon in SIDIS. Phys. Rev. D 2021, 103, 114011. [Google Scholar] [CrossRef]
- Collins, J.C.; Soper, D.E. Back-To-Back Jets in QCD. Nucl. Phys. B 1981, 193, 381, Erratum in Nucl. Phys. B 1983, 213, 543. [Google Scholar] [CrossRef]
- Collins, J. Foundations of Perturbative QCD; Cambridge University Press: Cambridge, UK, 2013; Volume 32, ISBN 978-1-107-64525-7. [Google Scholar]
- Collins, J.; Soper, D.E.; Sterman, G. Transverse Momentum Distribution in Drell–Yan Pair and W and Z Boson Production. Nucl. Phys. B 1985, 250, 199. [Google Scholar] [CrossRef] [Green Version]
- Ji, X.; Ma, J.-P.; Yuan, F. QCD factorization for spin-dependent cross sections in DIS and Drell–Yan processes at low transverse momentum. Phys. Lett. B 2004, 597, 299. [Google Scholar] [CrossRef] [Green Version]
- Collins, J. Fragmentation of transversely polarized quarks probed in transverse momentum distributions. Nucl. Phys. B 1993, 396, 161. [Google Scholar] [CrossRef] [Green Version]
- Airapetian, A.; Akopov, N.; Akopov, Z.; Amarian, M.; Andrus, A.; Aschenauer, E.C.; Augustyniak, W.; Avakian, R.; Avetissian, A.; Bacchetta, A.; et al. Single-spin asymmetries in semi-inclusive deep-inelastic scattering on a transversely polarized hydrogen target. Phys. Rev. Lett. 2005, 94, 012002. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Airapetian, A.; Akopov, N.; Akopov, Z.; Aschenauer, E.C.; Augustyniak, W.; Avetissian, A.; Avetisyan, E.; Bacchetta, A.; Ball, B.; Bianchi, N.; et al. Observation of the Naive-T-odd Sivers Effect in Deep-Inelastic Scattering. Phys. Rev. Lett. 2009, 103, 152002. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Airapetian, A.; Akopov, N.; Akopov, Z.; Aschenauer, E.; Augustyniak, W.; Avakian, R.; Avetissian, A.; Avetisyan, E.; Bacchetta, A.; Belostotski, S.; et al. Effects of transversity in deep-inelastic scattering by polarized protons. Phys. Lett. B 2010, 693, 11. [Google Scholar] [CrossRef]
- Airapetian, A.; Akopov, N.; Akopov, Z.; Aschenauer, E.C.; Augustyniak, W.; Avakian, R.; Avetissian, A.; Avetisyan, E.; Belostotski, S.; Blok, H.P.; et al. Azimuthal distributions of charged hadrons, pions, and kaons produced in deep-inelastic scattering off unpolarized protons and deuterons. Phys. Rev. D 2013, 87, 012010. [Google Scholar] [CrossRef] [Green Version]
- Adolph, C.; Alekseev, M.G.; Alexakhin, V.Y.; Alexandrov, Y.; Alexeev, G.D.; Amoroso, A.; Antonov, A.A.; Austregesilo, A.; Badełek, B.; Balestra, F.; et al. Experimental investigation of transverse spin asymmetries in muon-p SIDIS processes: Collins asymmetries. Phys. Lett. B 2012, 717, 376. [Google Scholar]
- Qian, X.; Allada, K.; Dutta, C.; Huang, J.; Katich, J.; Wang, Y.; Zhang, Y.; Aniol, K.; Ann, J.R.M.; Averett, T.; et al. Single Spin Asymmetries in Charged Pion Production from Semi-Inclusive Deep Inelastic Scattering on a Transversely Polarized 3He Target. Phys. Rev. Lett. 2011, 107, 072003. [Google Scholar] [CrossRef]
- Zhao, Y.X.; Wang, Y.; Allada, K.; Aniol, K.; Annand, J.R.M.; Averett, T.; Benmokhtar, F.; Bertozzi, W.; Bradshaw, P.C.; Bosted, P.; et al. Single spin asymmetries in charged kaon production from semi-inclusive deep inelastic scattering on a transversely polarized 3He target. Phys. Rev. C 2014, 90, 055201. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; D’Alesio, U.; Kotzinian, A.; Murgia, F.; Prokudin, A.; Türk, C. Transversity and Collins functions from SIDIS and e+ e- data. Phys. Rev. D 2007, 75, 054032. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; D’Alesio, U.; Kotzinian, A.; Murgia, F.; Prokudin, A.; Melis, S. Update on transversity and Collins functions from SIDIS and e+ e- data. Nucl. Phys. B Proc. Suppl. 2009, 191, 98. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; D’Alesio, U.; Melis, S.; Murgia, F.; Prokudin, A. Simultaneous extraction of transversity and Collins functions from new SIDIS and e+e- data. Phys. Rev. D 2013, 87, 094019. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; D’Alesio, U.; Hernandez, J.G.; Melis, S.; Murgia, F.; Prokudin, A. Collins functions for pions from SIDIS and new e+e− data: A first glance at their transverse momentum dependence. Phys. Rev. D 2015, 92, 114023. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; D’Alesio, U.; Hernandez, J.G.; Melis, S.; Murgia, F.; Prokudin, A. Extracting the Kaon Collins function from e+e− hadron pair production data. Phys. Rev. D 2016, 93, 034025. [Google Scholar] [CrossRef] [Green Version]
- Xue, S.-C.; Wang, X.; Li, D.-M.; Lu, Z. The Collins asymmetry in electroproduction of Kaon at the electron–ion colliders within TMD factorization. Eur. Phys. J. C 2020, 80, 685. [Google Scholar] [CrossRef]
- Abazov, V.M.; Abramov, V.; Afanasyev, L.G.; Akhunzyanov, R.R.; Akindinov, A.V.; Akopov, N.; Alekseev, I.G.; Aleshko, A.M.; Alexakhin, V.Y.; Alexeev, G.D.; et al. Conceptual design of the Spin Physics Detector. arXiv 2021, arXiv:2102.00442. [Google Scholar]
- Ji, X.; Ma, J.-P.; Yuan, F. QCD factorization for semi-inclusive deep-inelastic scattering at low transverse momentum. Phys. Rev. D 2005, 71, 034005. [Google Scholar] [CrossRef] [Green Version]
- Aybat, S.M.; Rogers, T.C. TMD Parton Distribution and Fragmentation Functions with QCD Evolution. Phys. Rev. D 2011, 83, 114042. [Google Scholar] [CrossRef] [Green Version]
- Collins, J.; Rogers, T. Equality of Two Definitions for Transverse Momentum Dependent Parton Distribution Functions. Phys. Rev. D 2013, 87, 034018. [Google Scholar] [CrossRef] [Green Version]
- Echevarría, M.G.; Idilbi, A.; Schäfer, A.; Scimemi, I. Model-Independent Evolution of Transverse Momentum Dependent Distribution Functions (TMDs) at NNLL. Eur. Phys. J. C 2013, 73, 2636. [Google Scholar] [CrossRef] [Green Version]
- Pitonyak, D.; Schlegel, M.; Metz, A. Polarized hadron pair production from electron-positron annihilation. Phys. Rev. D 2014, 89, 054032. [Google Scholar] [CrossRef] [Green Version]
- Boer, D. Angular dependences in inclusive two-hadron production at BELLE. Nucl. Phys. B 2009, 806, 23. [Google Scholar]
- Arnold, S.; Metz, A.; Schlegel, M. Dilepton production from polarized hadron hadron collisions. Phys. Rev. D 2009, 79, 034005. [Google Scholar] [CrossRef] [Green Version]
- Lambertsen, M.; Vogelsang, W. Drell–Yan lepton angular distributions in perturbative QCD. Phys. Rev. D 2016, 93, 114013. [Google Scholar] [CrossRef] [Green Version]
- Collins, J.; Hautmann, F. Infrared divergences and nonlightlike eikonal lines in Sudakov processes. Phys. Lett. B 2000, 472, 129. [Google Scholar] [CrossRef] [Green Version]
- Bacchetta, A.; D’Alesio, U.; Diehl, M.; Miller, C.A. Single-spin asymmetries: The Trento conventions. Phys. Rev. D 2004, 70, 117504. [Google Scholar] [CrossRef] [Green Version]
- Bacchetta, A.; Diehl, M.; Goeke, K.; Metz, A.; Mulders, P.J.; Schlegel, M. Semi-inclusive deep inelastic scattering at small transverse momentum. J. High Energy Phys. 2007, 02, 093. [Google Scholar] [CrossRef] [Green Version]
- Idilbi, A.; Ji, X.; Ma, J.-P.; Yuan, F. Collins-Soper equation for the energy evolution of transverse-momentum and spin dependent parton distributions. Phys. Rev. D 2004, 70, 074021. [Google Scholar] [CrossRef] [Green Version]
- Bacchetta, A.; Prokudin, A. Evolution of the helicity and transversity Transverse-Momentum-Dependent parton distributions. Nucl. Phys. B 2013, 875, 536. [Google Scholar] [CrossRef] [Green Version]
- Echevarria, M.G.; Idilbi, A.; Kang, Z.-B.; Vitev, I. QCD Evolution of the Sivers Asymmetry. Phys. Rev. D 2014, 89, 074013. [Google Scholar] [CrossRef] [Green Version]
- Kang, Z.-B.; Xiao, B.-W.; Yuan, F. QCD Resummation for Single Spin Asymmetries. Phys. Rev. Lett. 2011, 107, 152002. [Google Scholar] [CrossRef] [Green Version]
- Aybat, S.M.; Collins, J.C.; Qiu, J.W.; Rogers, T.C. The QCD Evolution of the Sivers Function. Phys. Rev. D 2012, 85, 034043. [Google Scholar] [CrossRef] [Green Version]
- Echevarria, M.G.; Idilbi, A.; Scimemi, I. Unified treatment of the QCD evolution of all (un-)polarized transverse momentum dependent functions: Collins function as a study case. Phys. Rev. D 2014, 90, 014003. [Google Scholar] [CrossRef]
- Landry, F.; Brock, R.; Nadolsky, P.M.; Yuan, C.P. Tevatron Run-1 Z boson data and Collins-Soper-Sterman resummation formalism. Phys. Rev. D 2003, 67, 073016. [Google Scholar] [CrossRef] [Green Version]
- Qiu, J.; Zhang, X. QCD prediction for heavy boson transverse momentum distributions. Phys. Rev. Lett. 2001, 86, 2724. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Konychev, A.V.; Nadolsky, P.M. Universality of the Collins-Soper-Sterman nonperturbative function in gauge boson production. Phys. Lett. B 2006, 633, 710. [Google Scholar] [CrossRef] [Green Version]
- Davies, C.; Webber, B.; Stirling, W. Drell–Yan Cross-Sections at Small Transverse Momentum. Nucl. Phys. B 1984, 1, I.95. [Google Scholar] [CrossRef] [Green Version]
- Ellis, R.K.; Ross, D.; Veseli, S. Vector boson production in hadronic collisions. Nucl. Phys. B 1997, 503, 309. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; Melis, S. A Strategy towards the extraction of the Sivers function with TMD evolution. Phys. Rev. D 2012, 86, 014028. [Google Scholar] [CrossRef] [Green Version]
- Kang, Z.-B.; Prokudin, A.; Sun, P.; Yuan, F. Extraction of Quark Transversity Distribution and Collins Fragmentation Functions with QCD Evolution. Phys. Rev. D 2016, 93, 014009. [Google Scholar] [CrossRef] [Green Version]
- Lai, H.-L.; Guzzi, M.; Huston, J.; Li, Z.; Nadolsky, P.M.; Pumplin, J.; Yuan, C.P. New parton distributions for collider physics. Phys. Rev. D 2010, 82, 074024. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Lu, Z.; Schmidt, I. Transverse polarization of the Λ hyperon from unpolarized quark fragmentation in the diquark model. Phys. Rev. D 2017, 96, 034010. [Google Scholar] [CrossRef] [Green Version]
- Botje, M. QCDNUM: Fast QCD Evolution and Convolution. Comput. Phys. Commun. 2011, 182, 490. [Google Scholar] [CrossRef] [Green Version]
- de Florian, D.; Sassot, R.; Stratmann, M.; Vogelsang, W. Extraction of Spin-Dependent Parton Densities and Their Uncertainties. Phys. Rev. D 2009, 80, 034030. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Yang, Y.; Lu, Z. Double Collins effect in e+e−→ΛX process in a diquark spectator model. Phys.Rev. D 2018, 97, 114015. [Google Scholar] [CrossRef] [Green Version]
- Anselmino, M.; Boglione, M.; Hernandez, J.O.G.; Melis, S.; Prokudin, A. Unpolarised Transverse Momentum Dependent Distribution and Fragmentation Functions from SIDIS Multiplicities. J. High Energy Phys. 2014, 4, 5. [Google Scholar] [CrossRef] [Green Version]
- Accardi, A.; Albacete, J.L.; Anselmino, M.; Armesto, N.; Aschenauer, E.C.; Bacchetta, A.; Boer, D.; Brooks, W.K.; Burton, T.; Chang, N.-B.; et al. Electron–ion collider: The Next QCD Frontier. Eur. Phys. J. A 2016, 52, 268. [Google Scholar] [CrossRef] [Green Version]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, S.; Xu, Q.; Zhang, Y.; Wang, X. The Collins Asymmetry in Λ Hyperon Produced SIDIS Process at Electron–Ion Colliders. Symmetry 2023, 15, 841. https://doi.org/10.3390/sym15040841
Yang S, Xu Q, Zhang Y, Wang X. The Collins Asymmetry in Λ Hyperon Produced SIDIS Process at Electron–Ion Colliders. Symmetry. 2023; 15(4):841. https://doi.org/10.3390/sym15040841
Chicago/Turabian StyleYang, Shuailiang, Qi Xu, Yateng Zhang, and Xiaoyu Wang. 2023. "The Collins Asymmetry in Λ Hyperon Produced SIDIS Process at Electron–Ion Colliders" Symmetry 15, no. 4: 841. https://doi.org/10.3390/sym15040841




