Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction
Abstract
:1. Introduction
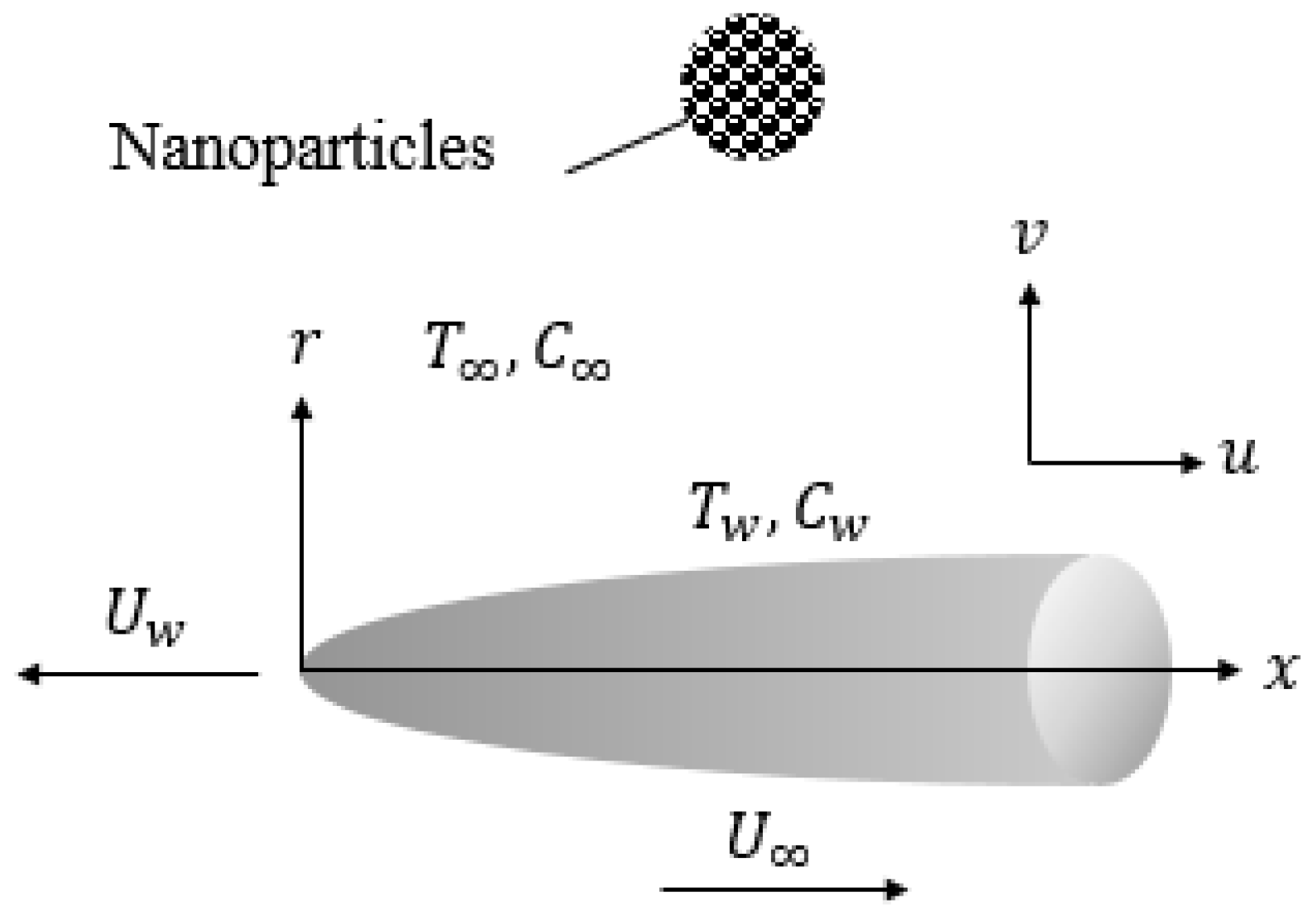
2. Governing Formula and Modeling
3. Stability Analysis
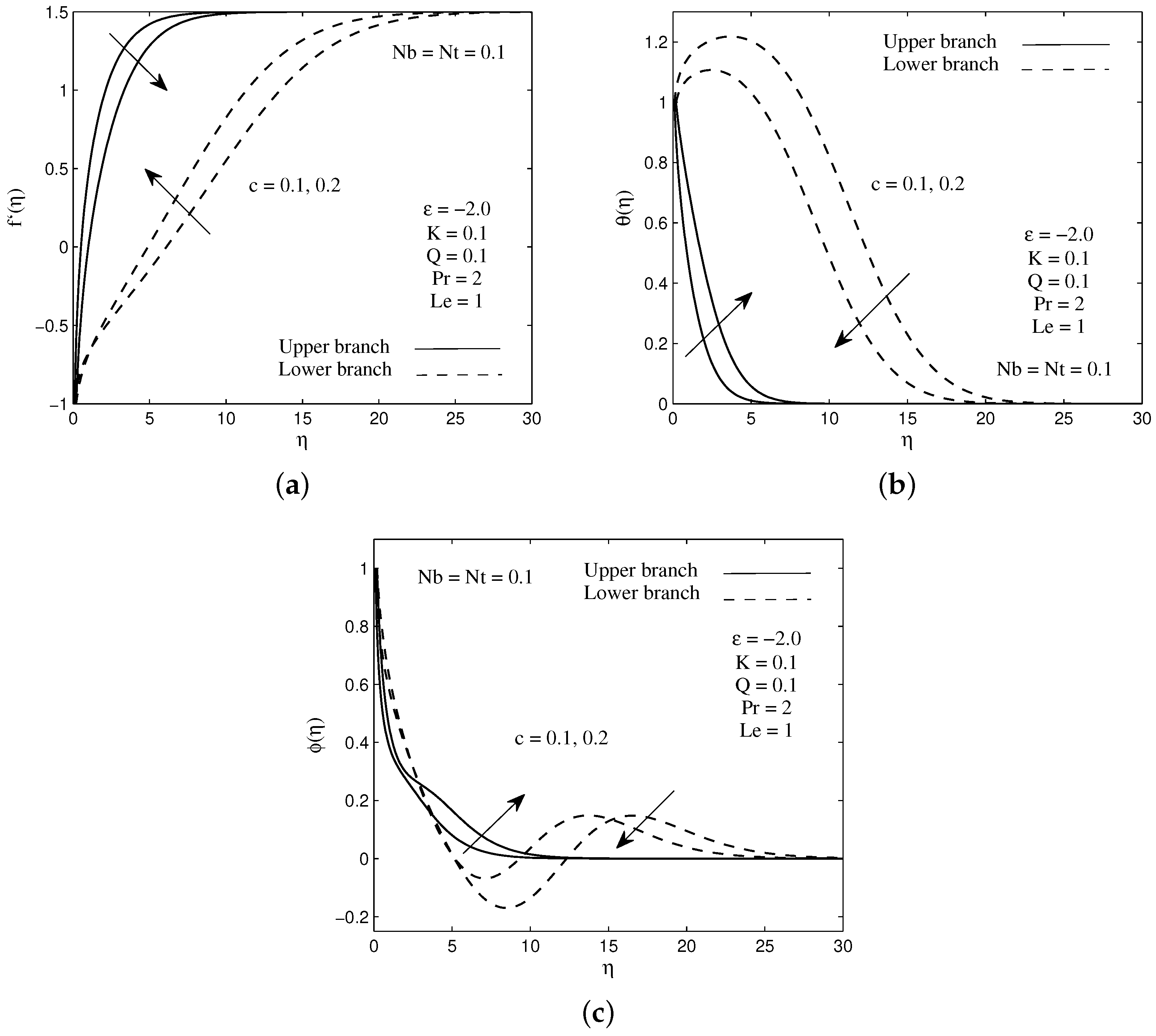
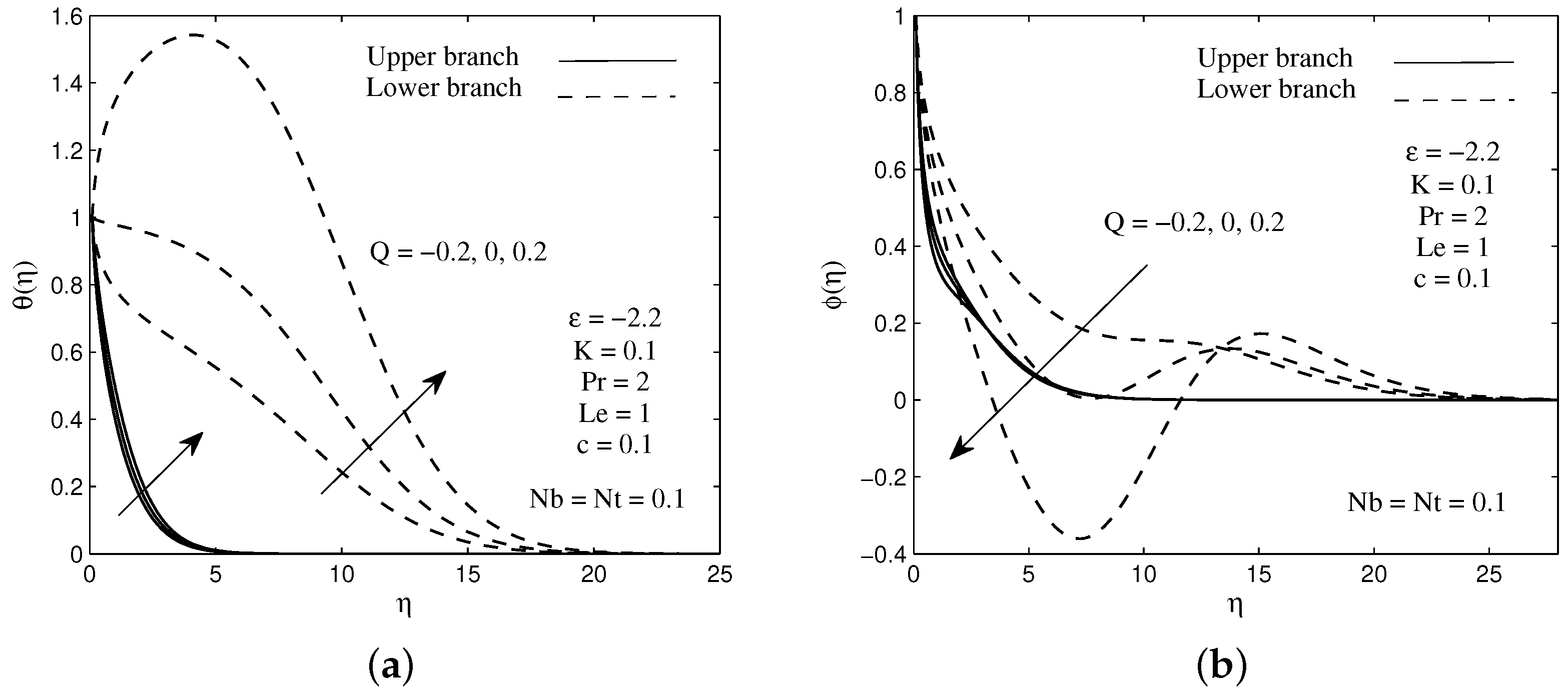
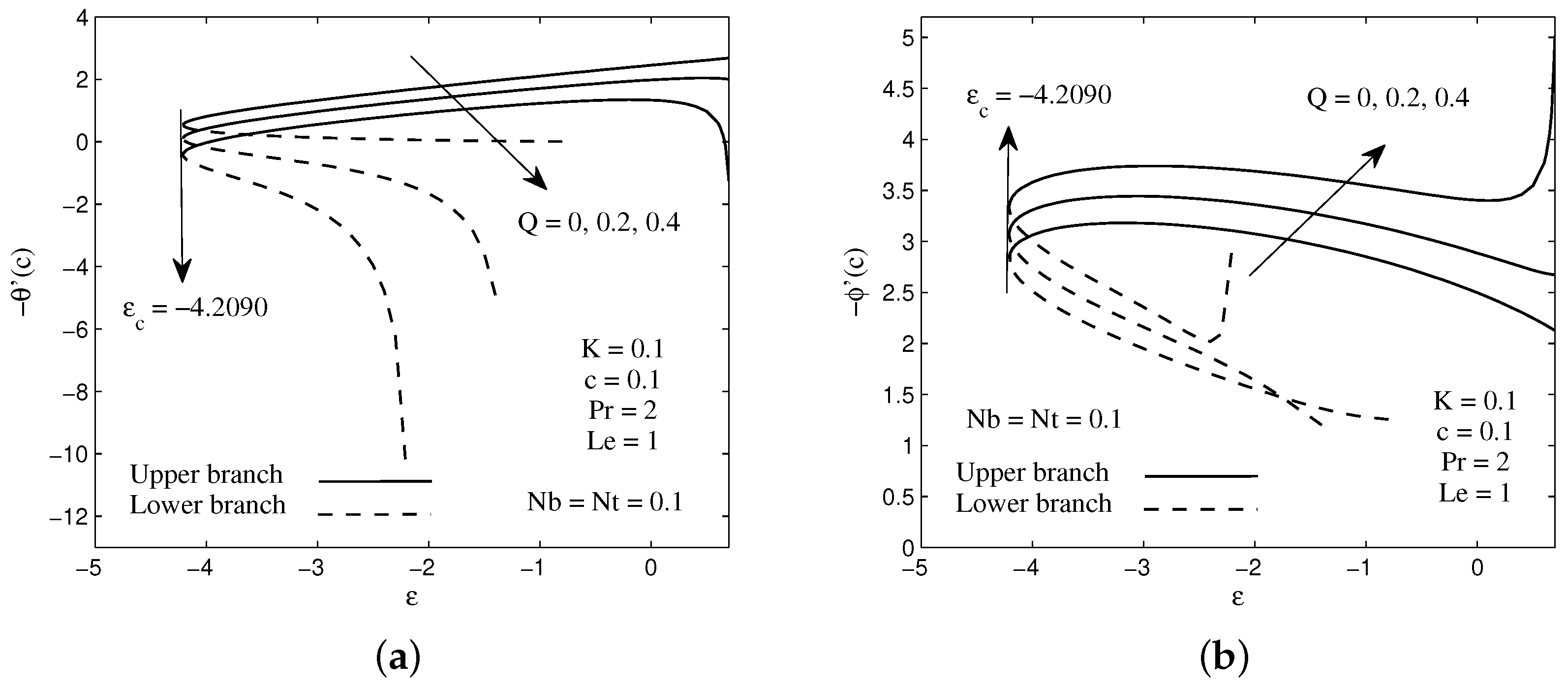
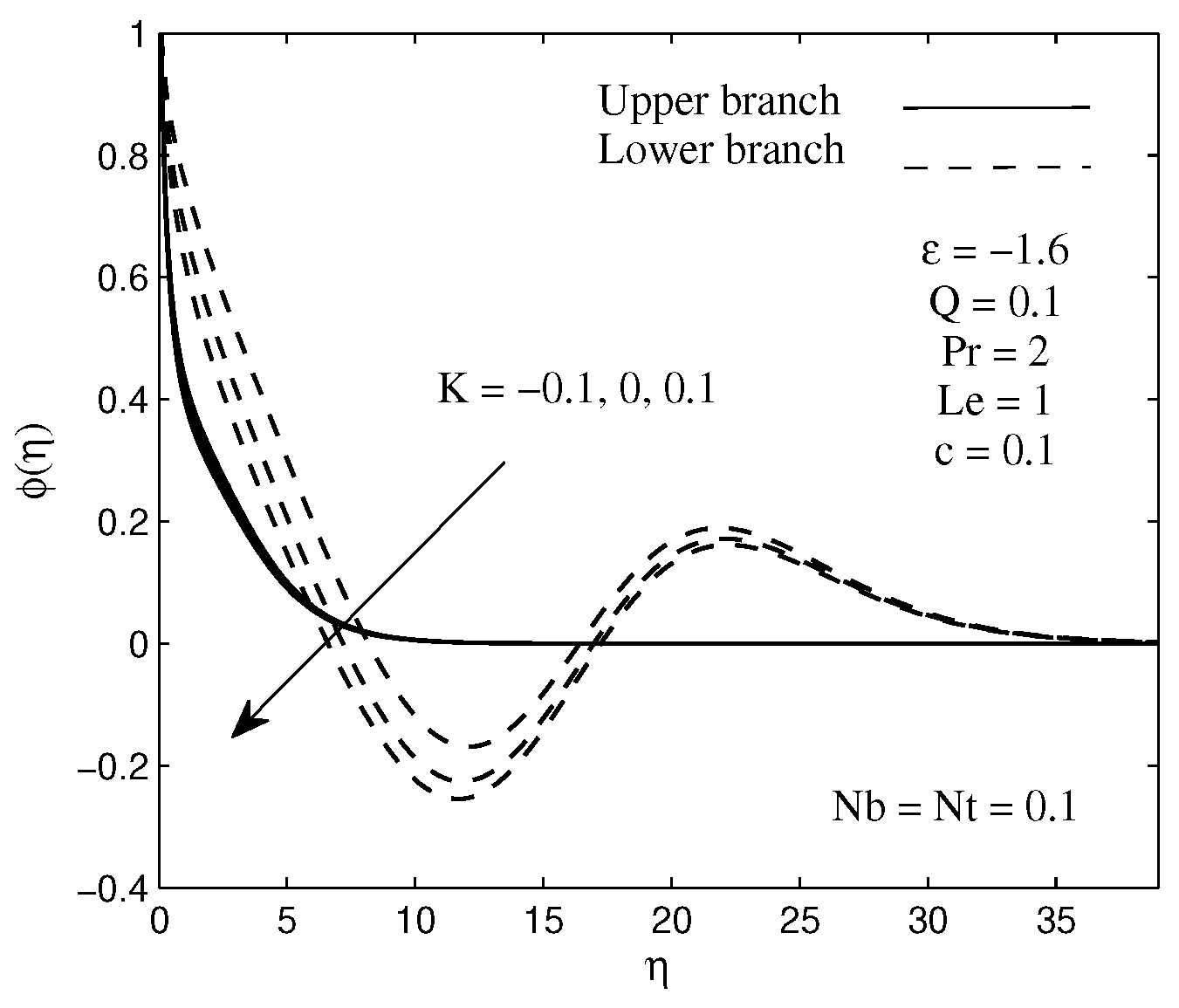
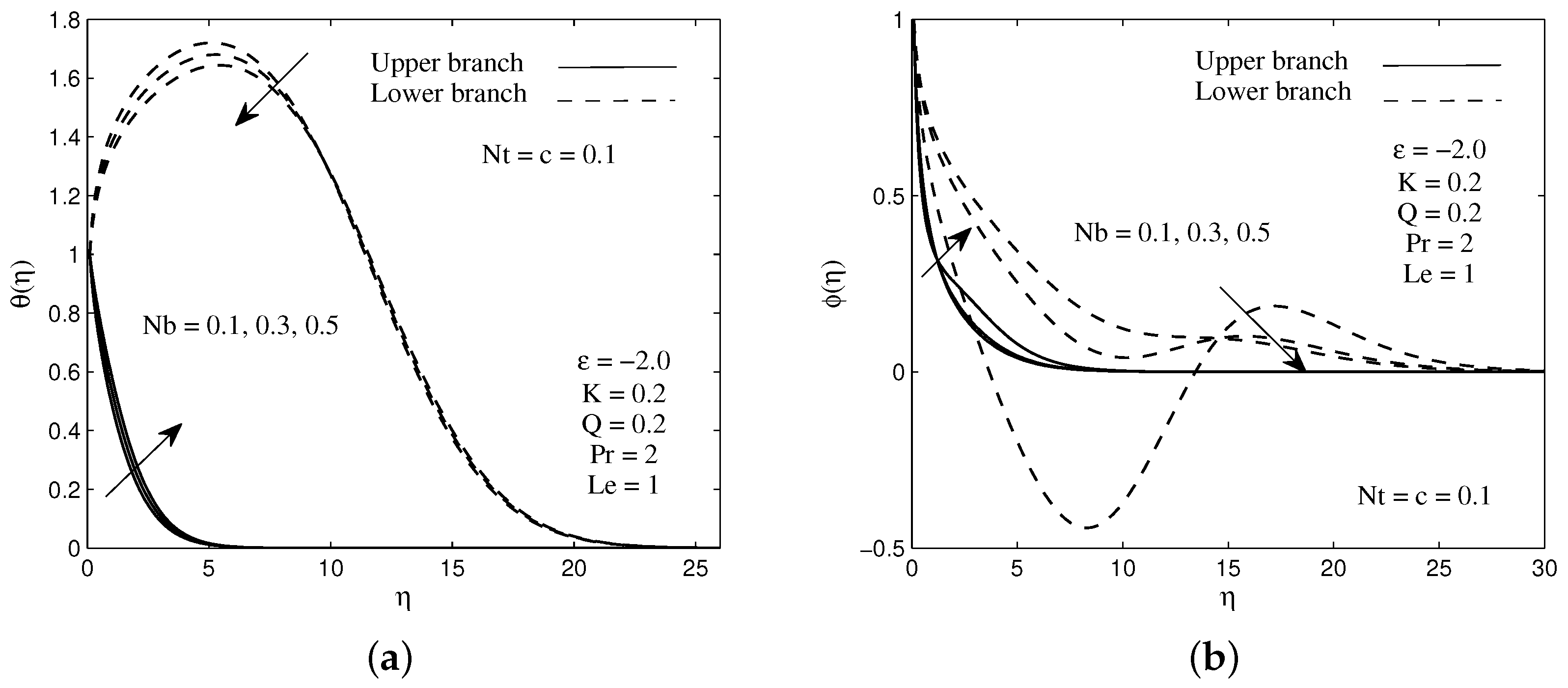
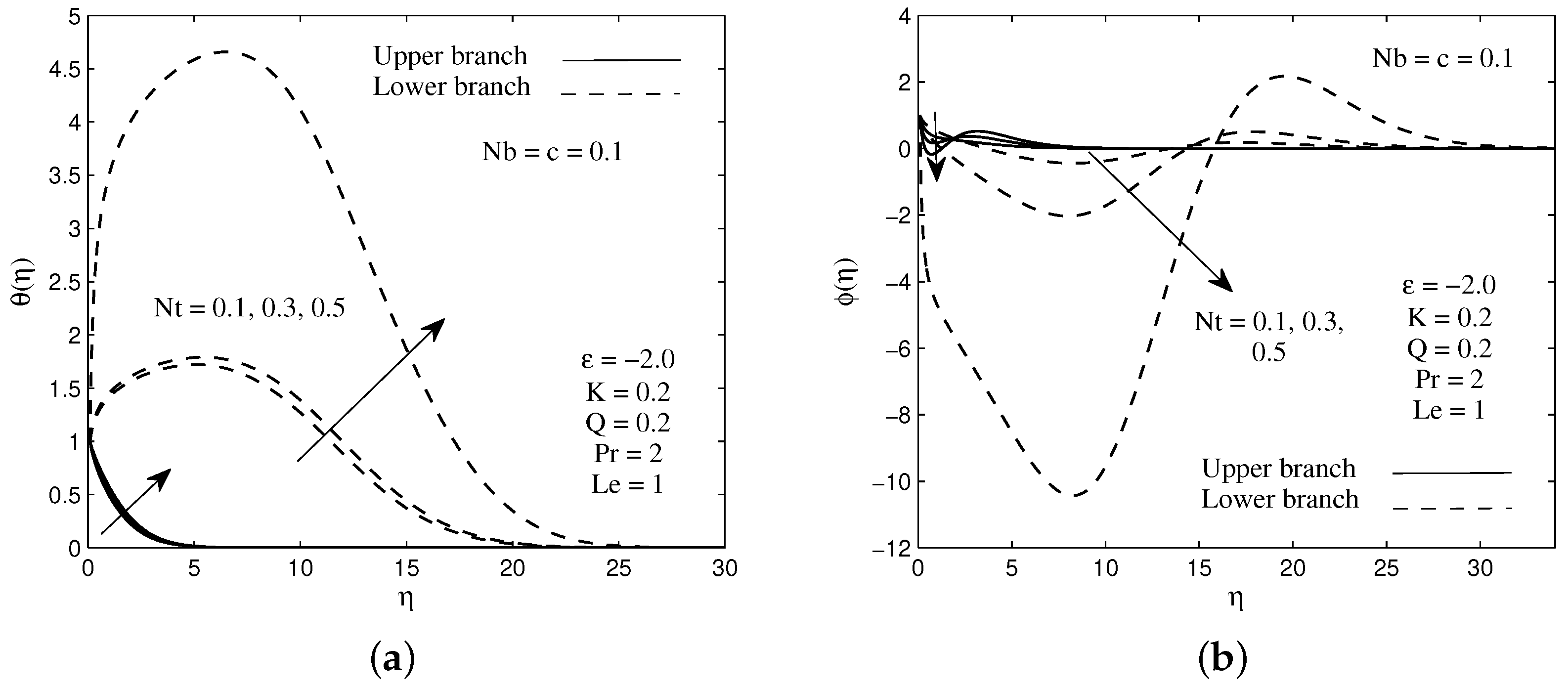
4. Graphical Results and Discussion
5. Final Remarks
- The heat generation parameter reduces the local heat flux as well as the rate of heat transfer.
- The presence of a chemical reaction increases the rate of mass transfer on the needle surface.
- The Brownian motion parameter diminishes the rate of heat and mass transfers from the needle surface to the flow.
- An increase in the thermophoresis parameter results in an increase in the mass transfer rate, while the reverse effect is noted for the heat transfer rate.
- An increment in the needle thickness leads to decrease the magnitudes of the surface shear stress, local heat flux and local mass flux.
- The dual solutions are likely to exist when the needle surface moves against the free-stream direction, .
- The upper branch solution exhibits stable flow (or solution) and lower branch solution exhibits unstable flow.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| c | Needle size |
| C | Fluid concentration (kg m) |
| Skin friction coefficient | |
| Ambient nanoparticle volume fraction | |
| Surface volume fraction | |
| Specific heat at constant pressure | |
| Brownian diffusion coefficient (m s) | |
| Thermophoretic diffusion coefficient (m s) | |
| f | Similarity function for velocity |
| K | Chemical reaction parameter |
| Chemical reaction coefficient | |
| Dimensionless reaction rate | |
| Lewis number | |
| Brownian motion parameter | |
| Thermophoresis parameter | |
| Local Nusselt number | |
| Prandtl number | |
| Q | Heat generation parameter |
| Heat generation coefficient | |
| Dimensionless heat generation | |
| r | Cartesian coordinate |
| Local Reynolds number | |
| Local Sherwood number | |
| T | Fluid temperature (K) |
| Wall temperature (K) | |
| Ambient temperature (K) | |
| U | Composite velocity (ms) |
| Wall velocity (ms) | |
| Ambient velocity (ms) | |
| u | Velocity in x direction (ms) |
| v | Velocity in r direction (ms) |
| x | Cartesian coordinate |
| Thermal diffusivity (m s) | |
| Similarity independent variable | |
| Dimensionless temperature | |
| Velocity ratio parameter | |
| Ratio of effective heat capacity of nanofluid | |
| Volumetric heat capacity (J K) | |
| Kinematic viscosity (m s) | |
| Dynamic viscosity (kg ms) | |
| Fluid density (kg m) | |
| Dimensionless solid volume fraction | |
| w | Condition at the wall |
| ∞ | Ambient condition |
| Differentiative with respect to |
References
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. Am. Soc. Mech. Eng. Fluids Eng. Div. 1995, 231, 99–105. [Google Scholar]
- Wong, K.V.; Leon, O.D. Applications of Nanofluids: Current and Future. Adv. Mech. Eng. 2010, 2010, 519659. [Google Scholar] [CrossRef]
- Saidur, R.; Leong, K.Y.; Mohammad, H.A. A review on applications and challenges of nanofluids. Renew. Sustain. Energy Rev. 2011, 15, 1646–1668. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. Application of nanofluids in heat exchangers: A review. Renew. Sustain. Energy Rev. 2012, 16, 5625–5638. [Google Scholar] [CrossRef]
- Colangelo, G.; Favale, E.; Milanese, M.; Risi, A.D.; Laforgia, D. Cooling of electronic devices: Nanofluids contribution. Appl. Ther. Eng. 2017, 127, 421–435. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Trans. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Trans. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Trans. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. Boundary layer flow of a nanofluid past a stretching sheet with a convective boundary condition. Int. J. Ther. Sci. 2011, 50, 1326–1332. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Unsteady boundary-layer flow and heat transfer of a nanofluid over a permeable stretching/shrinking sheet. Int. J. Heat Mass Trans. 2012, 55, 2102–2109. [Google Scholar] [CrossRef]
- Das, K.; Duari, P.R.; Kundu, P.K. Nanofluid flow over an unsteady stretching surface in presence of thermal radiation. Alex. Eng. J. 2014, 53, 737–745. [Google Scholar] [CrossRef] [Green Version]
- Mabood, F.; Khan, W.A.; Ismail, A.I.M. MHD boundary layer flow and heat transfer of nanofluids over a nonlinear stretching sheet: A numerical study. J. Magn. Magn. Mater. 2015, 374, 569–576. [Google Scholar] [CrossRef]
- Naramgari, S.; Sulochana, C. MHD flow over a permeable stretching/shrinking sheet of a nanofluid with suction/injection. Alex. Eng. J. 2016, 55, 819–827. [Google Scholar] [CrossRef] [Green Version]
- Pandey, A.K.; Kumar, M. Boundary layer flow and heat transfer analysis on Cu-water nanofluid flow over a stretching cylinder with slip. Alex. Eng. J. 2017, 56, 671–677. [Google Scholar] [CrossRef]
- Mustafa, M. MHD nanofluid flow over a rotating disk with partial slip effects: Buongiorno model. Int. J. Heat Mass Trans. 2017, 108, 1910–1916. [Google Scholar] [CrossRef]
- Jyothi, K.; Reddy, P.S.; Reddy, M.S. Influence of magnetic field and thermal radiation on convective flow of SWCNTs-water and MWCNTs-water nanofluid between rotating stretchable disks with convective boundary conditions. Adv. Powder Technol. 2018, 331, 326–337. [Google Scholar] [CrossRef]
- Bakar, N.A.A.; Bachok, N.; Arifin, N.M.; Pop, I. Stability analysis on the flow and heat transfer of nanofluid past a stretching/shrinking cylinder with suction effect. Results Phys. 2018, 9, 1335–1344. [Google Scholar] [CrossRef]
- Griffith, R.M. Velocity, temperature and concentration distributions during fiber spinning. Ind. Eng. Chem. Fundam. 1964, 3, 245–250. [Google Scholar] [CrossRef]
- Chin, D.T. Mass transfer to a continuousmoving sheet electrode. J. Electrochem. Soc. 1975, 122, 643–646. [Google Scholar] [CrossRef]
- Gorla, R.S.R. Unsteady mass transfer in the boundary layer on a continuous moving sheet electrode. J. Electrochem. Soc. 1978, 125, 865–869. [Google Scholar] [CrossRef]
- Damseh, R.A.; Al-Odat, M.Q.; Chamkha, A.J.; Shannak, B.A. Combined effect of heat generation or absorption and first-order chemical reaction on micropolar fluid flows over a uniformly stretched permeable surface. Int. J. Therm. Sci. 2009, 48, 1658–1663. [Google Scholar] [CrossRef]
- Magyari, E.; Chamkha, A.J. Combined effect of heat generation or absorption and first-order chemical reaction on micropolar fluid flows over a uniformly stretched permeable surface: The full analytical solution. Int. J. Therm. Sci. 2010, 49, 1821–1828. [Google Scholar] [CrossRef]
- Mabood, F.; Shateyi, S.; Rashidi, M.M.; Momoniat, E.; Freidoonimehr, N. MHD stagnation point flow heat and mass transfer of nanofluids in porous medium with radiation, viscous dissipation and chemical reaction. Adv. Powder Technol. 2016, 27, 742–749. [Google Scholar] [CrossRef]
- Eid, M.R. Chemical reaction effect on MHD boundary-layer flow of two-phase nanofluid model over an exponentially stretching sheet with a heat generation. J. Mol. Liq. 2016, 220, 718–725. [Google Scholar] [CrossRef]
- Ibrahim, S.M.; Lorenzini, G.; Vijaya Kumar, P.; Raju, C.S.K. Influence of chemical reaction and heat source on dissipative MHD mixed convection flow of a Casson nanofluid over a nonlinear permeable stretching sheet. Int. J. Heat Mass Trans. 2017, 111, 346355. [Google Scholar] [CrossRef]
- Nayak, M.K.; Akbar, N.S.; Tripathi, D.; Khan, Z.H.; Pandey, V.S. MHD 3D free convective flow of nanofluid over an exponentially stretching sheet with chemical reaction. Adv. Powder Technol. 2017, 28, 2159–2166. [Google Scholar] [CrossRef]
- Sithole, H.; Mondal, H.; Goqo, S.; Sibanda, P.; Motsa, S. Numerical simulation of couple stress nanofluid flow in magneto-porous medium with thermal radiation and a chemical reaction. Appl. Math. Comput. 2018, 339, 820–836. [Google Scholar] [CrossRef]
- Khan, M.; Shahid, A.; Malik, M.Y.; Salahuddin, T. Chemical reaction for Carreau-Yasuda nanofluid flow past a nonlinear stretching sheet considering Joule heating. Results Phys. 2018, 8, 1124–1130. [Google Scholar] [CrossRef]
- Zeeshan, A.; Shehzad, N.; Ellahi, R. Analysis of activation energy in Couette-Poiseuille flow of nanofluid in the presence of chemical reaction and convective boundary conditions. Results Phys. 2018, 8, 502–512. [Google Scholar] [CrossRef]
- Hayat, T.; Kiyani, M.Z.; Alsaedi, A.; Ijaz Khan, M.; Ahmad, I. Mixed convective three-dimensional flow of Williamson nanofluid subject to chemical reaction. Int. J. Heat Mass Trans. 2018, 127, 422–429. [Google Scholar] [CrossRef]
- Lee, L.L. Boundary layer over a thin needle. Phys. Fluids 1967, 10, 1820–1822. [Google Scholar] [CrossRef]
- Narain, J.P.; Uberoi, S.M. Combined forced and free-convection heat transfer from vertical thin needles in a uniform stream. Phys. Fluids 1973, 15, 1879–1882. [Google Scholar] [CrossRef]
- Wang, C.Y. Mixed convection on a vertical needle with heated tip. Phys. Fluids 1990, 2, 622–625. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Boundary layer flow over a continuously moving thin needle in a parallel free stream. Chin. Phys. Lett. 2007, 24, 2895–2897. [Google Scholar] [CrossRef]
- Ahmad, S.; Arifin, N.M.; Nazar, R.; Pop, I. Mixed convection boundary layer flow along vertical thin needles: Assisting and opposing flows. Int. Commun. Heat Mass Trans. 2008, 35, 157–162. [Google Scholar] [CrossRef]
- Afridi, M.I.; Qasim, M. Entropy generation and heat transfer in boundary layer flow over a thin needle moving in a parallel stream in the presence of nonlinear Rosseland radiation. Int. J. Therm. Sci. 2018, 123, 117–128. [Google Scholar] [CrossRef]
- Grosan, T.; Pop, I. Forced Convection Boundary Layer Flow Past Nonisothermal Thin Needles in Nanofluids. J. Heat Trans. 2011, 133. [Google Scholar] [CrossRef]
- Trimbitas, R.; Grosan, T.; Pop, I. Mixed convection boundary layer flow along vertical thin needles in nanofluids. Int. J. Numer. Methods Heat Fluid Flow 2014, 24, 579–594. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Farooq, M.; Yasmeen, T.; Alsaedi, A. Water-carbon nanofluid flow with variable heat flux by a thin needle. J. Mol. Liq. 2016, 224, 786–791. [Google Scholar] [CrossRef]
- Soid, S.K.; Ishak, A.; Pop, I. Boundary layer flow past a continuously moving thin needle in a nanofluid. Appl. Therm. Eng. 2017, 114, 58–64. [Google Scholar] [CrossRef]
- Krishna, P.M.; Sharma, R.P.; Sandeep, N. Boundary layer analysis of persistent moving horizontal needle in Blasius and Sakiadis magnetohydrodynamic radiative nanofluid flows. Nucl. Eng. Technol. 2017, 49, 1654–1659. [Google Scholar] [CrossRef]
- Ahmad, R.; Mustafa, M.; Hina, S. Buongiorno’s model for fluid flow around a moving thin needle in a flowing nanofluid: A numerical study. Chin. J. Phys. 2017, 55, 1264–1274. [Google Scholar] [CrossRef]
- Salleh, S.N.A.; Bachok, N.; Arifin, N.M.; Ali, F.M.; Pop, I. Magnetohydrodynamics flow past a moving vertical thin needle in a nanofluid with stability analysis. Energies 2018, 11, 3297. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Rosca, A.V.; Pop, I. Flow and heat transfer over a vertical permeable stretching/shrinking sheet with a second order slip. Int. J. Heat Mass Trans. 2013, 60, 355–364. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]


| c | Ahmad et al. [42] | Salleh et al. [43] | Current Study |
|---|---|---|---|
| 0.01 | 8.4924360 | 8.4924452 | 8.4924453 |
| 0.1 | 1.2888171 | 1.2888299 | 1.2888300 |
| 0.15 | - | - | 0.9383388 |
| 0.2 | - | - | 0.7515725 |
| Heat Generation Parameter | Thermphoresis Parameter | |||
|---|---|---|---|---|
| 0.1 | 0.1 | 1.208880 | 0.959223 | 0.749362 |
| 0.3 | 0.989170 | 0.777343 | 0.601318 | |
| 0.5 | 0.805833 | 0.627349 | 0.480535 | |
| 0.2 | 0.1 | 1.078738 | 0.832762 | 0.628219 |
| 0.3 | 0.863788 | 0.656596 | 0.486510 | |
| 0.5 | 0.685682 | 0.512462 | 0.371923 | |
| Chemical Reaction Parameter | Thermphoresis Parameter | |||
|---|---|---|---|---|
| 0.1 | 0.1 | 2.005009 | 1.825444 | 1.781448 |
| 0.3 | 3.271205 | 2.343083 | 2.135890 | |
| 0.5 | 5.055702 | 2.990494 | 2.546082 | |
| 0.2 | 0.1 | 2.085015 | 1.898138 | 1.852426 |
| 0.3 | 3.362939 | 2.418572 | 2.207592 | |
| 0.5 | 5.144680 | 3.063527 | 2.615317 | |
| K = Q | c | Upper Branch | Lower Branch | |
|---|---|---|---|---|
| 0.1 | 0.1 | −4.1994 | 0.0471 | −0.0449 |
| −4.199 | 0.0481 | −0.0458 | ||
| −4.19 | 0.0668 | −0.0625 | ||
| 0.2 | −2.7424 | 0.0150 | −0.0147 | |
| −2.742 | 0.0175 | −0.0170 | ||
| −2.74 | 0.0265 | −0.0255 | ||
| 0.2 | 0.1 | −4.1246 | 0.1444 | −0.1254 |
| −4.124 | 0.1449 | −0.1258 | ||
| −4.12 | 0.1484 | −0.1284 | ||
| 0.2 | −2.7136 | 0.0793 | −0.0706 | |
| −2.713 | 0.0801 | −0.0713 | ||
| −2.71 | 0.0841 | −0.0744 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salleh, S.N.A.; Bachok, N.; Arifin, N.M.; Ali, F.M. Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction. Symmetry 2019, 11, 543. https://doi.org/10.3390/sym11040543
Salleh SNA, Bachok N, Arifin NM, Ali FM. Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction. Symmetry. 2019; 11(4):543. https://doi.org/10.3390/sym11040543
Chicago/Turabian StyleSalleh, Siti Nur Alwani, Norfifah Bachok, Norihan Md Arifin, and Fadzilah Md Ali. 2019. "Numerical Analysis of Boundary Layer Flow Adjacent to a Thin Needle in Nanofluid with the Presence of Heat Source and Chemical Reaction" Symmetry 11, no. 4: 543. https://doi.org/10.3390/sym11040543






