Evaluation of BDS-2 and BDS-3 Satellite Atomic Clock Products and Their Effects on Positioning
Abstract
:1. Introduction
2. Methods
2.1. Satellite Clock Stability Assessment and BDS-2/BDS-3 Clock Datum Discrepancy
2.2. Ionosphere-Free PPP and Receiver Clock ISB
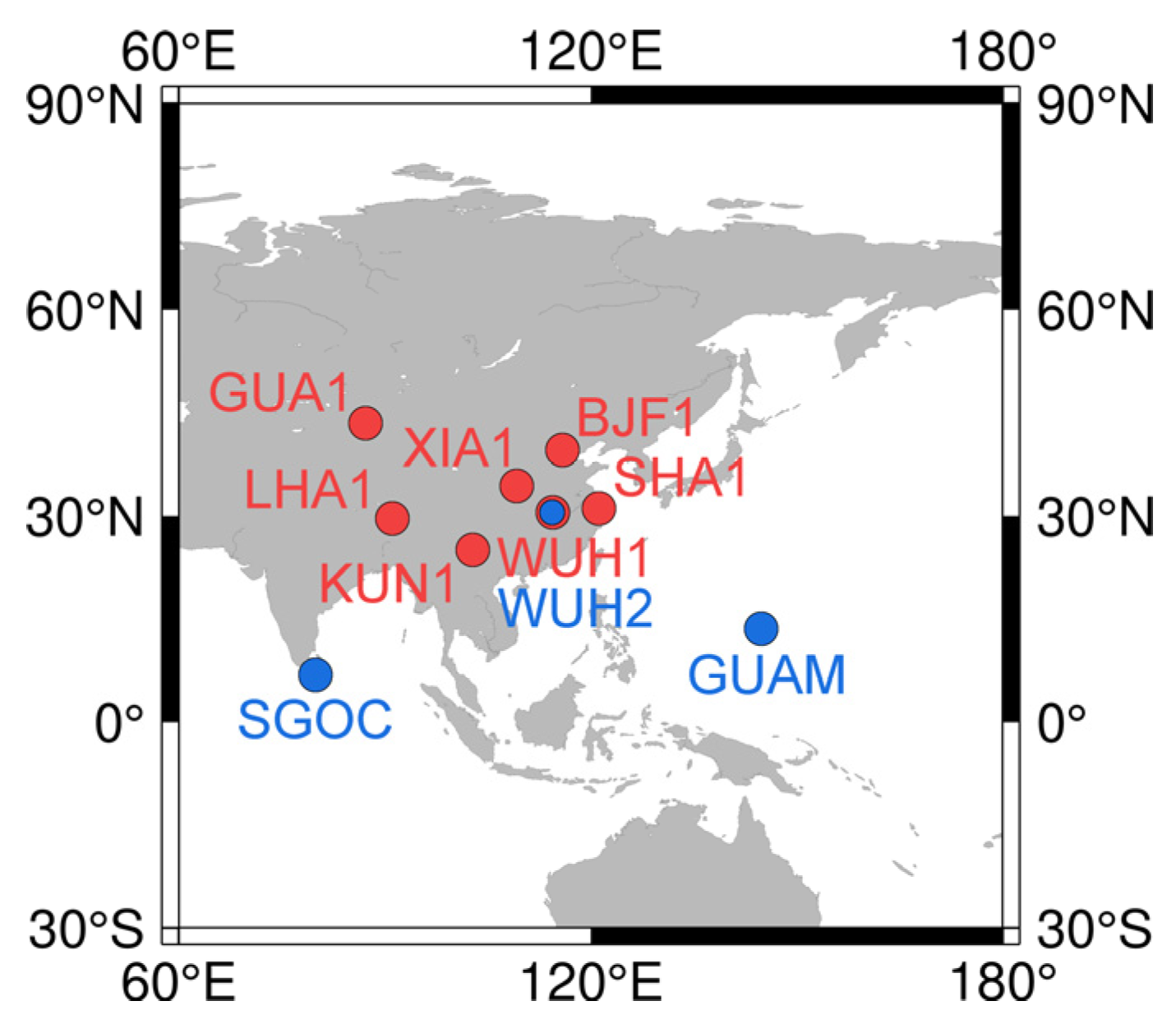
3. Data Collection
4. BDS-2/BDS-3 Clock Stabilities and Datum Discrepancy
4.1. BDS-2/BDS-3 Clock Stabilities
4.2. Comparison of Clock Datum in Different Products
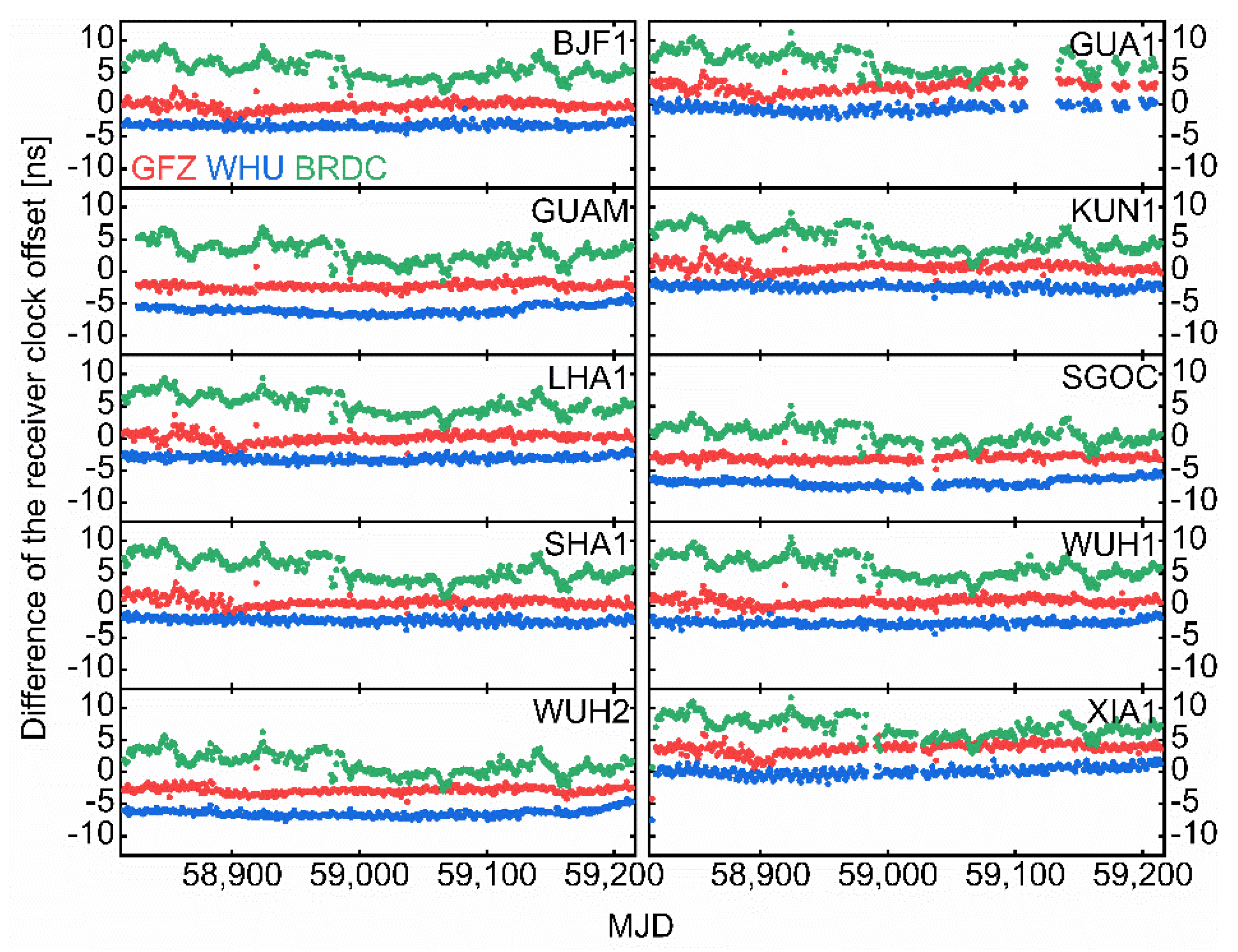
5. BDS-2 and BDS-3 ISB Analysis and PPP Solutions
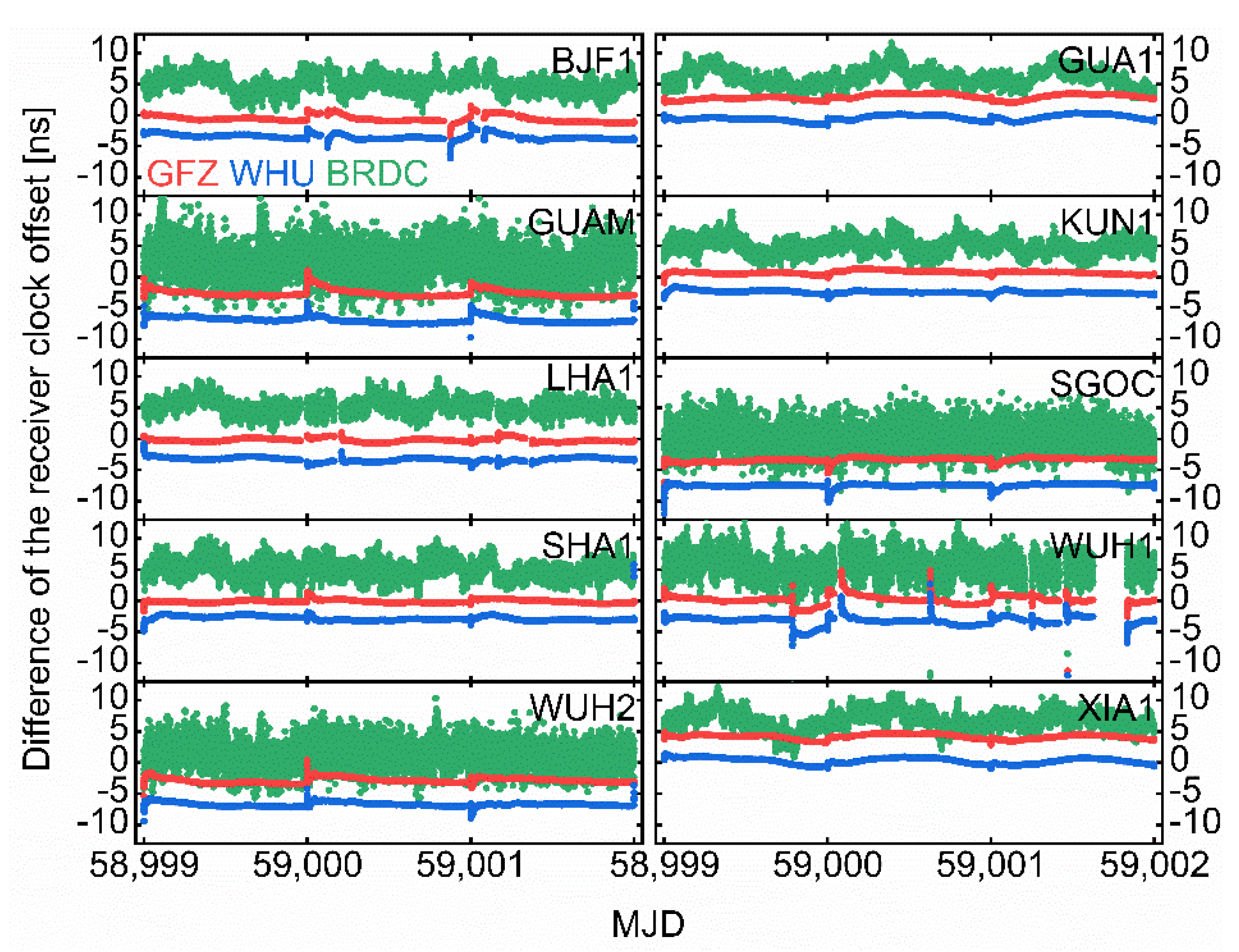
5.1. Comparison of Receiver Clock Offset
5.2. PPP Soulutions
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- CSNO. Development of BeiDou Navigation Satellite System (Version 4.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201912/P020191227430565455478.pdf (accessed on 10 December 2021).
- Li, X.; Li, X.; Liu, G.; Yuan, Y.; Freeshah, M.; Zhang, K.; Zhou, F. BDS multi-frequency PPP ambiguity resolution with new B2a/B2b/B2a + b signals and legacy B1I/B3I signals. J. Geod. 2020, 94, 107. [Google Scholar] [CrossRef]
- CSNO. Report on the Development of BeiDou Navigation Satellite System (Version 2.1). Available online: http://www.beidou.gov.cn/xt/gfxz/201805/P020180509588857259014.pdf (accessed on 10 December 2021).
- Gu, S.; Lou, Y.; Shi, C.; Liu, J. BeiDou phase bias estimation and its application in precise point positioning with triple-frequency observable. J. Geod. 2015, 89, 979–992. [Google Scholar] [CrossRef]
- Gu, S.; Wang, Y.; Zhao, Q.; Zheng, F.; Gong, X. BDS-3 differential code bias estimation with undifferenced uncombined model based on triple-frequency observation. J. Geod. 2020, 94, 45. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Yang, Y.; Hu, X.; Tang, C.; Guo, R.; Zhou, Z.; Xu, J.; Pan, J.; Su, M. BeiDou-3 broadcast clock estimation by integration of observations of regional tracking stations and inter-satellite links. GPS Solut. 2021, 25, 57. [Google Scholar] [CrossRef]
- Jaduszliwer, B.; Camparo, J. Past, present and future of atomic clocks for GNSS. GPS Solut. 2021, 25, 27. [Google Scholar] [CrossRef]
- Bock, H.; Dach, R.; Jäggi, A.; Beutler, G. High-rate GPS clock corrections from CODE: Support of 1 Hz applications. J. Geod. 2009, 83, 1083–1094. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.; Gu, S.; Gong, X.; Song, W.; Lou, Y.; Liu, J. Regional BDS satellite clock estimation with triple-frequency ambiguity resolution based on undifferenced observation. GPS Solut. 2019, 23, 33. [Google Scholar] [CrossRef]
- Phelan, J.; Dass, T.; Freed, G.; Rajan, J.; D’Agostino, J.; Epstein, M. GPS block IIR clocks in space: Current performance and plans for the future. In Proceedings of the 2005 IEEE International Frequency Control Symposium and Exposition, Vancouver, BC, Canada, 29–31 August 2005; p. 19, ISBN 0-7803-9053-9. [Google Scholar]
- Senior, K.L.; Ray, J.R.; Beard, R.L. Characterization of periodic variations in the GPS satellite clocks. GPS Solut. 2008, 12, 211–225. [Google Scholar] [CrossRef]
- Griggs, E.; Kursinski, E.R.; Akos, D. An investigation of GNSS atomic clock behavior at short time intervals. GPS Solut. 2014, 18, 443–452. [Google Scholar] [CrossRef]
- Hauschild, A.; Montenbruck, O.; Steigenberger, P. Short-term analysis of GNSS clocks. GPS Solut. 2013, 17, 295–307. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hauschild, A.; Steigenberger, P.; Hugentobler, U.; Teunissen, P.; Nakamura, S. Initial assessment of the COMPASS/BeiDou-2 regional navigation satellite system. GPS Solut. 2013, 17, 211–222. [Google Scholar] [CrossRef]
- Steigenberger, P.; Hugentobler, U.; Hauschild, A.; Montenbruck, O. Orbit and clock analysis of Compass GEO and IGSO satellites. J. Geod. 2013, 87, 515–525. [Google Scholar] [CrossRef]
- Ai, Q.; Maciuk, K.; Lewinska, P.; Borowski, L. Characteristics of Onefold Clocks of GPS, Galileo, BeiDou and GLONASS Systems. Sensors 2021, 21, 2396. [Google Scholar] [CrossRef]
- Wang, B.; Lou, Y.; Liu, J.; Zhao, Q.; Su, X. Analysis of BDS satellite clocks in orbit. GPS Solut. 2016, 20, 783–794. [Google Scholar] [CrossRef]
- Qin, W.; Ge, Y.; Wei, P.; Dai, P.; Yang, X. Assessment of the BDS-3 on-board clocks and their impact on the PPP time transfer performance. Measurement 2020, 153, 107356. [Google Scholar] [CrossRef]
- Cao, Y.; Huang, G.; Xie, W.; Xie, S.; Wang, H. Assessment and comparison of satellite clock offset between BeiDou-3 and other GNSSs. Acta Geod. Geophys. 2021, 56, 303–319. [Google Scholar] [CrossRef]
- Wu, Z.; Zhou, S.; Hu, X.; Liu, L.; Shuai, T.; Xie, Y.; Tang, C.; Pan, J.; Zhu, L.; Chang, Z. Performance of the BDS3 experimental satellite passive hydrogen maser. GPS Solut. 2018, 22, 48. [Google Scholar] [CrossRef]
- Yang, Y.; Mao, Y.; Sun, B. Basic performance and future developments of BeiDou global navigation satellite system. Satell. Navig. 2020, 1, 1. [Google Scholar] [CrossRef] [Green Version]
- Shi, J.; Ouyang, C.; Huang, Y.; Peng, W. Assessment of BDS-3 global positioning service: Ephemeris, SPP, PPP, RTK, and new signal. GPS Solut. 2020, 24, 81. [Google Scholar] [CrossRef]
- Wang, N.; Li, Z.; Montenbruck, O.; Tang, C. Quality assessment of GPS, Galileo and BeiDou-2/3 satellite broadcast group delays. Adv. Space Res. 2019, 64, 1764–1779. [Google Scholar] [CrossRef]
- Zhang, Y.; Kubo, N.; Chen, J.; Chu, F.-Y.; Wang, A.; Wang, J. Apparent clock and TGD biases between BDS-2 and BDS-3. GPS Solut. 2019, 24, 27. [Google Scholar] [CrossRef]
- Gong, X.; Lou, Y.; Zheng, F.; Gu, S.; Shi, C.; Liu, J.; Jing, G. Evaluation and calibration of BeiDou receiver-related pseudorange biases. GPS Solut. 2018, 22, 98. [Google Scholar] [CrossRef]
- Zheng, F.; Gong, X.; Lou, Y.; Gu, S.; Jing, G.; Shi, C. Calibration of BeiDou Triple-Frequency Receiver-Related Pseudorange Biases and Their Application in BDS Precise Positioning and Ambiguity Resolution. Sensors 2019, 19, 3500. [Google Scholar] [CrossRef] [Green Version]
- Montenbruck, O.; Hauschild, A.; Steigenberger, P. Differential Code Bias Estimation using Multi-GNSS Observations and Global Ionosphere Maps. J. Inst. Navig. 2014, 61, 191–201. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, J.; Gong, X.; Chen, Q. The update of BDS-2 TGD and its impact on positioning. Adv. Space Res. 2020, 65, 2645–2661. [Google Scholar] [CrossRef]
- Li, X.; Xie, W.; Huang, J.; Ma, T.; Zhang, X.; Yuan, Y. Estimation and analysis of differential code biases for BDS3/BDS2 using iGMAS and MGEX observations. J. Geod. 2019, 93, 419–435. [Google Scholar] [CrossRef]
- Jiao, G.; Song, S.; Liu, Y.; Su, K.; Cheng, N.; Wang, S. Analysis and Assessment of BDS-2 and BDS-3 Broadcast Ephemeris: Accuracy, the Datum of Broadcast Clocks and Its Impact on Single Point Positioning. Remote Sens. 2020, 12, 2081. [Google Scholar] [CrossRef]
- Jiao, G.; Song, S.; Jiao, W. Improving BDS-2 and BDS-3 joint precise point positioning with time delay bias estimation. Meas. Sci. Technol. 2020, 31, 25001. [Google Scholar] [CrossRef]
- Qin, W.; Ge, Y.; Zhang, Z.; Su, H.; Wei, P.; Yang, X. Accounting BDS3–BDS2 inter-system biases for precise time transfer. Measurement 2020, 156, 107566. [Google Scholar] [CrossRef]
- Zhao, W.; Chen, H.; Gao, Y.; Jiang, W.; Liu, X. Evaluation of Inter-System Bias between BDS-2 and BDS-3 Satellites and Its Impact on Precise Point Positioning. Remote. Sens. 2020, 12, 2185. [Google Scholar] [CrossRef]
- Allan, D.W. Statistics of atomic frequency standards. Proc. IEEE 1966, 54, 221–230. [Google Scholar] [CrossRef] [Green Version]
- Allan, D.; Hellwig, H.; Kartaschoff, P.; Vanier, J.; Vig, J.; Winkler, G.; Yannoni, N.F. Standard terminology for fundamental frequency and time metrology. In Proceedings of the 42nd Annual Frequency Control Symposium, Baltimore, MD, USA, 1–3 June 1988; pp. 419–425. [Google Scholar]
- Lou, Y.; Gong, X.; Gu, S.; Zheng, F.; Feng, Y. Assessment of code bias variations of BDS triple-frequency signals and their impacts on ambiguity resolution for long baselines. GPS Solut. 2017, 21, 177–186. [Google Scholar] [CrossRef]
- Gu, S.; Zheng, F.; Gong, X.; Lou, Y.; Shi, C. Fusing: A Distributed Software Platform for Real-Time High Precision Multi-GNSS Service. In Proceedings of the IGS Workshop, Wuhan, China, 29 October–2 November 2018; Available online: https://files.igs.org/pub/resource/pubs/workshop/2018/IGSWS-2018-PY09-05.pdf?_ga=2.181681828.2033109928.1639203343-1862730709.1639203343 (accessed on 10 December 2021).
- Gong, X.; Gu, S.; Lou, Y.; Zheng, F.; Ge, M.; Liu, J. An efficient solution of real-time data processing for multi-GNSS network. J. Geod. 2018, 92, 797–809. [Google Scholar] [CrossRef]
- Gu, S.; Dai, C.; Fang, W.; Zheng, F.; Wang, Y.; Zhang, Q.; Lou, Y.; Niu, X. Multi-GNSS PPP/INS tightly coupled integration with atmospheric augmentation and its application in urban vehicle navigation. J. Geod. 2021, 95, 64. [Google Scholar] [CrossRef]
- Luo, X.; Lou, Y.; Gu, S.; Li, G.; Xiong, C.; Song, W.; Zhao, Z. Local ionospheric plasma bubble revealed by BDS Geostationary Earth Orbit satellite observations. GPS Solut. 2021, 25, 117. [Google Scholar] [CrossRef]
- Kudrys, J.; Skorupa, B.; Malkin, Z. Testing the product quality of Galileo and GPS on-board oscillators. Measurement 2021, 167, 108261. [Google Scholar] [CrossRef]
- Wu, W.; Guo, F.; Zheng, J. Analysis of Galileo signal-in-space range error and positioning performance during 2015–2018. Satell. Navig. 2020, 1, 6. [Google Scholar] [CrossRef] [Green Version]
- Montenbruck, O.; Steigenberger, P.; Hauschild, A. Broadcast versus precise ephemerides: A multi-GNSS perspective. GPS Solut. 2015, 19, 321–333. [Google Scholar] [CrossRef]
- Hauschild, A.; Montenbruck, O. A study on the dependency of GNSS pseudorange biases on correlator spacing. GPS Solut. 2016, 20, 159–171. [Google Scholar] [CrossRef]
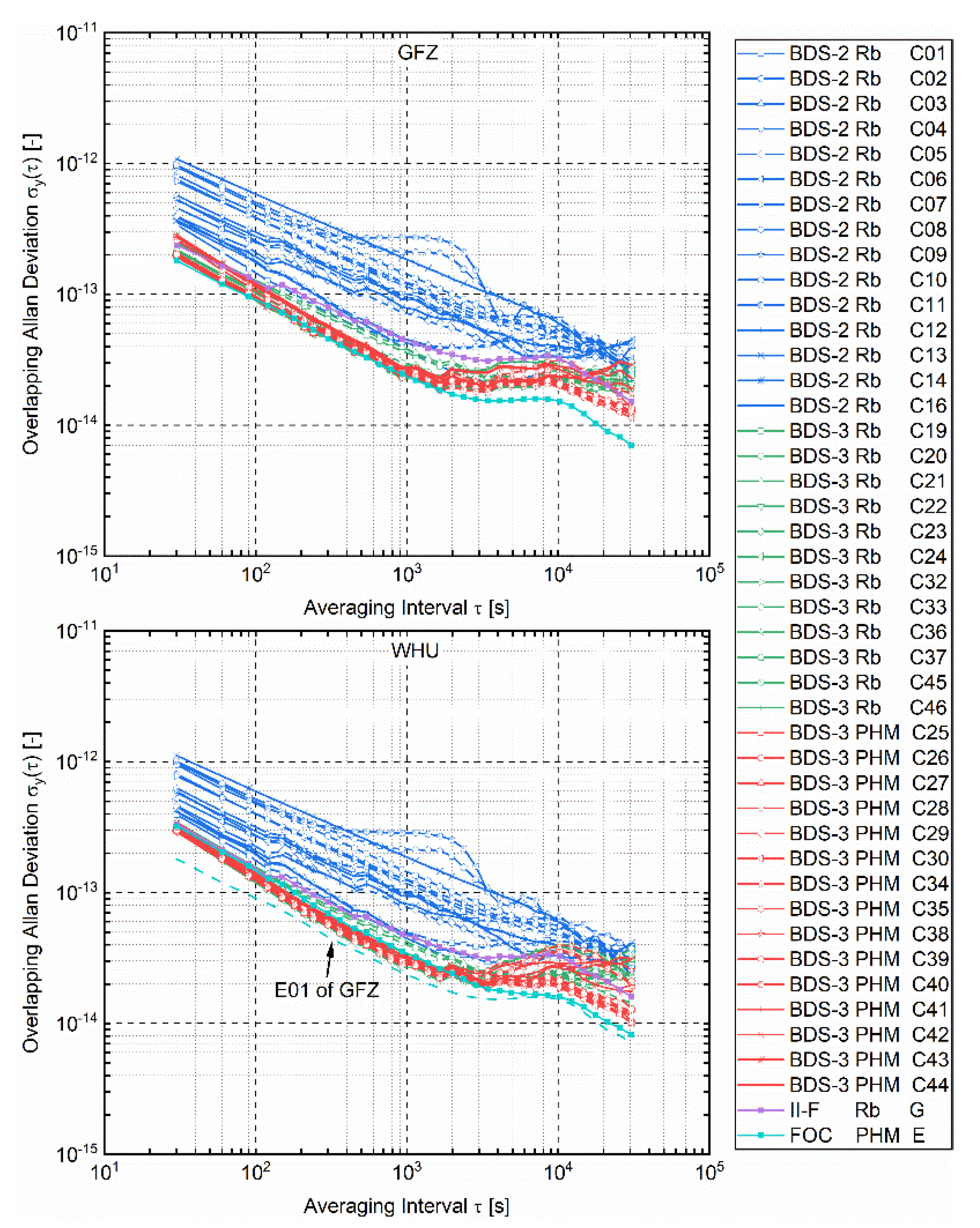
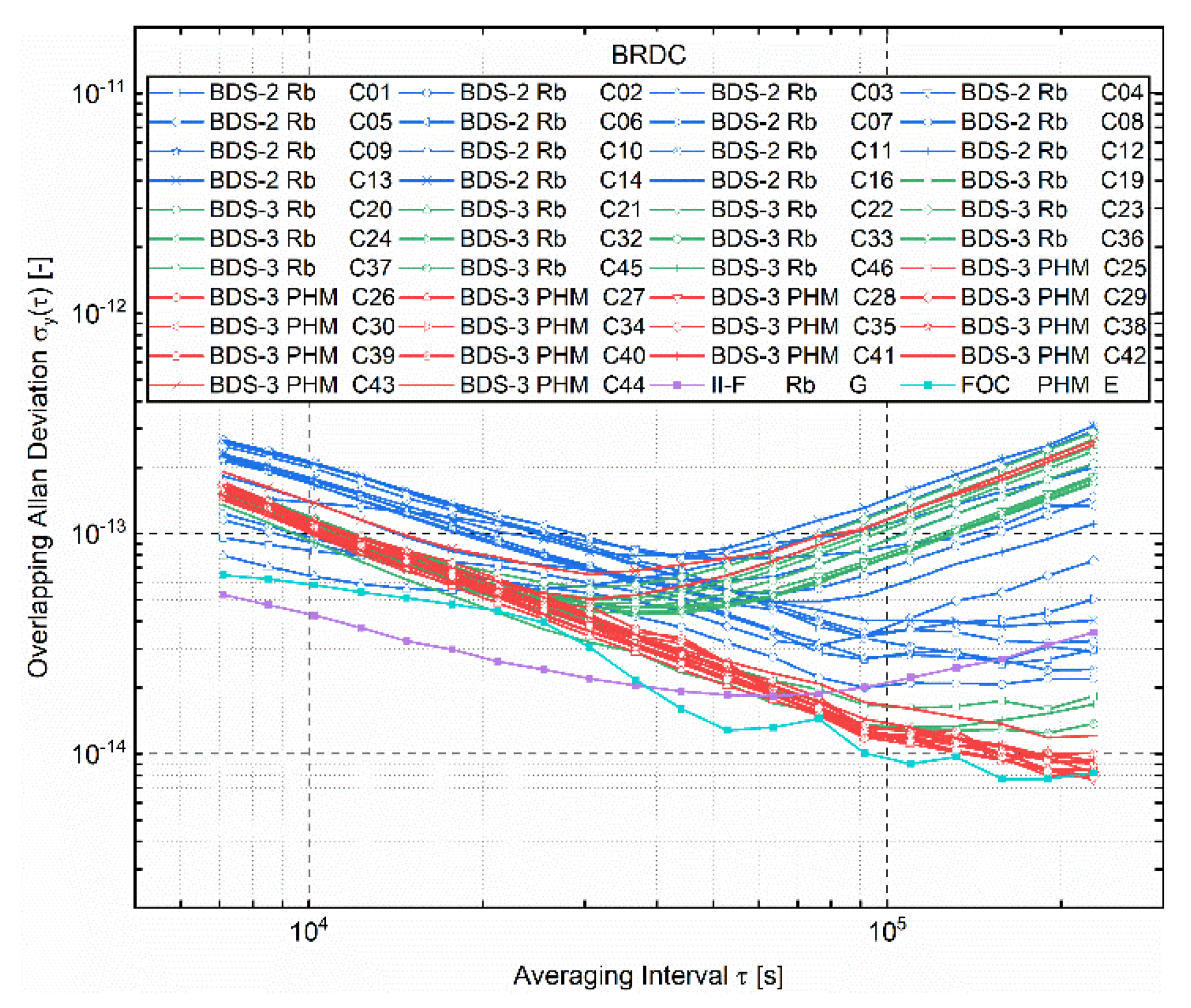


| System | PRN | Type | Manuf | Clock Type |
|---|---|---|---|---|
| BDS-2 | C01 C02 C03 C04 C05 | GEO | CASC | Rb |
| C06 C07 C08 C09 C10 C13 C16 | IGSO | CASC | Rb | |
| C11 C12 C14 | MEO | CASC | Rb | |
| BDS-3 | C19 C20 C21 C22 C23 C24 C32 C33 C36 C37 C45 C46 | MEO | CASC | Rb |
| C41 C42 | MEO | CASC | PHM | |
| C38 C39 C40 | IGSO | CASC | PHM | |
| C59 C60 C61(Testing) | GEO | CASC | PHM | |
| C25 C26 C27 C28 C29 C30 C34 C35 C43 C44 | MEO | SECM | PHM |
| System | STD (ns) |
|---|---|
| GPS | 0.13 |
| BDS-2 | 0.16 |
| BDS-3 | 0.07 |
| Galileo | 0.07 |
| Station | Rec Type | GFZ | WHU | BRDC | GFZ-WHU |
|---|---|---|---|---|---|
| BJF1 | CETC | −0.21 | −3.24 | 5.51 | 3.03 |
| GUA1 | BD070 | 2.69 | −0.58 | 6.66 | 3.27 |
| GUAM | JAVAD TRE_3 | −2.25 | −6.03 | 2.98 | 3.78 |
| KUN1 | UB4B0 | 0.68 | −2.42 | 4.86 | 3.1 |
| LHA1 | CETC | 0.11 | −3.12 | 5.37 | 3.23 |
| SGOC | JAVAD TRE_3 | −3.06 | −6.90 | 0.54 | 3.84 |
| SHA1 | UB4B0 | 0.48 | −2.35 | 5.63 | 2.83 |
| WUH1 | CETC | 0.58 | −2.72 | 5.91 | 3.3 |
| WUH2 | JAVAD TRE_3 | −2.83 | −6.45 | 1.30 | 3.62 |
| XIA1 | BD070 | 3.73 | 0.10 | 7.07 | 3.63 |
| Item | Strategy |
|---|---|
| Observation | IF combination observation of B1I/B3I pseudorange and carrier phase |
| Solution | Restart per hour |
| Ephemeris | Broadcast ephemeris and precise products of GFZ, WHU |
| Receiver clocks | Estimated as white noise process |
| ISB | Estimated as random constant |
| Troposphere | Prior model with remaining estimated as a random walk process |
| Satellite code bias | Corrected with TGD of broadcast ephemeris |
| Ambiguity | Float values |
| Elevation angle cutoff | 7° |
| Weighting | 3 dm for pseudorange and 3 mm for phase, double for BDS-2 Concerning elevation-dependent weighting, 1 for E > 30°, otherwise 2sin(E); the weighting of broadcast ephemeris is determined according to Table 2 |
| Products | Strategy | Vertical | Horizontal |
|---|---|---|---|
| Broadcast ephemeris | GPS | 0.86 | 0.52 |
| BDS-3 | 0.53 | 0.46 | |
| BDS | 0.50 | 0.43 | |
| BDS-23 | 0.46 | 0.41 | |
| Constant ISB | 0.46 | 0.39 | |
| Precise products | GFZ | 0.11 | 0.15 |
| WHU | 0.11 | 0.15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, S.; Mao, F.; Gong, X.; Lou, Y.; Xu, X.; Zhou, Y. Evaluation of BDS-2 and BDS-3 Satellite Atomic Clock Products and Their Effects on Positioning. Remote Sens. 2021, 13, 5041. https://doi.org/10.3390/rs13245041
Gu S, Mao F, Gong X, Lou Y, Xu X, Zhou Y. Evaluation of BDS-2 and BDS-3 Satellite Atomic Clock Products and Their Effects on Positioning. Remote Sensing. 2021; 13(24):5041. https://doi.org/10.3390/rs13245041
Chicago/Turabian StyleGu, Shengfeng, Feiyu Mao, Xiaopeng Gong, Yidong Lou, Xueyong Xu, and Ye Zhou. 2021. "Evaluation of BDS-2 and BDS-3 Satellite Atomic Clock Products and Their Effects on Positioning" Remote Sensing 13, no. 24: 5041. https://doi.org/10.3390/rs13245041






