New Insights into Pore Structure and Hydraulic Conductivity of Sodium Hydroxide Alkali-Activated Slag through Advanced Modelling
Abstract
:1. Introduction
2. Experimental Procedure
2.1. Materials
2.2. Methods
2.2.1. Open Porosity
2.2.2. Water Vapour Desorption
2.2.3. BET Method
2.2.4. BJH Method
2.2.5. Hydraulic Conductivity
Boundary Conditions
3. Results and Discussion
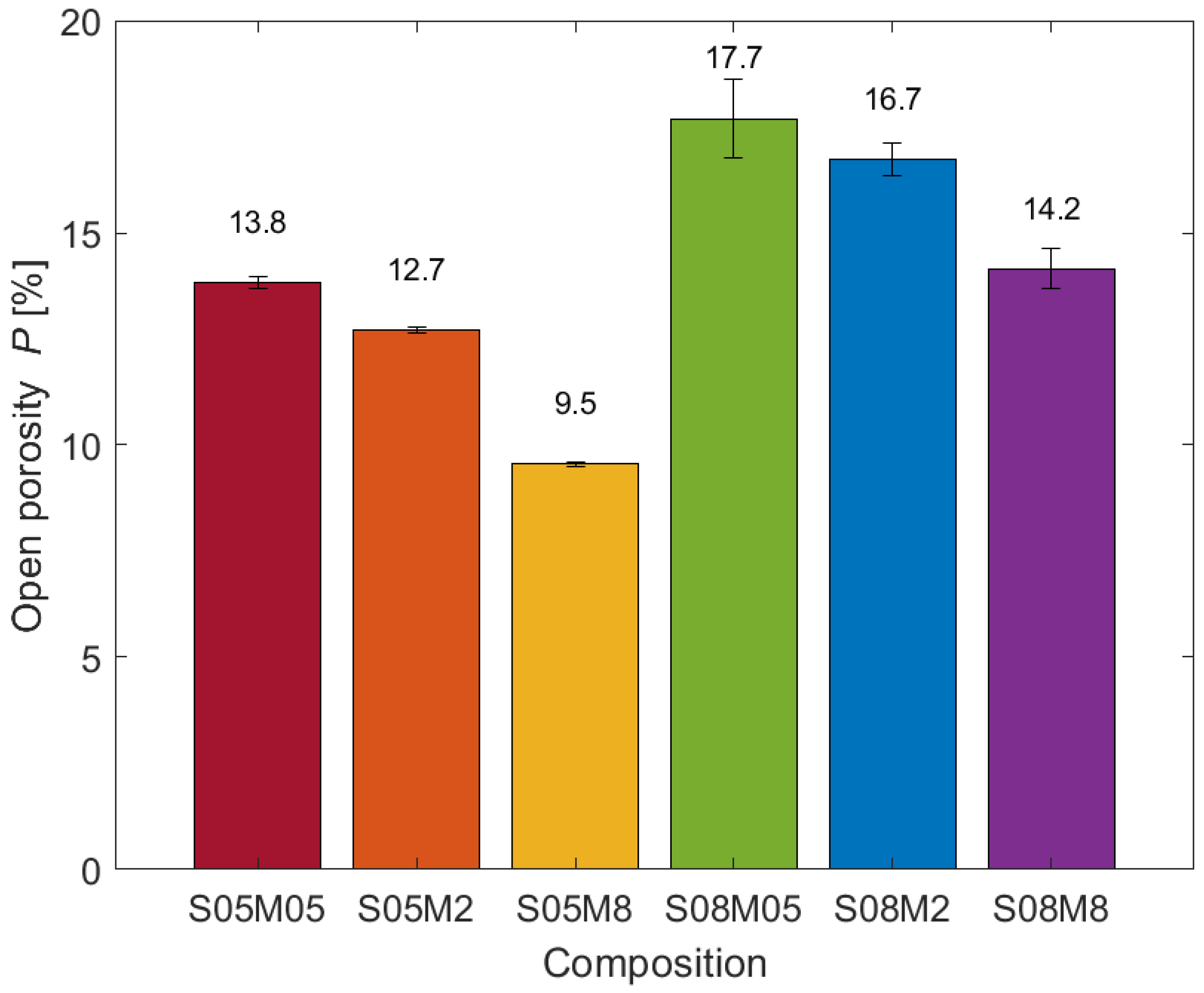
3.1. Open Porosity
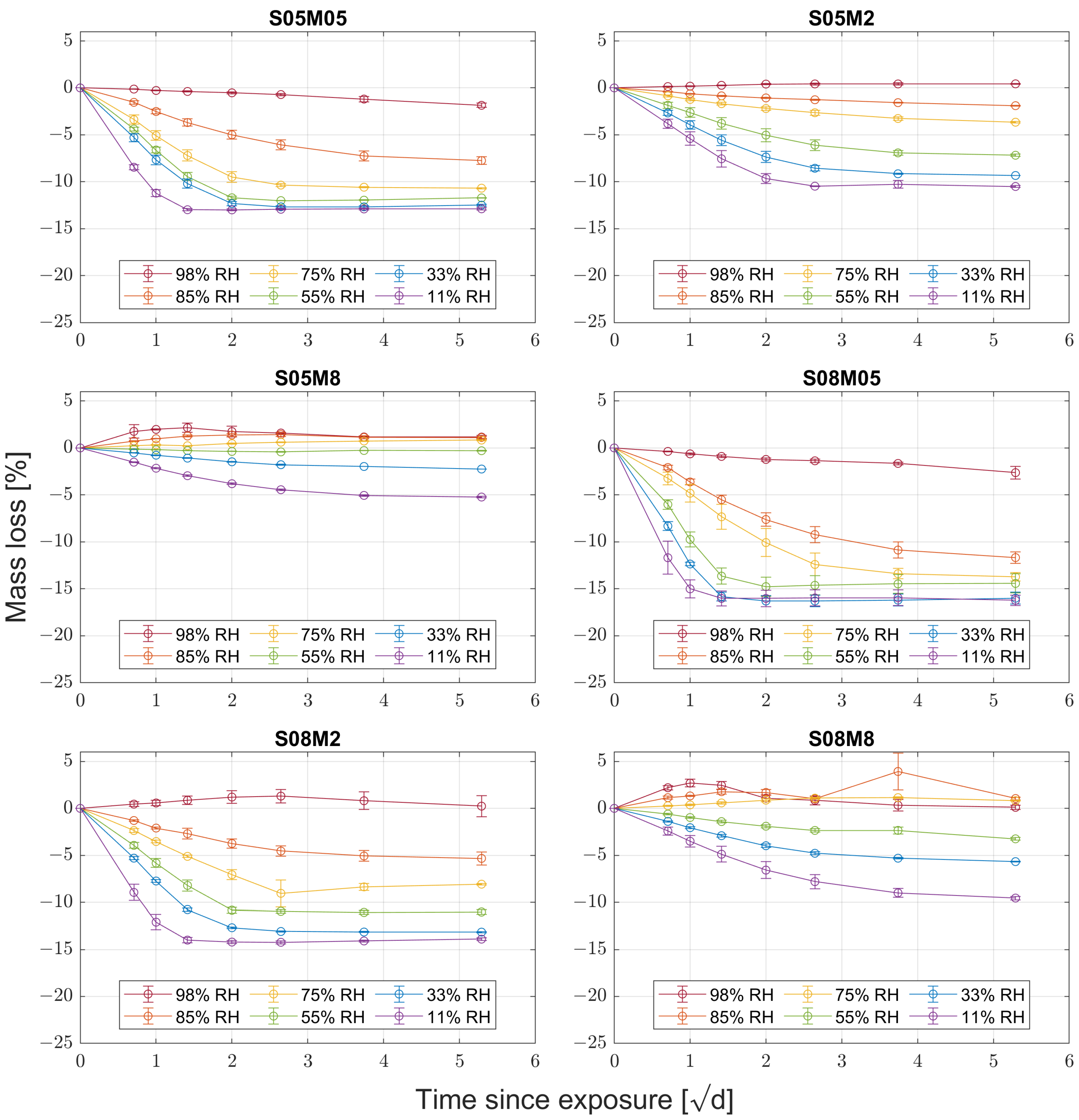
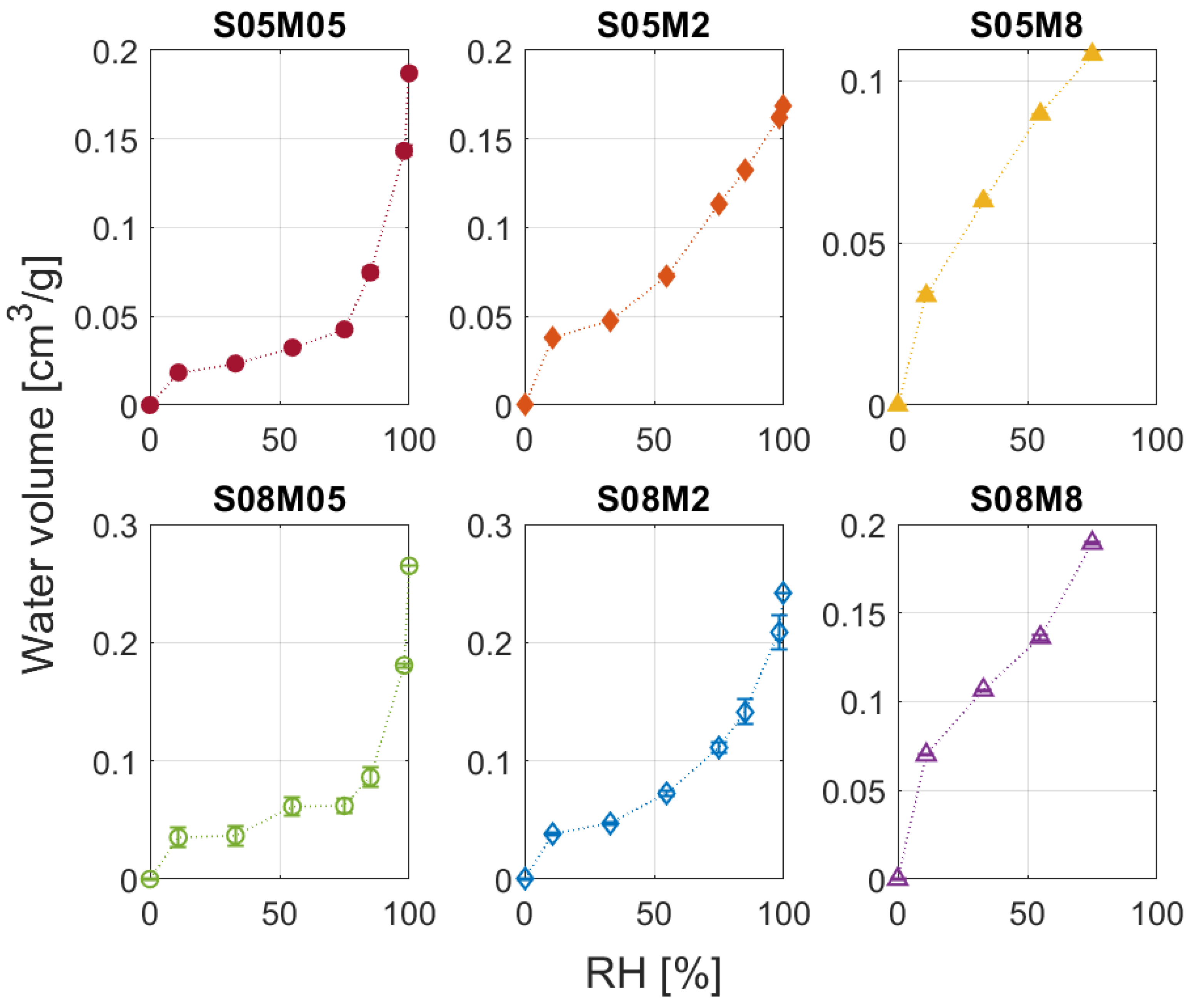
3.2. Water Vapour Desorption

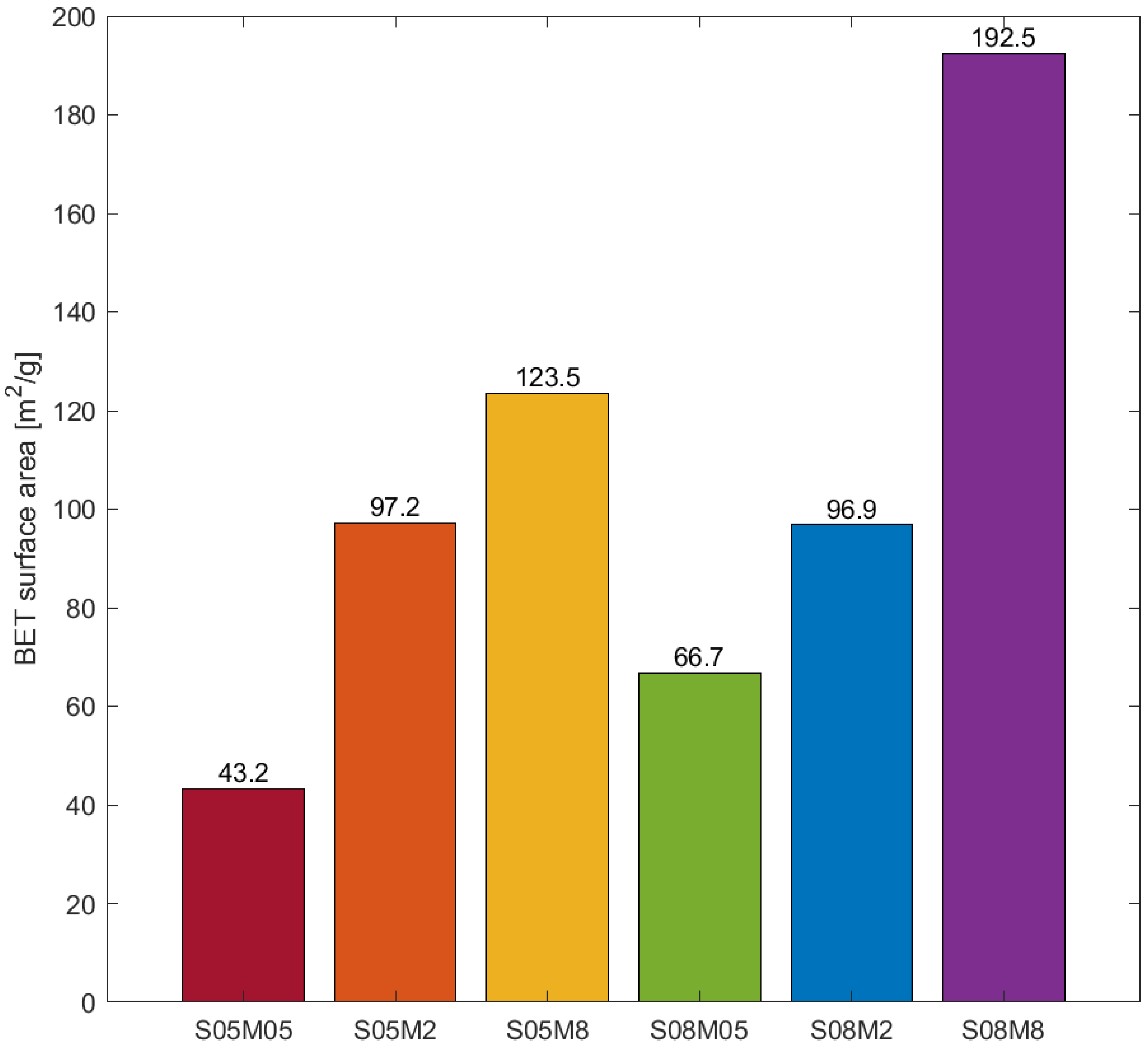
3.3. BET Surface Area
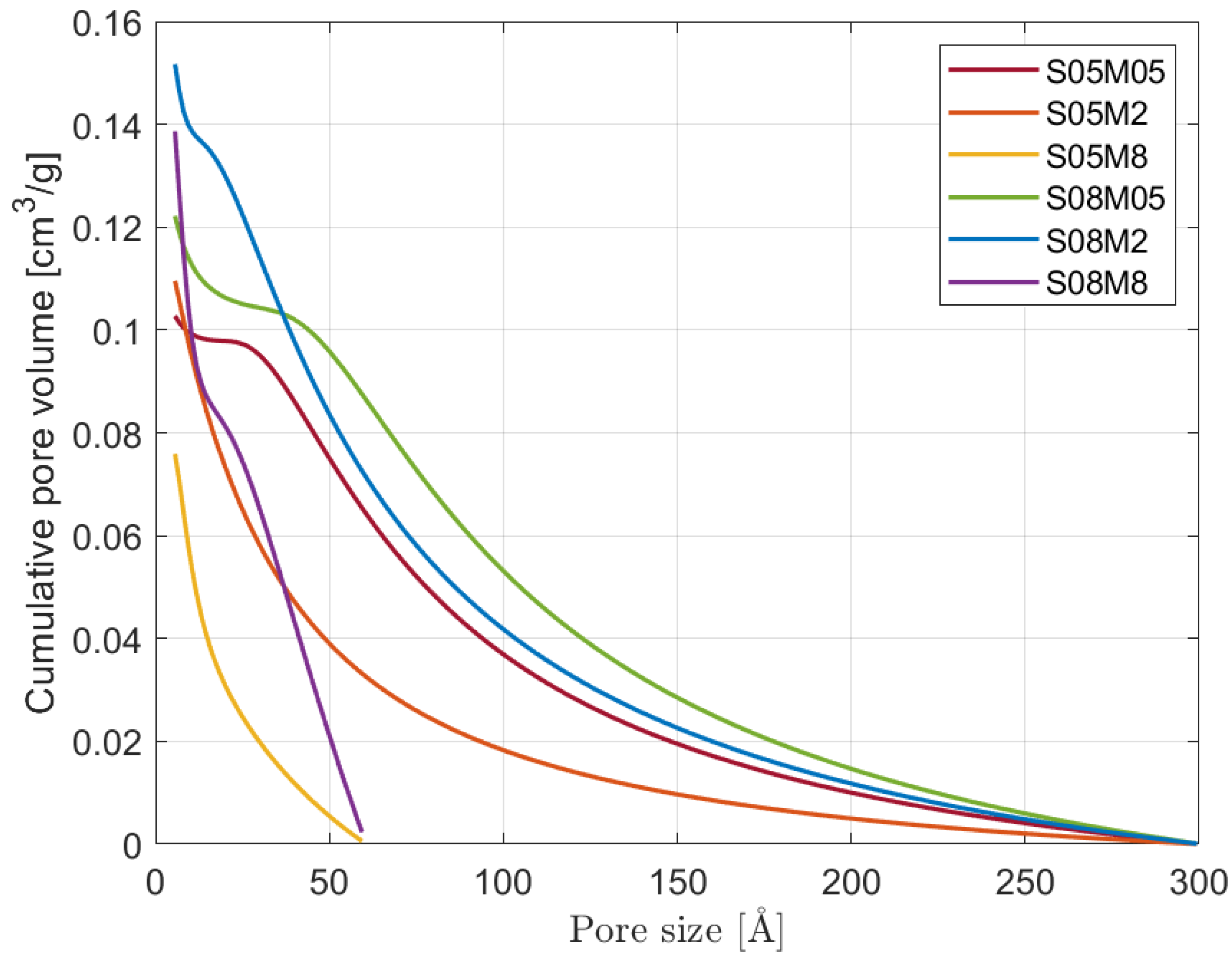
3.4. BJH Pore Size Distribution
- Note that 0.5 M compositions present a small number of micropores compared to the other compositions. The results are in line with what was observed for the porosity and the BET surface area: even though S08M05 presents the highest porosity, its surface area is relatively small due to a coarser pore structure, as visible in Figure 10.
- S08M05 does not present the highest open porosity value as it did during the open porosity test; a possible reason for this is that preliminary tests showed very low mechanical strength that may have allowed for a higher cracking formation during the drying phase in the oven, causing an increase in the number of connected pores and a subsequent higher mass loss. Specifically, the shrinkage experienced by the material due to the drying process leads to the appearance of tensile stresses that can cause the formation of microcracks, especially for materials with very low tensile strength [5]. As a consequence, the microcracks may increase the volume of open pores observed during the test [77,78]. S05M05 shows the same behaviour if compared to low s/b compositions.
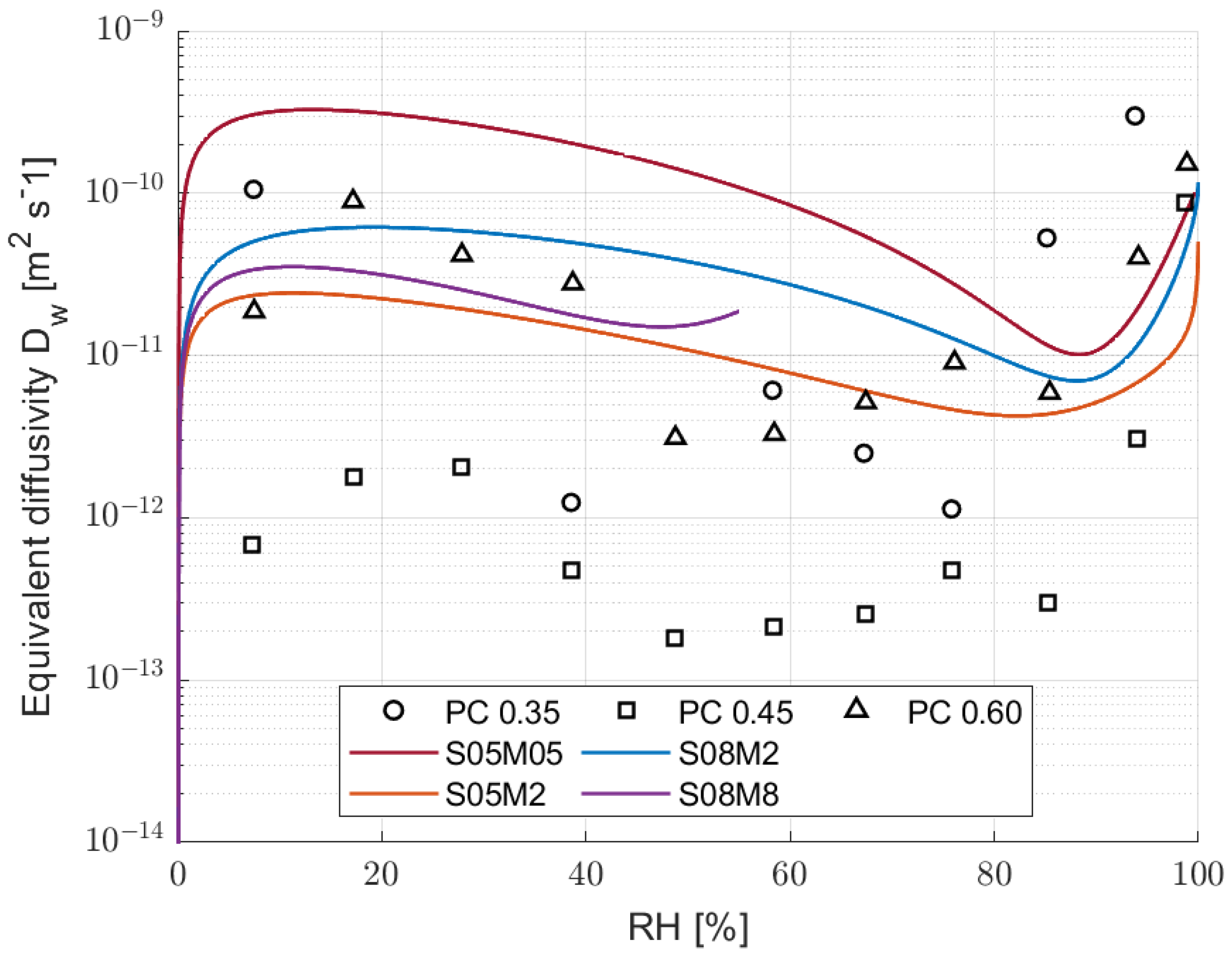
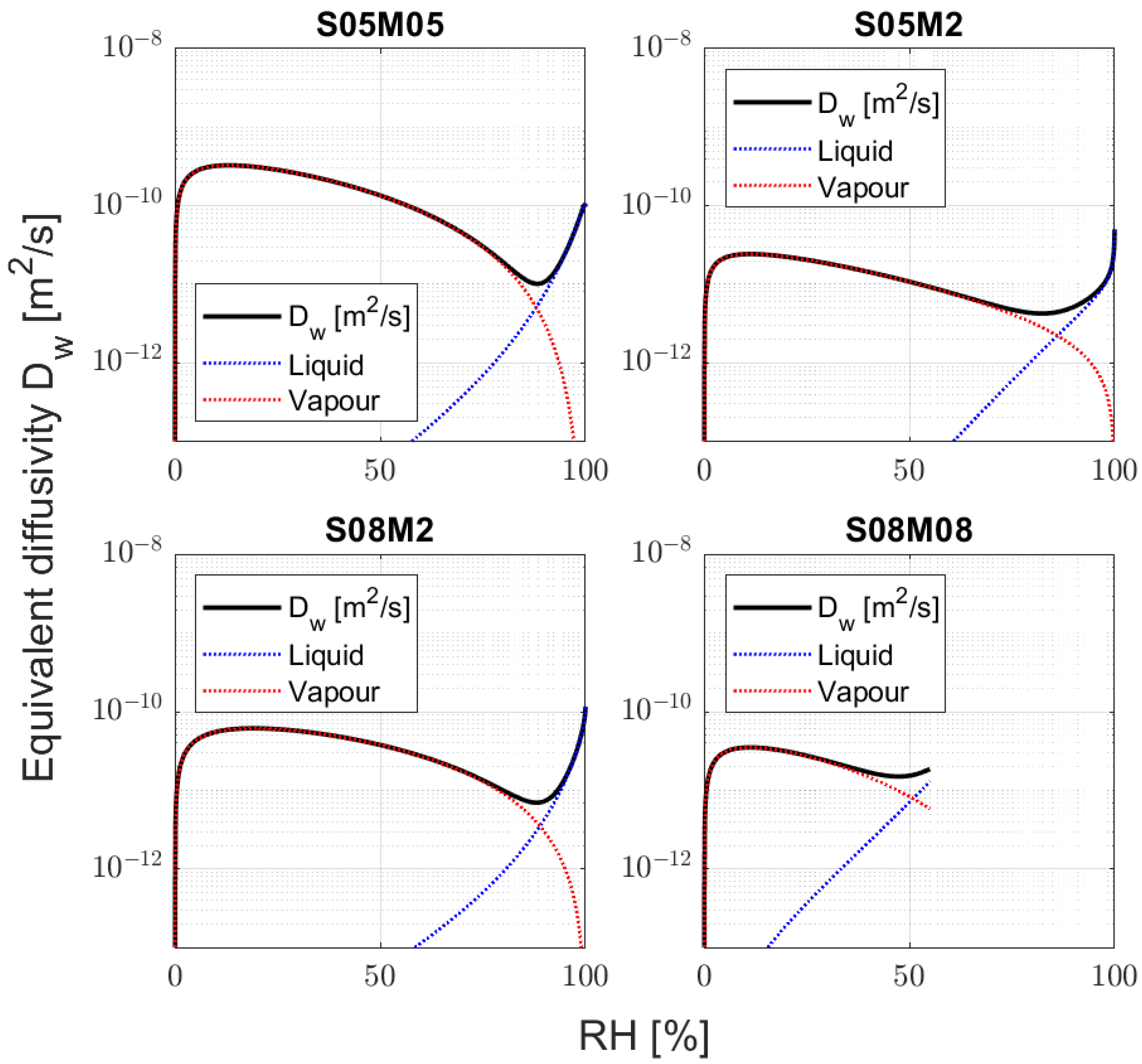
3.5. Hydraulic Conductivity
4. Conclusions
- Increasing the molarity of the activating solution refines the pore structure of the material.
- Increasing the solution-to-binder ratio not only increases the total porosity but also seems to refine the pore structure itself.
- High molarity compositions are not suitable for tests in high relative humidity conditions where carbonation takes place, as deliquescence greatly affects the results obtained.
- The tests on the total porosity of 0.5 M compositions show a discrepancy, possibly due to the test conditions and the low mechanical strength.
- The model proved to be able to predict the desorption isotherms from short drying experiments for AAS as well.
- The leading drying mechanism of alkali-activated slag is water vapour transport, making it fundamentally different from that of PC.
- The kinetics of drying strongly depends on external relative humidity, especially between 85 and 50%, which is the typical external RH.
- The modelling of the hydraulic conductivity is not applicable to high molarity conditions due to mechanical and modelling reasons, but also for the chemical activity—namely, carbonation and deliquescence—which fall out of the applicability of the model used, as it considers a fixed and unreactive microstructure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| AAS | alkali-activated slag |
| OPC | ordinary Portland cement |
| s/b | solution-to-binder ratio |
| RH | relative humidity |
| WVD | water vapour desorption |
| DoS | degree of saturation |
| BET | Brunauer–Emmett–Teller |
| BJH | Barret–Joyner–Halenda |
| HSDB | Hazardous Substances Data Bank |
References
- Turner, L.K.; Collins, F.G. Carbon Dioxide Equivalent (CO2-e) Emissions: A Comparison between Geopolymer and OPC Cement Concrete. Constr. Build. Mater. 2013, 43, 125–130. [Google Scholar] [CrossRef]
- Benhelal, E.; Zahedi, G.; Shamsaei, E.; Bahadori, A. Global Strategies and Potentials to Curb CO2 Emissions in Cement Industry. J. Clean. Prod. 2013, 51, 142–161. [Google Scholar] [CrossRef]
- Naqi, A.; Jang, J.G. Recent Progress in Green Cement Technology Utilizing Low-Carbon Emission Fuels and Raw Materials: A Review. Sustainability 2019, 11, 537. [Google Scholar] [CrossRef]
- Scrivener, K.L.; Kirkpatrick, R.J. Innovation in use and research on cementitious material. Cem. Concr. Res. 2008, 38, 128–136. [Google Scholar] [CrossRef]
- Sirotti, M.; Delsaute, B.; Staquet, S. New Experimental Evidence for Drying Shrinkage of Alkali-Activated Slag with Sodium Hydroxide. Materials 2023, 16, 5659. [Google Scholar] [CrossRef] [PubMed]
- Tian, B.; Li, X.; Lv, Y.; Xu, J.; Ma, W.; He, C.; Chen, Y.; Jian, S.; Wang, W.; Zhang, C.; et al. Effect of Rice Husk Ash on the Properties of Alkali-Activated Slag Pastes: Shrinkage, Hydration and Mechanical Property. Materials 2023, 16, 3148. [Google Scholar] [CrossRef] [PubMed]
- Ye, H.; Radlińska, A. Shrinkage Mechanisms of Alkali-Activated Slag. Cem. Concr. Res. 2016, 88, 126–135. [Google Scholar] [CrossRef]
- Jang, J.; Ahn, Y.; Souri, H.; Lee, H. A Novel Eco-Friendly Porous Concrete Fabricated with Coal Ash and Geopolymeric Binder: Heavy Metal Leaching Characteristics and Compressive Strength. Constr. Build. Mater. 2015, 79, 173–181. [Google Scholar] [CrossRef]
- Thomas, R.J.; Ye, H.; Radlińska, A.; Peethamparan, S. Alkali-Activated Slag Cement Concrete. Concr. Int. 2016, 38, 33–38. [Google Scholar]
- Juenger, M.; Winnefeld, F.; Provis, J.; Ideker, J. Advances in alternative cementitious binders. Cem. Concr. Res. 2011, 41, 1232–1243. [Google Scholar] [CrossRef]
- Hojati, M.; Rajabipour, F.; Radlińska, A. Drying Shrinkage of Alkali Activated Fly Ash: Effect of Activator Composition and Ambient Relative Humidity. In Proceedings of the Fourth International Conference on Sustainable Construction Materials and Technologies, Las Vegas, NV, USA, 7–11 August 2016; p. 11. [Google Scholar]
- Azenha, M. Numerical Simulation of the Structural Behaviour of Concrete Since Its Early Ages. Ph.D. Thesis, Faculdade de Engenharia da Universidade do Porto, Porto, Portugal, 2009. [Google Scholar]
- Delsaute, B.; Staquet, S. Monitoring of the Thermal and Autogenous Strain. In Advanced Techniques for Testing of Cement-Based Materials; Serdar, M., Gabrijel, I., Schlicke, D., Staquet, S., Azenha, M., Eds.; Springer International Publishing: Berlin/Heidelberg, Germany, 2020; pp. 135–176. [Google Scholar] [CrossRef]
- Brooks, J.J.; Johari, M.A.M. Effect of Metakaolin on Creep and Shrinkage of Concrete. Cem. Concr. Compos. 2001, 23, 495–502. [Google Scholar] [CrossRef]
- Powers, T.C. The Thermodynamics of Volume Change and Creep. Mater. Constr. 1968, 1, 487–507. [Google Scholar] [CrossRef]
- Lura, P. Autogenous Deformation and Internal Curing of Concrete. Ph.D. Thesis, DUP, Delft, The Netherlands, 2003. [Google Scholar]
- Mehta, P.K.; Monteiro, P.J.M.; Monteiro, P.J. Concrete: Microstructure, Properties, and Materials; [CD-ROM with 1000+ Powerpoint Slides, Videos, Bonus Material, and More], 3rd ed.; Fully Rev. and Updated ed.; McGraw-Hill: New York, NY, USA, 2005. [Google Scholar]
- Hojati, M. Shrinkage and Creep of Alkali-Activated Binders. Ph.D. Thesis, The Pennsylvania State University, State College, PA, USA, 2017. [Google Scholar]
- Ye, H.; Cartwright, C.; Rajabipour, F.; Radlińska, A. Understanding the drying shrinkage performance of alkali-activated slag mortars. Cem. Concr. Compos. 2017, 76, 13–24. [Google Scholar] [CrossRef]
- Hojati, M.; Rajabipour, F.; Radlińska, A. Drying Shrinkage of Alkali-Activated Cements: Effect of Humidity and Curing Temperature. Mater. Struct. 2019, 52, 118. [Google Scholar] [CrossRef]
- Melo Neto, A.A.; Cincotto, M.A.; Repette, W. Drying and Autogenous Shrinkage of Pastes and Mortars with Activated Slag Cement. Cem. Concr. Res. 2008, 38, 565–574. [Google Scholar] [CrossRef]
- Ben Haha, M.; Le Saout, G.; Winnefeld, F.; Lothenbach, B. Influence of Activator Type on Hydration Kinetics, Hydrate Assemblage and Microstructural Development of Alkali Activated Blast-Furnace Slags. Cem. Concr. Res. 2011, 41, 301–310. [Google Scholar] [CrossRef]
- Fernández-Jiménez, A.; Palomo, A. Composition and Microstructure of Alkali Activated Fly Ash Binder: Effect of the Activator. Cem. Concr. Res. 2005, 35, 1984–1992. [Google Scholar] [CrossRef]
- Shi, C. Strength, Pore Structure and Permeability of Alkali-Activated Slag Mortars. Cem. Concr. Res. 1996, 26, 1789–1799. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, H. Analysing the Relation between Pore Structure and Permeability of Alkali-Activated Concrete Binders. In Handbook of Alkali-Activated Cements, Mortars and Concretes; Pacheco-Torgal, F., Labrincha, J., Leonelli, C., Palomo, A., Chindaprasirt, P., Eds.; Woodhead Publishing: Sawston, UK, 2014; pp. 235–264. [Google Scholar] [CrossRef]
- Criado, M.; Fernández Jiménez, A.; Sobrados, I.; Palomo, A.; Sanz, J. Effect of Relative Humidity on the Reaction Products of Alkali Activated Fly Ash. J. Eur. Ceram. Soc. 2012, 32, 2799–2807. [Google Scholar] [CrossRef]
- Baroghel-Bouny, V. Water Vapour Sorption Experiments on Hardened Cementitious Materials. Cem. Concr. Res. 2007, 37, 414–437. [Google Scholar] [CrossRef]
- Wu, M.; Johannesson, B.; Geiker, M. Application of Water Vapor Sorption Measurements for Porosity Characterization of Hardened Cement Pastes. Constr. Build. Mater. 2014, 66, 621–633. [Google Scholar] [CrossRef]
- Zeng, Q.; Zhang, D.; Sun, H.; Li, K. Characterizing Pore Structure of Cement Blend Pastes Using Water Vapor Sorption Analysis. Mater. Charact. 2014, 95, 72–84. [Google Scholar] [CrossRef]
- Snoeck, D.; Velasco, L.; Mignon, A.; Van Vlierberghe, S.; Dubruel, P.; Lodewyckx, P.; De Belie, N. The Influence of Different Drying Techniques on the Water Sorption Properties of Cement-Based Materials. Cem. Concr. Res. 2014, 64, 54–62. [Google Scholar] [CrossRef]
- Snoeck, D.; Velasco, L.; Mignon, A.; Van Vlierberghe, S.; Dubruel, P.; Lodewyckx, P.; De Belie, N. The Effects of Superabsorbent Polymers on the Microstructure of Cementitious Materials Studied by Means of Sorption Experiments. Cem. Concr. Res. 2015, 77, 26–35. [Google Scholar] [CrossRef]
- Odler, I. The BET-specific Surface Area of Hydrated Portland Cement and Related Materials. Cem. Concr. Res. 2003, 33, 2049–2056. [Google Scholar] [CrossRef]
- Alderete, N.; Villagrán, Y.; Mignon, A.; Snoeck, D.; De Belie, N. Pore Structure Description of Mortars Containing Ground Granulated Blast-Furnace Slag by Mercury Intrusion Porosimetry and Dynamic Vapour Sorption. Constr. Build. Mater. 2017, 145, 157–165. [Google Scholar] [CrossRef]
- Brunauer, S.; Emmett, P.H.; Teller, E. Adsorption of Gases in Multimolecular Layers. J. Am. Chem. Soc. 1938, 60, 309–319. [Google Scholar] [CrossRef]
- Garci Juenger, M.C.; Jennings, H.M. The Use of Nitrogen Adsorption to Assess the Microstructure of Cement Paste. Cem. Concr. Res. 2001, 31, 883–892. [Google Scholar] [CrossRef]
- Langmuir, I. The Adsorption of Gases on Plane Surfaces of Glass, Mica, and Platinum. J. Am. Chem. Soc. 1918, 40, 1361–1403. [Google Scholar] [CrossRef]
- Dollimore, D.; Spooner, P.; Turner, A. The Bet Method of Analysis of Gas Adsorption Data and Its Relevance to the Calculation of Surface Areas. Surf. Technol. 1976, 4, 121–160. [Google Scholar] [CrossRef]
- Barrett, E.P.; Joyner, L.G.; Halenda, P.P. The Determination of Pore Volume and Area Distributions in Porous Substances. I. Computations from Nitrogen Isotherms. J. Am. Chem. Soc. 1951, 73, 373–380. [Google Scholar] [CrossRef]
- Snoeck, D.; Pel, L.; De Belie, N. Comparison of Different Techniques to Study the Nanostructure and the Microstructure of Cementitious Materials with and without Superabsorbent Polymers. Constr. Build. Mater. 2019, 223, 244–253. [Google Scholar] [CrossRef]
- Wheeler, A. Presentations at Catalysis Symposia. In Proceedings of the Gibson Island A. A. A. S. Conferences, Gibson Island, MD, USA, June 1945. [Google Scholar]
- Carette, J.; Soleilhet, F.; Benboudjema, F.; Ma, X.; Nahas, G.; Abahri, K.; Darquennes, A.; Bennacer, R. Identifying the mechanisms of concrete drying: An experimental-numerical approach. Constr. Build. Mater. 2020, 230, 117001. [Google Scholar] [CrossRef]
- Sbartaï, Z.M.; Breysse, D.; Larget, M.; Balayssac, J.P. Combining NDT Techniques for Improved Evaluation of Concrete Properties. Cem. Concr. Compos. 2012, 34, 725–733. [Google Scholar] [CrossRef]
- Sbartaï, Z.; Laurens, S.; Rhazi, J.; Balayssac, J.; Arliguie, G. Using Radar Direct Wave for Concrete Condition Assessment: Correlation with Electrical Resistivity. J. Appl. Geophys. 2007, 62, 361–374. [Google Scholar] [CrossRef]
- Jerjen, I.; Poulikakos, L.D.; Plamondon, M.; Schuetz, P.; Luethi, T.; Flisch, A. Drying of Porous Asphalt Concrete Investigated by X-Ray Computed Tomography. Phys. Procedia 2015, 69, 451–456. [Google Scholar] [CrossRef]
- Popovics, S. Effects of Uneven Moisture Distribution on the Strength of and Wave Velocity in Concrete. Ultrasonics 2005, 43, 429–434. [Google Scholar] [CrossRef]
- Dai, X.; Aydın, S.; Yardımcı, M.Y.; Lesage, K.; De Schutter, G. Effects of Activator Properties and GGBFS/FA Ratio on the Structural Build-up and Rheology of AAC. Cem. Concr. Res. 2020, 138, 106253. [Google Scholar] [CrossRef]
- EN 196-1:1987; Methods of Testing Cement—Part 1: Determination of Strength. European Committee for Standardization: Brussels, Belgium, 2016.
- Safiuddin, M.; Hearn, N. Comparison of ASTM saturation techniques for measuring the permeable porosity of concrete. Cem. Concr. Res. 2005, 35, 1008–1013. [Google Scholar] [CrossRef]
- Greenspan, L. Humidity Fixed Points of Binary Saturated Aqueous Solutions. J. Res. Natl. Bur. Stand. Sect. Phys. Chem. 1977, 81A, 89. [Google Scholar] [CrossRef]
- Panesar, D.K.; Francis, J. Influence of Limestone and Slag on the Pore Structure of Cement Paste Based on Mercury Intrusion Porosimetry and Water Vapour Sorption Measurements. Constr. Build. Mater. 2014, 52, 52–58. [Google Scholar] [CrossRef]
- Cieśla, J.; Sokołowska, Z.; Witkowska-Walczak, B.; Skic, K. Adsorption of Water Vapour and the Specific Surface Area of Arctic Zone Soils (Spitsbergen). Int. Agrophysics 2018, 32, 19–27. [Google Scholar] [CrossRef]
- Badmann, R.; Stockhausen, N.; Setzer, M.J. The Statistical Thickness and the Chemical Potential of Adsorbed Water Films. J. Colloid Interface Sci. 1981, 82, 534–542. [Google Scholar] [CrossRef]
- PubChem, Sodium Hydroxide. Available online: https://pubchem.ncbi.nlm.nih.gov/compound/14798 (accessed on 17 January 2023).
- Baroghel-Bouny, V. Water Vapour Sorption Experiments on Hardened Cementitious Materials. Part II: Essential Tool for Assessment of Transport Properties and for Durability Prediction. Cem. Concr. Res. 2007, 37, 438–454. [Google Scholar] [CrossRef]
- Mainguy, M.; Coussy, O.; Baroghel-Bouny, V. Role of Air Pressure in Drying of Weakly Permeable Materials. J. Eng. Mech. 2001, 127, 582–592. [Google Scholar] [CrossRef]
- Mualem, Y. A New Model for Predicting the Hydraulic Conductivity of Unsaturated Porous Media. Water Resour. Res. 1976, 12, 513–522. [Google Scholar] [CrossRef]
- Monlouis-Bonnaire, J.; Verdier, J.; Perrin, B. Prediction of the Relative Permeability to Gas Flow of Cement-Based Materials. Cem. Concr. Res. 2004, 34, 737–744. [Google Scholar] [CrossRef]
- Poyet, S.; Charles, S.; Honoré, N.; L’hostis, V. Assessment of the Unsaturated Water Transport Properties of an Old Concrete: Determination of the Pore-Interaction Factor. Cem. Concr. Res. 2011, 41, 1015–1023. [Google Scholar] [CrossRef]
- Zhou, C. Predicting Water Permeability and Relative Gas Permeability of Unsaturated Cement-Based Material from Hydraulic Diffusivity. Cem. Concr. Res. 2014, 58, 143–151. [Google Scholar] [CrossRef]
- Thiery, M.; Baroghel-Bouny, V.; Bourneton, N.; Villain, G.; Stéfani, C. Modélisation du séchage des bétons: Analyse des différents modes de transfert hydrique. Rev. Eur. Genie Civ. 2007, 11, 541–577. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A Closed-form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Chen, X.; Wu, S. Influence of Water-to-Cement Ratio and Curing Period on Pore Structure of Cement Mortar. Constr. Build. Mater. 2013, 38, 804–812. [Google Scholar] [CrossRef]
- Ma, Y.; Ye, G. The Shrinkage of Alkali Activated Fly Ash. Cem. Concr. Res. 2015, 68, 75–82. [Google Scholar] [CrossRef]
- Gijbels, K.; Pontikes, Y.; Samyn, P.; Schreurs, S.; Schroeyers, W. Effect of NaOH Content on Hydration, Mineralogy, Porosity and Strength in Alkali/Sulfate-Activated Binders from Ground Granulated Blast Furnace Slag and Phosphogypsum. Cem. Concr. Res. 2020, 132, 106054. [Google Scholar] [CrossRef]
- Aliabdo, A.A.; Abd Elmoaty, A.E.M.; Emam, M.A. Factors Affecting the Mechanical Properties of Alkali Activated Ground Granulated Blast Furnace Slag Concrete. Constr. Build. Mater. 2019, 197, 339–355. [Google Scholar] [CrossRef]
- Saludung, A.; Azeyanagi, T.; Ogawa, Y.; Kawai, K. Effect of Silica Fume on Efflorescence Formation and Alkali Leaching of Alkali-Activated Slag. J. Clean. Prod. 2021, 315, 128210. [Google Scholar] [CrossRef]
- Škvára, F.; Šmilauer, V.; Hlaváček, P.; Kopecký, L.; Cílová, Z. A Weak Alkali Bond in (N, K)–A–S–H Gels: Evidence from Leaching and Modeling. Ceram. Silik. 2012, 56, 9. [Google Scholar]
- Tang, D.; Yang, C.; Li, X.; Zhu, X.; Yang, K.; Yu, L. Mitigation of Efflorescence of Alkali-Activated Slag Mortars by Incorporating Calcium Hydroxide. Constr. Build. Mater. 2021, 298, 123873. [Google Scholar] [CrossRef]
- Kang, S.P.; Kwon, S.J. Effects of Red Mud and Alkali-Activated Slag Cement on Efflorescence in Cement Mortar. Constr. Build. Mater. 2017, 133, 459–467. [Google Scholar] [CrossRef]
- Zhang, Z.; Provis, J.L.; Reid, A.; Wang, H. Fly Ash-Based Geopolymers: The Relationship between Composition, Pore Structure and Efflorescence. Cem. Concr. Res. 2014, 64, 30–41. [Google Scholar] [CrossRef]
- Rörig-Dalgaard, I. Direct Measurements of the Deliquescence Relative Humidity in Salt Mixtures Including the Contribution from Metastable Phases. ACS Omega 2021, 6, 16297–16306. [Google Scholar] [CrossRef]
- Liu, C.F.; Shih, S.M.; Yang, J.H. Reactivities of NaOH Enhanced Iron Blast Furnace Slag/Hydrated Lime Sorbents toward SO2 at Low Temperatures: Effects of the Presence of CO2, O2, and NOx. Ind. Eng. Chem. Res. 2010, 49, 515–519. [Google Scholar] [CrossRef]
- Reches, Y. Concrete on Mars: Options, challenges, and solutions for binder-based construction on the Red Planet. Cem. Concr. Compos. 2019, 104, 103349. [Google Scholar] [CrossRef]
- Ye, H.; Cartwright, C.; Rajabipour, F.; Radlińska, A. Effect of Drying Rate on Shrinkage of Alkali-Activated Slag Cements. In Proceedings of the International Conference on the Durability of Concrete Structures, West Lafayette, IN, USA, 24–26 July 2014. [Google Scholar]
- Auroy, M.; Poyet, S.; Le Bescop, P.; Torrenti, J.M.; Charpentier, T.; Moskura, M.; Bourbon, X. Impact of Carbonation on Unsaturated Water Transport Properties of Cement-Based Materials. Cem. Concr. Res. 2015, 74, 44–58. [Google Scholar] [CrossRef]
- Zhang, Z.; Yao, X.; Zhu, H. Potential Application of Geopolymers as Protection Coatings for Marine concreteII. Microstructure and Anticorrosion Mechanism. Appl. Clay Sci. 2010, 49, 7–12. [Google Scholar] [CrossRef]
- Wang, X.; Peng, Y.; Wang, J.; Zeng, Q. Pore Structure Damages in Cement-Based Materials by Mercury Intrusion: A Non-Destructive Assessment by X-Ray Computed Tomography. Materials 2019, 12, 2220. [Google Scholar] [CrossRef] [PubMed]
- Shi, D.; Winslow, D.N. Contact Angle and Damage during Mercury Intrusion into Cement Paste. Cem. Concr. Res. 1985, 15, 645–654. [Google Scholar] [CrossRef]
- Naqi, A.; Delsaute, B.; Königsberger, M.; Staquet, S. Monitoring early age elastic and viscoelastic properties of alkali-activated slag mortar by means of repeated minute-long loadings. Dev. Built Environ. 2023, 16, 100275. [Google Scholar] [CrossRef]
- Wang, S.D.; Scrivener, K.L.; Pratt, P. Factors Affecting the Strength of Alkali-Activated Slag. Cem. Concr. Res. 1994, 24, 1033–1043. [Google Scholar] [CrossRef]
- Dai, X.; Aydin, S.; Yardimci, M.Y.; Lesage, K.; De Schutter, G. Early Age Reaction, Rheological Properties and Pore Solution Chemistry of NaOH-activated Slag Mixtures. Cem. Concr. Compos. 2022, 133, 104715. [Google Scholar] [CrossRef]
- Pihlajavaara, S.E. Some Results of the Effect of Carbonation on the Porosity and Pore Size Distribution of Cement Paste. Mater. Constr. 1968, 1, 521–527. [Google Scholar] [CrossRef]
- Houst, Y.F.; Wittmann, F.H. Influence of Porosity and Water Content on the Diffusivity of CO2 and O2 through Hydrated Cement Paste. Cem. Concr. Res. 1994, 24, 1165–1176. [Google Scholar] [CrossRef]
- Puertas, F.; Palacios, M.; Vázquez, T. Carbonation Process of Alkali-Activated Slag Mortars. J. Mater. Sci. 2006, 41, 3071–3082. [Google Scholar] [CrossRef]
- Ye, H.; Radlińska, A. Carbonation-Induced Volume Change in Alkali-Activated Slag. Constr. Build. Mater. 2017, 144, 635–644. [Google Scholar] [CrossRef]
- Humad, A.M.; Provis, J.L.; Habermehl-Cwirzen, K.; Rajczakowska, M.; Cwirzen, A. Creep and Long-Term Properties of Alkali-Activated Swedish-Slag Concrete. J. Mater. Civ. Eng. 2021, 33, 04020475. [Google Scholar] [CrossRef]
- Zhang, Z.; Thiery, M.; Baroghel-Bouny, V. Investigation of Moisture Transport Properties of Cementitious Materials. Cem. Concr. Res. 2016, 89, 257–268. [Google Scholar] [CrossRef]
- Xiong, Q.X.; Tong, L.y.; Zhang, Z.; Shi, C.; Liu, Q.f. A New Analytical Method to Predict Permeability Properties of Cementitious Mortars: The Impacts of Pore Structure Evolutions and Relative Humidity Variations. Cem. Concr. Compos. 2023, 137, 104912. [Google Scholar] [CrossRef]
- Xu, Z.; Zhang, Z.; Huang, J.; Yu, K.; Zhong, G.; Chen, F.; Chen, X.; Yang, W.; Wang, Y. Effects of temperature, humidity and CO2 concentration on carbonation of cement-based materials: A review. Constr. Build. Mater. 2022, 346, 128399. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Raftshol, W.J. Effect of Cracking in Drying and Shrinkage Specimens. Cem. Concr. Res. 1982, 12, 209–226. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Şener, S.; Kim, J.K. Effect of Cracking on Drying Permeability and Diffusivity of Concrete. ACI Mater. J. 1987, 85, 351–357. [Google Scholar]
- Zhang, J.; Bian, F.; Zhang, Y.; Fang, Z.; Fu, C.; Guo, J. Effect of Pore Structures on Gas Permeability and Chloride Diffusivity of Concrete. Constr. Build. Mater. 2018, 163, 402–413. [Google Scholar] [CrossRef]
- Sarott, F.A.; Bradbury, M.; Pandolfo, P.; Spieler, P. Diffusion and Adsorption Studies on Hardened Cement Paste and the Effect of Carbonation on Diffusion Rates. Cem. Concr. Res. 1992, 22, 439–444. [Google Scholar] [CrossRef]




| Oxide | CaO | SiO2 | Al2O3 | MgO | SO3 | TiO2 | Fe2O3 | K2O | Na2O | MnO | BaO |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Content [%] | 40.8 | 33.3 | 12.3 | 7.8 | 2.3 | 1.3 | 0.4 | 0.7 | 0.4 | 0.4 | 0.3 |
| Composition | Solution-to-Binder Ratio | NaOH Concentration [M] | Sand-to-Paste Ratio | Water-to-Binder Ratio |
|---|---|---|---|---|
| S05M05 | 0.5 | 0.5 | 1 | 0.49 |
| S05M2 | 2 | 0.44 | ||
| S05M8 | 8 | 0.29 | ||
| S08M05 | 0.8 | 0.5 | 0.77 | |
| S08M2 | 2 | 0.69 | ||
| S08M8 | 8 | 0.43 |
| RH | 98% | 85% | 75% | 55% | 33% | 11% |
|---|---|---|---|---|---|---|
| Salt | K2SO4 | KCl | NaCl | Mg(NO3)2 | MgCl2 | LiCl |
| S05M05 | S05M2 | S05M8 | S08M05 | S08M2 | S08M8 |
|---|---|---|---|---|---|
| Yes | Yes | No | No | Yes | Only between 11 and 55% RH |
| Parameter | S05M05 | S05M2 | S05M8 | S08M05 | S08M2 | S08M8 | PC | Min | Max |
|---|---|---|---|---|---|---|---|---|---|
| 1.94 × 10−21 | 1.11 × 10−23 | 3.23 × 10−22 | 3.57 × 10−22 | 7.22 × 10−22 | 2.31 × 10−20 | 4.68 × 10−22 | - | - | |
| 10.23 | 1.37 | 1.05 | 0.82 | 1.03 | 1.95 | 1.65 | - | - | |
| 0.91 | 1.37 | 1.05 | 0.82 | 1.03 | 1.95 | 1.65 | - | - | |
| 1.09 | 0.99 | 0.28 | 1.72 | 0.54 | 0.20 | 0.39 | - | - | |
| 1.09 | 0.99 | 0.28 | 1.72 | 0.54 | 0.20 | 0.39 | - | - | |
| 4.11 | 5.00 | 5.00 | 1.62 | 4.68 | 5.00 | −1.57 | −4.00 | 5.00 | |
| 2.02 | 2.72 | 3.02 | 2.70 | 2.61 | 1.63 | 3.00 | 1.30 | 2.74 | |
| 3.48 | 0.86 | 4.88 | 4.29 | 1.38 | 2.81 | 3.72 | 3.30 | 4.20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sirotti, M.; Carette, J.; Staquet, S. New Insights into Pore Structure and Hydraulic Conductivity of Sodium Hydroxide Alkali-Activated Slag through Advanced Modelling. Materials 2024, 17, 363. https://doi.org/10.3390/ma17020363
Sirotti M, Carette J, Staquet S. New Insights into Pore Structure and Hydraulic Conductivity of Sodium Hydroxide Alkali-Activated Slag through Advanced Modelling. Materials. 2024; 17(2):363. https://doi.org/10.3390/ma17020363
Chicago/Turabian StyleSirotti, Marco, Jérôme Carette, and Stéphanie Staquet. 2024. "New Insights into Pore Structure and Hydraulic Conductivity of Sodium Hydroxide Alkali-Activated Slag through Advanced Modelling" Materials 17, no. 2: 363. https://doi.org/10.3390/ma17020363







