Elastic Critical Resistance of the Simple Beam Grillage Resulting from the Lateral Torsional Buckling Condition: FEM Modelling and Analytical Considerations
Abstract
:1. Introduction
- (i)
- A departure from fork support, classically adopted in beam stability analysis;
- (ii)
- Taking into account the interaction of component beams and joint properties (including the stiffening type) in the ECR analysis of H-type grillage;
- (iii)
- Proposing a method for the determination of the indexes of the critical beam elastic restraint in stiffening beams;
- (iv)
- Demonstrating that the ECR of H-type grillage can be determined based on the critical moment of the critical beam. Further, on the basis of Mcr, it is possible to determine the so-called relative slenderness and reduction factor for LTB (taking into account the equivalent imperfection according to [1]) of the design resistance of the beam against LTB;
- (v)
- Demonstrating that the approach based on the critical beam analysis offers a more accurate representation of the SBG’s actual performance in the elastic LTB phase while using a relatively simple analytical model.
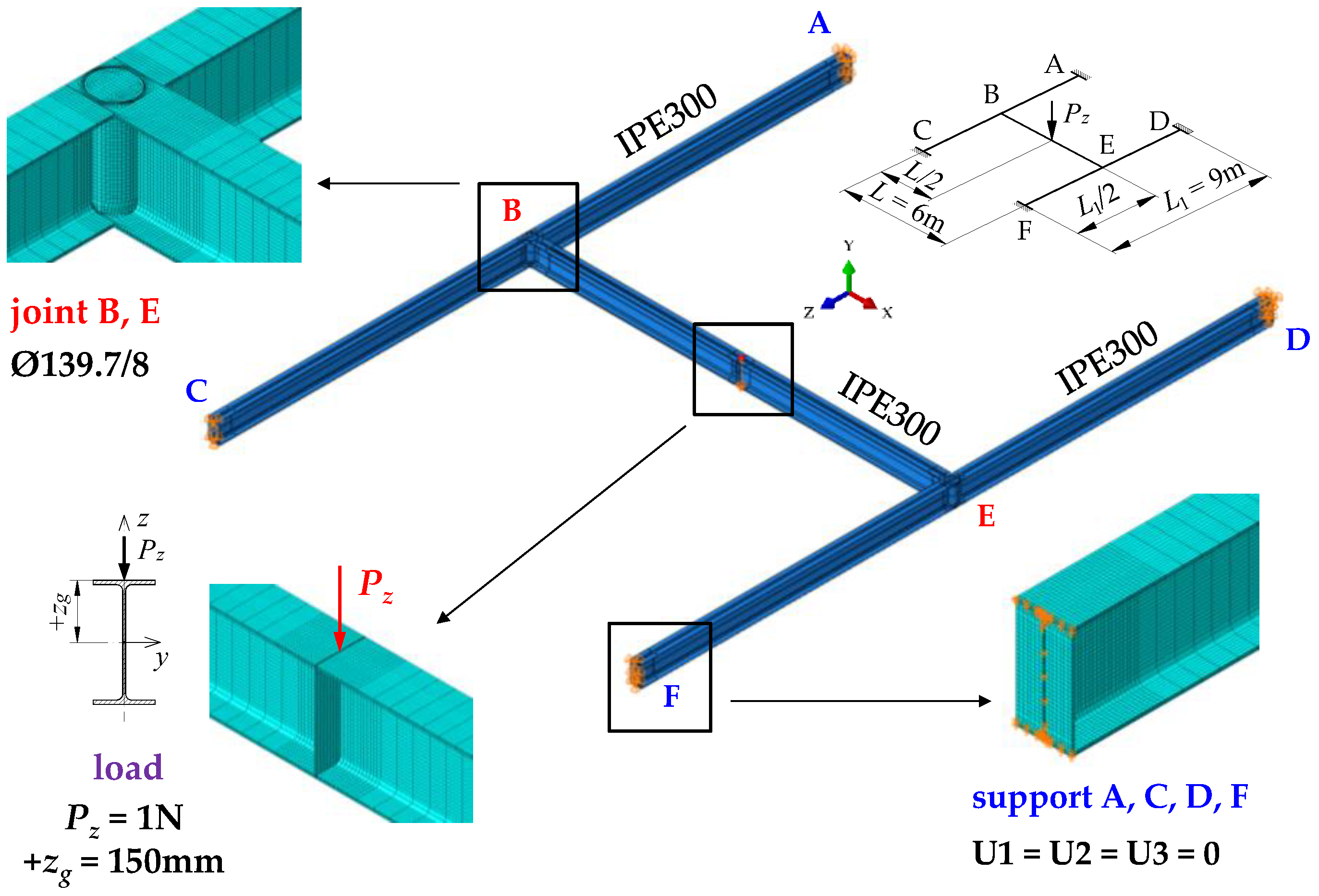
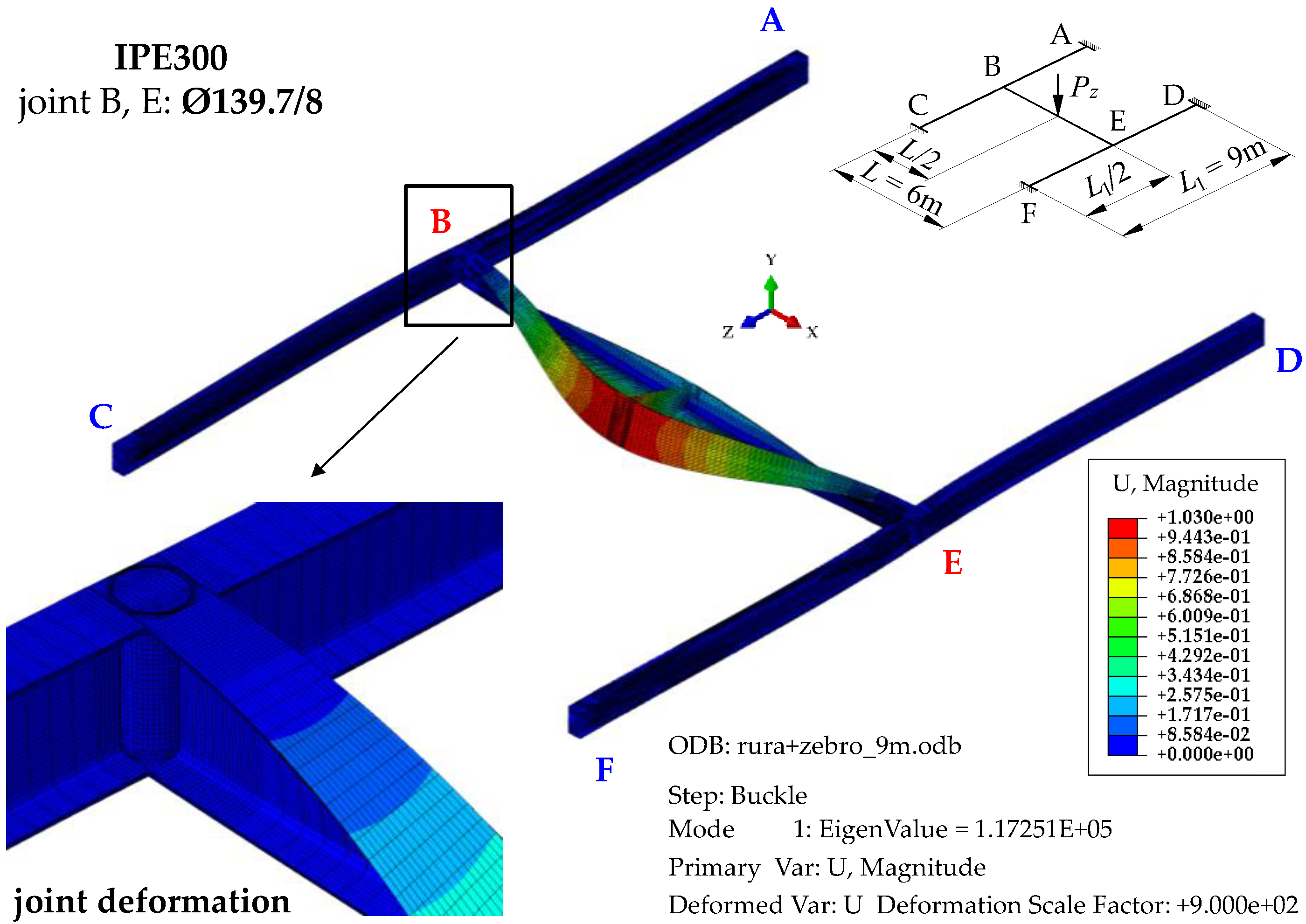
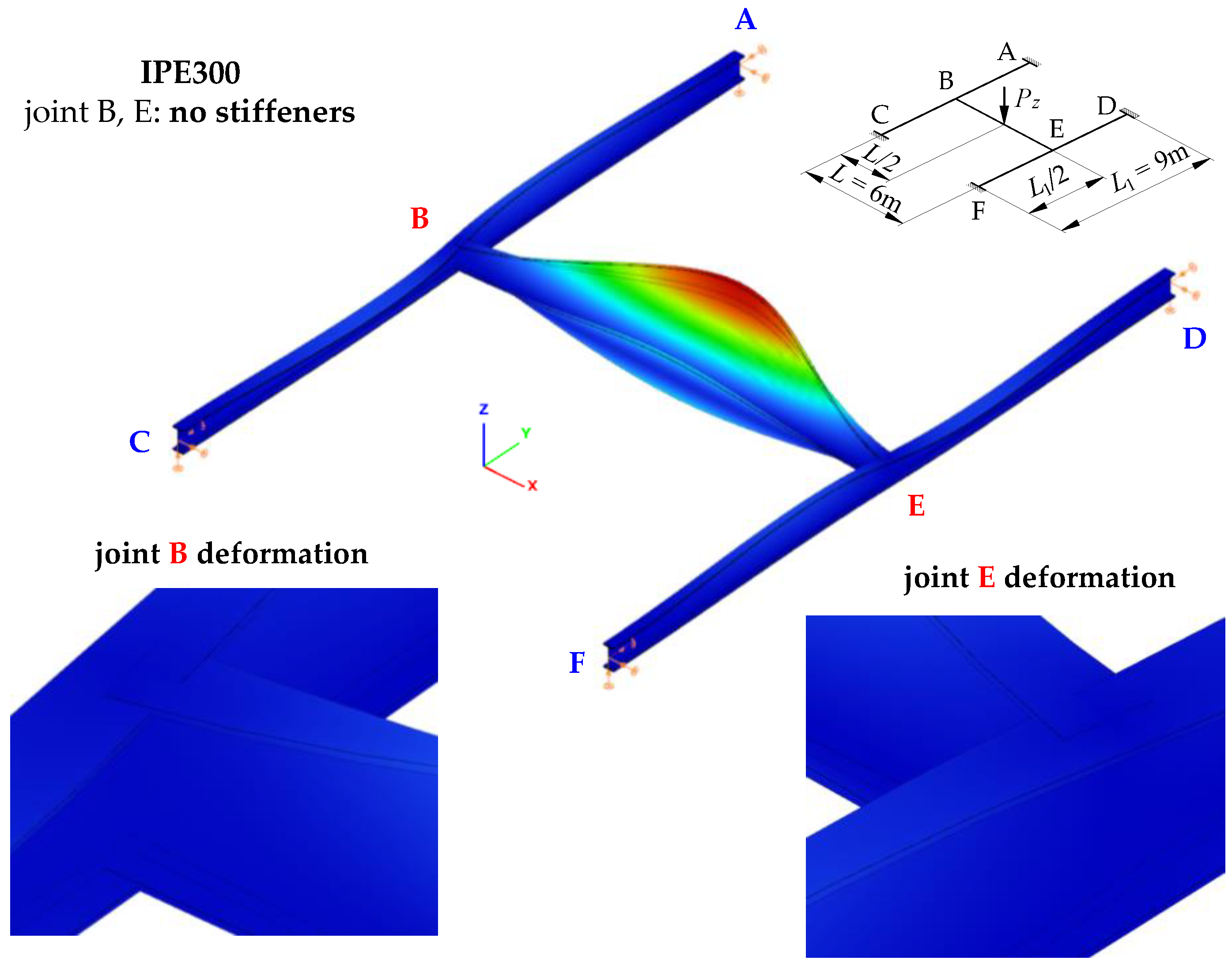
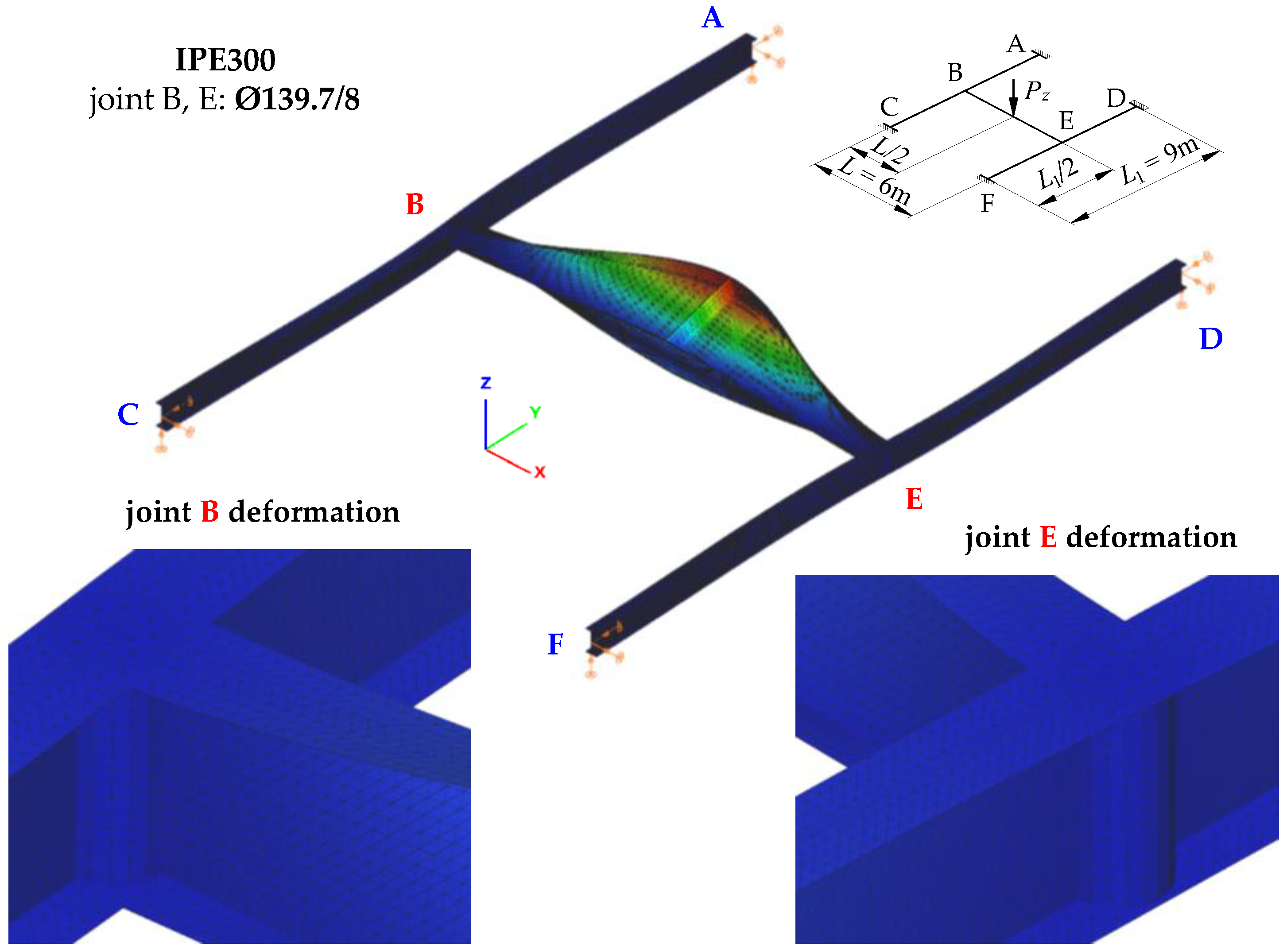
2. Diagram of the Analysed Structure
3. FEM Simulations in Abaqus Software (Method 1)
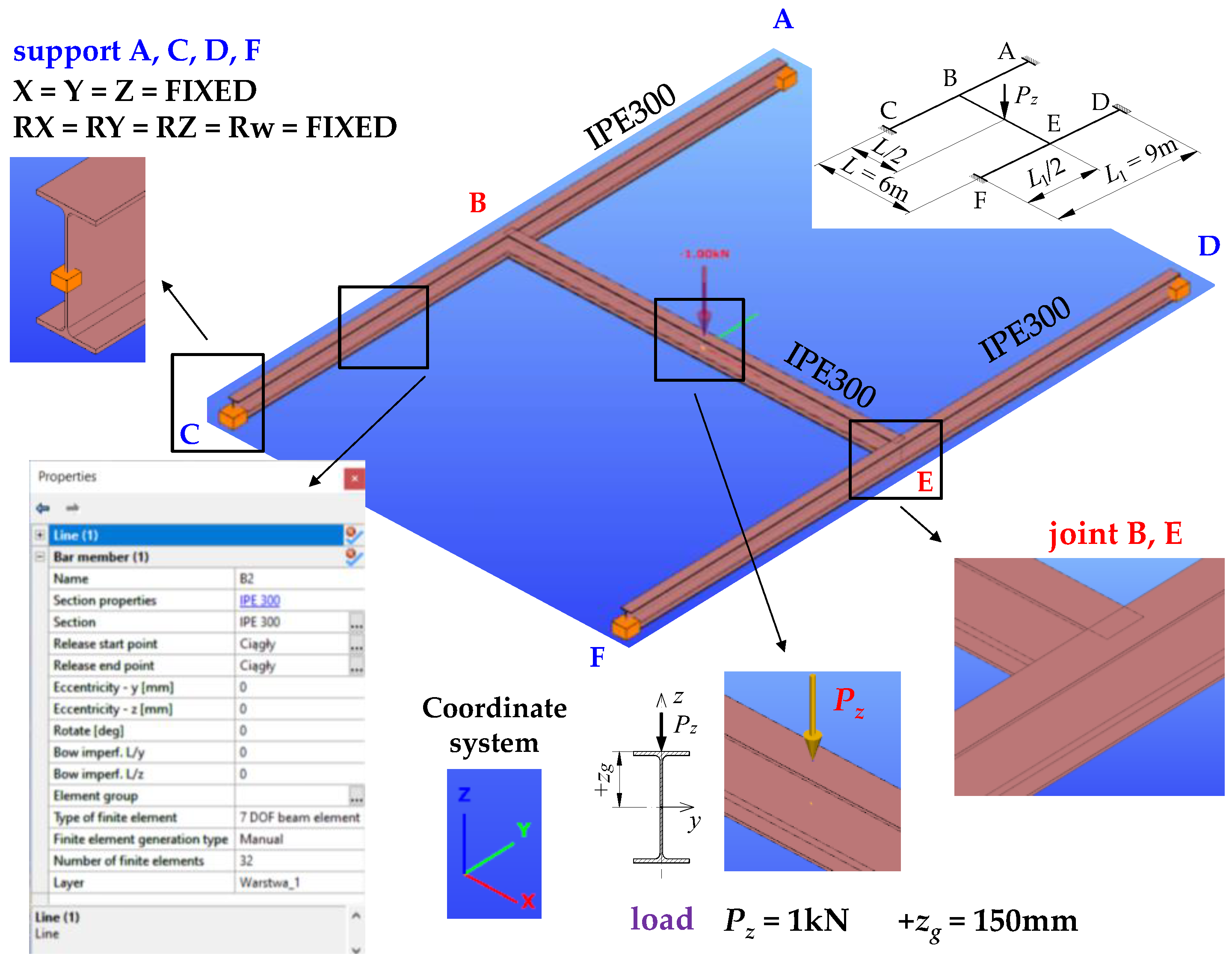
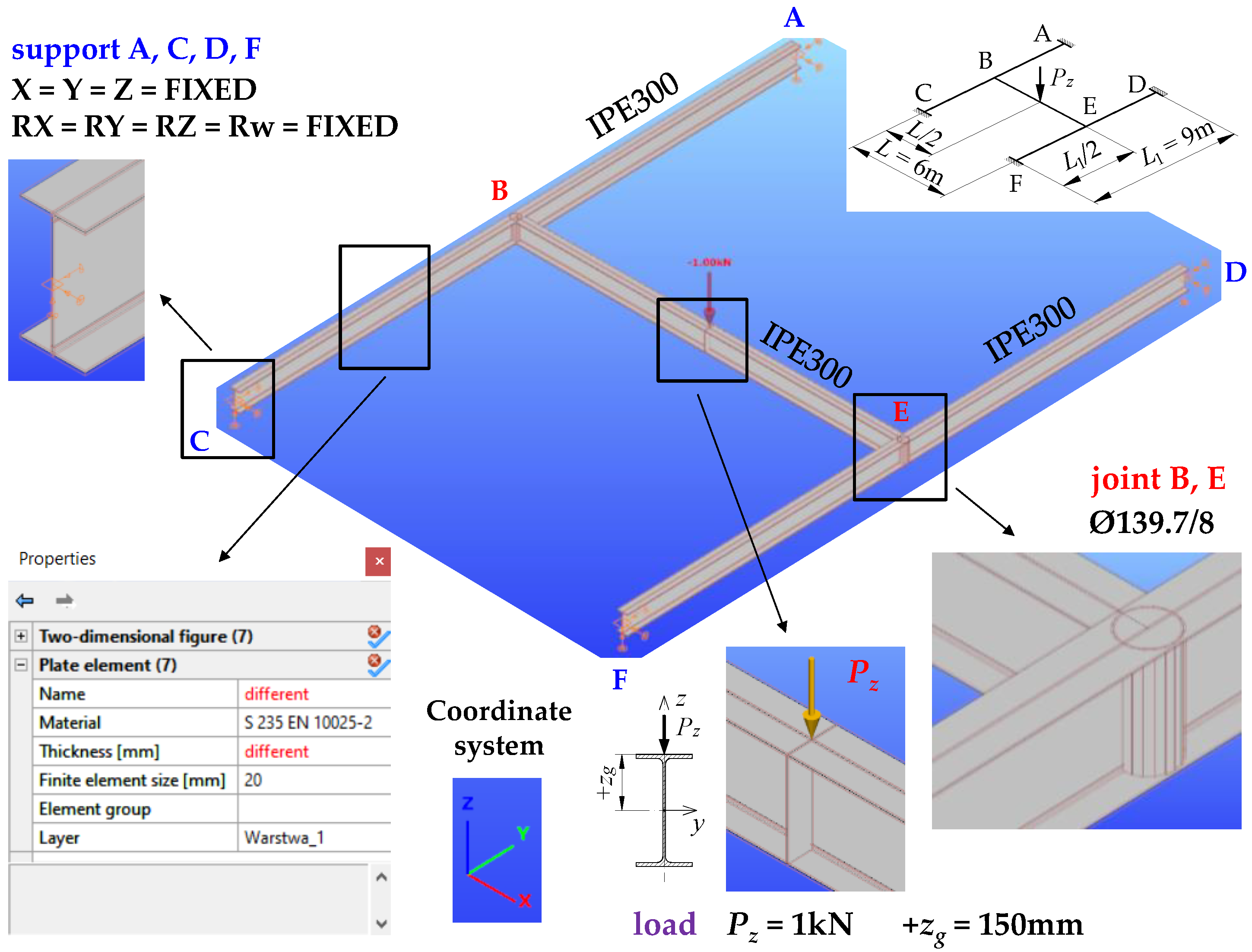
4. FEM Simulations in ConSteel Software (Method 2)
4.1. The Bar Model
4.2. The Shell Model
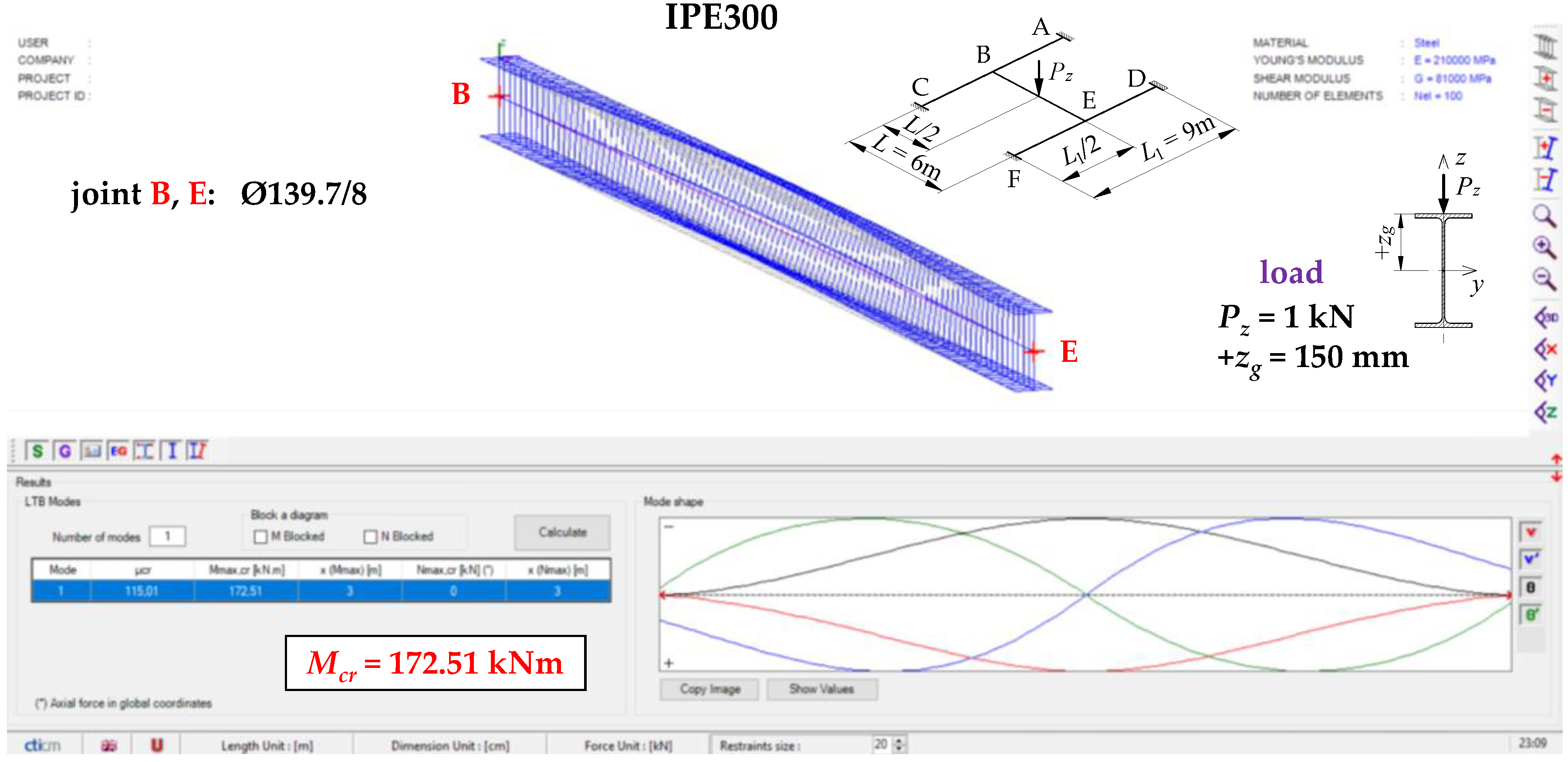
5. The Model of Designated Critical Beam (Method 3)
5.1. Conditions of Elastic Restraint
5.2. The Critical Moment of the Lateral Torsional Buckling of the H-Grillage Main Beam
5.2.1. The Analytical Model—The Energy Method
5.2.2. Analytical Model—Approximation Formula
5.2.3. Thin-Walled Bar FEM Model—LTBeam, LTBemN
6. Selected Results of Computations
6.1. Method 1—Volumetric Model—Abaqus
6.2. Method 2—ConSteel
6.2.1. The Bar Model (Thin-Walled Bar Elements)
6.2.2. The Shell Model
6.3. Method 3—Designated Critical Beam
6.3.1. Analytical Models
6.3.2. The Bar Model (FEM)—Thin-Walled Elements (LTBeamN)
7. Comparative Analysis
8. Summary and Conclusions
- (1)
- When the interaction of beams in the H-grillage joints is taken into consideration in the computational model, it is possible to provide a more accurate representation of the actual performance of this structure class under load. Consequently, a more precise determination of the ECR is available, compared with estimation based on the fork support, which is most common in engineering design. It is obvious that when taking into account the effect of elastic restraint at joints, engineers must be cautious and check the actual stiffness of the joints.
- (2)
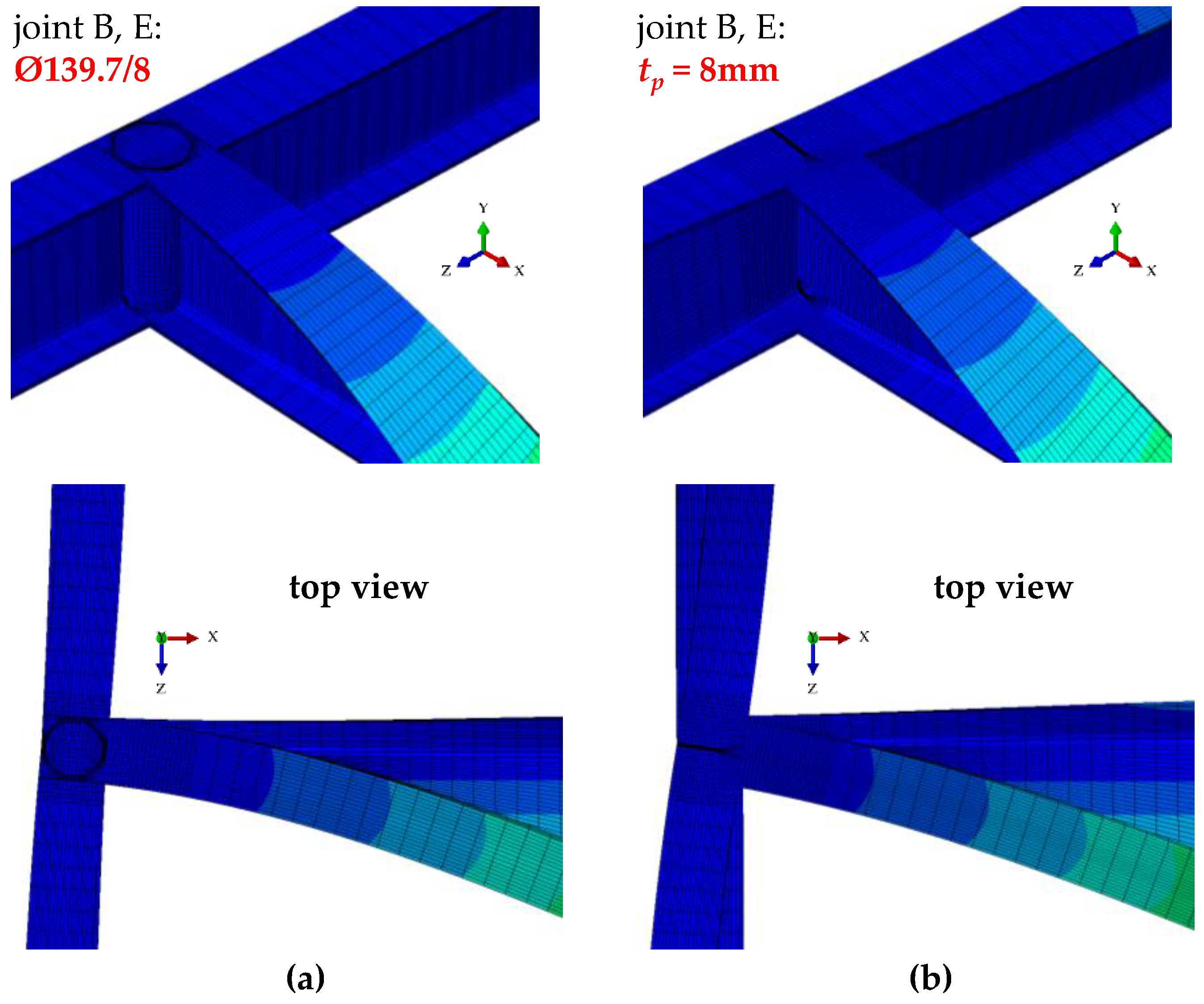
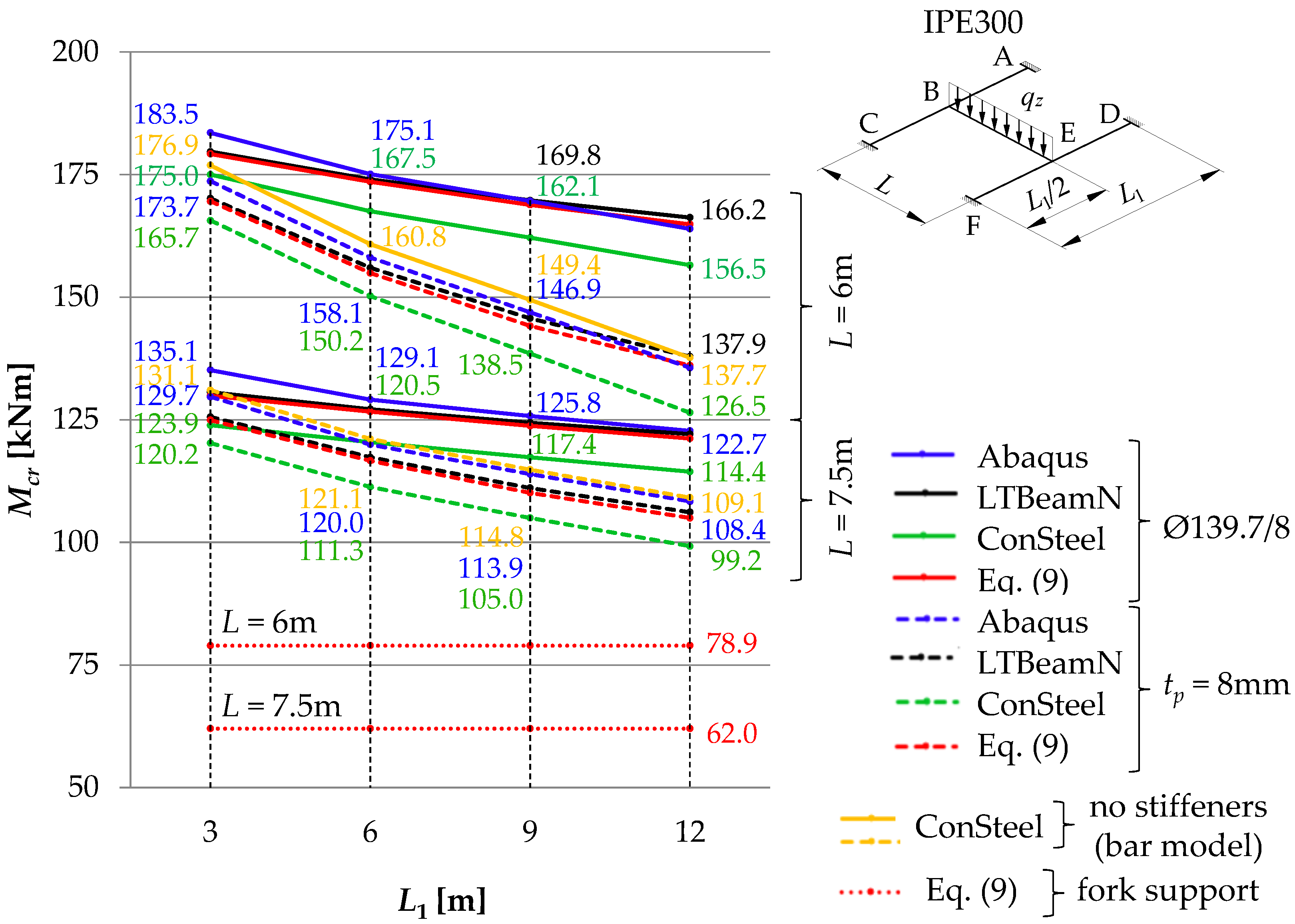
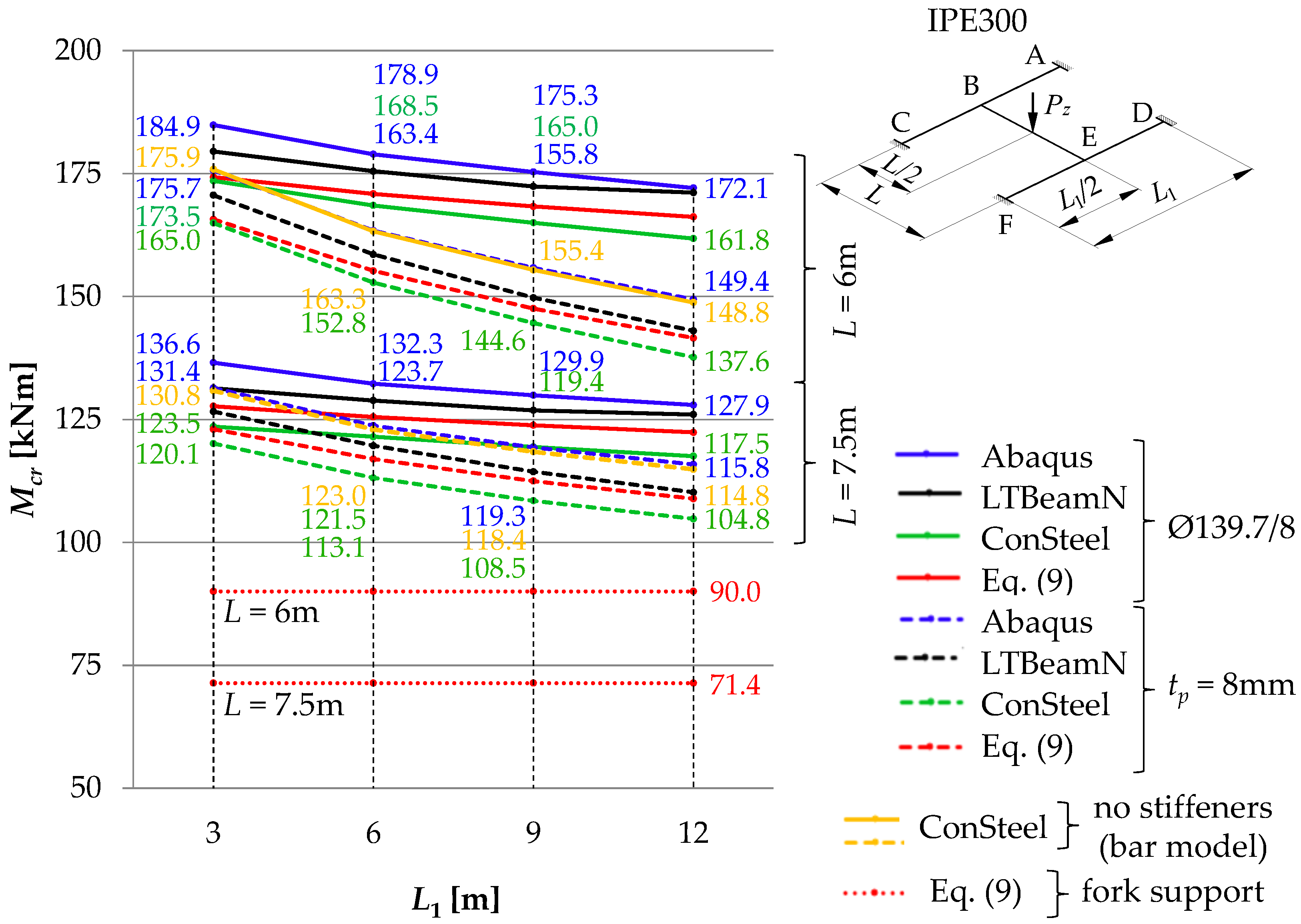
- As the span L1 of the supporting beams increases, the Mcr of the critical beam decreases. The greatest fall in Mcr, related to the increase in the span of the supporting beams, was obtained for flat stiffeners (dashed lines in Figure 11, Figure 12 and Figure 13), especially for the main beam span L = 6 m. In this case, closed-section stiffeners should be used to increase Mcr.
- (3)
- The warping stiffness αω of the welded grillage joints depends on their stiffening. The use of closed-section stiffeners considerably increases the degree of section fixity against warping. It also restricts the transmission of the bimoment between the joint component beams and enhances the grillage ECR. The use of classic flat stiffeners, however, practically does not increase Mcr. In the analysed H-type grillage of concern, the use of closed-section stiffeners resulted in an increase in the ECR from 3.9% (L = 7.5 m, L1 = 3 m) to 15.2% (L = 6 m, L1 = 12 m) according to Abaqus (Table 6, Column VI), and also from 2.9% (L = 7.5 m, L1 = 3 m) to 17.6% (L = 6 m, L1 = 12 m) according to ConSteel—shell model (Table 6, Column VIII).
- (4)
- The percentage increase in the ECR in the grillage with closed-section stiffeners (relative to grillage with flat stiffeners) is inversely proportional to the increase in the span L1 of supporting beams. Enhanced stiffness of the joints mitigates the decrease in Mcr associated with a diminished stiffness of the supporting beams in the grillage plane. The greatest efficiency in the mitigation of the Mcr drop due to the use of closed-section stiffeners was obtained for the span L = 6 m of the main beam and L1 = 12 m of the supporting beams (Figure 11, Figure 12 and Figure 13). The contribution of closed-section stiffeners increases with a decrease in the main beam span L.
- (5)
- The means of determining the stiffness of the elastic action of the grillage joints reported in this paper, and the approximation Formula (9) proposed in [11], which at the same time takes into account the effect of elastic restraint against warping and against the lateral rotation of the grillage’s weakest (critical) beam, make it possible to produce a very good estimation of Mcr compared with computations based on advanced FEM models (Abaqus, ConSteel). Determined in this way, Mcr allows for an estimation of grillage ECR. Additionally, Mcr can also be successfully applied to verify the FEM results.
- (6)
- In the case of significant strength utilisation in the supporting (stiffening) beams relative to their critical buckling resistance (Mcr,u), the reduction in the stiffness restraint of the critical beam can be conservatively estimated from Formulas (7) and (8). A more detailed approach to this issue will be the subject of further research and will be presented by the authors in another study.
- (7)
- The results obtained from the FEM numerical simulations (Abaqus—solid model, ConSteel—shell model) show (Figure 11, Figure 12 and Figure 13) that the grillage ECR is conditioned by the lateral torsion buckling of the B–E critical beam. The critical resistance is nonlinearly dependent on the grillage geometry and the method of stiffening of welded intermediate joints B and E. The use of closed-section stiffeners resulted in ECR increasing from a few to over 21% compared with flat stiffeners (Table 6).
- (8)
- From an engineering point of view, the comparison of results obtained with: Formula (9) [11], MLTB,EL,2 algorithm, and Abaqus (FEM) indicates their very good congruity (Table 7 and Table 8, Figure 11, Figure 12 and Figure 13). The LTB critical moments were obtained for (1) different variants of the coupling beam span (L) and supporting beams (L1), (2) different variants of nodal stiffening, and (3) different loading diagrams of the coupling beam.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
- (a)
- Variant 1—acc. Formulas (3), (4): αω = 47.00 kNm3/rad, αu = 2254.93 kNm
- (b)
- Variant 2—acc. Formulas (4), (5), (6): αω = 384.41 kNm3/rad, αu = 2254.93 kNm
- (a)
- Variant 1: κω = 0.842, κu = 0.842, κω* = 0.828, κu* = 0.828
- (b)
- Variant 2: κω = 0.978, κu = 0.842, κω* = 0.975, κu* = 0.828
- (a)
- Variant 1: Mcr(1) = 147.54 kNm, Mcr(1)* = 145.47 kNm (−1.4%)
- (b)
- Variant 2: Mcr(2) = 168.41 kNm, Mcr(2)* = 167.07 kNm (−0.8%)
- (a)
- Variant 1: λLT(1) = 1.158, αLT(1) = 0.21, ФLT(1) = 1.271, χLT(1) = 0.557
- (b)
- Variant 2: λLT(2) = 1.084, αLT(2) = 0.21, ФLT(2) = 1.180, χLT(2) = 0.607
- (a)
- Variant 1: Mb,Rd(1) = 124.55 kNm, Mb,Rd(1)* = 123.43 kNm (−0.9%)
- (b)
- Variant 2: Mb,Rd(2) = 135.73 kNm, Mb,Rd(2)* = 135.05 kNm (−0.5%)
- (a)
- Variant 1: MEd/Mb,Rd(1) = 0.843 < 1.0 (load capacity fulfilled)
- (b)
- Variant 2: MEd/Mb,Rd(2) = 0.774 < 1.0 (load capacity fulfilled)
- (1)
- Taking into account the conditions of elastic restraint of the main (coupling) beam in the supporting beams allowed for a more economical assessment of its design lateral buckling resistance and fulfilment of the load capacity condition according to EN 1993-1-1 [1].
- (2)
- The use of closed-section stiffeners with at joints B and E of the H-grillage, compared to the use of flat stiffeners, resulted in an increase in Mcr by 14% and an increase in the design load capacity Mb,Rd by 9%.
- (3)
- Analytical consideration of the spatial interaction of the grillage beams made it possible to maintain the resistance condition of the main (coupling) beam B–E without the need to increase its cross-section.
- (4)
- Due to the significant difference between Mcr for the critical (main) beam in relation to Mcr,u for the supporting (stiffening) beams, the reduction in the design resistance was only −0.9% (variant 1) and −0.5% (variant 2). In the case of greater strength utilisation of the supporting beams, the degree of reduction of joint stiffness can be conservatively determined from Formulas (7) and (8).
- (5)
- In computations, the use of the fork support model for the main (coupling) beam design resulted in a conservative assessment of the beam resistance (Mb,Rd(3)). Due to the simple analytical model, such a simplification is often found in engineering practice. This is a safe estimate, giving a lower determination of the lateral buckling resistance of the beam. However, as already mentioned, due to the spatial interaction of the component beams and the stiffeners of the joints of H-grillage, especially closed-section stiffeners, use of the fork support model for the weakest (critical) beam is not an economical solution. In the computational example, adopting such a method of supporting the main beam resulted in failure to meet the design condition according to EN 1993-1-1 [1]. In order to satisfy the condition above, it would be necessary to increase the beam cross-section to at least IPE360.
References
- EN 1993-1-1; Eurocode 3: Design of Steel Structures—Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2005.
- EN 1993-1-8; Eurocode 3: Design of Steel Structures—Part 1-8: Design of joints. European Committee for Standardization: Brussels, Belgium, 2005.
- Koczubiej, S.; Cichoń, C. Global static and stability analysis of thin-walled structures with open cross-section using FE shell-beam models. Thin-Walled Struct. 2014, 82, 196–211. [Google Scholar] [CrossRef]
- Shayan, S.; Rasmussen, K. A model for warping transmission through joints of steel frames. Thin-Walled Struct. 2014, 82, 1–12. [Google Scholar] [CrossRef]
- Kujawa, M. Static and sensitivity analysis of grids made of thin-walled members. In Theoretical Analysis and Experimental Research, 1st ed.; Gdansk University of Technology Publishers: Gdansk, Poland, 2009. (In Polish) [Google Scholar]
- Szymczak, C.; Kreja, I.; Mikulski, T.; Kujawa, M. Sensitivity Analysis of Beams and Frames Made of Thin-Walled Members; Gdansk University of Technology Publishers: Gdansk, Poland, 2003. [Google Scholar]
- Vaszilievits-Sömjén, B.; Szalai, J. Simple Superelement Model of Warping Transfer in Moment Connections Between I Sections. In Proceedings of the Ninth International Conference on Advances in Steel Structures, Hong Kong, China, 5–7 December 2018. [Google Scholar] [CrossRef]
- Vaszilievits-Sömjén, B.; Szalai, J.; Rad, M.M. Validation of simple superelement model of warping transfer in moment connections of portal frames. Ce/Pap. Online Collect. Conf. Pap. Civ. Eng. 2019, 3, 373–378. [Google Scholar] [CrossRef]
- Szychowski, A. Computation of Thin-Walled Cross-Section Resistance to Local Buckling with the Use of the Critical Plate Method. Arch. Civ. Eng. 2016, 62, 229–264. [Google Scholar] [CrossRef]
- Giżejowski, M. Lateral buckling of steel beams with limited rotation ability at supports. Inżynieria I Bud. 2001, 10, 589–594. (In Polish) [Google Scholar]
- Piotrowski, R.; Szychowski, A. Lateral Torsional Buckling of Steel Beams Elastically Restrained at the Support Nodes. Appl. Sci. 2019, 9, 1944. [Google Scholar] [CrossRef]
- Amara, S.; Kerdal, D.E.; Jaspart, J.P. Effect of end connection restraints on the stability of steel beams in bending. Adv. Steel Constr. 2008, 4, 243–259. [Google Scholar]
- Piotrowski, R.; Szychowski, A. The Effect of Steel Beam Elastic Restraint on the Critical Moment of Lateral Torsional Buckling. Materials 2022, 15, 1275. [Google Scholar] [CrossRef]
- Lee, H.E.; Nguyen, C.T.; Moon, J.H.; Joo, H.S. Lateral-torsional buckling of discretely-braced i-girder. Procedia Eng. 2011, 14, 264–271. [Google Scholar] [CrossRef]
- Nguyen, C.T.; Moon, J.; Le, V.N.; Lee, H.E. Lateral–torsional buckling of I-girders with discrete torsional bracings. J. Constr. Steel Res. 2010, 66, 170–177. [Google Scholar] [CrossRef]
- Valentino, J.; Trahair, N.S. Torsional Restraint against Elastic Lateral Buckling. J. Struct. Eng. 1998, 124, 1217–1225. [Google Scholar] [CrossRef]
- Stroetmann, R. Lateral torsional and distortional buckling of cross-connected beams. In Proceedings of the Eighth International Conference on Advances in Steel Structures, Lisbon, Portugal, 21–24 July 2015. [Google Scholar]
- Piotrowski, R.; Siedlecka, M. Point Protection of Primary Beams of Steel Grillages Against Lateral Torsional Buckling. Adv. Sci. Technol. Res. J. 2020, 14, 1–8. [Google Scholar] [CrossRef]
- Yura, J. Fundamentals of beam bracing. Eng. J. 2001, 38, 11–26. [Google Scholar]
- Gosowski, B. Non-uniform torsion of stiffened open thin-walled members of steel structures. J. Constr. Steel Res. 2007, 63, 849–865. [Google Scholar] [CrossRef]
- Gosowski, B.; Redecki, M. Lateral-torsional buckling moments for I-beams with local lateral restraints. Inżynieria I Bud. 2012, 68, 140–144. (In Polish) [Google Scholar]
- Park, Y.-M.; Hwang, S.-Y.; Hwang, M.-O.; Choi, B.H. Inelastic buckling of torsionally braced I-girders under uniform bending: I. Numerical parametric studies. J. Constr. Steel Res. 2010, 66, 304–316. [Google Scholar] [CrossRef]
- Gull, J.H.; Azizinamini, A.; Helwig, T.A. Comparison of detailing methods for straight skewed steel I-girder bridges. J. Constr. Steel Res. 2017, 136, 24–34. [Google Scholar] [CrossRef]
- Yura, J.; Helwig, T.; Herman, R.; Zhou, C. Global Lateral Buckling of I-Shaped Girder Systems. J. Struct. Eng. 2008, 134, 1487–1494. [Google Scholar] [CrossRef]
- Zhao, Q.; Yu, B.; Burdette, E.G. Effects of Cross-Frame on Stability of Double I-Girder System under Erection. Transp. Res. Board 2010, 2152, 57–62. [Google Scholar] [CrossRef]
- Adamakos, T.; Vayas, I.; Petridis, S.; Iliopoulos, A. Modeling of curved composite I-girder bridges using spatial systems of beam elements. J. Constr. Steel Res. 2011, 67, 462–470. [Google Scholar] [CrossRef]
- Gocál, J.; Odrobiňák, J. On the Influence of Corrosion on the Load-Carrying Capacity of Old Riveted Bridges. Materials 2020, 13, 717. [Google Scholar] [CrossRef]
- Rageh, A.; Sun, C.; Linzell, D.G.; Puckett, J.A. Dataset for large-scale, lateral-torsional buckling tests of continuous beams in a grillage system. Data Brief 2022, 44, 108532. [Google Scholar] [CrossRef]
- Sun, C.; Linzell, D.G.; Puckett, J.A.; Akintunde, E.; Rageh, A. Experimental Study of Continuous-Beam Lateral Torsional-Buckling Resistance with a Noncomposite Concrete Deck. J. Struct. Eng. 2022, 148, 04022026. [Google Scholar] [CrossRef]
- Kong, X.; Zhou, H.; Zheng, C.; Pei, Z.; Yuan, T.; Wu, W. Research on the dynamic buckling of a typical deck grillage structure subjected to in-plane impact Load. Mar. Struct. 2021, 78, 103003. [Google Scholar] [CrossRef]
- Piotrowski, R.; Szychowski, A. Impact of support closed section ribs on the critical moment for lateral torsional buckling of steel beams. Struct. Environ. 2018, 10, 5–18. [Google Scholar] [CrossRef]
- Pi, Y.-L.; Trahair, N.S. Distortion and warping at beam supports. J. Struct. Eng. 2000, 126, 1279–1287. [Google Scholar] [CrossRef]
- Piotrowski, R.; Szychowski, A. Critical resistance of a beam grillage in FEM simulations and analytical calculations. In Proceedings of the International Conference Selected Issues in Building Structures Design, Kielce—Cedzyna, Poland, 14–15 July 2022. [Google Scholar]
- Abaqus 6.12. In Abaqus/CAE User’s Manual; PDF Documentation; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2012; Available online: http://130.149.89.49:2080/v6.12/pdf_books/CAE.pdf (accessed on 1 March 2022).
- ConSteel User Manuals. Available online: https://consteelsoftware.com/downloads/ (accessed on 1 March 2022).
- Vlasov, V.Z. Thin-Walled Elastic Beams, 2nd ed.; Israel Program for Scientific Translations: Jerusalem, Israel, 1961. [Google Scholar]
- Cross, H. Analysis of Continuous Frames By Distributing Fixed-End Moments. Am. Soc. Civ. Eng. 1932, 96, 1–10. [Google Scholar] [CrossRef]
- Galéa, Y. Moment critique de déversement élastique de poutres fléchies. Présentation du logiciel LTBEAM. Rev. Constr. Métallique. CTICM 2003, 40, 47–76. [Google Scholar]



| Item | L [m] | L1 [m] | zg [mm] | qz,cr [kN/m] Acc. Abaqus | % [-] VI-V | |
|---|---|---|---|---|---|---|
| Stiffener tp = 8 mm | Stiffener Ø139.7/8 | |||||
| I | II | III | IV | V | VI | VII |
| 1 | 6 | 3 | 150 | 38.6 | 40.8 | 5.7 |
| 2 | 6 | 35.1 | 38.9 | 10.8 | ||
| 3 | 9 | 32.6 | 37.7 | 15.6 | ||
| 4 | 12 | 30.1 | 36.4 | 20.9 | ||
| 5 | 7.5 | 3 | 18.4 | 19.2 | 4.3 | |
| 6 | 6 | 17.1 | 18.4 | 7.6 | ||
| 7 | 9 | 16.2 | 17.9 | 10.5 | ||
| 8 | 12 | 15.4 | 17.4 | 13.0 | ||
| Item | L [m] | L1 [m] | zg [mm] | qz,cr [kN/m] Acc. ConSteel —Bar Model |
|---|---|---|---|---|
| No Stiffener | ||||
| I | II | III | IV | V |
| 1 | 6 | 3 | 150 | 39.31 |
| 2 | 6 | 35.73 | ||
| 3 | 9 | 33.21 | ||
| 4 | 12 | 30.59 | ||
| 5 | 7.5 | 3 | 18.64 | |
| 6 | 6 | 17.22 | ||
| 7 | 9 | 16.33 | ||
| 8 | 12 | 15.52 |
| Item | L [m] | L1 [m] | zg [mm] | qz,cr [kN/m] Acc. ConSteel - Shell Model | % [-] VI-V | |
|---|---|---|---|---|---|---|
| Stiffener tp = 8 mm | Stiffener Ø139.7/8 | |||||
| I | II | III | IV | V | VI | VII |
| 1 | 6 | 3 | 150 | 36.81 | 38.89 | 5.7 |
| 2 | 6 | 33.38 | 37.23 | 11.5 | ||
| 3 | 9 | 30.78 | 36.03 | 17.1 | ||
| 4 | 12 | 28.10 | 34.78 | 23.8 | ||
| 5 | 7.5 | 3 | 17.10 | 17.62 | 3.0 | |
| 6 | 6 | 15.83 | 17.13 | 8.2 | ||
| 7 | 9 | 14.93 | 16.69 | 11.8 | ||
| 8 | 12 | 14.11 | 16.27 | 15.3 | ||
| Item | L [m] | L1 [m] | zg [mm] | Mcr [kNm] Acc. Analytical Method | % [-] VIII-VI | |||
|---|---|---|---|---|---|---|---|---|
| Stiffener tp = 8 mm | Stiffener Ø139.7/8 | |||||||
| MLTB,EL,2 | Equation (9) [11] | MLTB,EL,2 | Equation (9) [11] | |||||
| I | II | III | IV | V | VI | VII | VIII | IX |
| 1 | 6 | 3 | 150 | 170.5 | 169.6 | 180.2 | 179.2 | 5.7 |
| 2 | 6 | 156.3 | 154.9 | 174.5 | 173.5 | 12.0 | ||
| 3 | 9 | 145.9 | 144.2 | 170.1 | 168.8 | 17.1 | ||
| 4 | 12 | 138.0 | 136.1 | 166.5 | 164.9 | 21.2 | ||
| 5 | 7.5 | 3 | 125.9 | 124.9 | 131.0 | 129.9 | 4.0 | |
| 6 | 6 | 117.6 | 116.6 | 127.5 | 126.6 | 8.6 | ||
| 7 | 9 | 111.3 | 110.1 | 124.7 | 123.8 | 12.4 | ||
| 8 | 12 | 106.3 | 105.0 | 122.3 | 121.2 | 15.4 | ||
| Item | L [m] | L1 [m] | zg [mm] | Mcr [kNm] Acc. Bar Model | % [-] VI-V | |
|---|---|---|---|---|---|---|
| Stiffener tp = 8 mm | Stiffener Ø139.7/8 | |||||
| I | II | III | IV | V | VI | VII |
| 1 | 6 | 3 | 150 | 170.1 | 179.7 | 5.6 |
| 2 | 6 | 156.0 | 174.1 | 11.6 | ||
| 3 | 9 | 145.7 | 169.8 | 16.5 | ||
| 4 | 12 | 137.9 | 166.2 | 20.5 | ||
| 5 | 7.5 | 3 | 125.6 | 130.6 | 4.0 | |
| 6 | 6 | 117.4 | 127.2 | 8.3 | ||
| 7 | 9 | 111.1 | 124.4 | 12.0 | ||
| 8 | 12 | 106.2 | 122.1 | 15.0 | ||
| Item | Stiffener | L [m] | L1 [m] | zg [mm] | Pz,cr [kN] | % [-] | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Method 1 | Method 2 | VII-VI | VIII-VI | VIII-VII | ||||||
| Abaqus– Solid Model | ConSteel– Bar Model | ConSteel— Shell Model | ||||||||
| I | II | III | IV | V | VI | VII | VIII | IX | X | XI |
| 1 | tp = 8 mm | 6 | 3 | 150 | 117.11 | 117.25 | 109.97 | 0.1 | −6.1 | −6.2 |
| 2 | 6 | 108.93 | 108.84 | 101.88 | −0.1 | −6.5 | −6.4 | |||
| 3 | 9 | 103.89 | 103.60 | 96.41 | −0.3 | −7.2 | −6.9 | |||
| 4 | 12 | 99.61 | 99.19 | 91.76 | −0.4 | −7.9 | −7.5 | |||
| 5 | 7.5 | 3 | 70.09 | 69.78 | 64.05 | −0.4 | −8.6 | −8.2 | ||
| 6 | 6 | 65.98 | 65.59 | 60.32 | −0.6 | −8.6 | −8.0 | |||
| 7 | 9 | 63.64 | 63.16 | 57.84 | −0.8 | −9.1 | −8.4 | |||
| 8 | 12 | 61.78 | 61.25 | 55.88 | −0.9 | −9.6 | −8.8 | |||
| 9 | Ø139.7/8 | 6 | 3 | 150 | 123.25 | 117.25 | 115.69 | −4.9 | −6.1 | −1.3 |
| 10 | 6 | 119.29 | 108.84 | 112.35 | −8.8 | −5.8 | 3.2 | |||
| 11 | 9 | 116.89 | 103.60 | 110.00 | −11.4 | −5.9 | 6.2 | |||
| 12 | 12 | 114.73 | 99.19 | 107.87 | −13.5 | −6.0 | 8.8 | |||
| 13 | 7.5 | 3 | 72.83 | 69.78 | 65.89 | −4.2 | −9.5 | −5.6 | ||
| 14 | 6 | 70.53 | 65.59 | 64.79 | −7.0 | −8.1 | −1.2 | |||
| 15 | 9 | 69.29 | 63.16 | 63.68 | −8.8 | −8.1 | 0.8 | |||
| 16 | 12 | 68.23 | 61.25 | 62.69 | −10.2 | −8.1 | 2.4 | |||
| Item | Stiffener | L [m] | L1 [m] | zg [mm] | Mcr [kNm] | % [-] | Mcr [kNm] | % [-] | Mcr [kNm] | % [-] | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Abaqus | LTBeamN | VII-VI | MLTB,EL,2 | IX-VI | IX-VII | Equation (9) [11] | XII-VI | XII-VII | |||||
| I | II | III | IV | V | VI | VII | VIII | IX | X | XI | XII | XIII | XIV |
| 1 | tp * | 6 | 3 | 150 | 173.7 | 170.1 | −2.1 | 170.5 | −1.8 | 0.2 | 169.6 | −2.4 | −0.3 |
| 2 | 6 | 158.1 | 156.0 | −1.3 | 156.3 | −1.1 | 0.2 | 154.9 | −2.0 | −0.7 | |||
| 3 | 9 | 146.9 | 145.7 | −0.8 | 145.9 | −0.7 | 0.1 | 144.2 | −1.8 | −1.0 | |||
| 4 | 12 | 135.6 | 137.9 | 1.7 | 138.0 | 1.8 | 0.1 | 136.1 | 0.4 | −1.3 | |||
| 5 | 7.5 | 3 | 129.7 | 125.6 | −3.2 | 125.9 | −2.9 | 0.2 | 124.9 | −3.7 | −0.6 | ||
| 6 | 6 | 120.0 | 117.4 | −2.2 | 117.6 | −2.0 | 0.2 | 116.6 | −2.8 | −0.7 | |||
| 7 | 9 | 113.9 | 111.1 | −2.5 | 111.3 | −2.3 | 0.2 | 110.1 | −3.3 | −0.9 | |||
| 8 | 12 | 108.4 | 106.2 | −2.0 | 106.3 | −1.9 | 0.1 | 105.0 | −3.1 | −1.1 | |||
| 9 | Ø ** | 6 | 3 | 150 | 183.5 | 179.7 | −2.1 | 180.2 | −1.8 | 0.3 | 179.2 | −2.3 | −0.3 |
| 10 | 6 | 175.1 | 174.1 | −0.6 | 174.5 | −0.3 | 0.2 | 173.5 | −0.9 | −0.3 | |||
| 11 | 9 | 169.6 | 169.8 | 0.1 | 170.1 | 0.3 | 0.2 | 168.8 | −0.5 | −0.6 | |||
| 12 | 12 | 163.9 | 166.2 | 1.4 | 166.5 | 1.6 | 0.2 | 164.9 | 0.6 | −0.8 | |||
| 13 | 7.5 | 3 | 135.1 | 130.6 | −3.3 | 131.0 | −3.0 | 0.3 | 129.9 | −3.8 | −0.5 | ||
| 14 | 6 | 129.1 | 127.2 | −1.5 | 127.5 | −1.2 | 0.2 | 126.6 | −1.9 | −0.5 | |||
| 15 | 9 | 125.8 | 124.4 | −1.1 | 124.7 | −0.9 | 0.2 | 123.8 | −1.6 | −0.5 | |||
| 16 | 12 | 122.7 | 122.1 | −0.5 | 122.3 | −0.3 | 0.2 | 121.2 | −1.2 | −0.7 | |||
| Loading Diagram of the B–E Beam | zg [mm] | Stiffener | LTBeamN vs. Abaqus [%] | MLTB,EL,2 vs. Abaqus [%] | MLTB,EL,2 vs. LTBeamN [%] | Equation (9) [11] vs. Abaqus [%] | Equation (9) [11] vs. LTBeamN [%] |
|---|---|---|---|---|---|---|---|
| I | II | III | IV | V | VI | VII | VIII |
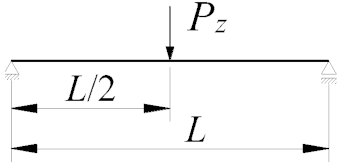 | 150 | tp = 8 mm | −4.8 ÷ −2.9 | −3.4 ÷ −1.1 | 1.4 ÷ 1.9 | −6.4 ÷ −5.0 | −2.9 ÷ −1.0 |
| Ø139.7/8 | −3.9 ÷ −0.6 | −1.9 ÷ 0.3 | 0.9 ÷ 2.0 | −6.5 ÷ −3.4 | −2.9 ÷ −2.4 | ||
 | 150 | tp = 8 mm | −3.2 ÷ 1.7 | −2.9 ÷ 1.8 | 0.1 ÷ 0.3 | −3.6 ÷ 0.3 | −1.3 ÷ −0.3 |
| Ø139.7/8 | −3.3 ÷ 1.4 | −3.0 ÷ 1.6 | 0.2 ÷ 0.3 | −3.9 ÷ 0.6 | −0.8 ÷ −0.3 | ||
 | 150 | tp = 8 mm | −3.0 ÷ 3.1 | −2.3 ÷ 3.6 | 0.6 ÷ 0.7 | −2.7 ÷ 2.6 | −0.5 ÷ 0.5 |
| Ø139.7/8 | −3.1 ÷ 2.0 | −2.5 ÷ 2.5 | 0.5 ÷ 0.7 | −2.9 ÷ 1.9 | 0.1 ÷ 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piotrowski, R.; Szychowski, A.; Vičan, J. Elastic Critical Resistance of the Simple Beam Grillage Resulting from the Lateral Torsional Buckling Condition: FEM Modelling and Analytical Considerations. Materials 2023, 16, 1346. https://doi.org/10.3390/ma16041346
Piotrowski R, Szychowski A, Vičan J. Elastic Critical Resistance of the Simple Beam Grillage Resulting from the Lateral Torsional Buckling Condition: FEM Modelling and Analytical Considerations. Materials. 2023; 16(4):1346. https://doi.org/10.3390/ma16041346
Chicago/Turabian StylePiotrowski, Rafał, Andrzej Szychowski, and Josef Vičan. 2023. "Elastic Critical Resistance of the Simple Beam Grillage Resulting from the Lateral Torsional Buckling Condition: FEM Modelling and Analytical Considerations" Materials 16, no. 4: 1346. https://doi.org/10.3390/ma16041346






