Graded and Anisotropic Porous Materials for Broadband and Angular Maximal Acoustic Absorption
Abstract
:1. Introduction
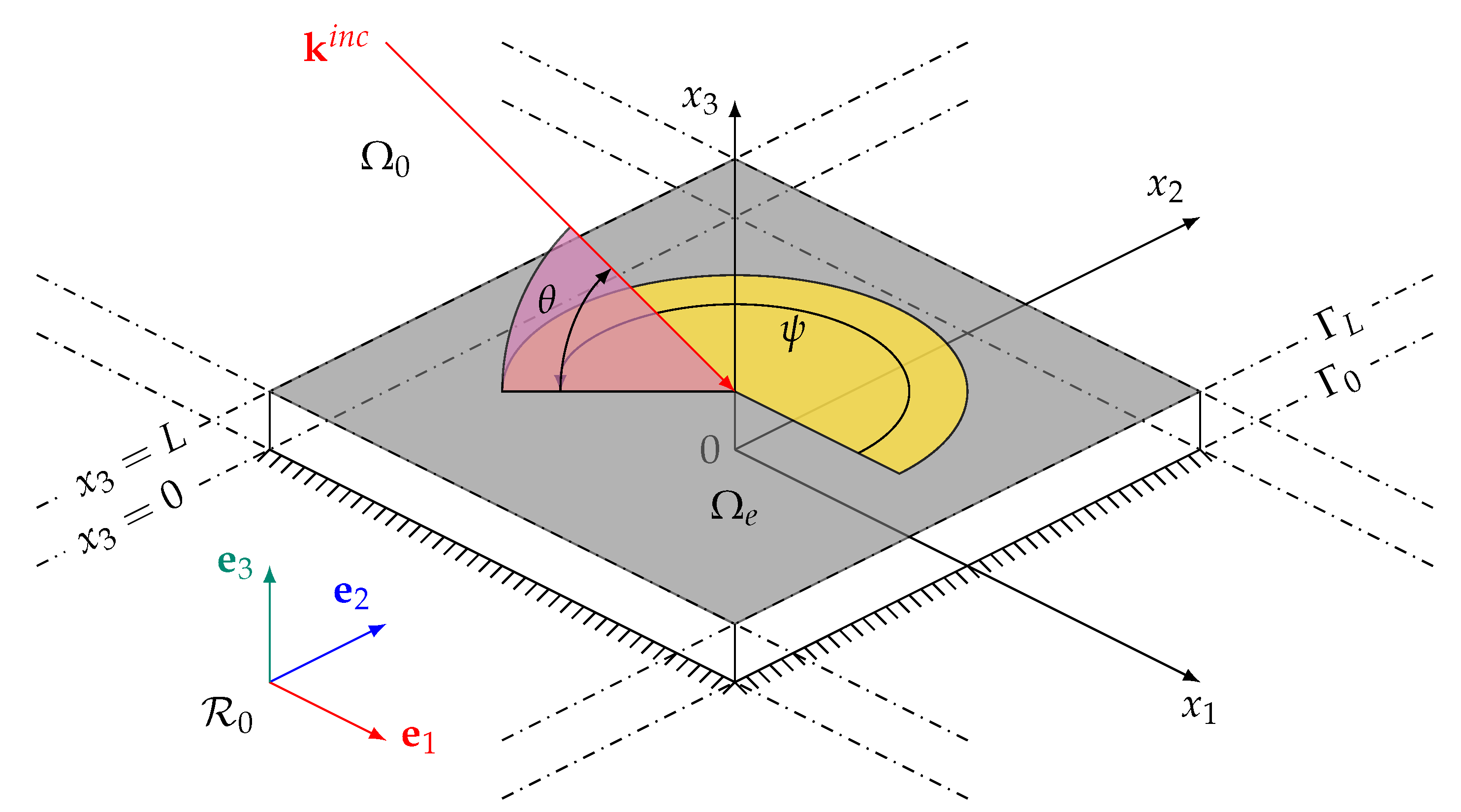
2. Reminder on Anisotropic and Graded Porous Materials
3. Average and Diffuse Field Absorptions
4. Optimization Procedures
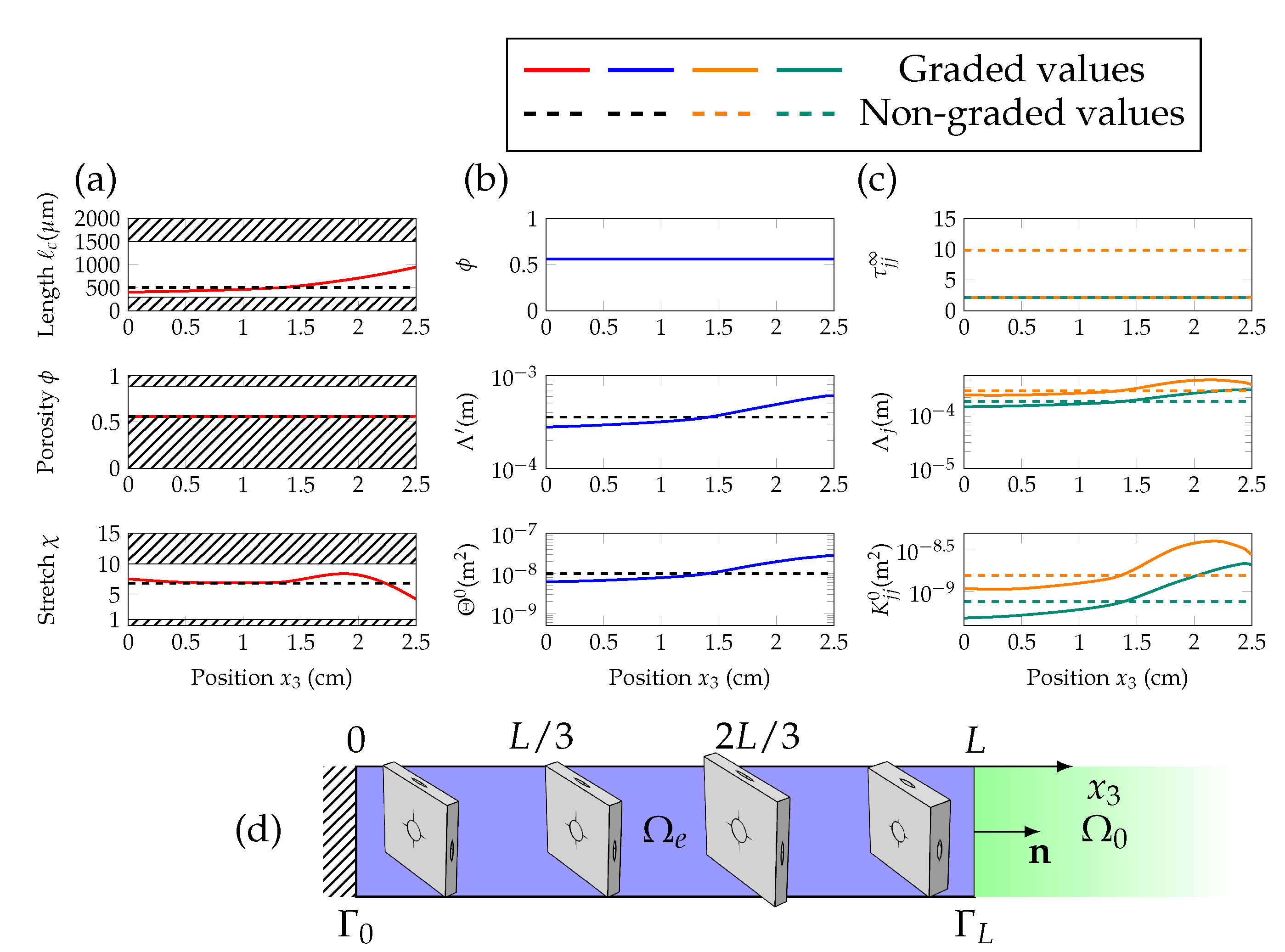
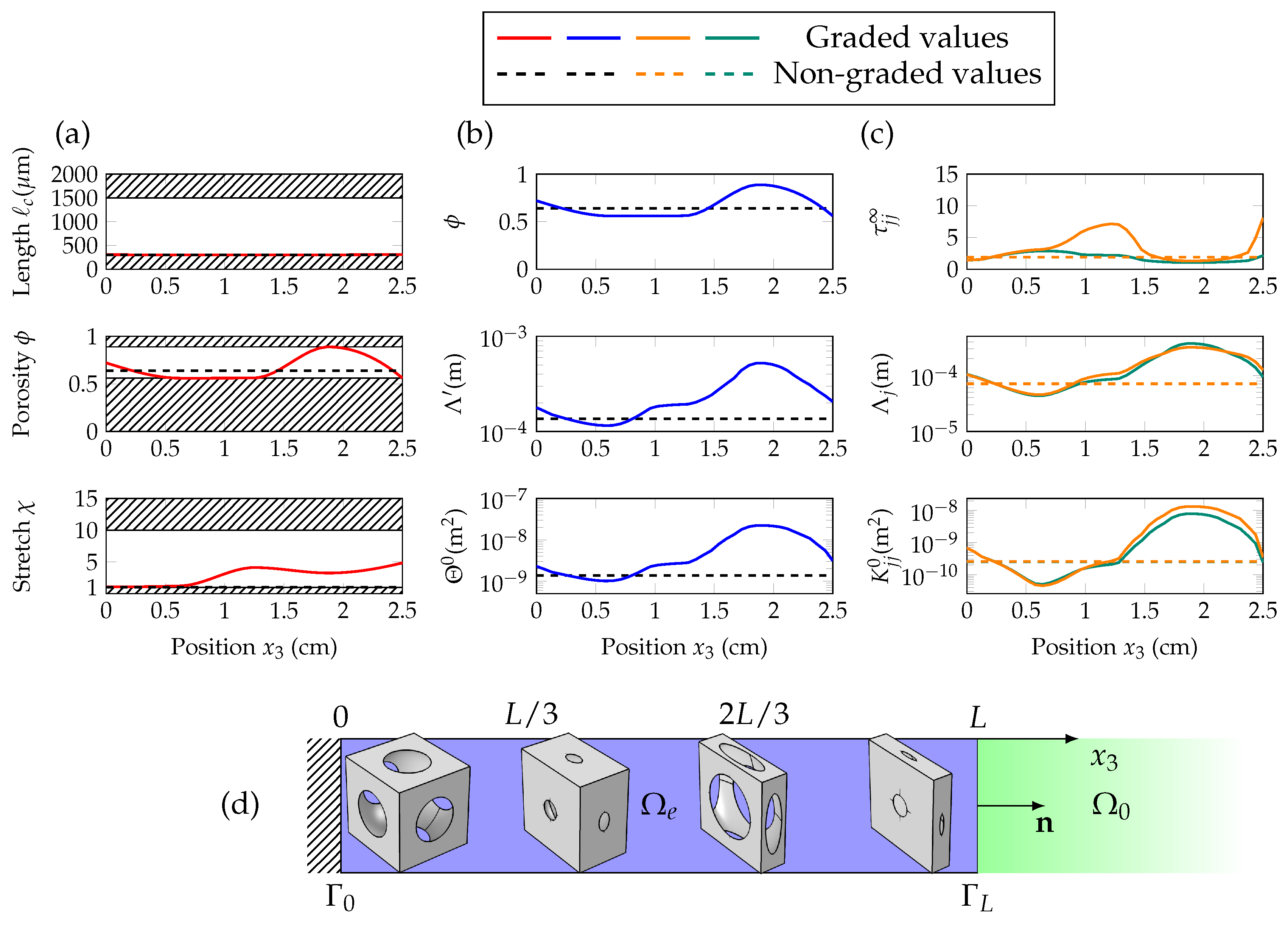
4.1. Interpolated Database of Unit Cells
4.2. Macro-Modulation of Effective Properties
4.3. Optimization of Geometric Properties
5. Results
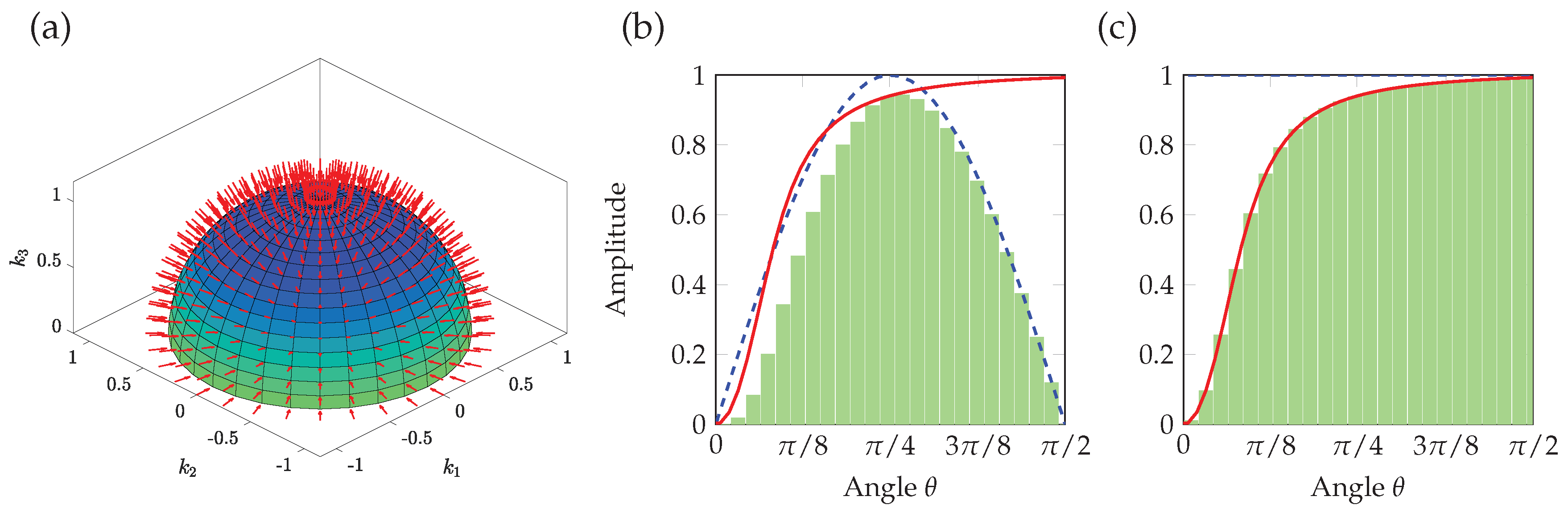
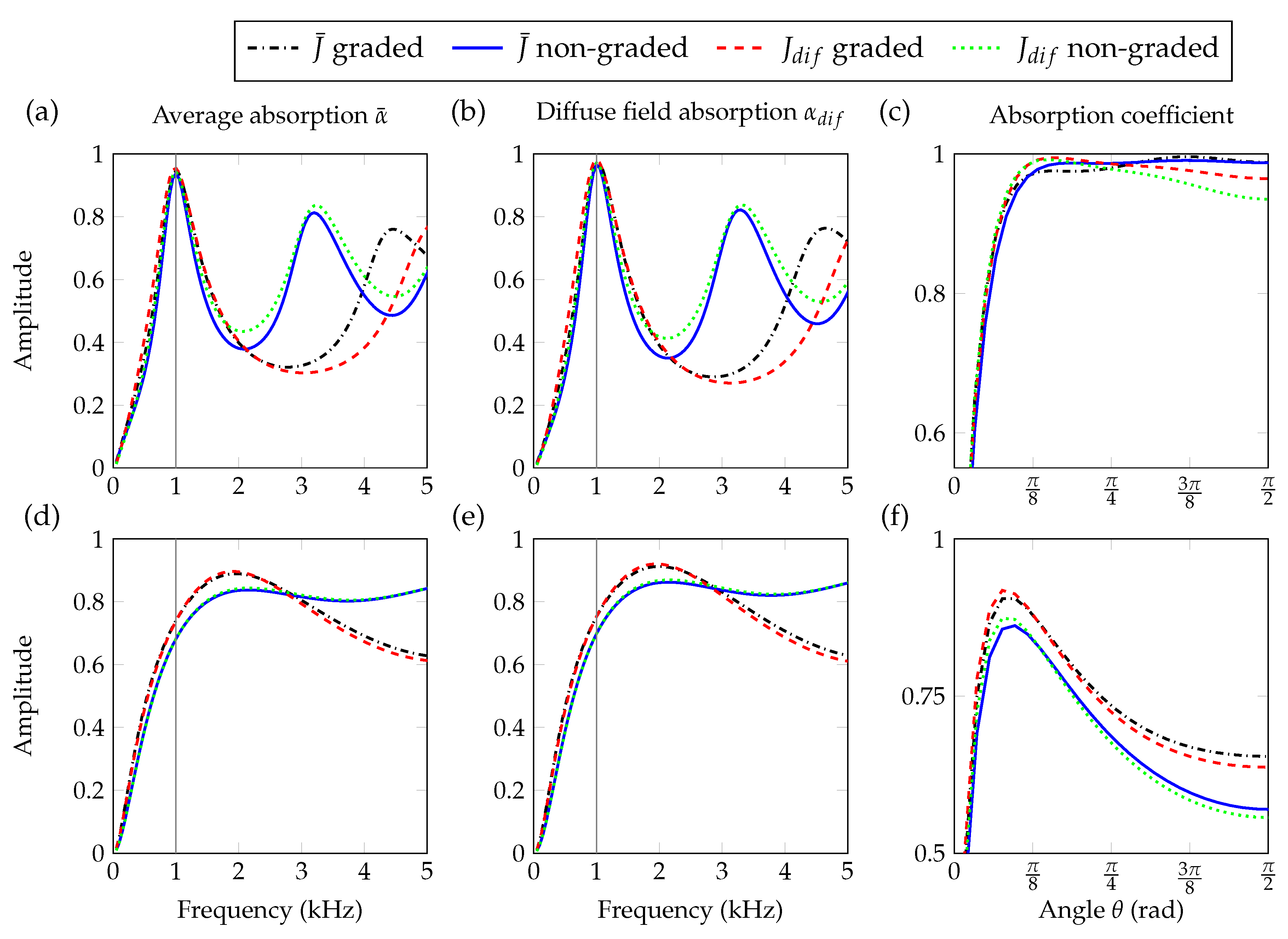
5.1. Sub–Wavelength Acoustic Absorption
5.2. Broadband Acoustic Absorption
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| TMM | Transfer matrix method |
| FEM | Finite elements method |
| PS | Peano series |
| WSTGF | Wave-splitting transfer Green functions |
| PCHIP | Piecewise cubic Hermite interpolating polynomial |
| JCAL | Johnson–Champoux–Allard–Lafarge |
References
- Zwikker, C.; Kosten, C.W. Sound Absorbing Materials; Elsevier: New York, NY, USA, 1949. [Google Scholar]
- Attenborough, K. Acoustical characteristics of porous materials. Phys. Rep. 1982, 82, 179–227. [Google Scholar] [CrossRef]
- Eijk, J.V.D.; Kosten, C.W.; Kok, W. Sound absorption by porous materials I. Appl. Sci. Res. Sect. B 1950, 1, 50–62. [Google Scholar] [CrossRef] [Green Version]
- Paris, E. On the coefficient of sound-absorption measured by the reverberation method. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1928, 5, 489–497. [Google Scholar] [CrossRef]
- Ingard, K.U. Noise Reduction Analysis; Physics Series; Jones and Bartlett Publ: Sudbury, MA, USA, 2010. [Google Scholar]
- Cox, T.J.; D’Antonio, P. Acoustic Absorbers and Diffusers: Theory, Design And Application, 3rd ed.; CRC Press, Taylor & Francis: Boca Raton, FL, USA, 2017. [Google Scholar]
- Pierce, A.D. Acoustics: An Introduction to Its Physical Principles and Applications; Springer International Publishing: Cham, Switzerland, 2019. [Google Scholar] [CrossRef]
- Allard, J.F. Propagation of Sound in Porous Media: Modelling Sound Absorbing Materials; Elsevier Applied Science: London, UK; New York, NY, USA, 1993. [Google Scholar]
- Lafarge, D.; Lemarinier, P.; Allard, J.F.; Tarnow, V. Dynamic compressibility of air in porous structures at audible frequencies. J. Acoust. Soc. Am. 1997, 102, 1995–2006. [Google Scholar] [CrossRef] [Green Version]
- Auriault, J.L.; Boutin, C.; Geindreau, C. Homogenization of Coupled Phenomena in Heterogenous Media; John Wiley & Sons: London, UK; Hoboken, NJ, USA, 2009. [Google Scholar]
- Tarnow, V. Measured anisotropic air flow resistivity and sound attenuation of glass wool. J. Acoust. Soc. Am. 2002, 111, 2735–2739. [Google Scholar] [CrossRef] [Green Version]
- Van der Kelen, C.; Göransson, P. Identification of the full anisotropic flow resistivity tensor for multiple glass wool and melamine foam samples. J. Acoust. Soc. Am. 2013, 134, 4659–4669. [Google Scholar] [CrossRef]
- Terroir, A.; Schwan, L.; Cavalieri, T.; Romero-García, V.; Gabard, G.; Groby, J.P. General method to retrieve all effective acoustic properties of fully-anisotropic fluid materials in three dimensional space. J. Appl. Phys. 2019, 125, 025114. [Google Scholar] [CrossRef] [Green Version]
- Boulvert, J.; Cavalieri, T.; Costa-Baptista, J.; Schwan, L.; Romero-García, V.; Gabard, G.; Fotsing, E.R.; Ross, A.; Mardjono, J.; Groby, J.P. Optimally graded porous material for broadband perfect absorption of sound. J. Appl. Phys. 2019, 126, 175101. [Google Scholar] [CrossRef]
- Zieliński, T.G.; Venegas, R.; Perrot, C.; Červenka, M.; Chevillotte, F.; Attenborough, K. Benchmarks for microstructure-based modelling of sound absorbing rigid-frame porous media. J. Sound Vib. 2020, 483, 115441. [Google Scholar] [CrossRef]
- Krueger, R.; Ochs, R. A green’s function approach to the determination of internal fields. Wave Motion 1989, 11, 525–543. [Google Scholar] [CrossRef]
- Borzdov, G.N. Frequency domain wave-splitting techniques for plane stratified bianisotropic media. J. Math. Phys. 1997, 38, 6328–6366. [Google Scholar] [CrossRef]
- Shuvalov, A.; Poncelet, O.; Deschamps, M. General formalism for plane guided waves in transversely inhomogeneous anisotropic plates. Wave Motion 2004, 40, 413–426. [Google Scholar] [CrossRef]
- Baron, C. Matricant Peano Series Development to Study Elastic Waves Propagation in Continuously Varying Properties Materials. Ph.D. Thesis, Université de Bordeaux, Bordeaux, France, 2005. [Google Scholar]
- Gautier, G.; Kelders, L.; Groby, J.P.; Dazel, O.; De Ryck, L.; Leclaire, P. Propagation of acoustic waves in a one-dimensional macroscopically inhomogeneous poroelastic material. J. Acoust. Soc. Am. 2011, 130, 1390–1398. [Google Scholar] [CrossRef] [Green Version]
- Geslain, A.; Groby, J.P.; Dazel, O.; Mahasaranon, S.; Horoshenkov, K.V.; Khan, A. An application of the Peano series expansion to predict sound propagation in materials with continuous pore stratification. J. Acoust. Soc. Am. 2012, 132, 208–215. [Google Scholar] [CrossRef] [PubMed]
- Cavalieri, T.; Boulvert, J.; Schwan, L.; Gabard, G.; Romero-Garcìa, V.; Groby, J.P.; Escouflaire, M.; Mardjono, J. Acoustic wave propagation in effective graded fully anisotropic fluid layers. J. Acoust. Soc. Am. 2019, 146, 3400–3408. [Google Scholar] [CrossRef]
- Pease, M.C. Methods of Matrix Algebra; Academic Press: New York, NY, USA, 1965. [Google Scholar]
- Brouard, B.; Lafarge, D.; Allard, J.F. A general method of modelling sound propagation in layered media. J. Sound Vib. 1995, 183, 129–142. [Google Scholar] [CrossRef]
- Teperik, T.V.; García de Abajo, F.J.; Borisov, A.G.; Abdelsalam, M.; Bartlett, P.N.; Sugawara, Y.; Baumberg, J.J. Omnidirectional absorption in nanostructured metal surfaces. Nat. Photonics 2008, 2, 299–301. [Google Scholar] [CrossRef] [Green Version]
- Fritsch, F.N.; Carlson, R.E. Monotone Piecewise Cubic Interpolation. SIAM J. Numer. Anal. 1980, 17, 238–246. [Google Scholar] [CrossRef]
- Darcy, H. Les Fontaines Publiques de la ville de Dijon: Exposition et application des principes à suivre et des formules à employer dans les questions de distribution d’eau; Hachette Livre BNF: Paris, France, 1856. [Google Scholar]
- Nelder, J.A.; Mead, R. A Simplex Method for Function Minimization. Comput. J. 1965, 7, 308–313. [Google Scholar] [CrossRef]
- Press, W.H. (Ed.) Numerical Recipes: The Art of Scientific Computing, 3rd ed.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2007. [Google Scholar]
- Romero-García, V.; Theocharis, G.; Richoux, O.; Pagneux, V. Use of complex frequency plane to design broadband and sub-wavelength absorbers. J. Acoust. Soc. Am. 2016, 139, 3395–3403. [Google Scholar] [CrossRef] [Green Version]
- Groby, J.P.; Pommier, R.; Aurégan, Y. Use of slow sound to design perfect and broadband passive sound absorbing materials. J. Acoust. Soc. Am. 2016, 139, 1660–1671. [Google Scholar] [CrossRef] [PubMed]
- Luk, T.S.; Campione, S.; Kim, I.; Feng, S.; Jun, Y.C.; Liu, S.; Wright, J.B.; Brener, I.; Catrysse, P.B.; Fan, S.; et al. Directional perfect absorption using deep subwavelength low-permittivity films. Phys. Rev. B 2014, 90, 085411. [Google Scholar] [CrossRef] [Green Version]
- Romero-García, V.; Theocharis, G.; Richoux, O.; Merkel, A.; Tournat, V.; Pagneux, V. Perfect and broadband acoustic absorption by critically coupled sub-wavelength resonators. Sci. Rep. 2016, 6, 19519. [Google Scholar] [CrossRef] [PubMed]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cavalieri, T.; Boulvert, J.; Gabard, G.; Romero-García, V.; Escouflaire, M.; Regnard, J.; Groby, J.-P. Graded and Anisotropic Porous Materials for Broadband and Angular Maximal Acoustic Absorption. Materials 2020, 13, 4605. https://doi.org/10.3390/ma13204605
Cavalieri T, Boulvert J, Gabard G, Romero-García V, Escouflaire M, Regnard J, Groby J-P. Graded and Anisotropic Porous Materials for Broadband and Angular Maximal Acoustic Absorption. Materials. 2020; 13(20):4605. https://doi.org/10.3390/ma13204605
Chicago/Turabian StyleCavalieri, Théo, Jean Boulvert, Gwénaël Gabard, Vicent Romero-García, Marie Escouflaire, Josselin Regnard, and Jean-Philippe Groby. 2020. "Graded and Anisotropic Porous Materials for Broadband and Angular Maximal Acoustic Absorption" Materials 13, no. 20: 4605. https://doi.org/10.3390/ma13204605




